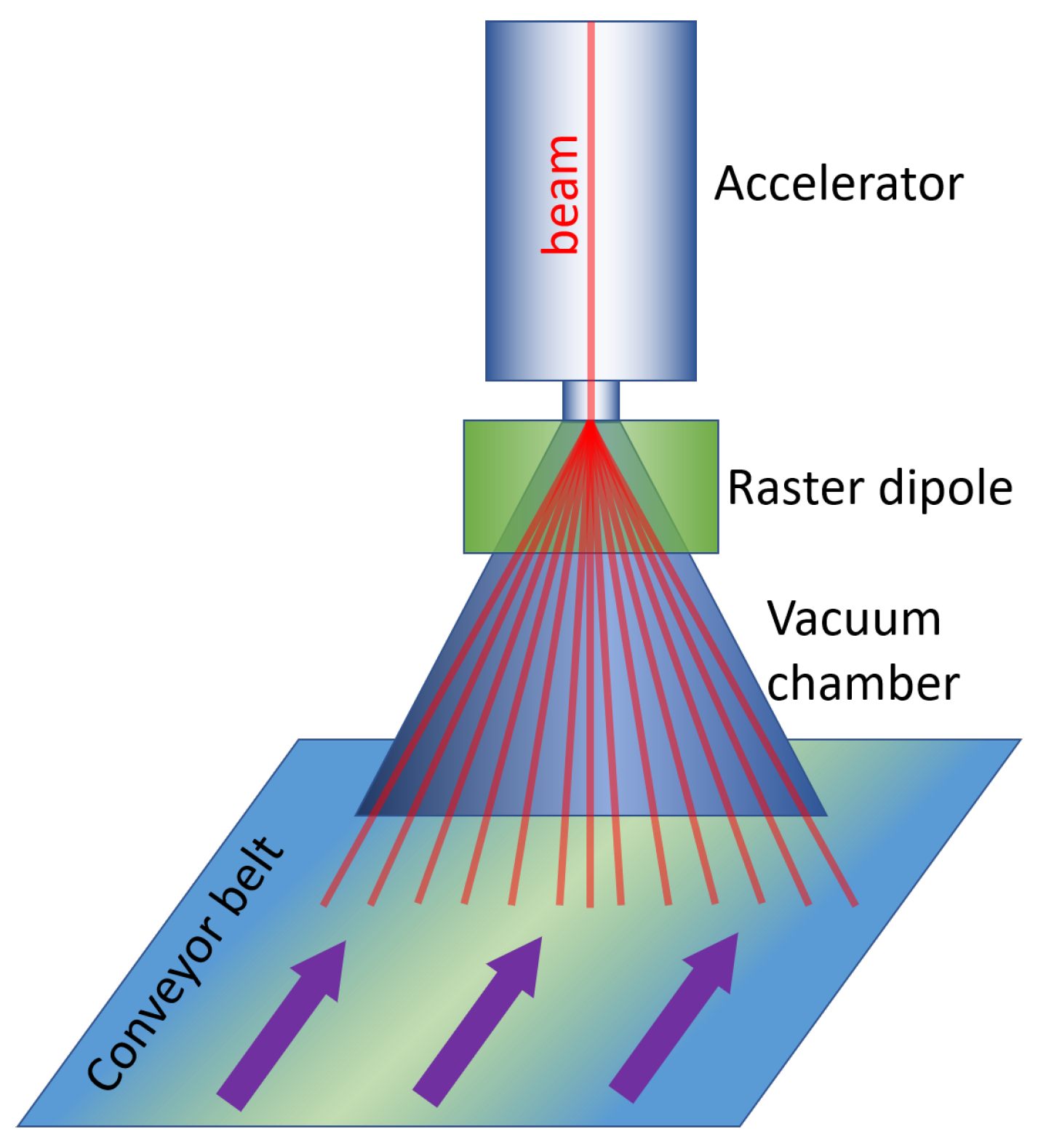
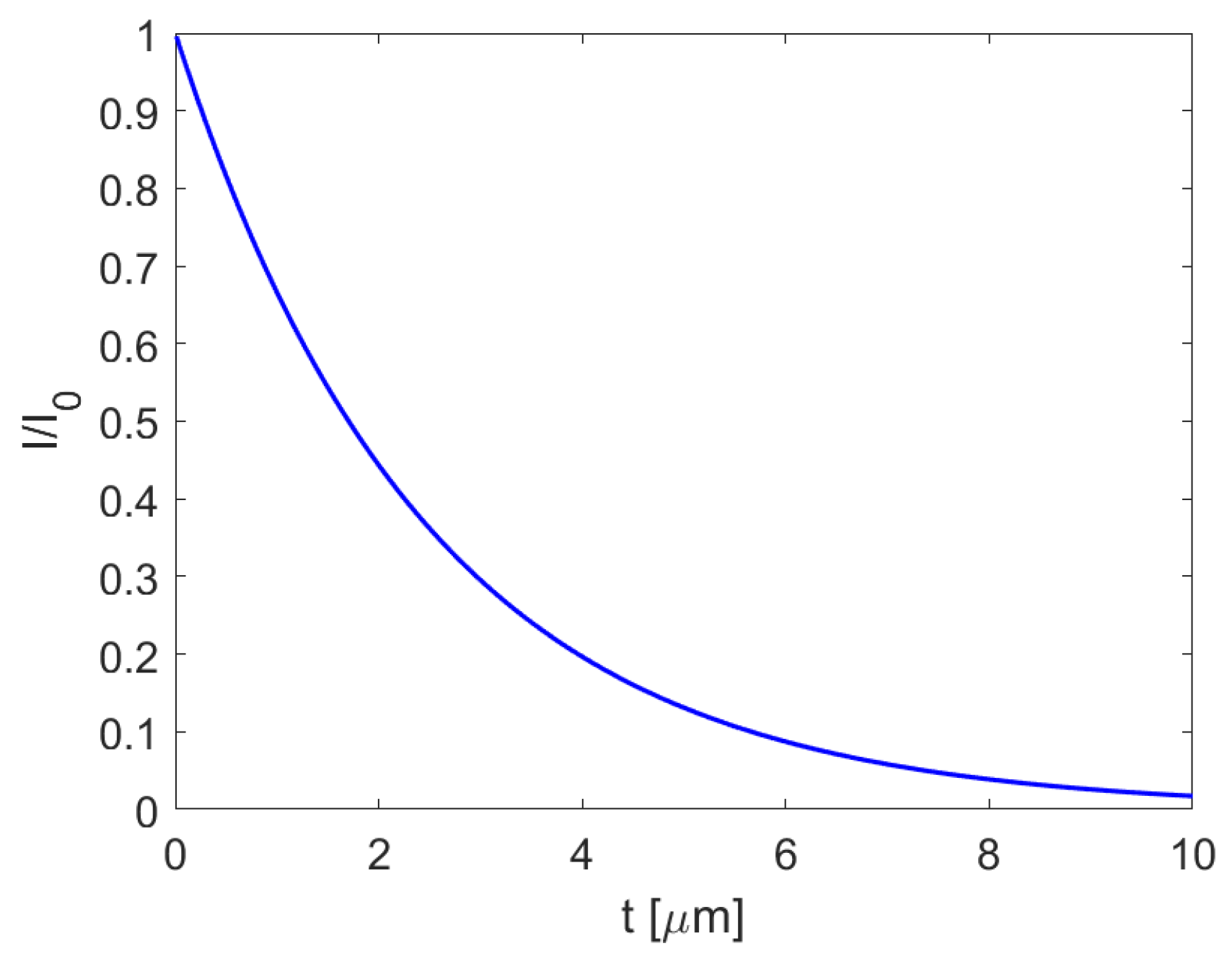
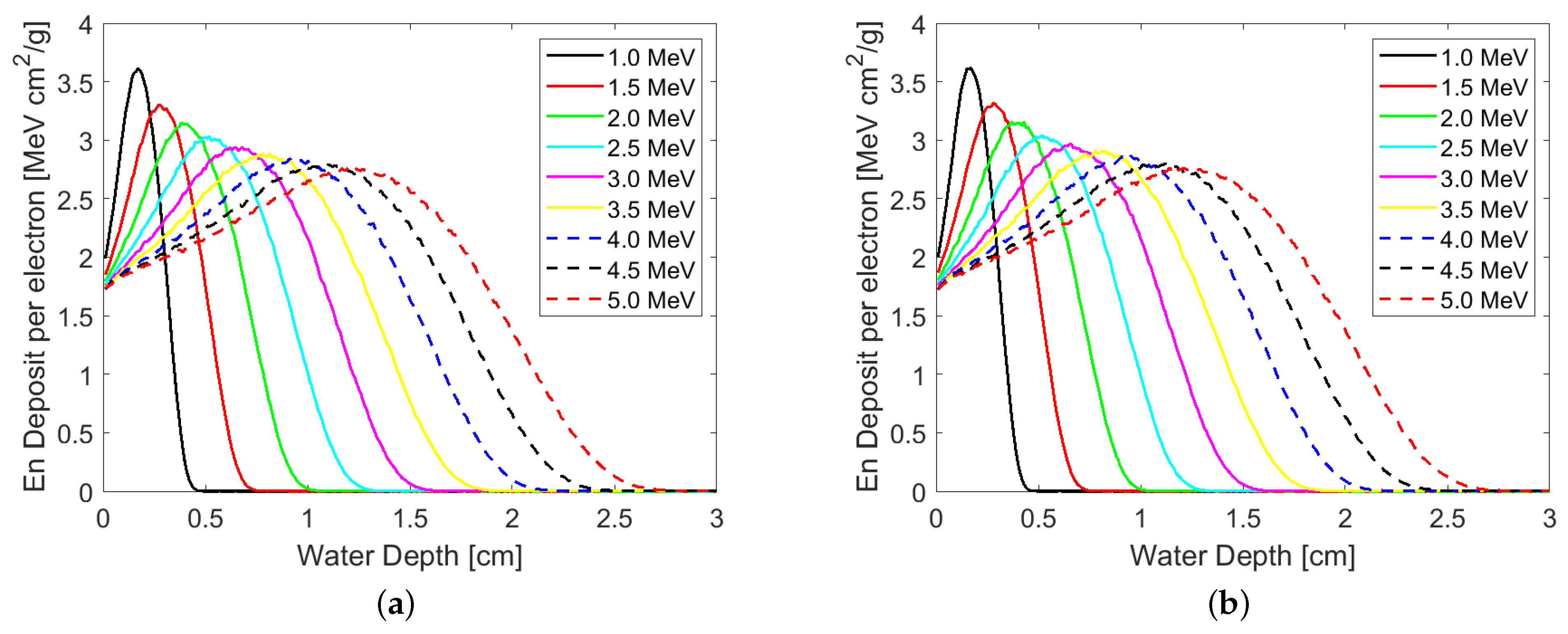
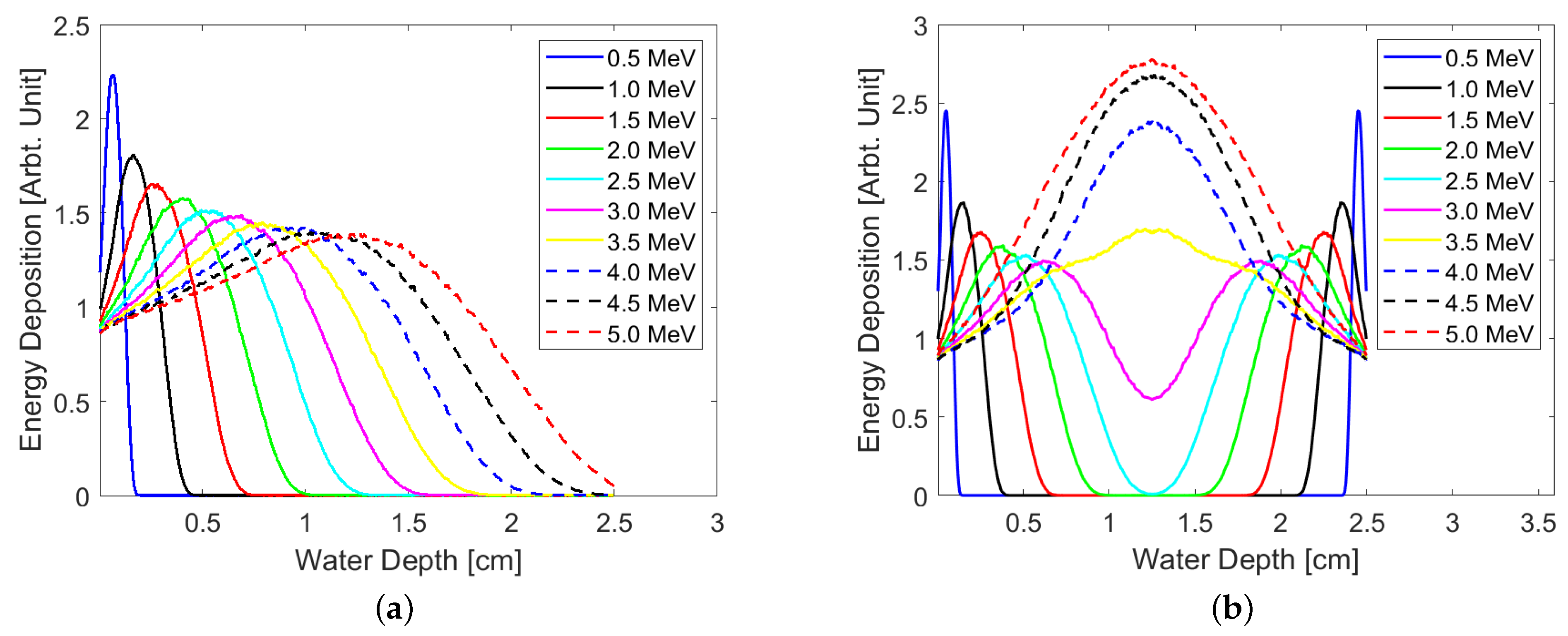
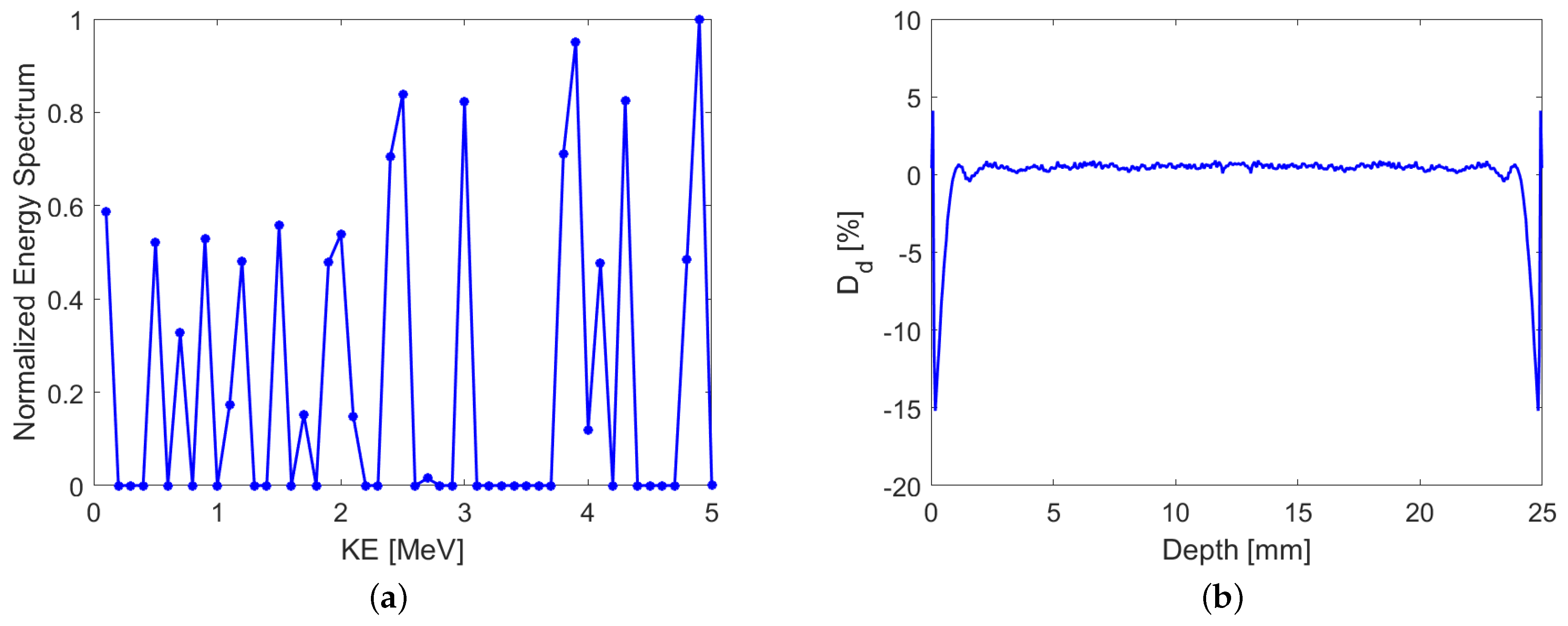
2.2. Orientation of the Target with Respect to the Beam
As we discuss in this paper, the dose deposition curve of an electron beam can be altered by either changing the energy of the beam or by varying the angle of incidence of the electron beam on the target. The simulation studies shown in the next section indicate that, qualitatively, varying the energy and the angle of incidence has a similar effect on the dose deposition.
We made no assumptions on the preferred means of varying the dose deposition through the hide; instead, we simply present different possibilities that allow different options to be considered in later studies. This section considers the possibilities for varying the angle of incidence of the electron beam, taking into account the challenges faced.
A whole hide from a fully grown bull is approximately
m
in size, and if the intention is to irradiate the entire hide in one piece, then the electron beam must be scanned over the entire width. When a charged particle travels through a uniform magnetic field orientated perpendicular to the direction of motion, as with a dipole magnet, the particle adopts a circular path, with radius of curvature
.
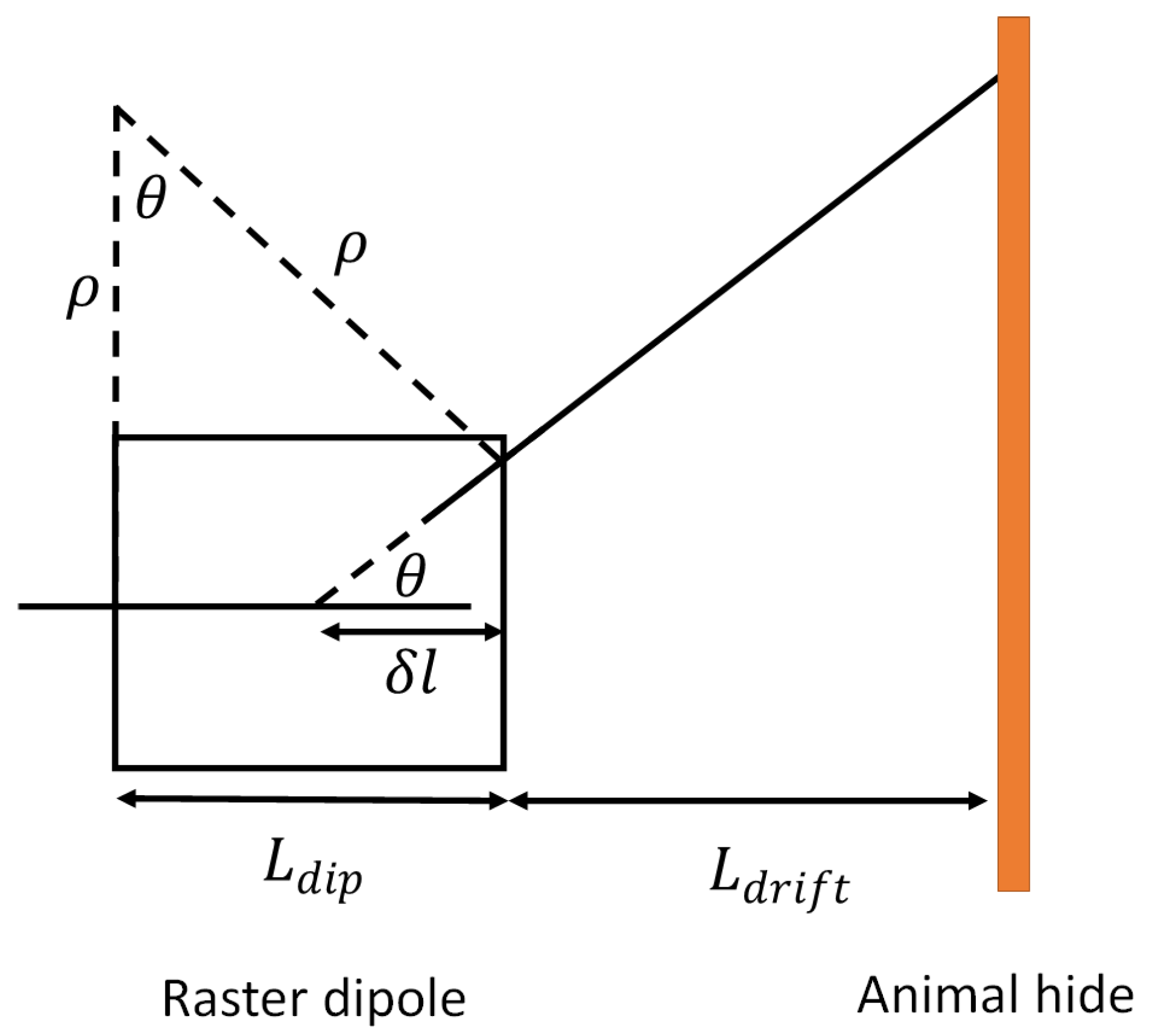
Figure 2 shows a schematic diagram of the geometry of the beam trajectory through the raster scanning magnet to the hide. We define
as the bending angle,
as the dipole length and
as the distance between the scanning magnet and the centre of the hide.
Figure 2 considers the position,
z, to be the apparent point of rotation of the beam. That is to say that we know that before and after the dipole magnet, the electron beam trajectory is a straight line, so rather than considering the true curved path of the trajectory through the dipole magnet, we can consider that at some location, the beam is rotated by an angle
. We know that the radius of curvature is given as:
We also know that the offset of the electron beam at the exit of the raster magnet (where we take
to be to the right of the beam at the entrance of the raster magnet) is given as:
In terms of
, the gradient of the line after the raster magnet is given as
, and from this, we obtain:
From this, if we define the position of the beam at the start of the raster magnet as , then the point of rotation is given as . The point of rotation is not at a fixed position, but rather, it varies with the angle, with the position changing more as increases.
In order to ensure that we achieve uniform tanning across the hide, we need to ensure that the angle of incidence between the beam and the normal to the surface of the hide is constant across the full scanning range of the beam. It should also be noted that when designing the magnetic rastering scheme, we shall also require that the beam scans over the surface of the hide at a constant rate as well.
We define the angle of incidence of the beam,
, to be the angle between the beam trajectory and the normal to the surface of the hide. For two lines with gradients
and
, where the angle of intersection between the lines is
, then we have:
from which we obtain the gradient of the hide as a function of
and
as:
The choice of sign in Equation (
5) is based on which direction we define angles to be positive. For this paper, we chose the positive sign convention, but this is a completely free choice. We also know the equation of the line defining the trajectory of the beam between the raster magnet and the hide must be:
Let us now assume that points
and
are points on the surface of the hide that are sufficiently close together such that we can assume a straight line between them, then from Equations (
5) and (
6), we have:
From Equations (
5) and (
7), it can be shown that:
The differential equations given in Equation (
8) need to be solved numerically; however, we can make some approximations to allow us to gain some insight into the approximate form of the solution. If we assume that
, then we can neglect the variation in the point of rotation as the deflection angle of the raster magnet is varied. In this assumption, we obtain the simplified differential equations:
which can easily be solved and provide the general solution:
This solution describes the general equation for an exponential spiral, which is a set of curves, including the Golden Spiral, which have the property that for a line passing through the origin and intersecting the spiral, the angle of intersection is a constant. We see that when we apply simplifications to Equation (
8), it produces the correct result as expected.
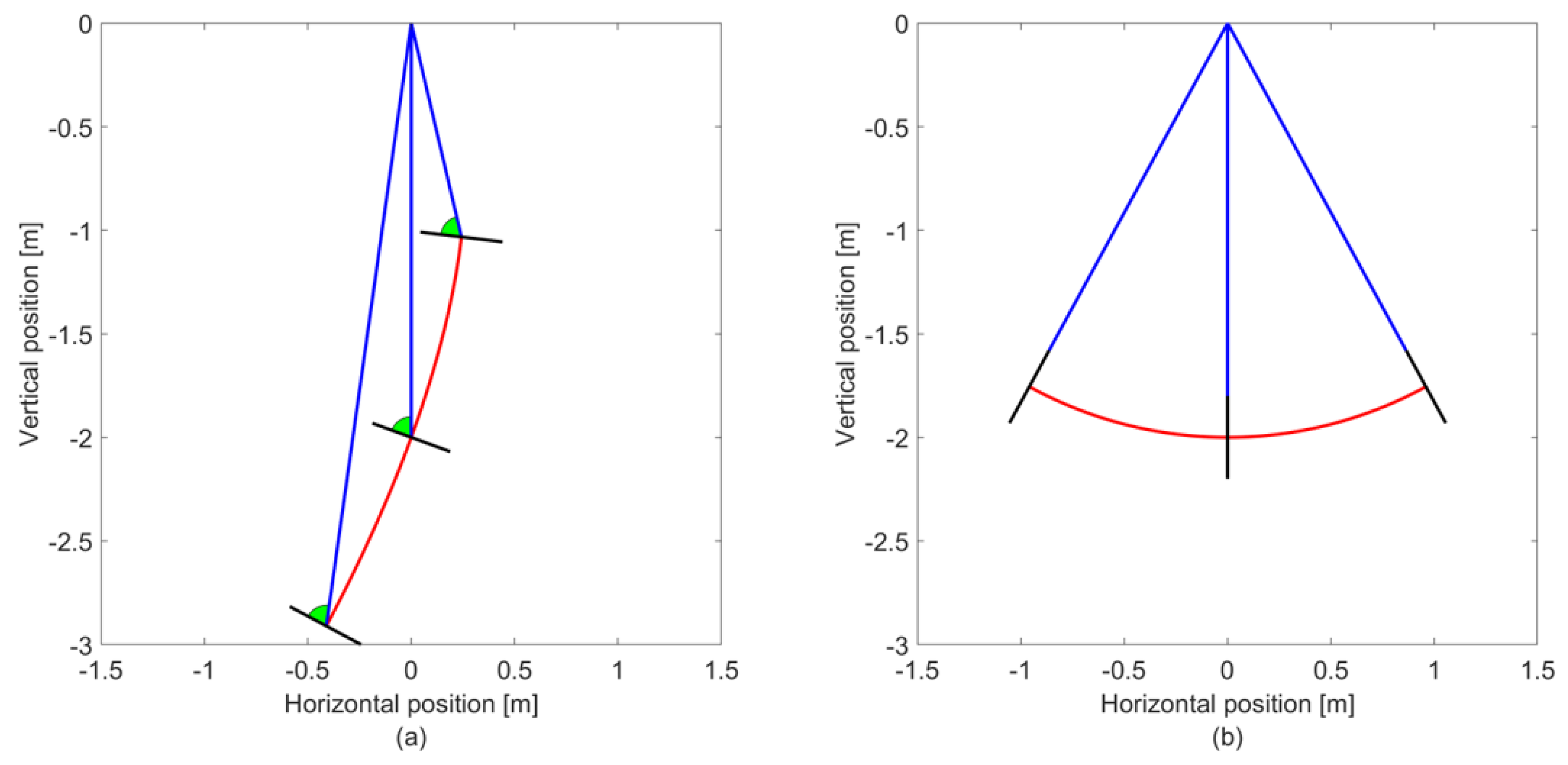
Figure 3 shows a diagram of the hide orientation for an angle of incidence of
(a) and
(b), based on Equation (
10) and assuming that the point of rotation is independent of the deflection angle, which is a reasonable assumption in this case. In both figures, we can see that the angle of incidence remains constant regardless of the deflection angle of the beam. The blue lines represent the direction of the electron beam, the black lines the normal to the hide surface and the red line the hide, and the green areas demonstrate that the angle of incidence remains constant for non-zero angles of incidence.
When , the angular range of the beam will not be symmetric. We would either have to shift the midpoint of the hide transversely or apply a DC offset current to the scanning magnet to shift the range of the angles. In practice, the latter option is likely to be the preferred solution.
So far, we considered the case where the hide is curved in the deflecting plane of the beam in order to ensure that the angle of incidence remains constant. We could also imagine inclining the plane of the hide as it passes through the electron beam; however, we would still need to ensure that the angle of incidence in the deflecting plane is zero to ensure we have the angle of incidence we expect; thus, we would need to solve Equation (
8) for
.
2.3. Design of the Rastering Scheme
The rastering scheme needs to be able to ensure that the beam is swept over the surface of the hide in a uniform manner. For this, there are several key considerations we need to take into account. Firstly, the beam should scan over the surface of the hide at a constant speed, which means that we need to determine how the sweeping speed of the beam depends on the deflection angle. Secondly, we need to consider what raster pattern will ensure a uniform dose distribution.
To determine the speed of the beam as it sweeps across the surface of the hide, we need to determine
, where
s is the arc length along the curve describing the hide. The arc length for a curve is given as:
From Equation (
5), we know
in terms of the angle of incidence and the magnetic deflection angle; thus:
Furthermore, we also have an expression relating
to
from Equation (
8):
Due to the fact that Equation (
8) describes an inseparable set of differential equations, it needs to be solved numerically. However, if we use the approximated results from Equation (
10), we can solve this as a simplified form:
In Equation (
14),
is taken to be the deflection angle required to direct the beam to one end of the hide and
is the angle of some arbitrary point on the hide surface. We can use Equation (
13) or Equation (
14) to determine the speed of the beam as a function of the deflection angle:
Equations (
15) and (
16) show the equations for the scanning rate of the raster magnet for the exact solution and where
, respectively.
is the scanning speed of the beam on the surface of the hide, which is taken to be a constant.
From now on, we will assume that
as we are able to analyse this case. In a real system, we would expect
1–2 m, whereas
0.1 m; therefore, this assumption is valid in this situation. We see that in order to obtain a constant scanning speed,
must vary exponentially with angle, although when
, the curve becomes a circular arc and
constant. Given that we want the midpoint of the hide to coincide with the beam when
for all values of
, we can define the corresponding range of deflection angles as:
where
is the width of the hide; however, in practice, it is likely that the beam would be swept slightly further than the width of the hide. If
, the general form of the integral breaks down as
if
. Therefore, in this case, the range of angles becomes
. From Equation (
17), we can also place some constraints on the geometry of the system. Firstly,
, and we also obtain the condition that
; if not, the upper bound on deflection angle becomes complex, which is unphysical.
Equation (
16) can be solved for
:
The negative sign in Equation (
18) is due to the fact that in our definition, a positive angular deflection corresponds to a negative transverse position. This equation defines the required deflection as a function of time in order to sweep across the surface of the hide at a constant rate. In addition to this, we need to take into account the fact that the hide is also moving longitudinally as the beam is being swept across it. As such, we need to give a deflection in the longitudinal direction as well to account for this motion. Furthermore, we need to ensure that the raster pattern forms a closed cycle.
There is an infinite number of choices for the magnetic rastering scheme, but we considered the scheme that minimises the rate of change of magnetic field for the longitudinal raster magnet. For the remainder of this paper, we refer to the x-direction as the direction across the width of the hide and the z-direction as the direction of motion.
We also need to consider the constraints on the width of the rather pitch,
, which will clearly depend on the beam size,
. If we assume that the beam profile is approximately Gaussian, then as we can see from
Figure 4b, we require that
.
For the longitudinal raster magnet, it can be relatively easily shown that the dependence on
z with respect to deflection angle is given as:
Note that we have now changed our previous definition of
to
, and the deflection angle in
z is
.
is the additional distance that the longitudinal raster magnet is away from the hide relative to the horizontal raster magnet; it should be noted that
can be negative if the longitudinal raster magnet is downstream of the horizontal raster magnet. In this paper, we neglected
, as it was assumed to be small. Given that, once again, we wanted the beam to move in
z at a constant speed, which is equal to the speed that the hide moves along the conveyor, which is
. We can show that the longitudinal deflection angle as a function of time is given as:
We now defined the required time dependence of the horizontal and longitudinal raster magnets; however, in order to complete a cycle, we need to consider the beam at the edges of the hide, such that the beam spot shifts by one pitch length relative to the hide before scanning across again. To consider the scans from left to right and right to left,
simply switches sign. To provide time for the longitudinal raster to shift down one raster, we need to include an overshoot region beyond the end of the hide width. This overshoot region also prevents us from delivering an excessive dose to the edge of the hide. The width of this overshoot is somewhat arbitrary; however, it is sensible to ensure that it is at least 5–6 RMS beam sizes away from the edge of the hide to avoid excessive dose deposition on the edge of the hide. In order for this scheme to work and to provide a closed raster scheme that provides a uniform dose distribution to the surface of the hide, we require:
where
is the average time to irradiate one hide,
is the length of the hide and
is the width of the overshoot region. It should be noted that the faster we make
, the higher the required raster frequency, which creates challenges on how to vary the magnetic field fast enough. However, we need to ensure that
is fast enough to produce the required leather throughput to compete with conventional techniques. While the conventional process is a batch process, it typically tans approximately one hide every 90–360 s. In order to minimise the raster frequency, while ensuring that the hide is irradiated within
, we require that Equation (
21) be an equality, which gives us:
If we define the beam momentum in MeV/
c, then the required magnetic field for a raster magnet is given as:
Based on all the information we now have, we can fully define the magnetic raster scheme as long as we know the hide width, beam size, overshoot width, tanning time and beam momentum. For this particular application,
Table 1 shows the assumed system parameters.
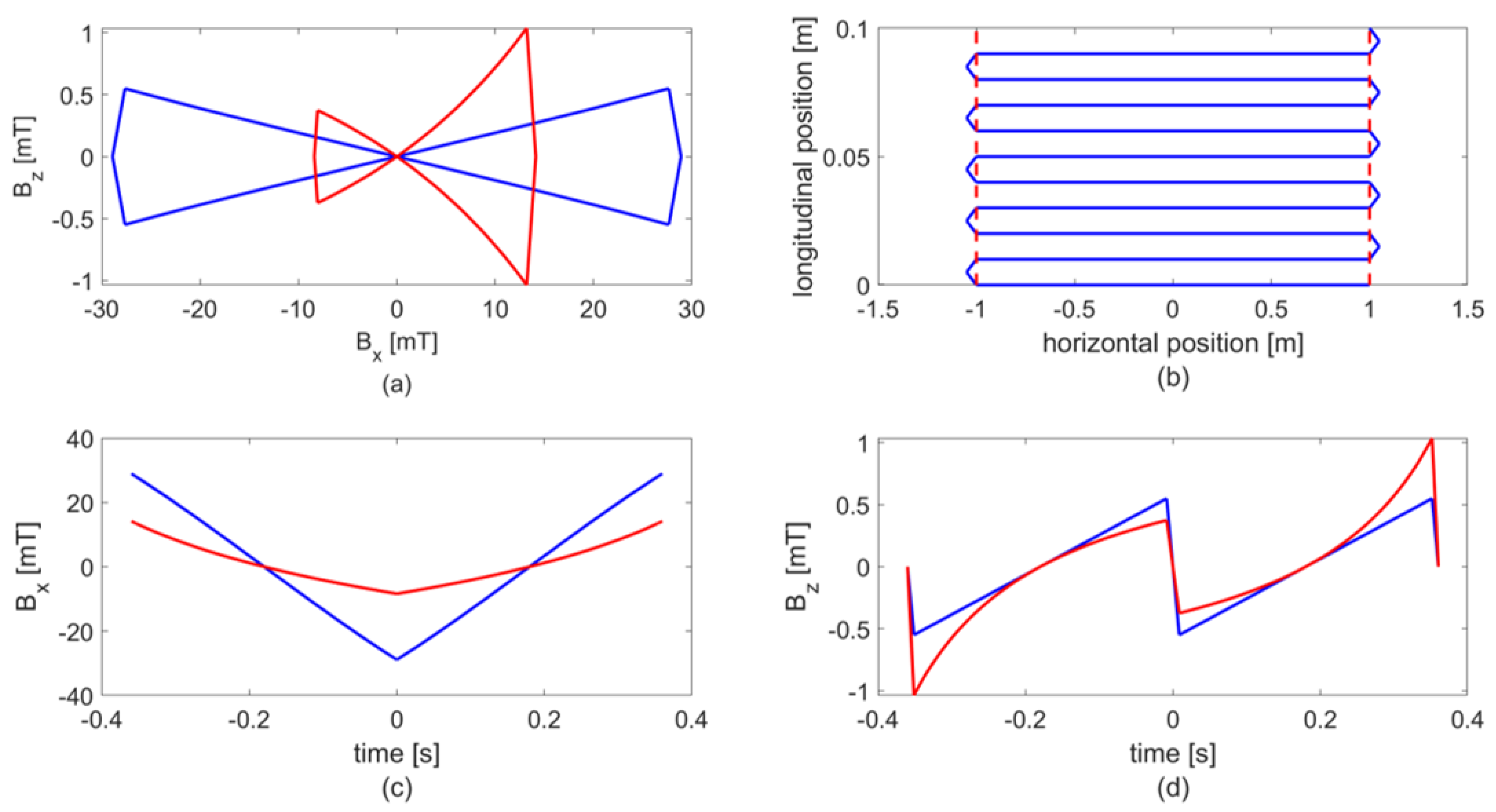
Based on the stated design parameters,
Figure 4 shows the magnetic fields for the horizontal (
x-direction) and longitudinal (
z-direction) raster magnets in the rest frame and the resulting raster pattern as seen on the surface of the hide. In
Figure 4b, the red lines represent the edge of the hide width, to show the overshoot region.
Figure 4c,d shows
and
as a function of time. Note that for this particular choice of raster pattern, the longitudinal raster magnet oscillates at double the frequency of the horizontal raster. In doing so, the raster pattern in the rest frame forms a figure eight pattern, and this setup allowed us to minimise the range of movement in the longitudinal direction. We could, however, design a raster pattern that would appear as a triangle in the rest frame, but this would double the dynamic range for the longitudinal raster magnet.