Research on the Influence of Spatial Dimensions on the Stability of Large-Scale Slopes under Heavy Rainfall
Abstract
1. Introduction
2. Unsaturated Seepage Analysis in FLAC3D
3. FDM with the Strength Reduction Method
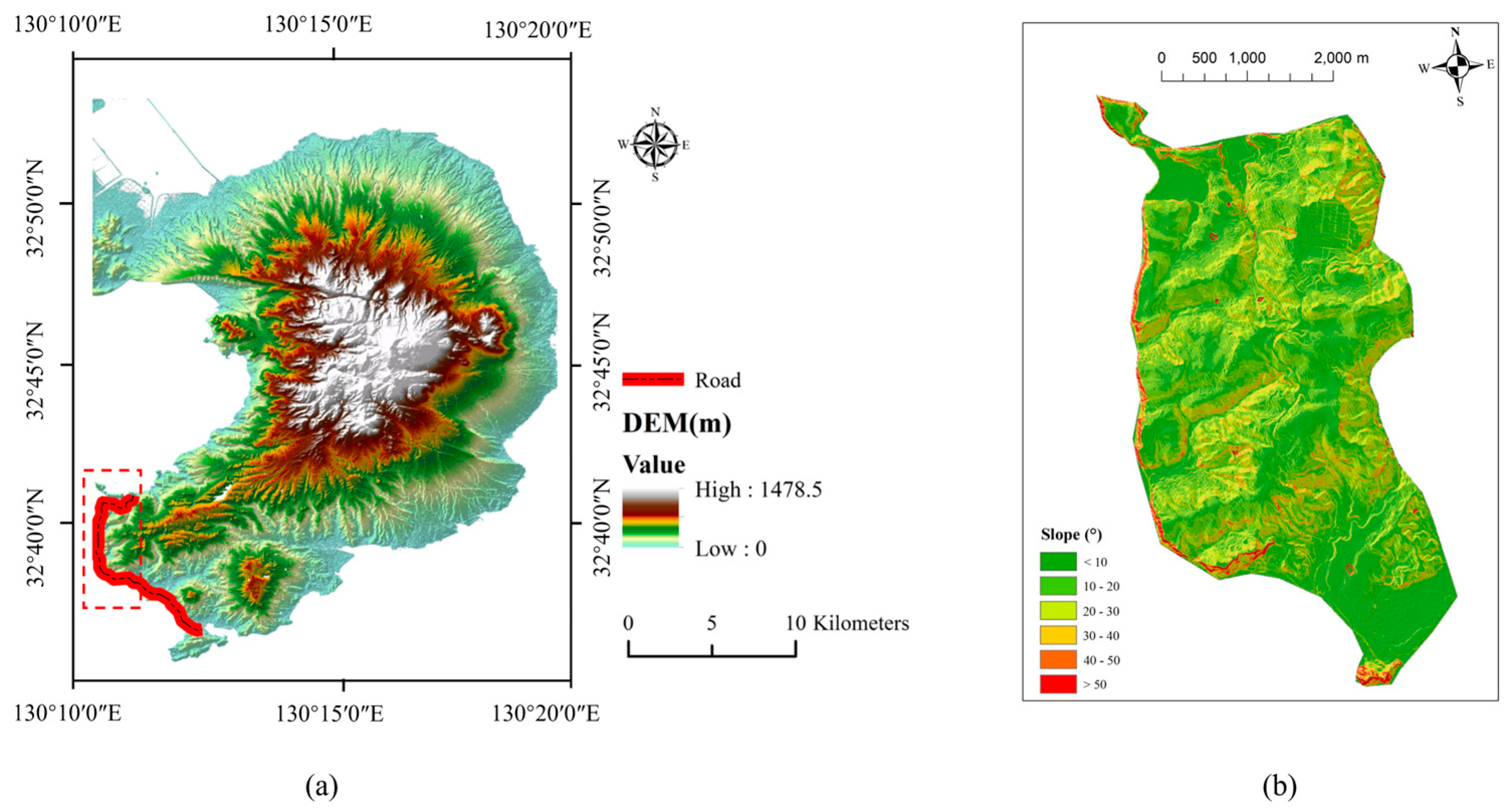
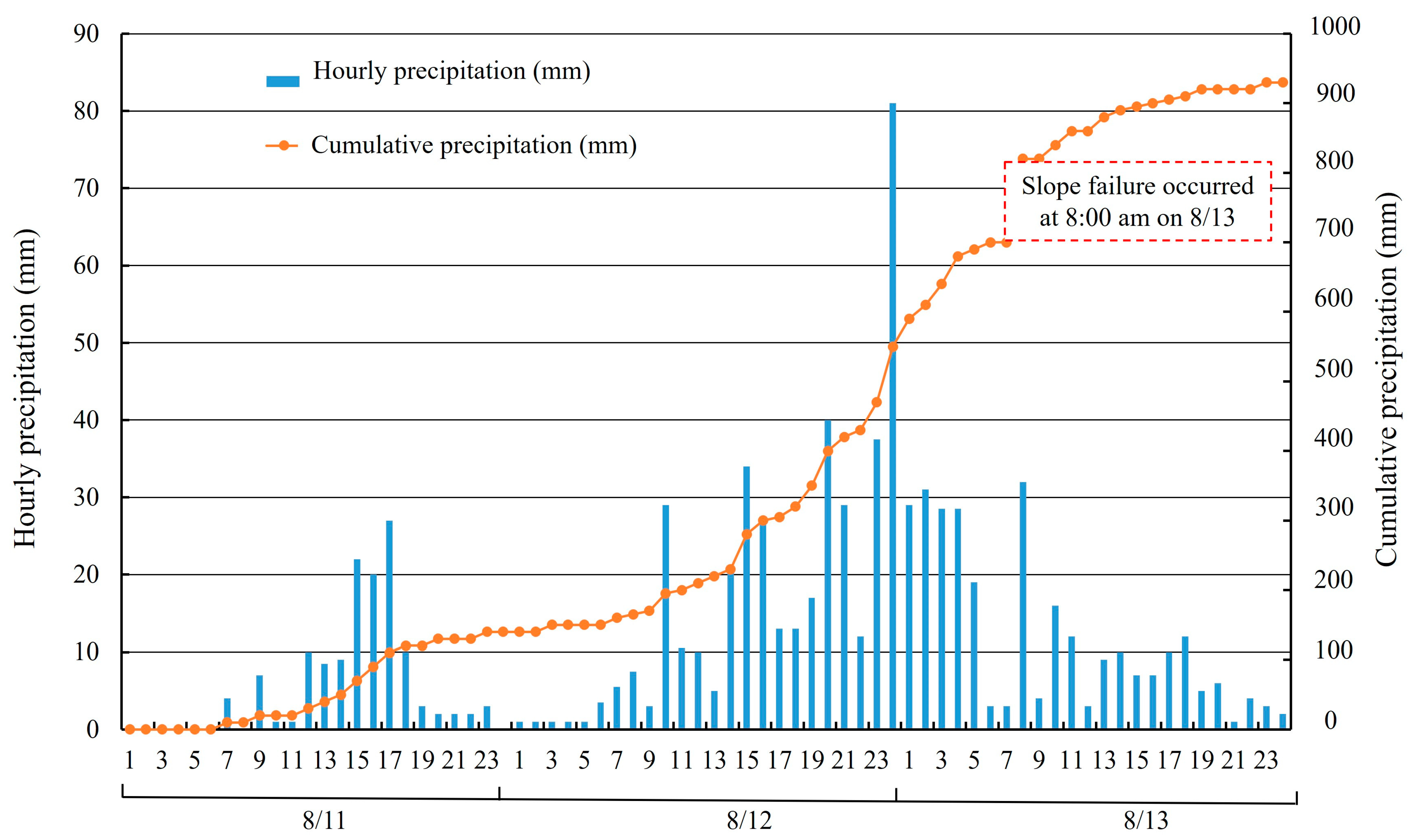
4. Characteristics of the Study Area
5. Methodology
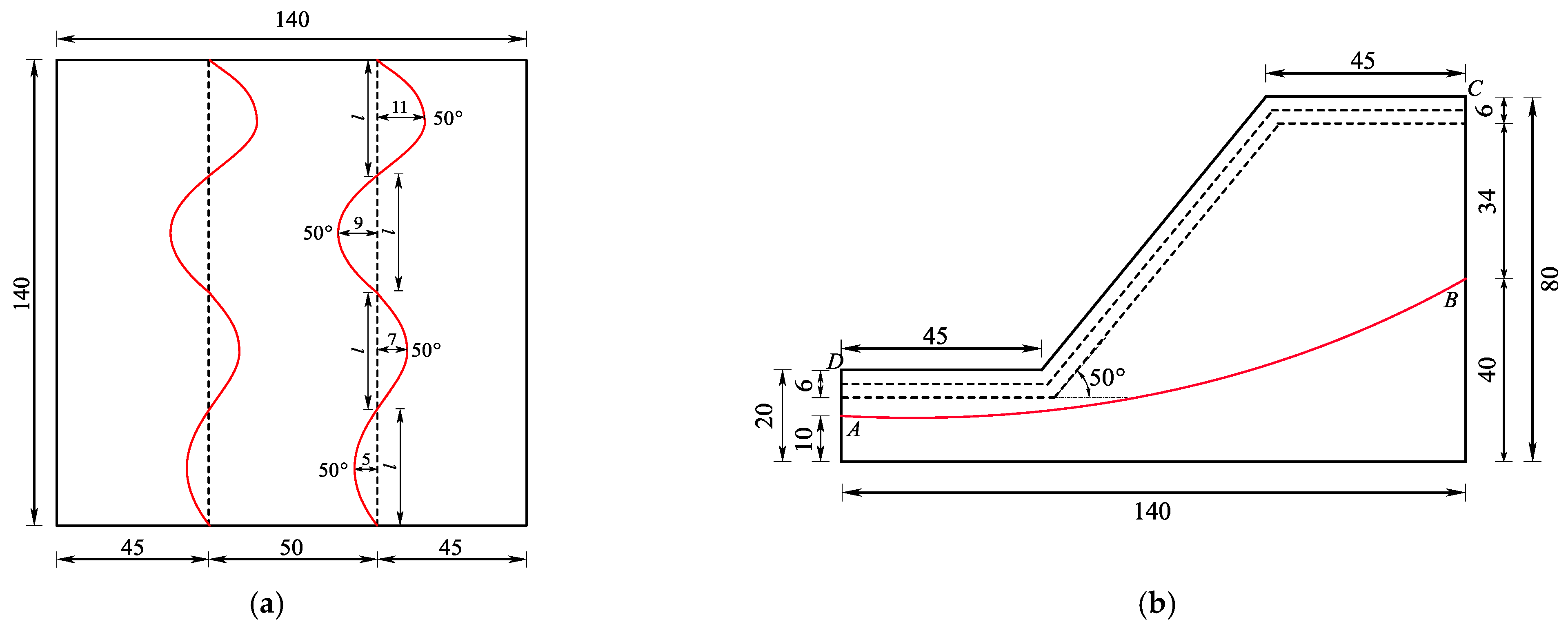
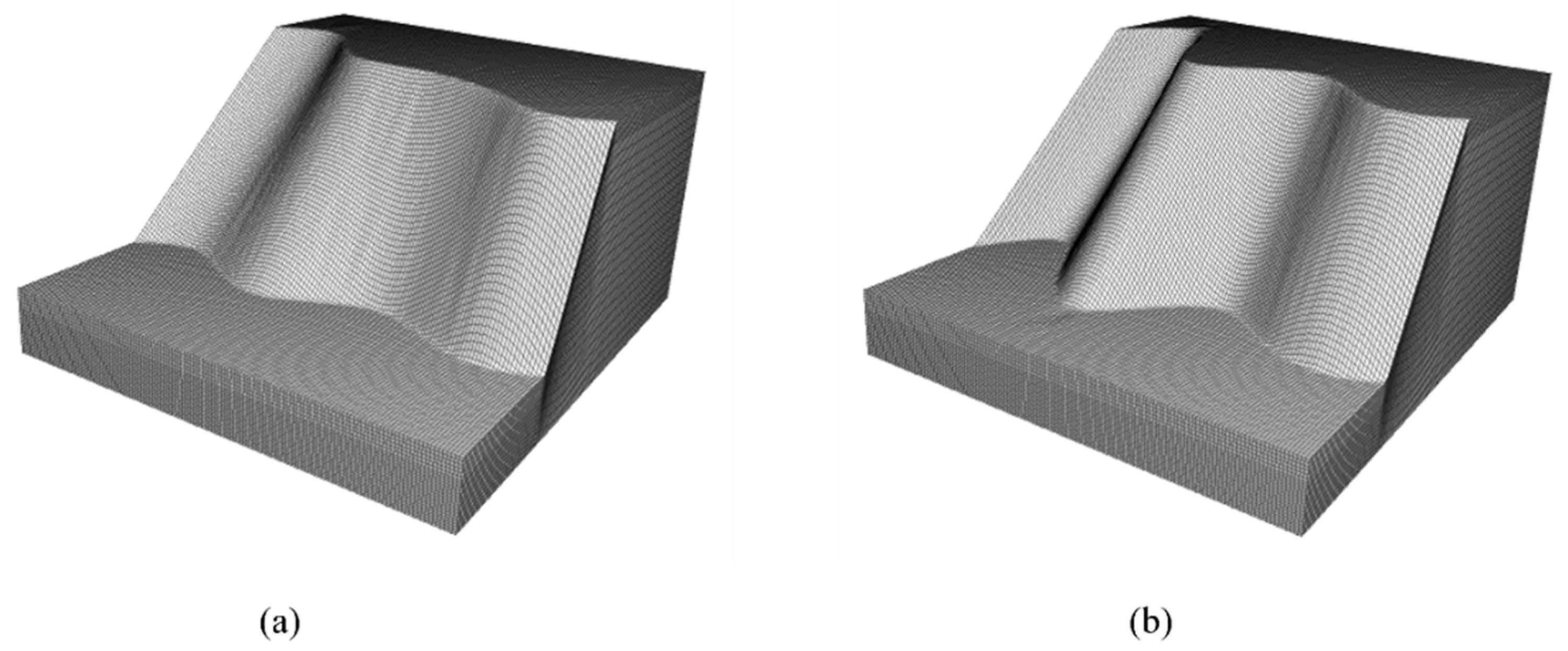
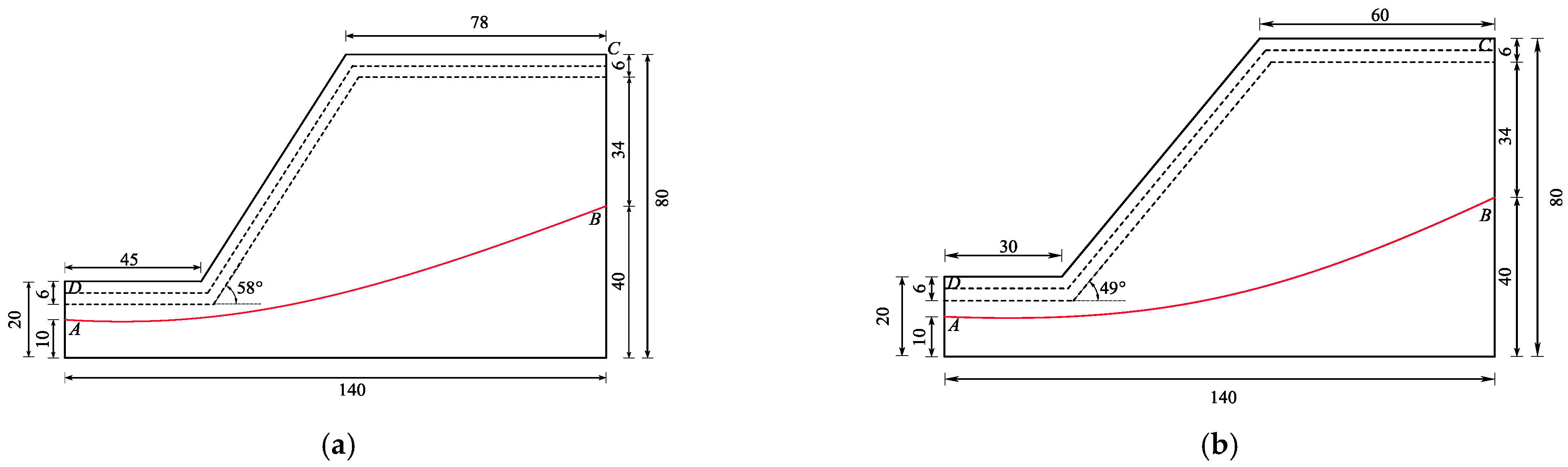
5.1. Slopes Parallel to the Road
5.2. Slopes Parallel to the Road with Different Gradients
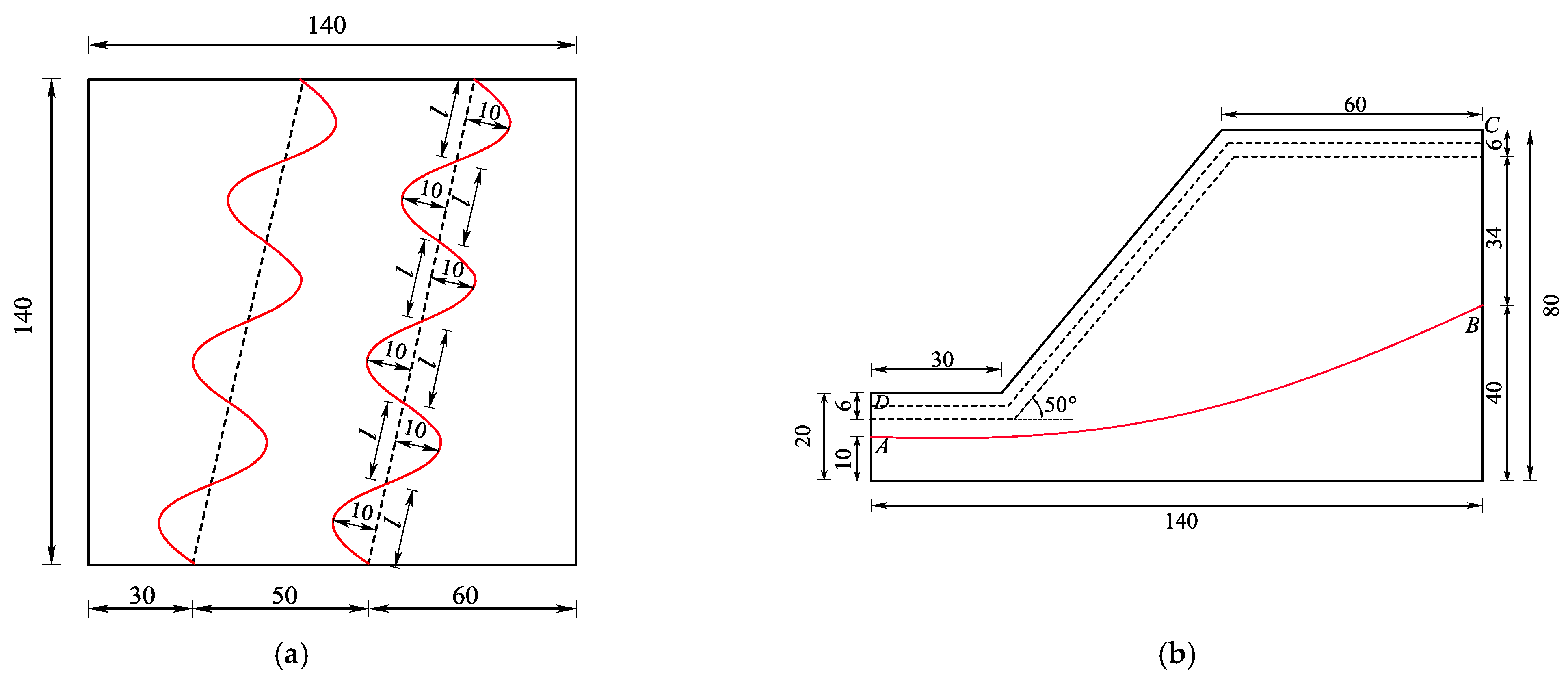
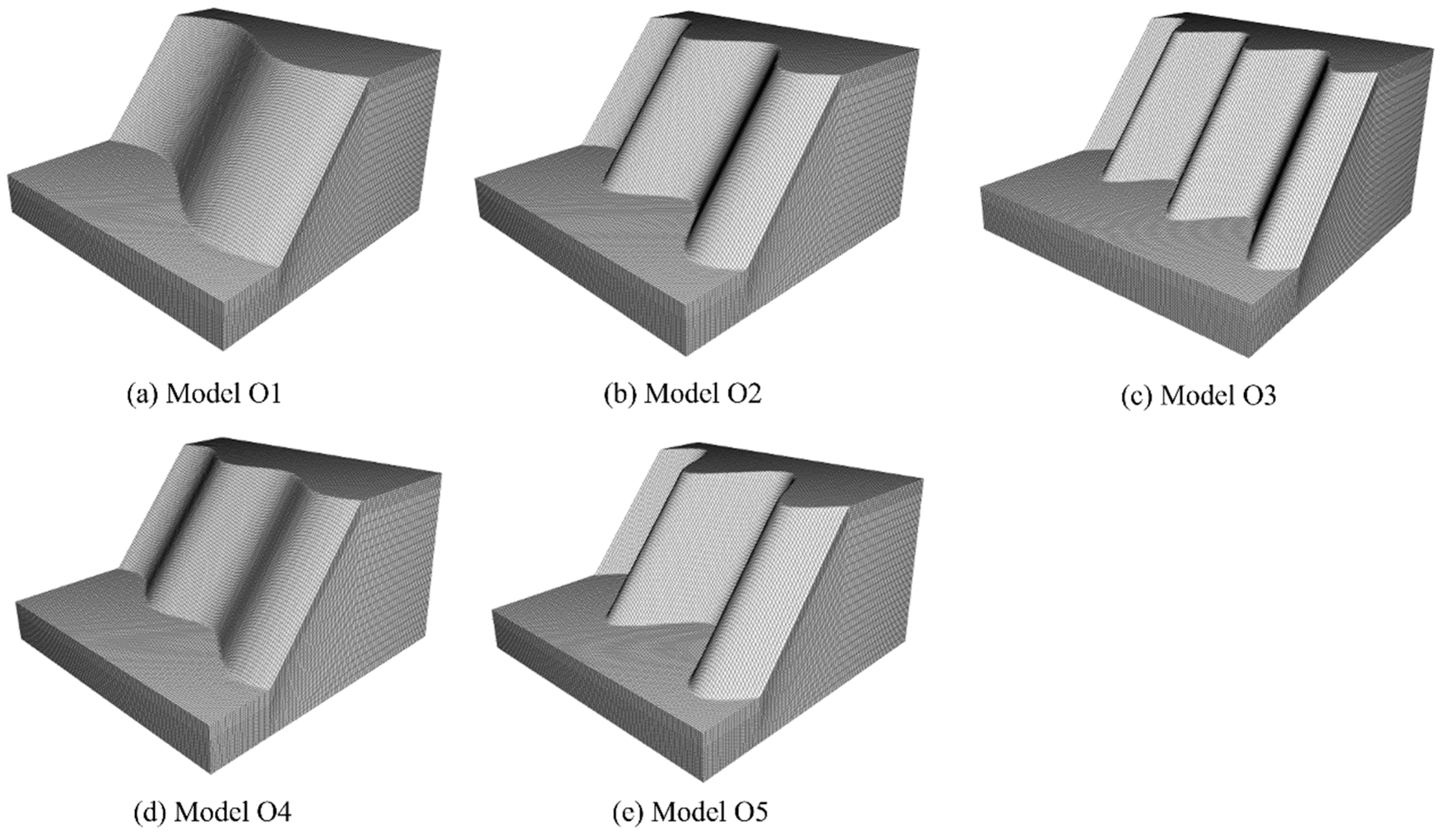
5.3. Slopes Oblique to the Road
6. Results
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sheikh, M.R.; Nakata, Y.; Shitano, M.; Kaneko, M. Rainfall-Induced Unstable Slope Monitoring and Early Warning through Tilt Sensors. Soils Found. 2021, 61, 1033–1053. [Google Scholar] [CrossRef]
- Chen, H.; Qin, S.; Xue, L.; Xu, C. Why the Xintan Landslide Was Not Triggered by the Heaviest Historical Rainfall: Mechanism and Review. Eng. Geol. 2021, 294, 106379. [Google Scholar] [CrossRef]
- Cho, M.T.T.; Chueasamat, A.; Hori, T.; Saito, H.; Kohgo, Y. Effectiveness of Filter Gabions against Slope Failure Due to Heavy Rainfall. Soils Found. 2021, 61, 480–495. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, G.; Zheng, L.; Li, Y.; Zhuang, X. Effects of Geometries on Three-Dimensional Slope Stability. Can. Geotech. J. 2013, 50, 233–249. [Google Scholar] [CrossRef]
- Jaboyedoff, M.; Carrea, D.; Derron, M.-H.; Oppikofer, T.; Penna, I.M.; Rudaz, B. A Review of Methods Used to Estimate Initial Landslide Failure Surface Depths and Volumes. Eng. Geol. 2020, 267, 105478. [Google Scholar] [CrossRef]
- Azizian, A.; Popescu, R. Three-Dimensional Seismic Analysis of Submarine Slopes. Soil. Dyn. Earthq. Eng. 2006, 26, 870–887. [Google Scholar] [CrossRef]
- Leong, E.C.; Rahardjo, H. Two and Three-Dimensional Slope Stability Reanalyses of Bukit Batok Slope. Comput. Geotech. 2012, 42, 81–88. [Google Scholar] [CrossRef]
- Wines, D. A Comparison of Slope Stability Analyses in Two and Three Dimensions. J. S. Afr. Inst. Min. Metall. 2016, 116, 399–406. [Google Scholar] [CrossRef]
- Griffiths, D.V.; Marquez, R.M. Three-Dimensional Slope Stability Analysis by Elasto-Plastic Finite Elements. Géotechnique 2007, 57, 537–546. [Google Scholar] [CrossRef]
- Wei, W.B.; Cheng, Y.M.; Li, L. Three-Dimensional Slope Failure Analysis by the Strength Reduction and Limit Equilibrium Methods. Comput. Geotech. 2009, 36, 70–80. [Google Scholar] [CrossRef]
- Zhang, L.L.; Fredlund, M.D.; Fredlund, D.G.; Lu, H.; Wilson, G.W. The Influence of the Unsaturated Soil Zone on 2-D and 3-D Slope Stability Analyses. Eng. Geol. 2015, 193, 374–383. [Google Scholar] [CrossRef]
- Zhou, Y.; Qi, S.-C.; Fan, G.; Chen, M.-L.; Zhou, J.-W. Topographic Effects on Three-Dimensional Slope Stability for Fluctuating Water Conditions Using Numerical Analysis. Water 2020, 12, 615. [Google Scholar] [CrossRef]
- Yeh, P.-T.; Lee, K.Z.-Z.; Chang, K.-T. 3D Effects of Permeability and Strength Anisotropy on the Stability of Weakly Cemented Rock Slopes Subjected to Rainfall Infiltration. Eng. Geol. 2020, 266, 105459. [Google Scholar] [CrossRef]
- Sabzevari, T.; Noroozpour, S. Effects of Hillslope Geometry on Surface and Subsurface Flows. Hydrogeol. J. 2014, 22, 1593–1604. [Google Scholar] [CrossRef]
- Talebi, A.; Troch, P.A.; Uijlenhoet, R. A Steady-state Analytical Slope Stability Model for Complex Hillslopes. Hydrol. Process. 2008, 22, 546–553. [Google Scholar] [CrossRef]
- Wang, J.; Chen, L. The Effect of Hillslope Geometry on Hortonian Rainfall-Infiltration-Runoff Processes. J. Hydrol. 2021, 594, 125962. [Google Scholar] [CrossRef]
- Xu, J.; Zhao, X.; Li, P.; Zhang, M. Stability of a 3D Unsaturated Vertical Cut Slope Subjected to Variable Rainfall Infiltration. Comput. Geotech. 2021, 134, 104110. [Google Scholar] [CrossRef]
- Zhang, L.; Gong, W.; Li, X.; Tan, X.; Zhao, C.; Wang, L. A Comparison Study between 2D and 3D Slope Stability Analyses Considering Spatial Soil Variability. J. Zhejiang Univ. Sci. A 2022, 23, 208–224. [Google Scholar] [CrossRef]
- Tozato, K.; Dolojan, N.L.J.; Touge, Y.; Kure, S.; Moriguchi, S.; Kawagoe, S.; Kazama, S.; Terada, K. Limit Equilibrium Method-Based 3D Slope Stability Analysis for Wide Area Considering Influence of Rainfall. Eng. Geol. 2022, 308, 106808. [Google Scholar] [CrossRef]
- Zheng, H. A Three-dimensional Rigorous Method for Stability Analysis of Landslides. Eng. Geol. 2012, 145–146, 30–40. [Google Scholar] [CrossRef]
- Li, A.J.; Merifield, R.S.; Lyamin, A.V. Three-Dimensional Stability Charts for Slopes Based on Limit Analysis Methods. Can. Geotech. J. 2010, 47, 1316–1334. [Google Scholar] [CrossRef]
- Michalowski, R.L. Limit Analysis and Stability Charts for 3D Slope Failures. J. Geotech. Geoenviron. Eng. 2010, 136, 583–593. [Google Scholar] [CrossRef]
- Nian, T.-K.; Huang, R.-Q.; Wan, S.-S.; Chen, G.-Q. Three-Dimensional Strength-Reduction Finite Element Analysis of Slopes: Geometric Effects. Can. Geotech. J. 2012, 49, 574–588. [Google Scholar] [CrossRef]
- Ugai, K.; Leshchinsky, D. Three-Dimensional Limit Equilibrium and Finite Element Analyses: A Comparison of Results. Soils Found. 1995, 35, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Zienkiewicz, O.C.; Humpheson, C.; Lewis, R.W. Associated and Non-Associated Visco-Plasticity and Plasticity in Soil Mechanics. Géotechnique 1975, 25, 671–689. [Google Scholar] [CrossRef]
- Sun, C.; Chai, J.; Xu, Z.; Qin, Y. 3D Stability Charts for Convex and Concave Slopes in Plan View with Homogeneous Soil Based on the Strength-Reduction Method. Int. J. Geomech. 2017, 17, 06016034. [Google Scholar] [CrossRef]
- Ietto, F.; Perri, F.; Cella, F. Weathering Characterization for Landslides Modeling in Granitoid Rock Masses of the Capo Vaticano Promontory (Calabria, Italy). Landslides 2018, 15, 43–62. [Google Scholar] [CrossRef]
- Perri, F.; Ietto, F.; Le Pera, E.; Apollaro, C. Weathering Processes Affecting Granitoid Profiles of Capo Vaticano (Calabria, Southern Italy) Based on Petrographic, Mineralogic and Reaction Path Modelling Approaches. Geol. J. 2016, 51, 368–386. [Google Scholar] [CrossRef]
- van Genuchten, M.T. A Closed-Form Equation for Predicting the Hydraulic Conductivity of Unsaturated Soils. Soil. Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Pan, Y.; Wu, G.; Zhao, Z.; He, L. Analysis of Rock Slope Stability under Rainfall Conditions Considering the Water-Induced Weakening of Rock. Comput. Geotech. 2020, 128, 103806. [Google Scholar] [CrossRef]
- Dawson, E.M.; Roth, W.H.; Drescher, A. Slope Stability Analysis by Strength Reduction. Géotechnique 1999, 49, 835–840. [Google Scholar] [CrossRef]
- Shen, H.; Klapperich, H.; Abbas, S.M.; Ibrahim, A. Slope Stability Analysis Based on the Integration of GIS and Numerical Simulation. Autom. Constr. 2012, 26, 46–53. [Google Scholar] [CrossRef]
- Xu, Y.; Li, J.; Fan, H.; Chen, L.; Zhao, Y.; Li, L. Stability Analysis of Clastic Rock Slope with Mudstone Interlayer Under Rainfall Infiltration. Geotech. Geol. Eng. 2017, 35, 1871–1883. [Google Scholar] [CrossRef]
- Rahardjo, H.; Ong, T.H.; Rezaur, R.B.; Leong, E.C. Factors Controlling Instability of Homogeneous Soil Slopes under Rainfall. J. Geotech. Geoenviron. Eng. 2007, 133, 1532–1543. [Google Scholar] [CrossRef]







| Stratum | Designation | Classification | Description | |
|---|---|---|---|---|
| Quaternary | Colluvial soil | Dt | Sandy soil | Coarse-grained soil with sub-angular gravel of sandstone. |
| Neogene | Weathered sandstone | WSs | Weathered rock | The hardness of weathered rock allows it to be broken with light hammer strikes. |
| Weathered Mudstone | Ms | Soft rock | Fragile mudstone interlayered with weathered sandstone. Some parts of the core are so weakened that they can be crumbled by hand. | |
| Sandstone | Ss | Soft rock | Fresh fine-grained sandstone. The hardness of rock allows it to be broken under medium hammer strikes. | |
| Friction Angle ° | Cohesion kPa | Poisson’s Ratio | Density kg/m3 | Young’s Modulus MPa | Permeability m/s | |
|---|---|---|---|---|---|---|
| Strongly weathered sandstone | 38 | 60 | 0.36 | 1800 | 200 | 6 × 10−6 |
| Moderately weathered sandstone | 40 | 80 | 0.30 | 2100 | 300 | 1 × 10−6 |
| Bedrock | 42 | 100 | 0.30 | 2200 | 600 | 5 × 10−7 |
| Group | Model | Undulation Degrees (m) Slope Gradient (50°) | FoS Rainfall Duration (h) | DF (%) | ||||
|---|---|---|---|---|---|---|---|---|
| 0 h | 24 h | 48 h | 0 h | 24 h | 48 h | |||
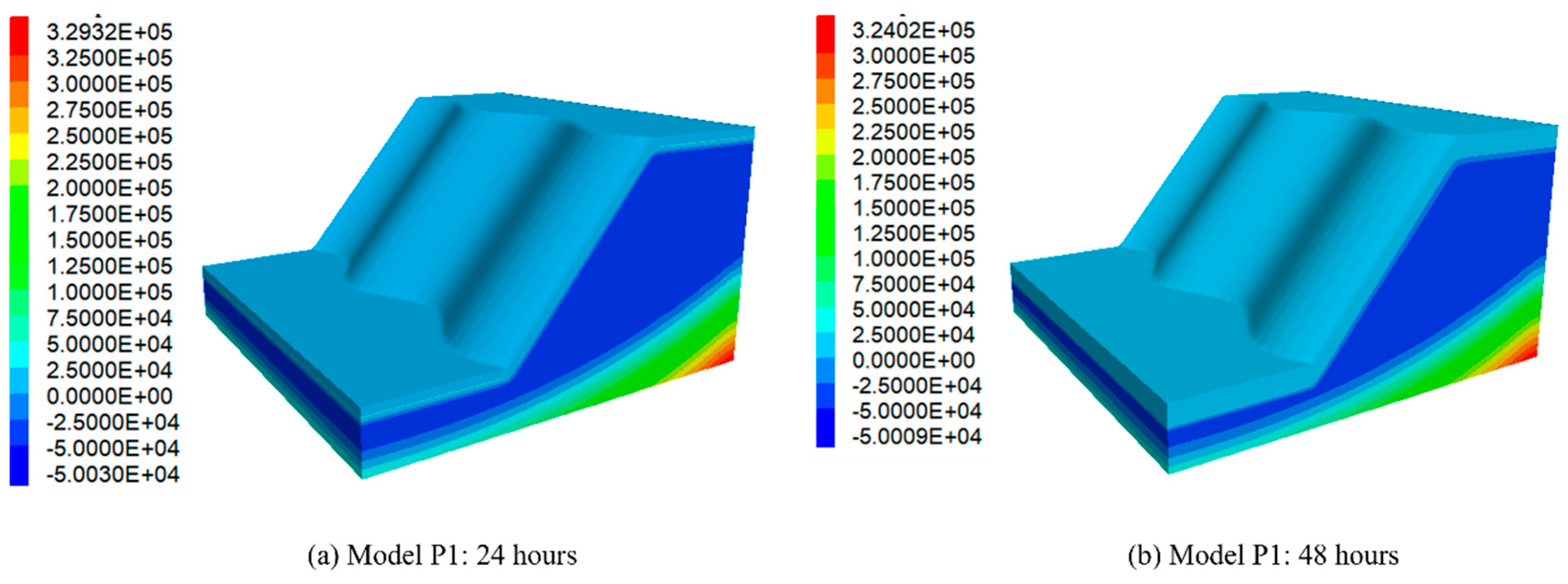
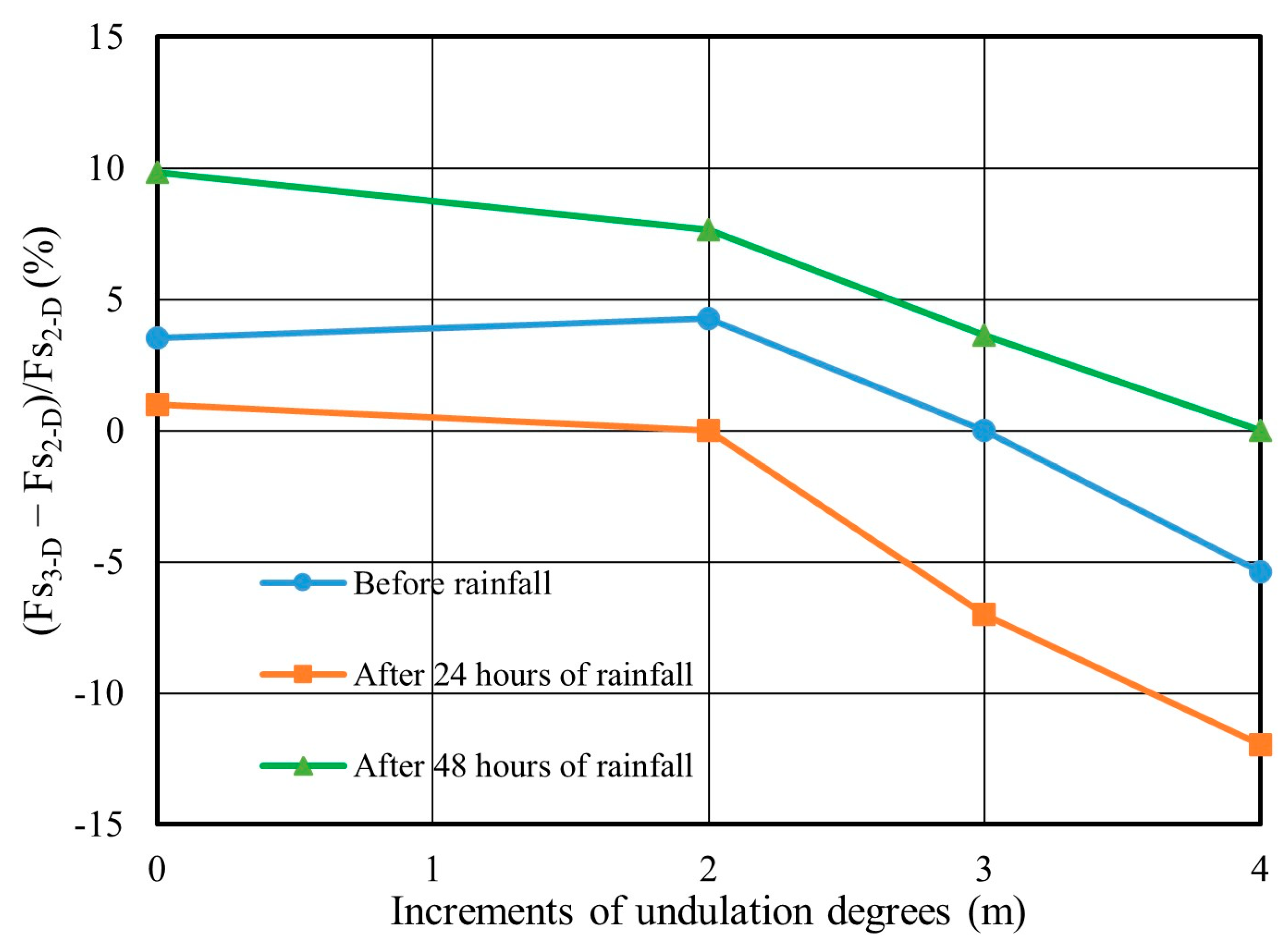
| 1 | P1 | 5, 5, 5, 5 | 2.133 | 1.629 | 1.395 | 3.54 | 0.99 | 9.84 |
| P2 | 5, 7, 9, 11 | 2.148 | 1.613 | 1.367 | 4.27 | 0.00 | 7.64 | |
| P3 | 5, 8, 11, 14 | 2.06 | 1.50 | 1.316 | 0.00 | −7.01 | 3.62 | |
| P4 | 5, 9, 13, 17 | 1.949 | 1.42 | 1.27 | −5.39 | −11.97 | 0.00 | |
| 2D analysis (slope gradient: 50°) | 2.06 | 1.613 | 1.27 | |||||
| Variation of slope gradients (°) | ||||||||
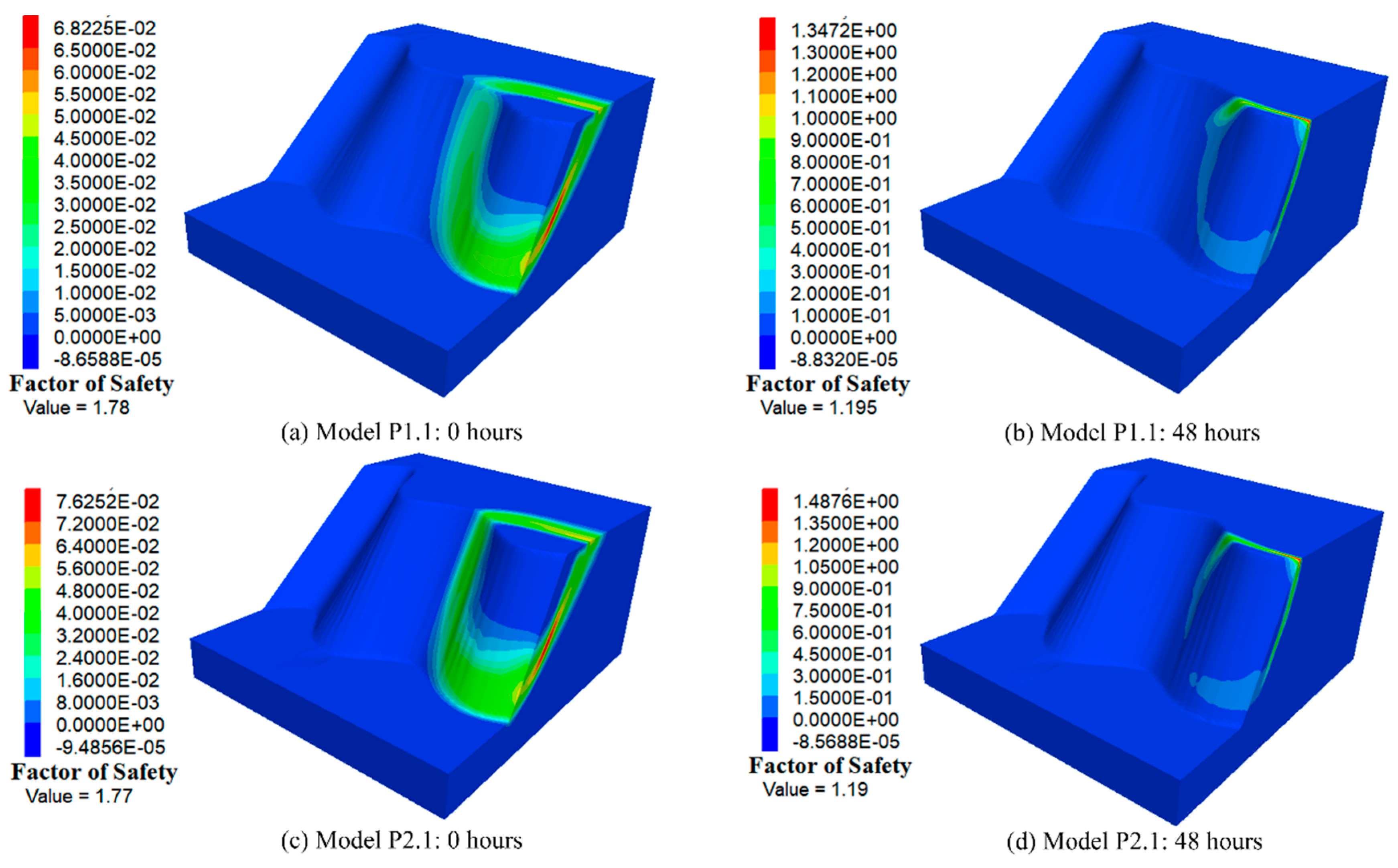
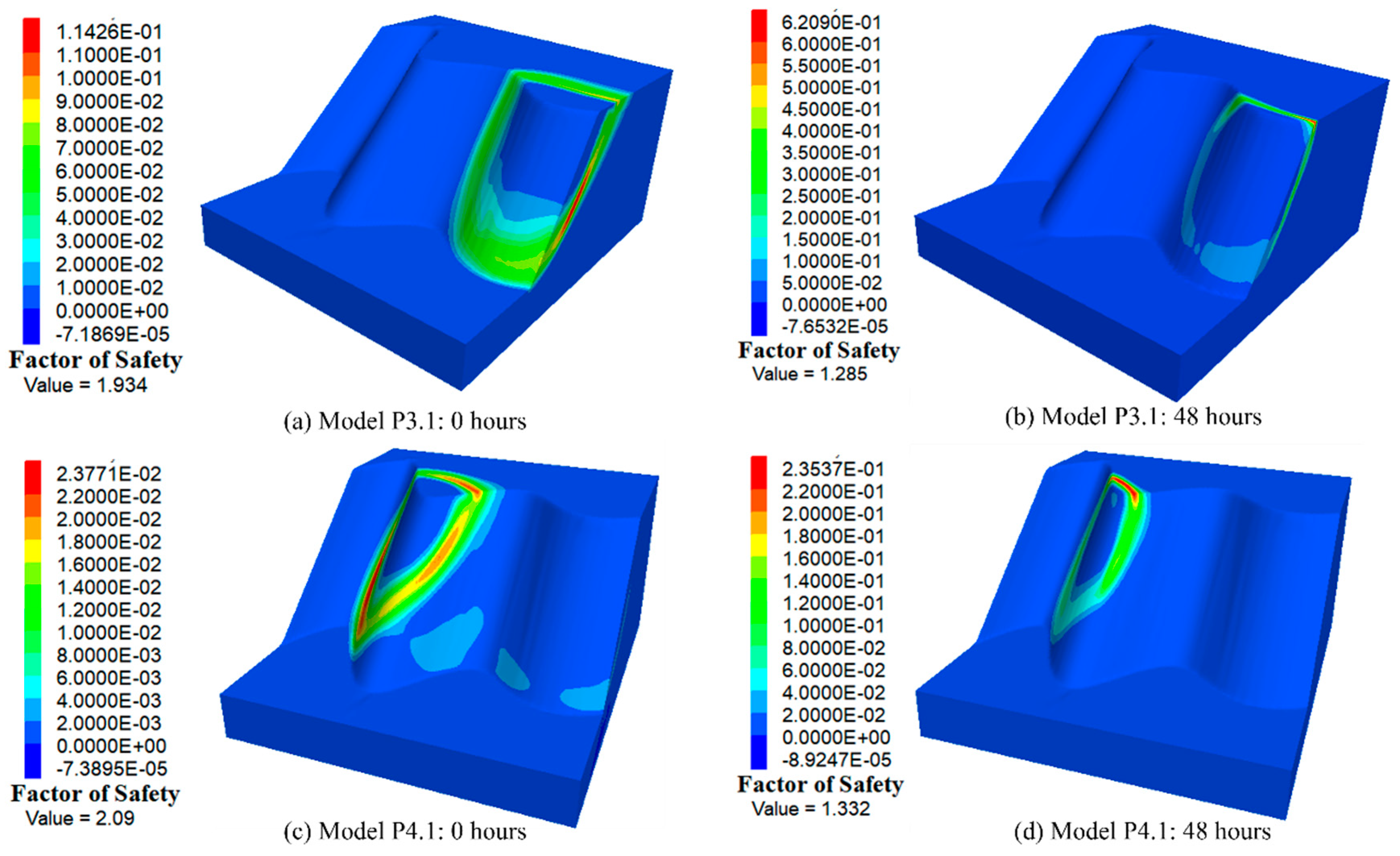
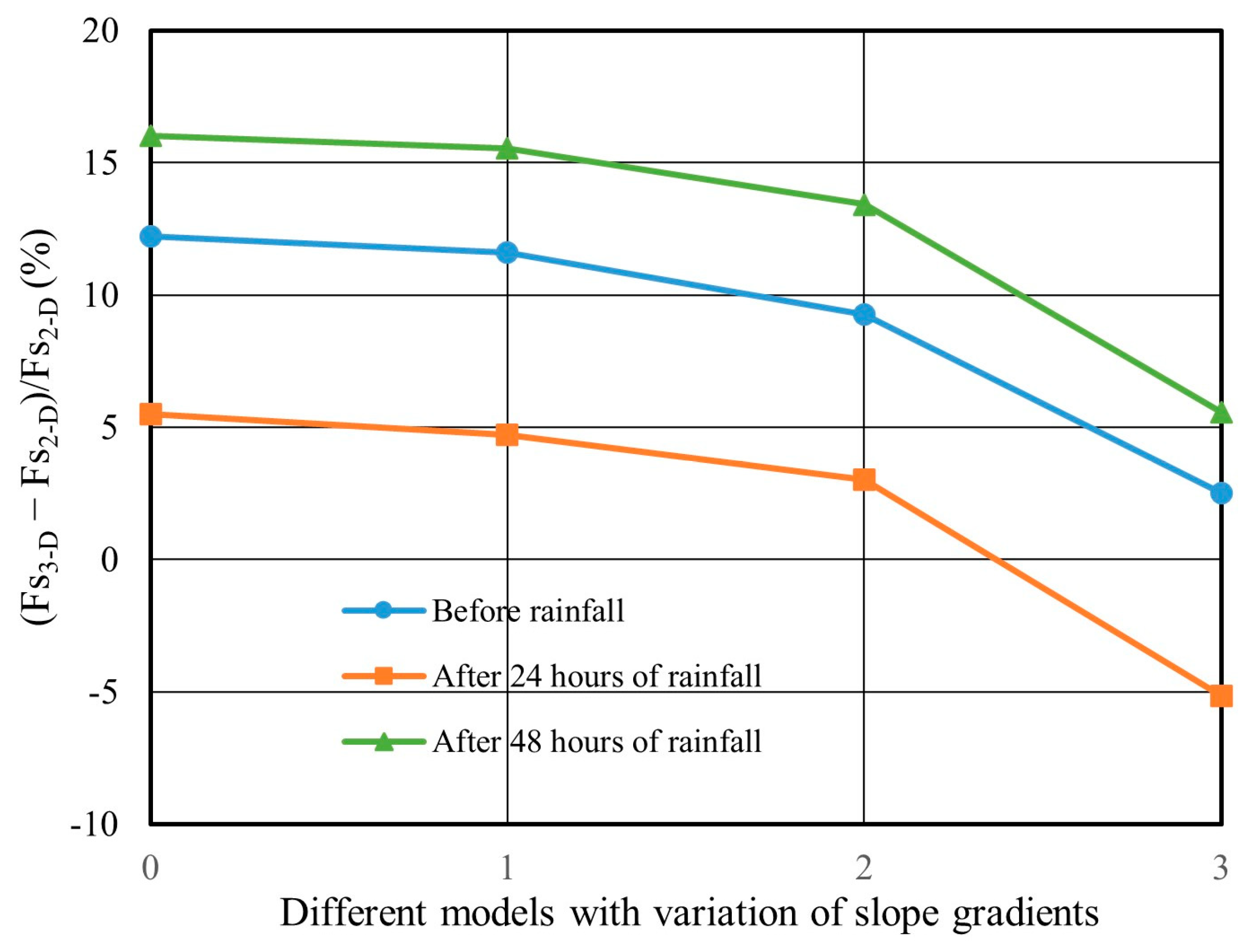
| 2 | P1.1 | 40, 48, 56, 64 | 1.78 | 1.340 | 1.195 | 12.23 | 5.51 | 16.02 |
| P2.1 | 40, 48, 56, 64 | 1.77 | 1.33 | 1.19 | 11.60 | 4.72 | 15.53 | |
| P3.1 | 40, 46, 52, 58 | 1.934 | 1.461 | 1.285 | 9.27 | 3.03 | 13.42 | |
| P4.1 | 40, 43, 46, 49 | 2.09 | 1.527 | 1.332 | 2.50 | −5.16 | 5.55 | |
| 2D analysis | 49 | 2.039 | 1.61 | 1.262 | ||||
| 58 | 1.77 | 1.418 | 1.133 | |||||
| 64 | 1.586 | 1.270 | 1.03 | |||||
| Group | Model | Frequencies | Degrees (m) | FoS Rainfall Duration (h) | DF (%) | ||||
|---|---|---|---|---|---|---|---|---|---|
| 0 h | 24 h | 48 h | 0 h | 24 h | 48 h | ||||
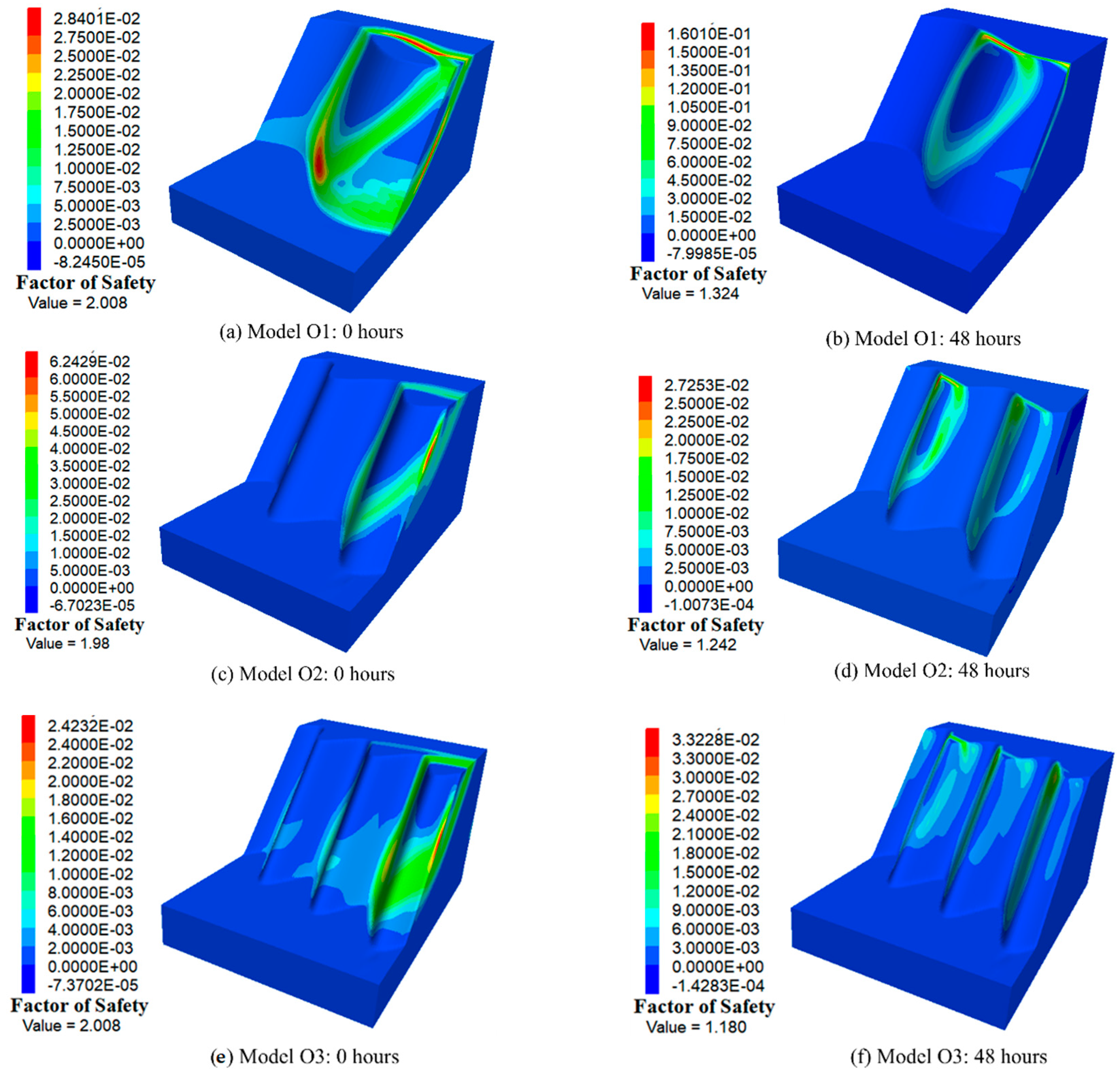
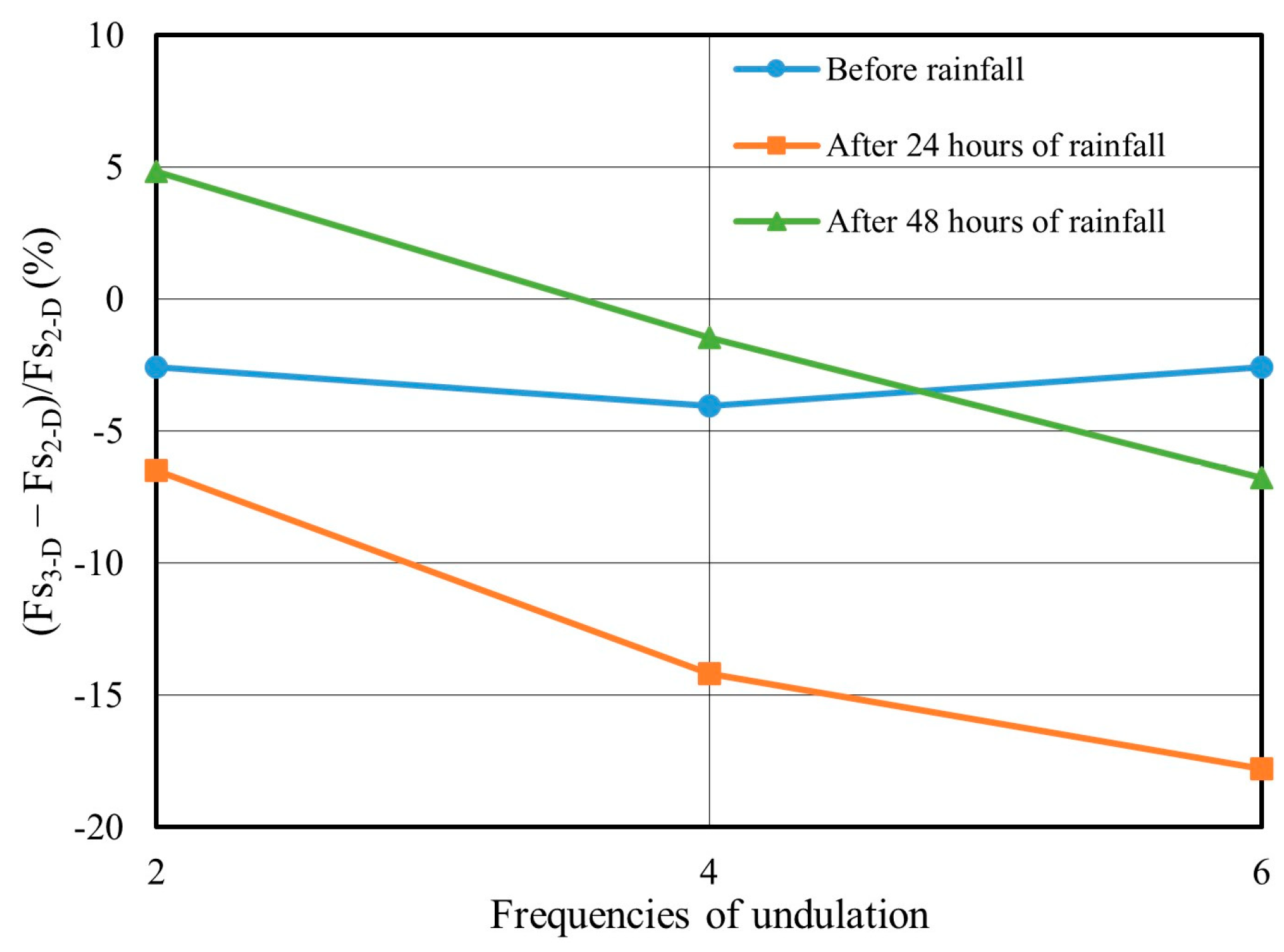
| 4 | O1 | 2 | 10 | 2.008 | 1.512 | 1.324 | −2.58 | −6.48 | 4.83 |
| O2 | 4 | 10 | 1.98 | 1.410 | 1.242 | −4.04 | −14.18 | −1.45 | |
| O3 | 6 | 10 | 2.008 | 1.367 | 1.180 | −2.58 | −17.78 | −6.78 | |
| 2D analysis | 2.06 | 1.61 | 1.26 | ||||||
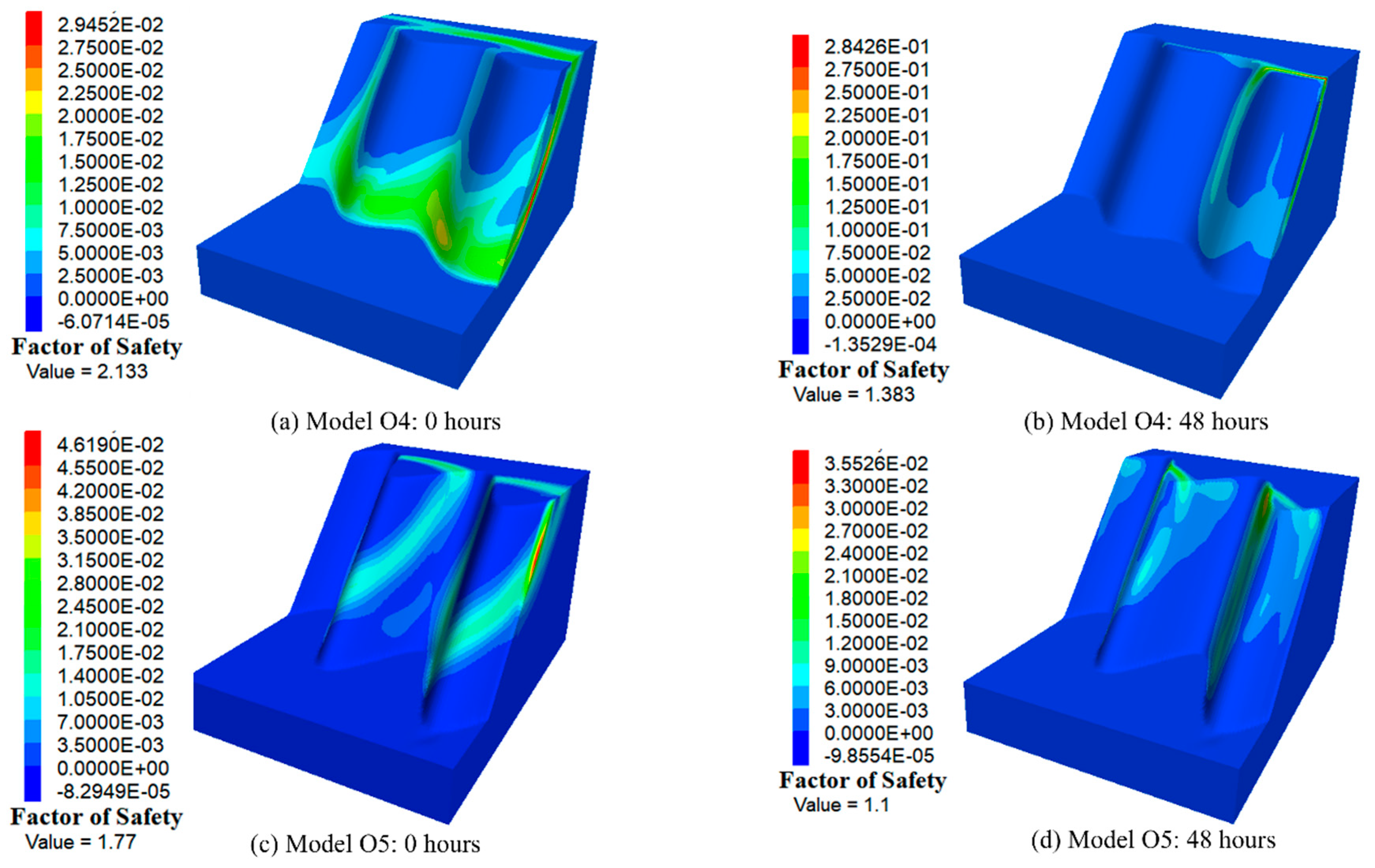
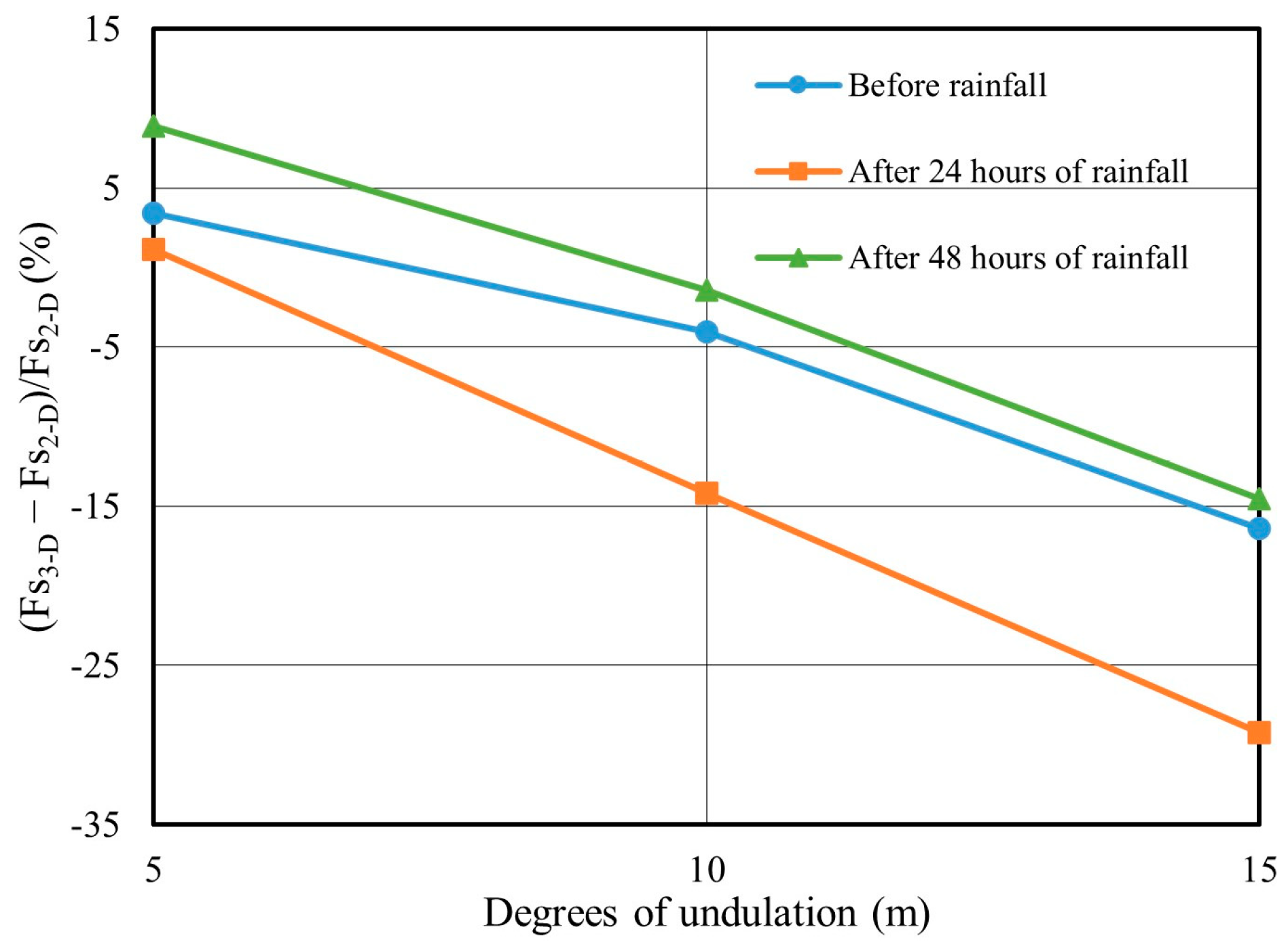
| 5 | O4 | 4 | 5 | 2.133 | 1.629 | 1.383 | 3.42 | 1.16 | 8.89 |
| O5 | 4 | 15 | 1.77 | 1.246 | 1.10 | −16.4 | −29.21 | −14.54 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Jiang, Y.; Sugimoto, S. Research on the Influence of Spatial Dimensions on the Stability of Large-Scale Slopes under Heavy Rainfall. GeoHazards 2024, 5, 732-754. https://doi.org/10.3390/geohazards5030037
Li X, Jiang Y, Sugimoto S. Research on the Influence of Spatial Dimensions on the Stability of Large-Scale Slopes under Heavy Rainfall. GeoHazards. 2024; 5(3):732-754. https://doi.org/10.3390/geohazards5030037
Chicago/Turabian StyleLi, Xun, Yujing Jiang, and Satoshi Sugimoto. 2024. "Research on the Influence of Spatial Dimensions on the Stability of Large-Scale Slopes under Heavy Rainfall" GeoHazards 5, no. 3: 732-754. https://doi.org/10.3390/geohazards5030037
APA StyleLi, X., Jiang, Y., & Sugimoto, S. (2024). Research on the Influence of Spatial Dimensions on the Stability of Large-Scale Slopes under Heavy Rainfall. GeoHazards, 5(3), 732-754. https://doi.org/10.3390/geohazards5030037








