Sideband Peak Count in a Vibro-Acoustic Modulation Method for Crack Detection
Abstract
:1. Introduction
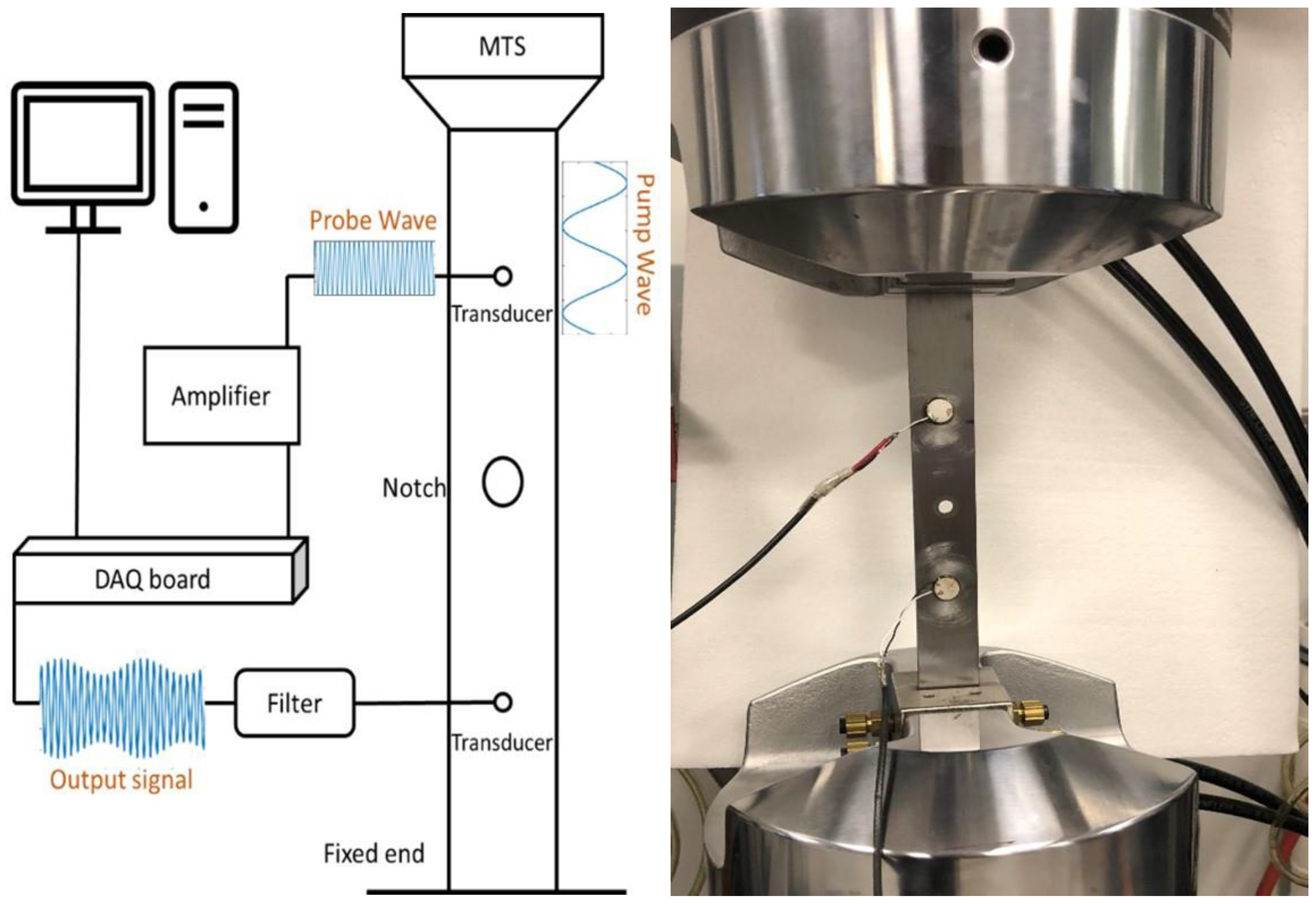
2. Configuration of the Experimental Setup
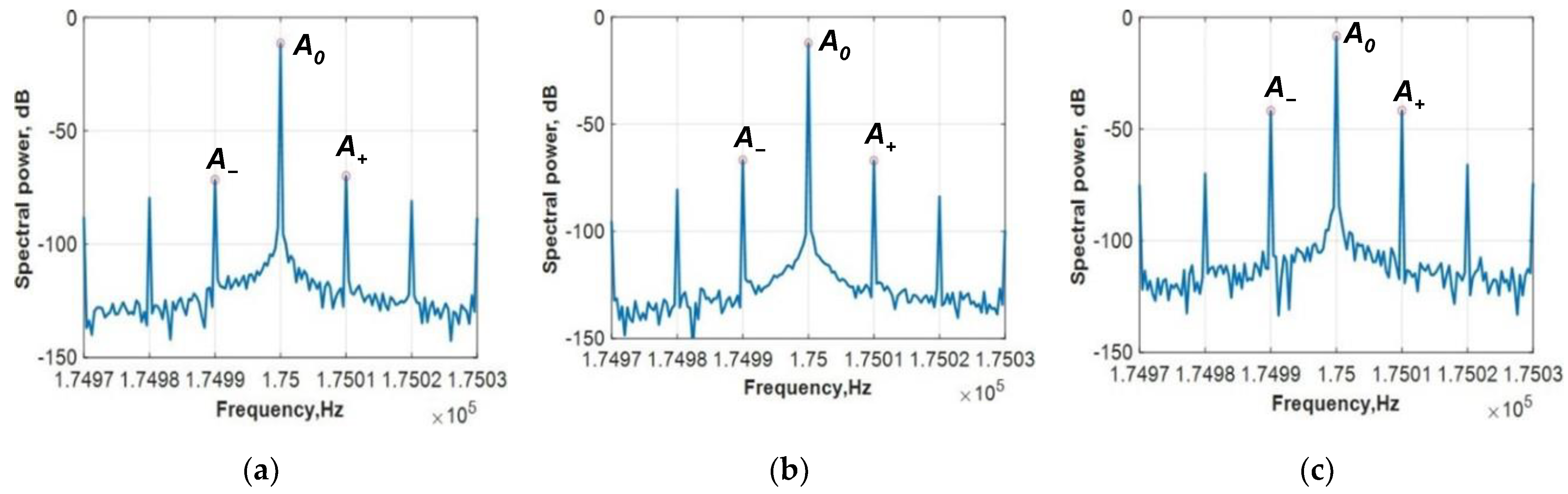
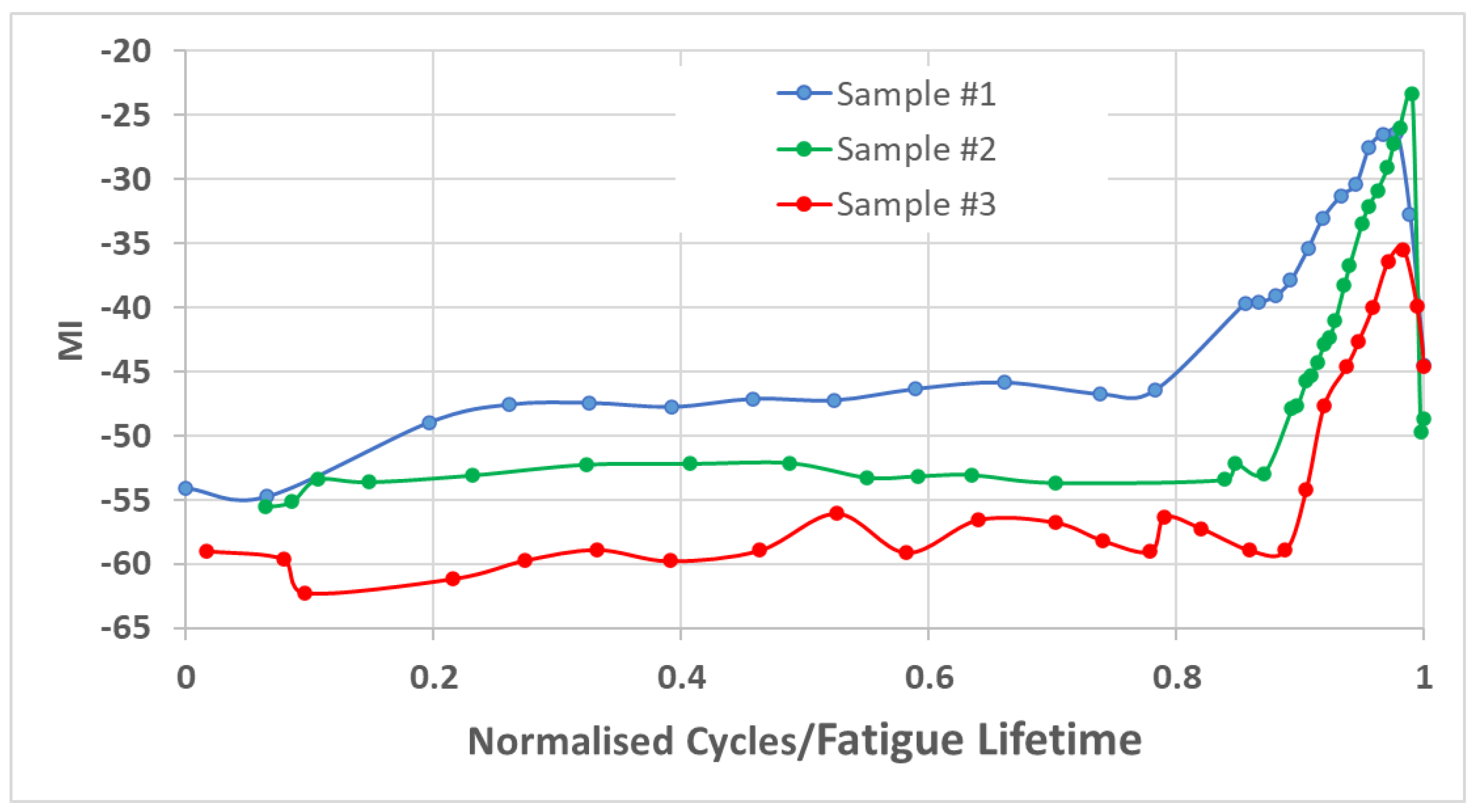
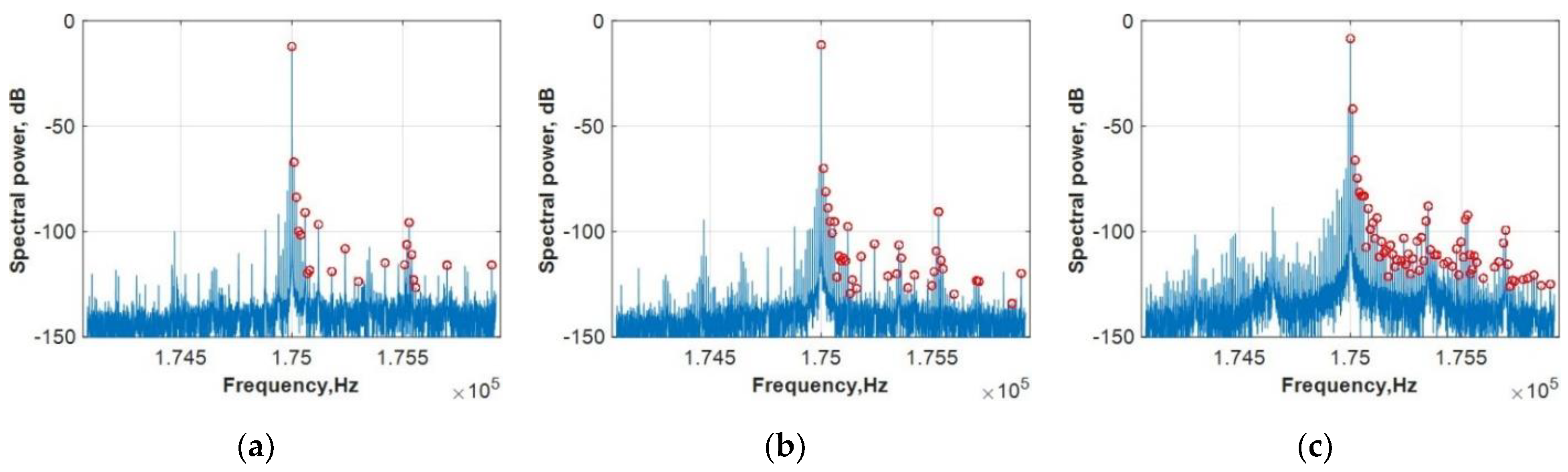
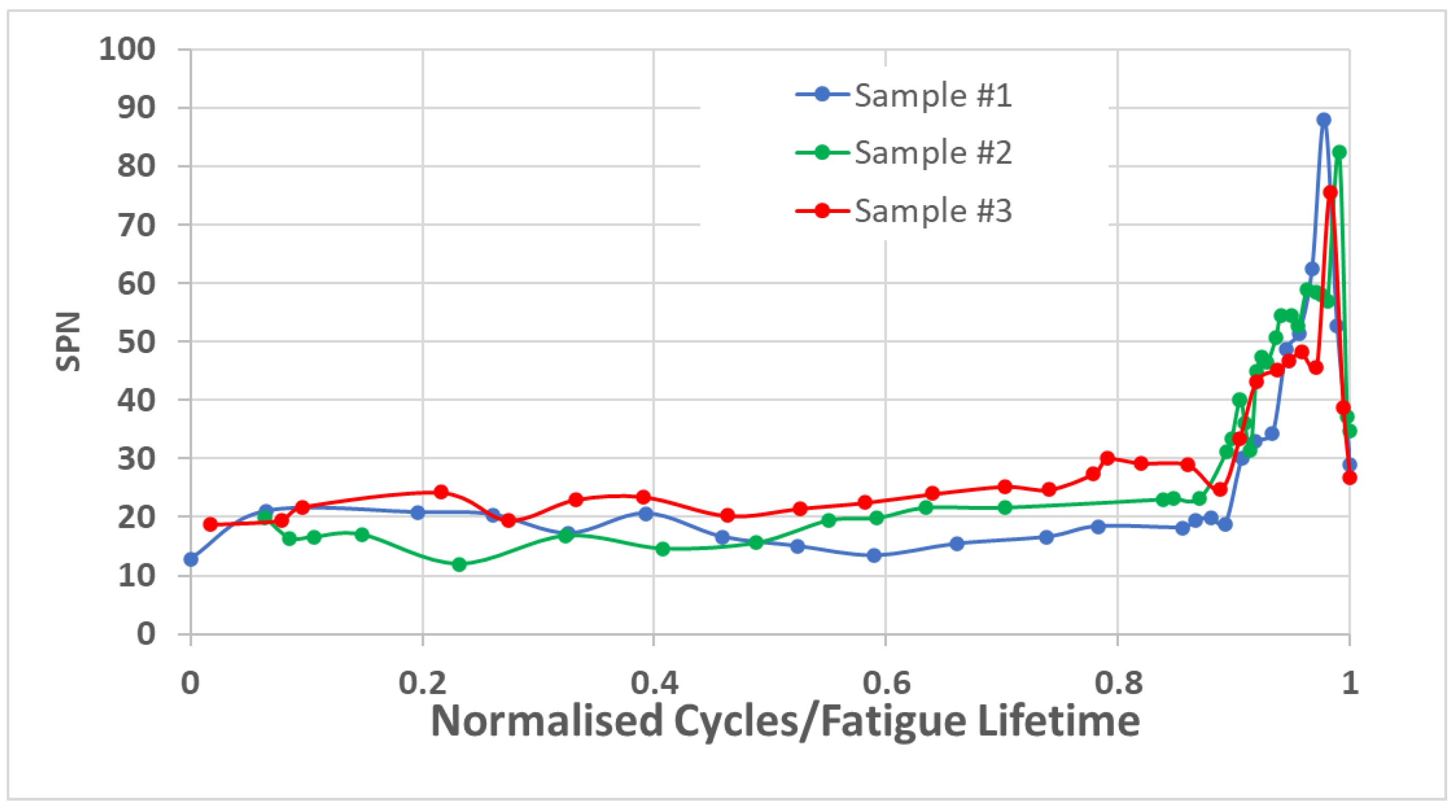
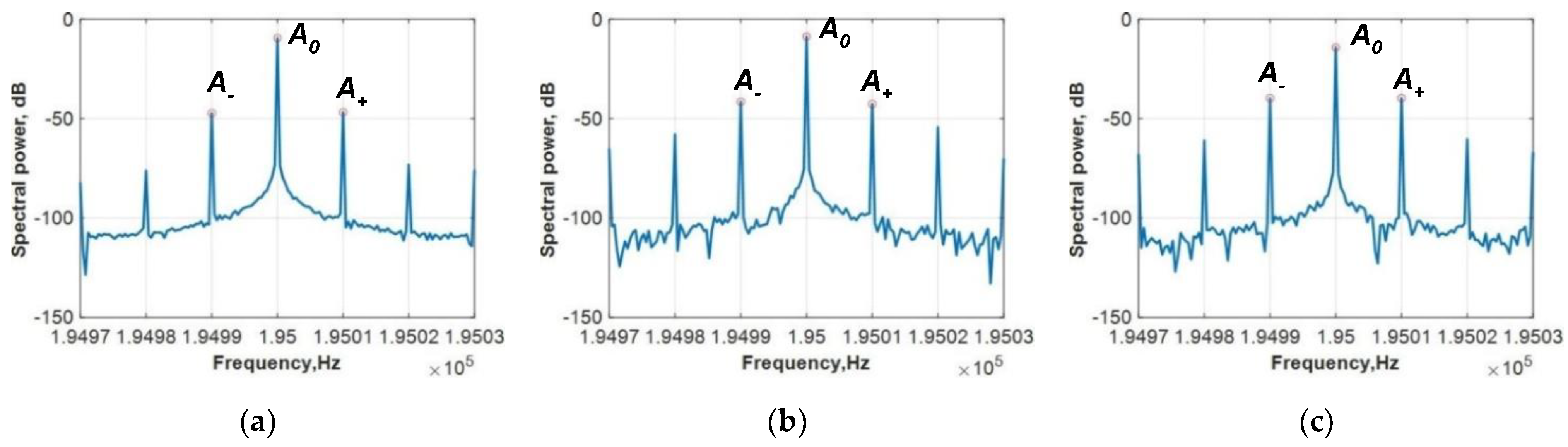
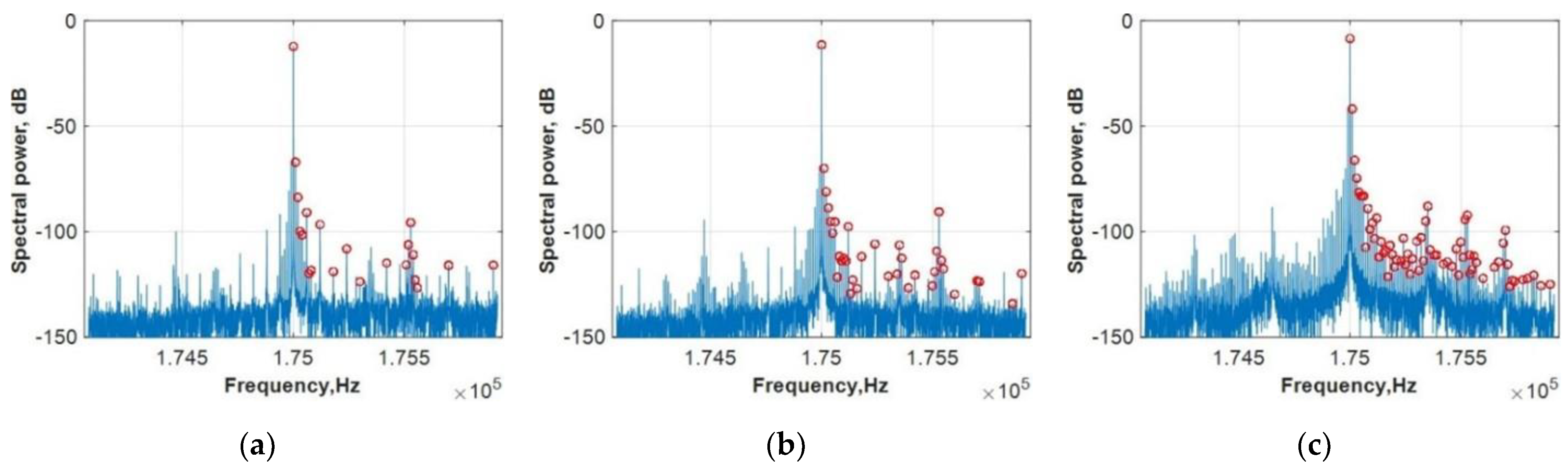
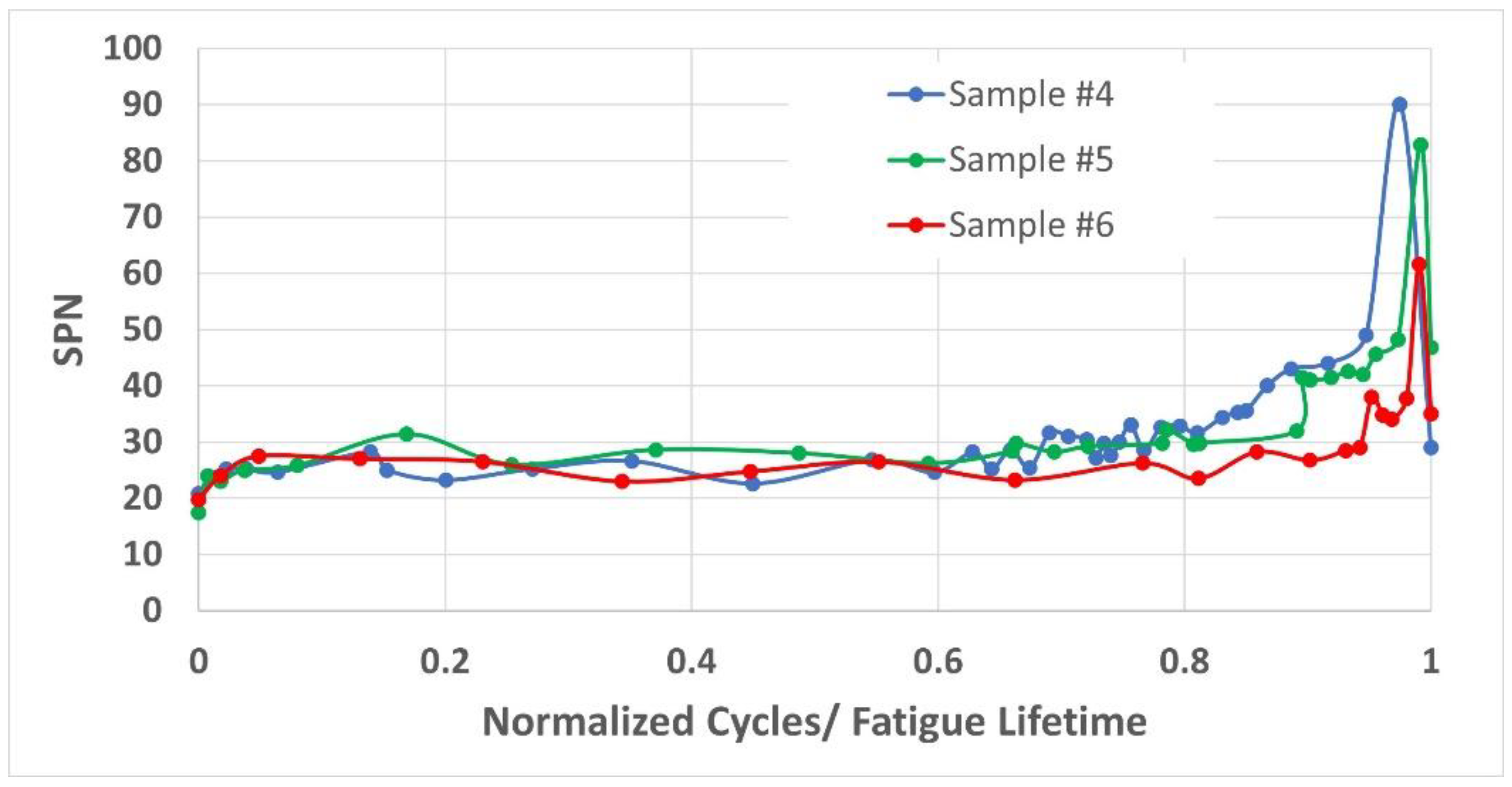
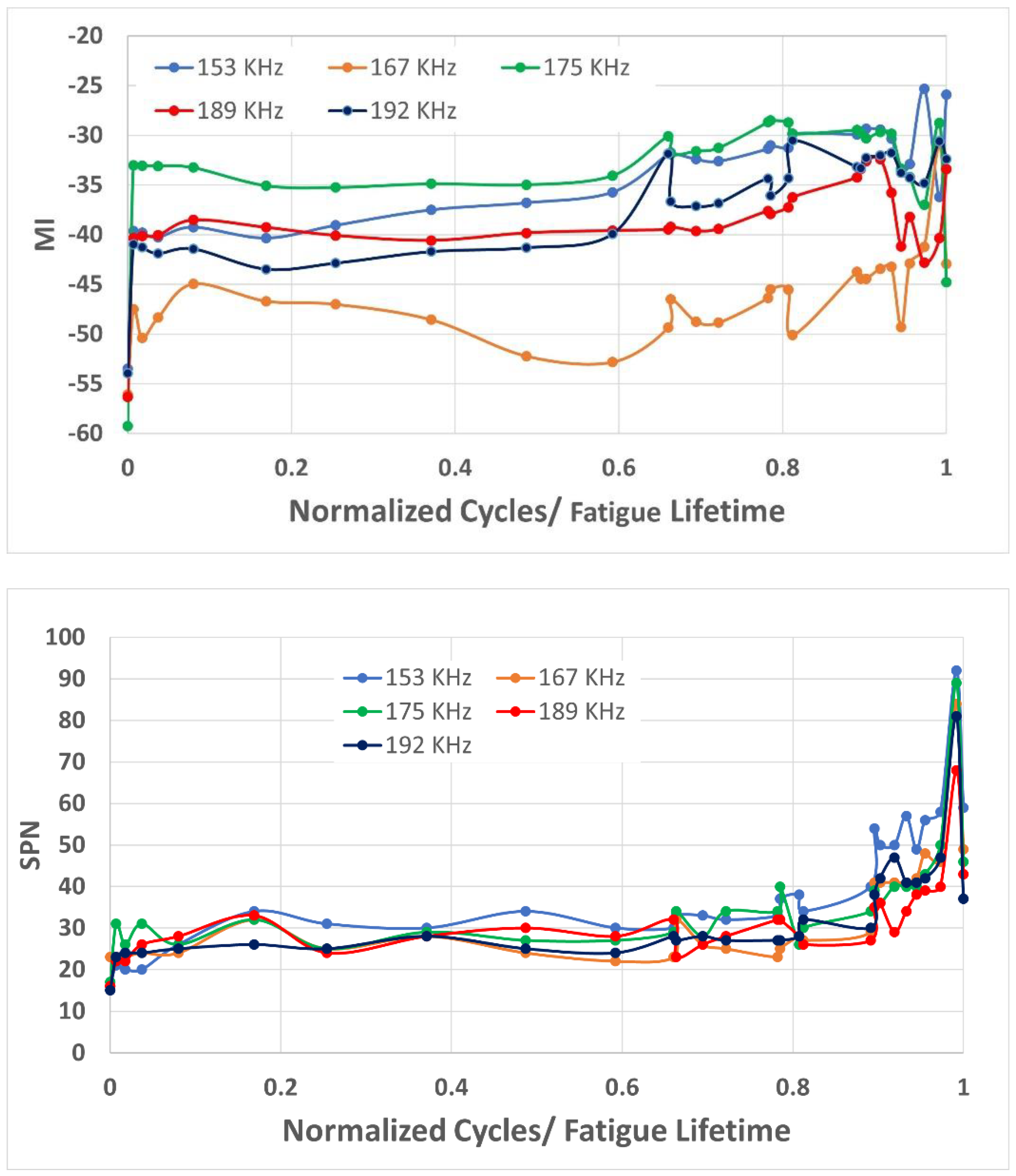
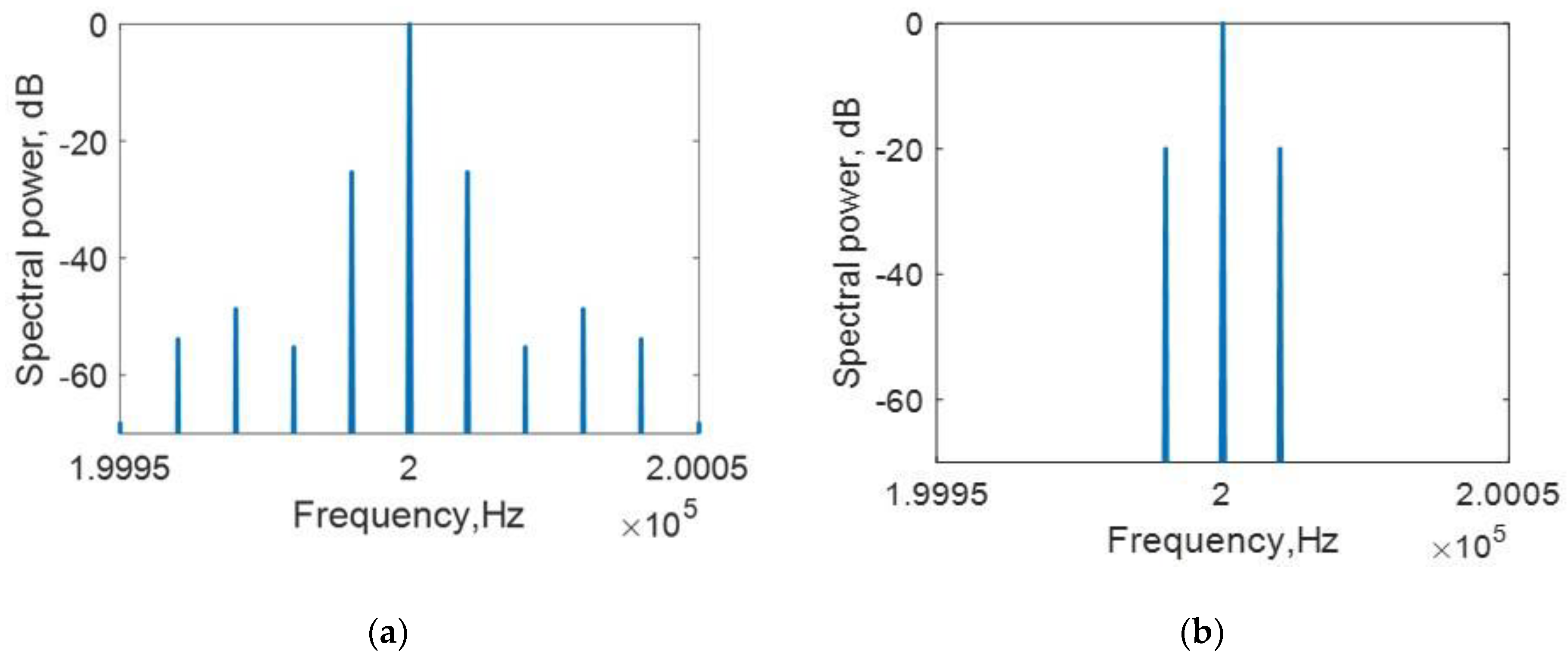
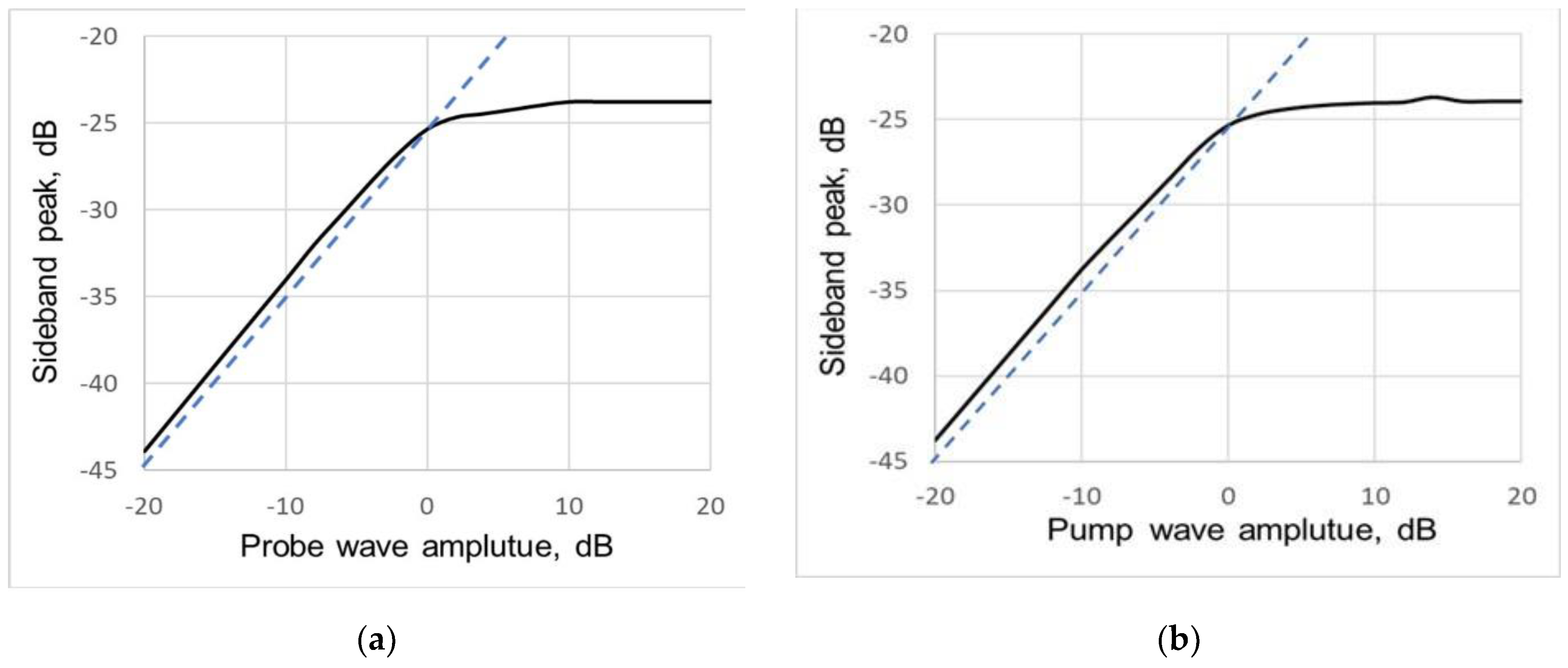
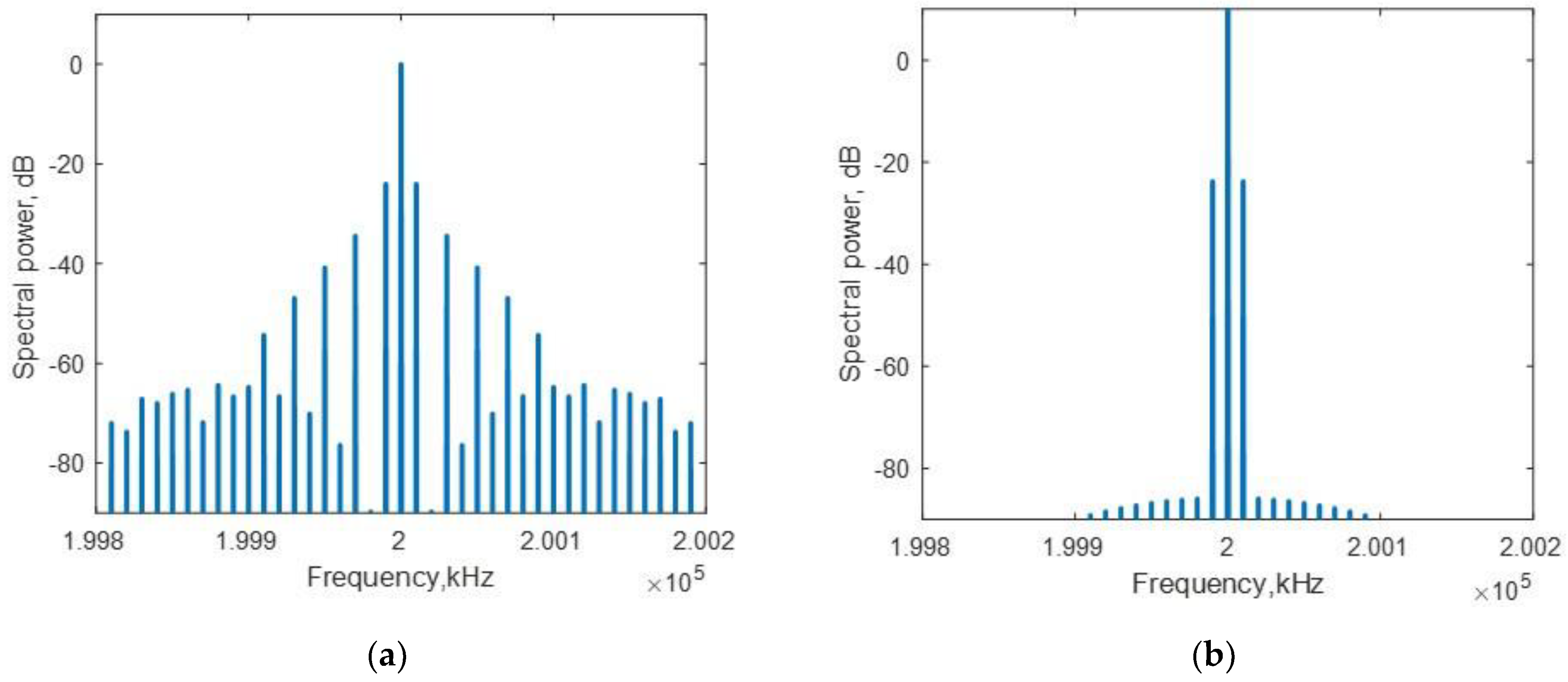
3. Results
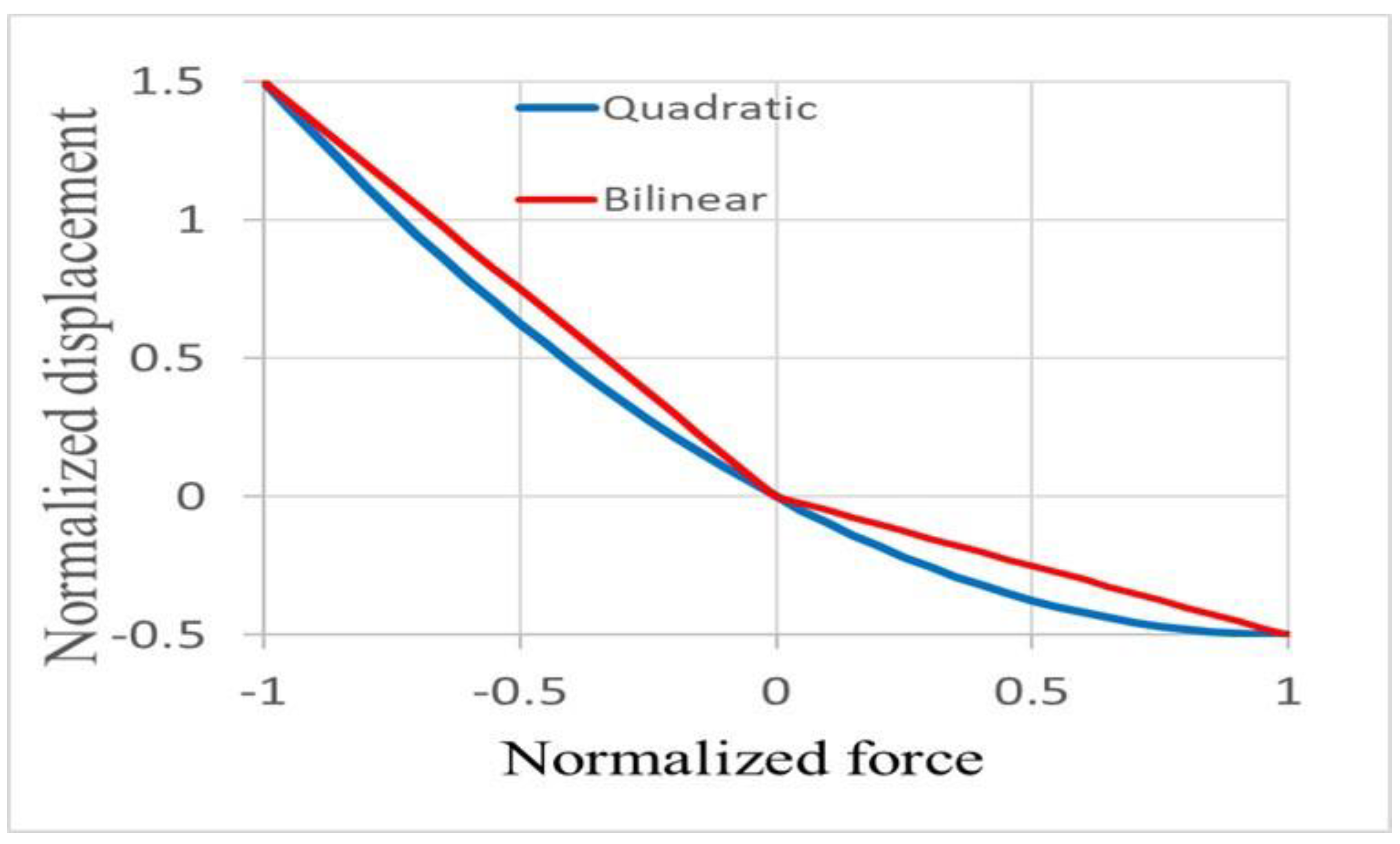
4. Bilinear Crack Model of Multiple Sideband Peak Interpretation
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Buck, O. Nonlinear Acoustic Properties of Structural Materials—A Review. In Review of Progress in Quantitative Nondestructive Evaluation; Thompson, D.O., Chimenti, D.E., Eds.; Springer: Boston, MA, USA, 1990; pp. 1677–1684. ISBN 978-1-4684-5774-2. [Google Scholar]
- Zheng, Y.; Maev, R.G.; Solodov, I.Y. Review/Sythèse Nonlinear Acoustic Applications for Material Characterization: A Review. Can. J. Phys. 2000, 77, 927–967. [Google Scholar] [CrossRef]
- Broda, D.; Staszewski, W.J.; Martowicz, A.; Uhl, T.; Silberschmidt, V.V. Modelling of Nonlinear Crack–Wave Interactions for Damage Detection Based on Ultrasound—A Review. J. Sound Vib. 2014, 333, 1097–1118. [Google Scholar] [CrossRef]
- Sutin, A.M.; Salloum, H. Interaction of Acoustic and Electromagnetic Waves in Nondestructive Evaluation and Medical Applications. Radiophys. Quantum Electron. 2020, 63, 40–54. [Google Scholar] [CrossRef]
- Klepka, A.; Pieczonka, L.; Dziedziech, K.; Staszewski, W.J.; Aymerich, F.; Uhl, T. Structural Damage Detection Based on Nonlinear Acoustics: Application Examples. In Nonlinear Ultrasonic and Vibro-Acoustical Techniques for Nondestructive Evaluation; Kundu, T., Ed.; Springer International Publishing: Cham, Switzerland, 2019; pp. 139–174. ISBN 978-3-319-94476-0. [Google Scholar]
- Krohn, N.; Pfleiderer, K.; Stoessel, R.; Solodov, I.; Busse, G. Nonlinear Acoustic Imaging: Fundamentals, Methodology, and NDE-Applications. In Proceedings of the Acoustical Imaging; Arnold, W., Hirsekorn, S., Eds.; Springer: Dordrecht, The Netherlands, 2004; pp. 91–98. [Google Scholar]
- Guyer, R.A.; Johnson, P.A. Nonlinear Mesoscopic Elasticity: The Complex Behaviour of Rocks, Soil, Concrete; John Wiley & Sons: Hoboken, NJ, USA, 2009; ISBN 978-3-527-40703-3. [Google Scholar]
- Kundu, T.; Eiras, J.N.; Li, W.; Liu, P.; Sohn, H.; Payá, J. Fundamentals of Nonlinear Acoustical Techniques and Sideband Peak Count. In Nonlinear Ultrasonic and Vibro-Acoustical Techniques for Nondestructive Evaluation; Kundu, T., Ed.; Springer International Publishing: Cham, Switzerland, 2019; pp. 1–88. ISBN 978-3-319-94476-0. [Google Scholar]
- Jin, J.; Rivière, J.; Ohara, Y.; Shokouhi, P. Dynamic Acousto-Elastic Response of Single Fatigue Cracks with Different Microstructural Features: An Experimental Investigation. J. Appl. Phys. 2018, 124, 075303. [Google Scholar] [CrossRef]
- Bergman, R.H.; Shahbender, R.A. Effect of Statically Applied Stresses on the Velocity of Propagation of Ultrasonic Waves. J. Appl. Phys. 1958, 29, 1736–1738. [Google Scholar] [CrossRef]
- Toupin, R.A.; Bernstein, B. Sound Waves in Deformed Perfectly Elastic Materials. Acoustoelastic Effect. J. Acoust. Soc. Am. 1961, 33, 216–225. [Google Scholar] [CrossRef]
- Korotkov, A.S.; Slavinskij, M.M.; Sutin, A.M. Variations of acoustic nonlinear parameters with the concentration of defects in steel. Akust. Zurnal 1994, 40, 84–87. [Google Scholar]
- Klepka, A.; Dziedziech, K.; Mrówka, J.; Górski, J. Experimental Investigation of Modulation Effects for Contact-Type Interfaces in Vibro-Acoustic Modulation Tests. Struct. Health Monit. 2021, 20, 917–930. [Google Scholar] [CrossRef]
- Hu, H.F.; Staszewski, W.J.; Hu, N.Q.; Jenal, R.B.; Qin, G.J. Crack Detection Using Nonlinear Acoustics and Piezoceramic Transducers—Instantaneous Amplitude and Frequency Analysis. Smart Mater. Struct. 2010, 19, 065017. [Google Scholar] [CrossRef]
- Li, N.; Wang, F.; Song, G. New Entropy-Based Vibro-Acoustic Modulation Method for Metal Fatigue Crack Detection: An Exploratory Study. Measurement 2020, 150, 107075. [Google Scholar] [CrossRef]
- Ballad, E.M.; Vezirov, S.Y.; Pfleiderer, K.; Solodov, I.Y.; Busse, G. Nonlinear Modulation Technique for NDE with Air-Coupled Ultrasound. Ultrasonics 2004, 42, 1031–1036. [Google Scholar] [CrossRef] [PubMed]
- Lee, S.E.; Lim, H.J.; Sohn, H.; Hong, J.W. Excitation Conditions for Nonlinear Ultrasonic Wave Modulation Technique. In KKHTCNN; Chulalongkorn University: Bangkok, Thailand, 2015. [Google Scholar]
- Dziedziech, K.; Klepka, A.; Roemer, J.; Pieczonka, L. Experimental Study of Thermo-Acoustic Wave Modulation in a Cracked Plate. J. Sound Vib. 2021, 498, 115970. [Google Scholar] [CrossRef]
- Golchinfar, B.; Ramezani, M.G.; Donskoy, D.; Saboonchi, H. Vibro-Acoustic Modulation Technique Comparison with Conventional Nondestructive Evaluation Methods. In Health Monitoring of Structural and Biological Systems XIV; SPIE: Bellingham, WC, USA, 2020; Volume 11381, pp. 187–198. [Google Scholar]
- Korotkov, A.S.; Sutin, A.M. Modulation of Ultrasound by Vibrations in Metal Constructions with Cracks. Acoust. Lett. 1994, 18, 59–62. [Google Scholar]
- Sutin, A.M.; Donskoy, D.M. Vibro-Acoustic Modulation Nondestructive Evaluation Technique. In Nondestructive Evaluation of Aging Aircraft, Airports, and Aerospace Hardware II; SPIE: Bellingham, WC, USA, 1998; Volume 3397, pp. 226–237. [Google Scholar]
- Duffour, P.; Morbidini, M.; Cawley, P. Comparison between a Type of Vibro-Acoustic Modulation and Damping Measurement as NDT Techniques. NDT E Int. 2006, 39, 123–131. [Google Scholar] [CrossRef]
- Meyer, J.J.; Adams, D.E. Theoretical and Experimental Evidence for Using Impact Modulation to Assess Bolted Joints. Nonlinear Dyn. 2015, 81, 103–117. [Google Scholar] [CrossRef]
- Donskoy, D.; Zagrai, A.; Chudnovsky, A.; Golovin, E.; Agarwala, V. Damage Assessment with Nonlinear Vibro-Acoustic Modulation Technique. In In American Society of Mechanical Engineers Digital Collection, Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Las Vegas, NV, USA, 4–7 September 2007; ASME: New York, NY, USA, 2009; pp. 1949–1956. [Google Scholar]
- Liu, B.; Luo, Z.; Gang, T. Influence of Low-Frequency Parameter Changes on Nonlinear Vibro-Acoustic Wave Modulations Used for Crack Detection. Struct. Health Monit. 2018, 17, 218–226. [Google Scholar] [CrossRef]
- Jia, J.; Hu, H.; Tao, L.; Yang, D. Analysis of Load Effect on Nonlinear Vibro-Acoustic Modulation Used in on-Line Monitoring of Fatigue Cracks. Smart Mater. Struct. 2017, 26, 095048. [Google Scholar] [CrossRef]
- Boll, B.; Willmann, E.; Fiedler, B.; Meißner, R.H. Weak Adhesion Detection—Enhancing the Analysis of Vibroacoustic Modulation by Machine Learning. Compos. Struct. 2021, 273, 114233. [Google Scholar] [CrossRef]
- Castellano, A.; Fraddosio, A.; Piccioni, M.D.; Kundu, T. Linear and Nonlinear Ultrasonic Techniques for Monitoring Stress-Induced Damages in Concrete. J. Nondestruct. Eval. Diagn. Progn. Eng. Syst. 2021, 4, 041001. [Google Scholar] [CrossRef]
- Donskoy, D.; Golchinfar, B.; Ramezani, M.; Rutner, M.; Hassiotis, S. Vibro-acoustic amplitude and frequency modulations during fatigue damage evolution. In AIP Conference Proceedings; AIP Publishing LLC: Melville, NY, USA, 2019; Volume 2102, p. 040004. [Google Scholar]
- Ramezani, M.G.; Golchinfar, B.; Donskoy, D.; Hassiotis, S.; Venkiteela, G. Steel Material Degradation Assessment via Vibro-Acoustic Modulation Technique. Transp. Res. Rec. 2019, 2673, 579–585. [Google Scholar] [CrossRef]
- Donskoy, D.M. Nonlinear Acoustic Methods. In Encyclopedia of Structural Health Monitoring; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2009; ISBN 978-0-470-06162-6. [Google Scholar]
- Liu, P.; Sohn, H. Damage Detection Using Sideband Peak Count in Spectral Correlation Domain. J. Sound Vib. 2017, 411, 20–33. [Google Scholar] [CrossRef]
- Alnuaimi, H.; Amjad, U.; Park, S.; Russo, P.; Lopresto, V.; Kundu, T. An Improved Nonlinear Ultrasonic Technique for Detecting and Monitoring Impact Induced Damage in Composite Plates. Ultrasonics 2022, 119, 106620. [Google Scholar] [CrossRef] [PubMed]
- Frau, A.; Aymerich, F.; Porcu, M.; Pieczonka, L.; Klepka, A.; Staszewski, W. Probing WaveFrequency Selection for the Nonlinear Vibro-Acoustic Wave Modulation Tests. AIAS–Assoc. Ital. L’analisi Sollecitazioni 2014, 43, 9–12. [Google Scholar]
- Zagrai, A.; Donskoy, D.; Lottiaux, J. N-Scan®: New Vibro-Modulation System for Crack Detection, Monitoring and Characterization. In AIP Conference Proceedings; AIP Publishing LLC: Melville, NY, USA, 2004; Volume 700, pp. 1414–1421. [Google Scholar] [CrossRef]
- Zaitsev, V.Y.; Matveev, L.A.; Matveyev, A.L. Elastic-Wave Modulation Approach to Crack Detection: Comparison of Conventional Modulation and Higher-Order Interactions. NDT E Int. 2011, 44, 21–31. [Google Scholar] [CrossRef]
- Dimarogonas, A.D. Vibration of Cracked Structures: A State of the Art Review. Eng. Fract. Mech. 1996, 55, 831–857. [Google Scholar] [CrossRef]
- Bovsunovsky, A.; Surace, C. Non-Linearities in the Vibrations of Elastic Structures with a Closing Crack: A State of the Art Review. Mech. Syst. Signal Processing 2015, 62–63, 129–148. [Google Scholar] [CrossRef]
- Wauer, J. On the Dynamics of Cracked Rotors: A Literature Survey. Appl. Mech. Rev. 1990, 43, 13–17. [Google Scholar] [CrossRef]
- Chu, Y.C.; Shen, M.-H.H. Analysis of Forced Bilinear Oscillators and the Application to Cracked Beam Dynamics. AIAA J. 1992, 30, 2512–2519. [Google Scholar] [CrossRef]
- Ostrovsky, L.A.; Starobinets, I.M. Transitions and Statistical Characteristics of Vibrations in a Bimodular Oscillator. Chaos 1995, 5, 496–500. [Google Scholar] [CrossRef]


| Mechanical Properties | Value |
|---|---|
| Density | 0.284 Ib/in3 |
| Ultimate Tensile Strength | 63,800 psi |
| Yield Tensile Strength | 53,700 psi |
| Shear Strength | 43,500 psi |
| Melting Point | 2590–2670 °F |
| Sample | Number of Cycles to Failure |
|---|---|
| Sample #1 | 46,102 |
| Sample #2 | 120,074 |
| Sample #3 | 52,106 |
| Sample #4 | 67,637 |
| Sample #5 | 67,712 |
| Sample #6 | 70,603 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alnutayfat, A.; Hassiotis, S.; Liu, D.; Sutin, A. Sideband Peak Count in a Vibro-Acoustic Modulation Method for Crack Detection. Acoustics 2022, 4, 74-86. https://doi.org/10.3390/acoustics4010005
Alnutayfat A, Hassiotis S, Liu D, Sutin A. Sideband Peak Count in a Vibro-Acoustic Modulation Method for Crack Detection. Acoustics. 2022; 4(1):74-86. https://doi.org/10.3390/acoustics4010005
Chicago/Turabian StyleAlnutayfat, Abdullah, Sophia Hassiotis, Dong Liu, and Alexander Sutin. 2022. "Sideband Peak Count in a Vibro-Acoustic Modulation Method for Crack Detection" Acoustics 4, no. 1: 74-86. https://doi.org/10.3390/acoustics4010005
APA StyleAlnutayfat, A., Hassiotis, S., Liu, D., & Sutin, A. (2022). Sideband Peak Count in a Vibro-Acoustic Modulation Method for Crack Detection. Acoustics, 4(1), 74-86. https://doi.org/10.3390/acoustics4010005






