Pre-Sabine Room Acoustic Guidelines on Audience Rake, Stage Acoustics, and Dimension Ratios
Abstract
1. Introduction
- Acoustically dissonant (“kat-echountes”). This would be associated with prevalent diffusion or scattering.
- Reverberant (“peri-echountes”).
- Echoic (“anti-echountes”). This would be associated with noticeable, unwanted echoes.
- Acoustically consonant (“syn-echountes”). This would be associated with a supportive acoustic, beneficial reflections, being desirable for theatres.
2. Audience Rake
2.1. Marcus Vitruvius Pollio (20 BC)
2.2. Johann Rhode (1800)
Here it is proper to inquire whether in a theater of curvilinear rake in which the seats are arranged as steps, one above another, any echo of the sound can at all become perceptible. I deny the possibility of it. The sound in such a building never encounters at the same instant of time a resisting mass from which a reflection, sufficient to be perceptible to the hearing, could be possible. It strikes the first range of seats before the second, and the echo from the first is lost before that from the second has arisen, and so on up to the top. The ancients, inasmuch as all their theatres had this arrangement, cannot have depended at all upon the reflex echo of sound.
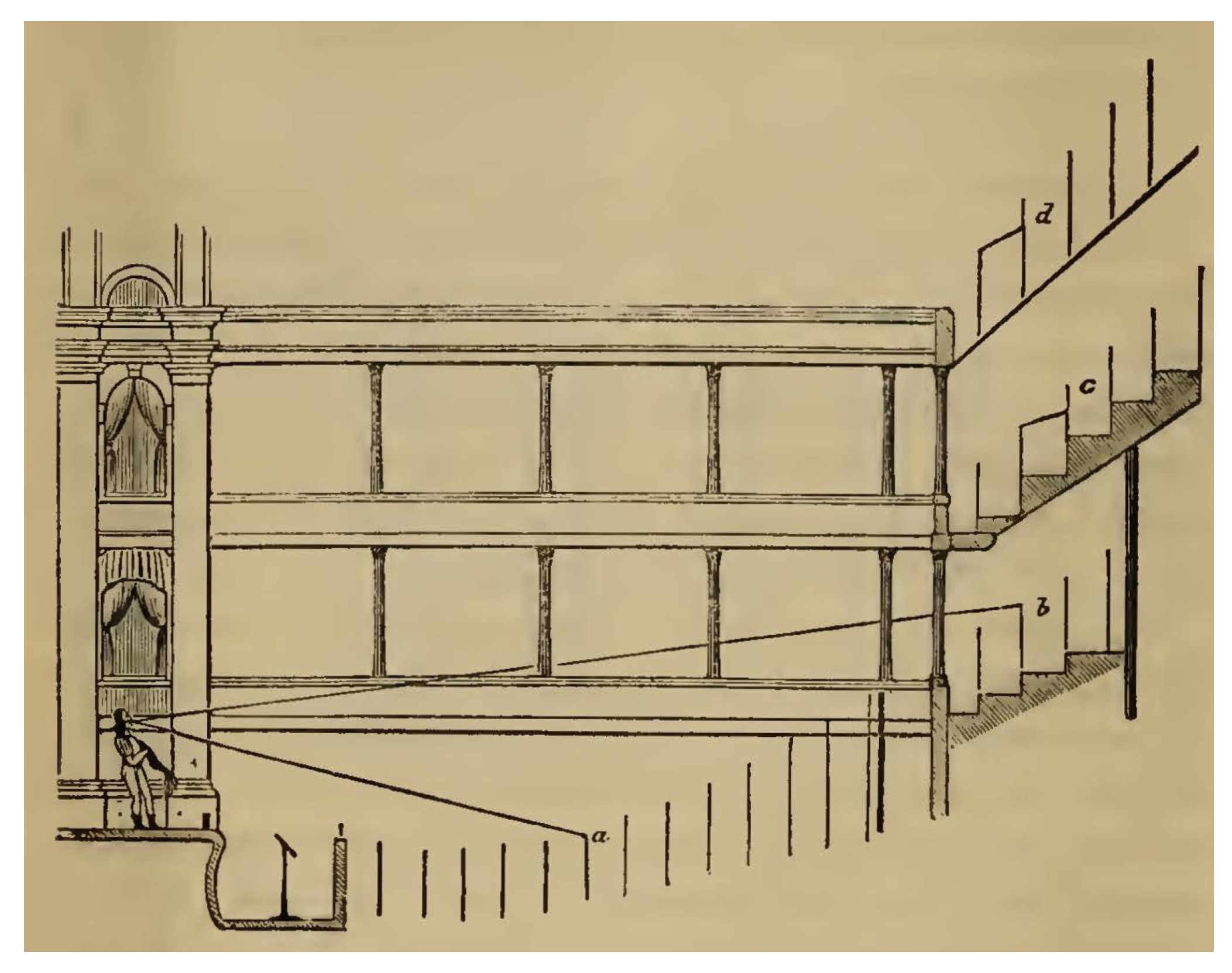
2.3. John Scott Russell (1839)
- The position and height of the speaking person and of the auditor occupying the first seat are determined.
- A line is drawn from the speaking person’s mouth touching the point where the top of the first auditor’s head comes.
- At the proper distance the situation of the seat for the second auditor is to be marked and on the line indicating the back of it a height of 1 foot 6 inches ( ) or less is to be set off upwards from the point where the ray from the speaker’s mouth touched; this is to mark the position of the top of the head of the second auditor.
- By repeating this process, adding 18 inches ( ) at each seat, a series of points are found all ranged in a curve (which Russell called the isacoustic, or equally-hearing curve).
- Each seat will be placed about 2 ft 9 inches ( ) below the mark indicating the top of the head of its occupant, and the floor on which each stands should be about 1 foot 6 inches ( ) lower still.
2.4. Théodore Lachèz (1848)
- The more the object to be seen is elevated, the less inclination the seats for the auditory require; the more the seats for the auditory are elevated, the lower the object to be seen can be placed.
- But the distance from the auditory to the object to be seen exercises a considerable influence upon the inclination of the seats, and the elevation of the point of sight.
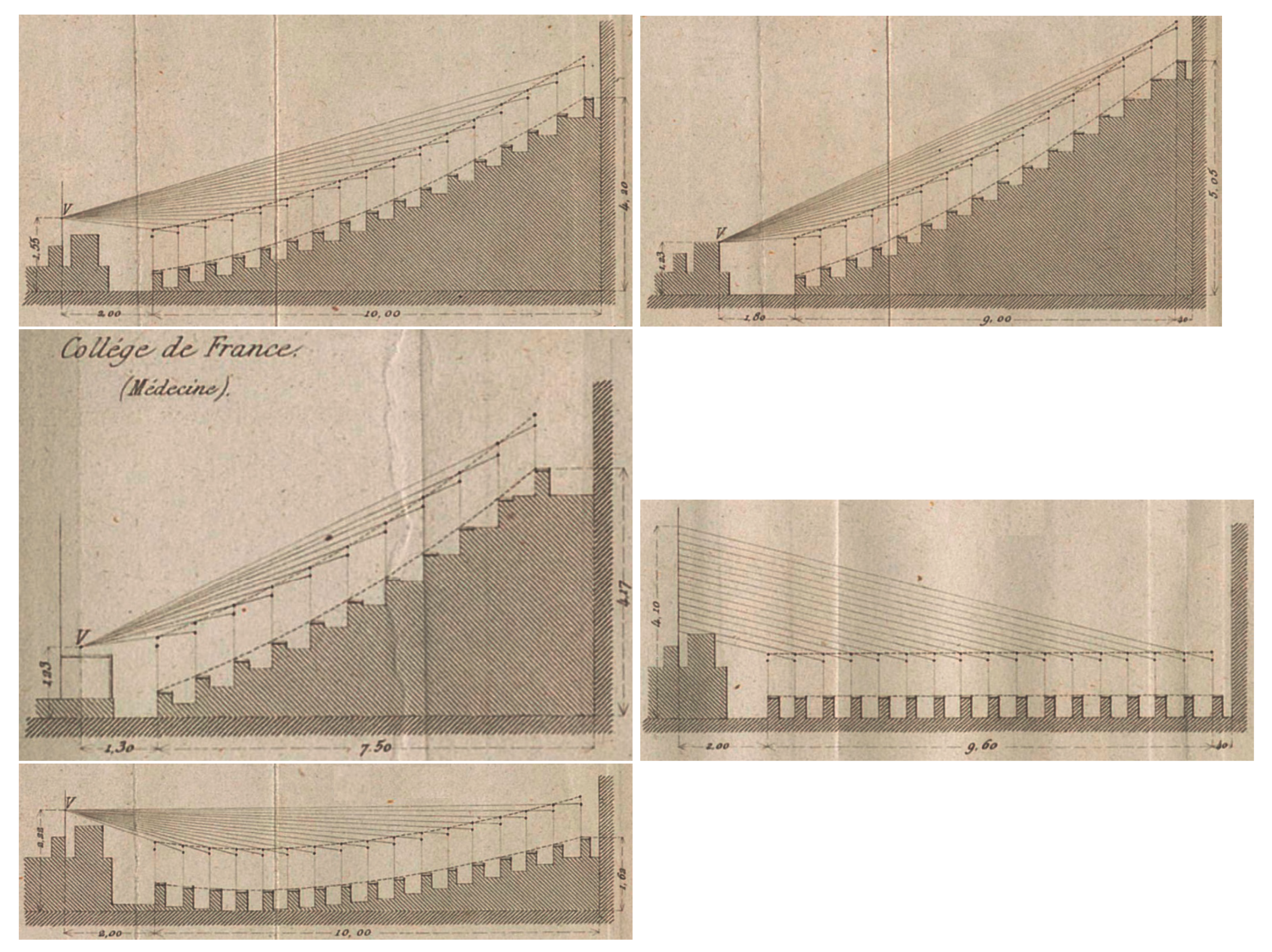
2.5. Practical Examples
- Two of the rooms in the building for the Collegiate Department of the University of Pennsylvania were designed by Professor A.D. Bache in 1830 following this principle [19].
- After the forming of the Free Church of Scotland in 1843, several new churches adopted Scott Russell’s curve for the seating layout [14].
- One of the classrooms attached to St. Martin’s Hall, designed by Richard Westmacott (1847–1867), used this principle to ensure that the teacher could be distinctly heard and seen by the entire class [14].
- The orchestra and choir seating for the Handel Festival at the Crystal Palace in London (1854) adopted a rake according to this principle [14] (Note, it is quite possible that John Scott Russell was involved in the design of this event as he was secretary to the building committee for its original erection in 1851 and closely involved in its re-erection at Sydenham Hill in 1854. However, direct evidence for this theorem was not uncovered during the course of this study). The acoustics of this concert hall has received mixed reactions [20].
- The houses of Parliament in Vienna (1883) designed by Baron von Hansen (The architect of the Vienna Musikverein) [21].
- The Chicago Auditorium (1889), designed by architects Louis Sullivan and Dankmar Adler [22]. The Auditorium Theatre is known for its good acoustics.
- New Welsh presbyterian chapel, Ruthin, North Wales (1889) [23].
3. Stage Acoustics and Proscenium Opening Width
3.1. Carl Friedrich Langhans (1810)
- Places without a sufficient view of the stage should be avoided.
- Places on the sides of the stage should not be as far away as those directly facing the stage, in order to decrease the number of audience members who have a view of the stage and the scenery from the side.
- The stage opening width should not be too large, otherwise, due to the orientation of the actor to the audience at the sides, the intelligibility and transmission of the speech from an actor on the extreme side of the stage would be disadvantageous.
3.2. Ernst Florenz Friedrich Chladni (1826)
Not long after the inauguration of the Leipzig concert hall, the conductor Hiller in a rehearsal positioned both choirs during C.P.E. Bach’s two-choir Heilig Cantata away from one other, the one on the stage and the other in the loges opposite, to see its acoustical effect. He stood in the middle of the hall. He was unable to establish ensemble singing between both choirs, as one choir heard the singing of the other too late. He came to the conclusion that both choirs need to be positioned on the stage.
3.3. Johann Wetter (1829)
3.4. Practical Examples
- The Iffland Theater, Berlin (1802–1817): The architect, Carl Gotthard Langhans, stated that the size of a theater depends primarily on the stage opening width [29]. He looked at other theaters in Europe and found that in Paris, in London, and in all of Italy, none of the stage opening widths were over 40 to 43 ft ( to ), except for St. Carlos in Naples which according to Langhans Sr. was 48 ft ( ). He ignored this theater as it was merely an opera house. Based on the limitation of the stage opening width and historical voice directivity experiments [7], Langhans chose the theater’s elliptical shape. He stated that since the stage opening determines the diameter of the circular line, one must still try to gain as much space as possible, it follows quite naturally that this results in an ellipse, which also followed the contemporary rules of room acoustics. Despite the design’s intentions, the acoustics turned out to be poor, with disturbing perceivable focusing effects [26].
- Academy of Music, Philadelphia (1857): The architects Napoleon LeBrun and Gustavus Runge designed the opera house with a stage opening of 48 ft () [30]. They employed the rationale that direct sound and first order reflections should reach the auditor as loudly as possible and that disturbing reflections should be prevented. In order to reinforce the first order reflections, they employed “resonating” materials for the stage area. In order to determine which reflections were disturbing they employed “echo theory”. Finally, the architects considered a greater stage opening width detrimental for the acoustics, as it complicates ensemble playing of the actors and the sound “would lose itself in the stage” instead of reaching the audience area. Various conductors have given mixed reviews on the quality of the orchestral sound in the hall [2].
- Victoria Theater, Berlin (1859–1891): The architectural output of Langhans Jr. includes several rooms with specific acoustical demands such as the Stadttheater in Liegnitz (now Legnica, 1839), the Opera in Breslau (now Wroclaw, 1841), Herzogliches Hoftheater in Dessau-Roßlau (1856–1922), the Victoria Theater in Berlin (1859–1891), and the Stadttheater in Leipzig (1867–1945). During the course of this study no documents were uncovered that describe the acoustic concepts behind the Liegnitz, Breslau and Dessau rooms. However, Langhans did describe the acoustic concept of the Victoria Theater in the Zeitschrift für Bauwesen of 1860 [31]. The Victoria Theater was an original architectural experiment which combined a summer and winter theater (i.e., an auditorium with two stages of which one was in the open). Langhans reduced the stage opening from 50 to 38 ft (15.2 to 11.6 ).
4. Length-Width-Height Ratio
4.1. William Gardiner (1841)
4.2. Scott Russell (1847)
4.3. Jabez Upham (1852)
The proportions as well as the form of a music room, are not a matter of indifference. We have already noticed the tendency of one vibrating body or medium to throw another, in contact or in its immediate vicinity, into a similar state of vibration. In this way the oscillations of the contained air of a room, communicated to its walls, produce therein a sympathetic vibration, which will be more or less perfect according as the structure of these walls, their subdivisions and general relations of length, width, and height, approximate to the acoustic conditions required. From this comes resonance, as we understand, the existence of which, to a considerable extent, in some rooms gives to the voice that peculiar brilliancy and resilient power which every singer must have noticed.
4.4. Thomas Roger Smith (1861)
Suppose, now, that the dimensions of the room bear to one another some simple ratio—say that of height 1, width 2, and length 4—it will follow that the repetitions caused by these echoes will strike the ear of any hearer in any part of the room at equally recurring intervals. For instance, in a room 28 ft ( ) high, 56 ft ( ) wide, and 112 ft ( ) long, the echo in each direction would follow paths of which that from floor to ceiling and back would be 56 ft long, and consequently could be traversed 20 times in a second; that from one side to the other and back would be 112 ft long ( ) and traversed 10 times in a second; and that from end to end and back 224 ft ( ), and traversed 5 times in a second. There would be other echoes audible, varying with the relative positions of the hearer, and the point where the sound was made, but the equable recurrence of those just enumerated would be what would excite sonorous agitation in the air.
Supposing, however, the dimensions such, that reflections, as they successively strike the ear, come always at irregular and quite incommensurable intervals, that is to say that the pulsation passing and repassing from side to side will very seldom coincide with those passing from end to end, or from floor to ceiling, the result will be that, from the moment that the sound proper to the room and excited in it by the echoes of any other sound, begins to appear, a noise, and not a note, will be audible.
4.5. Practical Examples
- Theater of the Royal Institution (1802): The lecture hall’s dimension are in very simple relation to one another, the height, length, and width being in the ratio 2:3:4 respectively, and within a few inches giving dimensions of 30, 45, and 60 feet (, , and ). Smith [14] called this one of the reasons for its room acoustic excellence.
- Boston Music Hall (1852): Based on the previously presented theory, the dimensions of the Boston Music Hall were designed to be 130 ft () long, 78 ft () wide, and 65 ft () high, a ratio of 10:6:5 [35]. Higginson (owner of the Boston Symphony Orchestra) once remarked “[O]ur present hall [Boston Music Hall] gives a piano better than a forte, gives an elegant rather than a forcible return of the instruments—noble but weak—I want both”.
- Reid Concert Hall (1859): This Edinburgh concert hall, by Professor John Donaldson (1789–1865) and Scottish Architect David Cousin, was purposely designed to abide by the principle of harmonic ratios. Its main dimensions are 30 feet, 48 feet, and 90 feet (, , and ), a ratio of 5:8:15 [14].
- Wiener Musikverein (1870): This concert hall has proportions similar to its predecessor, the Redoutensaal [36]. From opening on, the Musikverein’s acoustics have been renowned.
- Neues Gewandhaus (1884–1944): The acoustic concept of the Neues Gewandhaus was based on the original Gewandhaus and the Palais du Trocadero [37]. Architects Martin Gropius and Heino Schmieden copied the dimensions from the existing Gewandhaus and scaled them up by approximately . One can assume that as the original Gewandhaus’ acoustics were well-liked, and the architects attempted to recreate its “sympathetic vibration” in the newly erected concert hall. The acoustics of the Neue Gewandhaus were well received by the German press [38]. The concert hall became an example of excellence in acoustics.
- Amsterdam Concertgebouw (1888): This concert hall has a length of 44 , width of 28 , and a height of 17 , close to a common multiple of 9 [2]. While not directly stated by its architect, one can assume that these dimensions were based on the previously described theory. The acoustics were initially part to mixed reviews, however a stage renovation in 1899 heralded its renowned acoustic reputation [39].
5. Current Practices in Room Acoustic Design
5.1. Audience Rake and Sightlines
5.2. Stage Acoustics
5.3. Length-Width-Height Ratios
...the most definite and often repeated statements are such as the following, that the dimensions of a room should be in the ratio 2:3:5, or according to some writers, 1:1:2, and others, 2:3:4; it is probable that the basis of these suggestions is the ratios of the harmonic intervals in music, but the connection is untraced and remote.
6. Discussion
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sabine, W. Collected Papers on Acoustics; Harvard University Press: Cambridge, UK, 1922; pp. 1–588. [Google Scholar]
- Beranek, L. How They Sound: Concert and Opera Halls; Acoustical Society of America: Melville, NY, USA, 1996; pp. 1–643. [Google Scholar]
- Barbieri, P. The acoustics of Italian opera houses and auditoriums (ca. 1450–1900). Recercare 1998, 10, 263–328. [Google Scholar]
- Barbieri, P. The state of architectural acoustics in the late renaissance. In Architettura e Musica Nella Venezia del Rinascimento; Howard, D., Moretti, L., Eds.; Bruno Mondadori: Milan, Italy, 2006; pp. 53–78. [Google Scholar]
- Morgan, M. The Ten Books on Architecture. Vitruvius, Translated by Josephus Gwilt; Harvard University Press: Cambridge, UK; Humphrey Milford: London, UK; Oxford University Press: Oxford, UK, 1914; pp. 1–413. [Google Scholar]
- Vassilantonopoulos, S.L.; Mourjopoulos, J. A Study of Ancient Greek and Roman Theater Acoustics. Acta Acust. United Acust. 2003, 89, 123–136. [Google Scholar]
- Postma, B.; Jouan, S.; Katz, B. Pre-Sabine room acoustic design guidelines based on human voice directivity. J. Acoust. Soc. Am. 2018, 143, 2428–2437. [Google Scholar] [CrossRef]
- Postma, B. A History of the Use of Time Intervals After the Direct Sound in Concert Hall Design Before the Reverberation Formula of Sabine Became Generally Accepted. Build. Acoust. 2013, 20, 157–176. [Google Scholar] [CrossRef]
- Postma, B.; Katz, B. A History of the Use of Reflections Arrival Time in Pre-Sabinian Concert Hall Design. In Proceedings of the Forum Acusticum, Krakow, Poland, 7–12 September 2014; pp. 1–6. [Google Scholar]
- Postma, B.; Dubouilh, S.; Katz, B.F.G. An archeoacoustic study of the history of the Palais du Trocadero (1878–1937). J. Acoust. Soc. Am. 2019, 145, 2810–2821. [Google Scholar] [CrossRef] [PubMed]
- Postma, B.; Katz, B.F.G. Pre-Sabine room acoustic assumptions on reverberation and their influence on room acoustic design. J. Acoust. Soc. Am. 2020, 147, 2478–2487. [Google Scholar] [CrossRef] [PubMed]
- D’Orazio, D.; Nannini, S. Towards Italian Opera Houses: A Review of Acoustic Design in Pre-Sabine Scholars. Acoustics 2019, 1, 252–280. [Google Scholar] [CrossRef]
- Cocci, A. Theatre design in ancient times: Science or opportunity? Acta Acust. United Acust. 2013, 99, 14–20. [Google Scholar] [CrossRef]
- Smith, T. A Rudimentary Treatise on the Acoustics of Public Buildings; John Weale: London, UK, 1861; pp. 1–163. [Google Scholar]
- Rhode, J. Theorie der Verbreitung des Schalles Für Baukünstler; English Title: Theory of the Propagation of Sound for Architects; Heinrich Frölich: Berlin, Germany, 1800; pp. 1–82. [Google Scholar]
- Russell, J. Elementary Considerations if some Principles in the Construction of Buildings designed to accommodate Spectators and Auditors. Edinb. New Philos. J. 1839, 27, 131–136. [Google Scholar]
- Lachèz, T. Acoustique et Optique des Salles de Réunion Publiques. Théâtres et Amphithéâtres, Spectacles, Concerts etc.; English Title: Acoustics and Optics of Public Meeting Rooms. Theaters and Amphitheatres, Performances, Concerts, etc.; Lemoine: Paris, France, 1848. [Google Scholar]
- Smith, T. On acoustics. Civ. Eng. Archit. J. 1861, 24, 46–53. [Google Scholar]
- Anonymous. Form of Lecture Rooms. J. Frankl. Inst. 1843, 5, 419. [Google Scholar]
- Smith, T.R. Architecture and Building: The Laws of Sound as Applied to the Arrangments of Buildings; Crosby Lockwood and Son: London, UK, 1895. [Google Scholar]
- Farrow, F. The Godwin Bursary: Report of a visit to Vienna and Budapest. Transactions 1885, 1, 55–64. [Google Scholar]
- Anonymous. Buffalo hot blast system of heating and ventilating, application to theaters. Illus. Gen. Cat. Buffalo Steel Plate Steam Pulley Fans 1892, 1, 84–85. [Google Scholar]
- Anonymous. New welsh presbyterian chapel, Ruthin. Br. Archit. J. Archit. Its Accessory Arts 1889, 32, 193. [Google Scholar]
- Patte, P. Essai sur L’architecture Théatrale; Chez Moutard: Paris, France, 1782; pp. 1–212. [Google Scholar]
- Saunders, G. Treatise on Theaters; I and J Taylor: London, UK, 1790; pp. 1–94. [Google Scholar]
- Langhans, C. Über Theater oder Bemerkungen über Katakustik; In English: About Theaters or Remarks about Catacoustics; Gottfried Hayn: Berlin, Germany, 1810; pp. 1–64. [Google Scholar]
- Chladni, E. Über vortheilhafte Einrichtung eines Locals für gute Wirkung des Schalles. English Title: About the Beneficial Setup of Rooms for the Good Effect of Sound. Allg. Musik. Ztg. 1826, 35, 565–570. [Google Scholar]
- Wetter, J. Untersuchungen über die Wichtigsten Gegenstände der Theaterbaukunst; In English: Studies about the most important theater construction subjects; Joseph Stenz: Mainz, Germany, 1829. [Google Scholar]
- Langhans, K. Vergleichung des Neuen Schauspielhauses zu Berlin mit Verschiedenen Ältern und Neuen Schauspielhuäsern in Rucksicht auf akustische und Optische Grundfäse; In English: Comparison of the New Theater in Berlin with Various Older and Newer Theaters Regarding Acoustics and Optics; Johann Friedrich Unger: Berlin, Germany, 1800; pp. 1–21. [Google Scholar]
- Runge, G. Das neue Opernhaus “Academy of music” in Philidelphia. English Title: The new opera house “Academy of music” in Philidelphia. Z. FÜr Bauwes. 1860, 10, 145–156. [Google Scholar]
- Langhans, C. Das Victoria Theater in Berlin. English Title: The Victoria-Theater in Berlin. Z. Bauwes. 1860, 10, 315–342. [Google Scholar]
- Pintore, A. Musical Symbolism in the Works of Leon Battista Alberti. Nexus Netw. J. 2004, 6, 49–70. [Google Scholar] [CrossRef][Green Version]
- Gardiner, W. The Music of Nature; J.H.Wilkins & R.B. Carter: Boston, MA, USA, 1841. [Google Scholar]
- Russell, J. The interior forms of buildings with reference to the laws of sounds. Builder 1847, 5, 82. [Google Scholar]
- Upham, J. A Consideration of Some of the Phenomena and Laws of Sound, Their Application in the Construction of Buildings, Designed Especially for musical Effects. Am. J. Sci. Art 1853, 65–66, 215–226. [Google Scholar]
- Barron, M. Auditorium Acoustics and Architectural Design; Taylor & Francis: London, UK, 1993; pp. 1–504. [Google Scholar]
- Schmieden, H. Das neue Gewandhaus in Leipzig. In English: The new Gewandhaus in Leipzig; In Zeitschrift Fur Bauwesen; Gewandhaus: Leipzig, Germany, 1886; pp. 1–14. [Google Scholar]
- Skoda, R. Neues Gewandhaus Leipzig. Baugeschichte und Gegenwart Eines Konzertgebäudes; In English: New Leipzig Gewandhaus. Building’s History and Present; Verlag Für Bauwesen: Berlin, Germany, 1985. [Google Scholar]
- van Royen, H. Historie en Kroniek van het Concertgebouw en het Concertgebouworkest 1888–1988. Dl. 1. Voorgeschiedenis 1888–1945; English Title: History and Chronicle of the Concertgebouw and the Concertgebouw Orchestra 1888–1988. part 1. History 1888–1945; Walburg pers: Zutphen, The Netherlands, 1989. [Google Scholar]
- JGJ 57-2016. Code for Design of Theater Building. In Code of China; China Construction Industry Press: Beijjing, China, 2016. [Google Scholar]
- Gade, A. Acoustics of the Orchestra Platform from the Musicians’ Point of View. In Proceedings of Acoustics for Choir and Orchestra, Royal Swedish Academy of Music No. 52; Royal Swedish Academy of Music: Stockholm, Sweden, 1986. [Google Scholar]
- Rindel, J. Design of new ceiling reflectors for improved ensemble in a concert hall. Appl. Acoust. 1991, 34, 7–17. [Google Scholar] [CrossRef]
- Green, E.; Essert, R. On-Stage Hearing: Experience from Orchestra Hall, Minneapolis; Institute of Acoustics: Milton Keynes, UK, 2015; Volume 37. [Google Scholar]
- Taylor, L.; Claringbold, D. Acoustics of the Sydney Opera House concert hall. In Proceedings of the International Congress on Acoustics, Sydney, NSW, Australia, 23–27 August 2010; Volume 20, pp. 1–8. [Google Scholar]
- Jurkiewicz, Y.; Kahle, E.; Katz, B.F. Stavanger Concert Hall, acoustic design and measurement results. In Proceedings of the Auditorium Acoustics, Paris, France, 29–31 October 2015; Volume 37, pp. 300–307. [Google Scholar]
- Miller, G.; Giegold, C.; Pfeiffer, S.; Schuette, D.; Brill, L. Orchestral Preferences for Discrete Overhead and Side Wall On-Stage Reflections. In Proceedings of the International Symposium on Room Acoustics, Amsterdam, The Netherlands, 15–17 September 2019. [Google Scholar]
- Meyer, J. Acoustics and the Performance of Music; Springer: New York, NY, USA, 2009. [Google Scholar]
- Beranek, L.; Hidaka, T.; Masuda, S. Acoustical design of the opera house of the New National Theatre, Tokyo, Japan. J. Acoust. Soc. Am. 2000, 107, 355–367. [Google Scholar] [CrossRef] [PubMed]
- Thiele, R. Richtungsverteilung und Zeitfolge der Schallruckwurfe in Räumen. English title: Directional distribution and sequence of the sound reflections in rooms. Acustica 1953, 3, 291–302. [Google Scholar]
- Reichardt, W.; Abdel Alim, O.; Schmidt, W. Definition und Messgrunde eines objektiven Masses zur Ermittlung der Grenze zwischen brauchbarer und unbrauchbarer Durchsichtigkeit bei Musikarbietung. English title: Definition and Basis of Making an Objective Evaluation to Distinguish Between Useful and Useless Clarity Defining Musical Performances. Acustica 1975, 32, 126–137. [Google Scholar]
- Louden, M.M. Dimension Ratios of Rectangular Rooms with Good Distribution of Eigentones. Acustica 1971, 24, 101–104. [Google Scholar]
- Marshall, A. A Note on the Importance of Room Cross-Section in Concert Halls. J. Sound Vib. 1967, 5, 100–112. [Google Scholar] [CrossRef]
- Arau-Puchades, H. An Improved Reverberation Time Formula. Acustica 1988, 65, 163–180. [Google Scholar]
- Luizard, P.; Katz, B.F.; Guastavino, C. Perceptual thresholds for realistic double-slope decay reverberation in large coupled spaces. J. Acoust. Soc. Am. 2015, 137, 75–84. [Google Scholar] [CrossRef]
- Lokki, T.; Pätynen, J. Architectural Features That Make Music Bloom in Concert Halls. Acoustics 2019, 1, 439–449. [Google Scholar] [CrossRef]
- Lokki, T.; McLeod, L.; Kuusinen, A. Perception of loudness and envelopment for different orchestral dynamics. J. Acoust. Soc. Am. 2020, 148, 2137–2145. [Google Scholar] [CrossRef]
- Katz, B.F.G. In-situ calibration of the sound strength parameter G. J. Acoust. Soc. Am. 2015, 138, EL167–EL173. [Google Scholar] [CrossRef] [PubMed]
| Room | Stage Opening | |
|---|---|---|
| In Rheinl. Ft. | In Meters | |
| The opera in Bologna | 47 | 14.7 |
| The opera in Milan | 46 | 14.4 |
| The opera in Napels | 45.5 | 14.3 |
| The opera in Paris | 42 | 13.2 |
| The opera in Berlin | 40 | 12.6 |
| The theater in Vienna | 45 | 14.1 |
| The national theater in Berlin | 40 | 12.6 |
| The new theater in St. Petersburg | 44 | 13.8 |
| The theater in Karlsruhe | 42 | 13.2 |
| Room | Voice Directivity | “Echo Theory” | Reverberation | Audience Rake | Stage Opening Width | Length Width Height Ratio |
|---|---|---|---|---|---|---|
| Sheldonian Theater, Oxford (1669) | x | - | - | - | - | - |
| Lecture hall College of Physicians, London (1675) | x | - | - | - | - | - |
| Theater of the Royal institution, London (1802) | x | - | x | x | - | x |
| Iffland Theater, Berlin (1802) | x | x | x | - | x | - |
| Hoftheater, Karlsruhe (1809) | x | x | - | - | - | - |
| Drury lane theater, London (1813) | x | - | x | - | - | - |
| House of Commons (renovation), London (1834) | - | - | x | - | - | - |
| Crystal Palace concert hall, London (1851) | - | - | - | x | - | - |
| Boston Music Hall, Boston (1852) | - | - | x | - | - | x |
| Handel Orchestra in the Crystal palace, London (1854) | - | - | x | - | - | x |
| Lecture room in the Smithsonian, New York (1856) | x | x | x | - | - | - |
| Academy of music, New York (1854) | x | - | - | - | - | - |
| Academy of music, Philadelphia (1857) | - | x | x | - | x | - |
| Victoria Theater, Berlin (1859–1891) | - | - | - | - | x | - |
| Reid Concert Hall (1859) | - | - | - | - | - | x |
| Théâtre du Châtelet, Paris (1862) | - | - | x | - | - | - |
| Théâtre-Lyrique, Paris (1862) | - | - | x | - | - | - |
| Fourteenth Street Theater, New York (1866) | x | - | - | - | - | - |
| Wiener Musikverein, Vienna (1870) | - | - | - | - | - | x |
| Royal Albert Hall, London (1871) | x | - | x | - | - | - |
| Sanders Theater, Boston (1876) | x | - | - | - | - | - |
| Palais du Trocadero, Paris (1878–1937) | - | x | - | - | - | - |
| Neue Gewandhaus, Leipzig (1884–1944) | - | x | x | - | - | x |
| Concertgebouw, Amsterdam (1888) | - | x | x | - | - | x |
| The Chicago Auditorium, Chicago (1889) | - | - | - | x | - | - |
| New Welsh presbyterian chapel, Ruthin, North Wales (1889) | - | - | - | x | - | - |
| Salle Pleyel, Paris (1928) | - | x | - | - | - | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Postma, B.N.J.; Green, E.; Kahle, E.; Katz, B.F.G. Pre-Sabine Room Acoustic Guidelines on Audience Rake, Stage Acoustics, and Dimension Ratios. Acoustics 2021, 3, 235-251. https://doi.org/10.3390/acoustics3020017
Postma BNJ, Green E, Kahle E, Katz BFG. Pre-Sabine Room Acoustic Guidelines on Audience Rake, Stage Acoustics, and Dimension Ratios. Acoustics. 2021; 3(2):235-251. https://doi.org/10.3390/acoustics3020017
Chicago/Turabian StylePostma, Barteld N. J., Evan Green, Eckhard Kahle, and Brian F. G. Katz. 2021. "Pre-Sabine Room Acoustic Guidelines on Audience Rake, Stage Acoustics, and Dimension Ratios" Acoustics 3, no. 2: 235-251. https://doi.org/10.3390/acoustics3020017
APA StylePostma, B. N. J., Green, E., Kahle, E., & Katz, B. F. G. (2021). Pre-Sabine Room Acoustic Guidelines on Audience Rake, Stage Acoustics, and Dimension Ratios. Acoustics, 3(2), 235-251. https://doi.org/10.3390/acoustics3020017







