Atomistic Investigation of Material Deformation Behavior of Polystyrene in Nanoimprint Lithography
Abstract
1. Introduction
2. Materials and Methods
2.1. Polymer Formation
2.2. Conversion to LAMMPS Readable Format
2.3. Importance of Glass Transition Temperature
2.4. Molecular Dynamics Simulation
3. Results
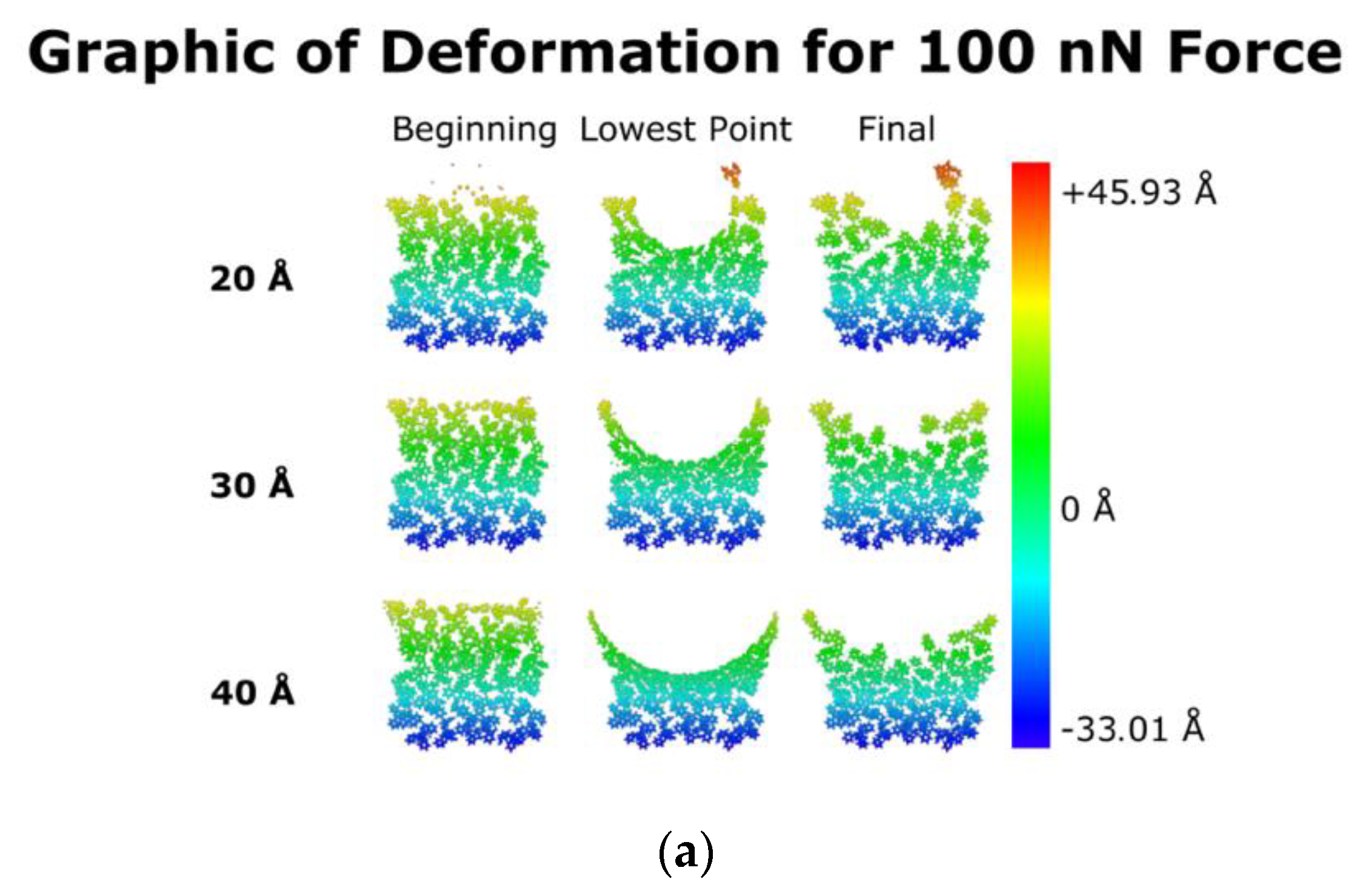
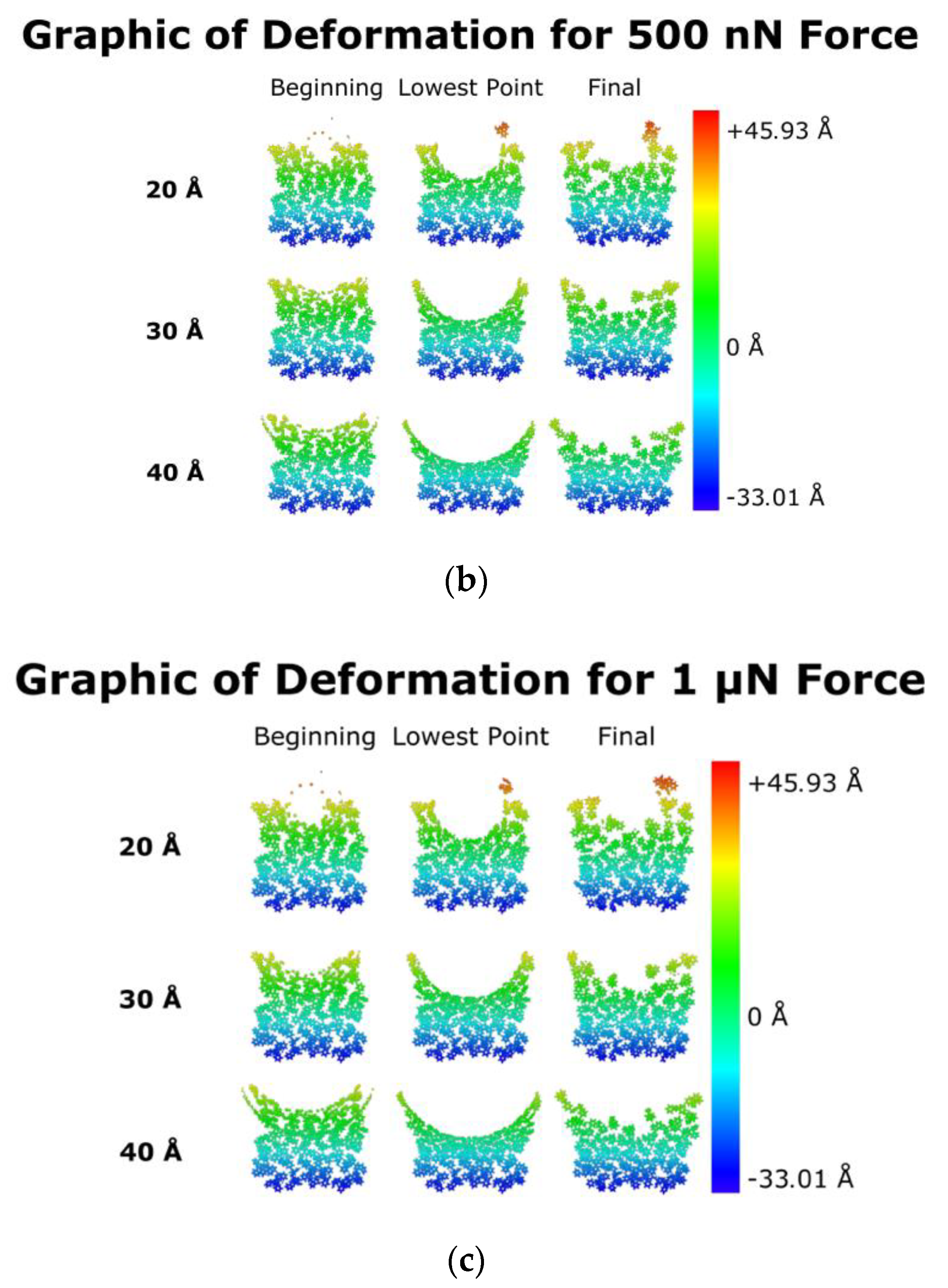
3.1. Effect of Indenter Size and Applied Force on Indentation Depth
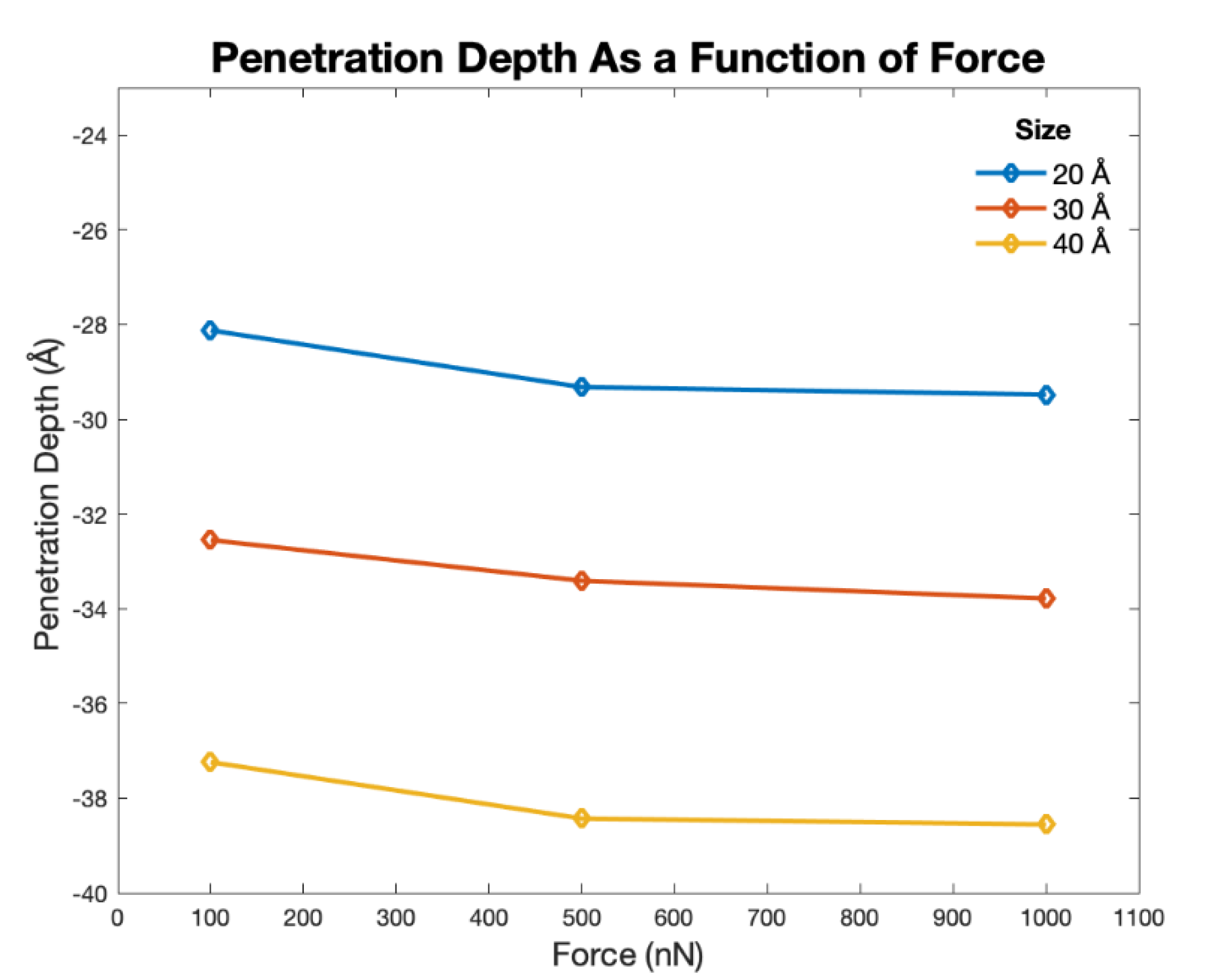
3.2. Effect on Penetration Depth
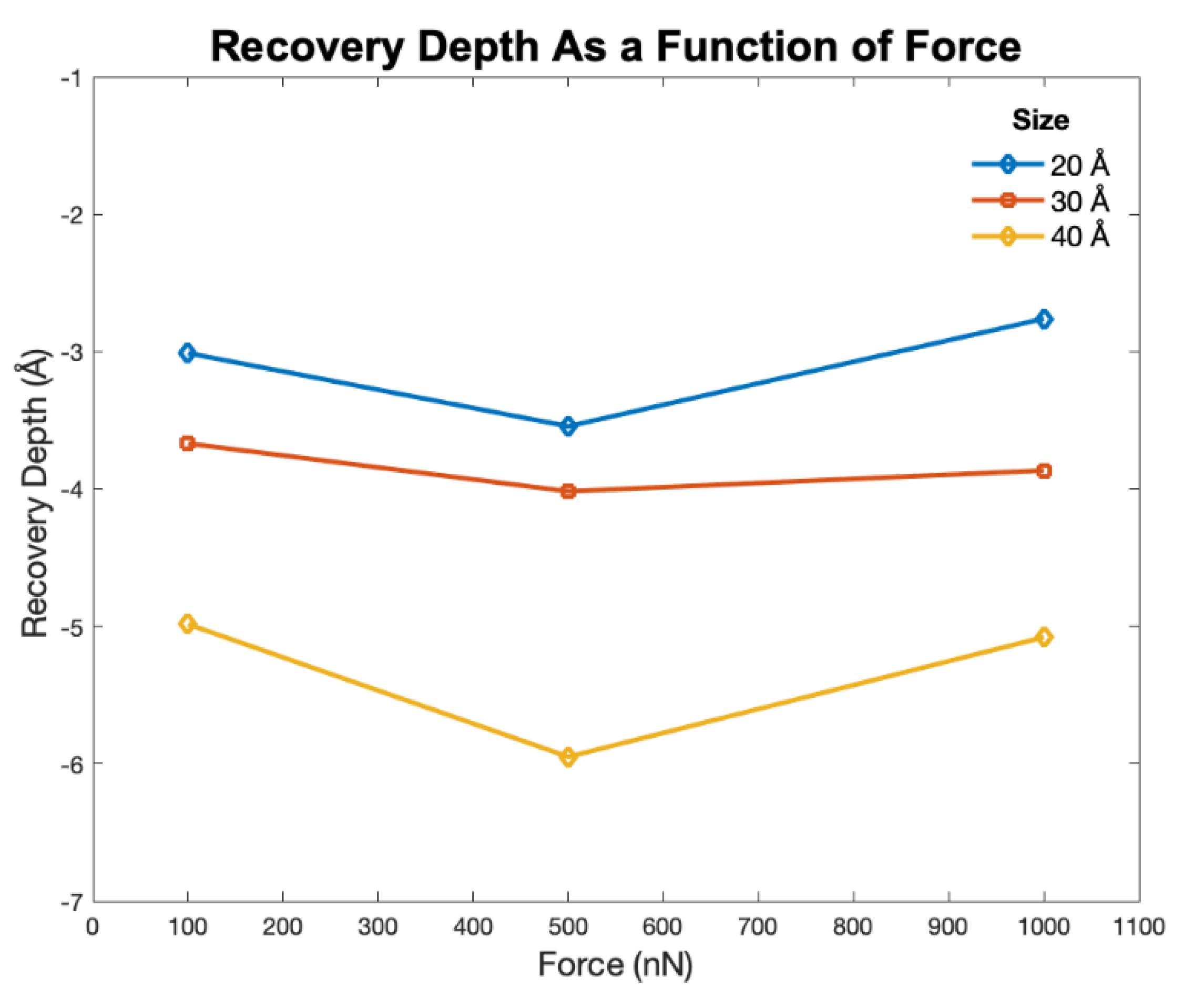
3.3. Recovery Behavior
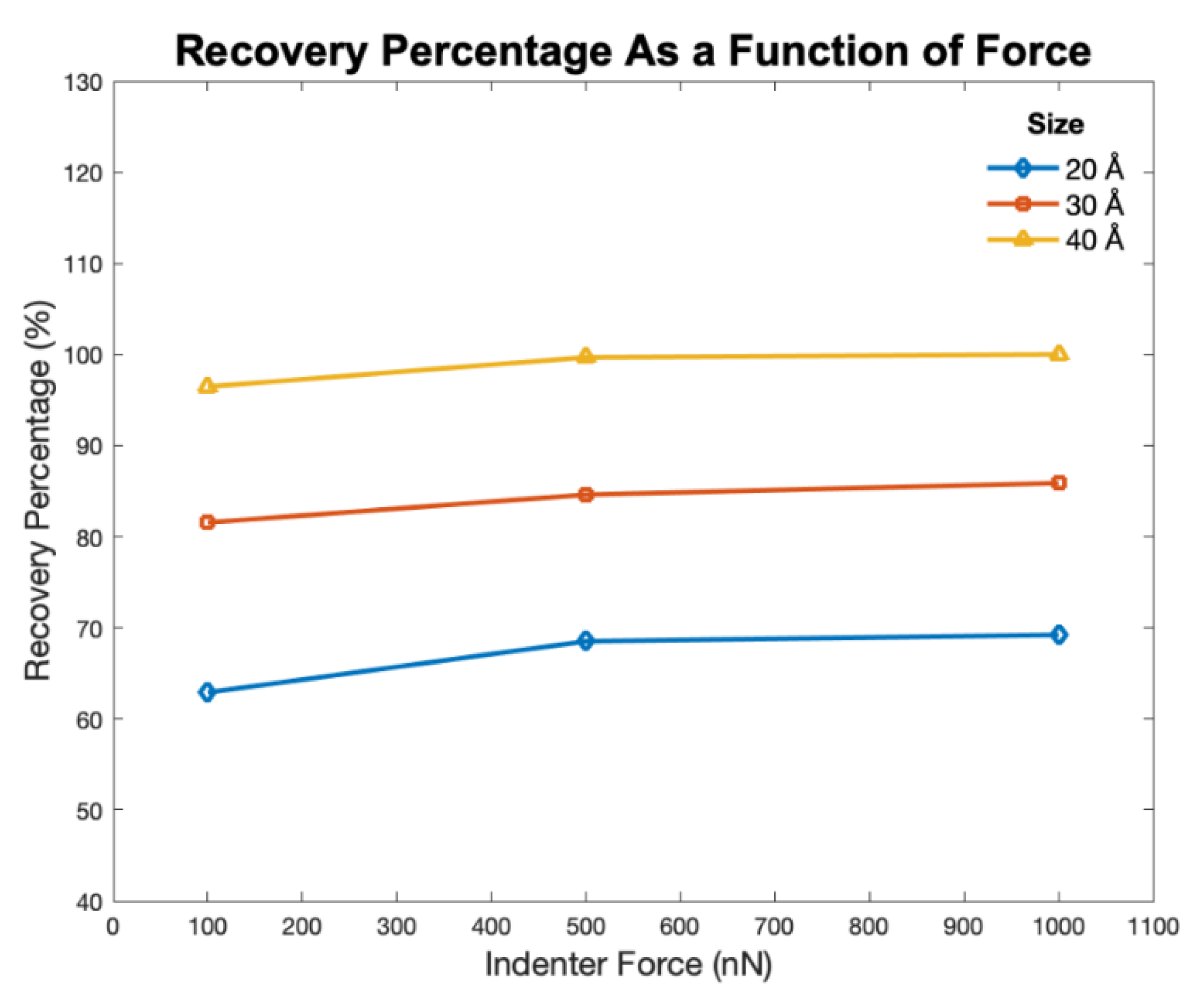
3.4. Recovery Percentage
3.5. Effect of Indenter Size and Indentation Hold Time
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Chou, S.Y.; Krauss, P.R.; Renstrom, P.J. Imprint of sub-25 nm vias and trenches in polymers. Appl. Phys. Lett. 1995, 67, 3114–3116. [Google Scholar] [CrossRef]
- Chou, S.Y.; Krauss, P.R.; Renstrom, P.J. Nanoimprint lithography. J. Vac. Sci. Technol. B Microelectron. Nanom. Struct. Process. Meas. Phenom. 1996, 14, 4129–4133. [Google Scholar] [CrossRef]
- Chou, S.Y.; Krauss, P.R.; Zhang, W.; Guo, L.; Zhuang, L. Sub-10 nm imprint lithography and applications. J. Vac. Sci. Technol. B Microelectron. Nanom. Struct. Process. Meas. Phenom. 1997, 15, 2897–2904. [Google Scholar] [CrossRef]
- Akter, T.; Desai, S. Developing a predictive model for nanoimprint lithography using artificial neural networks. Mater. Des. 2018, 160, 836–848. [Google Scholar] [CrossRef]
- Crawley, D.; Nikolic, K.; Forshaw, M. 3D Nanoelectronic Computer Architecture and Implementation (Series in Materials Science and Engineering); CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Gaikwad, A.; Clarke, J.; Desai, S. Atomistic Investigation of Process Parameter Variations on Material Deformation Behavior in Nanoimprint Lithography of Gold. Precis. Eng. 2020, 64, 7–19. [Google Scholar] [CrossRef]
- Kwon, B.; Kim, J.H. Importance of molds for nanoimprint lithography: Hard, soft, and hybrid molds. J. Nanosci. 2016, 2016, 1–12. [Google Scholar] [CrossRef]
- Shao, J.; Chen, X.; Li, X.; Tian, H.; Wang, C.; Lu, B. Nanoimprint lithography for the manufacturing of flexible electronics. Sci. China Technol. Sci. 2019, 62, 175–198. [Google Scholar] [CrossRef]
- Lyon, G.B.; Cox, L.M.; Goodrich, J.T.; Baranek, A.D.; Ding, Y.; Bowman, C.N. Remoldable thiol–ene vitrimers for photopatterning and nanoimprint lithography. Macromolecules 2016, 49, 8905–8913. [Google Scholar] [CrossRef]
- Odujole, J.I.; Desai, S. Molecular dynamics investigation of material deformation behavior of PMMA in nanoimprint lithography. AIP Adv. 2020, 10, 95102. [Google Scholar] [CrossRef]
- Koyama, M.; Shirai, M.; Kawata, H.; Hirai, Y.; Yasuda, M. Computational study on UV curing characteristics in nanoimprint lithography: Stochastic simulation. Jpn. J. Appl. Phys. 2017, 56, 06GL03. [Google Scholar] [CrossRef]
- Lee, H. Effect of imprinting pressure on residual layer thickness in ultraviolet nanoimprint lithography. J. Vac. Sci. Technol. B Microelectron. Nanom. Struct. Process. Meas. Phenom. 2005, 23, 1102–1106. [Google Scholar] [CrossRef]
- Jain, A.; Spann, A.; Bonnecaze, R.T. Effect of droplet size, droplet placement, and gas dissolution on throughput and defect rate in UV nanoimprint lithography. J. Vac. Sci. Technol. B Nanotechnol. Microelectron. Mater. Process. Meas. Phenom. 2017, 35, 11602. [Google Scholar] [CrossRef]
- Hirai, Y. Basic Mechanisms of Nanoimprint Lithography. Nanoimprinting Appl. 2019, 11, 83. [Google Scholar]
- Rowland, H.D.; Sun, A.C.; Schunk, P.R.; King, W.P. Impact of polymer film thickness and cavity size on polymer flow during embossing: Toward process design rules for nanoimprint lithography. J. Micromech. Microeng. 2005, 15, 2414. [Google Scholar] [CrossRef]
- Gaikwad, A.; Clarke, J. Molecular dynamics study of the quenching effect on direct nanoimprint of gold. In Proceedings of the 2019 IISE Annual Conference, Orlando, FL, USA, 18–21 May 2019. [Google Scholar]
- Odujole, J.; Desai, S. Molecular Dynamics Simulation of Poly Acrylic Acid as a Resist Material for Thermal Nanoimprint Lithography Processes. In Proceedings of the Industrial Engineers Research Conference 2020, New Orleans, LA, USA, 1 October 2020. [Google Scholar]
- Rodrigues, J.; Desai, S. The nanoscale Leidenfrost effect. Nanoscale 2019, 11, 12139–12151. [Google Scholar] [CrossRef] [PubMed]
- Marquetti, I.; Desai, S. Orientation effects on the nanoscale adsorption behavior of bone morphogenetic protein-2 on hydrophilic silicon dioxide. RSC Adv. 2019, 9, 906–916. [Google Scholar] [CrossRef]
- Gaikwad, A.; Desai, S. Understanding material deformation in nanoimprint of gold using molecular dynamics simulations. Am. J. Eng. Appl. Sci. 2018, 11, 837–844. [Google Scholar] [CrossRef][Green Version]
- Yuan, Y.; Sun, T.; Zhang, J.J.; Liu, C. Molecular Dynamics Study of Nanoimprint of Single Crystal Aluminium Thin Film. In Proceedings of the 2015 International Conference on Artificial Intelligence and Industrial Engineering, Phuket, Thailand, 26–27 July 2015. [Google Scholar]
- Lyulin, A.V.; Balabaev, N.K.; Mazo, M.A.; Michels, M.A.J. Molecular dynamics simulation of uniaxial deformation of glassy amorphous atactic polystyrene. Macromolecules 2004, 37, 8785–8793. [Google Scholar] [CrossRef]
- Lyulin, A.V.; Michels, M.A.J. Molecular dynamics simulation of bulk atactic polystyrene in the vicinity of T g. Macromolecules 2002, 35, 1463–1472. [Google Scholar] [CrossRef]
- Lyulin, A.V.; Balabaev, N.K.; Michels, M.A.J. Correlated segmental dynamics in amorphous atactic polystyrene: A molecular dynamics simulation study. Macromolecules 2002, 35, 9595–9604. [Google Scholar] [CrossRef]
- Eslami, H.; Müller-Plathe, F. Molecular dynamics simulation of sorption of gases in polystyrene. Macromolecules 2007, 40, 6413–6421. [Google Scholar] [CrossRef]
- Liu, W.; Yang, C.-L.; Zhu, Y.-T.; Wang, M. Interactions between single-walled carbon nanotubes and polyethylene/polypropylene/polystyrene/poly (phenylacetylene)/poly (p-phenylenevinylene) considering repeat unit arrangements and conformations: A molecular dynamics simulation study. J. Phys. Chem. C 2008, 112, 1803–1811. [Google Scholar] [CrossRef]
- Ayyagari, C.; Bedrov, D.; Smith, G.D. Structure of atactic polystyrene: A molecular dynamics simulation study. Macromolecules 2000, 33, 6194–6199. [Google Scholar] [CrossRef]
- Yang, F.; Ghosh, S.; Lee, L.J. Molecular dynamics simulation based size and rate dependent constitutive model of polystyrene thin films. Comput. Mech. 2012, 50, 169–184. [Google Scholar] [CrossRef]
- Pfaller, S.; Possart, G.; Steinmann, P.; Rahimi, M.; Müller-Plathe, F.; Böhm, M.C. Investigation of interphase effects in silica-polystyrene nanocomposites based on a hybrid molecular-dynamics–finite-element simulation framework. Phys. Rev. E 2016, 93, 52505. [Google Scholar] [CrossRef]
- Zharik, G.A.; Dagesyan, S.A.; Soldatov, E.S.; Presnov, D.E.; Krupenin, V.A. Nanometer Scale Lithography with Evaporated Polystyrene. Mosc. Univ. Phys. Bull. 2017, 72, 627–632. [Google Scholar] [CrossRef]
- Ma, S.; Con, C.; Yavuz, M.; Cui, B. Polystyrene negative resist for high-resolution electron beam lithography. Nanoscale Res. Lett. 2011, 6, 446. [Google Scholar] [CrossRef]
- Brazel, C.S.; Rosen, S.L. Fundamental Principles of Polymeric Materials; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Biovia, D.S. Materials Studio; Version 6.0; Dassault Systems: San Diego, CA, USA, 2012. [Google Scholar]
- Kang, J.-H.; Kim, K.-S.; Kim, K.-W. Molecular dynamics study on the effects of stamp shape, adhesive energy, and temperature on the nanoimprint lithography process. Appl. Surf. Sci. 2010, 257, 1562–1572. [Google Scholar] [CrossRef]
- Marquetti, I.; Rodrigues, J.; Desai, S.S. Ecological Impact of Green Computing Using Graphical Processing Units in Molecular Dynamics Simulations. Int. J. Green Comput. 2018, 9, 35–48. [Google Scholar] [CrossRef]
- Kwon, S.; Lee, Y.; Park, J.; Im, S. Molecular simulation study on adhesions and deformations for Polymethyl Methacrylate (PMMA) resist in nanoimprint lithography. J. Mech. Sci. Technol. 2011, 25, 2311. [Google Scholar] [CrossRef]
- Chang, K.-H. e-Design: Computer-Aided Engineering Design; Academic Press: Cambridge, MA, USA, 2016. [Google Scholar]
- Chang, Y.; Chang, S.-W. Full-atomistic simulations of poly (ϵ-caprolactone) diol models with CVFF and CGenFF. Multiscale Multiphys. Mech. 2016, 1, 327–340. [Google Scholar] [CrossRef]
- Awasthi, A.P.; Lagoudas, D.C.; Hammerand, D.C. Modeling of graphene–polymer interfacial mechanical behavior using molecular dynamics. Model. Simul. Mater. Sci. Eng. 2008, 17, 15002. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Rocha, J.R.; Yang, K.Z.; Hilbig, T.; Brostow, W.; Simoes, R. Polymer indentation with mesoscopic molecular dynamics. J. Mater. Res 2013, 28, 21. [Google Scholar] [CrossRef][Green Version]
- Xia, W.; Lan, T. Interfacial Dynamics Governs the Mechanical Properties of Glassy Polymer Thin Films. Macromolecules 2019, 52, 6547–6554. [Google Scholar] [CrossRef]
- Brostowl, W.; Simões, R. Tribological and mechanical behavior of metals and polymers simulated by molecular dynamics. J. Mater. Educ. 2005, 27, 19. [Google Scholar]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2009, 18, 15012. [Google Scholar] [CrossRef]
- Matlab, R; The MathWorks, Inc.: Natick, MA, USA, 2019.





| Masses | |||
|---|---|---|---|
| Atom | Atomic mass (amu) | ||
| C | 12.01115 | ||
| H | 1.00797 | ||
| Bond parameters | |||
| Bond type | r0 (Å) | KB (Kcal mole−1) | |
| H-C | 1.105 | 340.6175 | |
| C-C | 1.34 | 480.00 | |
| Angle parameters | |||
| Angle type | θ0 (degrees) | KA (Kcal mole−1) | |
| H-C-H | 106.4 | 39.5 | |
| H-C-C | 110.0 | 44.4 | |
| C-C-C | 110.5 | 46.6 | |
| Torsion parameters | |||
| Angle type | ϕ0 (degrees) | n | KA (Kcal mole−1) |
| H-C-C-C | 0 | 3 | 0.1581 |
| Van der Waals parameters | |||
| Atom type | A | B | |
| C | 0.389999952 | 3.875409636 | |
| H | 0.38000011 | 2.4499714540 | |
| Cut-off distance = 9.5 Å |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Odujole, J.; Desai, S. Atomistic Investigation of Material Deformation Behavior of Polystyrene in Nanoimprint Lithography. Surfaces 2020, 3, 649-663. https://doi.org/10.3390/surfaces3040043
Odujole J, Desai S. Atomistic Investigation of Material Deformation Behavior of Polystyrene in Nanoimprint Lithography. Surfaces. 2020; 3(4):649-663. https://doi.org/10.3390/surfaces3040043
Chicago/Turabian StyleOdujole, Jahlani, and Salil Desai. 2020. "Atomistic Investigation of Material Deformation Behavior of Polystyrene in Nanoimprint Lithography" Surfaces 3, no. 4: 649-663. https://doi.org/10.3390/surfaces3040043
APA StyleOdujole, J., & Desai, S. (2020). Atomistic Investigation of Material Deformation Behavior of Polystyrene in Nanoimprint Lithography. Surfaces, 3(4), 649-663. https://doi.org/10.3390/surfaces3040043






