1. Introduction
Recently, a certain number of papers, books and scientific blogs have given a vision of mathematics in the promotion of cultural heritage (see Reference [
1]). The connection between mathematics and art as well as mathematics and nature is appreciated from the tourist point of view. We suggest an itinerary in Matera (Italy) to see its peculiar forms from a mathematical point of view. This attempt also leads to research results. See References [
2,
3,
4] as refereed papers and References [
5,
6] as popularizing versions of these ideas. In particular, in Reference [
3] we gave a qualitative description of Matera by means of fractals sets; in Reference [
4] we carried out quantitative computation to support the thesis that Matera has fractal geometry. In Reference [
2], a comparison between the shape of Matera and Petra is illustrated. It is possible to compare these works with the definition of fractal city given in References [
7,
8], concluding that Matera is another example in which the application of fractal tools to urbanistic is possible and fruitful.
In this paper, we present another point of view: We try to explain a mathematical concept by seeing a city that contains its representation. The mathematical concept is the dimension and the city is still Matera. In
Section 2, we describe some places in this city where the guest can see Euclidean suggestions. In
Section 3, we briefly go back to the observations of References [
3,
4,
6] by fractal analysis and include these in the information that a touristic guide can give. In
Section 4, we suggest an application. In
Section 5, we present a combinatoric puzzle decorated with some pictures of Matera. This enables us to create a story explaining the concepts given in
Section 2 and
Section 3.
2. Going for a Walk in Euclidean Dimensions
In Matera, different stories of dimensions can be suggested starting from different points of observation. For example, the Museum of Contemporary Sculpture in Matera, called MUSMA, is the ideal point to observe the
fourth dimension. Some interesting installations can be seen by mathematicians as three-dimensional projections of four-dimensional objects. On the other hand, speaking to a curious tourist who likes scientific language, the guides must point out that this museum exhibits space–time. Not just space. Nor only time. Indeed, though Palazzo Pomarici was built in the 16th Century, today it houses a collection of modern works which concern the future. The proximity of the Sassi zone, with houses dug into limestone rock hundreds of years ago and contemporary sculpture, tell us about the ancient world. The stone itself gives this space–time shock, and one piece of evidence is Alberto Viani’s sculpture, see
Figure 1, and the way in which it collects rays of light and shadows as a physical universe. Indeed, it was inspired by a collection of mathematical surfaces used by scientists at the beginning of the 20th Century when a new cosmological vision appeared. See Reference [
9]. From our point of view, MUSMA is the place in Matera where space and time are intertwined.
Almost all installations in MUSMA are sculptures. Observing them in detail, we go down from the four dimensions to
three dimensions. Sculpure mainly plays with depth. We can see this, for example, in the facial expressions of the small
Birichino by Menardo Rosso (see
Figure 2). Without depth, we cannot see the core of the work. The same artist said that “Nothing is material in space, we are nothing but plays of light”. From a mathematical point of view, this statement refers to the theories on light paths (therefore shorter ones) in curved space. In this museum, there are also installations that realize the games of light deviated from more or less curved obstacles.
In MUSMA, the light flows on marble, bronze, iron, steel, terracotta, plaster, ceramic, papier-mâché, tuff, and wood, and it reveals by drawing the shadow. On the contrary, in many cases,
two dimensions are sufficient for an image.
Lucania 61, a large mural by Carlo Levi in Palazzo Lanfranchi shows the inhabitants of Basilicata in 1961. In this mural, the artist neglects depth, leaving to the viewer the task of finding it. See a detail in
Figure 3.
Let’s move to “Casa di Ortega”. The Spanish painter Josè Ortega tried to bring out the scream of pain in papier mache (see
Figure 4).
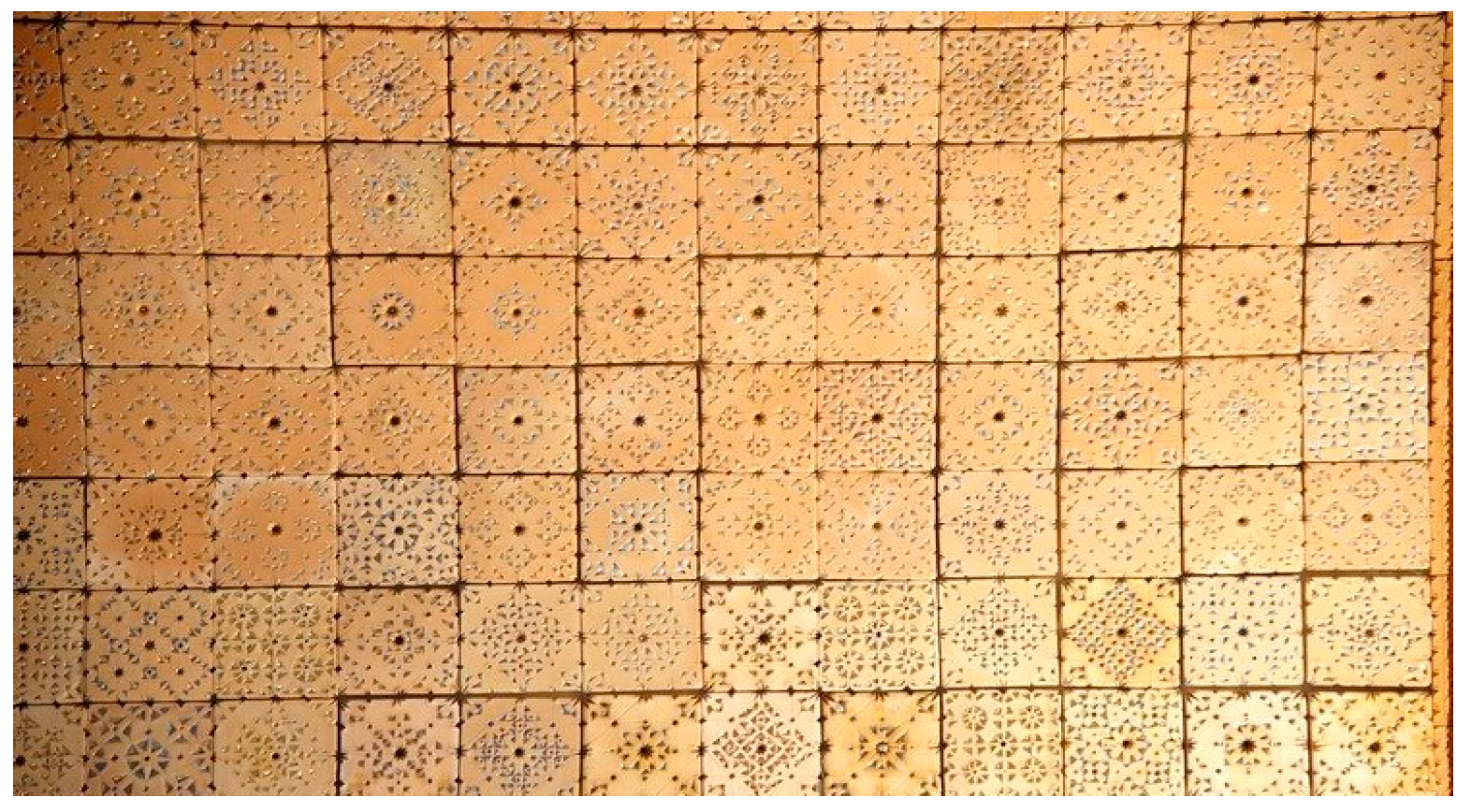
This technique allows the viewer to remain in the middle between the two dimensions of an image and the three dimensions of sculpture. On the contrary, the artisans who live in Matera filled the ceiling of Ortega’s house with a ceramic sky (see
Figure 5). For a mathematician, the crosses on these tiles suggest the tessellations of a surface but also the only two coordinates that are sufficient to find a point on a surface.
To reach Ortega’s house and MUSMA we pass stairs and slopes. The city of Matera is perched on a hill, it has an elevation of 401 m above sea level. In order to bring water into all houses, an underground city was needed. In Reference [
10], Pietro Laureano wrote that this city has an inverted pyramid, a sort of mirror of the tetrahedron of the Civita, the higher part of the city (see
Figure 6).
Matera and its water system appear as a
complex multi-dimensional world. As in the Gauss-2D complex space, we have four multi-quadrants. The origin of this complex system is in Piazza Vittorio Veneto where we can cross the horizontal and real plane of the city level. In addition, we can also think of an imaginary vertical plane between the populated city and the natural landscape (see
Figure 7). The canyon, here called “gravina”, is a thin line between rocks without humans and populated rocks. In all the other parts of the vision, human building and natural scenery are intertwined.
A tourist guide has to underline the ingenuity of those who used the empty spaces of nature to protect themselves from the cold and built empty spaces in the rock to save themselves from thirst. Mathematics was as always unconsciously allied with urban planning choices.
Going back to Piazza Vittorio Veneto, we can go down into Palombaro Lungo, the giant ancient cistern, (see
Figure 8). It is natural to ask about the aqueduct, observing that a water network and the way in which length, amplitude and number of conduits are settled. From the mathematical point of view, this is a graph: A set of points (called vertex) and lines (called arches) that eventually connect the point. In the Matera aqueduct, all vertices are connected and all arches are weighted by parameters that enable the water to be wisely dosed.
There is also an abstract graph in the city: The underground connections of the canals become the connections of the houses made by clothes lines or simply by citizens gazing at each other. The one dimensional concept is always present in Matera here, since an apparently chaotic city increases relations. A convex set is a set in which any two points are connected by a line which remains in the set. It is important to notice that any square is convex since it is the place of relations. In the Sassi zone, the houses were distributed in small groups of almost 10 which had a courtyard in common in which the life of everyone was controlled. This yard has a local name of “vicinato”. Like a theatre, it is convex. Any convex set is a connected set. This is a very important mathematical inclusion that is translated in any human agorà!
There are sets without connections and their dimension is less than one. For example, a point has zero dimension. However, very complicated sets of “separated” points are also zero-dimensional: The set of lights in Matera nights is one of these.
What is the best point to see the geometry of Matera? Everywhere around the Sassi zone and in every different point we can see different shapes.
Can a good definition of dimension become a tale for a tourist? We believe so. The guide is in dialogue with those who follow him. For example, after observing the sculptures, we show a cube and ask how we can break it into two equal parts. The visitor will tell us to do it through a plane. We will point out that we have divided a three dimensional object by a two dimensional object. Then, we show a sheet and ask how it can be broken into two equal parts. The visitor will tell us it can be done using a line. We will point out that we have divided a two dimensional object with an object of one dimension. Now, let us show a thread. It will be the same for a visitor to understand how a point, zero dimensional, is enough to divide an object of dimension one. In this descent, the visitor may wonder if the point can be divided. Mathematicians define
negative dimension as that of the empty set. It would be nice to give to the tourist this explanation on the balcony of Ortega’s house that overlooks two vacuums: the canyon, the void dug by water and the stone gardens, the void dug by the wind. See Reference [
11].
Then, one can come back from negative to zero, one, two, three, four dimensions and ask about higher ones. This is difficult to understand and we can only sketch that the higher dimension is related to groups of symmetry. The wheel of of the Cathedral of the city is a rare element of symmetry. Palazzo del Sedile, on the contrary, presents a symmetry broken by the absence of a clock (see
Figure 9). A good narrator can also explain the
infinite dimension by opening the windows of Ortegas’s house and mentioning that spaces with infinite coordinates are the right ones to study the motion of the flight of the nibbio.
3. Taking a Photo of a Fractal City
Fractional dimensions appeared in mathematics during the 20th Century. It was necessary to understand the measure of irregular surfaces or the length of irregular curves. Irregular means full of tips and cusps. For a surface and the volume in which its contained, not being regular means being full of voids. It is important in various contexts to understand the degree of regularity of a surface and if it is increasing or not during its evolution. The most irregular things that can be seen in Matera are the canyon and the rocks above it. Water and wind continuously act there. In Reference [
3], by means of a graphics program, we realized a fractal on an aerial picture of the canyon. We get a sequence of Julia sets, very complicated and unstable fractals, obtaining a figure that seems to runs along the gravina like a river. The comprehension of the rule that generates it and the computation of its fractal dimension is not easy, indeed it changes from point to point.
It is better to present to the visitor with something simpler, though chaotic. The inhabitants of Matera, from ancient times, have also acted by continuously excavating the rock. The Sassi zone is itself irregular, but “self-similar”.
Imagine many people in Matera each taking one photo of the Sassi zone from different points, for example our
Figure 6. Each photo would be different. Imagine putting these photos together and asking someone extraneous to this game to extract one of them. He or she would guess that the selected photo has been taken in the city of Matera. This possibility to go from local vision to the global one is only given in particular contexts. The same game would not be possible in Milan for example, since only images of particular monuments are characteristic of the city.
In order to explain why Matera is a fractal city, to speak about its topological and fractal dimension, we have to observe this “self-similarity”. The same form repeats many times but on a different scale, and it offers the pleasant effect that trees give us when passing from the trunks to the branches, (see
Figure 10). The same harmony that sea sponges have has been recreated in the magical continuous passage of concave and convex spaces especially in Sasso Caveoso (one of the two sections of the Sassi zone).
Here, we want to suggest to a tourist guide how to explain this comparison between Matera and a mathematical sponge in a better way. To do this, we draw attention to the continuous succession of edges. The number and disposition of edges determine whether a polyhedron is a cube or a prism. Looking at its edges, we claim that Matera has the shape of a children’s book! First of all, it must be clear that what we mean by “book form” is overlapping sheets of the same size to create a volume. This definition only applies to cities or skyscrapers. The request that the sheets be of equal size in a volume is not necessary. When the sheets were made from dried plants, the shape of the sheet varied according to the humidity of the air. Even an ancient book in which the pages are cut to separate them has different-shaped pages. Finally, some books for children that fill our bookcases today have recesses and holes and images printed on the edge that make each page a different shape from the previous one. Matera is a book of the latter kind. Its stone pages all have different shapes and this influences the volume of its houses, the number and the position of its edges, and therefore, its story. The word “volume” is used in publishing and in geometry: These multiple meanings hide a secret. In the history of mathematics, it took thousands of years to formalize the process that leads from the superimposition of very thin (two-dimensional) sheets to the concept of volume (three-dimensional). Likewise, if we write on a single page with lowercase, infinite lines (one-dimensional), we would fill the whole sheet. Mathematicians also know a special book in which the sheets are pierced so differently that the overlapping pages give zero volume. It is like leafing through those books in which you can see images moving very quickly. The images are replaced by appropriately distributed voids and the result is the so-called Menger Sponge (see Reference [
12] and
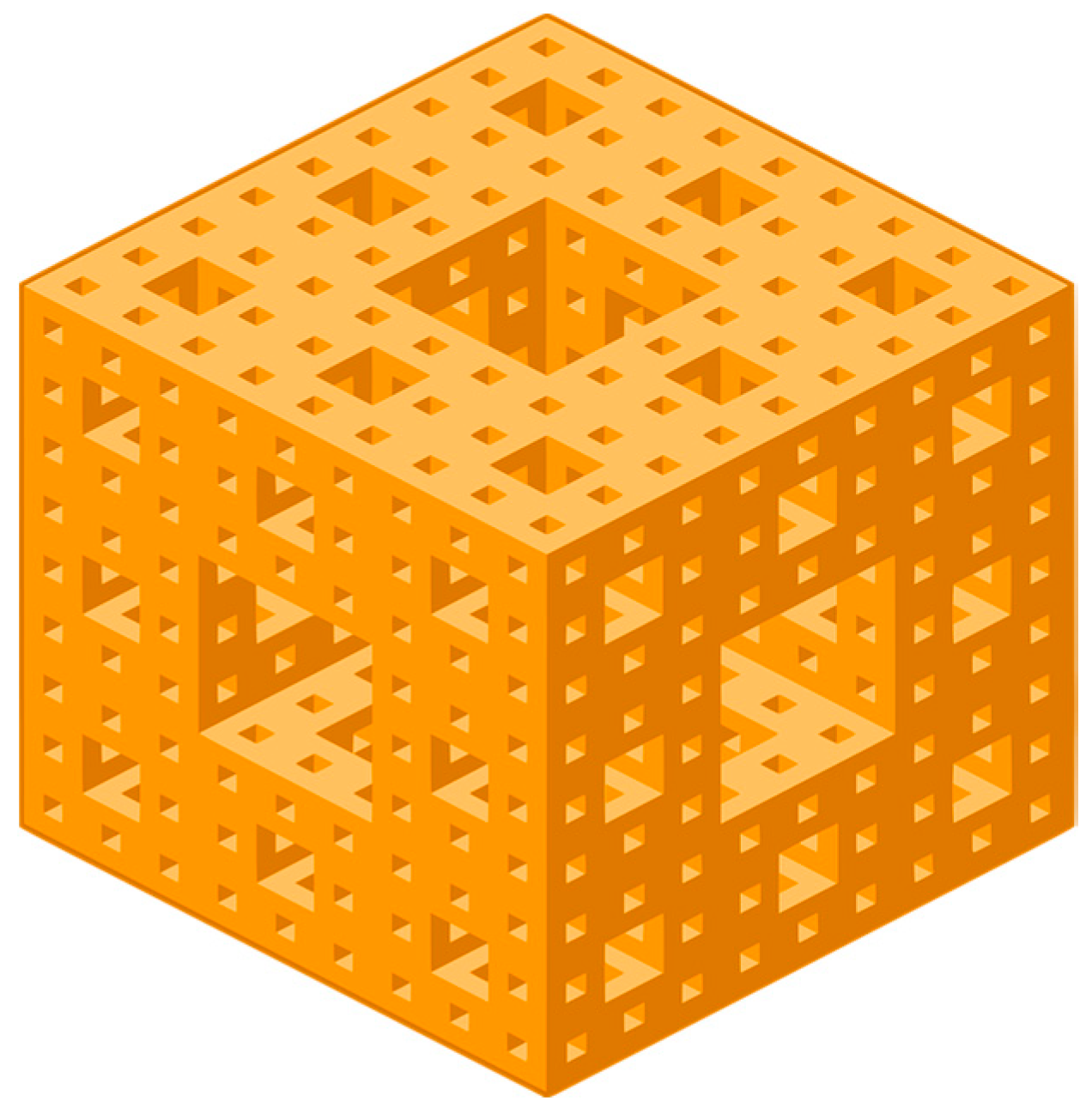
Figure 11). It can be seen as a book of blank pages with its infinite margins all annotated. It is an object of infinite perimeter and null volume. Among other things, for mathematicians this is the universal model of a one-dimensional whole (see Reference [
13] Section 4.4). It is a kind of continuous story to which all stories can be matched. This is a limiting process, we will never see it complete. Like the model of Matera, which can be seen at Palazzo Lanfranchi, it is nothing but an approximation of the city, so we can imagine figures closer and closer to that of the sponge.
After this informal description, let us detail the geometrical rule that gives this form starting from a cube. We make a triadic decomposition of the cube (this means it looks like a Rubiks Cube). If it has 27 smaller cubes and we remove 7: the central one and the 6 joined to its faces. There are 20 cubes left, on each one we can repeat this passage. You can then reapply the rule to the remaining 400 even smaller cubes. The resulting figure increases in complexity. The Fractal Menger Sponge is the limit figure of this process. One of the reasons why Matera is a fractal city is that the Sassi zone is obtained like this figure by repeating the same procedure on a different scale. Matera was born from excavated stone layers, the dwellings in the Sassi are the removed cubes whose floor-ceiling dividers are these stone pages impregnated with steps from remote and recent eras.
A Menger Sponge has a fractal dimension of ln(20)/ln 3 ≈ 2.7268, i.e., it is intermediate between two dimensions and three dimensions. For self-similar fractals, the dimension corresponds to the logarithm of the number of equal parts by which an object is subdivided and the logarithm of the scale factor that determines this division. The box-counting dimension can be used for a more complicated figure. In particular, using Benoit fractal analysis software, we can take a photo of Matera, transform it into a binary image and then trace a series of cross-links with ever closer meshes; the algorithm counts the number of boxes containing at least one element of the object. From the bi-logarithmic graph of the box number as a function of their width, the fractal dimension is determined as the slope of the regression line. One more dimension has to be added since we are starting from an image, while the object is in space. For Sasso Barisano (the other part of the Sassi zone), this gives 2.80, which is sufficiently close to that of an abstract Menger sponge, (for more details see Reference [
4]).
Now, let us imagine taking a photo at night. We would see a diffusion of lights from doors and windows. It corresponds to putting a light in every hole of the Menger sponge. If we asked for a name for this distribution we would invoke a Sierpinski carpet, that in
Figure 11 corresponds to a side of the Menger sponge.
Coming this time from global to local, we can concentrate on a very small zone of Matera, for example a wall. It is made of tuff. We can restrict our vision. Again, we see that it is full of emptiness. If we see a small part of tuff we soon understand that this rock is tuff. This kind of rock is described by a fractal called the Apollonius’ gasket. Therefore, Matera is contained in a fractal (the canyon), has a fractal urban structure (Peano curve for the map, see Reference [
4], Menger Sponge for the buildings) and contains fractals (the tuff and the distribution of light).