Projecting Mortality Rates to Extreme Old Age with the CBDX Model
Abstract
1. Introduction
2. The CBDX Model
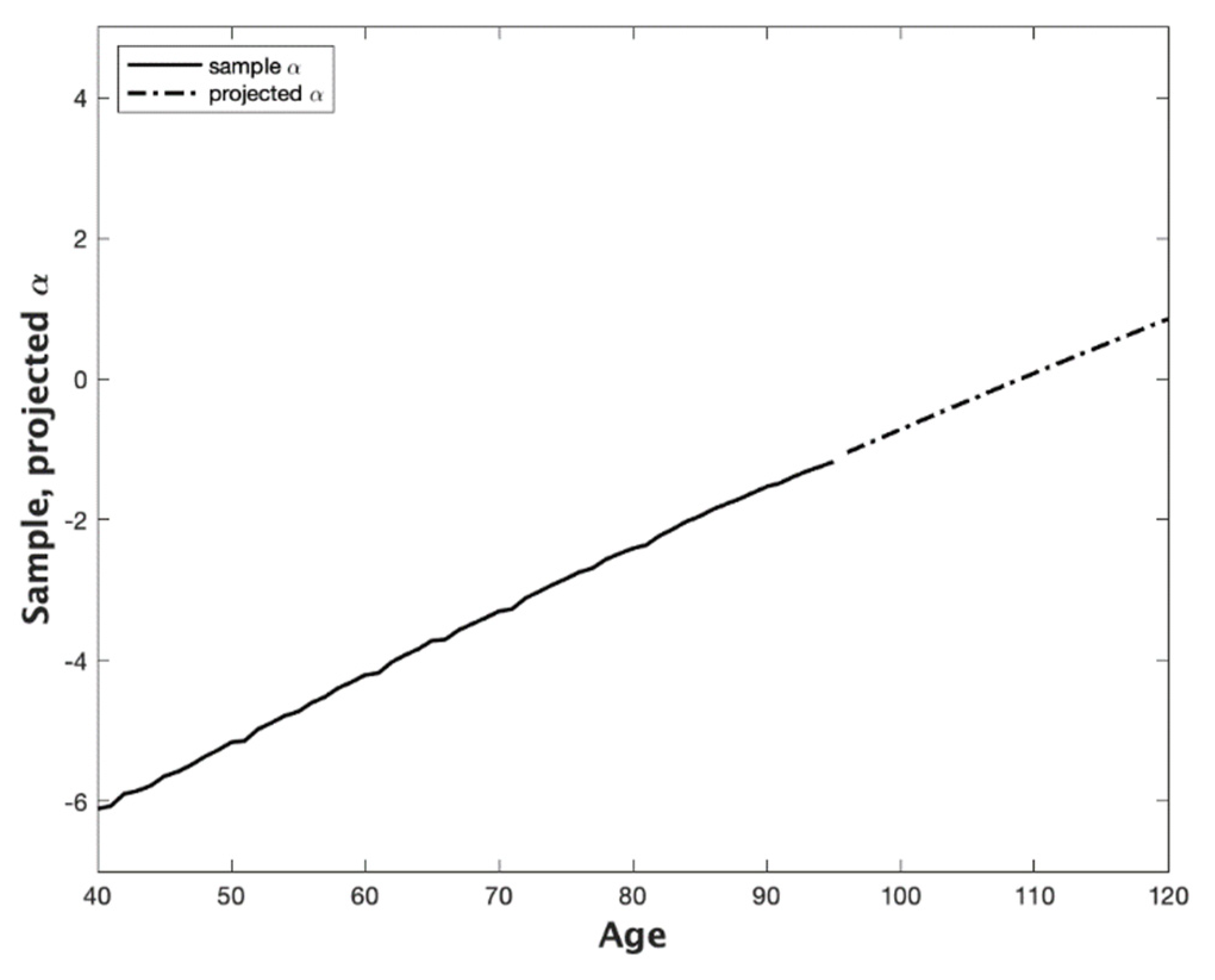
3. Projecting Mortality to Extreme Old Age with the CBDX Model: An Empirical Example Based on Australian Data
4. A Financial Application: Pricing a Life Annuity
5. Other Approaches to Projecting Mortality Rates to Older Ages
- A closure constraint of for all , on the grounds that ‘even if the human life span shows no sign of approaching a fixed limit imposed by biology or other factors, it seems reasonable to retain as a working assumption that the limit age 130 will not be exceeded’ (life tables have to be closed before projection by either truncating them at a specific age (e.g., 110, 120, or 130) or the (Kannisto, 1988, 1994, 1997 [15,16,17]) method is used to close a life table, as in some European regulatory life tables; DG assumed a maximum age of 130);
- An inflexion constraint for all , which makes the rate of mortality increase with age slow down at very old ages, consistent with the early empirical demographic data.
6. Conclusions
- Age 1—minimum age of the sample age range (we chose 40);
- Age 2—maximum age of the sample age range (we chose 95);
- Age 3—minimum age of the fitted age range (we chose 70);
- Age 4—maximum age of the fitted age range (we chose 95);
- Age 5—minimum age of the projection age range, i.e., the current age of the cohort being projected (we chose 70);
- Age 6—maximum age of the projection age range, i.e., the closing age of the life table (we chose 150).
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
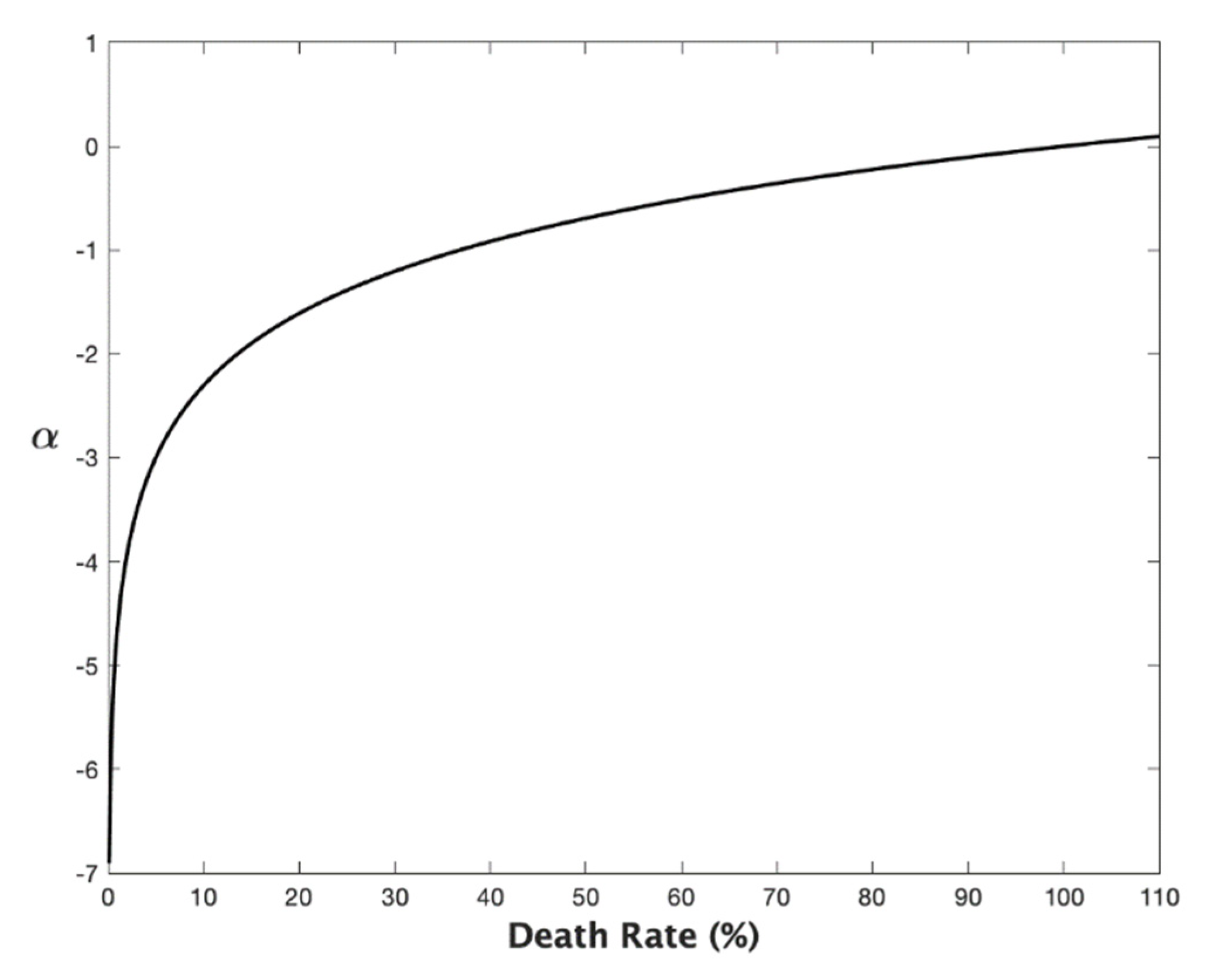
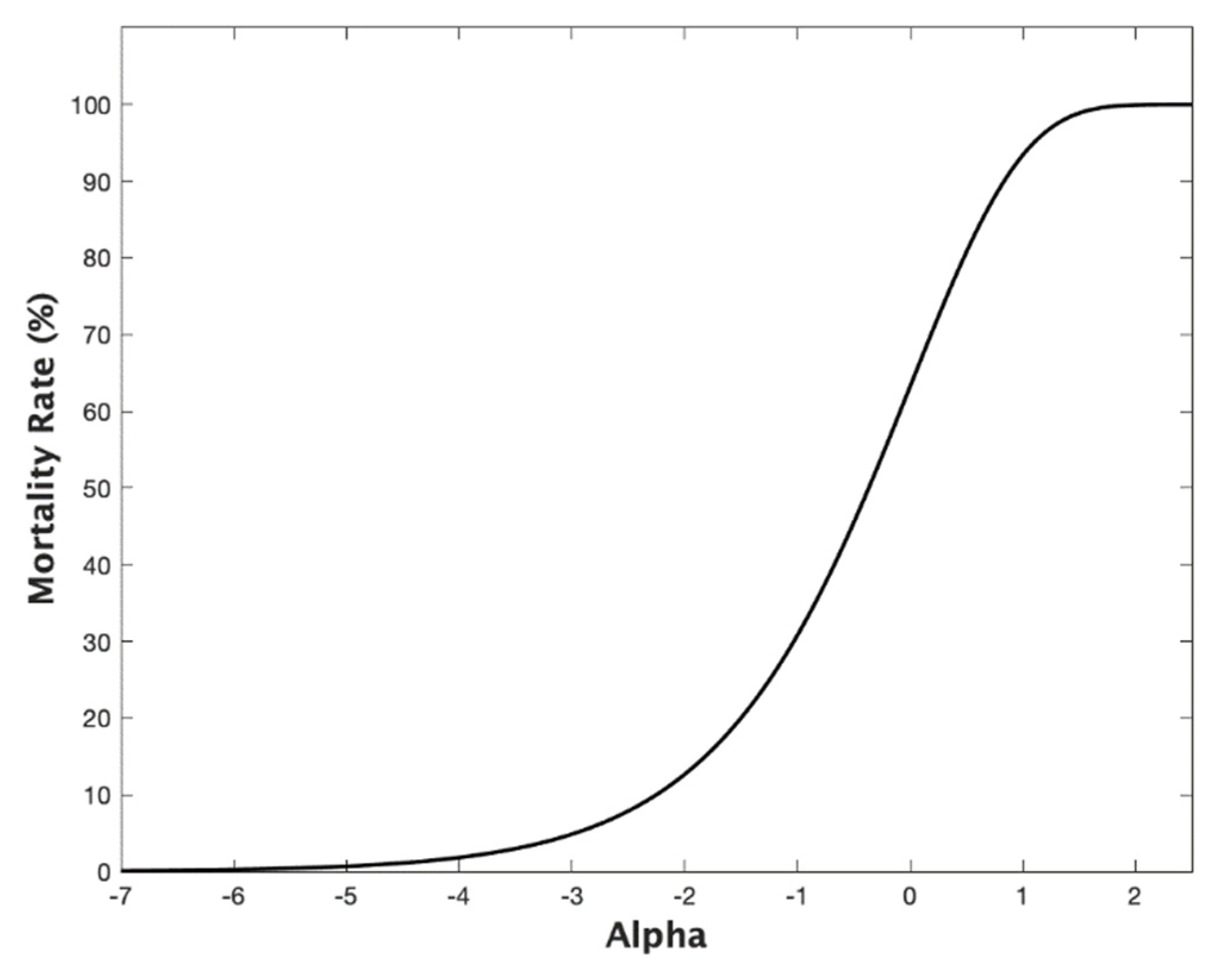
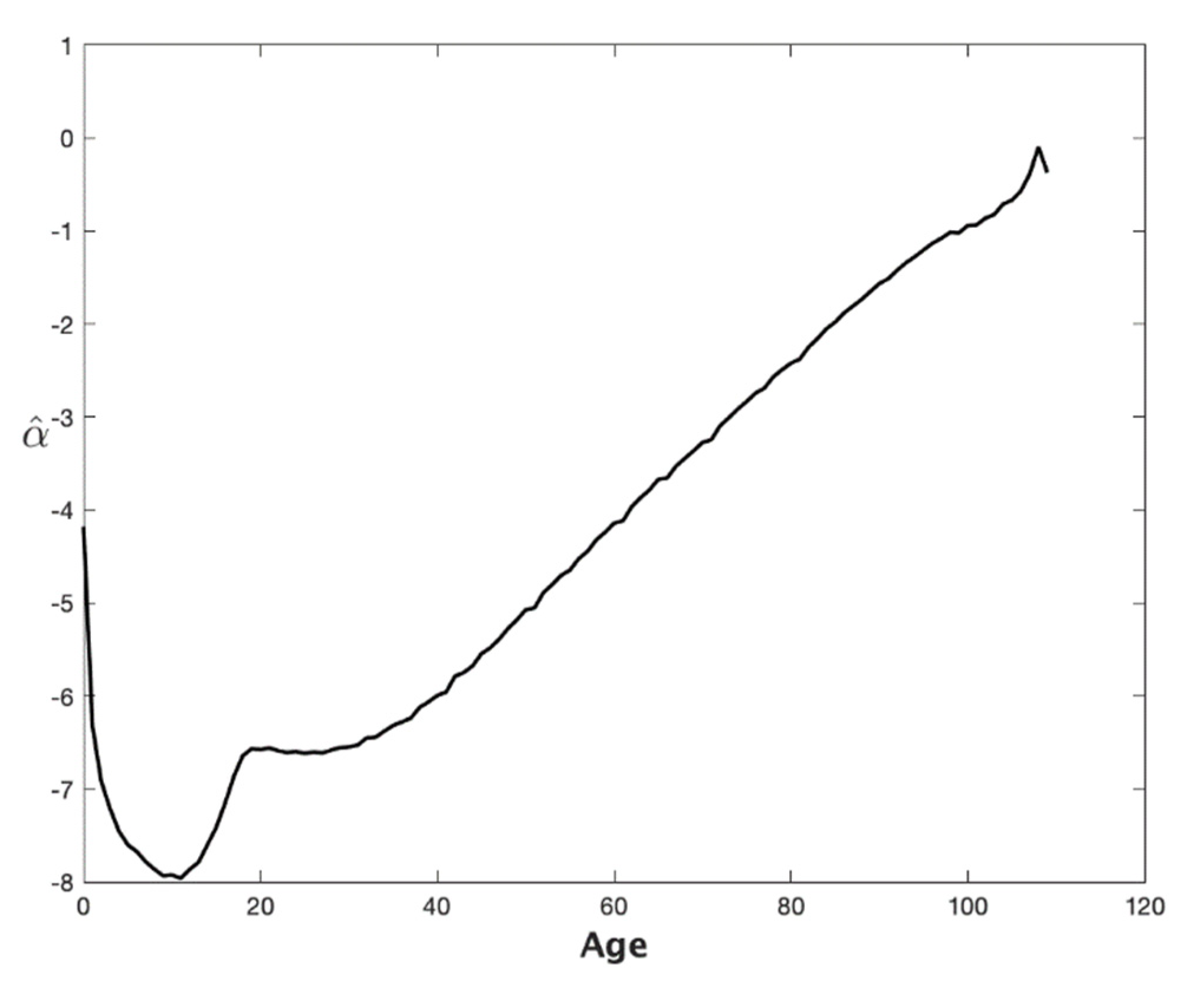
Appendix A. The Age State Variable, the Death Rate, and the Mortality Rate


References
- Dowd, K.; Cairns, A.J.G.; Blake, D. CBDX: A Workhorse Mortality Model from the Cairns-Blake-Dowd Family. Ann. Actuar. Sci. 2020, 14, 445–460. [Google Scholar] [CrossRef]
- Hunt, A.; Blake, D. A General Procedure for Constructing Mortality Models. N. Am. Actuar. J. 2014, 18, 116–138. [Google Scholar] [CrossRef]
- Cairns, A.J.G.; Blake, D.; Dowd, K. A Two-Factor Model for Stochastic Mortality with Parameter Uncertainty: Theory and Calibration. J. Risk Insur. 2006, 73, 687–718. [Google Scholar] [CrossRef]
- Cairns, A.J.G.; Blake, D.; Dowd, K.; Coughlan, G.D.; Epstein, D.; Ong, A.; Balevich, I. A Quantitative Comparison of Stochastic Mortality Models Using Data from England and Wales and the United States. N. Am. Actuar. J. 2009, 13, 1–35. [Google Scholar] [CrossRef]
- Currie, I.D. Modelling and Forecasting Mortality of the Very Old. ASTIN Bull. 2011, 41, 419–427. [Google Scholar]
- Lee, R.D.; Carter, L.R. Modeling and Forecasting U.S. Mortality. J. Am. Stat. Assoc. 1992, 87, 659–675. [Google Scholar] [CrossRef]
- Lindbergson, M. Mortality Among the Elderly in Sweden: 1988–1997. Scand. Actuar. J. 2001, 2001, 79–94. [Google Scholar] [CrossRef]
- Dowd, K.; Blake, D.; Cairns, A.J.G. The Myth of Methuselah and the Uncertainty of Death: The Mortality Fan Charts. Risks 2016, 4, 21. [Google Scholar] [CrossRef]
- Perks, W. On Some Experiments in the Graduation of Mortality Statistics. J. Inst. Actuar. 1932, 63, 12–57. [Google Scholar] [CrossRef]
- Denuit, M.; Goderniaux, A.-C. Closing and Projecting Life Tables using Loglinear Models. Bull. Swiss Assoc. Actuar. 2005, 1, 29–49. [Google Scholar]
- Gavrilova, N.S.; Gavrilov, L.A. Mortality Trajectories at Extreme Old Ages: A Comparative Study of Different Data Sources on U.S. Old-Age Mortality. Living 100 Monogr. 2014, 2014. Available online: https://www.soa.org/Library/Monographs/Life/Living-To-100/2014/mono-li14-3a-gavrilova.pdf (accessed on 20 January 2022).
- Gavrilov, L.A.; Gavrilova, N.S. New Trend in Old-Age Mortality: Gompertzialization of Mortality Trajectory. Gerontology 2019, 65, 451–457. [Google Scholar] [CrossRef] [PubMed]
- Thatcher, A.R.; Kannisto, V.; Andreev, K. The Survivor Ratio Method for Estimating Numbers at High Ages. Demogr. Res. 2002, 6, 1–18. [Google Scholar] [CrossRef]
- Vincent, P. La Mortalité des Vieillards. Population 1951, 6, 181–204. [Google Scholar] [CrossRef]
- Kannisto, V. On the Survival of Centenarians and the Span of Life. Popul. Stud. 1988, 42, 389–406. [Google Scholar] [CrossRef]
- Kannisto, V. Development of Oldest-Old Mortality, 1950–1990: Evidence from 28 Developed Countries; Odense Monographs on Population Aging No. 1; Odense University Press: Odense, Denmark, 1994. [Google Scholar]
- Kannisto, V. The Advancing Frontier of Survival; Odense Monographs on Population Aging No. 3; Odense University Press: Odense, Denmark, 1997. [Google Scholar]
- Horiuchi, S.; Wilmoth, J.R. Deceleration in the Age Pattern of Mortality at Older Ages. Demography 1998, 35, 391–412. [Google Scholar] [CrossRef] [PubMed]
- Thatcher, A.R.; Kannisto, V.; Vaupel, J.W. The Force of Mortality at Ages 80 to 120; Odense Monographs on Population Aging No. 5; Odense University Press: Odense, Denmark, 1998. [Google Scholar]
- Thatcher, A.R. The Long-term Pattern of Adult Mortality and the Highest Attained Age. J. R. Stat. Soc. Ser. A 1999, 162, 5–43. [Google Scholar] [CrossRef] [PubMed]
- Wilmoth, J.R.; Andreev, K.F.; Jdanov, D.A.; Glei, D.A. Methods Protocol for the Human Mortality Database: Version 5; Max Planck Institute for Demographic Research: Rostock, Germany, 2007. [Google Scholar]
- Gavrilov, L.A.; Gavrilova, N.S. The Biology of Life Span: A Quantitative Approach; Harwood Academic Publisher: New York, NY, USA, 1991. [Google Scholar]
- Preston, S.H.; Elo, I.T. Effects of Age Misreporting on Mortality Estimates at Older Ages. Popul. Stud. 1999, 53, 165–177. [Google Scholar] [CrossRef][Green Version]
- Newman, S.J. Errors as a Primary Cause of Late-life Mortality Deceleration and Plateaus. PLoS Biol. 2018, 16, e2006776. [Google Scholar] [CrossRef] [PubMed]
- Cairns, A.J.G.; Blake, D.; Dowd, K.; Kessler, A.R. Phantoms Never Die: Living with Unreliable Population Data. J. R. Stat. Soc. Ser. A 2016, 179, 975–1005. [Google Scholar] [CrossRef]



| Probability of survival to age 80 | 76.0% |
| Probability of survival to age 90 | 32.3% |
| Probability of survival to age 100 | 1.4% |
| Probability of survival to age110 | 1.20e−05% |
| Probability of survival to age 120 | 1.00e−18% |
| Probability of survival to age 130 | 6.30e−40% |
| Probability of survival to age 140 | 1.17e−65% |
| Probability of survival to age 150 | 5.84e−93% |
| Sample Years | Sample Ages | Fitted Ages | Annuity Price |
|---|---|---|---|
| 1921–2014 | 40–95 | 70–95 | 12.93 |
| 1921–2014 | 40–95 | 70–80 | 12.93 |
| 1921–2014 | 40–80 | 70–95 | 11.56 |
| 1921–2014 | 40–80 | 70–80 | 11.56 |
| 1950–2014 | 40–95 | 70–95 | 13.01 |
| 1950–2014 | 40–95 | 70–80 | 13.01 |
| 1950–2014 | 40–80 | 70–95 | 12.71 |
| 1950–2014 | 40–80 | 70–90 | 12.81 |
| Mean = 12.80; Minimum = 12.56; Maximum = 13.01 | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dowd, K.; Blake, D. Projecting Mortality Rates to Extreme Old Age with the CBDX Model. Forecasting 2022, 4, 208-218. https://doi.org/10.3390/forecast4010012
Dowd K, Blake D. Projecting Mortality Rates to Extreme Old Age with the CBDX Model. Forecasting. 2022; 4(1):208-218. https://doi.org/10.3390/forecast4010012
Chicago/Turabian StyleDowd, Kevin, and David Blake. 2022. "Projecting Mortality Rates to Extreme Old Age with the CBDX Model" Forecasting 4, no. 1: 208-218. https://doi.org/10.3390/forecast4010012
APA StyleDowd, K., & Blake, D. (2022). Projecting Mortality Rates to Extreme Old Age with the CBDX Model. Forecasting, 4(1), 208-218. https://doi.org/10.3390/forecast4010012