Semi-Dilute Dumbbells: Solutions of the Fokker–Planck Equation
Abstract
:1. Introduction
2. Materials and Methods
The Governing Equations
3. Results
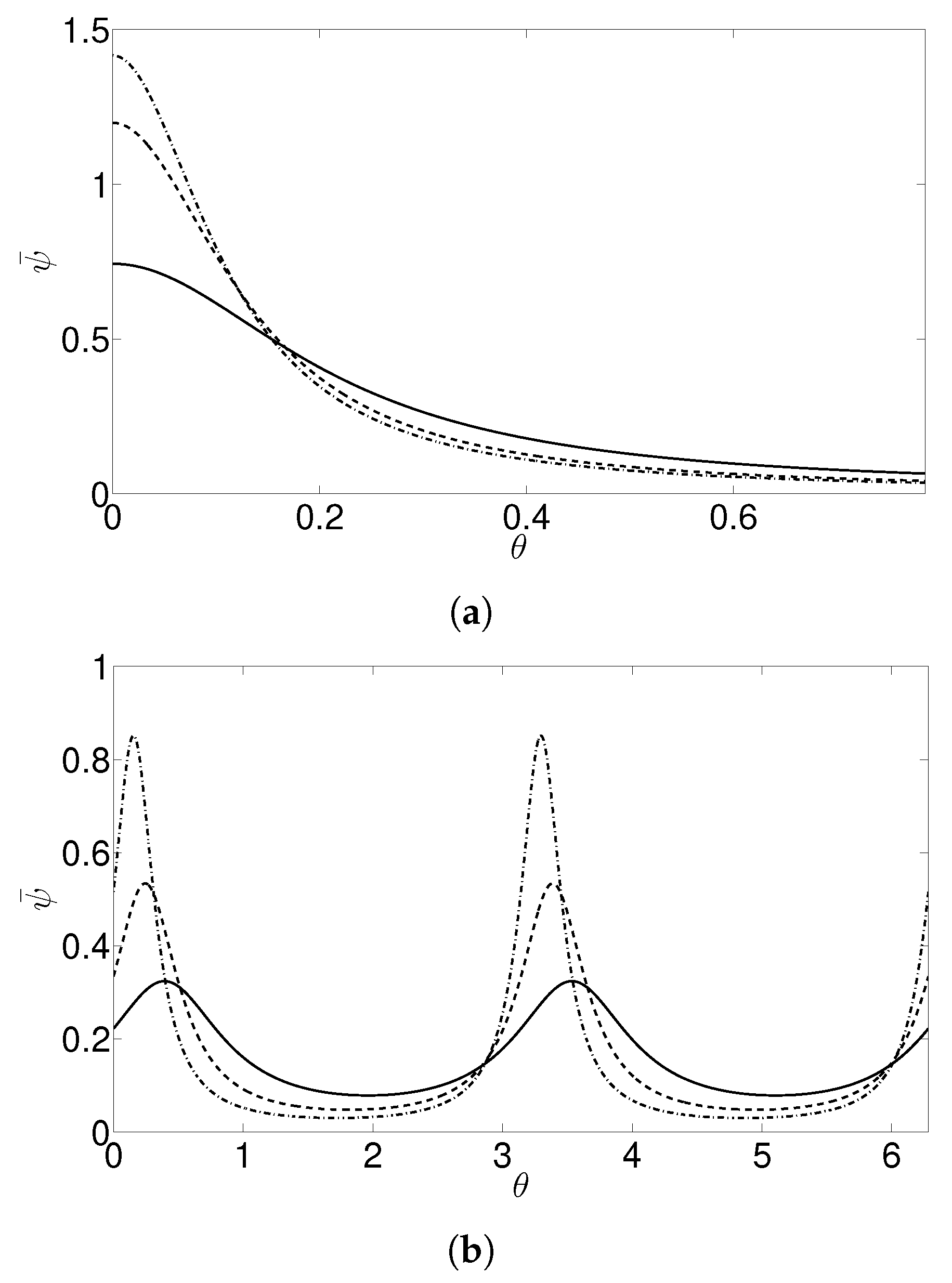
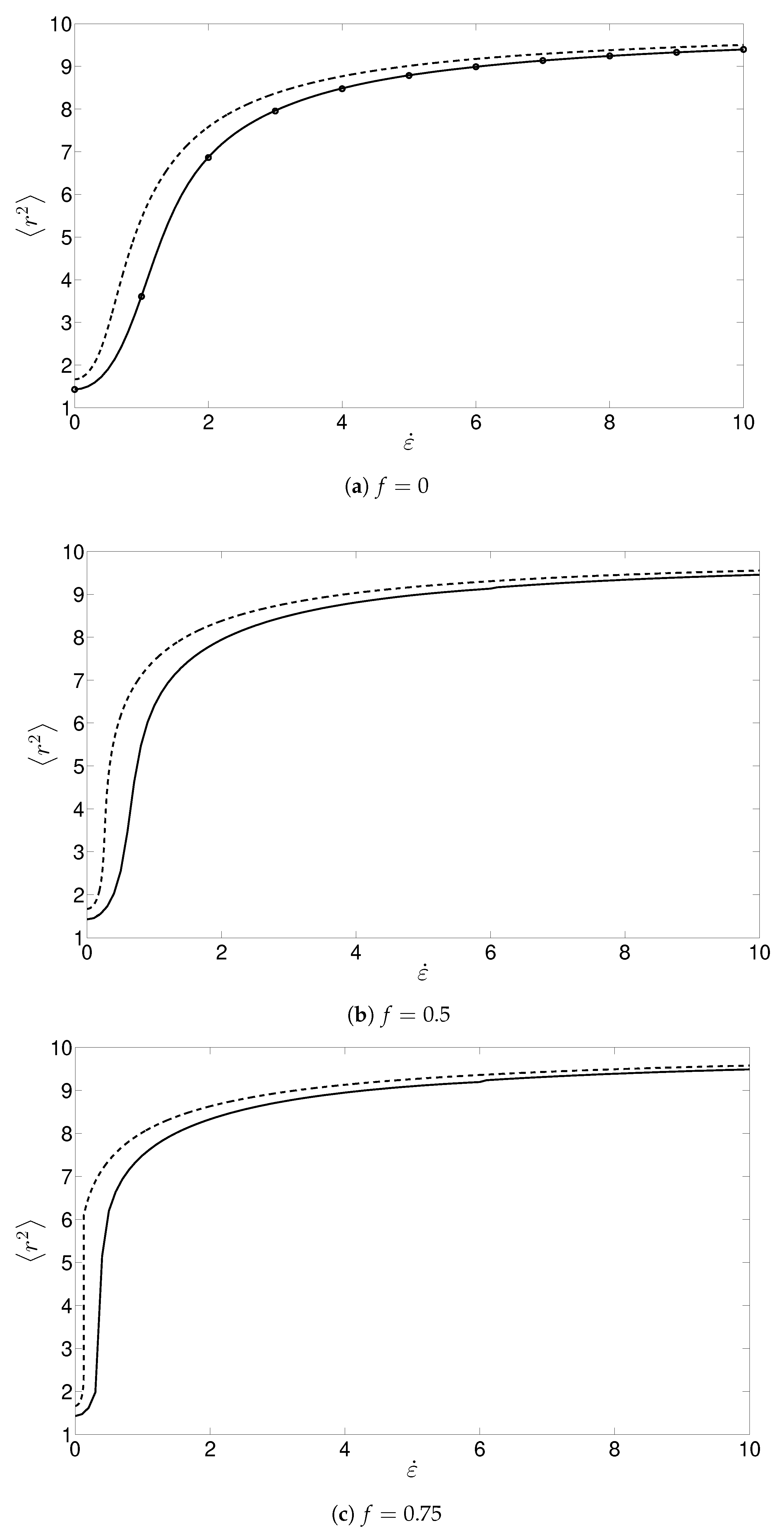
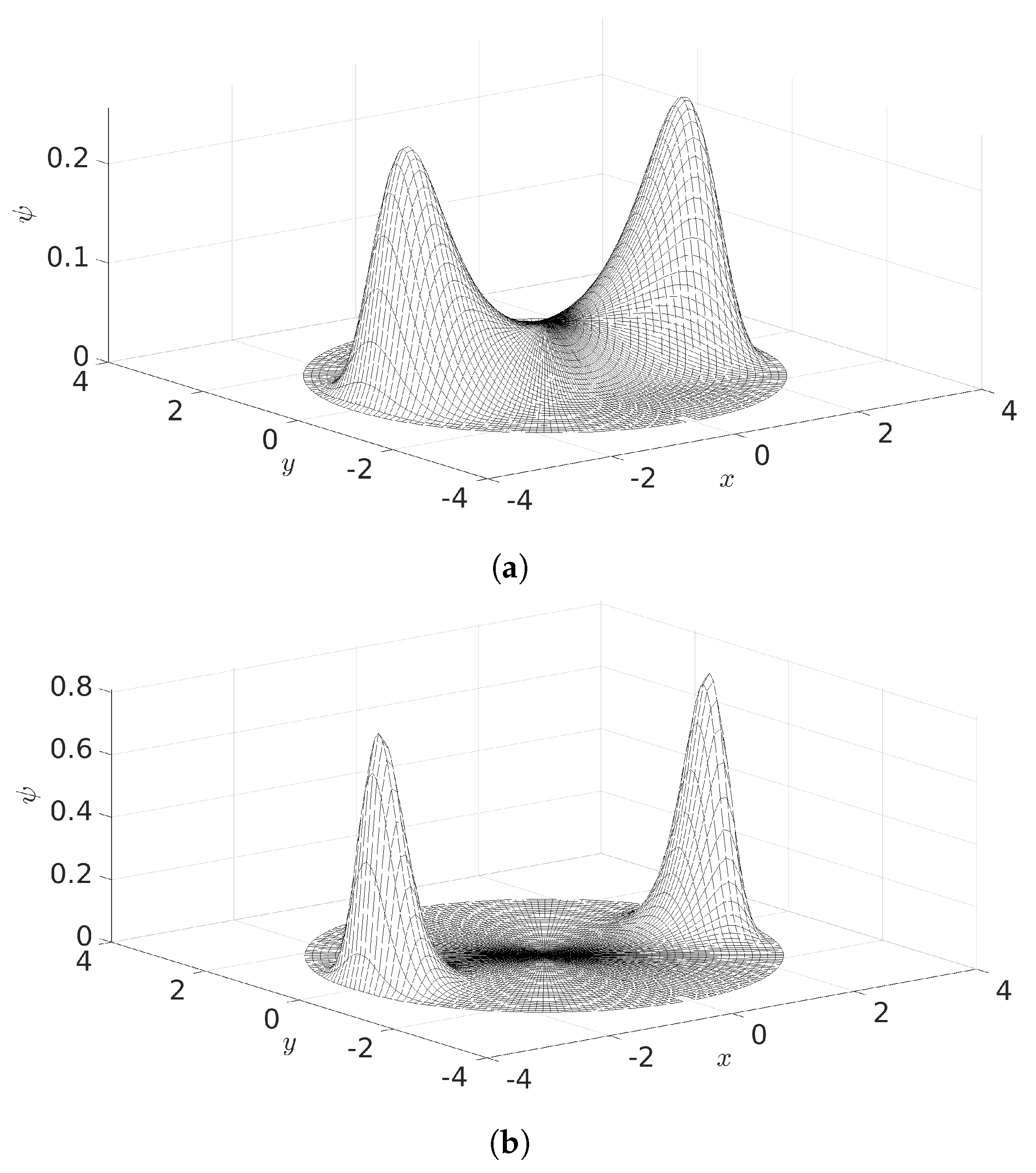
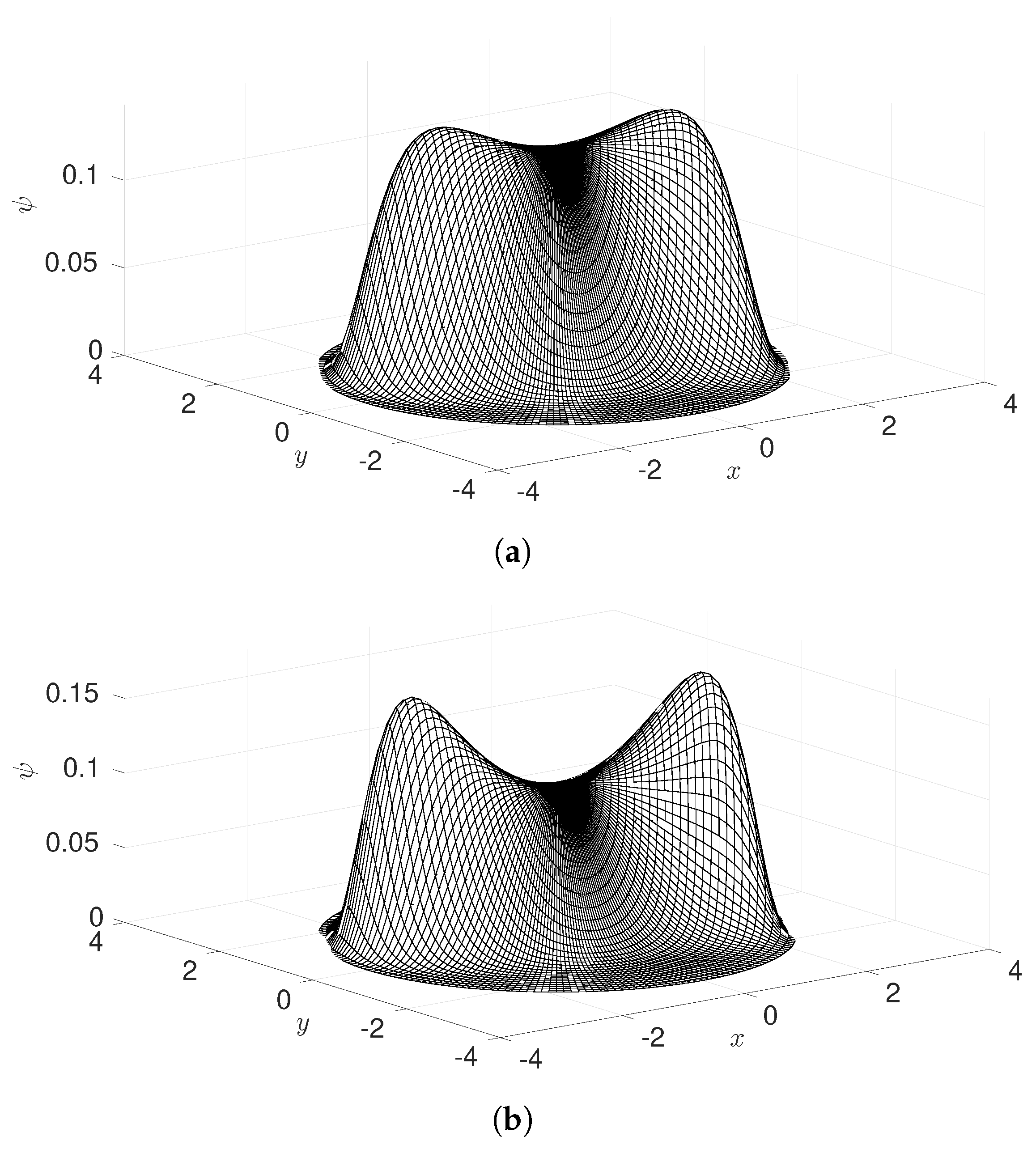
3.1. Mean-Field Force under Elongation
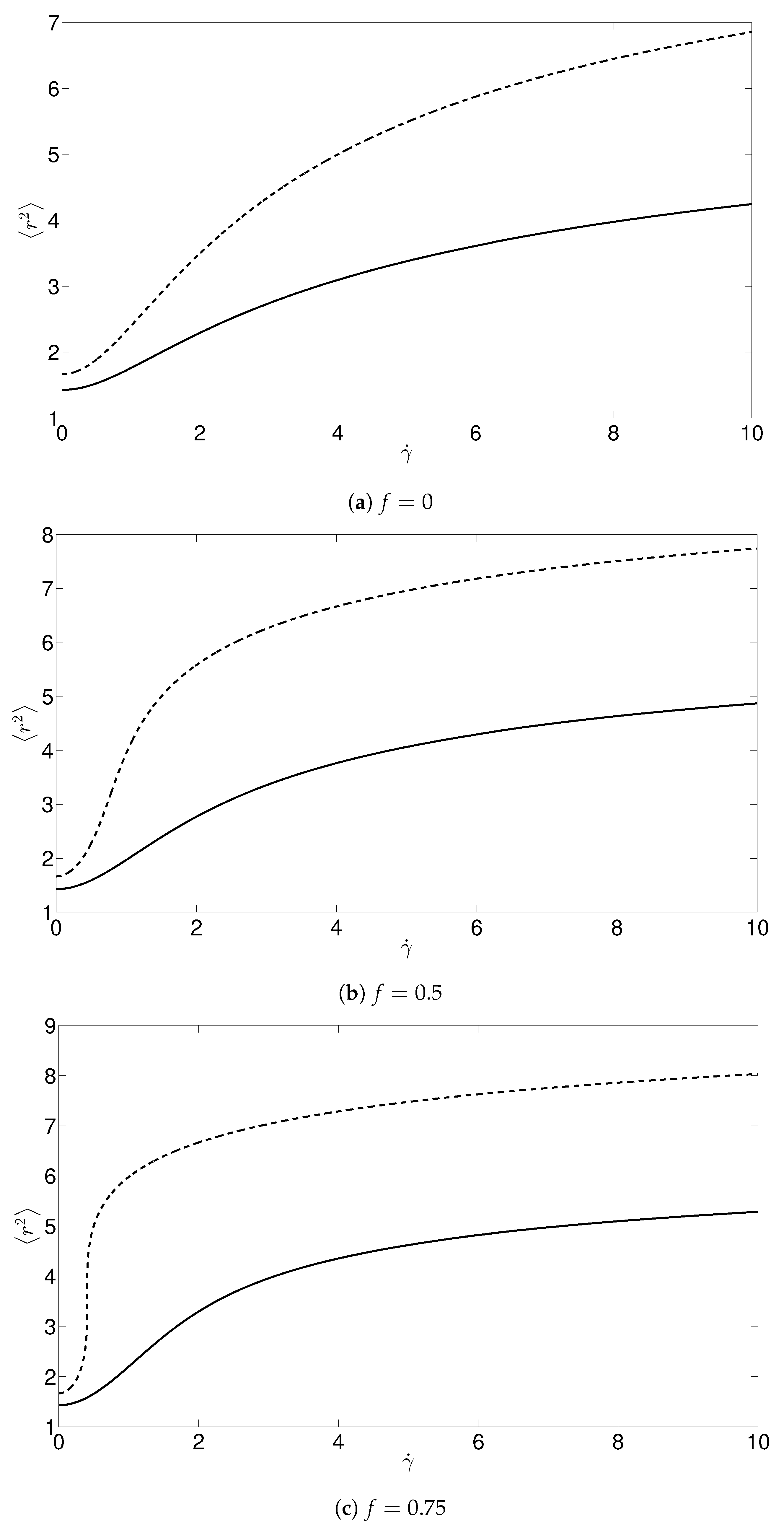
3.2. Mean-Field Force under Shear
3.3. FENE Solution
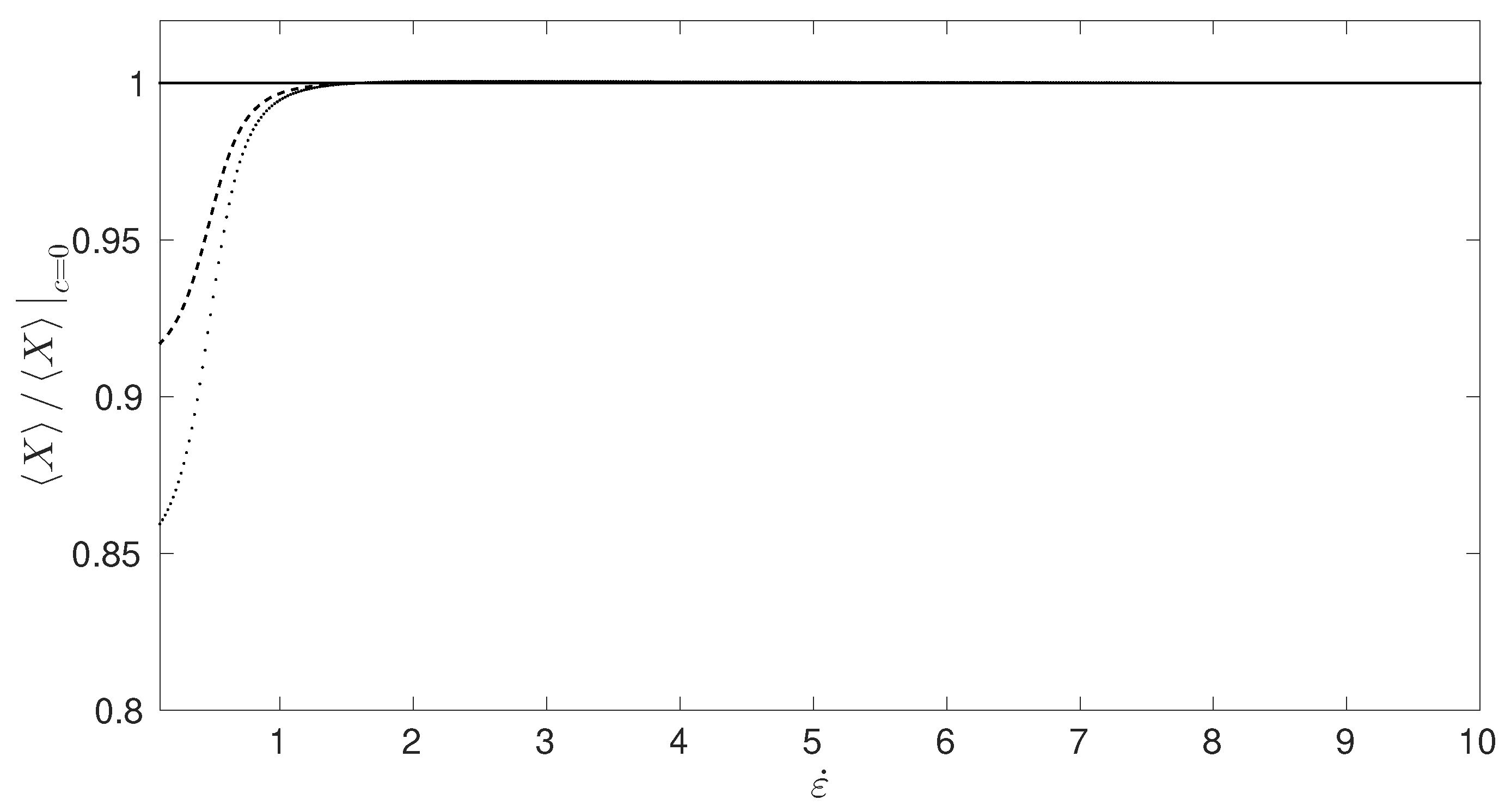
3.4. Comparison with Molecular Dynamics Simulations
4. Discussion
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| CDRT | Concentration dependent relaxation time |
| FENE | Finitely extensible nonlinear elastic |
| FENE-P | Finitely extensible nonlinear elastic with the Peterlin approximation |
| FP | Fokker–Planck |
References
- Warner, H.R. Kinetic theory and rheology of dilute suspensions of finitely extendible dumbbells. Ind. Eng. Chem. Fundam. 1972, 11, 379–387. [Google Scholar] [CrossRef]
- Adelman, S.A.; Freed, K.F. Microscopic theory of polymer internal viscosity: Mode coupling approximation for the rouse model. J. Chem. Phys. 1977, 64, 1380–1393. [Google Scholar] [CrossRef]
- Allegra, G. Internal viscosity in polymer chains: A critical analysis. J. Chem. Phys. 1986, 84, 5881–5890. [Google Scholar] [CrossRef]
- Farago, J.; Meyer, H.; Baschnagel, J.; Semenov, A.N. Hydrodynamic and viscoelastic effects in polymer diffusion. J. Condens. Matter Phys. 2012, 82, 284105–340000. [Google Scholar] [CrossRef]
- Ma, H.B.; Graham, M.D. Theory of shear-induced migration in dilute polymer solutions near solid boundaries. Phys. Fluids 2005, 17, 083103. [Google Scholar] [CrossRef] [Green Version]
- Ottinger, H.C. A model of dilute polymer solutions with hydrodynamic interaction and finite extensibility. I. Basic equations and series expansions. J. Non-Newton. Fluid Mech. 1987, 26, 207–246. [Google Scholar] [CrossRef]
- Bird, R.B.; Wiest, J.M. Anisotropic effects in dumbbell kinetic-theory. J. Rheol. 1985, 29, 519–532. [Google Scholar] [CrossRef]
- Delgado-Buscolioni, R. Dynamics of a single tethered polymer under shear flow. AIP Conf. Proc. 2007, 913, 114–120. [Google Scholar]
- Haber, C.; Ruiz, S.A.; Wirtz, D. Shape anisotropy of a single random-walk polymer. Proc. Natl. Acad. Sci. USA 2000, 97, 10792–10795. [Google Scholar] [CrossRef] [Green Version]
- Hur, J.S.; Shaqfeh, E.S.G.; Larson, R.G. Brownian dynamics simulations of single dna molecules in shear flow. J. Rheol. 2000, 44, 713–742. [Google Scholar] [CrossRef] [Green Version]
- Perkins, T.T.; Smith, D.E.; Chu, S. Single polymer dynamics in an elongational flow. Science 1997, 276, 2016–2021. [Google Scholar] [CrossRef] [Green Version]
- Schneggenburger, C.; Kröger, M.; Hess, S. An extended FENE dumbbell theory for concentration dependent shear-induced anisotropy in dilute polymer solutions. J. Non-Newton. Fluid Mech. 1996, 62, 235–251. [Google Scholar] [CrossRef]
- Kröger, M.; De Angelis, E. An extended FENE dumbbell model theory for concentration dependent shear-induced anisotropy in dilute polymer solutions: Addenda. J. Non-Newton. Fluid Mech. 2005, 125, 87–90. [Google Scholar] [CrossRef]
- Keunings, R. On the Peterlin approximation for finitely extensible dumbbells. J. Non-Newton. Fluid Mech. 1997, 68, 85–100. [Google Scholar] [CrossRef]
- Van Heel, A.P.G.; Hulsen, M.A.; van den Brule, B.H.A.A. On the selection of parameters in the fene-p model. J. Non-Newton. Fluid Mech. 1998, 75, 253–271. [Google Scholar] [CrossRef]
- Link, A.; Springer, J. Light-scattering from dilute polymer-solutions in shear-flow. Macromolecules 1993, 26, 464–471. [Google Scholar] [CrossRef]
- Babcock, H.P.; Smith, D.E.; Hur, J.S.; Shaqfeh, E.S.G.; Chu, S. Relating the microscopic and macroscopic response of a polymeric fluid in a shearing flow. Phys. Rev. Lett. 2000, 85, 2018–2021. [Google Scholar] [CrossRef] [PubMed]
- Hur, J.S.; Shaqfeh, E.S.G.; Babcock, H.P.; Smith, D.E.; Chu, S. Dynamics of dilute and semidilute DNA solutions in the start-up of shear flow. J. Rheol. 2001, 45, 421–450. [Google Scholar] [CrossRef]
- Clasen, C.; Plog, J.P.; Kulicke, W.M.; Owens, M.; Macosko, C.; Scriven, L.E.; Verani, M.; McKinley, G.H. How dilute are dilute solutions in extensional flows? J. Rheol. 2006, 50, 849–881. [Google Scholar] [CrossRef] [Green Version]
- Ng, R.C.Y.; Leal, L.G. Concentration effects on birefringence and flow modification of semidilute polymer-solutions in extensional flows. J. Rheol. 1993, 37, 443–468. [Google Scholar] [CrossRef]
- Feng, J.; Leal, L.G. Numerical simulations of the flow of dilute polymer solutions in a four-roll mill. J. Non-Newton. Fluid Mech. 1997, 72, 187–218. [Google Scholar] [CrossRef]
- Kai-Wen, H.; Sasmal, C.; Prakash, J.R.; Schroeder, C.M. Direct observation of DNA dynamics in semidilute solutions in extensional flow. J. Rheol. 2017, 61, 151–167. [Google Scholar]
- Stoltz, C.; de Pablo, J.J.; Graham, M.D. Concentration dependence of shear and extensional rheology of polymer solutions: Brownian dynamics simulations. J. Rheol. 2006, 50, 137–167. [Google Scholar] [CrossRef]
- Ng, R.C.Y.; Leal, L.G. A study of the interacting FENE dumbbell model for semidilute polymer-solutions in extensional flows. J. Rheol. 1993, 32, 25–35. [Google Scholar] [CrossRef]
- Bird, R.B.; Curtiss, C.F.; Armstrong, R.C.; Hassager, O. Dynamics of Polymeric Liquids, Volume 1: Fluid Mechanics, 2nd ed.; Wiley-Interscience: New York, NY, USA, 1987; pp. 1–672. [Google Scholar]
- Underhill, P.T.; Doyle, P.S. Accuracy of bead-spring chains in strong flows. J. Non-Newton. Fluid Mech. 2007, 145, 109–123. [Google Scholar] [CrossRef]
- Cohen, A. A Padé approximant to the inverse Langevin function. Rheol. Acta 1991, 30, 270–273. [Google Scholar] [CrossRef]
- Lielens, G.; Halin, P.; Jaumain, I.; Keunings, R.; Legat, V. New closure approximations for the kinetic theory of finitely extensible dumbbells. J. Non-Newton. Fluid Mech. 1998, 76, 249–279. [Google Scholar] [CrossRef]
- Lielens, G.; Keunings, R.; Legat, V. The FENE-L and FENE-LS closure approximations to the kinetic theory of finitely extensible dumbbells. J. Non-Newton. Fluid Mech. 1999, 87, 179–196. [Google Scholar] [CrossRef]
- Bird, R.B.; Curtiss, C.F.; Armstrong, R.C.; Hassager, O. Dynamics of Polymeric Liquids, Volume 2: Kinetic Theory, 2nd ed.; Wiley-Interscience: New York, NY, USA, 1987; pp. 1–464. [Google Scholar]
- Kröger, M. Models for Polymeric and Anisotropic Liquids, 1st ed.; Lecture Notes in Physics 675; Springer: Berlin/Heidelberg, Germany, 2005; pp. 1–215. [Google Scholar]
- Kuzuu, N. Constitutive equation for nematic liquid-crystals under weak velocity-gradient derived from a molecular kinetic-equation. J. Phys. Soc. Jpn. 1983, 52, 3486–3494. [Google Scholar] [CrossRef]
- Peterlin, A. Hydrodynamics of macromolecules in a velocity field with longitudinal gradient. J. Polym. Sci. B Polym. Phys. 1966, 4, 287–291. [Google Scholar] [CrossRef]
- Ottinger, H.C. Stochastic Processes in Polymeric Fluids: Tools and Examples for Developing Simulation Algorithms, 1st ed.; Springer: Berlin, Germany; New York, NY, USA, 1996; pp. 1–384. [Google Scholar]
- Chaffin, S. Non-Newtonian Fluids in Complex Geometries. Ph.D. Thesis, University of Sheffield, Sheffield, UK, 2016. [Google Scholar]
- Hess, S. Rheological properties via nonequilibrium molecular dynamics: From simple towards polymeric liquids. J. Non-Newton. Fluid Mech. 1987, 23, 305–319. [Google Scholar] [CrossRef]
- Herrchen, M. A detailed comparison of various fene dumbbell models. J. Non-Newton. Fluid Mech. 1997, 68, 17–42. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chaffin, S.; Rees, J. Semi-Dilute Dumbbells: Solutions of the Fokker–Planck Equation. J 2021, 4, 341-355. https://doi.org/10.3390/j4030026
Chaffin S, Rees J. Semi-Dilute Dumbbells: Solutions of the Fokker–Planck Equation. J. 2021; 4(3):341-355. https://doi.org/10.3390/j4030026
Chicago/Turabian StyleChaffin, Stephen, and Julia Rees. 2021. "Semi-Dilute Dumbbells: Solutions of the Fokker–Planck Equation" J 4, no. 3: 341-355. https://doi.org/10.3390/j4030026
APA StyleChaffin, S., & Rees, J. (2021). Semi-Dilute Dumbbells: Solutions of the Fokker–Planck Equation. J, 4(3), 341-355. https://doi.org/10.3390/j4030026





