On Some New Neighborhood Degree-Based Indices for Some Oxide and Silicate Networks
Abstract
:1. Introduction
2. Motivation
3. Computation of Topological Indices
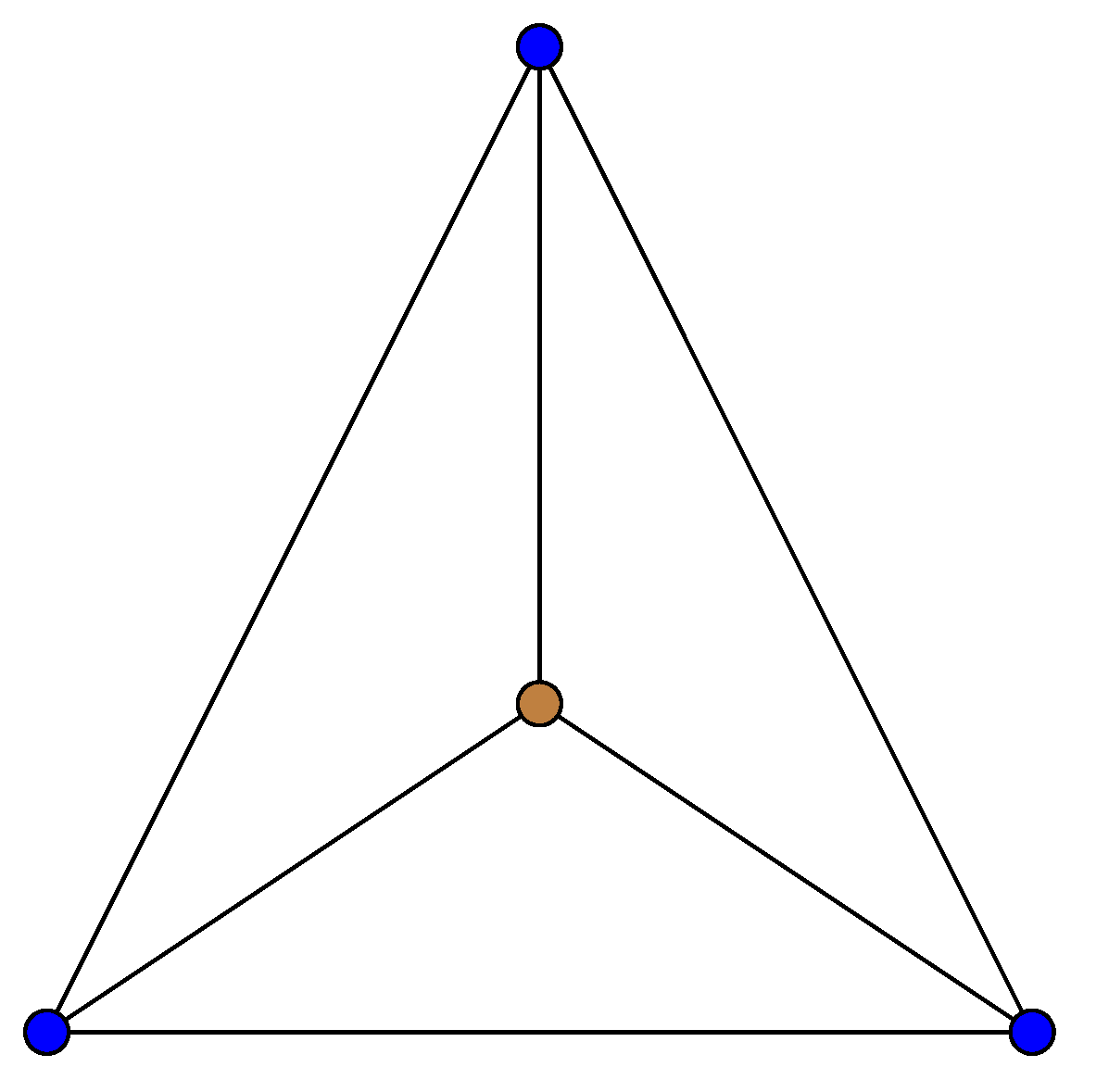
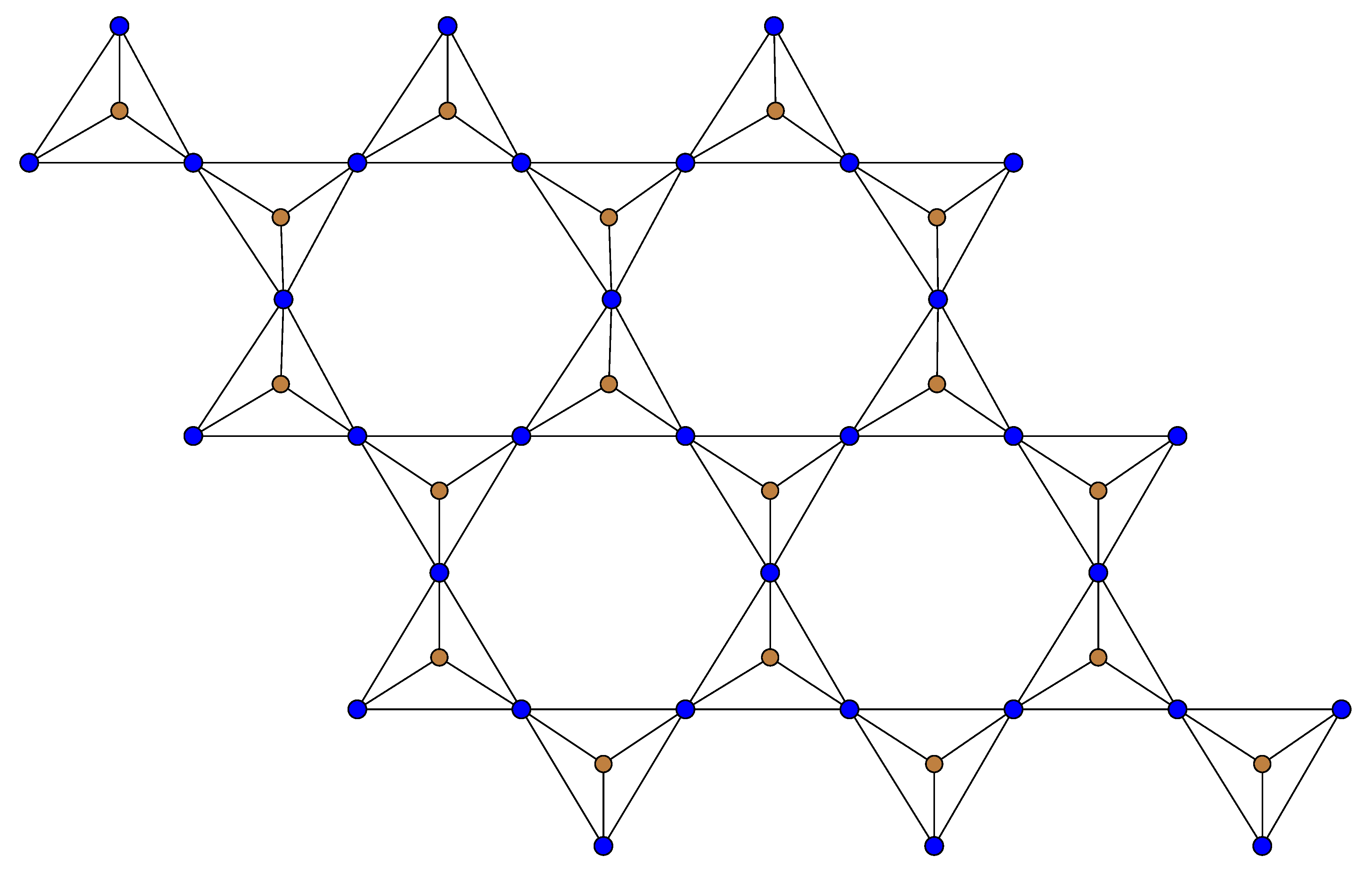
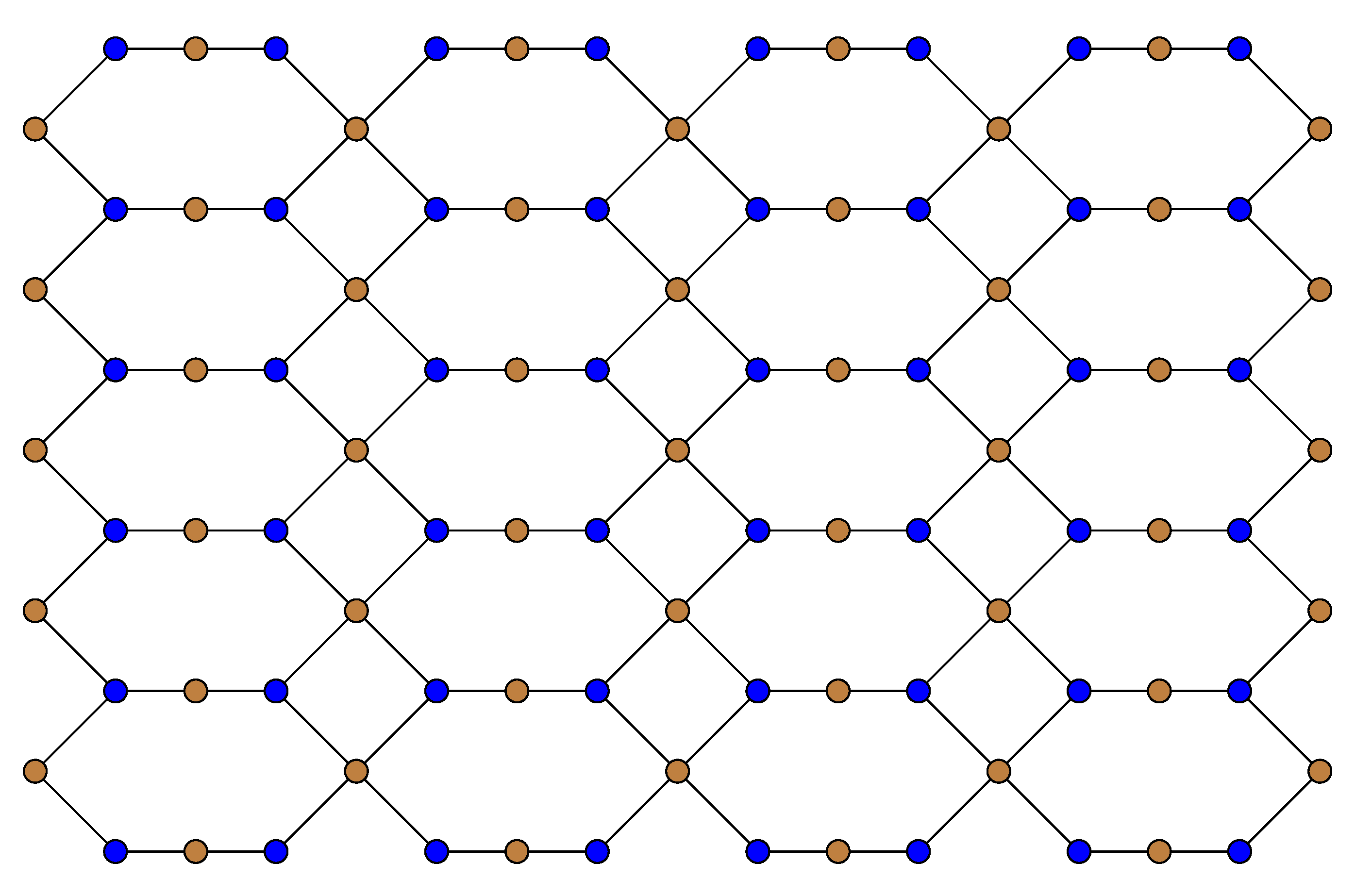
3.1. Dominating Silicate and Oxide )) Networks
- (i)
- (ii)
- (i)
- Let . Now, applying vertex partition (1) on the definition of the neighborhood Zagreb index, we get:Using Table 1, we obtain:
- (ii)
- Let . Now, using vertex partition (3) and Table 2 on the definition of the neighborhood Zagreb index, we obtain:After simplifying and arranging the terms, the required result can be obtained easily.
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
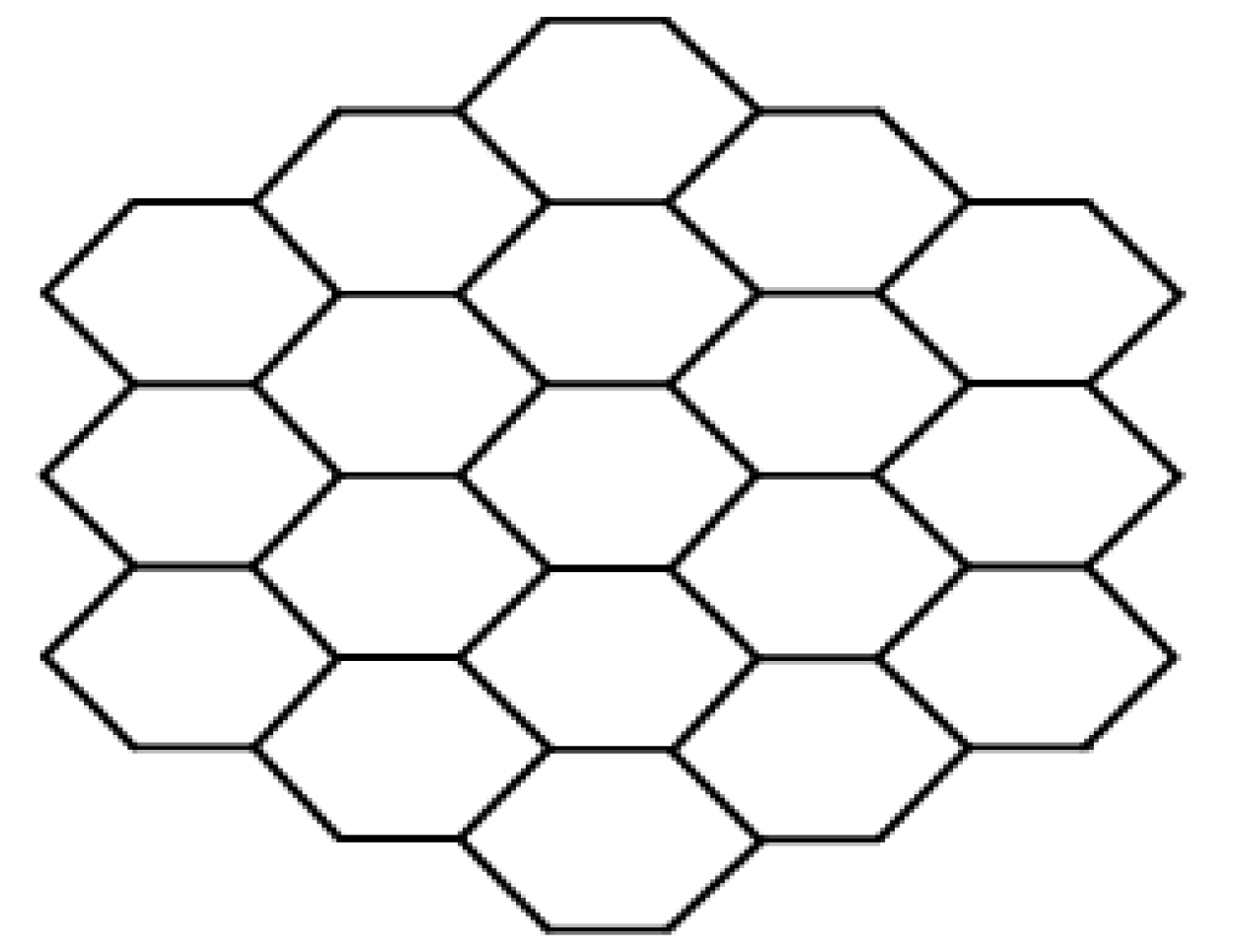
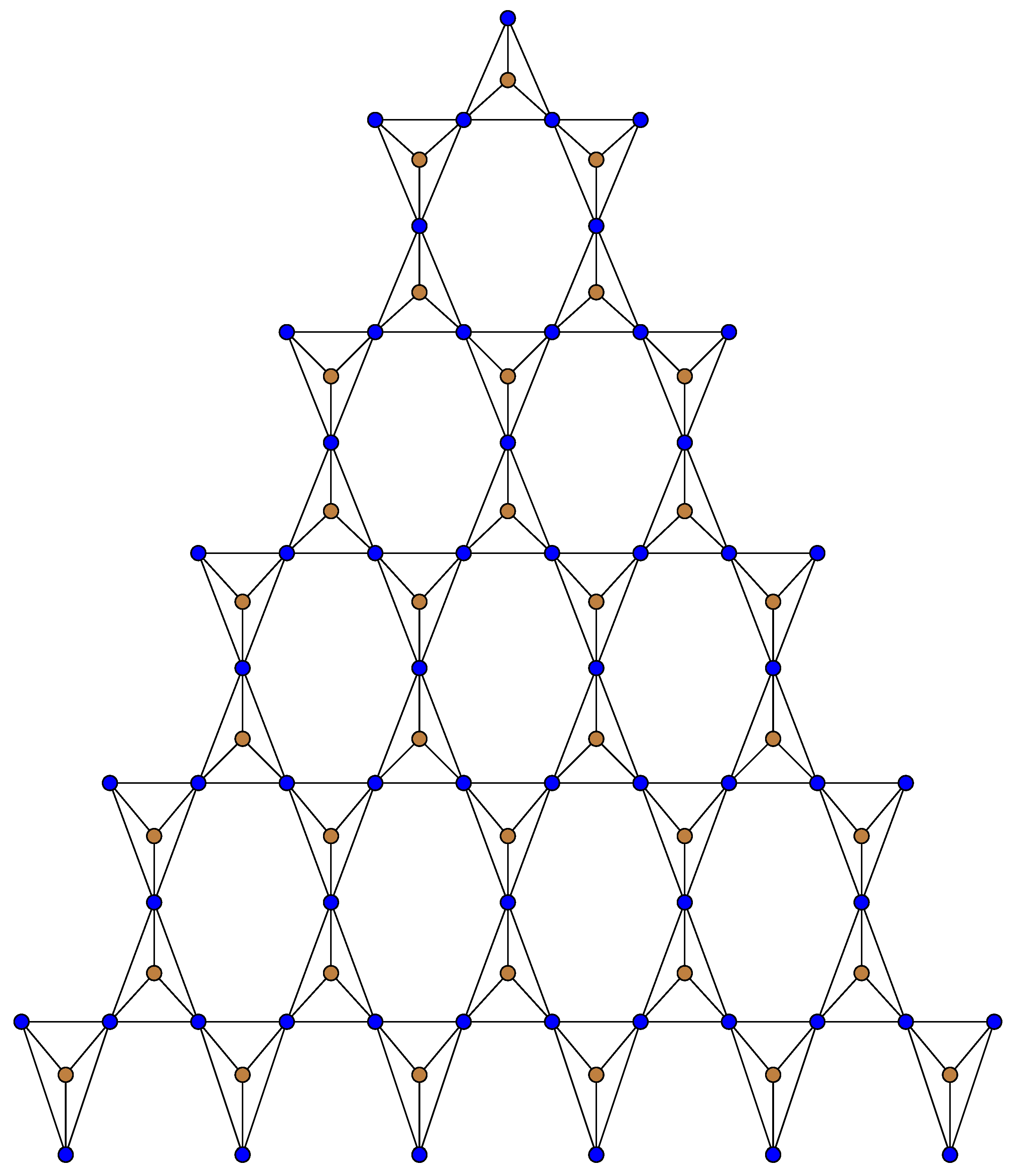
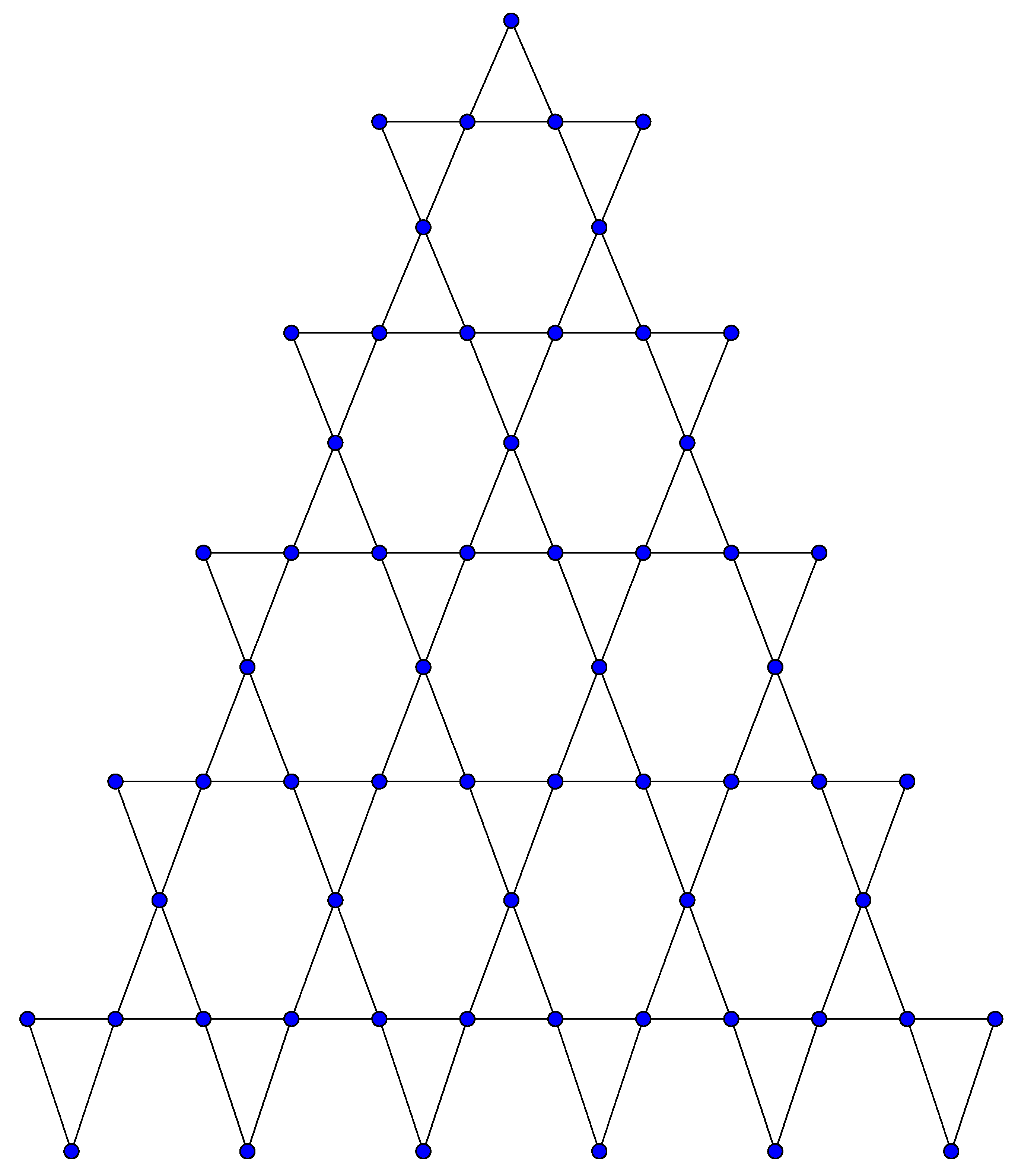
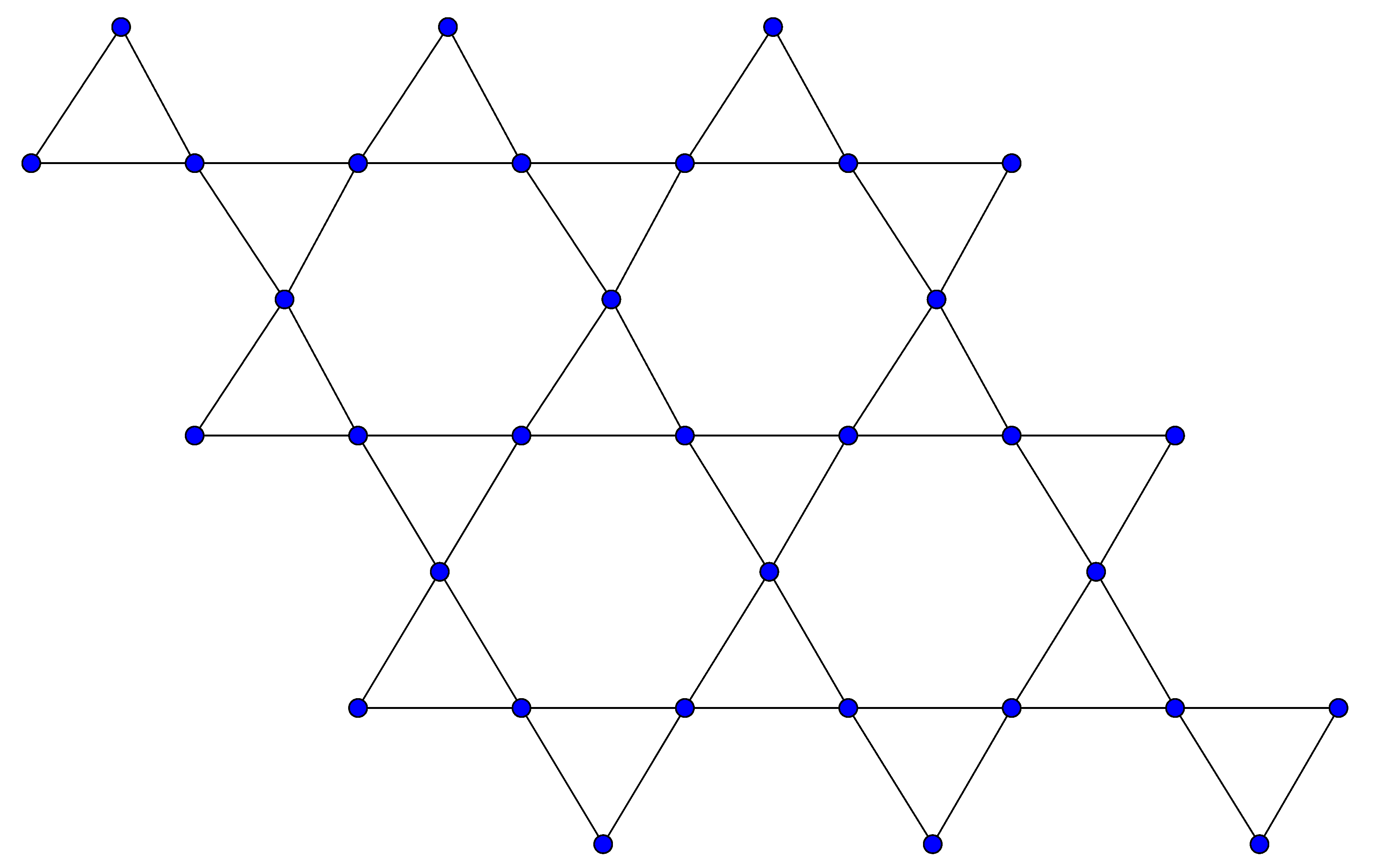
3.2. Regular Triangulene Silicate and Oxide ) Networks
- (i)
- (ii)
- (i)
- Let . Now, apply vertex partition (5) and Table 5 on the definition of the neighborhood Zagreb index, we get:After simplification, the desired result can be obtained easily.
- (ii)
- Let . Now, using vertex partition (7) and Table 6 on the definition of the neighborhood Zagreb index, we obtain:After simplifying and arranging the terms, the required result can be obtained easily.
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- After simplification and arranging the terms, we can get the desired result.
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
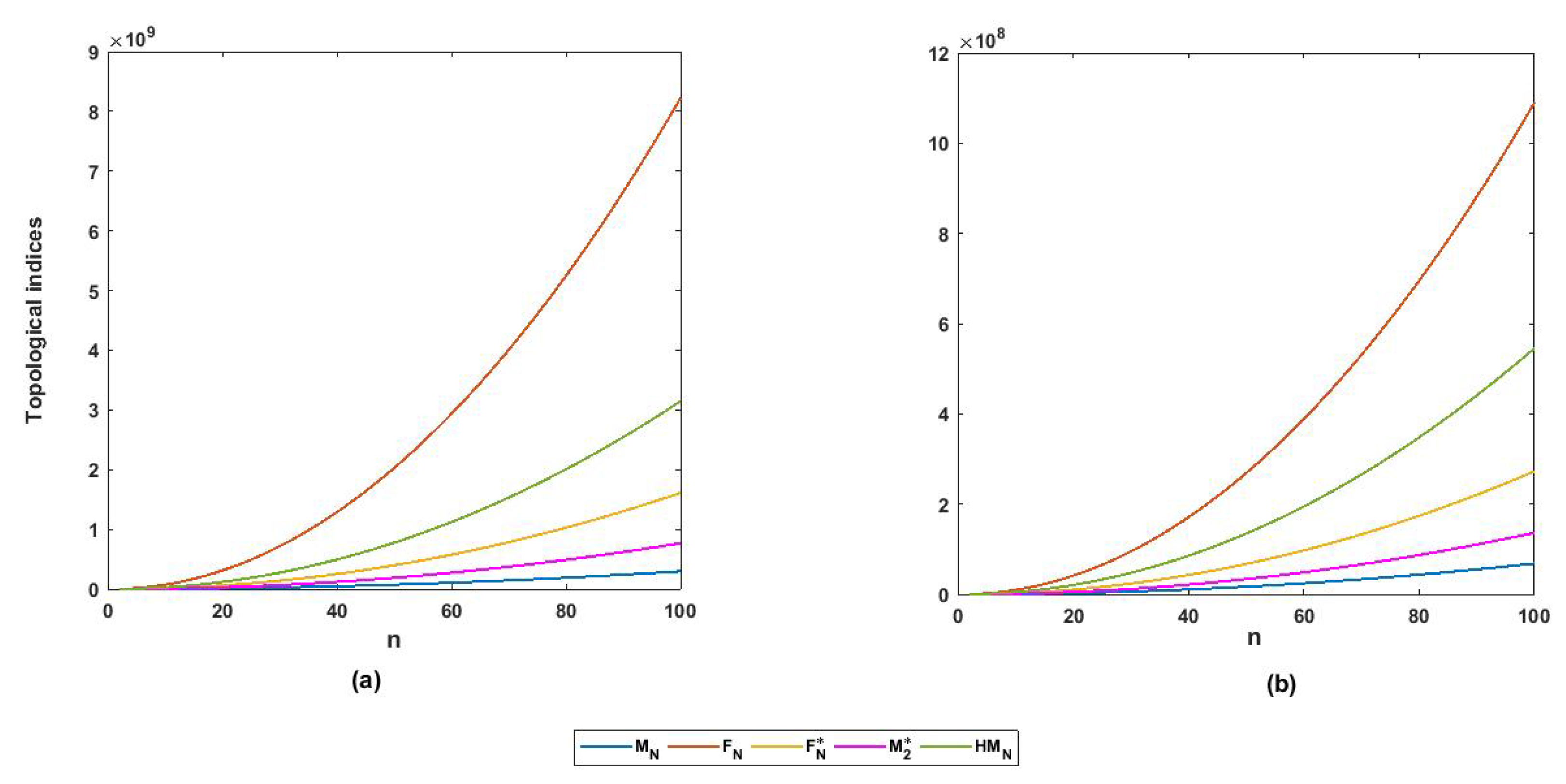
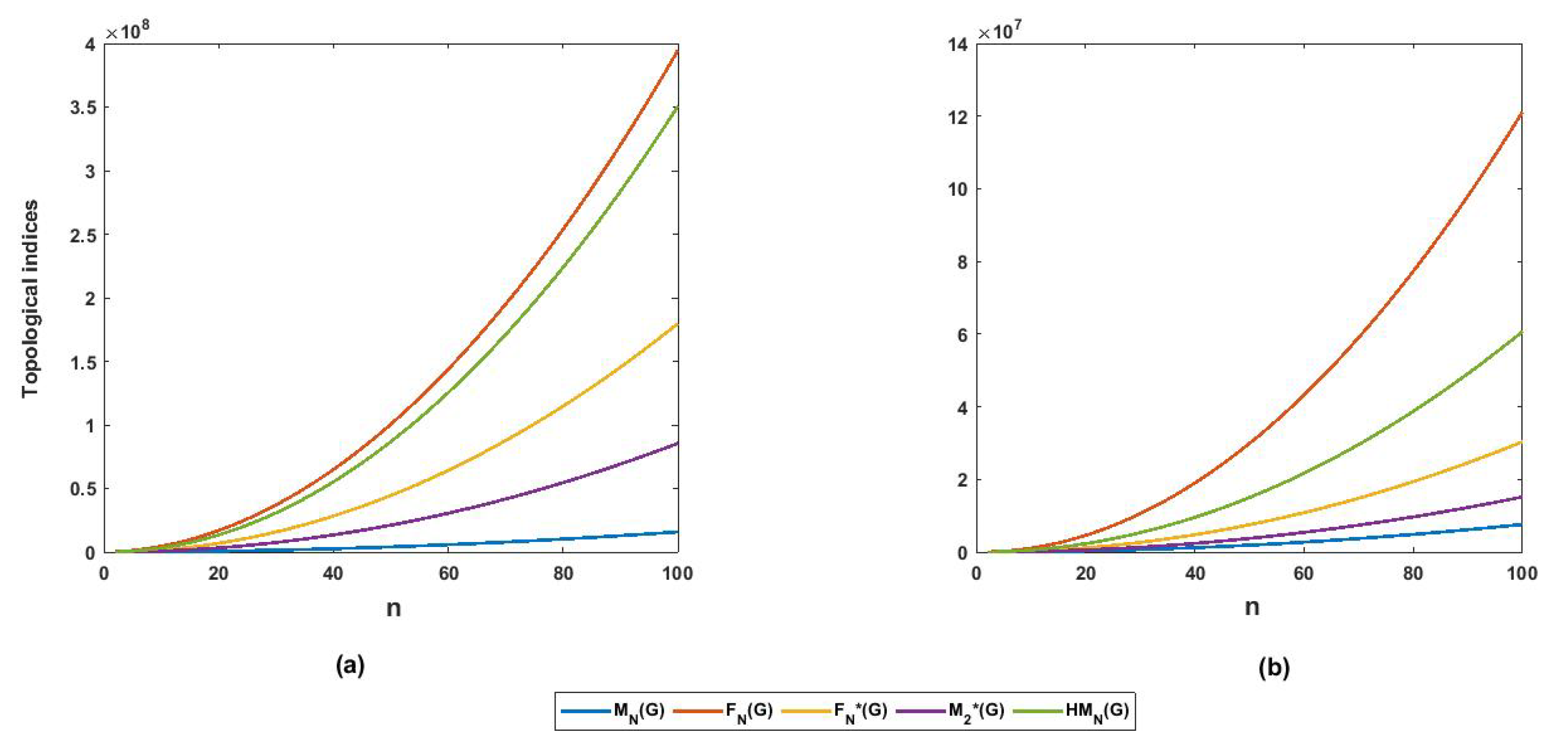
3.3. Rhombus Silicate () and Oxide () Networks
- (i)
- (ii)
- (i)
- (ii)
- (i)
- After simplification and arranging the terms, the required result is obtained.
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
- (i)
- (ii)
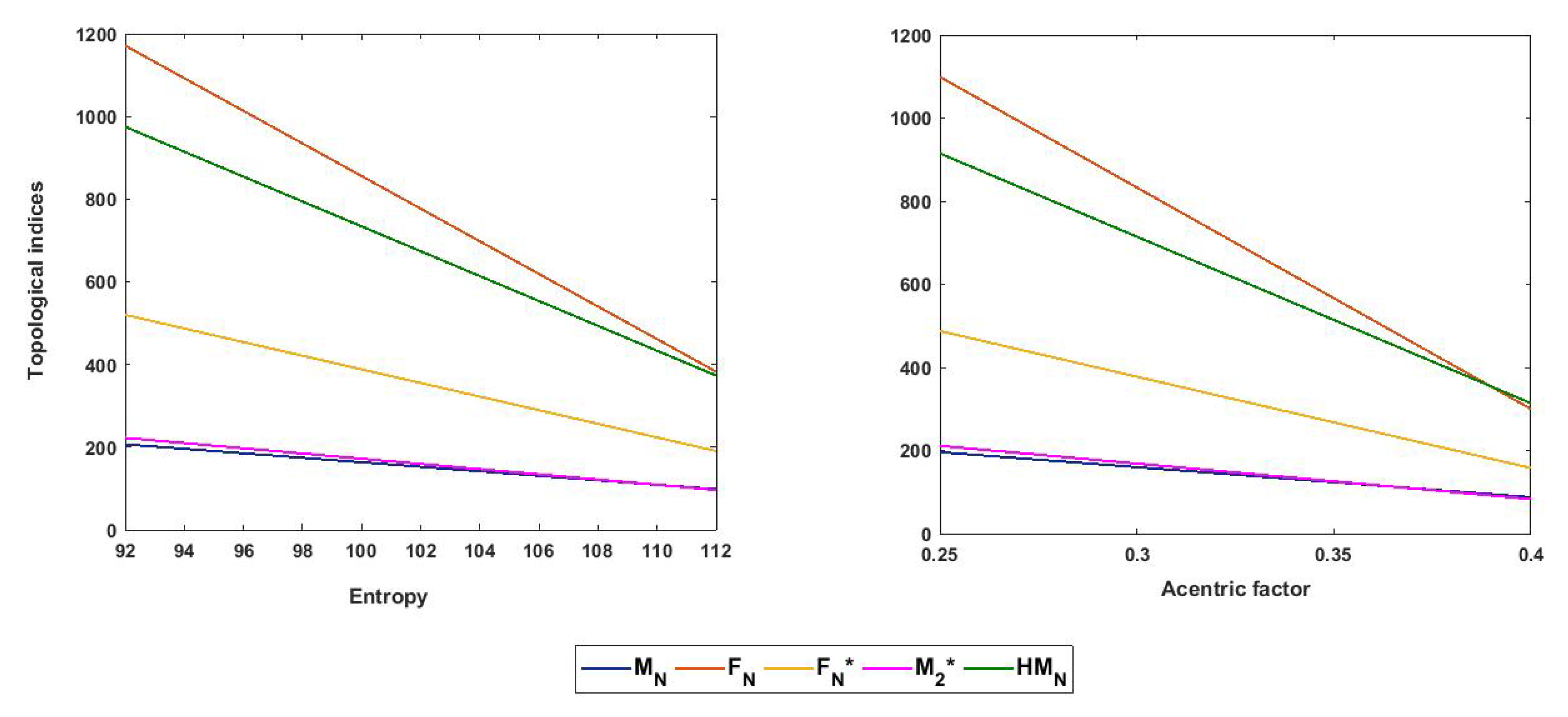
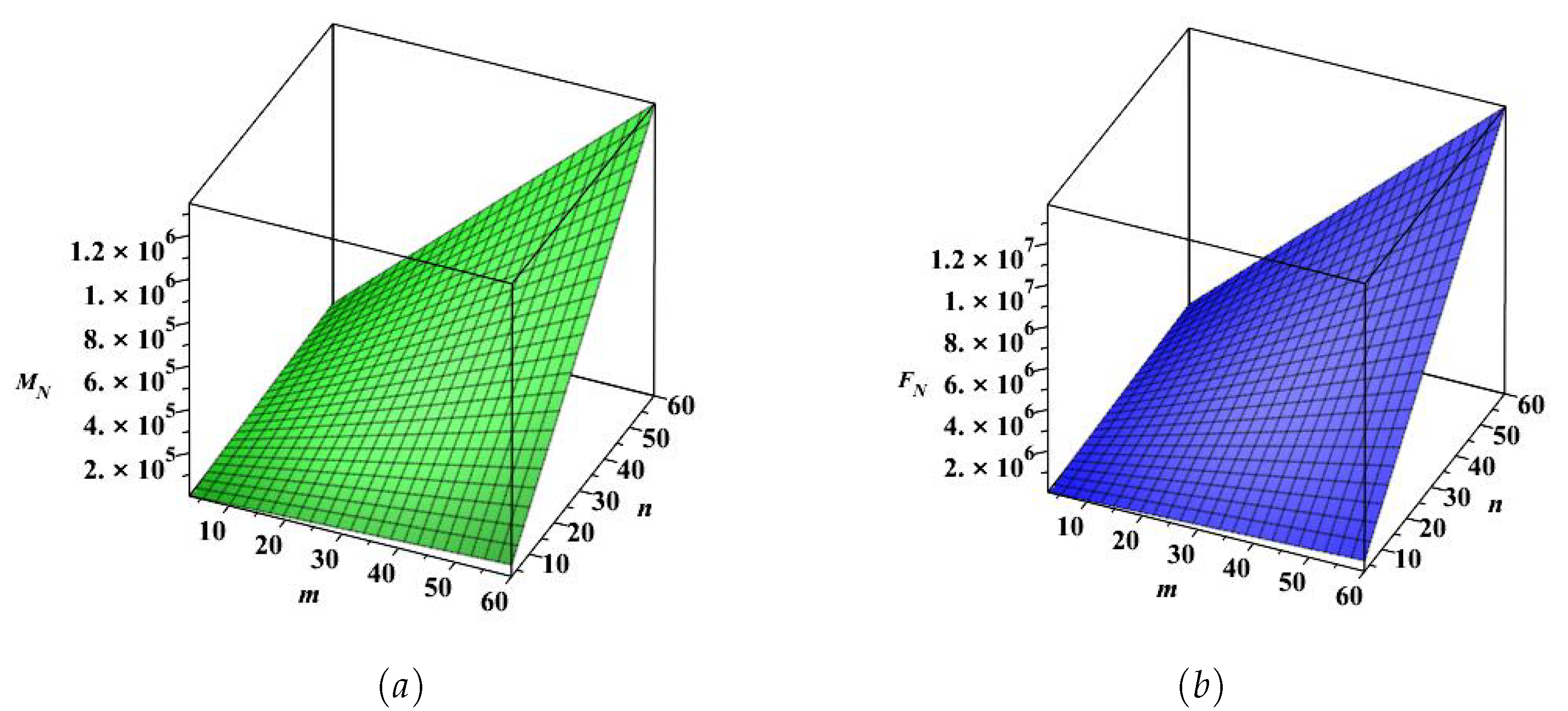
3.4. Copper (II) Oxide
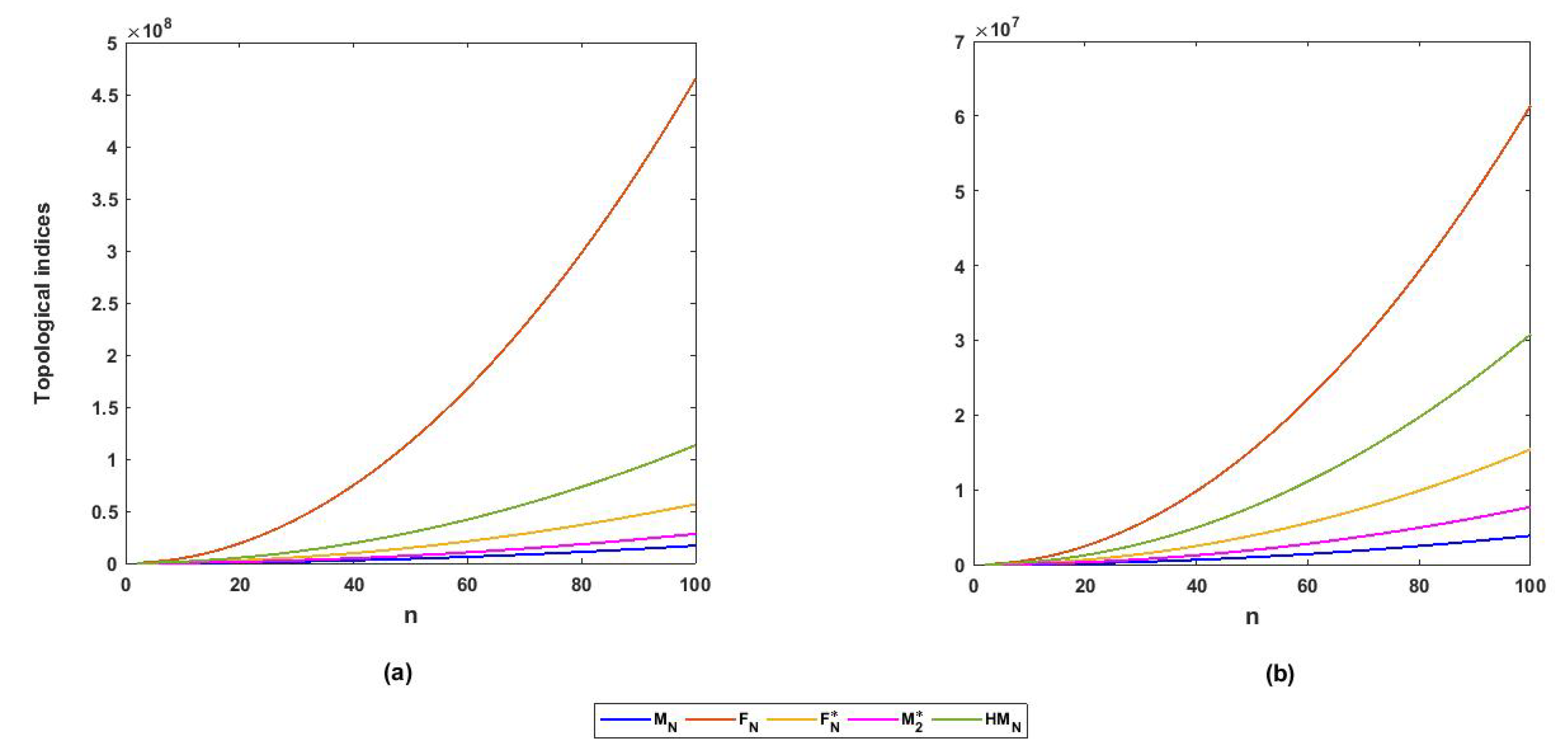
4. Remarks and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Borkow, G. Safety of Using Copper Oxide in Medical Devices and Consumer Products. Cur. Chem. Biol. 2012, 6, 86–92. [Google Scholar]
- Trinajstić, N. Chemical Graph Theory; CRC Press: Boca Raton, FL, USA, 1983. [Google Scholar]
- Gutman, I.; Polansky, O.E. Mathematical Concepts in Organic Chemistry; Springer: Berlin, Germany, 1986. [Google Scholar]
- Diudea, M.V.; Gutman, I.; Lorentz, J. Molecular Topology; Babes-Bolyai University: Cluj-Napoca, Romania, 2001. [Google Scholar]
- Wiener, H. Structural determination of the paraffin boiling points. J. Am. Chem. Soc. 1947, 69, 17–20. [Google Scholar] [CrossRef] [PubMed]
- Gutman, I.; Trinajstić, N. Graph theory and molecular orbitals. Total π-electron energy of alternate hydrocarbons. Chem. Phys. Lett. 1972, 17, 535–538. [Google Scholar] [CrossRef]
- Furtula, B.; Gutman, I. Forgotten topological index. J. Math. Chem. 2015, 53. [Google Scholar] [CrossRef]
- Shirdel, G.H.; Rezapour, H.; Sayadi, A.M. The hyper Zagreb index of graph operations. Iran. J. Math. Chem. 2013, 4, 213–220. [Google Scholar]
- Mondal, S.; De, N.; Pal, A. The Neighborhood Zagreb index of product graphs and its chemical interest. arXiv 2018, arXiv:1805.05273. [Google Scholar]
- Mondal, S.; De, N.; Pal, A. On some new neighborhood degree based indices. Acta Chem. IASI 2019. Accepted. [Google Scholar]
- Aslam, A.; Bashir, Y.; Ahmed, S.; Gao, W. On topological indices of certain dendrimer structures. Z. Naturforsch. 2017, 72, 559–566. [Google Scholar] [CrossRef]
- Imran, M.; Siddiqui, M.K.; Abunamous, A.A.E.; Adi, D.S.; Rafique, H.; Baig, A.Q. Eccentricity Based Topological Indices of an Oxide Network. Mathematics 2018, 6, 126. [Google Scholar] [CrossRef]
- De, N. On Molecular Topological Properties of TiO2 Nanotubes. J. Nanosci. 2016. [Google Scholar] [CrossRef]
- Bashir, Y.; Aslam, A.; Kamran, M.; Qureshi, M.I.; Jahangir, A.; Rafiq, M.; Bibi, N.; Muhammad, N. On Forgotten Topological Indices of Some Dendrimers Structure. Molecules 2017, 22, 867. [Google Scholar] [CrossRef]
- Akhter, S.; Imran, M.; Gao, W.; Farahani, M.R. On topological indices of honeycomb networks and graphene networks. Hacet. J. Math. Stat. 2018, 47, 19–35. [Google Scholar] [CrossRef]
- Kwun, Y.C.; Ahmad, J.; Nazeer, W.; Khalid, W.; Kang, S.M. Topological invariants of molecular graph of Graphene. JP J. Geom. Topol. 2018, 21, 95–103. [Google Scholar] [CrossRef]
- Jagadeesh, R.; Khanna, M.R.R.; Indumathi, R.S. Some results on topological indices of graphene. Nanomater. Nanotechnol. 2016, 6, 1–6. [Google Scholar]
- Akhtar, S.; Imran, M. On molecular topological properties of benzenoid structures. Can. J. Chem. 2016, 94, 687–698. [Google Scholar] [CrossRef] [Green Version]
- Shegehalli, V.S.; Kanabur, R. Computation of New Degree-Based Topological Indices of Graphene. J. Math. 2016, 2016, 4341919. [Google Scholar] [CrossRef]
- Baig, A.Q.; Imran, M.; Ali, H. On topological indices of poly oxide, poly silicate, DOX, and DSL networks. Can. J. Chem. 2015, 93, 730–739. [Google Scholar] [CrossRef]
- Javaid, M.; Rehman, M.U.; Cao, J. Topological indices of rhombus type silicate and oxide networks. Can. J. Chem. 2017, 95, 134–143. [Google Scholar] [CrossRef] [Green Version]
- Gao, W.; Baig, A.Q.; Khalid, W.; Farahani, M.R. Molecular description of copper(II) oxide. Maced. J. Chem. Chem. Eng. 2017, 36, 93–99. [Google Scholar] [Green Version]
- Gao, W.; Siddiqui, M.K. Molecular Descriptors of Nanotube, Oxide, Silicate, and Triangulene Networks. J. Chem. 2017. [Google Scholar] [CrossRef]
- Rajan, B.; William, A.; Grigorious, C.; Stephen, S. On Certain Topological Indices of Silicate, Honeycomb and Hexagonal Networks. J. Comput. Math. Sci. 2012, 3, 530–535. [Google Scholar]
- Ghorbani, M.; Hosseinzadeh, M.A. The third version of Zagreb index. Discret. Math. Algorithms Appl. 2013, 5. [Google Scholar] [CrossRef]
- Simonraj, F.; George, A. Topological Properties of few Poly Oxide, Poly Silicate, DOX and DSL Networks. Int. J. Future Comput. Commun. 2013, 2. [Google Scholar] [CrossRef]



| 6 |
| 6 |
| 4 | 4 |
| 4 | 4 |
| 2 | 4 | 4 | 10 | 2 | 1 | 6 |
| 2 | 4 | 4 | 1 | 6 |
| 6 | 4 |
| 4 | 4 |
| 6 | 6 | 8 | 2 | 8 |
| 2 | 4 | 4 | 8 |
| 4 |
| 4 | 4 | 4 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mondal, S.; De, N.; Pal, A. On Some New Neighborhood Degree-Based Indices for Some Oxide and Silicate Networks. J 2019, 2, 384-409. https://doi.org/10.3390/j2030026
Mondal S, De N, Pal A. On Some New Neighborhood Degree-Based Indices for Some Oxide and Silicate Networks. J. 2019; 2(3):384-409. https://doi.org/10.3390/j2030026
Chicago/Turabian StyleMondal, Sourav, Nilanjan De, and Anita Pal. 2019. "On Some New Neighborhood Degree-Based Indices for Some Oxide and Silicate Networks" J 2, no. 3: 384-409. https://doi.org/10.3390/j2030026
APA StyleMondal, S., De, N., & Pal, A. (2019). On Some New Neighborhood Degree-Based Indices for Some Oxide and Silicate Networks. J, 2(3), 384-409. https://doi.org/10.3390/j2030026





