Calculation of α Decay Half-Lives for Tl, Bi, and At Isotopes
Abstract
1. Introduction
2. Theoretical Framework
2.1. Deformed Relativistic Hartree–Bogoliubov Theory in Continuum
2.2. Empirical Formula for Decay Half-Lives
2.2.1. Royer Formula
2.2.2. AKRA Formula
2.2.3. Viola–Seaborg–Sobiczewski (VSS) Formula
2.2.4. Parkhomenko–Sobiczewski (SP) Formula
2.2.5. Universal Decay Law (UDL) Formula
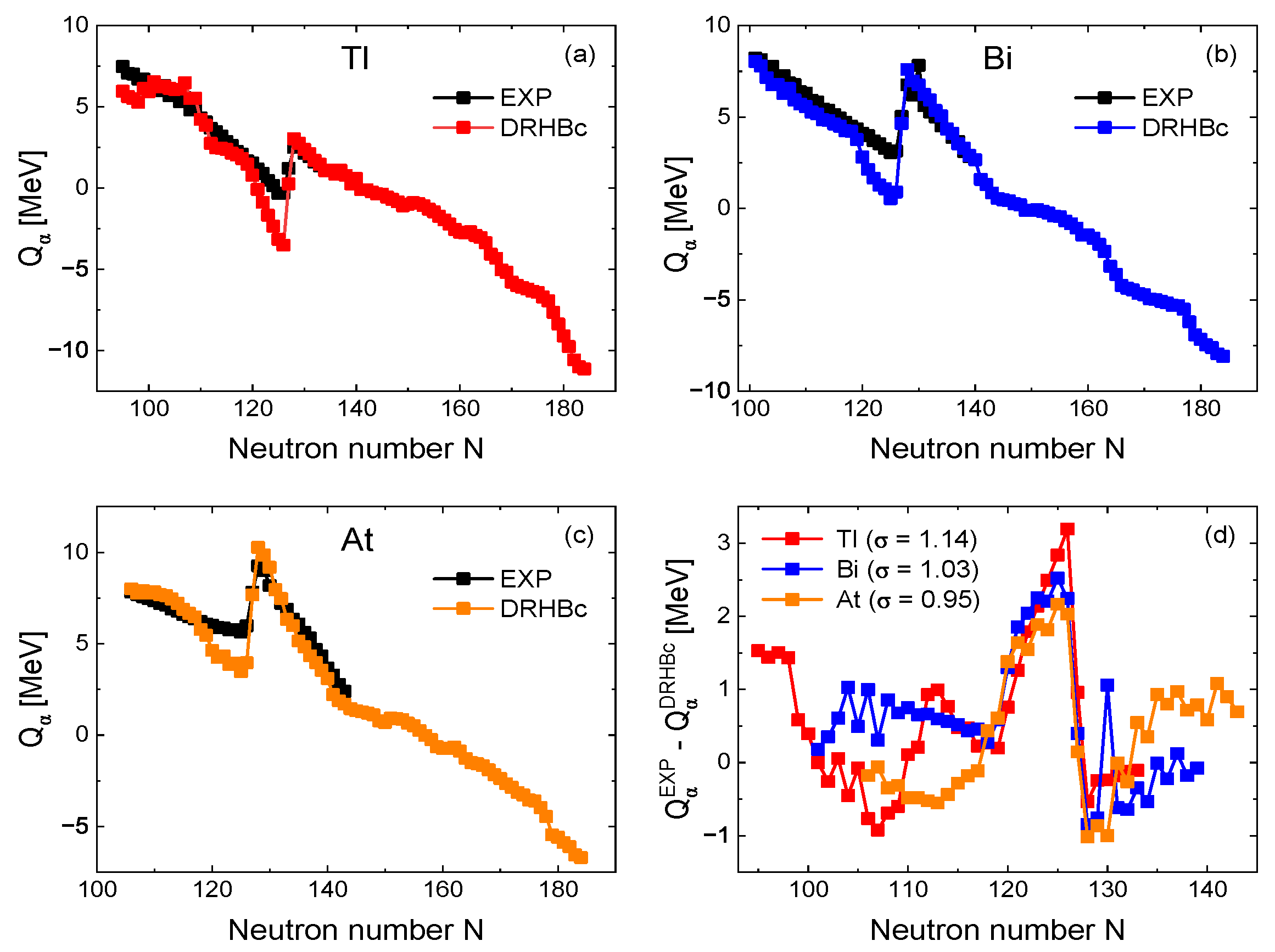
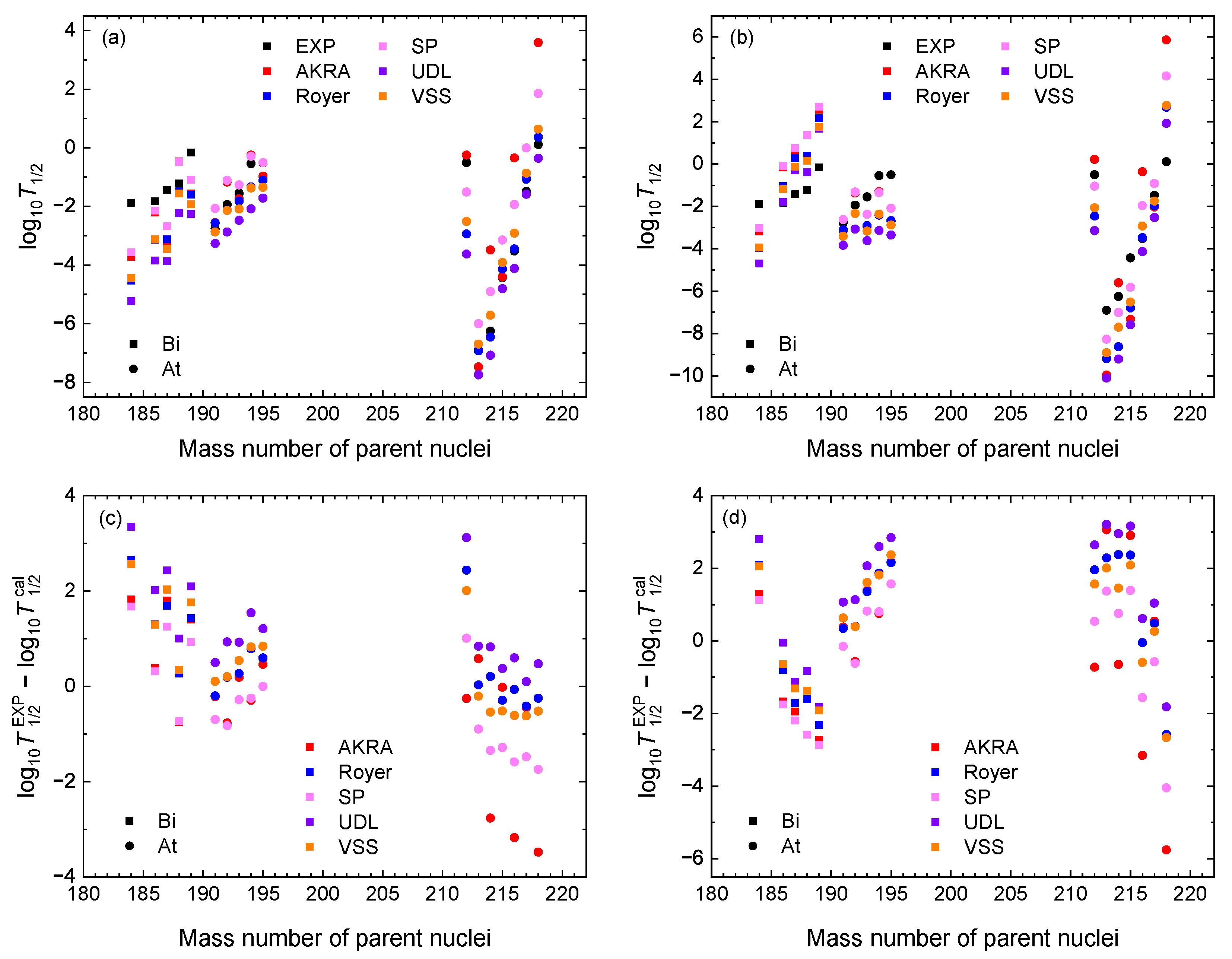
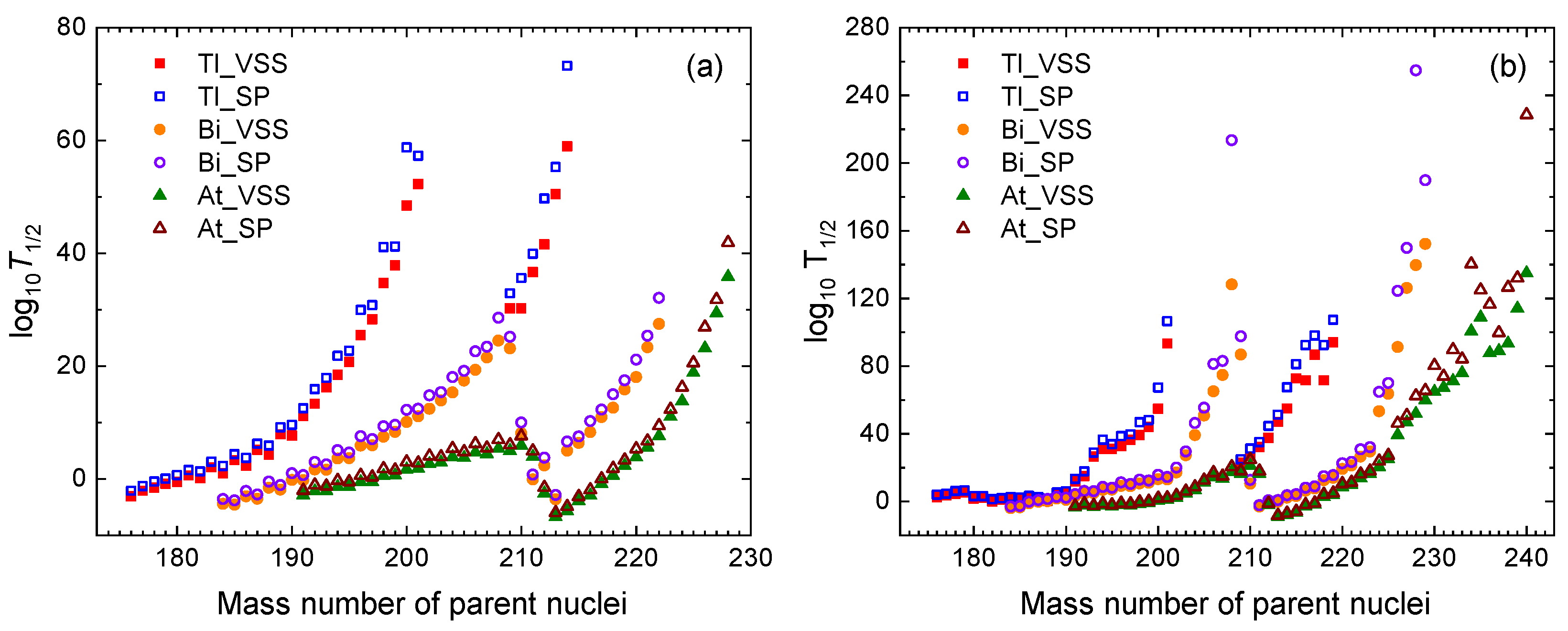
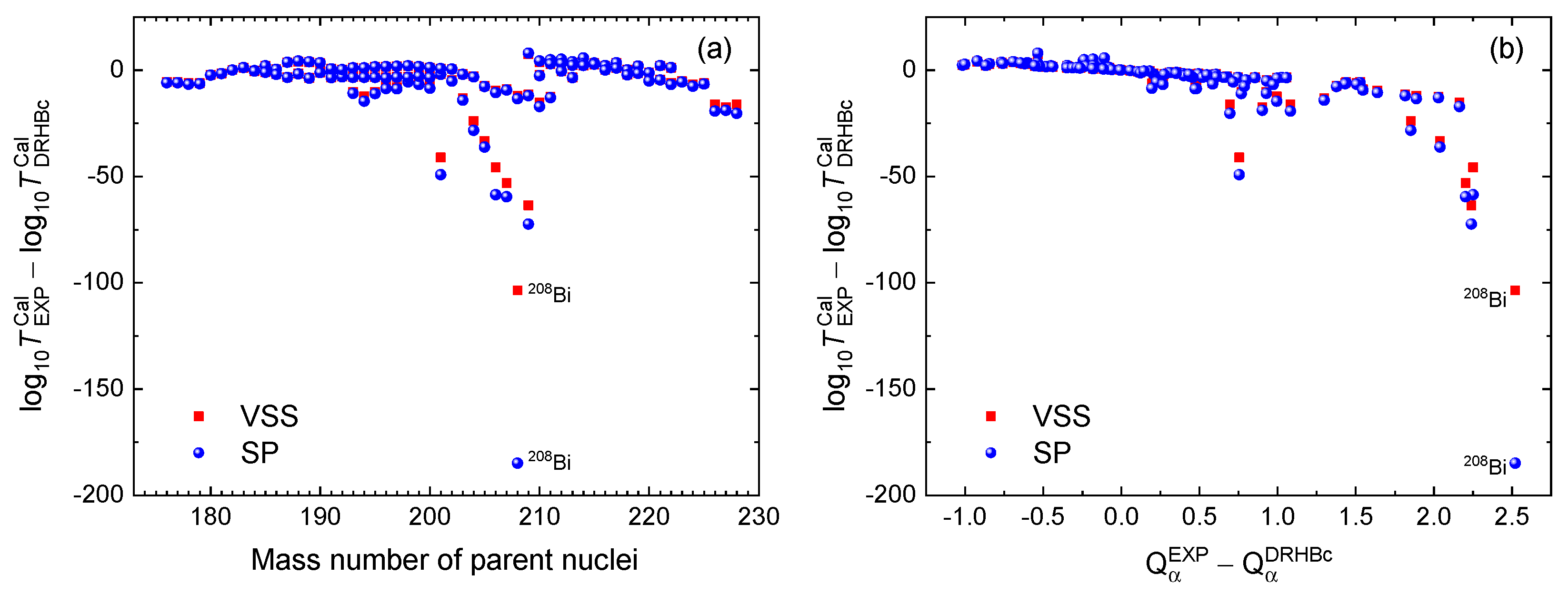
3. Results
4. Summary
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sobiczewski, A.; Pomorski, K. Description of structure and properties of superheavy nuclei. Prog. Part. Nucl. Phys. 2007, 58, 292. [Google Scholar]
- Zhang, Z.Y.; Yang, H.B.; Huang, M.H.; Gan, Z.G.; Yuan, C.X.; Qi, C.; Andreyev, A.N.; Liu, M.L.; Ma, L.; Zhang, M.M.; et al. New α-Emitting Isotope 214U and Abnormal Enhancement of α-Particle Clustering in Lightest Uranium Isotopes. Phys. Rev. Lett. 2021, 126, 152502. [Google Scholar]
- Oganessian, Y.T. Synthesis of the heaviest elements in 48Ca-induced reactions. Radiochim. Acta 2011, 99, 429. [Google Scholar]
- Basu, D.N. Role of effective interaction in nuclear disintegration processes. Phys. Lett. B 2003, 566, 90. [Google Scholar]
- Manjunatha, H.C.; Sowmya, N.; Damodara Gupta, P.S.; Sridhar, K.N.; Nagaraja, A.M.; Seenappa, L.; Alfred Cecil Raj, S. Investigation of decay modes of superheavy nuclei. Nucl. Sci. Tech. 2021, 32, 130. [Google Scholar]
- Royer, G. Alpha emission and spontaneous fission through quasi-molecular shapes. J. Phys. G 2000, 26, 1149. [Google Scholar]
- Akrawy, D.T.; Poenaru, D.N. Alpha decay calculations with a new formula. J. Phys. G 2017, 44, 105105. [Google Scholar]
- Viola, V.E., Jr.; Seaborg, G.T. Nuclear systematics of the heavy elements—II Lifetimes for alpha, beta and spontaneous fission decay. J. Inorg. Nucl. Chem. 1966, 28, 741. [Google Scholar]
- Sobiczewski, A.; Patyk, Z.; Cwiok, S. Deformed superheavy nuclei. Phys. Lett. B 1989, 224, 1. [Google Scholar]
- Parkhomenko, A.; Sobiczewski, A. Phenomenological formula for α-decay half-lives of heaviest nuclei. Acta Phys. Pol. B 2005, 36, 3095. [Google Scholar]
- Qi, C.; Xu, F.R.; Liotta, R.J.; Wyss, R. Universal Decay Law in Charged-Particle Emission and Exotic Cluster Radioactivity. Phys. Rev. Lett. 2009, 103, 072501. [Google Scholar] [CrossRef] [PubMed]
- Qi, C.; Xu, F.R.; Liotta, R.J.; Wyss, R.; Zhang, M.Y.; Asawatangtrakuldee, C.; Hu, D. Microscopic mechanism of charged-particle radioactivity and generalization of the Geiger-Nuttall law. Phys. Rev. C 2009, 80, 044326. [Google Scholar] [CrossRef]
- Zhang, K.; Cheoun, M.K.; Choi, Y.B.; Chong, P.S.; Dong, J.; Geng, L.; Ha, E.; He, X.; Heo, C.; Ho, M.C.; et al. Deformed relativistic Hartree-Bogoliubov theory in continuum with a point-coupling functional: Examples of even-even Nd isotopes. Phys. Rev. C 2020, 102, 024314. [Google Scholar] [CrossRef]
- Pan, C.; Cheoun, M.K.; Choi, Y.B.; Dong, J.; Du, X.; Fan, X.H.; Gao, W.; Geng, L.; Ha, E.; He, X.T.; et al. Deformed relativistic Hartree-Bogoliubov theory in continuum with a point-coupling functional. II. Examples of odd Nd isotopes. Phys. Rev. C 2022, 106, 014316. [Google Scholar] [CrossRef]
- Zhang, K.; Cheoun, M.K.; Choi, Y.B.; Chong, P.S.; Dong, J.; Dong, Z.; Du, X.; Geng, L.; Ha, E.; He, X.T.; et al. Nuclear mass table in deformed relativistic Hartree–Bogoliubov theory in continuum, I: Even–even nuclei. At. Data Nucl. Data Tables 2022, 144, 101488. [Google Scholar]
- Guo, P.; Cao, X.; Chen, K.; Chen, Z.; Cheoun, M.K.; Choi, Y.B.; Lam, P.C.; Deng, W.; Dong, J.; Du, P.; et al. Nuclear mass table in deformed relativistic Hartree–Bogoliubov theory in continuum, II: Even-Z nuclei. At. Data Nucl. Data Tables 2024, 158, 101661. [Google Scholar] [CrossRef]
- Sun, X.-X. Deformed two-neutron halo in 19B. Phys. Rev. C 2021, 103, 054315. [Google Scholar] [CrossRef]
- Yang, Z.H.; Kubota, Y.; Corsi, A.; Yoshida, K.; Sun, X.X.; Li, J.G.; Kimura, M.; Michel, N.; Ogata, K.; Yuan, C.X.; et al. Quasifree Neutron Knockout Reaction Reveals a Small s-Orbital Component in the Borromean Nucleus 17B. Phys. Rev. Lett. 2021, 126, 082501. [Google Scholar] [CrossRef]
- Kim, S.; Mun, M.-H.; Cheoun, M.-K.; Ha, E. Shape coexistence and neutron skin thickness of Pb isotopes by the deformed relativistic Hartree-Bogoliubov theory in continuum. Phys. Rev. C 2022, 105, 034340. [Google Scholar] [CrossRef]
- Zhang, K.Y.; Papakonstantinou, P.; Mun, M.-H.; Kim, Y.; Yan, H.; Sun, X.-X. Collapse of the N = 28 shell closure in the newly discovered 39Na nucleus and the development of deformed halos towards the neutron dripline. Phys. Rev. C 2023, 107, L041303. [Google Scholar] [CrossRef]
- Zhang, K.Y.; Yang, S.Q.; An, J.L.; Zhang, S.S.; Papakonstantinou, P.; Mun, M.H.; Kim, Y.; Yan, H. Missed prediction of the neutron halo in 37Mg. Phys. Lett. B 2023, 844, 138112. [Google Scholar] [CrossRef]
- Mun, M.-H.; Kim, S.; Cheoun, M.-K.; So, W.Y.; Choi, S.; Ha, E. Odd-even shape staggering and kink structure of charge radii of Hg isotopes by the deformed relativistic Hartree–Bogoliubov theory in continuum. Phys. Lett. B 2023, 847, 138298. [Google Scholar] [CrossRef]
- Guo, P.; Pan, C.; Zhao, Y.C.; Du, X.K.; Zhang, S.Q. Prolate-shape dominance in atomic nuclei within the deformed relativistic Hartree-Bogoliubov theory in continuum. Phys. Rev. C 2023, 108, 014319. [Google Scholar] [CrossRef]
- An, J.L.; Zhang, K.Y.; Lu, Q.; Zhong, S.Y.; Zhang, S.S. A unified description of the halo nucleus 37Mg from microscopic structure to reaction observables. Phys. Lett. B 2024, 849, 138422. [Google Scholar] [CrossRef]
- Mun, M.-H.; Ha, E.; Sagawa, H.; Colò, G.; Cheoun, M.-K. Symmetry energy from two-nucleon separation energies of Pb and Ca isotopes. Phys. Rev. C 2024, 110, 014314. [Google Scholar] [CrossRef]
- Taninah, A.; Agbemava, S.E.; Afanasjev, A.V. Covariant density functional theory input for r-process simulations in actinides and superheavy nuclei: The ground state and fission properties. Phys. Rev. C 2020, 102, 054330. [Google Scholar] [CrossRef]
- Choi, Y.-B.; Lee, C.-H.; Mun, M.-H.; Choi, S.C.; Kim, Y.M. α-decay half-lives for even-even isotopes of W to U. Phys. Rev. C 2024, 109, 054310. [Google Scholar] [CrossRef]
- Zhao, P.W.; Li, Z.P.; Yao, J.M.; Meng, J. New parametrization for the nuclear covariant energy density functional with a point-coupling interaction. Phys. Rev. C 2010, 82, 054319. [Google Scholar] [CrossRef]
- Zhou, S.G.; Meng, J.; Ring, P.; Zhao, E.G. Neutron halo in deformed nuclei. Phys. Rev. C 2010, 82, 011301. [Google Scholar] [CrossRef]
- Li, L.; Meng, J.; Ring, P.; Zhao, E.G.; Zhou, S.G. Deformed relativistic Hartree-Bogoliubov theory in continuum. Phys. Rev. C 2012, 85, 024312. [Google Scholar] [CrossRef]
- Li, L.; Meng, J.; Ring, P.; Zhao, E.G.; Zhou, S.G. Odd Systems in Deformed Relativistic Hartree Bogoliubov Theory in Continuum. Chin. Phys. Lett. 2012, 29, 042101. [Google Scholar] [CrossRef]
- Kucharek, H.; Ring, P.; Schuk, P.; Bengtsson, R.; Girod, M. Pairing properties of nuclear matter from the gogny force. Phys. Lett. B 1989, 216, 249. [Google Scholar] [CrossRef]
- Geiger, H.; Nuttall, J.M. LVII. The ranges of the α particles from various radioactive substances and a relation between range and period of transformation. Philos. Mag. Ser. 1911, 22, 613. [Google Scholar] [CrossRef]
- Deng, J.-G.; Zhang, H.-F.; Royer, G. Improved empirical formula for α-decay half-lives. Phys. Rev. C 2020, 101, 034307. [Google Scholar] [CrossRef]
- Rashidpour, Z.; Naderi, D. An empirical formula for the alpha decay half-lives. Int. J. Mod. Phys. E 2023, 32, 2350028. [Google Scholar] [CrossRef]
- Luo, S.; Xu, Y.-Y.; Zhu, D.-X.; He, B.; Chu, P.-C.; Li, X.-H. Improved Geiger–Nuttall law for α-decay half-lives of heavy and superheavy nuclei. Eur. Phys. J. A 2022, 58, 244. [Google Scholar] [CrossRef]
- Wang, M.; Huang, W.J.; Kondev, F.G.; Audi, G.; Naimi, S. The AME 2020 atomic mass evaluation (II). Tables, graphs and references. Chin. Phys. C 2021, 45, 030003. [Google Scholar] [CrossRef]
- NNDC (National Nuclear Data Center). Brookhaven National Laboratory. Available online: https://www.nndc.bnl.gov/nudat2/ (accessed on 2 April 2025).
- Gurvitz, S.A.; Kalbermann, G. Decay width and the shift of a quasistationary state. Phys. Rev. Lett. 1987, 59, 262. [Google Scholar] [CrossRef]
- Xu, C.; Ren, Z. New deformed model of α-decay half-lives with a microscopic potential. Phys. Rev. C 2006, 73, 041301. [Google Scholar] [CrossRef]
| Transition | EXP | AKRA | Royer | SP | UDL | VSS | |
|---|---|---|---|---|---|---|---|
| 184Bi →180Tl | 8.22 | −1.89 | −3.71 | −4.53 | −3.56 | −5.23 | −4.45 |
| 186Bi →182Tl | 7.76 | −1.83 | −2.21 | −3.13 | −2.15 | −3.85 | −3.12 |
| 187Bi →183Tl | 7.76 | −1.43 | −3.23 | −3.12 | −2.68 | −3.87 | −3.46 |
| 188Bi →184Tl | 7.26 | −1.22 | −0.47 | −1.49 | −0.49 | −2.23 | −1.57 |
| 189Bi →185Tl | 7.27 | −0.16 | −1.56 | −1.60 | −1.10 | −2.26 | −1.92 |
| 191At →187Bi | 7.82 | −2.77 | −2.55 | −2.57 | −2.07 | −3.27 | −2.87 |
| 192At →188Bi | 7.70 | −1.94 | −1.17 | −2.13 | −1.12 | −2.87 | −2.14 |
| 193At →189Bi | 7.57 | −1.55 | −1.74 | −1.82 | −1.27 | −2.47 | −2.09 |
| 194At →190Bi | 7.45 | −0.54 | −0.25 | −1.33 | −0.29 | −2.09 | −1.37 |
| 195At →191Bi | 7.34 | −0.51 | −0.97 | −1.11 | −0.50 | −1.72 | −1.35 |
| 212At →208Bi | 7.82 | −0.50 | −0.25 | −2.94 | −1.51 | −3.62 | −2.51 |
| 213At →209Bi | 9.25 | −6.90 | −7.48 | −6.93 | −6.00 | −7.75 | −6.70 |
| 214At →210Bi | 8.99 | −6.25 | −3.49 | −6.46 | −4.91 | −7.08 | −5.71 |
| 215At →211Bi | 8.18 | −4.43 | −4.41 | −4.14 | −3.15 | −4.81 | −3.91 |
| 216At →212Bi | 7.95 | −3.52 | −0.35 | −3.45 | −1.94 | −4.12 | −2.91 |
| 217At →213Bi | 7.20 | −1.49 | −1.04 | −1.07 | −0.01 | −1.58 | −0.87 |
| 218At →214Bi | 6.88 | 0.11 | 3.59 | 0.36 | 1.85 | −0.36 | 0.63 |
| standard deviation | 1.55 | 1.11 | 1.09 | 1.61 | 1.16 | ||
| Transition | EXP | AKRA | Royer | SP | UDL | VSS | |
|---|---|---|---|---|---|---|---|
| 184Bi →180Tl | 8.04 | −1.89 | −3.18 | −3.98 | −3.02 | −4.69 | −3.94 |
| 186Bi →182Tl | 7.15 | −1.83 | −0.16 | −1.03 | −0.08 | −1.79 | −1.19 |
| 187Bi →183Tl | 6.75 | −1.43 | 0.52 | 0.28 | 0.76 | −0.31 | −0.13 |
| 188Bi →184Tl | 6.77 | −1.22 | 1.36 | 0.38 | 1.36 | −0.39 | 0.15 |
| 189Bi →185Tl | 6.27 | −0.16 | 2.57 | 2.15 | 2.70 | 1.66 | 1.75 |
| 191At →187Bi | 8.00 | −2.77 | −3.15 | −3.11 | −2.62 | −3.84 | −3.40 |
| 192At →188Bi | 7.76 | −1.94 | −1.37 | −2.33 | −1.32 | −3.08 | −2.33 |
| 193At →189Bi | 7.92 | −1.55 | −2.94 | −2.91 | −2.37 | −3.62 | −3.16 |
| 194At →190Bi | 7.77 | −0.54 | −1.30 | −2.41 | −1.35 | −3.14 | −2.36 |
| 195At →191Bi | 7.83 | −0.51 | −2.69 | −2.67 | −2.08 | −3.35 | −2.88 |
| 212At →208Bi | 7.67 | −0.50 | 0.22 | −2.46 | −1.04 | −3.15 | −2.07 |
| 213At →209Bi | 10.27 | −6.90 | −9.97 | −9.19 | −8.27 | −10.11 | −8.91 |
| 214At →210Bi | 9.86 | −6.25 | −5.61 | −8.63 | −7.01 | −9.21 | −7.71 |
| 215At →211Bi | 9.18 | −4.43 | −7.34 | −6.79 | −5.82 | −7.59 | −6.52 |
| 216At →212Bi | 7.96 | −3.52 | −0.37 | −3.48 | −1.96 | −4.14 | −2.93 |
| 217At →213Bi | 7.46 | −1.49 | −2.03 | −1.97 | −0.91 | −2.52 | −1.75 |
| 218At →214Bi | 6.33 | 0.11 | 5.86 | 2.68 | 4.15 | 1.93 | 2.77 |
| standard deviation | 2.33 | 1.77 | 1.75 | 2.11 | 1.62 | ||
| standard deviation (+) | 6.07 | 6.62 | 5.59 | 7.38 | 6.24 | ||
| standard deviation (−) | 11.12 | 9.81 | 10.98 | 9.29 | 9.26 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mun, M.-H.; Heo, K.; Cheoun, M.-K. Calculation of α Decay Half-Lives for Tl, Bi, and At Isotopes. Particles 2025, 8, 42. https://doi.org/10.3390/particles8020042
Mun M-H, Heo K, Cheoun M-K. Calculation of α Decay Half-Lives for Tl, Bi, and At Isotopes. Particles. 2025; 8(2):42. https://doi.org/10.3390/particles8020042
Chicago/Turabian StyleMun, Myeong-Hwan, Kyoungsu Heo, and Myung-Ki Cheoun. 2025. "Calculation of α Decay Half-Lives for Tl, Bi, and At Isotopes" Particles 8, no. 2: 42. https://doi.org/10.3390/particles8020042
APA StyleMun, M.-H., Heo, K., & Cheoun, M.-K. (2025). Calculation of α Decay Half-Lives for Tl, Bi, and At Isotopes. Particles, 8(2), 42. https://doi.org/10.3390/particles8020042








