Masses and Quadrupole Deformations of Even-Z Nuclei Within a Triaxial Relativistic Hartree–Bogoliubov Model
Abstract
1. Introduction
2. Theoretical Framework and Numerical Details
3. Results and Discussion
4. Summary and Outlook
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Thoennessen, M. The Discovery of Isotopes; Springer: Cham, Switzerland, 2016. [Google Scholar]
- Wang, M. Nuclear Mass Measurement and Evaluation. Nucl. Phys. Rev. 2017, 34, 380–386. [Google Scholar]
- Wang, M.; Zhang, Y.H.; Zhou, X.H. Nuclear mass measurements. Sci. Sin. Phys. Mech. Astron. 2020, 50, 052006. [Google Scholar]
- Wang, M.; Huang, W.J.; Kondev, F.G.; Audi, G.; Naimi, S. The AME 2020 atomic mass evaluation (II). Tables, graphs and references. Chin. Phys. C 2021, 45, 030003. [Google Scholar] [CrossRef]
- Erler, J.; Birge, N.; Kortelainen, M.; Nazarewicz, W.; Olsen, E.; Perhac, A.M.; Stoitsov, M. The limits of the nuclear landscape. Nature 2012, 486, 509–512. [Google Scholar] [CrossRef]
- Xia, X.W.; Lim, Y.; Zhao, P.W.; Liang, H.Z.; Qu, X.Y.; Chen, Y.; Liu, H.; Zhang, L.F.; Zhang, S.Q.; Kim, Y.; et al. The limits of the nuclear landscape explored by the relativistic continuum Hartree—Bogoliubov theory. At. Data Nucl. Data Tables 2018, 121–122, 1–215. [Google Scholar] [CrossRef]
- Burbidge, E.M.; Burbidge, G.R.; Fowler, W.A.; Hoyle, F. Synthesis of the elements in stars. Rev. Mod. Phys. 1957, 29, 547. [Google Scholar] [CrossRef]
- Möller, P.; Myers, W.; Swiatecki, W.; Treiner, J. Nuclear mass formula with a finite-range droplet model and a folded-Yukawa single-particle potential. At. Data Nucl. Data Tables 1988, 39, 225–233. [Google Scholar] [CrossRef]
- Moller, P.; Nix, J.; Myers, W.; Swiatecki, W. Nuclear Ground-State Masses and Deformations. At. Data Nucl. Data Tables 1995, 59, 185–381. [Google Scholar] [CrossRef]
- Möller, P.; Sierk, A.; Ichikawa, T.; Sagawa, H. Nuclear ground-state masses and deformations: FRDM(2012). At. Data Nucl. Data Tables 2016, 109–110, 1–204. [Google Scholar] [CrossRef]
- Haustein, P.E. An overview of the 1986–1987 atomic mass predictions. At. Data Nucl. Data Tables 1988, 39, 185–200. [Google Scholar] [CrossRef]
- Wang, N.; Liu, M.; Wu, X.Z.; Meng, J. Surface diffuseness correction in global mass formula. Phys. Lett. B 2014, 734, 215–219. [Google Scholar] [CrossRef]
- Bender, M.; Heenen, P.H.; Reinhard, P.G. Self-consistent mean-field models for nuclear structure. Rev. Mod. Phys. 2003, 75, 121. [Google Scholar] [CrossRef]
- Samyn, M.; Goriely, S.; Heenen, P.H.; Pearson, J.; Tondeur, F. A hartree–fock–bogoliubov mass formula. Nucl. Phys. A 2002, 700, 142–156. [Google Scholar] [CrossRef]
- Stoitsov, M.; Dobaczewski, J.; Nazarewicz, W.; Pittel, S.; Dean, D. Systematic study of deformed nuclei at the drip lines and beyond. Phys. Rev. C—Nucl. Phys. 2003, 68, 054312. [Google Scholar] [CrossRef]
- Goriely, S.; Chamel, N.; Pearson, J. Skyrme-Hartree-Fock-Bogoliubov Nuclear Mass Formulas: Crossing the 0.6 MeV Accuracy Threshold with Microscopically Deduced Pairing. Phys. Rev. Lett. 2009, 102, 152503. [Google Scholar] [CrossRef]
- Goriely, S.; Chamel, N.; Pearson, J. Further explorations of Skyrme-Hartree-Fock-Bogoliubov mass formulas. XIII. The 2012 atomic mass evaluation and the symmetry coefficient. Phys. Rev. C—Nucl. Phys. 2013, 88, 024308. [Google Scholar] [CrossRef]
- Goriely, S.; Chamel, N.; Pearson, J. Hartree-Fock-Bogoliubov nuclear mass model with 0.50 MeV accuracy based on standard forms of Skyrme and pairing functionals. Phys. Rev. C—Nucl. Phys. 2013, 88, 061302. [Google Scholar] [CrossRef]
- Hilaire, S.; Girod, M. Large-scale mean-field calculations from proton to neutron drip lines using the D1S Gogny force. Eur. Phys. J. A 2007, 33, 237–241. [Google Scholar] [CrossRef]
- Goriely, S.; Hilaire, S.; Girod, M.; Peru, S. The Gogny-Hartree-Fock-Bogoliubov nuclear-mass model. Phys. Rev. Lett. 2009, 102, 242501. [Google Scholar] [CrossRef]
- Delaroche, J.P.; Girod, M.; Libert, J.; Goutte, H.; Hilaire, S.; Péru, S.; Pillet, N.; Bertsch, G. Structure of even-even nuclei using a mapped collective Hamiltonian and the D1S Gogny interaction. Phys. Rev. C—Nucl. Phys. 2010, 81, 014303. [Google Scholar] [CrossRef]
- Ring, P. Relativistic mean field theory in finite nuclei. Prog. Part. Nucl. Phys. 1996, 37, 193–263. [Google Scholar] [CrossRef]
- Vretenar, D.; Afanasjev, A.; Lalazissis, G.; Ring, P. Relativistic Hartree–Bogoliubov theory: Static and dynamic aspects of exotic nuclear structure. Phys. Rep. 2005, 409, 101–259. [Google Scholar] [CrossRef]
- Meng, J.; Toki, H.; Zhou, S.G.; Zhang, S.Q.; Long, W.H.; Geng, L.S. Relativistic continuum Hartree Bogoliubov theory for ground-state properties of exotic nuclei. Prog. Part. Nucl. Phys. 2006, 57, 470–563. [Google Scholar] [CrossRef]
- Nikšić, T.; Vretenar, D.; Ring, P. Relativistic nuclear energy density functionals: Mean-field and beyond. Prog. Part. Nucl. Phys. 2011, 66, 519–548. [Google Scholar] [CrossRef]
- Meng, J.; Peng, J.; Zhang, S.Q.; Zhao, P.W. Progress on tilted axis cranking covariant density functional theory for nuclear magnetic and antimagnetic rotation. Front. Phys. 2013, 8, 55–79. [Google Scholar] [CrossRef]
- Meng, J.; Zhou, S.G. Halos in medium-heavy and heavy nuclei with covariant density functional theory in continuum. J. Phys. G Nucl. Part. Phys. 2015, 42, 093101. [Google Scholar] [CrossRef]
- Zhou, S.G. Multidimensionally constrained covariant density functional theories—nuclear shapes and potential energy surfaces. Phys. Scr. 2016, 91, 063008. [Google Scholar] [CrossRef]
- Meng, J. Relativistic Density Functional for Nuclear Structure; World Scientific: Singapore, 2016; Volume 10. [Google Scholar]
- Shen, S.H.; Liang, H.Z.; Long, W.H.; Meng, J.; Ring, P. Towards an ab initio covariant density functional theory for nuclear structure. Prog. Part. Nucl. Phys. 2019, 109, 103713. [Google Scholar] [CrossRef]
- Zhao, P.W.; Li, Z.P.; Yao, J.M.; Meng, J. New parametrization for the nuclear covariant energy density functional with a point-coupling interaction. Phys. Rev. C 2010, 82, 054319. [Google Scholar] [CrossRef]
- Lu, K.Q.; Li, Z.X.; Li, Z.P.; Yao, J.M.; Meng, J. Global study of beyond-mean-field correlation energies in covariant energy density functional theory using a collective Hamiltonian method. Phys. Rev. C 2015, 91, 027304. [Google Scholar] [CrossRef]
- Yang, Y.L.; Wang, Y.K.; Zhao, P.W.; Li, Z.P. Nuclear landscape in a mapped collective Hamiltonian from covariant density functional theory. Phys. Rev. C 2021, 104, 054312. [Google Scholar] [CrossRef]
- Agbemava, S.; Afanasjev, A.; Ray, D.; Ring, P. Global performance of covariant energy density functionals: Ground state observables of even-even nuclei and the estimate of theoretical uncertainties. Phys. Rev. C 2014, 89, 054320. [Google Scholar] [CrossRef]
- Geng, L.S.; Toki, H.; Meng, J. Masses, Deformations and Charge Radii—Nuclear Ground-State Properties in the Relativistic Mean Field Model. Prog. Theor. Phys. 2005, 113, 785–800. [Google Scholar] [CrossRef]
- Yang, Y.L.; Zhao, P.W.; Li, Z.P. Shape and multiple shape coexistence of nuclei within covariant density functional theory. Phys. Rev. C 2023, 107, 024308. [Google Scholar] [CrossRef]
- Wang, Y.K. Yrast band of 109Ag described by tilted axis cranking covariant density functional theory with a separable pairing force. Phys. Rev. C 2017, 96, 054324. [Google Scholar] [CrossRef]
- Wang, Y.K. Magnetic rotations in 198Pb and 199Pb within covariant density functional theory with pairing correlations. Phy. Rev. C 2018, 97, 064321. [Google Scholar] [CrossRef]
- Zhao, P.W.; Wang, Y.K.; Chen, Q.B. Microscopic resolution of the nuclear chiral conundrum with crossing twin bands in 106Ag. Phys. Rev. C 2019, 99, 054319. [Google Scholar] [CrossRef]
- Wang, Y.K.; Zhao, P.W.; Meng, J. Relativistic configuration-interaction density functional theory: Nonaxial effects on nuclear ββ decay. Sci. Bull. 2024, 69, 2017–2020. [Google Scholar] [CrossRef] [PubMed]
- Guo, P.; Cao, X.J.; Chen, K.M.; Chen, Z.H.; Cheoun, M.K.; Choi, Y.B.; Lam, P.C.; Deng, W.M.; Dong, J.M.; Du, P.X.; et al. Nuclear mass table in deformed relativistic Hartree–Bogoliubov theory in continuum, II: Even-Z nuclei. At. Data Nucl. Data Tables 2024, 158, 101661. [Google Scholar] [CrossRef]
- Meng, J. Relativistic continuum Hartree-Bogoliubov theory with both zero range and finite range Gogny force and their application. Nucl. Phys. A 1998, 635, 3–42. [Google Scholar] [CrossRef]
- Kucharek, H.; Ring, P. Relativistic field theory of superfluidity in nuclei. Z. Für Phys. A Hadron. Nucl. 1991, 339, 23–35. [Google Scholar] [CrossRef]
- Gonzalez-Llarena, T.; Egido, J.; Lalazissis, G.; Ring, P. Relativistic Hartree-Bogoliubov calculations with finite range pairing forces. Phys. Lett. B 1996, 379, 13–19. [Google Scholar] [CrossRef]
- Serra, M.; Ring, P. Relativistic Hartree-Bogoliubov theory for finite nuclei. Phys. Rev. C 2002, 65, 064324. [Google Scholar] [CrossRef]
- Ring, P.; Schuck, P. The Many Body Nuclear Problem; Springer: Berlin/Heidelberg, Germany, 1980. [Google Scholar]
- Tian, Y.; Ma, Z.Y.; Ring, P. A finite range pairing force for density functional theory in superfluid nuclei. Phys. Lett. B 2009, 676, 44–50. [Google Scholar] [CrossRef]
- Pan, C.; Zhang, K.Y.; Zhang, S.Q. Nuclear magnetism in the deformed halo nucleus 31Ne. Phys. Lett. B 2024, 855, 138792. [Google Scholar] [CrossRef]
- Nikšić, T.; Paar, N.; Vretenar, D.; Ring, P. DIRHB—A relativistic self-consistent mean-field framework for atomic nuclei. Comput. Phys. Commun. 2014, 185, 1808–1821. [Google Scholar] [CrossRef]
- Zhao, Q.; Ren, Z.X.; Zhao, P.W.; Meng, J. Covariant density functional theory with localized exchange terms. Phys. Rev. C 2022, 106, 034315. [Google Scholar] [CrossRef]
- Heyde, K.; Wood, J.L. Shape coexistence in atomic nuclei. Rev. Mod. Phys. 2011, 83, 1467–1521. [Google Scholar] [CrossRef]
- Quan, S.; Liu, W.P.; Li, Z.P.; Smith, M.S. Microscopic core-quasiparticle coupling model for spectroscopy of odd-mass nuclei. Phys. Rev. C 2017, 96, 054309. [Google Scholar] [CrossRef]
- Pan, C.; Cheoun, M.K.; Choi, Y.B.; Dong, J.M.; Du, X.K.; Fan, X.H.; Gao, W.; Geng, L.S.; Ha, E.; He, X.T.; et al. Deformed relativistic Hartree-Bogoliubov theory in continuum with a point-coupling functional. II. Examples of odd Nd isotopes. Phys. Rev. C 2022, 106, 014316. [Google Scholar] [CrossRef]
- Li, Y.; Zhou, E.F.; Yao, J.M. Benchmarking rotational correction energies in odd-mass nuclei. arXiv 2024, arXiv:2409.12402. [Google Scholar]

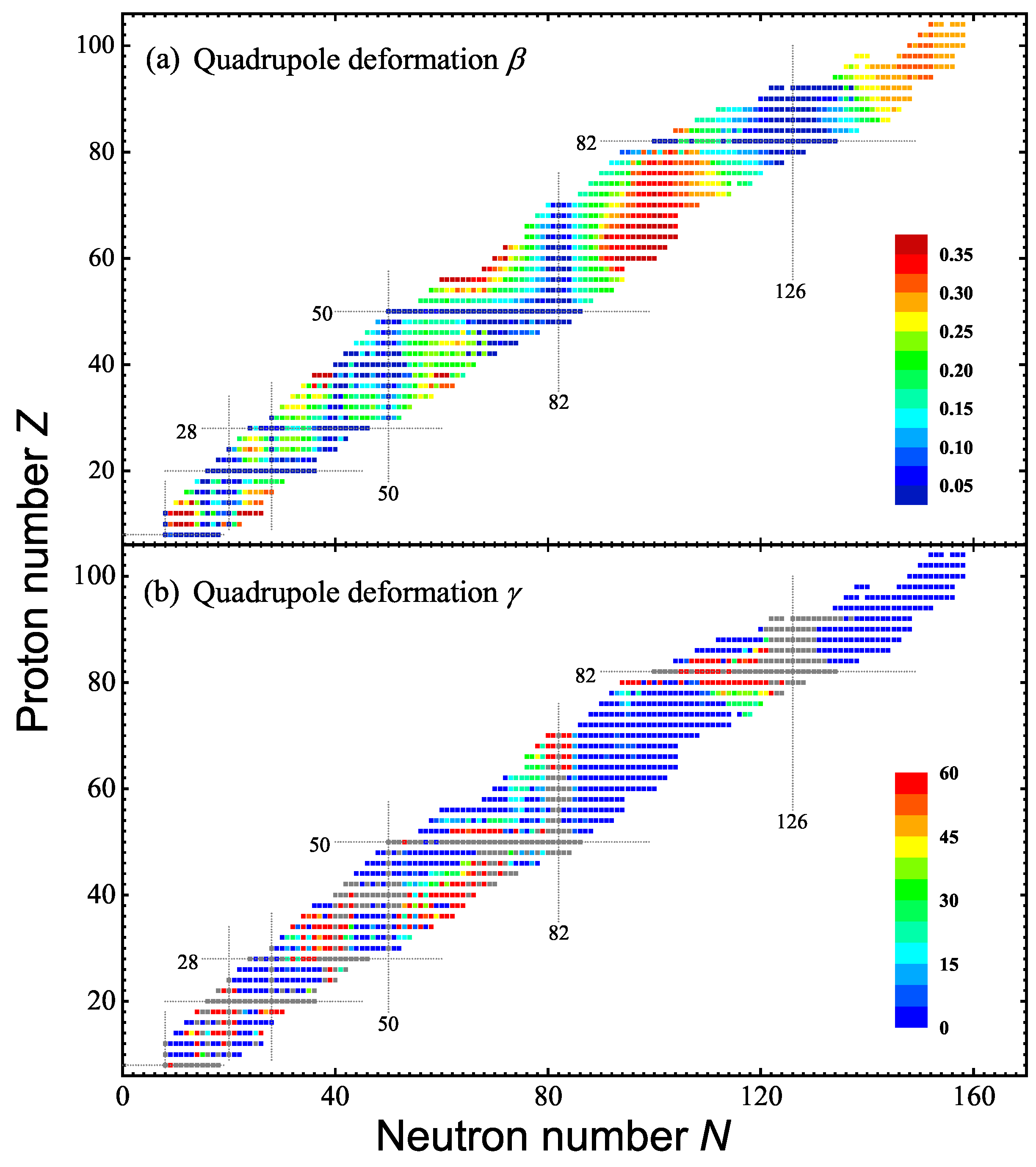
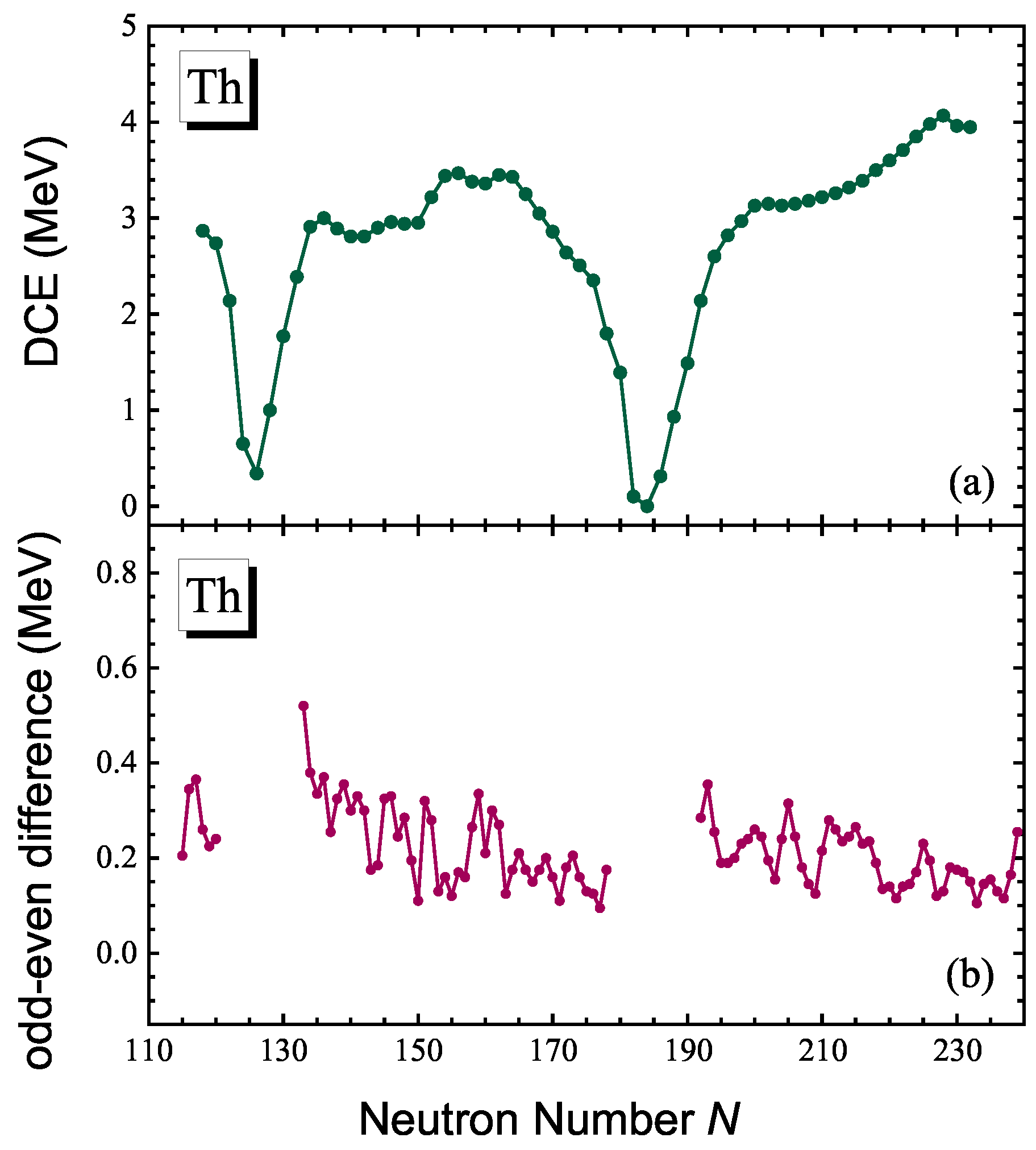

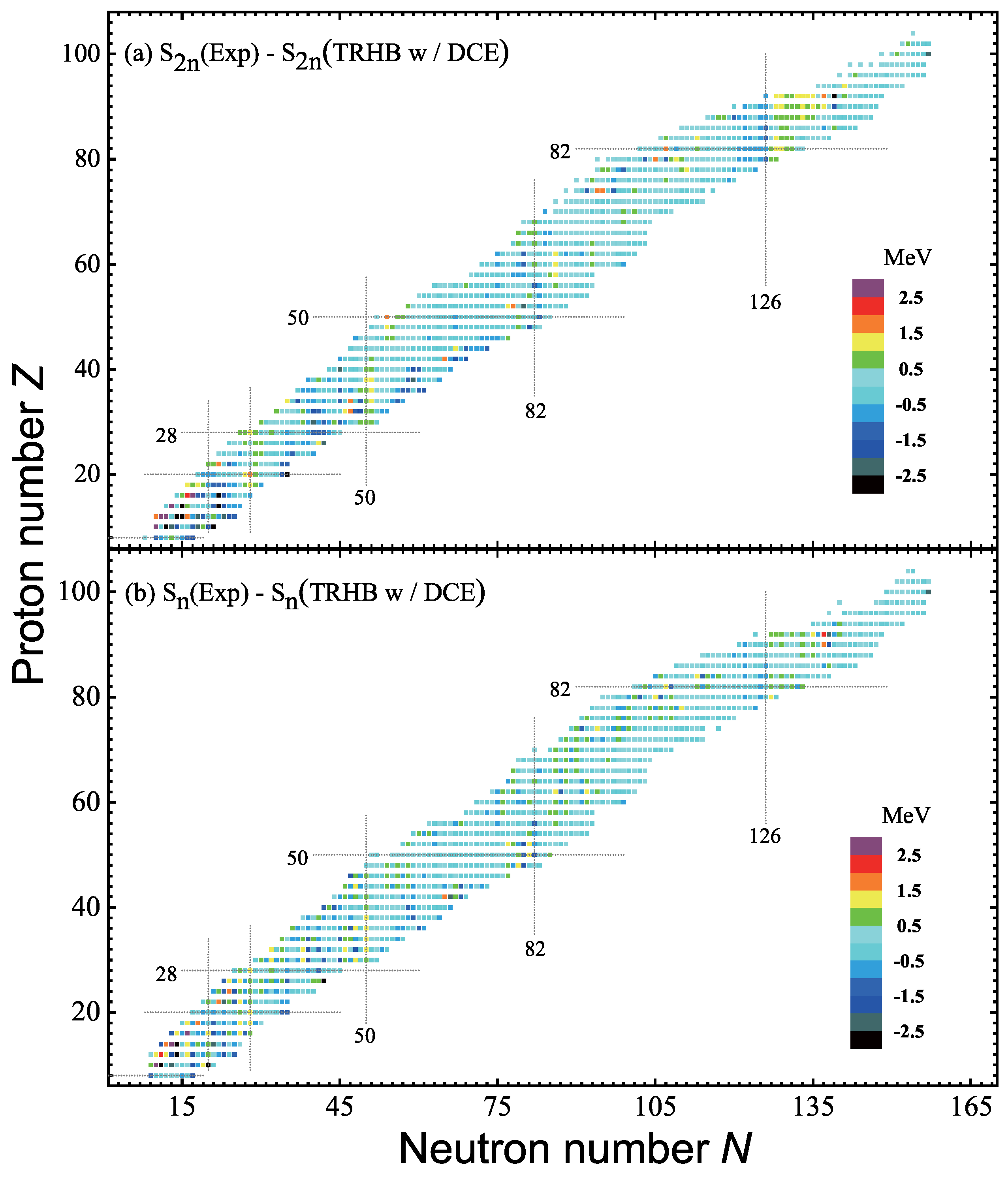
| DFTs | Date Number | |||
|---|---|---|---|---|
| TRHB w/o DCE | 2.56 | 0.98 | 0.75 | 1223 |
| TRHB w DCE | 1.36 | 0.75 | 0.65 | 1223 |
| TRHB w DCE* | 1.30 | 0.75 | 0.63 | 1223 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Q.; Li, Z. Masses and Quadrupole Deformations of Even-Z Nuclei Within a Triaxial Relativistic Hartree–Bogoliubov Model. Particles 2025, 8, 57. https://doi.org/10.3390/particles8020057
Zhou Q, Li Z. Masses and Quadrupole Deformations of Even-Z Nuclei Within a Triaxial Relativistic Hartree–Bogoliubov Model. Particles. 2025; 8(2):57. https://doi.org/10.3390/particles8020057
Chicago/Turabian StyleZhou, Qin, and Zhipan Li. 2025. "Masses and Quadrupole Deformations of Even-Z Nuclei Within a Triaxial Relativistic Hartree–Bogoliubov Model" Particles 8, no. 2: 57. https://doi.org/10.3390/particles8020057
APA StyleZhou, Q., & Li, Z. (2025). Masses and Quadrupole Deformations of Even-Z Nuclei Within a Triaxial Relativistic Hartree–Bogoliubov Model. Particles, 8(2), 57. https://doi.org/10.3390/particles8020057






