Development of a Combined Horizontal and Vertical Correcting Magnet for Siam Photon Source II
Abstract
:1. Introduction
1.1. Global Orbit Feedback System
1.2. Corrector Magnets for SOFB and FOFB
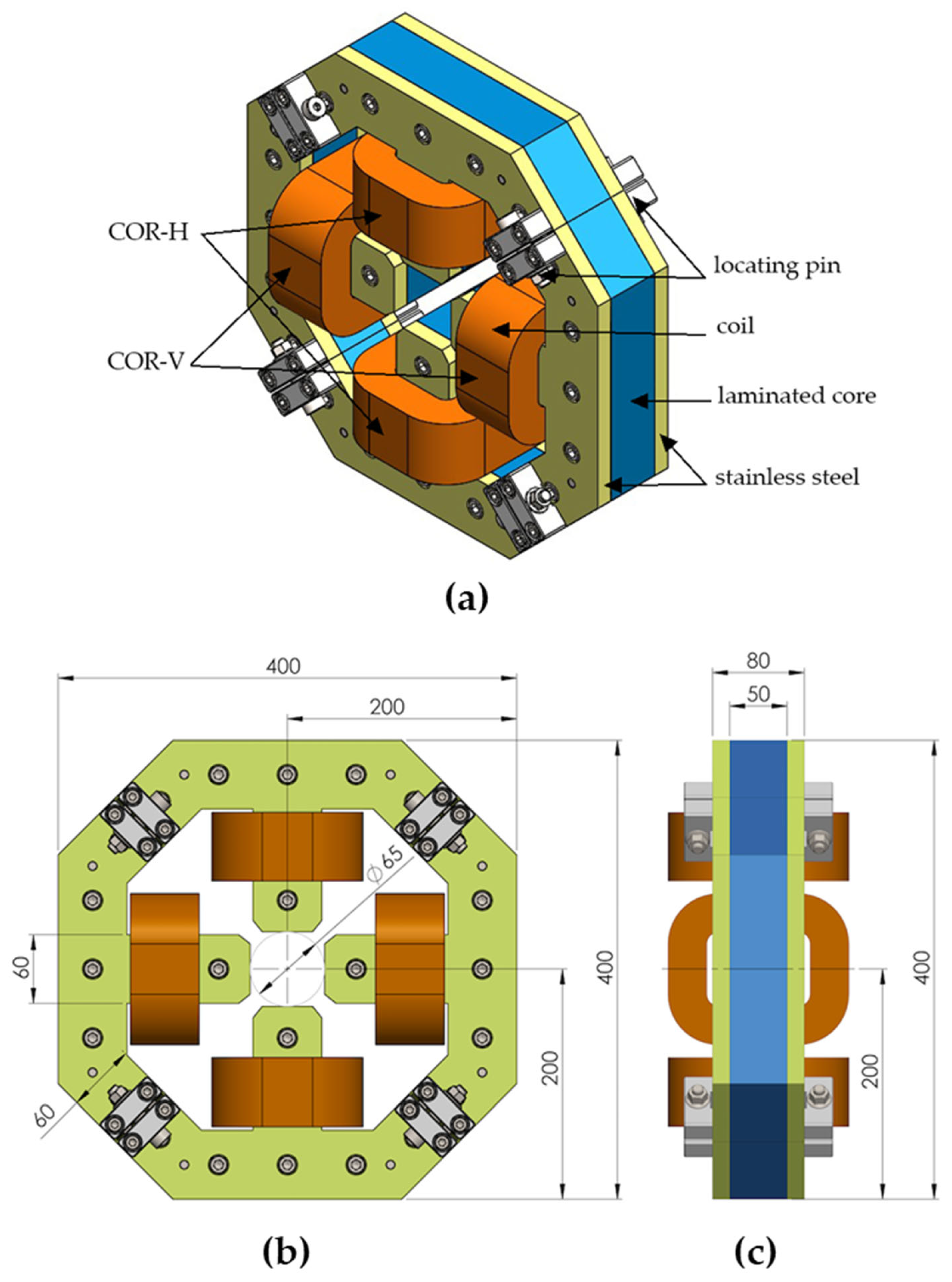
2. Combined Horizontal and Vertical Correcting Magnet Design
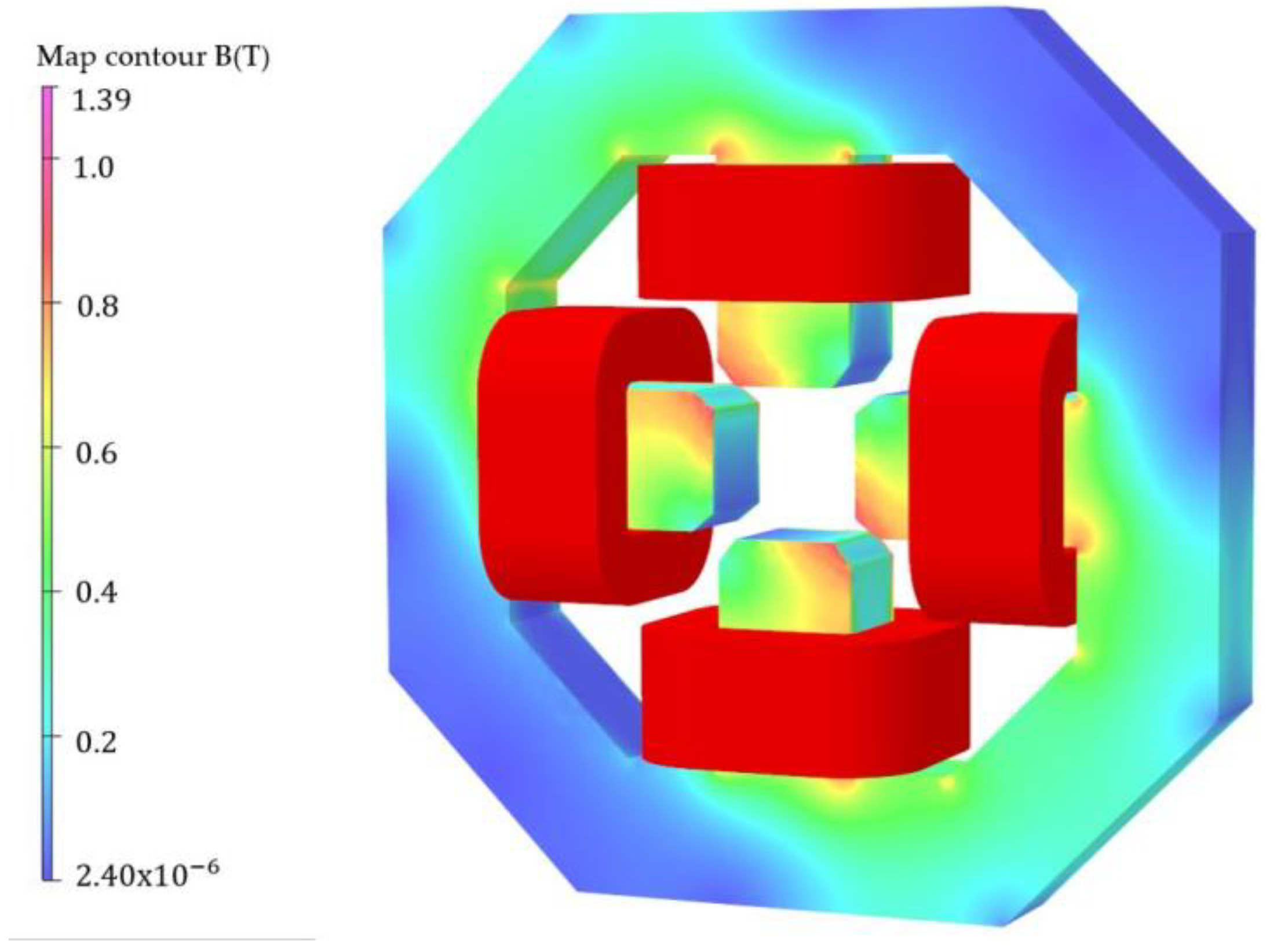
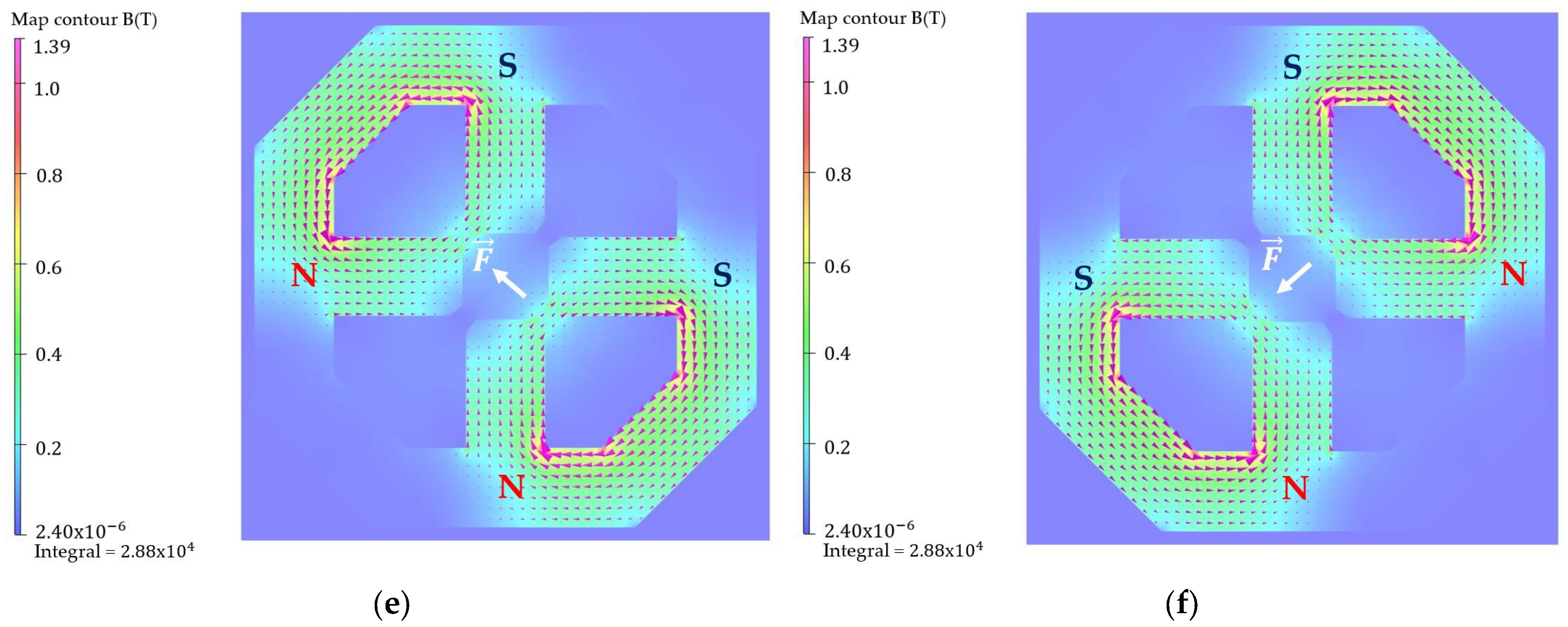
3. Magnetic Field Design
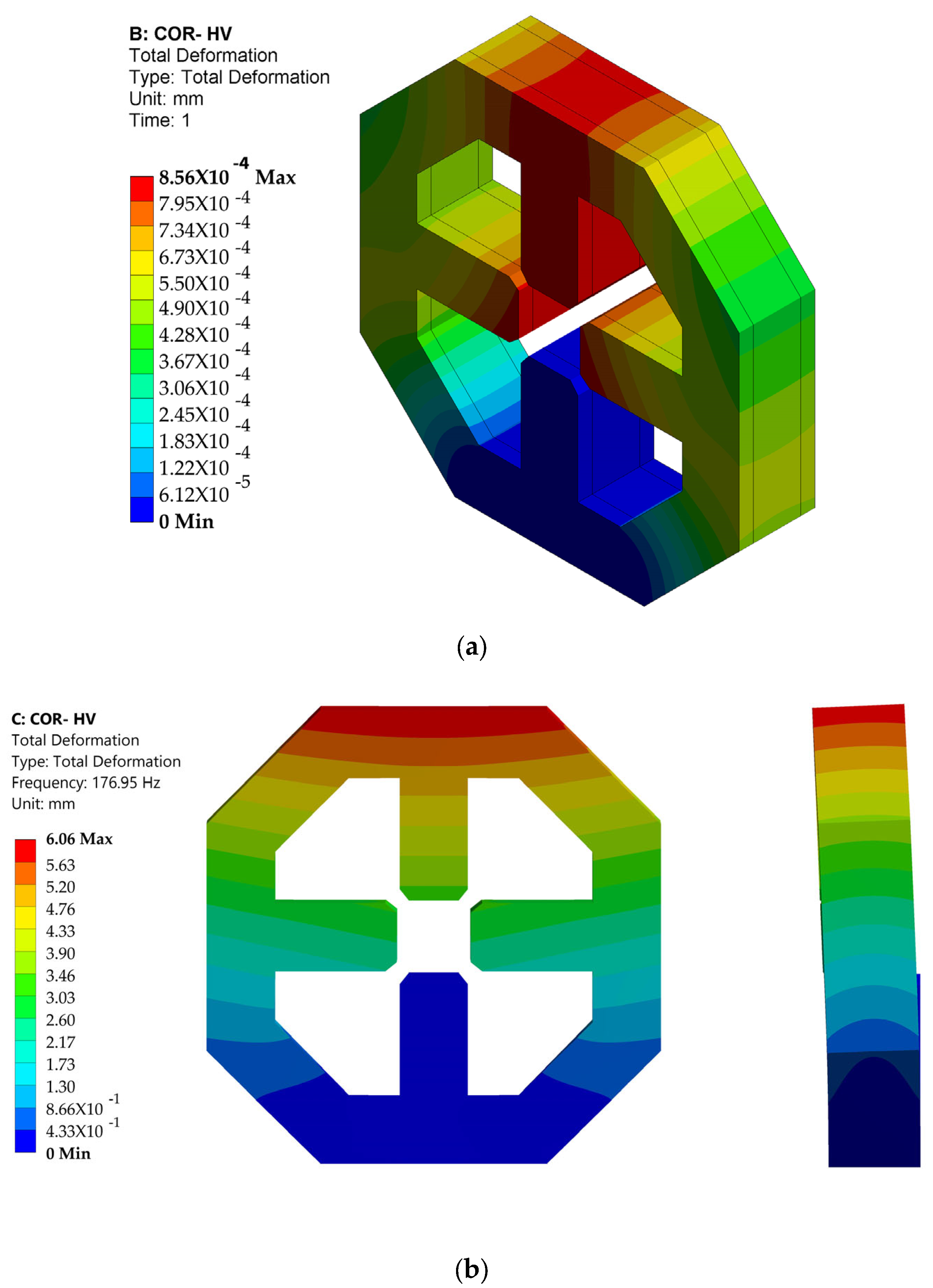
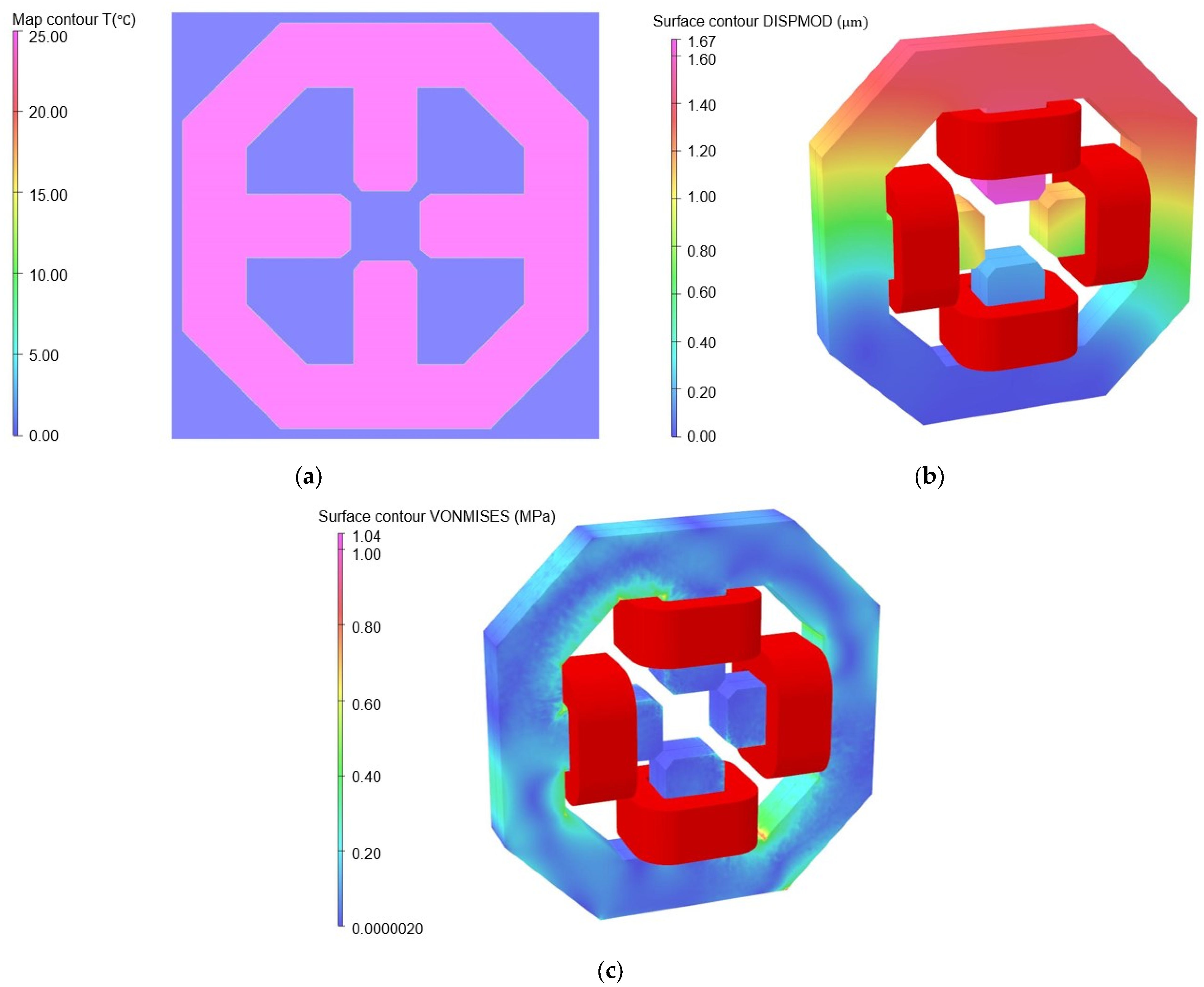
4. Mechanical Design
5. Manufacturing
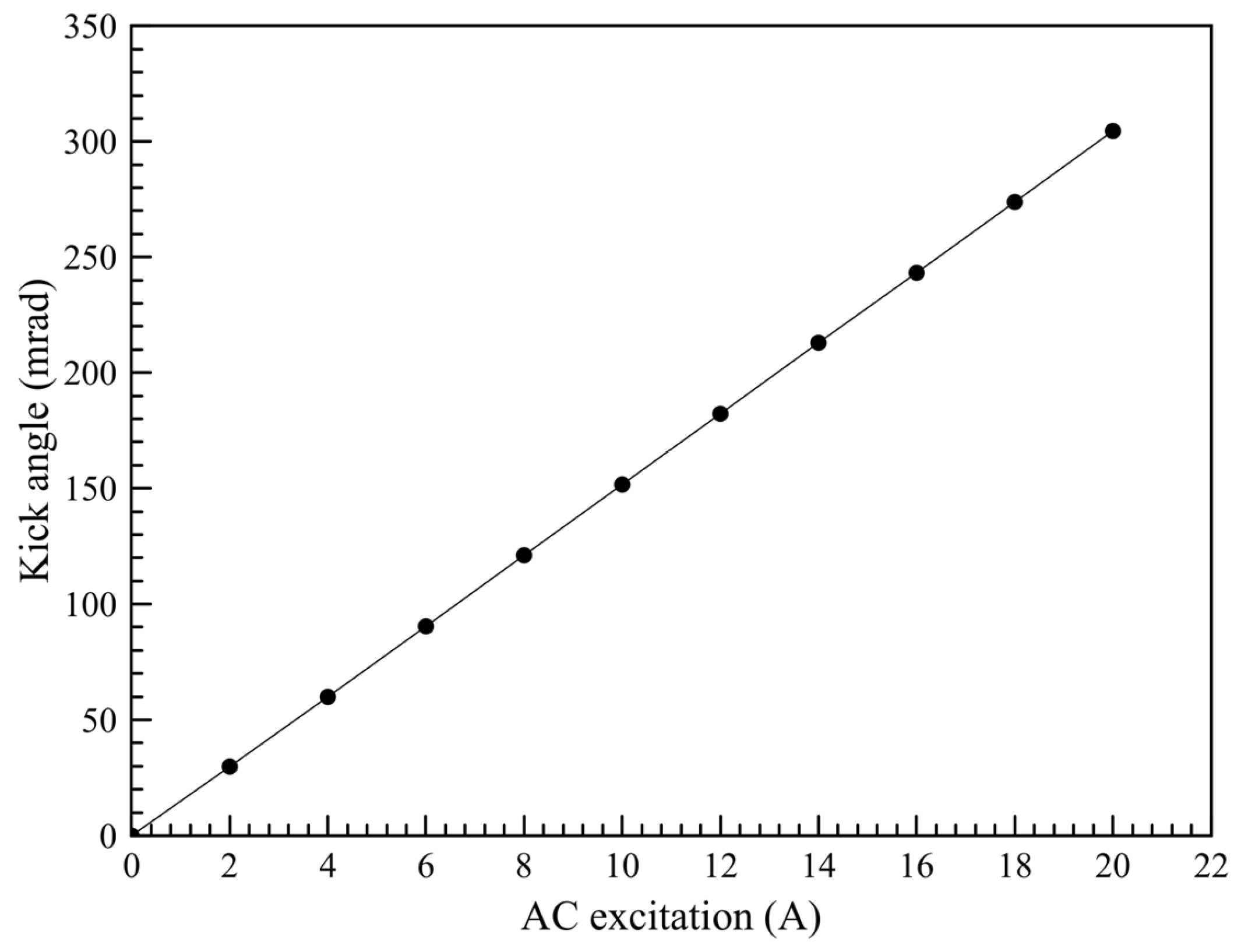
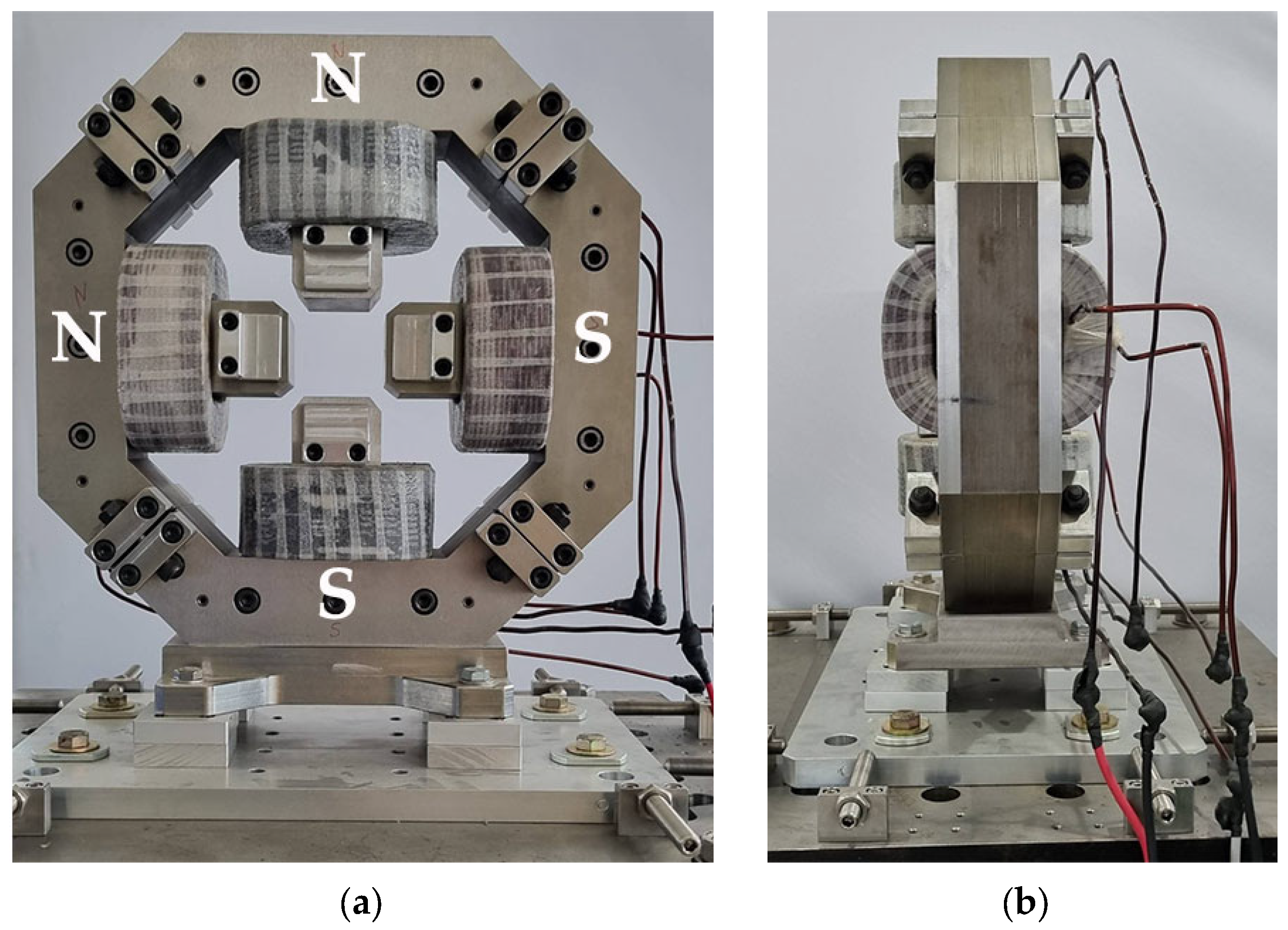
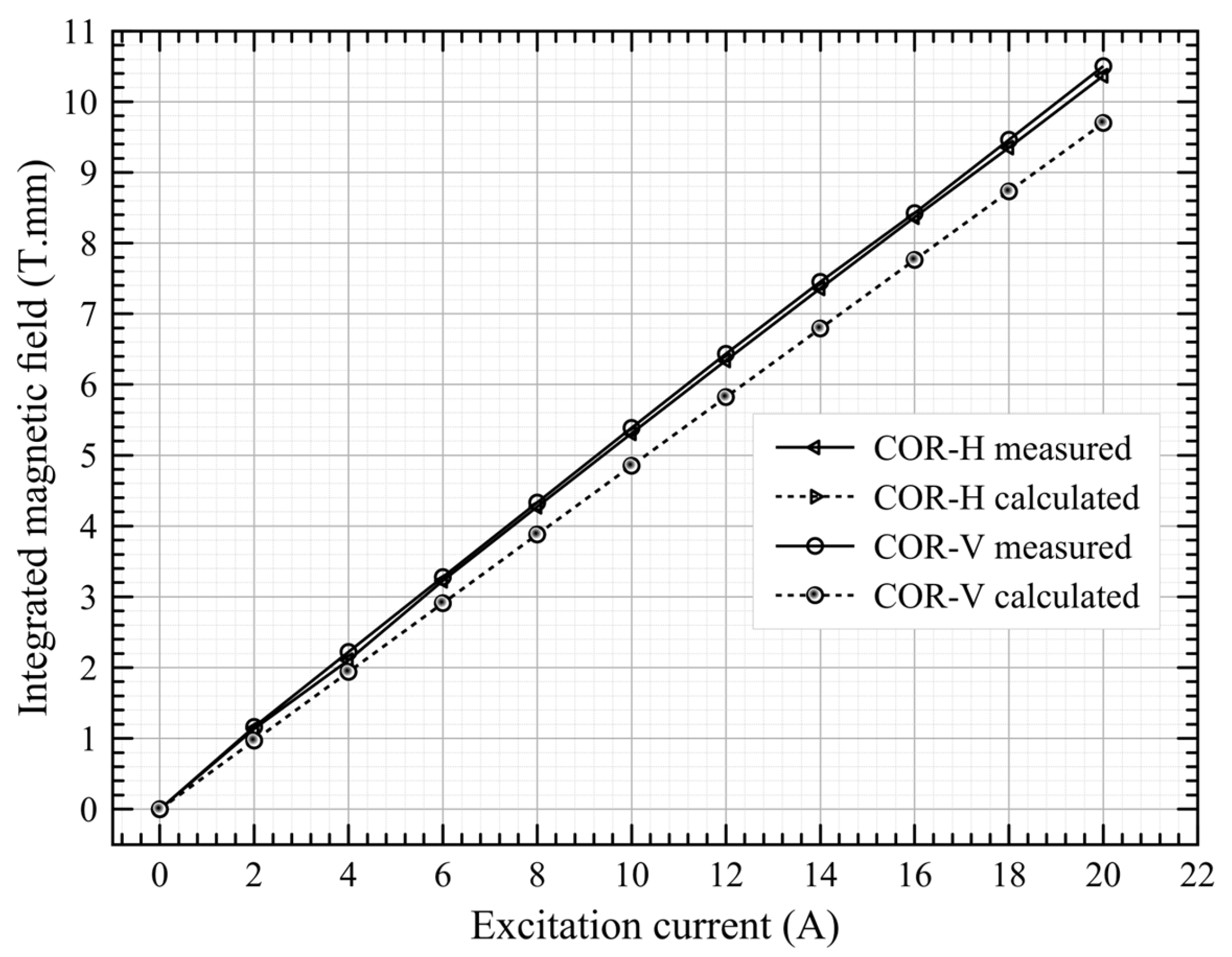
6. Magnetic Field Measurement
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Klysubun, P.; Pulampong, T.; Sudmuang, P. Design and Optimization of SPS-II storage ring. In Proceedings of the 13th International Particle Accelerator Conference, Copenhagen, Denmark, 14–19 May 2017; pp. 2773–2775, ISBN 978-3-95450-182-3. [Google Scholar]
- Wiedemann, H. Particle Accelerator Physics, 4th ed.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 140, 230, 494–499. ISBN 978-3-540-49043-2. [Google Scholar]
- Hubert, N.; Cassinari, L.; Denard, J.-C.; Nadji, A.; Nadolski, L. Global Orbit Feedback Systems down to DC Using Fast and Slow Correctors. In Proceedings of the 9th European Workshop on Beam Diagnostics and Instrumentation for Particle Accelerators, Basel, Switzerland, 25–27 May 2009; pp. 27–31. [Google Scholar]
- Hubert, N.; Cassinari, L.; Denard, J.-C.; Leclercq, N.; Nadji, A.; Nadolshi, L.; Pedeau, D. The SOLEIL BPM and Orbit Feedback Systems. In Proceedings of the 8th European Workshop on Beam Diagnostics and Instrumentation for Particle Accelerators, Venice, Italy, 20–23 May 2007; Abstract Number TUPC20. pp. 189–191. [Google Scholar]
- Schilcher, T.; Boge, M.; Keil, B.; Schlott, V. Commissioning of the Fast Orbit Feedback at SLS. In Proceedings of the 2003 Particle Accelerator Conference, Portland, OR, USA, 12–16 May 2003; ISBN 0-7803-7738-9. [Google Scholar]
- Boge, M.; Keil, B.; Krempasky, J.; Schilcher, T.; Schlott, V. Orbit Stability at the SLS; PSI Scientific and Technical Report; Paul Scherrer Institut: Villigen, Switzerland, 2003; Volume 4, pp. 11–13. [Google Scholar]
- Plouviez, E.; Uberto, F. The Orbit Correction Scheme of the new EBS of the ESRF. In Proceedings of the International Beam Instrumentation Conference IBIC 2016, Barcelona, Spain, 11–15 September 2016; pp. 51–54, ISBN 978-3-95450-177-9. [Google Scholar]
- Tanaka, H.; Aoyagi, H.; Daté, S.; Fukami, K.; Fukui, T.; Kudo, T.; Kumagai, N.; Matsui, S.; Nakatani, T.; Nakazato, T.; et al. Beam Orbit Stabilization at the Spring-8 Storage Ring. In Proceedings of the 7th International Workshop on Accelerator Alignment 2002, Nishi-Harima, Japan, 11–14 November 2002; pp. 1–8. [Google Scholar]
- Kongtawong, S.; Tian, Y.; Yu, L.H.; Yang, X.; Wang, G.; Ha, K.; Shaftan, T. Numerical Simulation of NSLS-II Fast Orbit Feedback System. Nucl. Instrum. Methods Phys. Res. Sect. A 2021, 997, 165175. [Google Scholar] [CrossRef]
- Brañas, B.; Castellanos, J.; Oliver, C.; Campmany, J.; Fernández, F.; García, M.; Kirpitchev, I.; Marcos, J.; Massana, V.; Mendez, P.; et al. Design and manufacturing of the combined quadrupole and corrector magnets for the LIPAc accelerator high energy beam transport line. Nucl. Fusion 2022, 62, 086024. [Google Scholar] [CrossRef]
- Klute, J.; Duhme, H.T.; Balewski, K.; Tiessen, H.; Wierzcholek, F. The Petra III Fast Orbit Feedback System. In Proceedings of the 10th European Workshop on Beam Diagnostics and Instrumentation for Particle Accelerators, Hamburg, Germany, 16–18 May 2011; pp. 221–223. [Google Scholar]
- Giachero, A.F.; Bruno, G.B.M.; Russo, L.M.; Tavares, D.O. Fast Orbit Corrector Power Supply in MTCA.4 Form Factor for Sirius Light Source. In Proceedings of the 12th International Particle Accelerator Conference, Campinas, Brazil, 24–28 May 2021; JACoW Publishing: Geneva, Switzerland, 2021. [Google Scholar] [CrossRef]
- Kallakut, P.; Carwardine, J.; Brill, A.; Sereno, N. Closed Loop Modeling of the APS-U Orbit Feedback System. In Proceedings of the North American Particle Accelerator Conference 2019, Lansing, MI, USA, 2–6 September 2019; JACoW Publishing: Geneva, Switzerland, 2019. [Google Scholar] [CrossRef]
- Tsoupas, N.; Berg, J.S.; Brooks, S.; Jain, A.; M’eot, F.; Mahler, G.; Trabocchi, S.; Trbojevic, D.; Tuozzolo, J. The Dipole Corrector Magnets for the FFAG Beam Line of the CBETA Accelerator; Brookhaven National Laboratory: Upton, NY, USA, 2019. [Google Scholar] [CrossRef]
- Yu, M.; Velev, G.; Harding, D. Dipole Corrector Magnets for the LBNE Beam Line. In Proceedings of the 2011 Particle Accelerator Conference, New York, NY, USA, 28 March–1 April 2011. [Google Scholar]
- Kashikhin, V.S.; Carson, J.A.; Harding, D.J.; Lackey, J.R.; Makarov, A.; Pellico, W.; Prebys, E.J. A New Correction Magnet Package for the Fermilab Booster Synchrotron. In Proceedings of the 2005 Particle Accelerator Conference, Knoxville, TN, USA, 16–20 May 2005; IEEE: Piscataway, NJ, USA, 2005. [Google Scholar] [CrossRef]
- Lonza, M.; Bulfone, D.; Forchi, V.; Gaio, G.; Pivetta, L. A Fast Orbit Feedback for the ELETTRA Storage Ring. In Proceedings of the 2007 International Conference on Accelerator and Large Experimental Physics Control Systems, Knoxville, TN, USA, 15–19 October 2007. [Google Scholar]
- Steier, C.; Biocca, A.; Domning, E.; Jacobson, S. Commissioning Results of the Fast Orbit Feedback at the ALS. In Proceedings of the 2003 Particle Accelerator Conference, Portland, OR, USA, 12–16 May 2003; IEEE: Piscataway, NJ, USA, 2004; pp. 3374–3376. [Google Scholar] [CrossRef]
- Boge, M.; Dehler, M.; Schilcher, T.; Schlott, V.; Ursic, R. Fast Closed Orbit Control in the SLS Storage Ring. In Proceedings of the 1999 Particle Accelerator Conference, New York, NY, USA, 27 March–2 April 1999; pp. 1129–1131. [Google Scholar] [CrossRef]
- Corbett, W.; Hettel, R.; Akre, R.; Bellomo, P.; Boyce, R.; Cadapan, L.; Cassel, R.; Choi, B.; Dell’Orco, D.; Evans, I.; et al. Spear 3 Upgrade Project: A Status Report. In Proceedings of the 2001 Particle Accelerator Conference, Chicago, IL, USA, 18–22 June 2001; pp. 2674–2676, ISBN 1-4244-0917-9. [Google Scholar]
- Smith, S.L.; Hill, S.L. Daresbury SRS Positional Feedback Systems. In Proceedings of the 4th European Workshop on Beam Diagnostics and Instrumentation for Particle Accelerator, Chester, UK, 16–18 May 1999; CT05. pp. 64–66. [Google Scholar]
- Brunelle, P.; Besson, J.-C.; Cassinari, L.; Cousin, J.-M.; Darpentigny, J.; Flynn, G.; Girault, F.; Lamarre, J.-F.; Nadji, A.; Rieul, B.; et al. News on Beam Dynamics at Super-Aco. In Proceedings of the 1999 Particle Accelerator Conference, New York, NY, USA, 27 March–2 April 1999; IEEE: Piscataway, NJ, USA, 1999. [Google Scholar] [CrossRef]
- Otter, A.J.; Reeve, P.A. Combined AC Corrector Magnets. In Proceedings of the 1993 Particle Accelerator Conference, Washington, DC, USA, 17–20 May 1993; IEEE: Piscataway, NJ, USA, 2002. [Google Scholar] [CrossRef]
- Nadolski, L.S.; Besson, J.-C.; Bouvet, F.; Brunelle, P.; Cassinari, L.; Denard, J.-C.; Filhol, J.-M.; Hubert, N.; Lamarre, J.-F.; Loulergue, A.; et al. Orbit Stability Status and Improvement at SOLEIL. In Proceedings of the 11th European Particle Accelerator Conference, Genoa, Italy, 23–27 June 2008. [Google Scholar]
- Opera-3d 18R2: Reference Manual; Dassault Systèmes UK Ltd.: Kidlington, UK, 2018; pp. 1–918.
- SOLIDWORKS Premium 2021 SP5.1 Online Help; Dassault Systèmes SOLIDWORKS Corp.: Waltham, MA, USA, 2021.
- Nunes, S.A.; Chadebec, O.; Kuo-Peng, P.; Dular, P.; Cucco, B. Contribution on the Source Field Calculation through the Biot-Savart Equation Using Curvilinear Elements and an Adaptive Process. Prog. Electromagn. Res. C 2018, 88, 145–161. [Google Scholar]
- ANSYS Workbench 2020 R2 Documentation; ANSYS Inc.: Canonsburg, PA, USA, 2020.




| Perturbation/Noise Sources | Frequency (Hz) | ) | ) |
|---|---|---|---|
| Air- and water-cooling temperature changes [3,6] | 0.0003 | 4 | - |
| Sun and moon tides [3] | low | - | 10–30 |
| Experimental hall and ground vibration [3,5,7] | 1–100 | 10 | 5 |
| Booster ramp [3,5,7] | 1–10 | 0.60 | 0.35 |
| Girder vibration mode [3] | 10–60 | 0.85 | 1.40 |
| Power supply main [5] | 50 and 60 | 1.20 | 0.45 |
| Power supply ripple [8] | 0.1–10, 30–40 | horizontal impact | - |
| Insertion device parameter change [7] | 0.1–1 | - | - |
| Vacuum chamber vibration [8] | 20–70, 50–100 | horizontal impact | vertical impact |
| Undefined [5] | 85 | 0.35 | 0.35 |
| Year | SR Facility | FB Scheme | Bandwidth (Hz) | Core Material | Fabrication | Corrector Type | ) |
|---|---|---|---|---|---|---|---|
| 2023 | SPS-II | SOFB + FOFB | SOFB (TBC) | Iron | Block | COR-HV + S | 0.27 |
| 2023 | SPS-II | SOFB + FOFB | FOFB (TBC) | Iron | Lamination (1 mm) | COR-HV | 0.27 |
| 2021 | IFMIF [10] | SOFB | low | Iron | Block | Q + COR | |
| 2021 | NSLS-II [9] | FOFB | H (250–400), V (250–300) | Air | COR | 0.5 | |
| 2021 | Petra III [11] | FOFB | DC-715 | Air | COR | ||
| 2021 | SIRIUS [12] | SOFB + FOFB | SOFB (DC-1) | Iron | Block | COR | |
| 2022 | SIRIUS [12] | SOFB + FOFB | FOFB (1000) | Iron | Lamination (0.5 mm) | COR-H, COR-V | |
| 2019 | APS [13] | FOFB | DC-30, DC-50, 1000 * | Air | 8-pole COR | <2, * <1 | |
| 2019 | BNL [14] | SOFB | Low | Iron | Block | Q + COR-H, Q + COR-V | |
| 2016 | ESRF [7] | SOFB + FOFB | SOFB (DC) | Iron | Block | S + COR | 0.6 |
| 2016 | ESRF [7] | SOFB + FOFB | FOFB (DC-500) | Iron | Lamination | COR | 0.6 |
| 2011 | FERMILAB [15] | FOFB | >15 | Steel | Lamination | COR-H | |
| 2009 | SOLEIL [3] | SOFB + FOFB | SOFB (DC-0.02) | Iron | Block | COR | |
| 2009 | SOLEIL [3] | SOFB + FOFB | FOFB (DC-250) | Air | COR | ||
| 2005 | FERMILAB [16] | FOFB | >15 | Iron | Lamination (0.25 mm) | COR-HV + NorSkQ+ NorSkS | |
| 2007 | ELETTRA [17] | FOFB | DC-70 | Air | COR | <0.2 | |
| 2003 | ALS [18] | FOFB | DC-100, * (0.1–500) | Iron + air | Chicane | O < 1, * Hor. < 3, * Ver. < 2 | |
| 2003 | SLS [6] | SOFB + FOFB | SOFB (<3) | Iron | Block | COR-H, COR-V | x < 1, y < 0.3 |
| 2003 | SLS [5,6,19] | SOFB + FOFB | FOFB (<100) | COR-H, COR-V | x < 0.7, y < 0.06 | ||
| 2001 | SPEAR3 * [20] | FOFB | DC-100 | Iron | Lamination | COR-HV | x < 20, y < 5 |
| 1999 | SRS [21] | Local FB | 0.03 | Iron | Block | COR-H, COR-V | 1 |
| 1999 | SUPER-ACO [22] | FOFB | DC-150 | Air | Q + COR | <5 | |
| 1993 | TRIUMP [23] | SOFB | Low | Iron | Block | COR + Q + S |
| Parameter | Value | Unit |
|---|---|---|
| Field integral | 8 | Tesla.mm |
| Effective length | 116.5 | mm |
| Physical length | 50 | mm |
| Magnet gap | 65 | mm |
| Coil turn number/pole | 140 | |
| Maximum DC current | 18 | A |
| Maximum AC current | 1 | A |
| Conductor size | 3 × 4 | |
| Air-cooled magnet |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Prawanta, S.; Sunwong, P.; Singthong, P.; Leetha, T.; Numanoy, P.; Tangyotkhajorn, W.; Kwankasem, A.; Sooksrimuang, V.; Kongtawong, S.; Klinkiew, S. Development of a Combined Horizontal and Vertical Correcting Magnet for Siam Photon Source II. Particles 2023, 6, 898-912. https://doi.org/10.3390/particles6040058
Prawanta S, Sunwong P, Singthong P, Leetha T, Numanoy P, Tangyotkhajorn W, Kwankasem A, Sooksrimuang V, Kongtawong S, Klinkiew S. Development of a Combined Horizontal and Vertical Correcting Magnet for Siam Photon Source II. Particles. 2023; 6(4):898-912. https://doi.org/10.3390/particles6040058
Chicago/Turabian StylePrawanta, Supachai, Prapaiwan Sunwong, Pariwat Singthong, Thongchai Leetha, Pajeeraphorn Numanoy, Warissara Tangyotkhajorn, Apichai Kwankasem, Visitchai Sooksrimuang, Sukho Kongtawong, and Supat Klinkiew. 2023. "Development of a Combined Horizontal and Vertical Correcting Magnet for Siam Photon Source II" Particles 6, no. 4: 898-912. https://doi.org/10.3390/particles6040058
APA StylePrawanta, S., Sunwong, P., Singthong, P., Leetha, T., Numanoy, P., Tangyotkhajorn, W., Kwankasem, A., Sooksrimuang, V., Kongtawong, S., & Klinkiew, S. (2023). Development of a Combined Horizontal and Vertical Correcting Magnet for Siam Photon Source II. Particles, 6(4), 898-912. https://doi.org/10.3390/particles6040058





