Development of Type A Quadrupole Magnet for Siam Photon Source II
Abstract
1. Introduction
2. Magnet Design
2.1. Requirements and Design Parameters
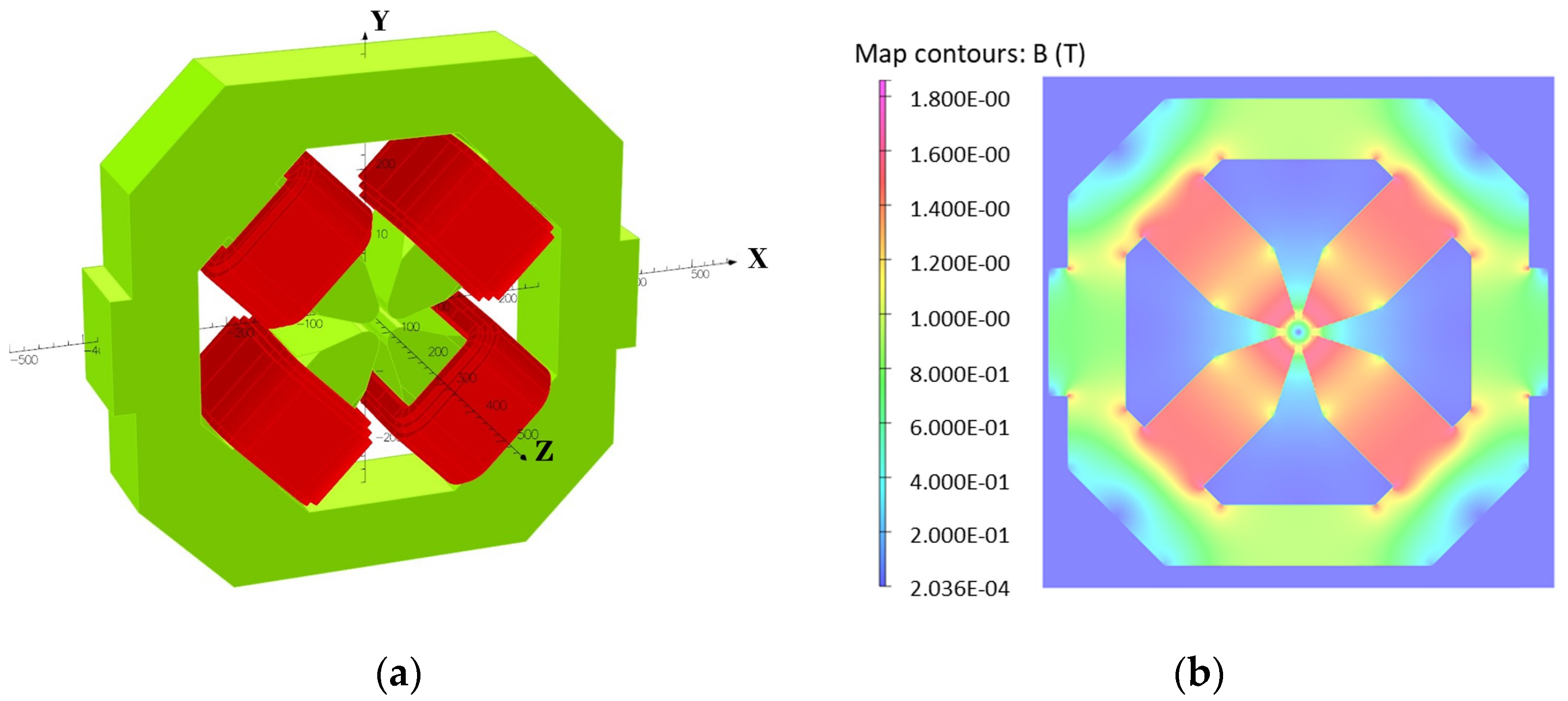
2.2. Magnetic Field Calculation
2.3. Mechanical Analysis
2.4. Hydraulic Calculation
3. Magnet Manufacturing
3.1. Magnet Yoke
3.2. Magnet Coils
4. Results and Discussion
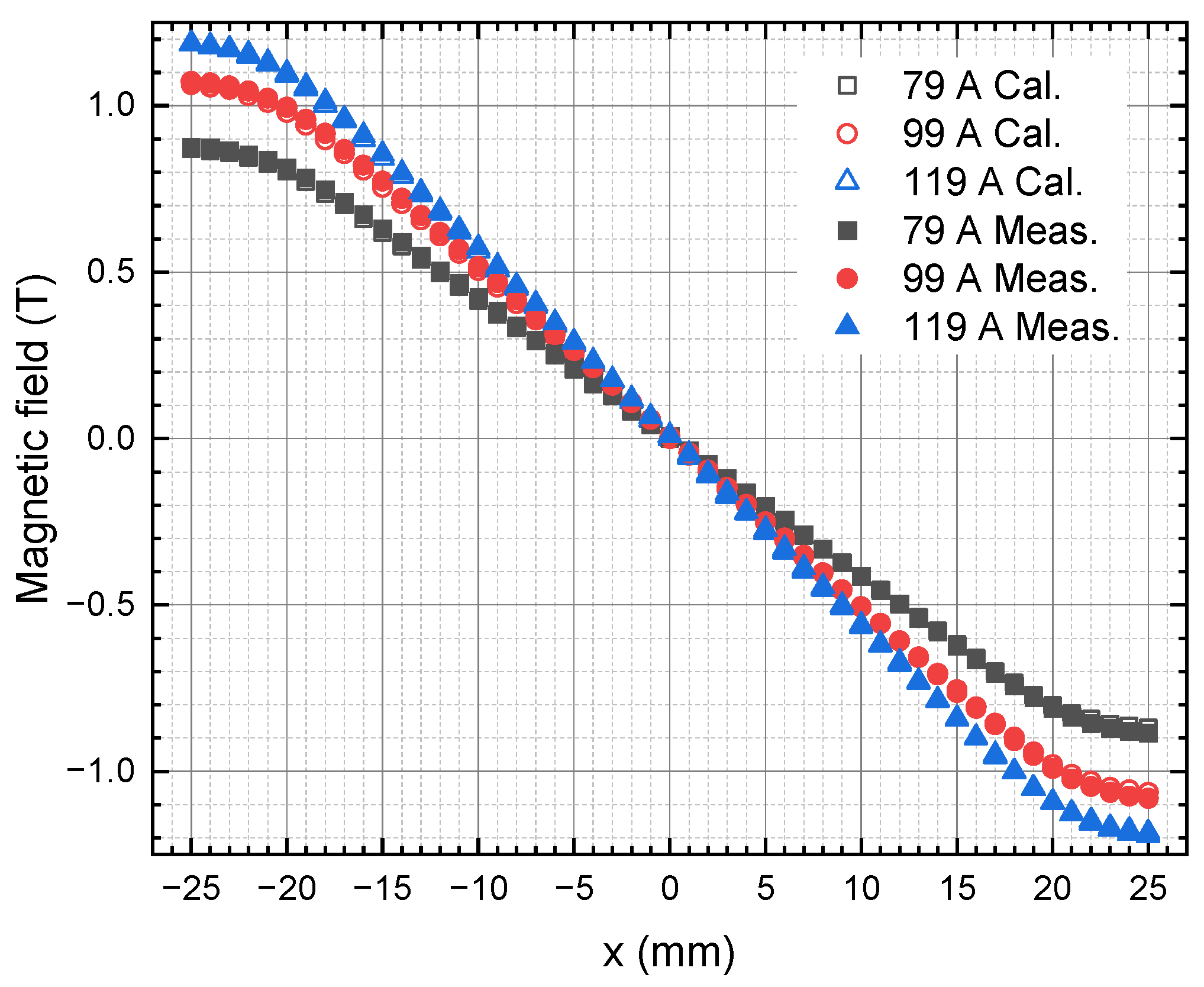
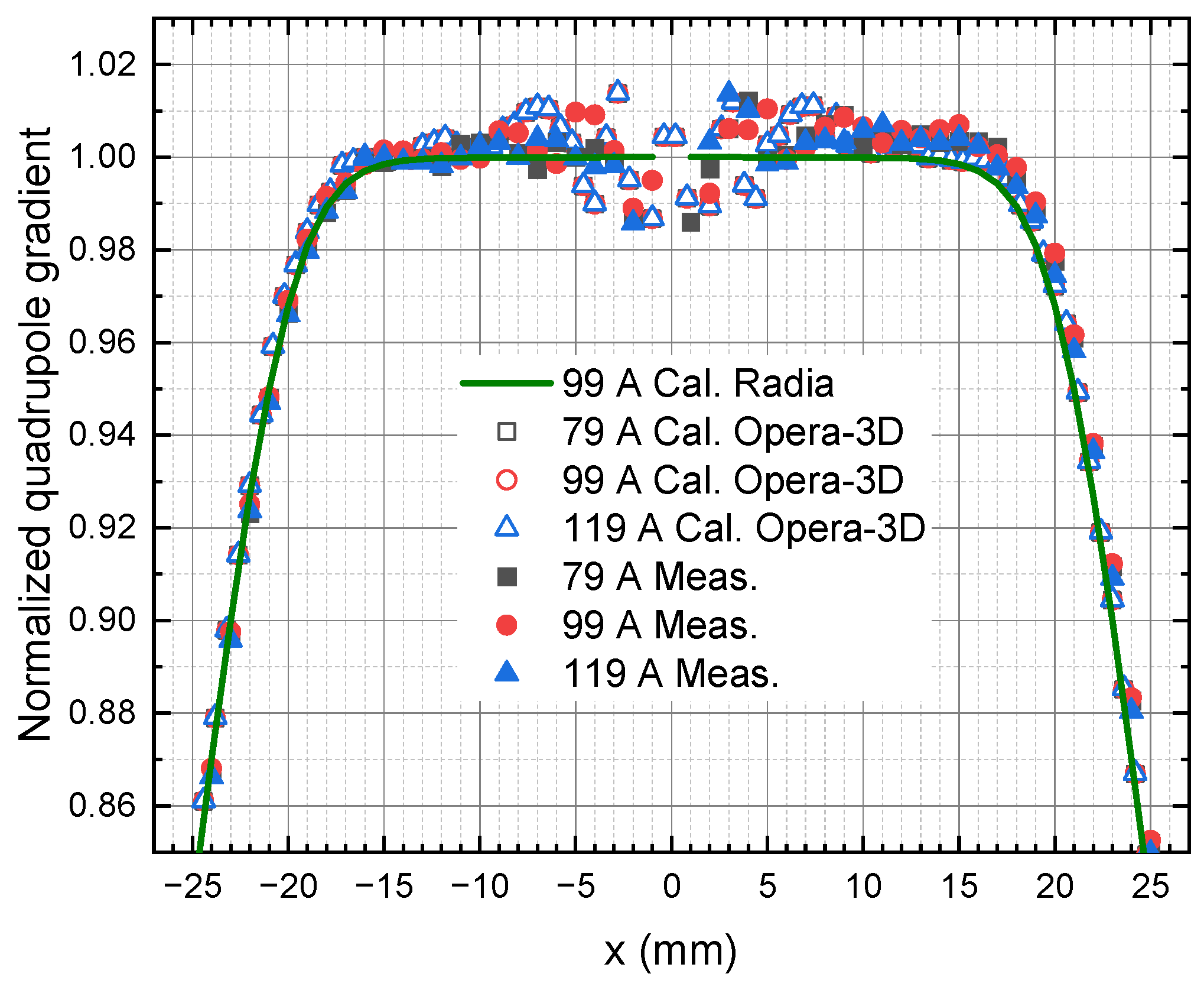
4.1. Magnetic Field Measurement
4.2. Temperature Measurement
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tavares, P.F.; Al-Dmour, E.; Andersson, Å.; Cullinan, F.; Jensen, B.N.; Olsson, D.; Olsson, D.K.; Sjöström, M.; Tarawneh, H.; Thorin, S.; et al. Commissioning and first-year operational results of the MAX IV 3 GeV ring. J. Synchrotron Rad. 2018, 25, 1291–1316. [Google Scholar] [CrossRef] [PubMed]
- Raimondi, P.; Carmignani, N.; Carver, L.R.; Chavanne, J.; Farvacque, L.; Le Bec, G.; Martin, D.; Liuzzo, S.M.; Perron, T.; White, S. Commissioning of the hybrid multibend achromat lattice at the European Synchrotron Radiation Facility. Phys. Rev. Accel. Beams. 2021, 24, 110701. [Google Scholar] [CrossRef]
- Liu, L.; Alves, M.B.; de Sa, F.H.; Oliveira, C.S.; Resende, X.R. Sirius commissioning results and operation status. In Proceedings of the 12th International Particle Accelerator Conference, Campinas, Brazil, 24–28 May 2021; pp. 13–18. [Google Scholar] [CrossRef]
- Klysubun, P.; Rugmai, S.; Rujirawat, S.; Cheedket, S.; Kwankasem, C.; Hoyes, G.; Oyamada, M. Operation and recent developments at the Siam Photon Source. In Proceedings of the Asian Particle Accelerator Conference, Indore, India, 29 January–2 February 2007; pp. 607–609. [Google Scholar] [CrossRef]
- Klysubun, P.; Sudmuang, P.; Pulampong, T.; Chanwattana, T.; Jummunt, S.; Sunwong, P.; Prawanta, S.; Kwankasem, A.; Juntong, N.; Phimsen, T.; et al. SPS-II: A 4th generation synchrotron light source in Southeast Asia. In Proceedings of the 13th International Particle Accelerator Conference, Bangkok, Thailand, 12–17 June 2022; pp. 764–768. [Google Scholar] [CrossRef]
- Klysubun, P.; Pulampong, T.; Sudmuang, P. Design and optimization of SPS-II storage ring. In Proceedings of the 8th International Particle Accelerator Conference, Copenhagen, Denmark, 14–19 May 2017; pp. 2773–2775. [Google Scholar] [CrossRef]
- Shin, S. New era of synchrotron radiation: Fourth-generation storage ring. AAPPS Bull. 2021, 31, 21. [Google Scholar] [CrossRef]
- Pulampong, T. (Synchrotron Light Research Institute, Nakhon Ratchasima, Thailand). SPS-II 3 GeV Storage Ring. Private communication, 2019.
- Vorozhtsov, A.; Modena, M. A New QF1 Magnet for ATF3. arXiv 2012, arXiv:1202.6301. [Google Scholar]
- Wiedemann, H. Particle Accelerator Physics; Springer: Berlin/Heidelberg, Germany, 2007; pp. 37–112. ISBN 978-3-540-49043-2. [Google Scholar]
- Tanabe, J. Iron Dominated Electromagnets: Design, Fabrication, Assembly and Measurements; World Scientific Publishing: Singapore, 2005; pp. 33–286. ISBN 9789813101982. [Google Scholar]
- Opera-3D 18R2 Reference Manual; Dassault Systèmes UK Ltd.: Kidlington, UK, 2018; pp. 1–918.
- Chubar, O.; Elleaume, P.; Chavanne, J. A three-dimensional magnetostatics computer code for insertion devices. J. Synchrotron Rad. 1998, 5, 481–484. [Google Scholar] [CrossRef] [PubMed]
- Sunwong, P.; Prawanta, S.; Phimsen, T.; Sudmuang, P.; Klysubun, P. Magnet design for Siam Photon Source II. In Proceedings of the 10th International Particle Accelerator Conference, Melbourne, Australia, 19–24 May 2019; pp. 4361–4363. [Google Scholar] [CrossRef]
- Rumiche, F.; Indacochea, J.E.; Wang, M.L. Assessment of the effect of Microstructure on the magnetic behavior of structural carbon steel using an electromagnetic sensor. J. Mater. Eng. Perf. 2008, 17, 586–593. [Google Scholar] [CrossRef]
- SOLIDWORKS Premium 2021 SP5 1 Online Help; Dassault Systèmes SOLIDWORKS Corp.: Waltham, MA, USA, 2021.
- ANSYS Workbench 2020 R2 Documentation; ANSYS, Inc.: Canonsburg, PA, USA, 2020.
- Johansson, M.; Anderberg, B.; Lindgren, L.J. Magnet design for a low-emittance storage ring. J. Synchrotron Rad. 2014, 21, 884–903. [Google Scholar] [CrossRef] [PubMed]
- Prawanta, S.; Sunwong, P.; Boonwanna, B.; Sooksrimuang, V.; Kwankasem, A.; Pongampai, S.; Sudmuang, P.; Klysubun, P. Design and construction of sextupole magnet prototype for Siam Photon Source II project. In Proceedings of the 10th International Particle Accelerator Conference, Melbourne, Australia, 19–24 May 2019; pp. 4295–4297. [Google Scholar] [CrossRef]
- Lake Shore Hall Probes. Available online: https://www.lakeshore.com/docs/default-source/product-downloads/catalog/hall-probes_l.pdf?sfvrsn=9e7b957d_6 (accessed on 29 May 2023).
- Le Bec, G.; Chavanne, J.; Benabderrahmane, C.; Farvacque, L.; Goirand, L.; Liuzzo, S.; Raimondi, P.; Villar, F. High gradient quadrupoles for low emittance storage rings. Phys. Rev. Accel. Beams. 2016, 19, 52401. [Google Scholar] [CrossRef]
- Buzio, M. Fabrication and calibration of search coils. arXiv 2011, arXiv:1104.0803. [Google Scholar]
- Walckiers, L. Magnetic measurement with coils and wires. arXiv 2011, arXiv:1104.3784. [Google Scholar]
- Le Bec, G.; Chavanne, J.; Pennel, C. Stretched wire measurement of multipole accelerator magnets. Phys. Rev. Accel. Beams 2012, 15, 22401. [Google Scholar] [CrossRef]
- Jan, J.C.; Kuo, C.Y.; Chang, C.H.; Chu, Y.L.; Yu, Y.T.; Lin, F.Y.; Chen, H.H.; Huang, H.M.; Yang, C.S.; Hwang, C.S. Magnetic field character of TPS booster magnets. In Proceedings of the 4th International Particle Accelerator Conference, Shanghai, China, 12–17 May 2013; pp. 3594–3596, ISBN 978-3-95450-122-9. [Google Scholar]
- Sunwong, P.; Boonwanna, B.; Chaichuay, S.; Klysubun, P.; Kwankasem, A.; Preecha, C.; Sooksrimuang, V. Design and fabrication of a combined function magnet prototype for Siam Photon Source. In Proceedings of the 9th International Particle Accelerator Conference, Vancouver, BC, Canada, 29 April–4 May 2018; pp. 3466–3468. [Google Scholar] [CrossRef]




| Parameter | Value |
|---|---|
| Quadrupole gradient | 51 T/m |
| Effective length | 162 mm |
| Physical length | 144 mm |
| Bore radius | 16 mm |
| Turn number per pole | 56 |
| Operating current | 99 A |
| Maximum current | 119 A |
| Field homogeneity | 5 × 10−4 within ±10 mm |
| Temperature rise at 119 A | 2.8 °C at 5.0 L/min |
| Multipole Fields | Radia | Opera−3D | Measurement |
|---|---|---|---|
| B0 (T) | +9.6 × 10−13 | −4.9 × 10−15 | +7.1 × 10−03 |
| B1 (T/m) | −5.1 × 10+01 | −5.1 × 10+01 | −5.1 × 10+01 |
| B2 (T/m2) | +2.5 × 10−06 | −6.2 × 10−11 | +2.6 × 10+01 |
| B3 (T/m3) | +1.7 × 10+02 | +2.0 × 10+05 | −1.3 × 10+05 |
| B4 (T/m4) | −1.8 × 1000 | +1.0 × 10−05 | −2.2 × 10+07 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Prawanta, S.; Leetha, T.; Singthong, P.; Numanoy, P.; Kwankasem, A.; Sooksrimuang, V.; Preecha, C.; Klinkiew, S.; Sunwong, P. Development of Type A Quadrupole Magnet for Siam Photon Source II. Particles 2023, 6, 664-673. https://doi.org/10.3390/particles6020039
Prawanta S, Leetha T, Singthong P, Numanoy P, Kwankasem A, Sooksrimuang V, Preecha C, Klinkiew S, Sunwong P. Development of Type A Quadrupole Magnet for Siam Photon Source II. Particles. 2023; 6(2):664-673. https://doi.org/10.3390/particles6020039
Chicago/Turabian StylePrawanta, Supachai, Thongchai Leetha, Pariwat Singthong, Pajeeraphorn Numanoy, Apichai Kwankasem, Visitchai Sooksrimuang, Chaiyut Preecha, Supat Klinkiew, and Prapaiwan Sunwong. 2023. "Development of Type A Quadrupole Magnet for Siam Photon Source II" Particles 6, no. 2: 664-673. https://doi.org/10.3390/particles6020039
APA StylePrawanta, S., Leetha, T., Singthong, P., Numanoy, P., Kwankasem, A., Sooksrimuang, V., Preecha, C., Klinkiew, S., & Sunwong, P. (2023). Development of Type A Quadrupole Magnet for Siam Photon Source II. Particles, 6(2), 664-673. https://doi.org/10.3390/particles6020039





