Λ Polarization and Vortex Rings in Heavy-Ion Collisions at NICA Energies
Abstract
1. Introduction
2. Global Polarization at NICA Energies
2.1. Thermal-Vorticity Polarization
2.2. Meson-Field Induced Polarization
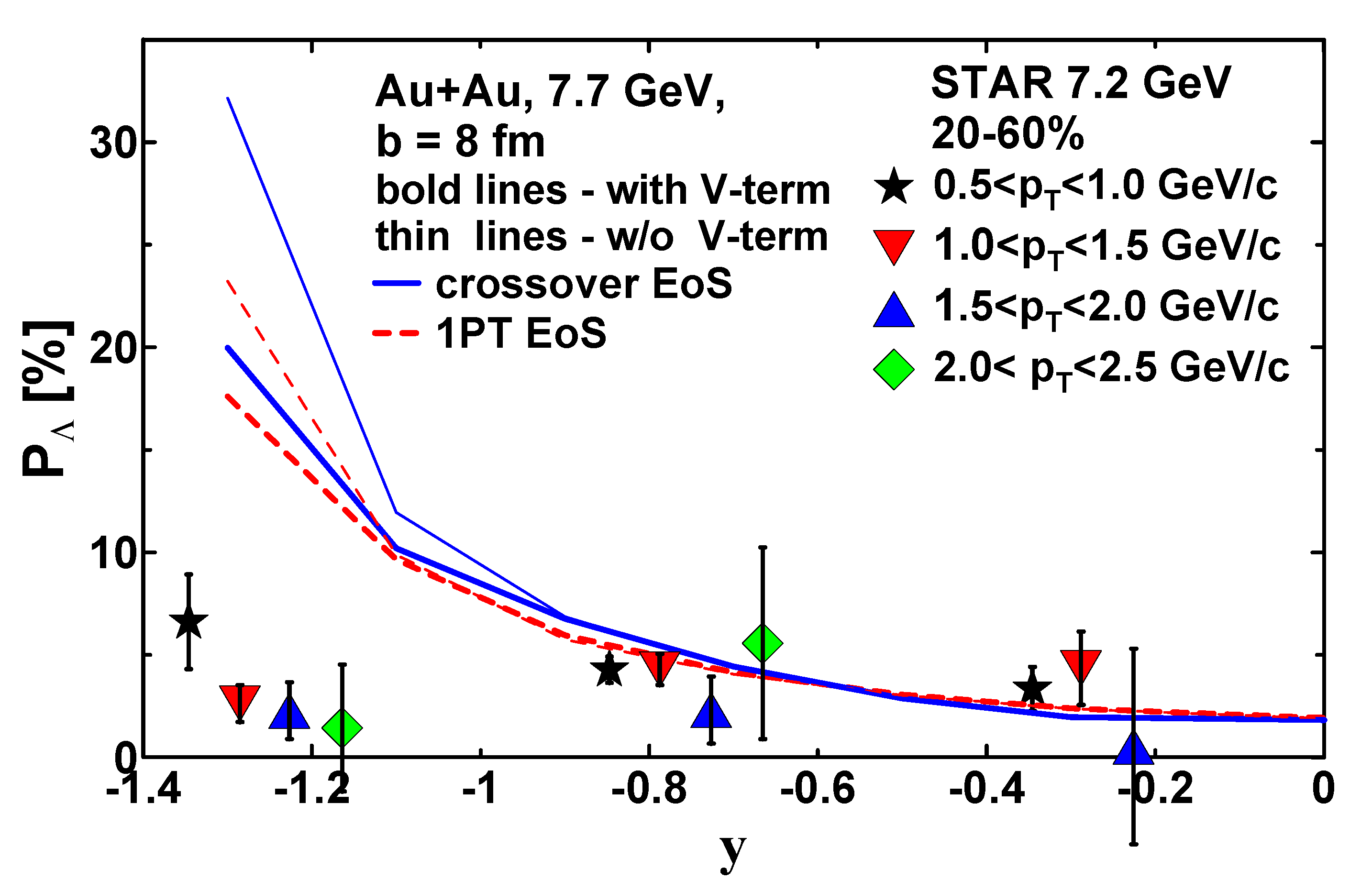
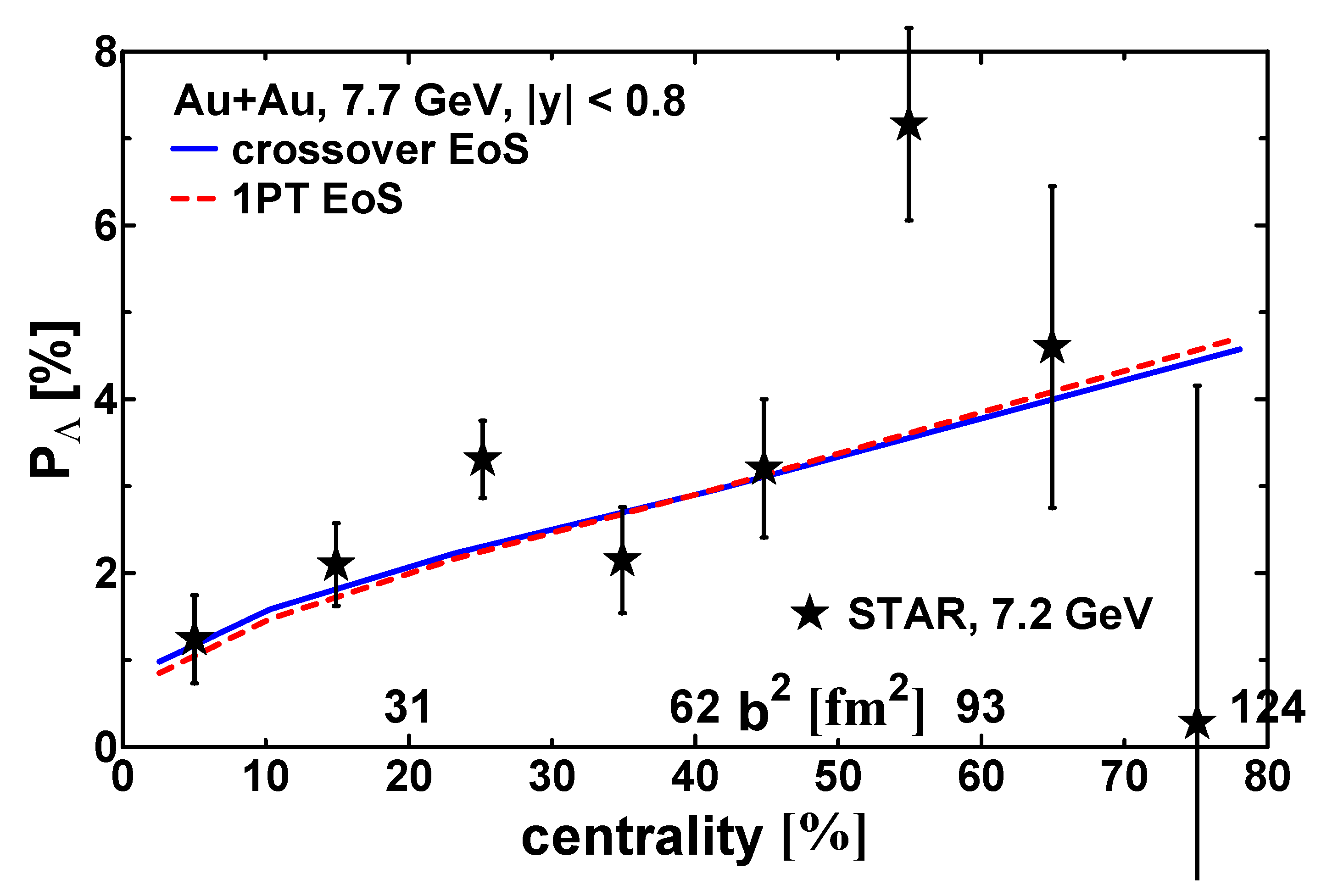
2.3. Results
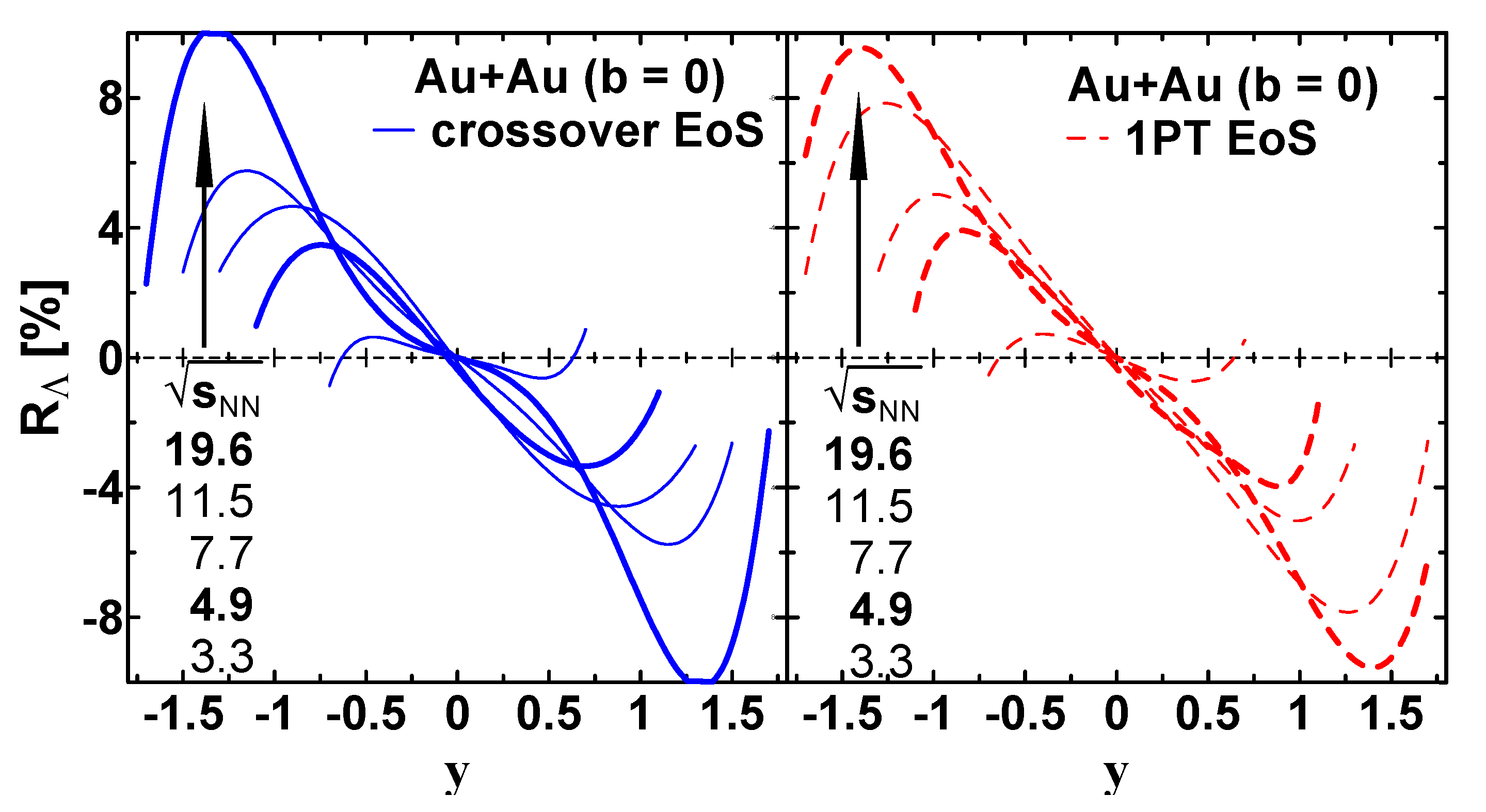
3. Vortical Rings
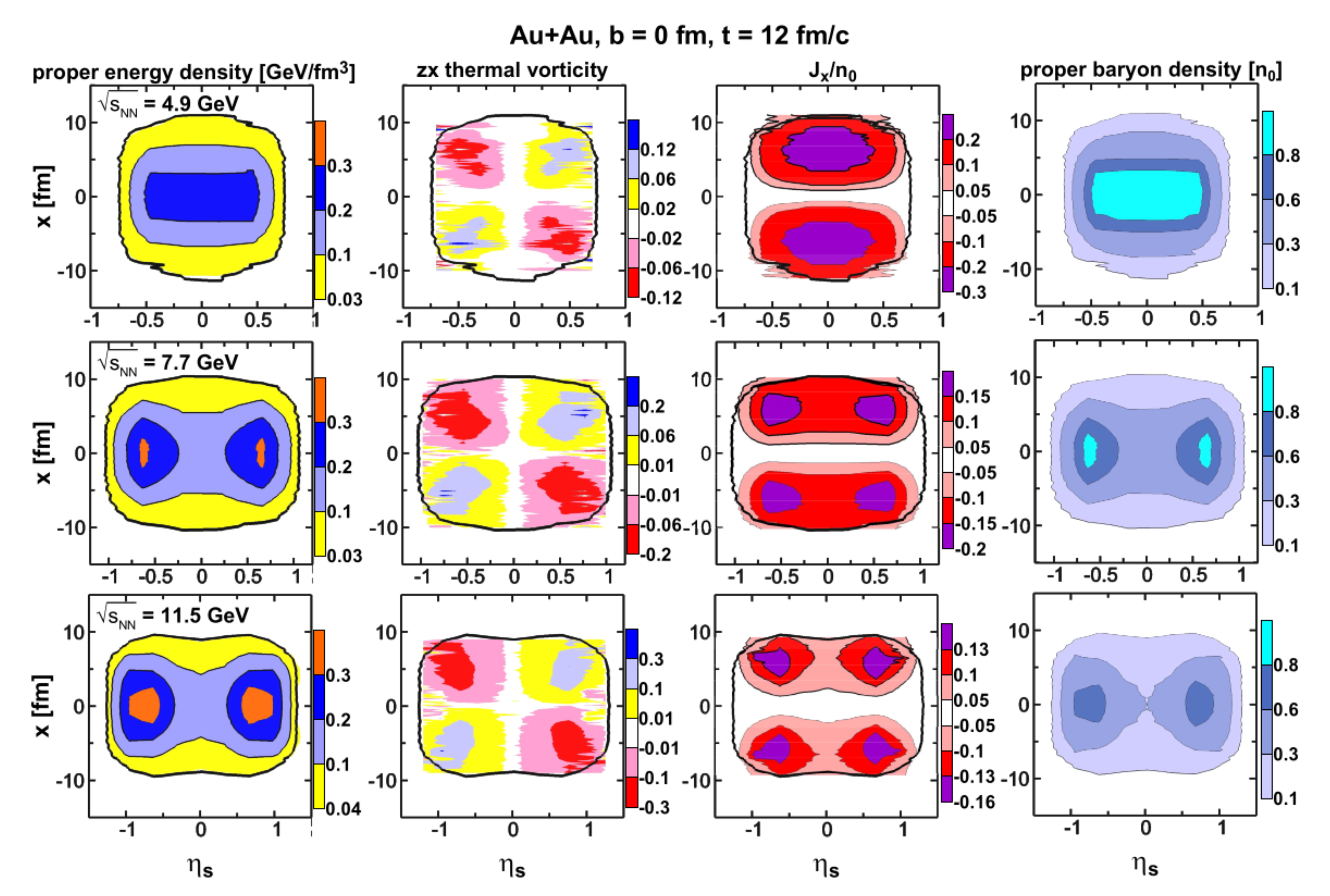
3.1. Vortical Rings in Central Collisions
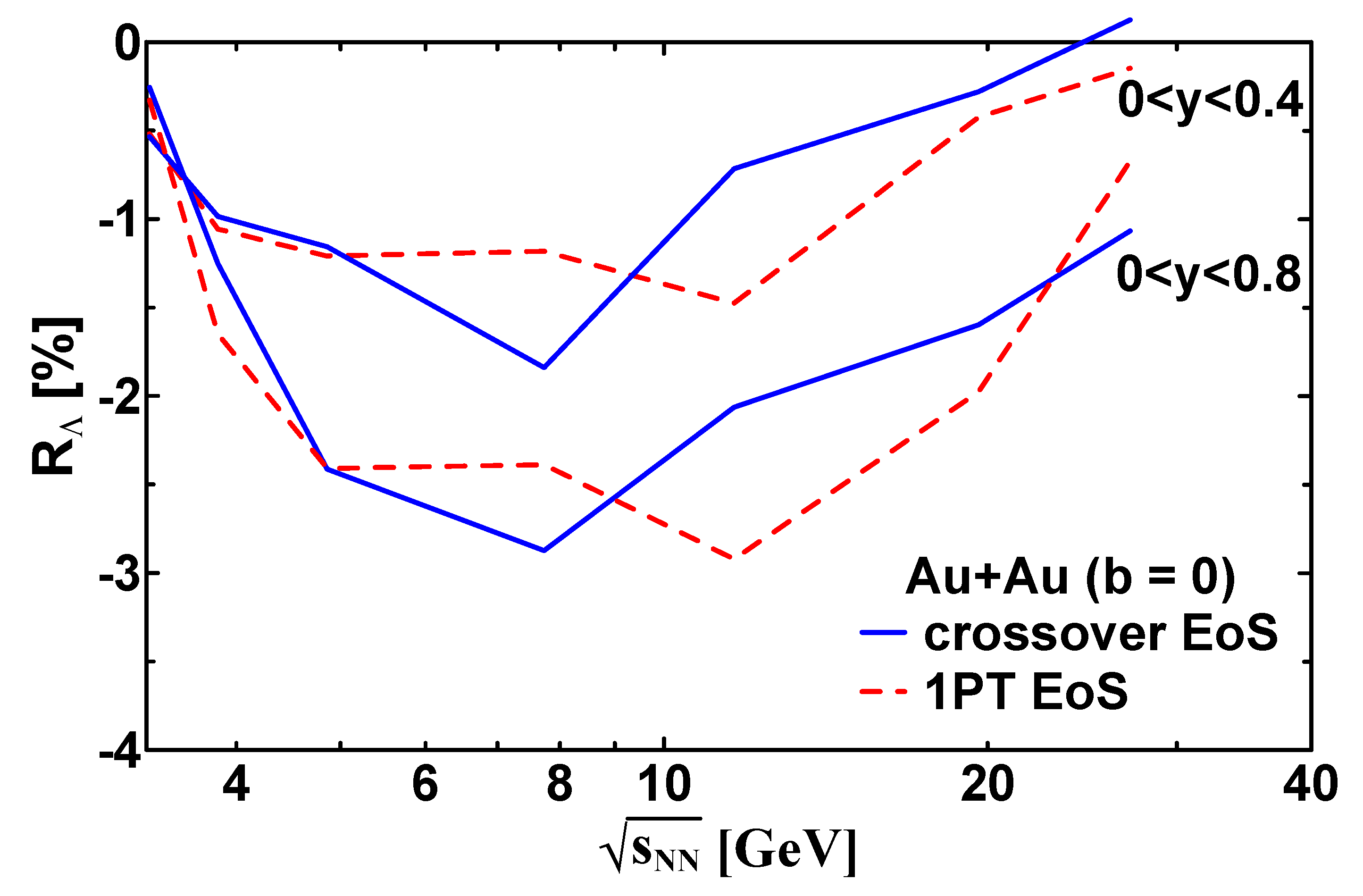
3.2. Ring Observable
4. Summary and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| NICA | Nuclotron-based Ion Collider Facility |
| RHIC | Relativistic Heavy Ion Collider |
| FAIR | Facility for Antiproton and Ion Research |
| FXT-STAR | STAR fixed-target program at RHIC |
| 3FD | three-fluid dynamics |
| EoS | equation of state |
| 1PT EoS | EoS with the first-order phase transition |
References
- Liang, Z.T.; Wang, X.N. Globally polarized quark-gluon plasma in non-central A+A collisions. Phys. Rev. Lett. 2005, 94, 102301, Erratum: [Phys. Rev. Lett. 2006, 96, 039901]. [Google Scholar] [CrossRef] [PubMed]
- Betz, B.; Gyulassy, M.; Torrieri, G. Polarization probes of vorticity in heavy ion collisions. Phys. Rev. C 2007, 76, 044901. [Google Scholar] [CrossRef]
- Gao, J.H.; Chen, S.W.; Deng, W.t.; Liang, Z.T.; Wang, Q.; Wang, X.N. Global quark polarization in non-central A+A collisions. Phys. Rev. C 2008, 77, 044902. [Google Scholar] [CrossRef]
- Barnett, S.J. Magnetization by rotation. Phys. Rev. 1915, 6, 239. [Google Scholar]
- Ivanov, Y.B.; Soldatov, A.A. Vortex rings in fragmentation regions in heavy-ion collisions at = 39 GeV. Phys. Rev. C 2018, 97, 044915. [Google Scholar] [CrossRef]
- Xia, X.L.; Li, H.; Tang, Z.B.; Wang, Q. Probing vorticity structure in heavy-ion collisions by local Λ polarization. Phys. Rev. C 2018, 98, 024905. [Google Scholar] [CrossRef]
- Wei, D.X.; Deng, W.T.; Huang, X.G. Thermal vorticity and spin polarization in heavy-ion collisions. Phys. Rev. C 2019, 99, 014905. [Google Scholar] [CrossRef]
- Ivanov, Y.B.; Toneev, V.D.; Soldatov, A.A. Vorticity and Particle Polarization in Relativistic Heavy-Ion Collisions. Phys. Atom. Nucl. 2020, 83, 179–187. [Google Scholar] [CrossRef]
- Zinchenko, A.; Sorin, A.; Teryaev, O.; Baznat, M. Vorticity structure and polarization of Λ hyperons in heavy-ion collisions. J. Phys. Conf. Ser. 2020, 1435, 012030. [Google Scholar] [CrossRef]
- Baznat, M.; Gudima, K.; Sorin, A.; Teryaev, O. Helicity separation in Heavy-Ion Collisions. Phys. Rev. C 2013, 88, 061901. [Google Scholar] [CrossRef]
- Baznat, M.I.; Gudima, K.K.; Sorin, A.S.; Teryaev, O.V. Femto-vortex sheets and hyperon polarization in heavy-ion collisions. Phys. Rev. C 2016, 93, 031902. [Google Scholar] [CrossRef]
- Tsegelnik, N.S.; Kolomeitsev, E.E.; Voronyuk, V. Helicity and vorticity in heavy-ion collisions at NICA energies. arXiv 2022, arXiv:2211.09219. [Google Scholar]
- Lisa, M.A.; Barbon, J.G.P.; Chinellato, D.D.; Serenone, W.M.; Shen, C.; Takahashi, J.; Torrieri, G. Vortex rings from high energy central p+A collisions. Phys. Rev. C 2021, 104, 011901. [Google Scholar] [CrossRef]
- Serenone, W.M.; Barbon, J.G.P.; Chinellato, D.D.; Lisa, M.A.; Shen, C.; Takahashi, J.; Torrieri, G. Λ polarization from thermalized jet energy. Phys. Lett. B 2021, 820, 136500. [Google Scholar] [CrossRef]
- Ivanov, Y.B.; Russkikh, V.N.; Toneev, V.D. Relativistic heavy-ion collisions within 3-fluid hydrodynamics: Hadronic scenario. Phys. Rev. C 2006, 73, 044904. [Google Scholar] [CrossRef]
- Ivanov, Y.B. Global Λ polarization in moderately relativistic nuclear collisions. Phys. Rev. C 2021, 103, L031903. [Google Scholar] [CrossRef]
- Ivanov, Y.B.; Soldatov, A.A. Global Λ polarization in heavy-ion collisions at energies 2.4–7.7 GeV: Effect of meson-field interaction. Phys. Rev. C 2022, 105, 034915. [Google Scholar] [CrossRef]
- Ivanov, Y.B. Vortex rings in heavy-ion collisions at energies = 3–30 GeV and possibility of their observation. arXiv 2022, arXiv:2211.17190. [Google Scholar]
- Ivanov, Y.B. Alternative Scenarios of Relativistic Heavy-Ion Collisions: I. Baryon Stopping. Phys. Rev. C 2013, 87, 064904. [Google Scholar] [CrossRef]
- Ivanov, Y.B. Baryon Stopping as a Probe of Deconfinement Onset in Relativistic Heavy-Ion Collisions. Phys. Lett. B 2013, 721, 123. [Google Scholar] [CrossRef]
- Ivanov, Y.B. Alternative Scenarios of Relativistic Heavy-Ion Collisions: II. Particle Production. Phys. Rev. C 2013, 87, 064905. [Google Scholar] [CrossRef]
- Ivanov, Y.B. Alternative Scenarios of Relativistic Heavy-Ion Collisions: III. Transverse Momentum Spectra. Phys. Rev. C 2014, 89, 024903. [Google Scholar] [CrossRef]
- Ivanov, Y.B.; Soldatov, A.A. Bulk Properties of the Matter Produced at Energies of the Beam Energy Scan Program. Phys. Rev. C 2018, 97, 024908. [Google Scholar] [CrossRef]
- Ivanov, Y.B.; Soldatov, A.A. Elliptic Flow in Heavy-Ion Collisions at Energies = 2.7–39 GeV. Phys. Rev. C 2015, 91, 024914. [Google Scholar] [CrossRef]
- Konchakovski, V.P.; Cassing, W.; Ivanov, Y.B.; Toneev, V.D. Examination of the directed flow puzzle in heavy-ion collisions. Phys. Rev. C 2014, 90, 014903. [Google Scholar] [CrossRef]
- Mishustin, I.N.; Russkikh, V.N.; Satarov, L.M. Fluid dynamical model of relativistic heavy ion collision. Sov. J. Nucl. Phys. 1991, 54, 260–314. (In Russian) [Google Scholar]
- Khvorostukin, A.S.; Skokov, V.V.; Toneev, V.D.; Redlich, K. Lattice QCD constraints on the nuclear equation of state. Eur. Phys. J. C 2006, 48, 531–543. [Google Scholar] [CrossRef]
- Adamczyk, L.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Alekseev, I.; Alford, J.; Anson, C.D.; Aparin, A.; Arkhipkin, D.; et al. Global Λ hyperon polarization in nuclear collisions: Evidence for the most vortical fluid. Nature 2017, 548, 62. [Google Scholar]
- Adam, J.; Adamczyk, L.; Adams, J.R.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Ajitanand, N.N.; Alekseev, I.; Anderson, D.M.; et al. Global polarization of Λ hyperons in Au+Au collisions at = 200 GeV. Phys. Rev. C 2018, 98, 014910. [Google Scholar] [CrossRef]
- Adam, J.; Adamczyk, L.; Adams, J.R.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Ajitanand, N.N.; Alekseev, I.; Anderson, D.M.; et al. Global Polarization of Ξ and Ω Hyperons in Au+Au Collisions at = 200 GeV. Phys. Rev. Lett. 2021, 126, 162301. [Google Scholar] [CrossRef]
- Adam, J.; Adamczyk, L.; Adams, J.R.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Ajitanand, N.N.; Alekseev, I.; Anderson, D.M.; et al. Polarization of Λ () hyperons along the beam direction in Au+Au collisions at = 200 GeV. Phys. Rev. Lett. 2019, 123, 132301. [Google Scholar] [CrossRef]
- Deng, X.G.; Huang, X.G.; Ma, Y.G.; Zhang, S. Vorticity in low-energy heavy-ion collisions. Phys. Rev. C 2020, 101, 064908. [Google Scholar] [CrossRef]
- Ayala, A.; Domínguez, I.; Maldonado, I.; Tejeda-Yeomans, M.E. Rise and fall of Λ and global polarization in semi-central heavy-ion collisions at HADES, NICA and RHIC energies from the core-corona model. Phys. Rev. C 2022, 105, 034907. [Google Scholar] [CrossRef]
- Guo, Y.; Liao, J.; Wang, E.; Xing, H.; Zhang, H. Hyperon polarization from the vortical fluid in low-energy nuclear collisions. Phys. Rev. C 2021, 104, L041902. [Google Scholar] [CrossRef]
- Abdallah, M.S.; Aboona, B.E.; Adam, J.; Adamczyk, L.; Adams, J.R.; Adkins, J.K.; Agakishiev, G.; Aggarwal, I.; Aggarwal, M.M.; Ahammed, Z.; et al. Global Λ-hyperon polarization in Au+Au collisions at = 3 GeV. Phys. Rev. C 2021, 104, L061901. [Google Scholar] [CrossRef]
- Okubo, K. Measurement of global polarization of Λ hyperons in Au+Au = 7.2 GeV fixed target collisions at RHIC-STAR experiment. EPJ Web Conf. 2022, 259, 06003. [Google Scholar] [CrossRef]
- Abou Yassine, R.; Adamczewski-Musch, J.; Asal, C.; Becker, M.; Belounnas, A.; Blanco, A.; Blume, C.; Chlad, L.; Chudoba, P.; Ciepa, I.; et al. Measurement of global polarization of Λ hyperons in few-GeV heavy-ion collisions. Phys. Lett. B 2022, 835, 137506. [Google Scholar] [CrossRef]
- Becattini, F.; Chandra, V.; Del Zanna, L.; Grossi, E. Relativistic distribution function for particles with spin at local thermodynamical equilibrium. Ann. Phys. 2013, 338, 32. [Google Scholar] [CrossRef]
- Becattini, F.; Karpenko, I.; Lisa, M.; Upsal, I.; Voloshin, S. Global hyperon polarization at local thermodynamic equilibrium with vorticity, magnetic field and feed-down. Phys. Rev. C 2017, 95, 054902. [Google Scholar] [CrossRef]
- Kolomeitsev, E.E.; Toneev, V.D.; Voronyuk, V. Vorticity and hyperon polarization at energies available at JINR Nuclotron-based Ion Collider fAcility. Phys. Rev. C 2018, 97, 064902. [Google Scholar] [CrossRef]
- Russkikh, V.N.; Ivanov, Y.B. Dynamical freeze-out in 3-fluid hydrodynamics. Phys. Rev. C 2007, 76, 054907. [Google Scholar] [CrossRef]
- Ivanov, Y.B.; Russkikh, V.N. On freeze-out problem in relativistic hydrodynamics. Phys. Atom. Nucl. 2009, 72, 1238. [Google Scholar] [CrossRef]
- Csernai, L.; Kapusta, J.; Welle, T. Λ and spin interaction with meson fields generated by the baryon current in high energy nuclear collisions. Phys. Rev. C 2019, 99, 021901. [Google Scholar] [CrossRef]
- Xie, Y.; Chen, G.; Csernai, L.P. A study of Λ and polarization splitting by meson field in PICR hydrodynamic model. Eur. Phys. J. C 2021, 81, 12. [Google Scholar] [CrossRef]
- Walecka, J.D. A theory of highly condensed matter. Ann. Phys. 1974, 83, 491. [Google Scholar] [CrossRef]
- Serot, B.D. Quantum hadrodynamics. Rep. Prog. Phys. 1992, 55, 1855. [Google Scholar] [CrossRef]
- Kapusta, J.I.; Gale, C. Finite Temperature Field Theory; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Weissenborn, S.; Chatterjee, D.; Schaffner-Bielich, J. Hyperons and massive neutron stars: Vector repulsion and SU(3) symmetry. Phys. Rev. C 2012, 85, 065802, [Erratum: Phys. Rev. C 2014, 90, 019904]. [Google Scholar] [CrossRef]
- Maslov, K.A.; Kolomeitsev, E.E.; Voskresensky, D.N. Relativistic Mean-Field Models with Scaled Hadron Masses and Couplings: Hyperons and Maximum Neutron Star Mass. Nucl. Phys. A 2016, 950, 64–109. [Google Scholar] [CrossRef]
- Cohen, J.; Weber, H.J. Relativistic sigma—Omega mean field theory for hyperons from a quark model. Phys. Rev. C 1991, 44, 1181–1187. [Google Scholar] [CrossRef]
- Web Interface for a Nuclear Overlap Calculation Code. Available online: http://web-docs.gsi.de/~misko/overlap/interface.html (accessed on 23 January 2023).
- Hauenstein, F.; Borodina, E.; Clement, H.; Doroshkevich, E.; Dzhygadlo, R.; Ehrhardt, K.; Eyrich, W.; Gast, W.; Gillitzer, A.; Grzonka, D.; et al. Measurement of polarization observables of the associated strangeness production in proton proton interactions. Eur. Phys. J. A 2016, 52, 337. [Google Scholar] [CrossRef]
- Agakishiev, G.; Arnold, O.; Balanda, A.; Belver, D.; Belyaev, A.V.; Berger-Chen, J.C.; Blanco, A.; Bohmer, M.; Boyard, J.L.; Cabanelas, P.; et al. Lambda hyperon production and polarization in collisions of p(3.5 GeV)+Nb. Eur. Phys. J. A 2014, 50, 81. [Google Scholar] [CrossRef]
- Adamczyk, L.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Alekseev, I.; Alford, J.; Anson, C.D.; Aparin, A.; Arkhipkin, D.; et al. Bulk Properties of the Medium Produced in Relativistic Heavy-Ion Collisions from the Beam Energy Scan Program. Phys. Rev. C 2017, 96, 044904. [Google Scholar] [CrossRef]
- Panagiotou, A.D. Λ0 Nonpolarization: Possible Signature of Quark Matter. Phys. Rev. C 1986, 33, 1999–2002. [Google Scholar] [CrossRef]
- Ayala, A.; Cuautle, E.; Herrera, G.; Montano, L.M. Λ0 polarization as a probe for production of deconfined matter in ultrarelativistic heavy ion collisions. Phys. Rev. C 2002, 65, 024902. [Google Scholar] [CrossRef]
- Bellwied, R. Transverse polarization of Lambda hyperons in relativistic Au—Au collisions at the AGS. Acta Phys. Hung. A 2002, 15, 437–444. [Google Scholar] [CrossRef]
- Adams, J.R. Differential measurements of Λ polarization in Au+Au collisions and a search for the magnetic field by STAR. Nucl. Phys. A 2021, 1005, 121864. [Google Scholar] [CrossRef]
- Nazarova, E.; Akhat, R.; Baznat, M.; Teryaev, O.; Zinchenko, A. Monte Carlo Study of Λ Polarization at MPD. Phys. Part. Nucl. Lett. 2021, 18, 429–438. [Google Scholar] [CrossRef]
- Aad, G.; Abbott, B.; Abdallah, J.; Abdel Khalek, S.; Abdinov, O.; Aben, R.; Abi, B.; Abolins, M.; AbouZeid, O.S.; Abramowicz, H.; et al. Measurement of the transverse polarization of Λ and hyperons produced in proton-proton collisions at = 7 TeV using the ATLAS detector. Phys. Rev. D 2015, 91, 032004. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ivanov, Y.B.; Soldatov, A.A. Λ Polarization and Vortex Rings in Heavy-Ion Collisions at NICA Energies. Particles 2023, 6, 245-261. https://doi.org/10.3390/particles6010014
Ivanov YB, Soldatov AA. Λ Polarization and Vortex Rings in Heavy-Ion Collisions at NICA Energies. Particles. 2023; 6(1):245-261. https://doi.org/10.3390/particles6010014
Chicago/Turabian StyleIvanov, Yuri B., and Alexei A. Soldatov. 2023. "Λ Polarization and Vortex Rings in Heavy-Ion Collisions at NICA Energies" Particles 6, no. 1: 245-261. https://doi.org/10.3390/particles6010014
APA StyleIvanov, Y. B., & Soldatov, A. A. (2023). Λ Polarization and Vortex Rings in Heavy-Ion Collisions at NICA Energies. Particles, 6(1), 245-261. https://doi.org/10.3390/particles6010014





