Approaches to Measuring Direct Photon Yield in A–A Collisions
Abstract
:1. Introduction
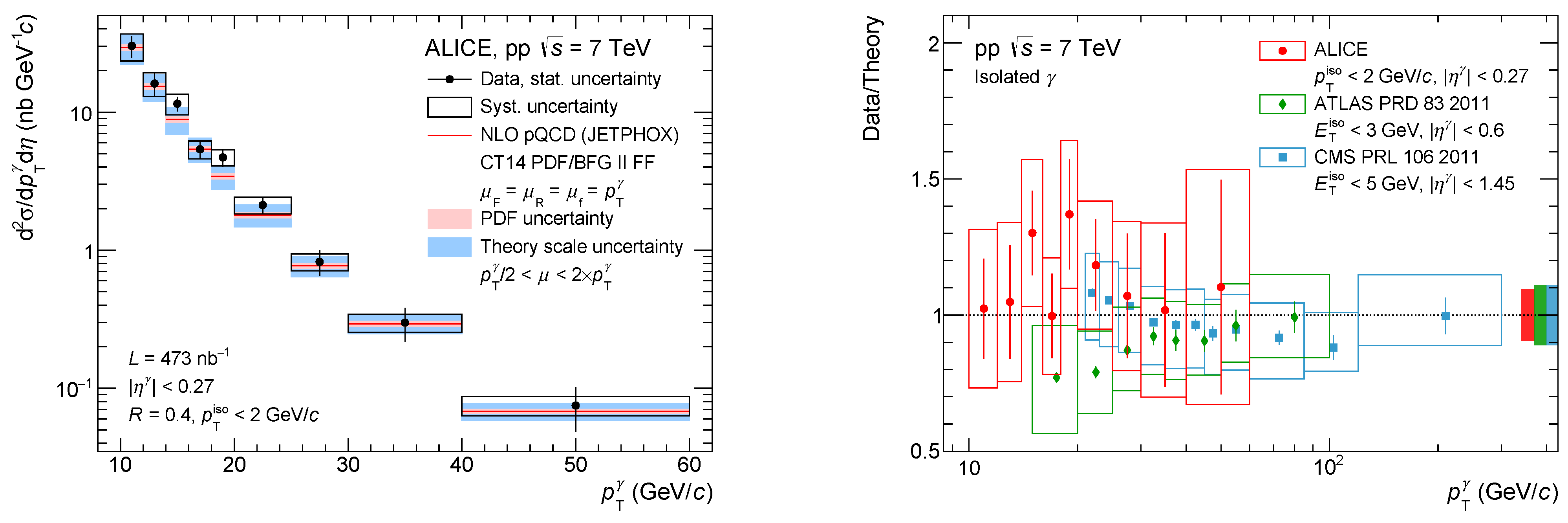
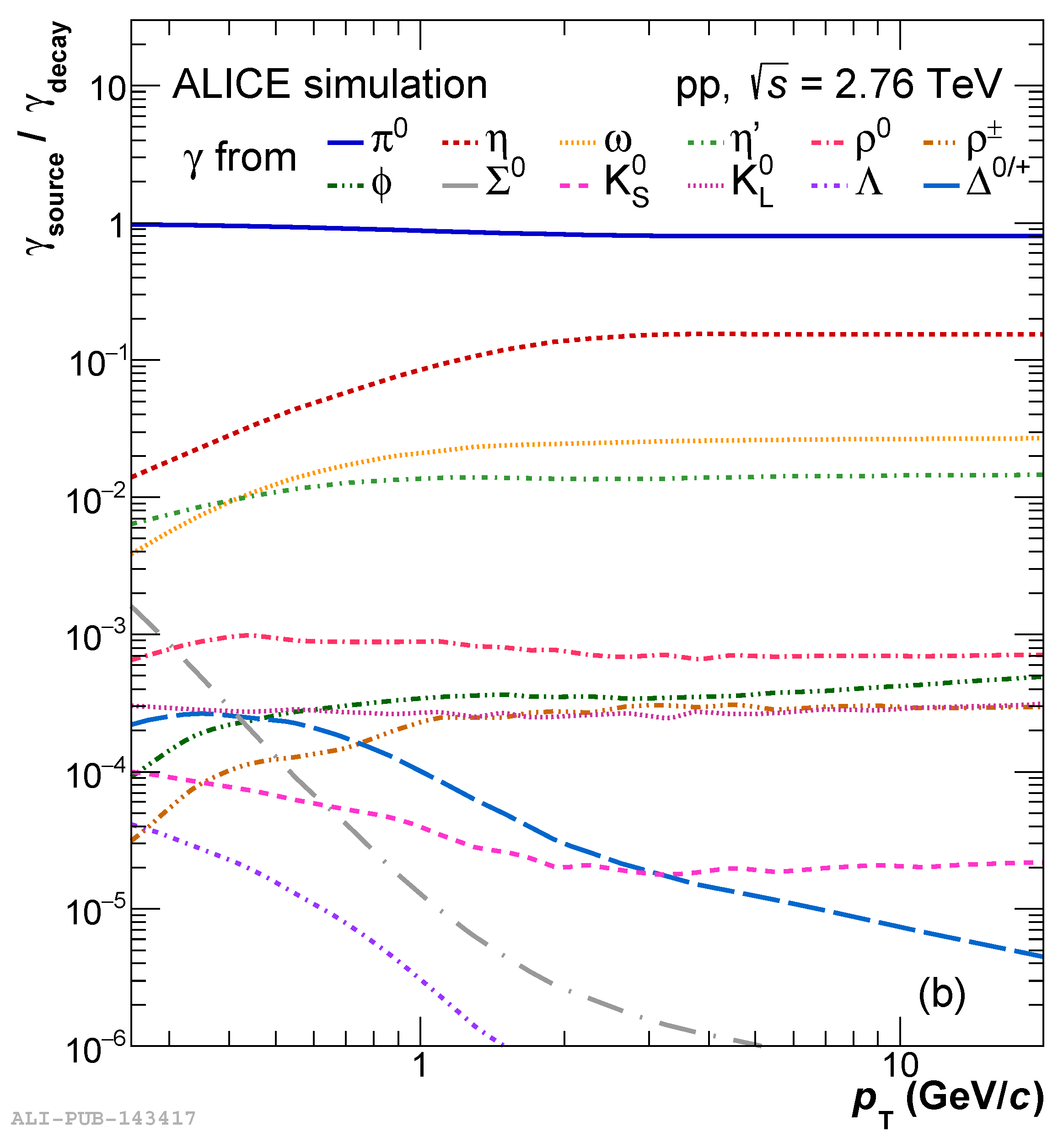
2. Isolated Direct Photons
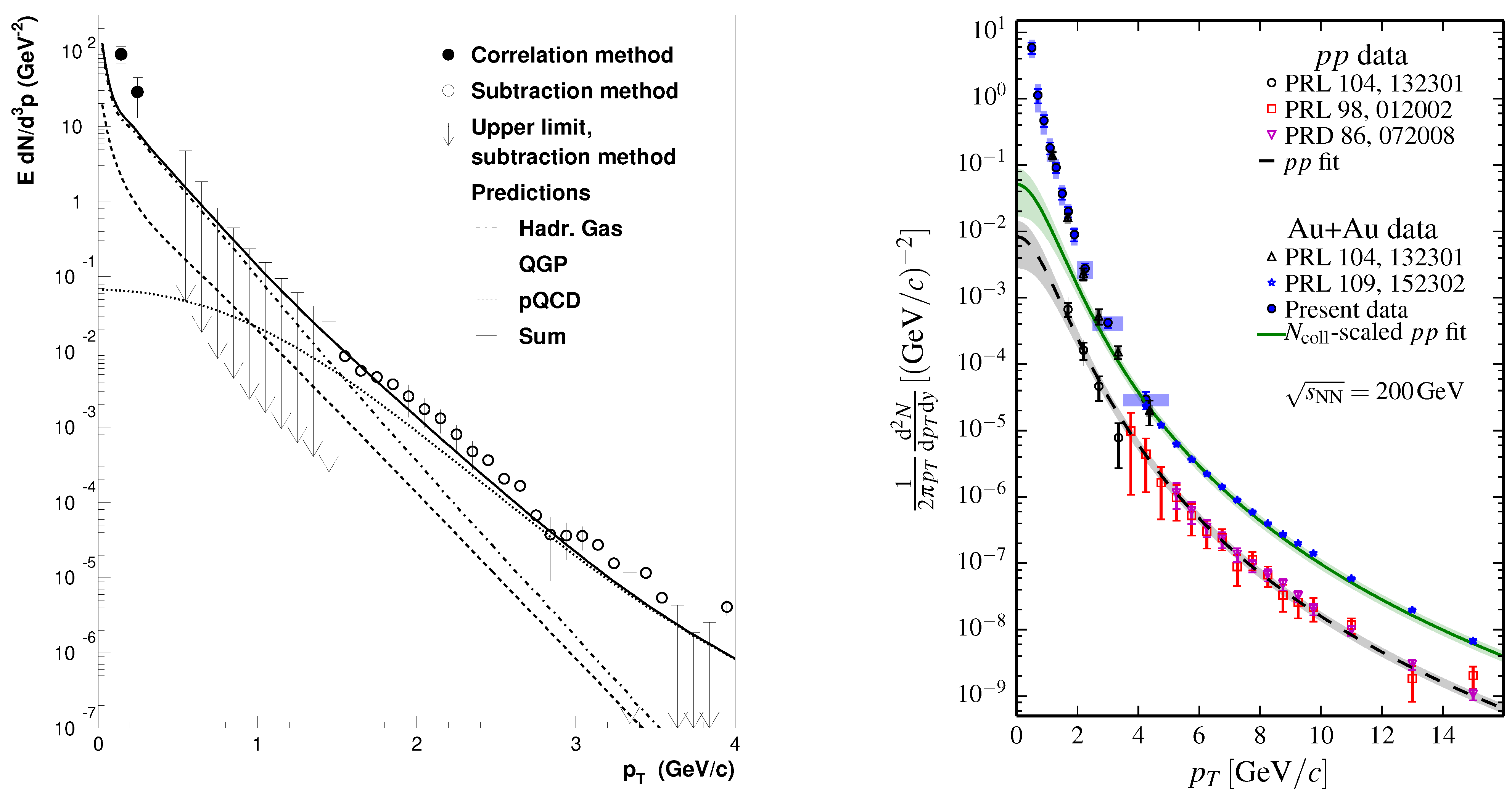
3. Statistical Method
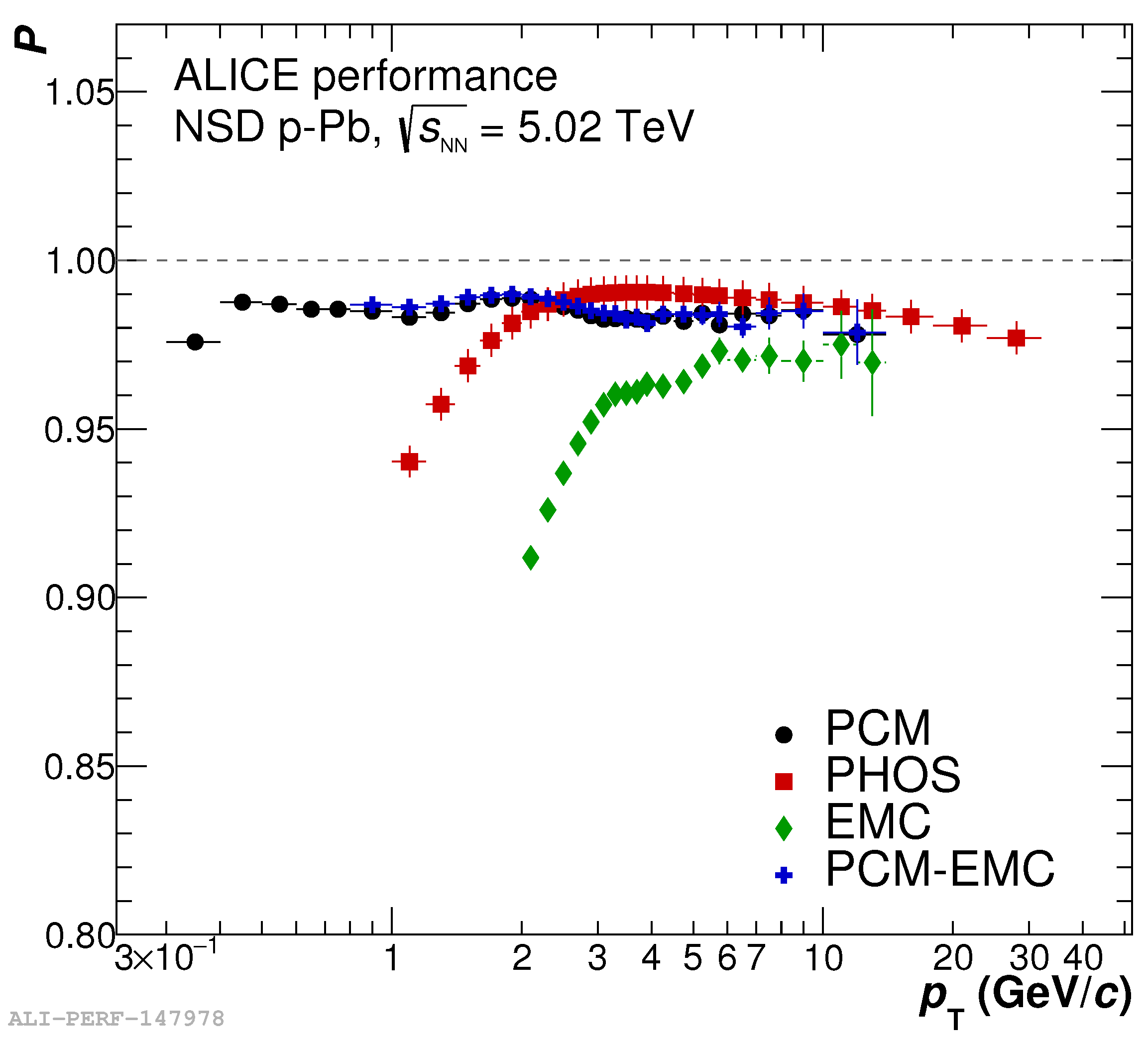
4. Photon Conversion Method
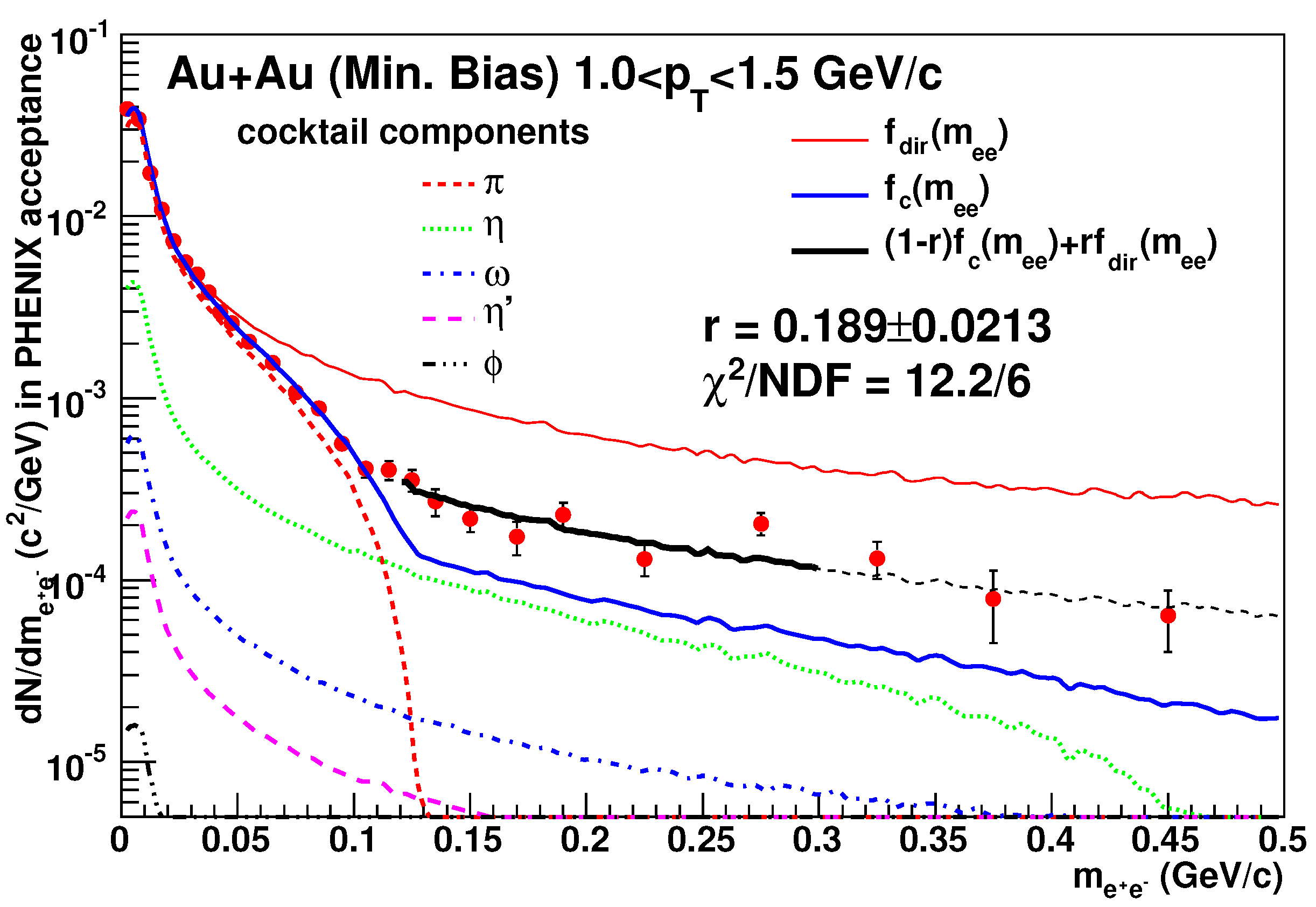
5. Internal Conversion Method
6. Bose–Einstein Correlations of Direct Photons
7. Method Comparison
8. Summary and Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Acharya, S.; Adamová, D.; Adler, A.; Adolfsson, J.; Aggarwal, M.M.; Aglieri Rinella, G.; Agnello, M.; Agrawal, N.; Ahammed, Z.; Ahmad, S.; et al. Measurement of isolated photon-hadron correlations in = 5.02 TeV pp and p-Pb collisions. Phys. Rev. C 2020, 102, 044908. [Google Scholar] [CrossRef]
- Aad, G.; Abajyan, T.; Abbott, B.; Abdallah, J.; Khalek, S.A.; Abdelalim, A.A.; Abdinov, O.; Aben, R.; Abi, B.; Abolins, M.; et al. Measurement of the inclusive isolated prompt photon cross section in pp collisions at = 7 TeV with the ATLAS detector. Phys. Rev. D 2011, 83, 052005. [Google Scholar] [CrossRef] [Green Version]
- Khachatryan, V.; Sirunyan, A.M.; Tumasyan, A.; Adam, W.; Bergauer, T.; Dragicevic, M.; Erö, J.; Fabjan, C.; Friedl, M.; Fruehwirth, R.; et al. Measurement of the Isolated Prompt Photon Production Cross Section in pp Collisions at = 7~TeV. Phys. Rev. Lett. 2011, 106, 082001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Acharya, S.; Adamová, D.; Adhya, S.P.; Adler, A.; Adolfsson, J.; Aggarwal, M.M.; Rinella, G.A.; Agnello, M.; Agrawal, N.; Ahammed, Z.; et al. Measurement of the inclusive isolated photon production cross section in pp collisions at = 7 TeV. Eur. Phys. J. C 2019, 79, 896. [Google Scholar] [CrossRef]
- Acharya, S.; Acosta, F.T.; Adamova, D.; Adolfsson, J.; Aggarwal, M.M.; Rinella, G.A.; Agnello, M.; Agrawal, N.; Ahammed, Z.; Ahn, S.U.; et al. Direct photon production at low transverse momentum in proton-proton collisions at = 2.76 and 8 TeV. Phys. Rev. C 2019, 99, 024912. [Google Scholar] [CrossRef] [Green Version]
- Aggarwal, M.M.; Agnihotri, A.; Ahammed, Z.; Angelis, A.L.S.; Antonenko, V.; Arefiev, V.; Astakhov, V.; Avdeitchikov, V.; Awes, T.C.; Baba, P.V.K.S.; et al. Observation of direct photons in central 158-A-GeV Pb-208 + Pb-208 collisions. Phys. Rev. Lett. 2000, 85, 3595–3599. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dellacasa, G.; Ramello, L.; Scalas, E.; Sitta, M.; Soave, C.; Ahmad, N.; Ahmad, S.; Ahmad, T.; Baritak, W.; Irfan, M.; et al. ALICE Technical Design Report of the Photon Spectrometer (PHOS). 1999. Available online: https://www.researchgate.net/publication/247768400_Alice_Technical_Design_Report_of_the_Photon_Spectrometer_PHOS (accessed on 3 May 2022).
- Cortese, P.; Dellacasa, G.; Gemme, R.; Ramello, L.; Sitta, M.; Ahmad, A.; Ahmad, N.; Danish Azmi, M.; Irfan, M.; Kamal, A.; et al. ALICE Electromagnetic Calorimeter Technical Design Report. 2008. Available online: https://cds.cern.ch/record/1121574 (accessed on 3 May 2022).
- Adare, A.; Afanasiev, S.; Aidala, C.; Ajitanand, N.N.; Akiba, Y.; Akimoto, R.; Al-Bataineh, H.; Al-Ta’ani, H.; Alexander, J.; Angerami, A.; et al. Centrality dependence of low-momentum direct-photon production in Au+Au collisions at = 200 GeV. Phys. Rev. C 2015, 91, 064904. [Google Scholar] [CrossRef] [Green Version]
- Schmidt, N. Direct Photon and Light Neutral Meson Production in Hadron Collisions at the LHC with ALICE. Ph.D. Thesis, Goethe University, Frankfurt am Main, Germany, 2021. [Google Scholar]
- Kroll, N.M.; Wada, W. Internal pair production associated with the emission of high-energy gamma rays. Phys. Rev. 1955, 98, 1355–1359. [Google Scholar] [CrossRef]
- Adare, A.; Afanasiev, S.; Aidala, C.; Ajitanand, N.N.; Akiba, Y.; Al-Bataineh, H.; Alexander, J.; Al-Jamel, A.; Aoki, K.; Aphecetche, L.; et al. Detailed measurement of the e+e− pair continuum in p+p and Au+Au collisions at = 200 GeV and implications for direct photon production. Phys. Rev. C 2010, 81, 034911. [Google Scholar] [CrossRef] [Green Version]
- Aggarwal, M.M.; Ahammed, Z.; Angelis, A.L.S.; Antonenko, V.G.; Arefev, V.; Astakhov, V.A.; Avdeichikov, V.; Awes, T.C.; Baba, P.V.K.S.; Badyal, S.K.; et al. Interferometry of direct photons in central Pb-208+Pb-208 collisions at 158-A-GeV. Phys. Rev. Lett. 2004, 93, 022301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bourhis, L.; Fontannaz, M.; Guillet, J.P. Quarks and gluon fragmentation functions into photons. Eur. Phys. J. C 1998, 2, 529–537. [Google Scholar] [CrossRef]
- Gluck, M.; Reya, E.; Vogt, A. Parton fragmentation into photons beyond the leading order. Phys. Rev. D 1993, 48, 116, Erratum in Phys. Rev. D 1995, 51, 1427. [Google Scholar] [CrossRef] [PubMed]
- Adare, A.; Afanasiev, S.; Aidala, C.; Ajitanand, N.N.; Akiba, Y.; Al-Bataineh, H.; Alexander, J.; Aoki, K.; Aphecetche, L.; Asai, J.; et al. Direct-Photon Production in p+p Collisions at = 200 GeV at Midrapidity. Phys. Rev. D 2012, 86, 072008. [Google Scholar] [CrossRef] [Green Version]


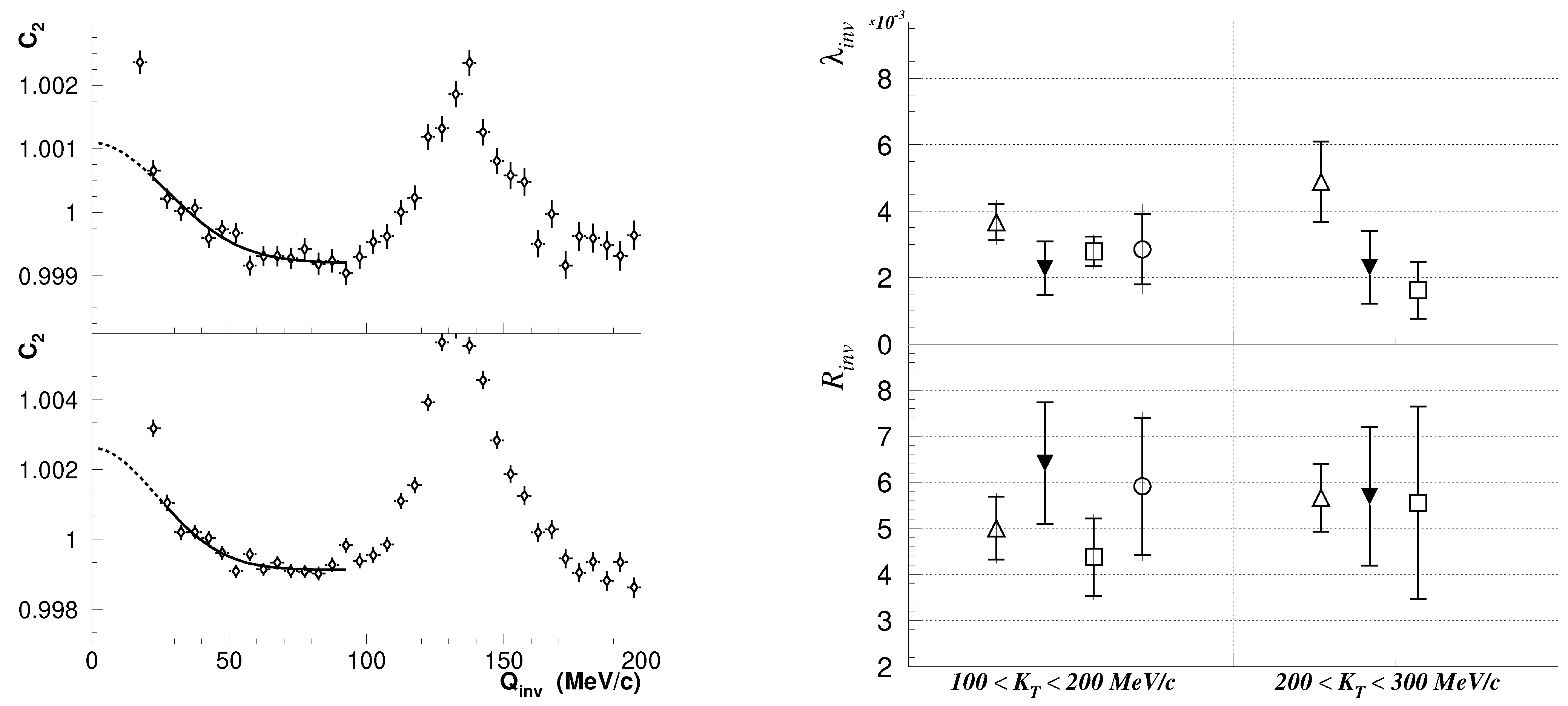

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peresunko, D. Approaches to Measuring Direct Photon Yield in A–A Collisions. Particles 2022, 5, 188-197. https://doi.org/10.3390/particles5020017
Peresunko D. Approaches to Measuring Direct Photon Yield in A–A Collisions. Particles. 2022; 5(2):188-197. https://doi.org/10.3390/particles5020017
Chicago/Turabian StylePeresunko, Dmitri. 2022. "Approaches to Measuring Direct Photon Yield in A–A Collisions" Particles 5, no. 2: 188-197. https://doi.org/10.3390/particles5020017
APA StylePeresunko, D. (2022). Approaches to Measuring Direct Photon Yield in A–A Collisions. Particles, 5(2), 188-197. https://doi.org/10.3390/particles5020017





