A Model for Assessing the Potential Impact Radius of Hydrogen Pipelines Based on Jet Fire Radiation
Abstract
1. Introduction
- (1)
- The pressure inside the hydrogen pipeline decays with time;
- (2)
- The rupture leads to a double-ended gas release.
2. Radiation Threshold for Potential Impact Radius
- (1)
- The people located outdoors when failure happens would be exposed to a low and finite chance of fatality.
- (2)
- The property represented by a typical wooden structure would not ignite and burn, thereby providing indefinite protection for people indoors when failure happens.
3. A Model for Assessing Potential Impact Radius
3.1. Equivalent Mass Release Rate
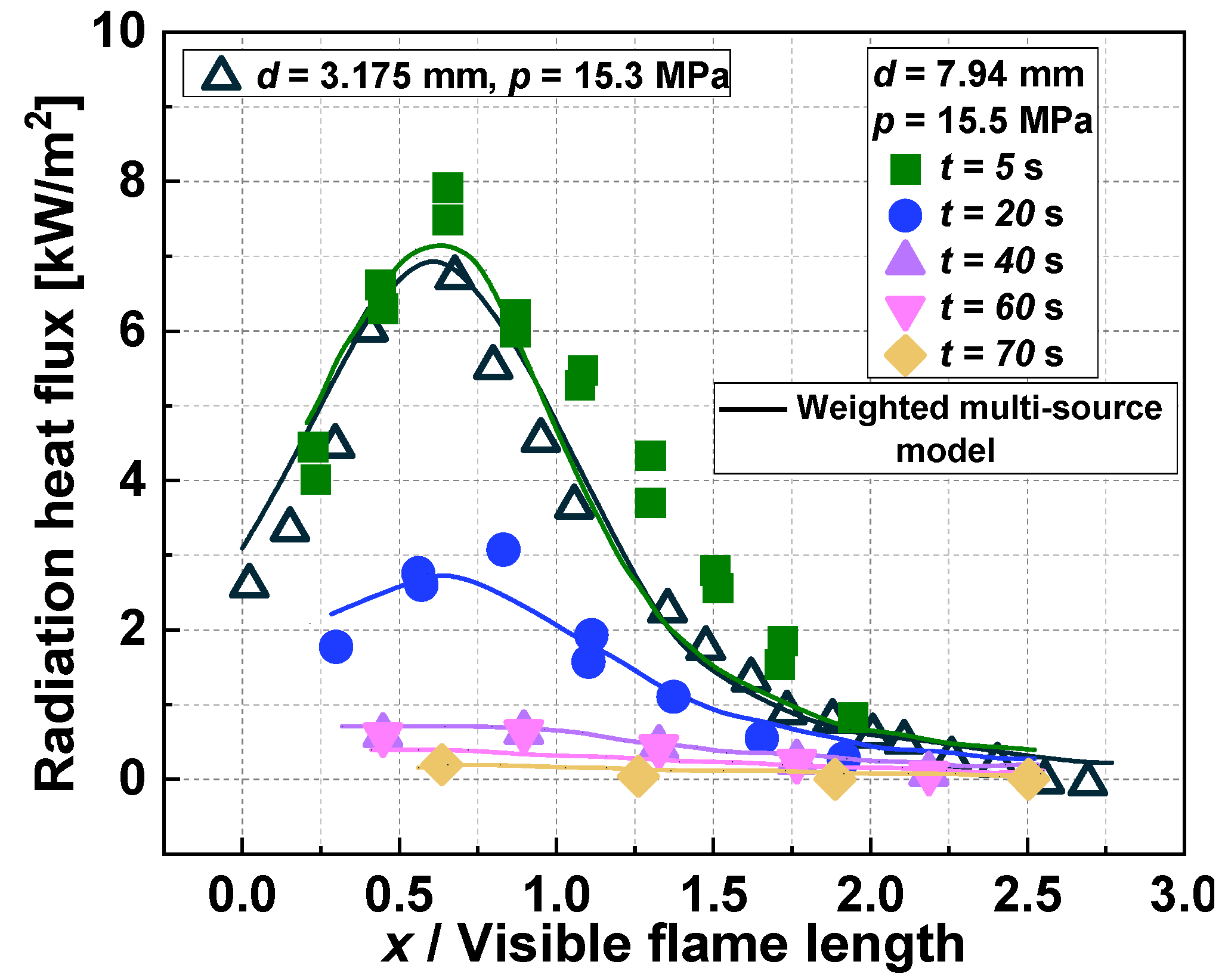
3.2. Flame Radiation Model
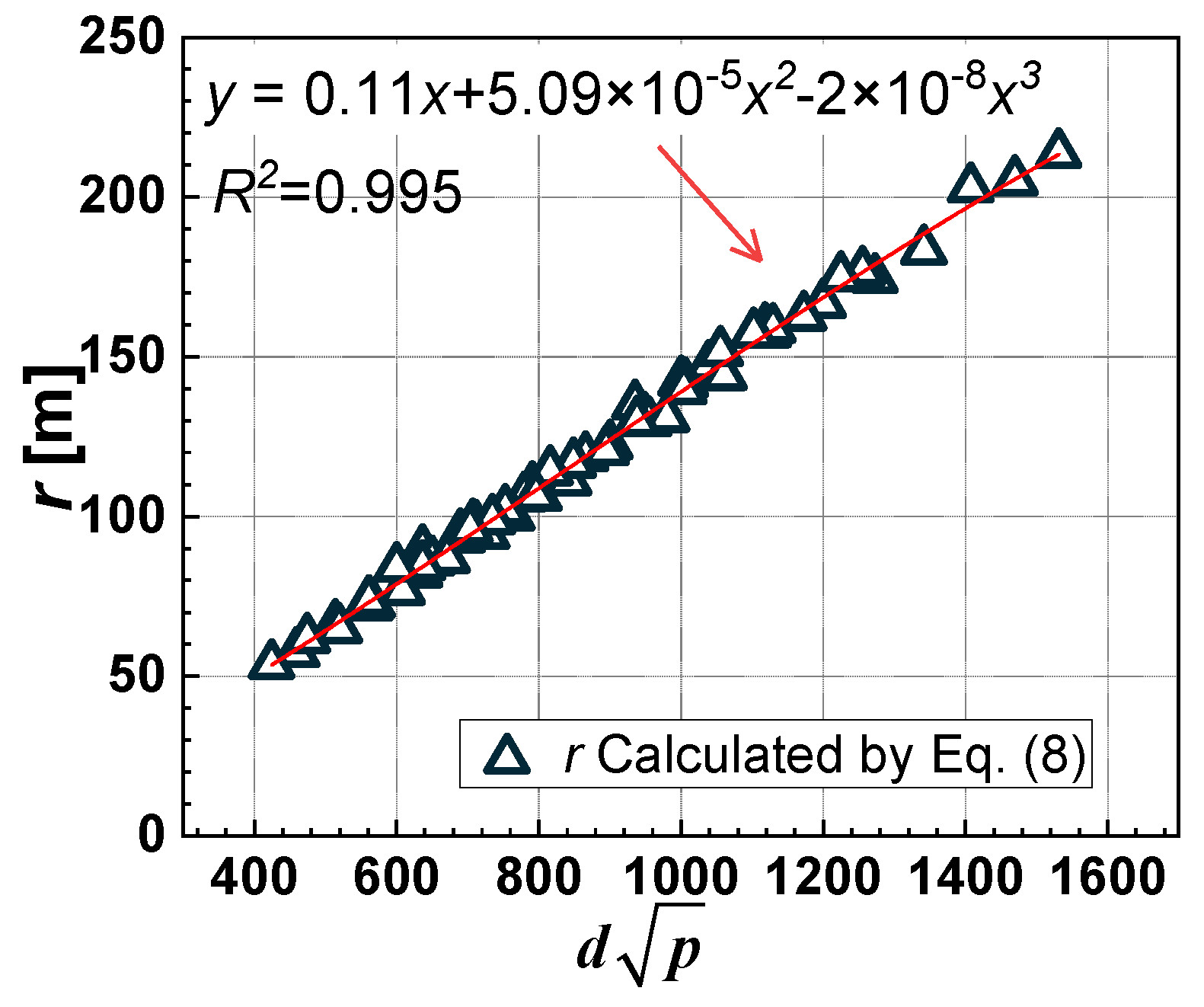
3.3. Potential Impact Radius for Hydrogen Pipelines
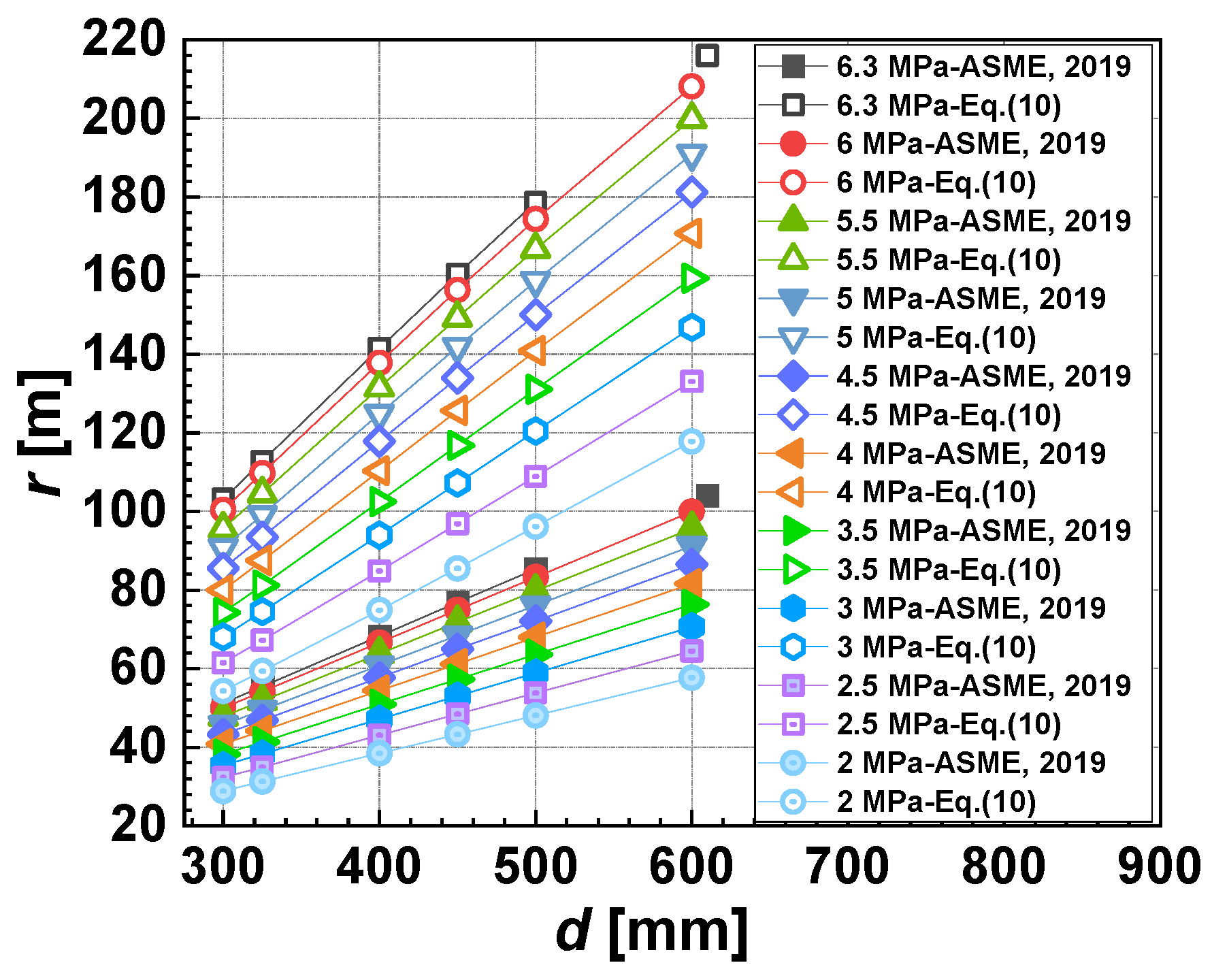
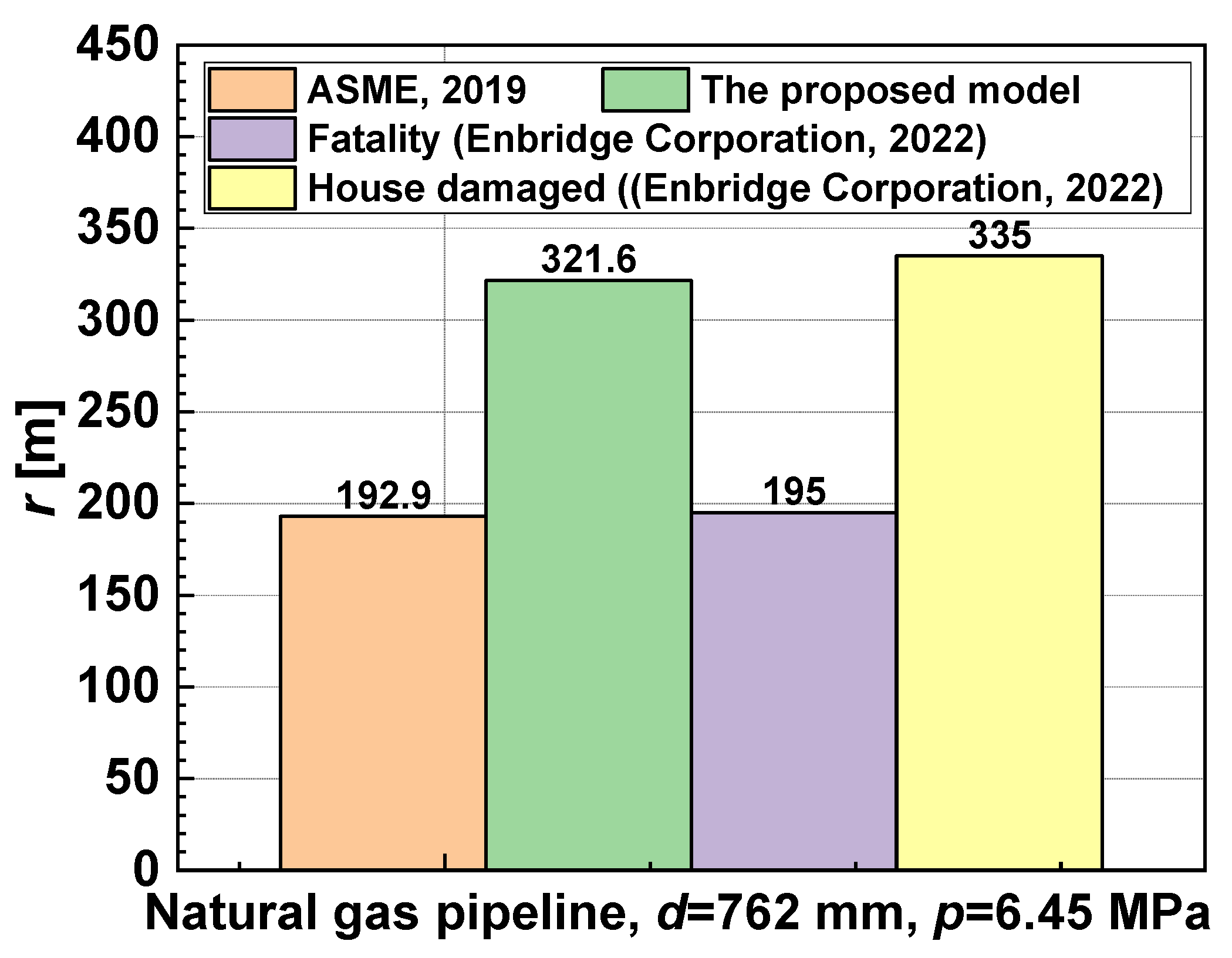
4. Results and Discussion
5. Conclusions
- (1)
- A model for assessing the potential impact radius is proposed, including an equivalent mass release rate that considers the pressure drop of the hydrogen pipeline leakage and a radiation model based on a weighted multi-source model;
- (2)
- A simplified correlation (Equation (10)) is proposed to calculate the potential impact radius and to provide a reference for industrial use. The proposed model consistently yields more accurate results than the single point source model. The validation against an actual pipeline leakage demonstrates good agreement with real-world scenarios.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Du, Y.; Liu, Y.; Zhou, F.; Li, Z.; Ma, L.; Liu, B. Evaluation of blast wave from hydrogen pipeline burst by a coupled fluid-structure-rupture approach. Int. J. Hydrogen Energy 2023, 55, 696–703. [Google Scholar] [CrossRef]
- Faye, O.; Szpunar, J.; Eduok, U. A critical review on the current technologies for the generation, storage, and transportation of hydrogen. Int. J. Hydrogen Energy 2022, 47, 13771–13802. [Google Scholar] [CrossRef]
- Crowl, D.; Jo, Y.D. The hazards and risks of hydrogen. J. Loss Prevent Proc. 2007, 20, 158–164. [Google Scholar] [CrossRef]
- Yu, X.; Kong, D.; He, X.; Ping, P. Risk Analysis of Fire and Explosion of Hydrogen-Gasoline Hybrid Refueling Station Based on Accident Risk Assessment Method for Industrial System. Fire 2023, 6, 181. [Google Scholar] [CrossRef]
- Virtue, B.; Salehi, F.; Abbassi, R. Safety Assessment of Hydrogen Jet Fire Scenarios within Semi-Confined Spaces. Fire 2023, 6, 29. [Google Scholar] [CrossRef]
- Ma, Q.; He, Y.; You, J.; Chen, J.; Zhang, Z. Probabilistic risk assessment of fire and explosion of onboard high-pressure hydrogen system. Int. J. Hydrogen Energy 2023, 50, 1261–1273. [Google Scholar] [CrossRef]
- Zhang, J.; Shi, M.; Lang, X.; You, Q.; Jing, Y.; Huang, D.; Dai, H.; Kang, J. Dynamic risk evaluation of hydrogen station leakage based on fuzzy dynamic Bayesian network. Int. J. Hydrogen Energy 2023, 50, 1131–1145. [Google Scholar] [CrossRef]
- Wang, X.; Gao, W. Hydrogen leakage risk assessment for hydrogen refueling stations. Int. J. Hydrogen Energy 2023, 48, 35795–35808. [Google Scholar] [CrossRef]
- Ramon, S.; Marcelo, H.; Adiel, T. Portfolio-based decision model for enhancing the mitigation of multidimensional risks in hydrogen pipeline sections. Process Saf. Environ. 2023, 175, 700–714. [Google Scholar]
- Francisco, F.; Marcelo, H.; Rodrigo, J.; Adiel, T. Multidimensional risk assessment and categorization of hydrogen pipelines. Int. J. Hydrogen Energy 2022, 47, 18424–18440. [Google Scholar]
- Bedford, T.; Wilson, K.; Daneshkhah, A. Assessing parameter uncertainty on coupled models using minimum information methods. Int. J. Hydrogen Energy 2014, 125, 3–12. [Google Scholar] [CrossRef][Green Version]
- Lins, P.; Almeida, A. Multidimensional risk analysis of hydrogen pipelines. Int. J. Hydrogen Energy 2012, 37, 13545–13554. [Google Scholar] [CrossRef]
- Stephens, M.; Leewis, K.; Moore, D. A Model for Sizing High Consequence Areas Associated with Natural Gas Pipelines. In Proceedings of the 4th International Pipeline Conference, Calgary, AB, Canada, 29 September–3 October 2002. [Google Scholar]
- Knowles, E.; Tweedle, F.; Van, L. The Background and Implications of IGE/TD/1 Edition 2; Gas Engineering and Management: London, UK, 1978; p. 247. [Google Scholar]
- Institute of Gas Engineers. Steel Pipelines for High Pressure Gas Transmission. Recommendations on Transmission and Distribution Practice IGE/TD/1 Edition 4; The Institution of Gas Engineers: London, UK, 1977. [Google Scholar]
- American Society of Mechanical Engineers. Hydrogen Piping and Pipelines; American Society of Mechanical Engineers: New York, NY, USA, 2019. [Google Scholar]
- Wang, C.; Wen, J.; Chen, Z.; Dembele, S. Predicting radiative characteristics of hydrogen and hydrogen/methane jet fires using FireFOAM. Int. J. Hydrogen Energy 2014, 39, 20560–20569. [Google Scholar] [CrossRef]
- Ehrhart, B.; Hecht, E. Hydrogen Plus Other Alternative Fuels Risk Assessment Models (HyRAM+) Version 4.1 Technical Reference Manual; SAND2022-5649; Sandia National Laboratories: Albuquerque, NW, USA, 2022.
- Miller, D. New model for predicting thermal radiation from flares and high pressure jet fires for hydrogen and syngas. Process Saf. Prog. 2017, 36, 237–251. [Google Scholar] [CrossRef]
- Bilo, M.; Kinsman, P. Thermal Radiation Criteria Used in Pipeline Risk Assessment. Pipes Pipelines Int. 1997, 42, 17–25. [Google Scholar]
- Eisenberg, N.; Lynch, C.; Breeding, R. Vulnerability Model: A Simulation System for Assessing Damage Resulting from Marine Spills. In Environmental Control; Report CG-D-136-75; Transportion Research Board: Rockville, MD, USA, 1975. [Google Scholar]
- Crane, C. Flow of Fluids through Valves, Fittings and Pipe, Metric Edition—SI Units; Technical Paper No. 410M; Crane: New York, NY, USA, 1981. [Google Scholar]
- TNO. Safety Study on the Transportation of Natural Gas and LPG by Underground Pipeline in the Netherlands; Netherlands Organization for Applied Scientific Research, Ref. No. 82-04180, File No. 8727-50960 [R]; Translation of a Report by the Division of Technology for Society; The Minister of Public Health and Environmental Hygiene: Enthe Hague, The Netherlands, 1982.
- Hankinson, G.; Lowesmith, B. A consideration of methods of determining the radiative characteristics of jet fires. Combust. Flame 2012, 159, 1165–1177. [Google Scholar] [CrossRef]
- Wayne, F. An economical formula for calculating atmospheric infrared transmissivities. J. Loss Prevent Proc. 1991, 4, 86–92. [Google Scholar] [CrossRef]
- Ekoto, I. Updated jet flame radiation modeling with buoyancy corrections. Int. J. Hydrogen Energy 2014, 39, 20570–20577. [Google Scholar] [CrossRef]
- Ehrhart, B.; Hecht, E.; Mohmand, J. Validation and Comparison of HyRAM Physics Models’ Sandia Report SAND2010-8676; US Department of Energy: Washington, DC, USA, 2021.
- Houf, W.; Schefer, R. Predicting radiative heat fluxes and flammability envelopes from unintended releases of hydrogen. Int. J. Hydrogen Energy 2007, 32, 136–151. [Google Scholar] [CrossRef]
- Enbridge Corporation. Natural Gas Transmission Pipeline Rupture and Fire (Pipeline Investigation Report: NTSB/PIR-22/02); Enbridge Corporation: Hillsboro, KY, USA, 2022. [Google Scholar]
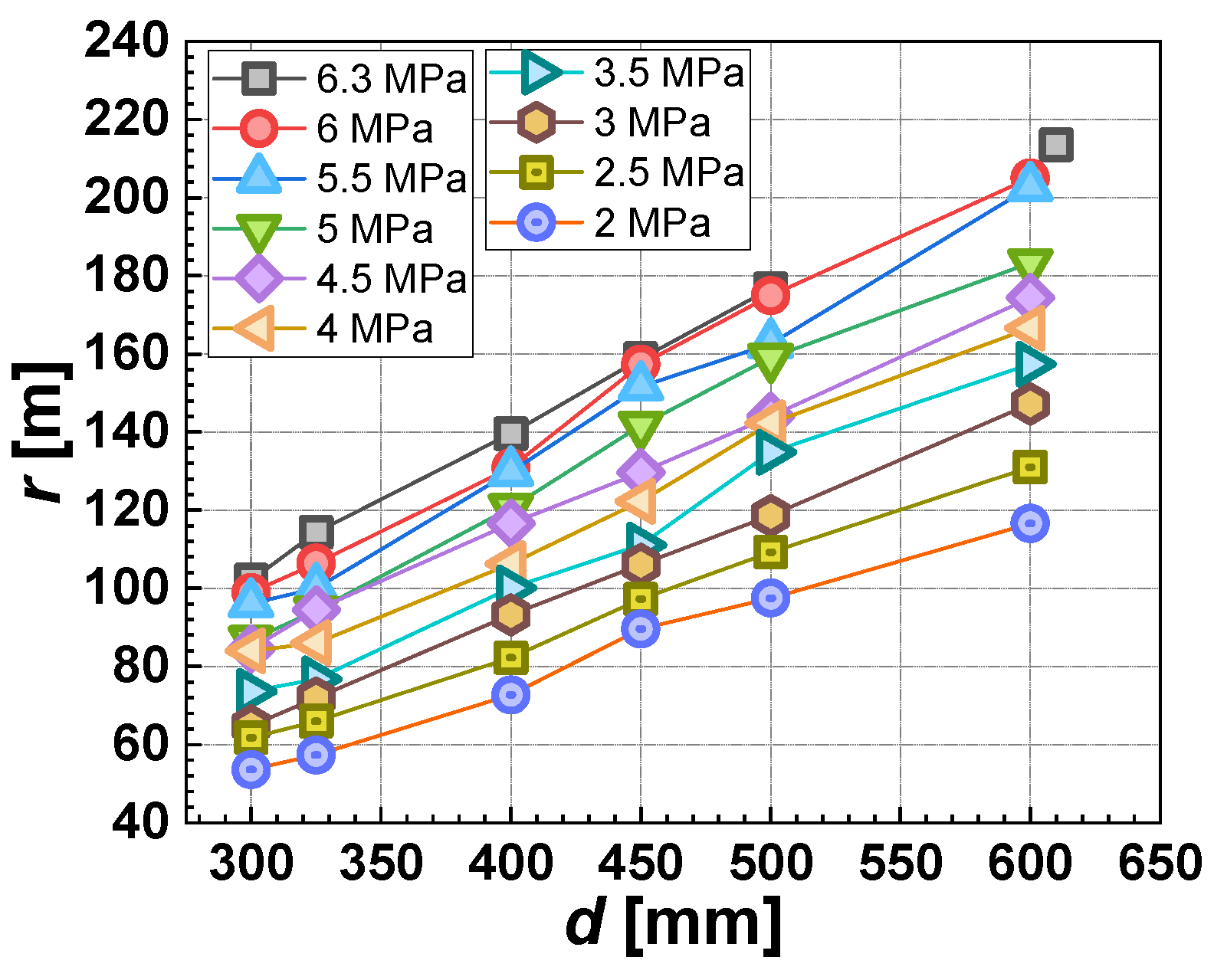
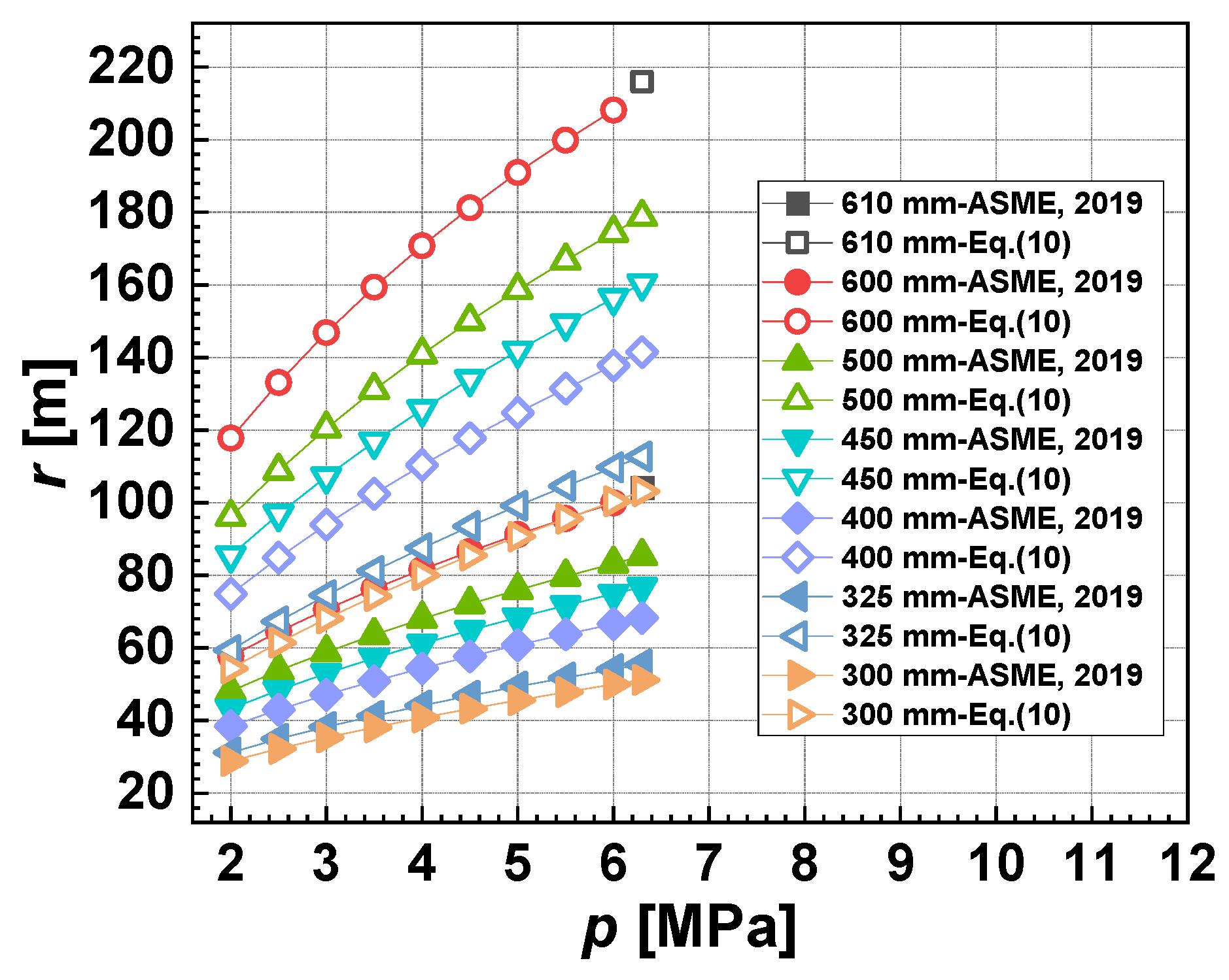
| Operation Pressure (MPa) | Pipeline Diameter (mm) |
|---|---|
| 6.3, 6, 5.5, 5, 4.5, 4, 3.5, 3, 2.5, 2 | 610, 600, 500, 450, 400, 325, 300 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, Y.; Yu, A.; Liu, Y.; Liu, X.; Zhang, Y.; Kuang, C.; Lu, Y.; Dang, W. A Model for Assessing the Potential Impact Radius of Hydrogen Pipelines Based on Jet Fire Radiation. Fire 2024, 7, 38. https://doi.org/10.3390/fire7020038
Lin Y, Yu A, Liu Y, Liu X, Zhang Y, Kuang C, Lu Y, Dang W. A Model for Assessing the Potential Impact Radius of Hydrogen Pipelines Based on Jet Fire Radiation. Fire. 2024; 7(2):38. https://doi.org/10.3390/fire7020038
Chicago/Turabian StyleLin, Yujie, Anfeng Yu, Yi Liu, Xiaolong Liu, Yang Zhang, Chen Kuang, Yuan Lu, and Wenyi Dang. 2024. "A Model for Assessing the Potential Impact Radius of Hydrogen Pipelines Based on Jet Fire Radiation" Fire 7, no. 2: 38. https://doi.org/10.3390/fire7020038
APA StyleLin, Y., Yu, A., Liu, Y., Liu, X., Zhang, Y., Kuang, C., Lu, Y., & Dang, W. (2024). A Model for Assessing the Potential Impact Radius of Hydrogen Pipelines Based on Jet Fire Radiation. Fire, 7(2), 38. https://doi.org/10.3390/fire7020038