An Alternative Approach for Predicting the Shelf Life of Energetic Materials

Abstract

The shelf life of energetic materials (EMs) is directly associated with safety and functionality. Therefore, a priori knowledge of this parameter is essential. The standard approach for predicting the shelf life of EMs is tremendously time and money consuming. It involves massive accelerated aging tests at temperatures typically between 40 and 80 °C for relatively long time periods—from months to years—with different aging time intervals, followed by analysis of the aging-induced changes. A subsequent kinetic analysis with Arrhenius evaluation provides the effective activation energy for calculating shelf life at lower storage temperatures. In this work, a much less time- and resource-intensive approach based on the kinetic analysis of decomposition data gathered by using thermal analysis techniques is discussed as a possible alternative for the shelf life prediction of EMs. The discussion is placed in the context of the few but promising works of literature on the subject that provide evidence and examples. On the path towards the practical application of this approach, the definition of procedures that allow for a realistic simulation of storage conditions not only in the accelerated aging tests—still needed but limited to the validation of the decomposition kinetics—but also in the thermal analysis experiments is highlighted as one of the main issues to be addressed.

1. Introduction

The existence of energetic materials (EMs) (i.e., explosives, propellants, and pyrotechnics) is only due to kinetic reasons—from a thermodynamic point of view, EMs are unstable substances. For most of them, slow chemical decomposition already occurs at room temperature and even more so at elevated temperatures. The chemical nature of the substance dictates its thermal stability. Aging of organic EMs often starts with unimolecular (homolytic) decay of the weakest bond, followed and then accompanied by self-accelerating parallel reactions involving the formed decomposition products—the activation energy of the homolytic decomposition can therefore be considered a good indicator of the thermal stability of the respective EM [1]. As a rule of thumb, EMs with an activation energy of decomposition higher than 170 kJ mol⁻¹ are stable for thousands of years at room temperature, whereas EMs with an activation energy of decomposition lower than 155 kJ mol⁻¹ have limited thermal stability [2].

Table 1. Thermal stability for different classes of organic EMs. (The values of activation energy were taken from [1]).

Class of Organic EMs	Activation Energy of Decomposition (kJ mol−1)	Thermal Stability
Aromatic nitro compounds	190–290	Relatively good
Aliphatic nitro compounds	230–260	Relatively good
Secondary nitramines	170–200	Relatively good
Organic azides	165–170	Relatively good
Aliphatic nitrate esters	155–190	Relatively poor 1

¹ This is especially true for nitrocellulose (NC) and nitroglycerine (NG) [1].

quantifies the thermal stability of different classes of organic EMs in terms of the activation energy of the homolytic decomposition. As can be seen from this table, aromatic and aliphatic nitro compounds, secondary nitramines, and organic azides are relatively stable, whereas aliphatic nitrate esters suffer from much lower stability.

The aging of EMs can affect their safety and functional features. The exposure of EMs to higher temperatures during storage (for instance, due to a fire) accelerates the aging process. The loss of thermal stability resulting from aging can lead to failure or accidental ignition with sometimes catastrophic consequences—think of the massive explosion that occurred at the port of Beirut in August 2020. Therefore, a priori knowledge of the shelf life of EMs, i.e., the time interval during which they can be stored, handled, and used without any danger [1], is essential.

Unfortunately, aging studies, which are relevant regarding assessing the safe and reliable use of EMs, cannot be conducted in a reasonable time at ambient temperature, at which the decomposition process usually proceeds at an extremely slow rate; thus, accelerated aging tests (i.e., artificial aging tests at higher temperatures) are generally used to reduce the time scale for such studies. The thermal stability of EMs can be investigated using different test methods based on accelerated aging. Vogelsanger [1] classified such methods according to the test structure and the type of aging phenomenon investigated, highlighting that the multi temperature aging method is the only method that allows for predicting shelf life. This method has the most elaborate test structure. It involves massive accelerated aging tests at temperatures typically between 40 and 80 °C for relatively long time periods—from months to years—with different aging time intervals, followed by analysis of the aging-induced changes. A subsequent kinetic analysis with Arrhenius evaluation provides the effective activation energy for calculating shelf life at lower storage temperatures. Ref. [3] describes a practical example of the application of this method to a nitrocellulose-based propellant whose accelerated aging process was tracked using measurements of the content of the stabilizer performed by high performance liquid chromatography (HPLC) analysis. Specifically, the content of the stabilizer was measured after accelerated aging of the propellant for up to 6, 23, and 91 days at 80, 70, and 60 °C, respectively. The kinetic evaluation gave the best fit for reaction order n = 0.58. The obtained activation energy value (135.3 kJ mol⁻¹) allowed reliable extrapolation to lower temperatures. Thus, a shelf life of 41 years at 25 °C was calculated, with the failure criterion defined as a 50% decrease in the content of the stabilizer.

The multi temperature aging method suffers from some important limitations, including its difficult application to non-isothermal aging (real life storage conditions often include a complex history of temperature variations—think, for example, of the diurnal and seasonal temperature variations) [4]. Furthermore, as can be easily inferred from the above discussion, it is tremendously time and money consuming [1]. More effective approaches for predicting the shelf life of EMs are therefore necessary.

Different thermal analysis techniques, such as thermogravimetry (TG), differential thermal analysis (DTA), and differential scanning calorimetry (DSC), have been widely used to investigate the decomposition of EMs, even as simultaneous techniques (i.e., TG/DTA and TG/DSC) and in combination with mass spectrometry (MS) and/or Fourier-transform infrared spectroscopy (FTIR) (these latter for identifying the reaction products) [5,6,7]. These techniques are usually operated under dynamic (i.e., non-isothermal) conditions and possess the advantage of a rapid reaction process. The kinetics of the thermal decomposition of EMs can be extracted from thermal analysis data and used to predict the shelf life—to this end, the failure threshold needs to be defined as a function of the limiting extent of conversion—once their reliability for extrapolation outside the range of calibration has been thoroughly assessed. This work aims at stimulating discussion on this approach, which is much less time and resource intensive than the previously described multi temperature aging method, as a possible alternative for the shelf life prediction of EMs. Evidence and examples to support this perspective are provided in the context of the literature on the subject (Section 2). The main implications for future research, from the authors’ point of view, are also highlighted (Section 3).

2. Evidence and Examples from the Literature

Evidence and examples to support the perspective outlined in this work come from few literature studies [8,9,10,11]. In such studies, both isoconversional [8,9] and model-based [10,11] methods were adopted to determine the decomposition kinetics of energetic materials (EMs) from the data of thermal analysis experiments performed under dynamic conditions at different heating rates. In the latter methods, the kinetic law is predetermined based on the thermal behavior of the substance under examination. This predetermination is not needed with the former methods. However, the isoconversional methods, also called “model-free” kinetic analyses, are not assumption-free methods. As highlighted for EMs of various classes by EL-Sayed [7] based on a review of about seventy literature works, the values of the kinetic parameters—pre-exponential factor and activation energy—can vary even significantly for the same material depending not only on the measurement technique but also on the adopted kinetic approach. The validation of the kinetics obtained from data gathered by using thermal analysis techniques, i.e., the assessment of their reliability for extrapolation outside the range of calibration, is therefore a key step, especially when they are intended to predict a very slow decomposition phenomenon such as the aging process. In this case, experimental aging data (i.e., low temperature, long term decomposition data) are needed.

In Refs. [8,9,10,11], kinetic predictions were compared with experimental data obtained on actual aged samples.

Table 2. Global overview of Refs. [8,9,10,11] in terms of investigated EMs, adopted kinetic approach, calculated kinetic predictions, and aging tests performed for validation. In the last column, remarks on the agreement between kinetic predictions and experimental aging data are also reported. “T” and “t” stand for temperature and time, respectively.

Ref.	EMs	Kinetic Approach	Kinetic Predictions	Aging Tests for Validation	Agreement
[8]	97.5% RDX, 95% HMX, and BPN	Isoconversional	Isothermal: reaction progress; T ranging from 30 to 180 °C; different t periods (even of 20 years). 1	Isothermal accelerated (only for BPN): T = 71 °C; aging t of 8, 16, 24, and 48 weeks.	Reasonable 2
[9]	TKX-50, RDX, HMX, CL-20, and PETN	Isoconversional	Isothermal: mass loss; T ranging from 0 to 100 °C; t period of 10 years. Climatic (of different countries): 3 mass loss; t period of 10 years.	Isothermal accelerated: T = 100 °C; aging t of 4, 14, and 28 days.	Consistency between kinetic predictions (of the mass loss) and aging data
[10]	NQ	Model-based	Isothermal: mass loss; T ranging from 150 to 210 °C; t period of 12 h.	Isothermal accelerated: T = 210 °C; aging t = 30 min.	Good
[11]	Picric Acid (PA)	Model-based	Isothermal: conversion; T ranging from 80 to 140 °C; different t periods (more than 90 years at 80 °C). 4	Isothermal accelerated: T ranging from 90 to 230 °C; aging t ranging from 25 min to 6 months. Non-isothermal accelerated: T following a complex history of variations between 120 and 265 °C over an aging t of 35 min. Natural aging: material stored at room temperature for more than 10 years.	Good 5

¹ Maximum storage temperature calculated, setting the reaction progress value of 0.01 after 20 years as the criterion for undecayed performance; ² Comparison limited to values of the remaining fraction of BPN very close to unity; ³ Six cities on four different continents, including Munich, Moscow, Washington D.C., Brasilia, Cairo, and Beijing, representing Europe, North America, South America, Africa, and Asia, respectively; ⁴ Shelf life calculated at T ranging from 20 to 140 °C, assuming 10% as the limiting extent of conversion; ⁵ Predictions also confirm that the decomposition of PA can occur at room temperature, but it is so slow that it becomes detectable only after thousands of years [2].

provides a global overview of these literature works in terms of investigated EMs, adopted kinetic approach, calculated kinetic predictions, and aging tests carried out for validating the kinetics at lower temperatures. In the last column of this table, remarks on the agreement between kinetic predictions and experimental aging data are also reported.

Kim et al. [8] applied an isoconversional method to differential scanning calorimetry (DSC) data to extract the kinetics of the thermal decomposition of 97.5% hexahydrotrinitrotriazine (RDX), 95% octahydrotetranitrotetrazine (HMX), and boron/potassium nitrate (BPN). Only this latter EM was subjected to isothermal accelerated aging tests at a single temperature of 71 °C for 8, 16, 24, and 48 weeks. There was agreement between experimental data and kinetic predictions that, even after 48 weeks, the remaining fraction of BPN was very close to unity.

In Ref. [9], the kinetic analysis of dihydroxylammonium-5,5′-bistetrazolyl-1,1′-diolat (TKX-50), RDX, HMX, hexanitrohexaazaisowurtzitane (CL-20), and pentaerythritoltetranitrate (PETN) was carried out applying an isoconversional method that fitted thermogravimetry (TG) data slightly better than a model-based method also investigated, but only for TKX-50. Isothermal predictions of the mass loss of each EM were calculated at various temperatures, and the predictions at 100 °C were compared with experimental mass loss data of samples subjected to isothermal accelerated aging at the same temperature for 4, 14, and 28 days. Consistency was found between kinetic predictions and experimental aging data.

Li and Cheng [10] investigated the thermal behavior of nitroguanidine (NQ) using simultaneous TG/DSC/mass spectrometry (MS)/Fourier-transform infrared spectroscopy (FTIR) analysis. They extracted the kinetics of the thermal decomposition of this EM in the frame of a model-based approach and used the kinetics to simulate the mass loss under isothermal conditions at a temperature of 210 °C for a total exposure time (i.e., an aging time) of 30 min. The simulated and TG curves almost overlapped. Isothermal simulations of the mass loss were also performed at lower temperatures (down to 150 °C) for a total exposure time of 12 h. Predictions show that before 170 °C, the mass loss rose to only 7%, whereas it shifted rapidly to much more after 170 °C. This is consistent with the change in the mechanism of the decomposition of NQ in the 160–170 °C temperature range deduced by Lee and Back [12] based on the comparison of the rate constants they obtained from accelerating rate calorimetry (ARC) experiments with literature rate constants derived from measurements performed using techniques other than ARC.

An extensive validation of kinetic predictions against experimental aging data was carried out in Ref. [11]. Specifically, attention was focused on picric acid (PA), an explosive belonging to the class of aromatic nitro compounds (like trinitrotoluene (TNT)). A schematic description of the adopted methodological path is shown in Figure 1. Dynamic DSC experiments were first performed at different heating rates. The gathered thermograms exhibited a single exothermic peak, and there was only one intersection point between curves collected at two different heating rates, confirming the autocatalytic nature of the process under examination [13,14]. A kinetic law consistent with this nature was thus assumed before extracting the kinetics of thermal decomposition—via a suitable procedure of parameter identification—from DSC data gathered at three different heating rates. After a preliminary validation against isothermal and further dynamic DSC experiments, kinetic predictions were compared with experimental aging data, i.e., the conversions of aged PA. Such data were obtained for PA subjected not only to isothermal accelerated aging—under such conditions, wide ranges of temperature (from 90 to 230 °C) and exposure time (from 25 min to 6 months) were explored—but also to non-isothermal accelerated aging (with temperature following a complex history of variations between 120 and 265 °C over a time period of 35 min) and natural aging (during more than 10 years of storage at room temperature). Another element of novelty introduced was the use of high performance liquid chromatography (HPLC) analysis—instead of heat release [8] or mass loss [9,10] measurements—to obtain the effective conversion of aged PA. 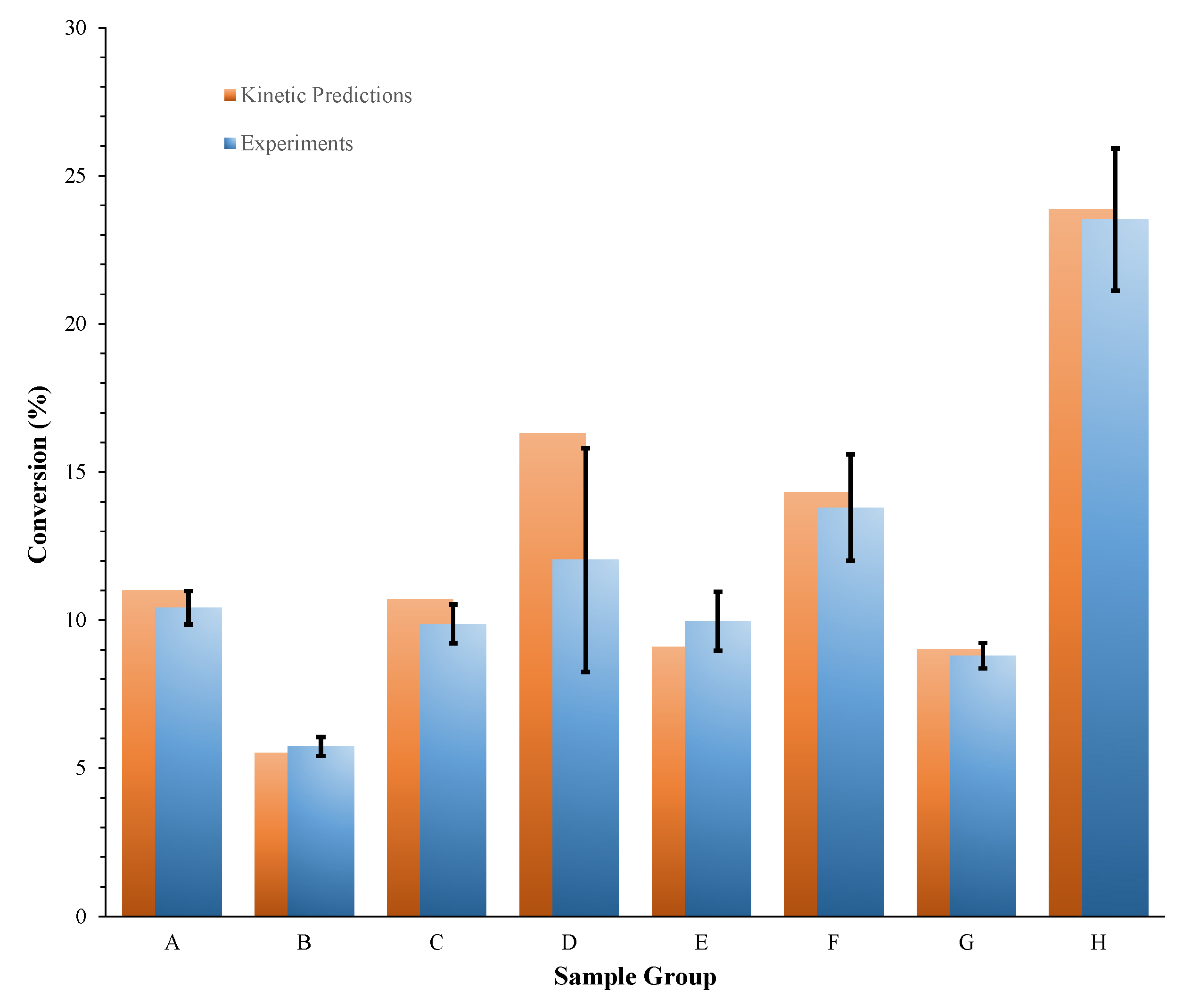Predictions were found to be in good agreement with experimental data; Figure 2 shows the comparison under high temperature isothermal conditions. For naturally aged PA, a negligible conversion was found—this was consistent with the almost overlap of the dynamic DSC thermograms recorded for a sample of this material and a sample of fresh (i.e., unaged) PA [15] (Figure 3). The good agreement between predictions and experimental aging data proves the reliability of the kinetics extracted for PA, also supporting the extension of the adopted model-based approach to the prediction of the shelf life of EMs that, like PA, decompose in an autocatalytic manner.

In the DSC experiments of Ref. [11], hermetically sealed stainless steel pans, which were able to withstand high internal pressure, were used as sample holders. The use of a closed system was mandatory with PA due to its great tendency to evaporate (the same behavior was also observed with TNT [14]). This approach is generally more conservative—the closed system retains the products of the decomposition that can cause a marked acceleration in the rate of decomposition [16,17]. However, not every EM obeys this rule. For example, for PETN, open systems—DSC with an open pan and TG/MS—were found to be more conservative than the closed system—DSC with a sealed pan [18].

Finally, it is worth highlighting that, in Ref. [11], the same sample holder was used in both DSC experiments and accelerated aging tests, and this represents a further point of strength for the performed validation.

3. Conclusions

Based on the literature results presented in the previous section, it can be concluded that the application of the kinetic analysis of decomposition data gathered by using thermal analysis techniques to the shelf life prediction of energetic materials (EMs) is worthy of further consideration. Overall, the studies carried out so far on this subject, although few in number, constitute a good premise, having shown consistency/agreement between kinetic predictions and experimental data obtained on actual aged samples. Starting from this premise, future research should broaden the range of investigated EMs, thoroughly assessing the reliability of kinetic predictions against aging data. This assessment is definitely the most delicate point to deal with as it involves accelerated aging tests. However, the efforts associated with such tests can be suitably optimized through a reasoned planning of the experimental campaign based on indications coming from the kinetic predictions themselves. For example, should test conditions result, even after relatively long aging times (months or years), in values of conversion not detectable by the available measurement techniques, then they would be rejected a priori. The same is true in the case of conversions well above the value corresponding to the failure threshold. From this perspective, the shelf life prediction of EMs via kinetic analysis of their thermal decomposition can be a viable alternative to the standard multi temperature aging method, which, in comparison, is much more time and resource intensive as it involves massive accelerated aging tests. On the path towards the practical application of this alternative approach, one of the main issues to be addressed is the definition of procedures that allow for a realistic simulation of storage conditions not only in the accelerated aging tests but also in the thermal analysis experiments. In this regard, several aspects come into play and should be carefully considered, including those related to the EM itself (degree of purity, particle size and morphology, moisture content, etc.), the atmosphere (inert or oxidative/reactive, wet or dry, etc.), the sample holder (open or closed, and, in this latter case, the verification of the tightness of the seal), the material of the sample holder and its possible interaction with the EM, the loading density, and the contact area.