Advanced Diagnostics of Electrons Escaping from Laser-Produced Plasma
Abstract
1. Introduction
2. Diagnostics for Low-Intensity Laser Interaction
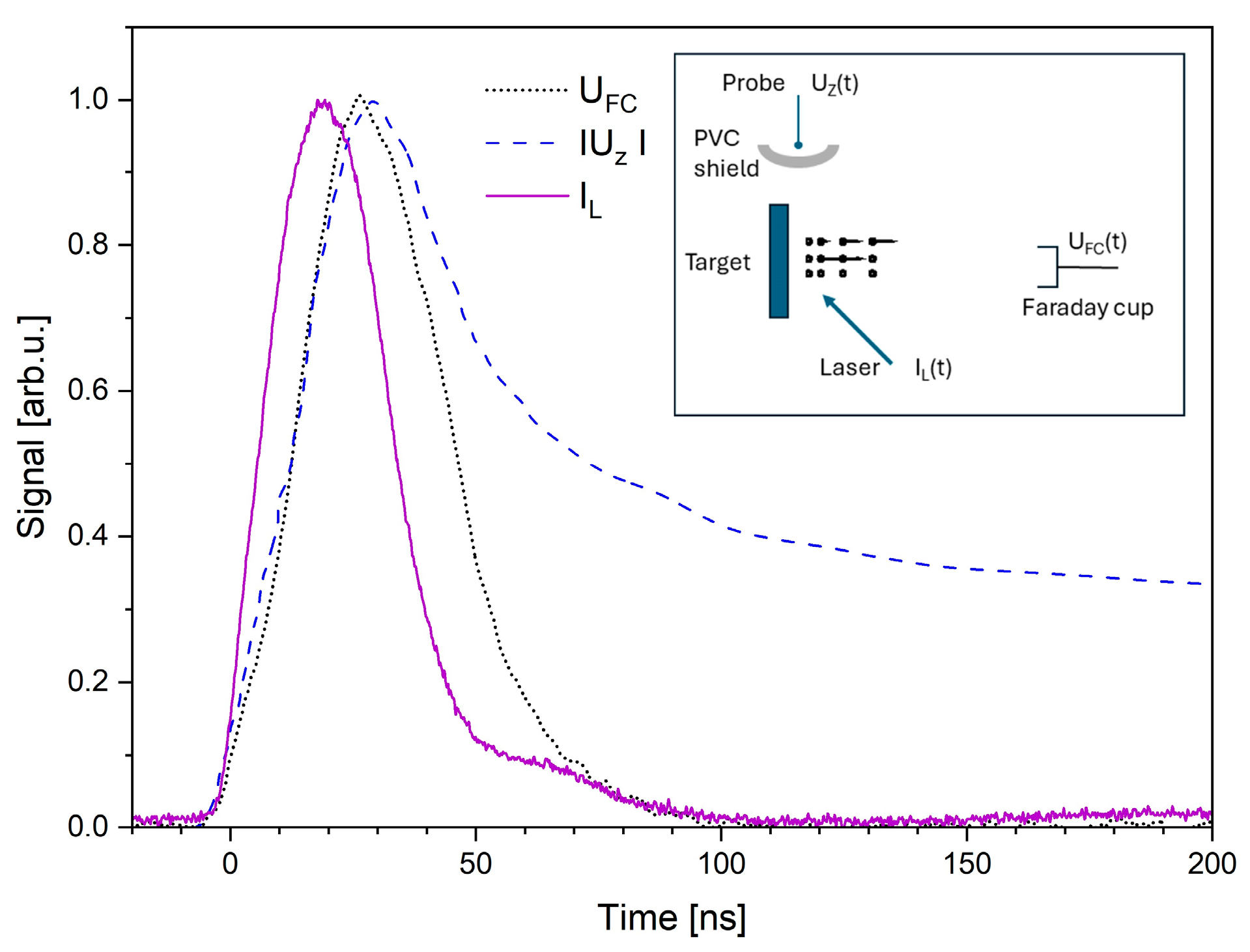
2.1. Electric Field near the Target
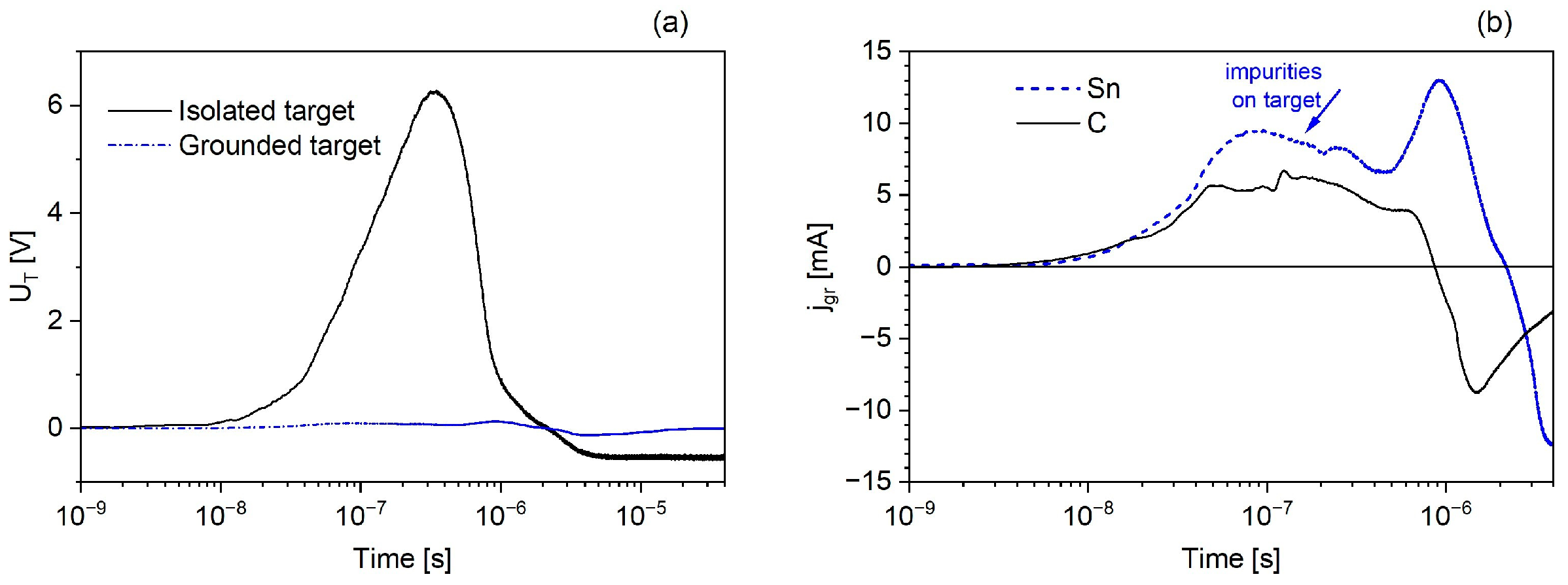
2.2. Return Target Current at Low Laser Intensity
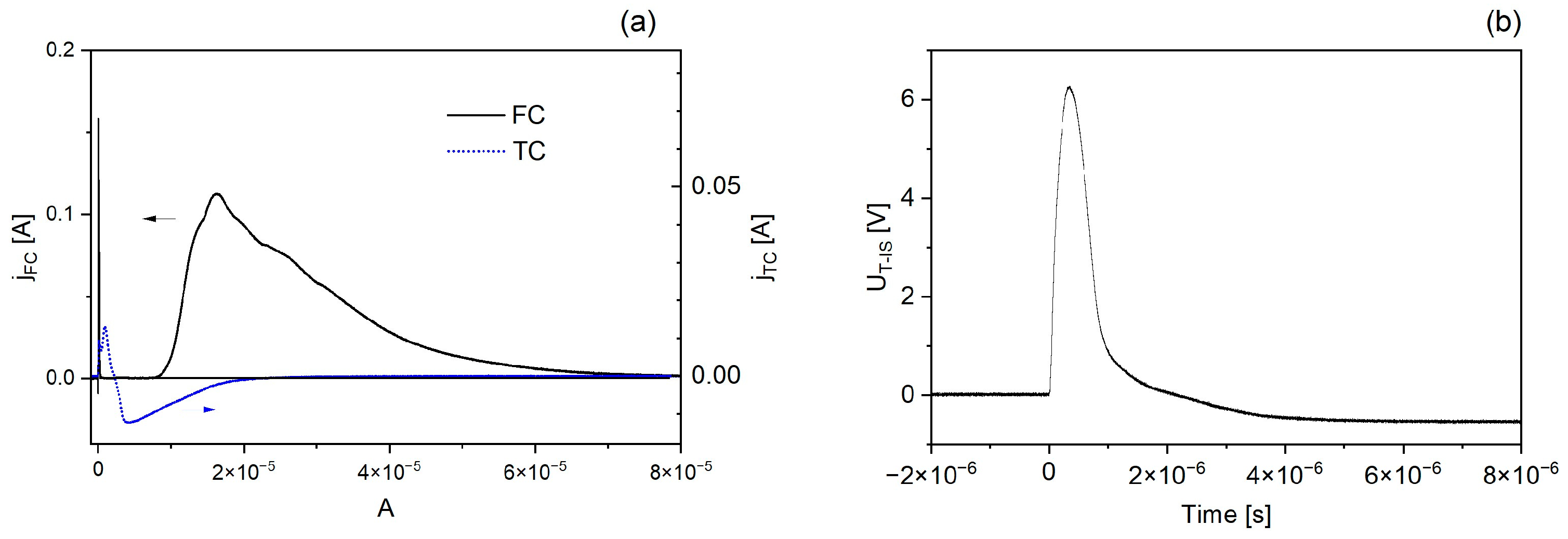
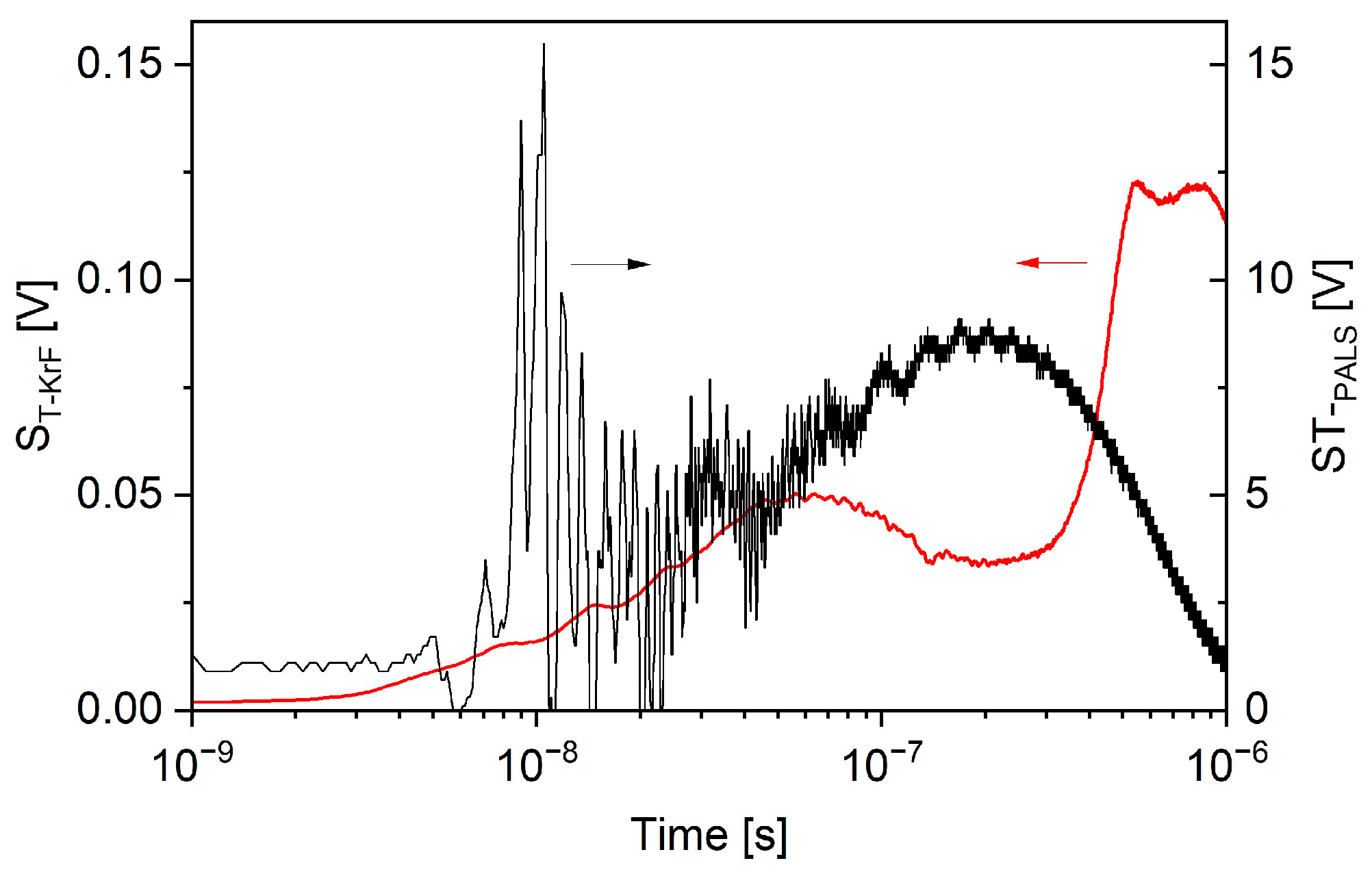
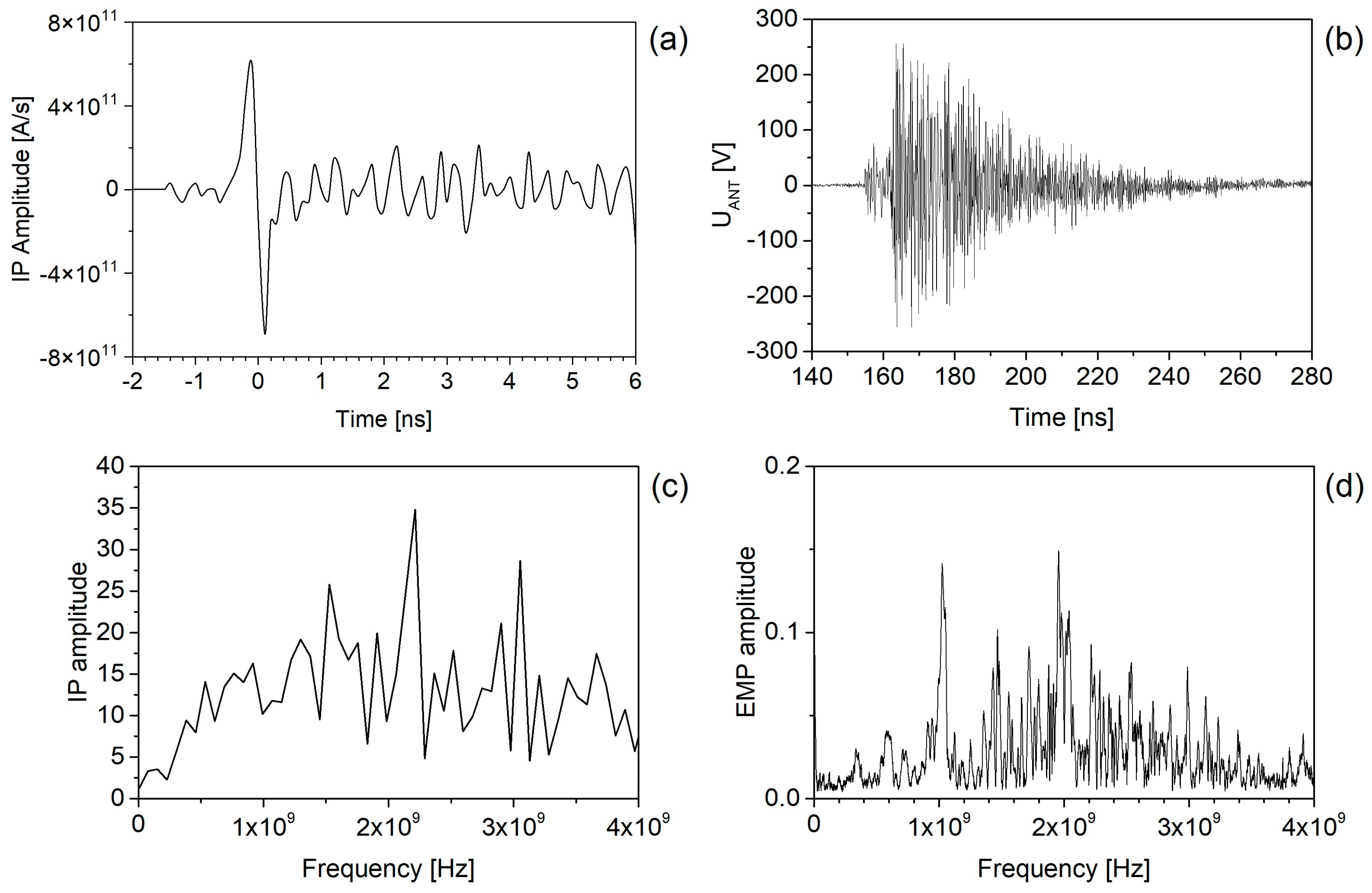
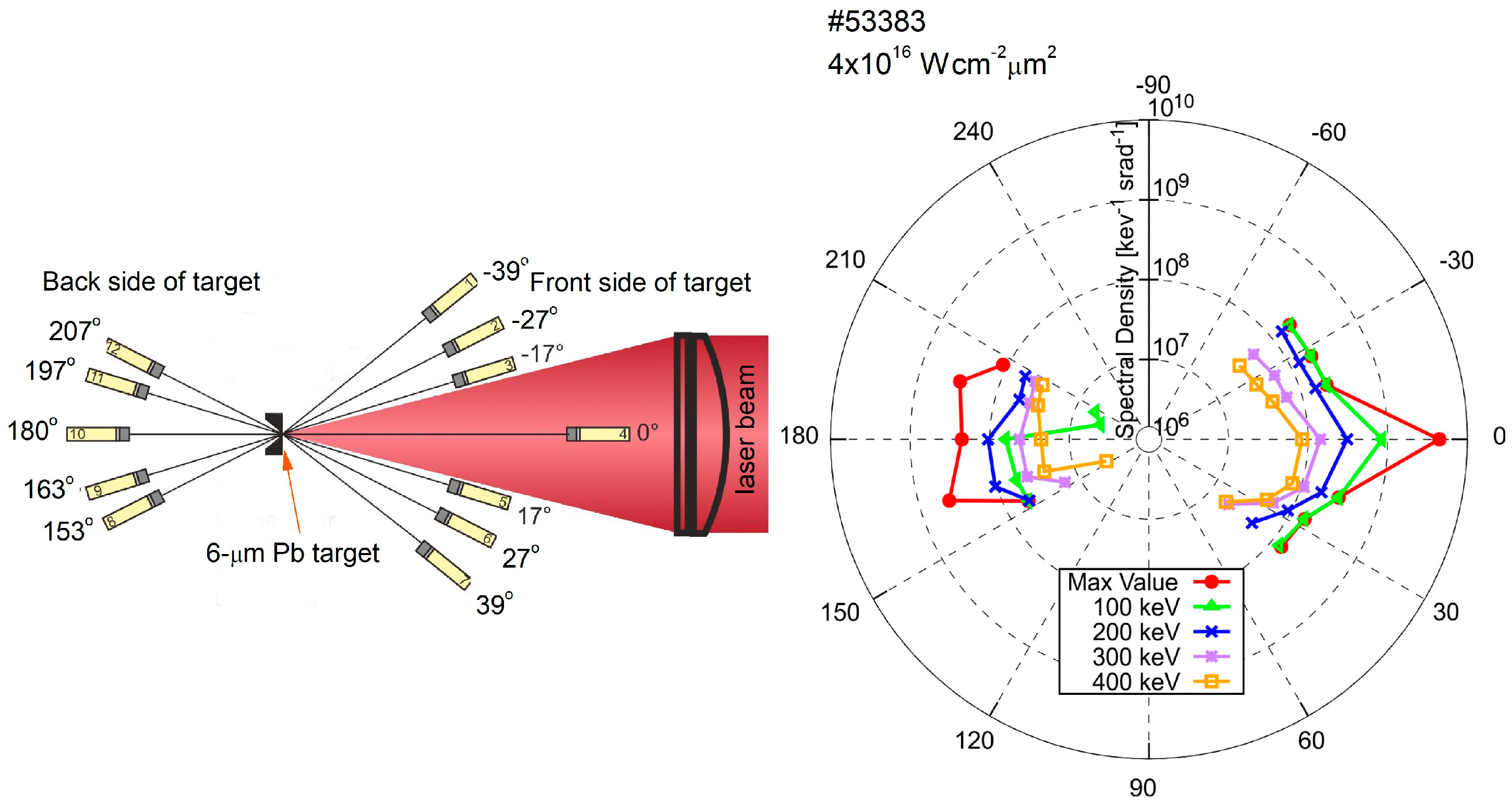
3. Diagnostics of Return Target Current at High Laser Intensity
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pathak, V.B.; Lee, S.K.; Pae, K.H.; Hojbota, C.I.; Kim, C.M.; Nam, C.H. Strong field physics pursued with petawatt lasers. AAPPS Bull. 2021, 31, 4. [Google Scholar] [CrossRef]
- Macchi, A.; Borghesi, M.; Passoni, M. Ion acceleration by superintense laser-plasma interaction. Rev. Mod. Phys. 2013, 85, 751–793. [Google Scholar] [CrossRef]
- Tajima, T.; Yan, X.Q.; Ebisuzak, T. Wakefield acceleration. Rev. Mod. Plasma Phys. 2020, 4, 7. [Google Scholar] [CrossRef]
- Tabak, M.; Hammer, J.; Glinsky, M.E.; Kruer, W.L.; Wilks, S.C.; Woodworth, J.; Campbell, E.M.; Perry, M.D.; Mason, R.J. Ignition and high gain with ultrapowerful lasers. Phys. Plasmas 1994, 1, 1626–1634. [Google Scholar] [CrossRef]
- Malka, V.; Faure, J.; Marques, J.; Amiranoff, F.; Rousseau, J.-P.; Ranc, S.; Chambaret, J.P.; Walton, B.; Mora, P.; Solodov, A. Characterization of electron beams produced by ultrashort (30 fs) laser pulses. Phys. Plasmas 2001, 8, 2605–2608. [Google Scholar] [CrossRef]
- Lécz, Z.; Polanek, R.; Andreev, A.; Sharma, A.; Papp, D.; Hafz, N.; Kamperidis, C. Hybrid acceleration of compact ion bunches by few-cycle laser pulses in gas jets of two atomic species. Phy. Rev. Res. 2023, 5, 023169. [Google Scholar] [CrossRef]
- Liu, Q.; Ma, M.; Zhang, X.; Lv, C.; Song, J.; Wang, Z.; Yang, G.; Yang, Y.; Wang, J.; Li, Q.; et al. Characteristic diagnosis of supersonic gas jet target for laser wakefield acceleration with high spatial-temporal resolution Nomarski interference system. Front. Phys. 2023, 11, 1203946. [Google Scholar] [CrossRef]
- Bradley, D.; Sheppard, C.G.W.; Suardjaja, I.M.; Woolley, R. Fundamentals of high-energy spark ignition with lasers. Combust. Flame 2004, 138, 55–77. [Google Scholar] [CrossRef]
- Kim, K.Y.; Glownia, J.H.; Taylor, A.J.; Rodriguez, G. Terahertz emission from ultrafast ionizing air in symmetry-broken laser fields. Opt. Express 2007, 15, 4577–4584. [Google Scholar] [CrossRef]
- Englesbe, A.; Elle, J.; Schwartz, R.; Garrett, T.; Woodbury, D.; Jang, D.; Kim, K.-Y.; Milchberg, H.; Reid, R.; Lucero, A.; et al. Ultrabroadband microwave radiation from near- and mid-infrared laser-produced plasmas in air. Phys. Rev. A 2021, 104, 013107. [Google Scholar] [CrossRef]
- Brinckerhoff, W.B.; Managadze, G.G.; McEntire, R.W.; Cheng, A.F.; Green, W.J. Laser time-of-flight mass spectrometry for space. Rev. Sci. Instrum. 2000, 71, 536–545. [Google Scholar] [CrossRef]
- Zayhowski, J.J. Passively Q-switched Nd: YAG microchip lasers and applications. J. Alloys Comp. 2000, 303, 393–400. [Google Scholar] [CrossRef]
- Hahn, D.W.; Omenetto, N. Laser-induced breakdown spectroscopy (LIBS), part II: Review of instrumental and methodological approaches to material analysis and applications to different fields. Appl. Spectrosc. 2012, 66, 347–419. [Google Scholar] [CrossRef]
- Hegelich, B.M.; Albright, B.J.; Cobble, J.; Flippo, K.; Letzring, S.; Paffett, M.; Ruhl, H.; Schreiber, J.; Schulze, R.K.; Fernández, J.C. Laser acceleration of quasi-monoenergetic MeV ion beams. Nature 2006, 439, 441–444. [Google Scholar] [CrossRef]
- Labaune, C.; Baccou, C.; Depierreux, S.; Goyon, C.; Loisel, G.; Yahia, V.; Rafelski, J. Fusion reactions initiated by laser-accelerated particle beams in a laser-produced plasma. Nat. Commun. 2013, 4, 2506. [Google Scholar] [CrossRef]
- Consoli, F.; Tikhonchuk, V.T.; Bardon, M.; Bradford, P.; Carroll, D.C.; Cikhardt, J.; Cipriani, M.; Clarke, R.J.; Cowan, T.E.; Danson, C.N.; et al. Laser produced electromagnetic pulses: Generation, detection, and mitigation. High Power Laser Sci. Eng. 2020, 8, e22. [Google Scholar] [CrossRef]
- Bradford, P.; Woolsey, N.C.; Scott, G.G.; Liao, G.; Liu, H.; Zhang, Y.; Zhu, B.; Armstrong, C.; Astbury, S.; Brenner, C.; et al. EMP control and characterization on high-power laser systems. High Power Laser Sci. Eng. 2018, 6, e21. [Google Scholar] [CrossRef]
- Kugland, N.L.; Aurand, B.; Brown, C.G.; Constantin, C.G.; Everson, E.T.; Glenzer, S.H.; Schaeffer, D.B.; Tauschwitz, A.; Niemann, C. Demonstration of a low electromagnetic pulse laser-driven argon gas jet x-ray source. Appl. Phys. Lett. 2012, 101, 024102. [Google Scholar] [CrossRef]
- Dubois, J.L.; Rączka, P.; Hulin, S.; Rosiński, M.; Ryć, L.; Parys, P.; Zaraś-Szydłowska, A.; Makaruk, D.; Tchórz, P.; Badziak, J.; et al. Experimental demonstration of an electromagnetic pulse mitigation concept for a laser driven proton source. Rev. Sci. Instrum. 2018, 89, 103301. [Google Scholar] [CrossRef]
- Gitomer, S.J.; Jones, R.D.; Begay, F.; Ehler, A.W.; Kephart, J.F.; Kristal, R. Fast ions and hot electrons in the laser–plasma interaction. Phys. Fluids 1986, 29, 2679–2688. [Google Scholar] [CrossRef]
- Courtois, C.; Ash, A.D.; Chambers, D.M.; Grundy, R.A.D.; Woolsey, N.C. Creation of a uniform high magnetic-field strength environment for laser-driven experiments. J. Appl. Phys. 2005, 98, 054913. [Google Scholar] [CrossRef]
- Brown, C.J., Jr.; Throop, A.; Eder, D.; Kimbrough, J. Electromagnetic pulses at short-pulse laser facilities. J. Phys. Conf. Ser. 2008, 112, 032025. [Google Scholar] [CrossRef]
- Dubois, J.L.; Lubrano-Lavaderci, F.; Raffestin, D.; Ribolzi, J.; Gazave, J.; Compant La Fontaine, A.; D’Humières, E.; Hulin, S.; Nicolaï, P.; Poyé, A.; et al. Target charging in short-pulse-laser–plasma experiments. Phys. Rev. E 2014, 89, 013102. [Google Scholar] [CrossRef]
- Nassisi, V.; Delle Side, D.; Monteduro, L.; Giuffreda, E. Mapping of acceleration field in FSA configuration of a LIS. J. Instrum. 2016, 11, C05014. [Google Scholar] [CrossRef]
- Jackson, J.D. Classical Electrodynamics; John Wiley & Sons: New York, NY, USA, 1975. [Google Scholar]
- Mead, M.J.; Neely, D.; Gauoin, J.; Heathcote, R.; Patel, P. Electromagnetic pulse generation within a petawatt laser target chamber. Rev. Sci. Instrum. 2004, 75, 4225. [Google Scholar] [CrossRef]
- Mendel, C.W., Jr. Apparatus for measuring rapidly varying electric fields in plasmas. Rev. Sci. Instrum. 1975, 46, 847–850. [Google Scholar] [CrossRef]
- Borghesi, M.; Sarri, G.; Cecchetti, C.A.; Kourakis, I.; Hoarty, D.; Stevenson, R.M.; James, S.; Brown, C.D.; Hobbs, P.; Lockyear, J.; et al. Progress in proton radiography for diagnosis of ICF-relevant plasmas. Laser Part. Beams 2010, 28, 277–284. [Google Scholar] [CrossRef]
- Manuel, M.E.; Sinenian, N.; Séguin, F.H.; Li, C.K.; Frenje, J.A.; Rinderknecht, H.G.; Casey, D.T.; Zylstra, A.B.; Petrasso, R.D.; Beg, F.N. Mapping return currents in laser-generated Z-pinch plasmas using proton deflectometry. Appl. Phys. Lett. 2012, 100, 203505. [Google Scholar] [CrossRef]
- Krása, J.; Delle Side, D.; Giuffreda, E.; Nassisi, V. Characteristics of target polarization by laser ablation. Laser Part. Beams 2015, 33, 601–605. [Google Scholar] [CrossRef]
- Eliezer, S.; Hora, H. Double layers in laser-produced plasmas. Phys. Rep. 1989, 172, 339–407. [Google Scholar] [CrossRef]
- Eliezer, S.; Nissim, N.; Val, J.M.M.; Mima, K.; Hora, H. Double layer acceleration by laser radiation. Laser Part. Beams 2014, 32, 211–216. [Google Scholar] [CrossRef]
- Bulgakova, N.M.; Bulgakov, A.V. Pulsed laser ablation of solids: Transition from normal vaporization to phase explosion. Appl. Phys. A 2001, 73, 199–208. [Google Scholar] [CrossRef]
- Marla, D.; Bhandarkar, U.V.; Joshi, S.S. A model of laser ablation with temperature-dependent material properties, vaporization, phase explosion and plasma shielding. Appl. Phys. A 2014, 116, 273–285. [Google Scholar] [CrossRef]
- Pearlman, J.S.; Dahlbacka, G.H. Charge separation and target voltages in laser produced plasmas. Appl. Phys. Lett. 1977, 31, 414–417. [Google Scholar] [CrossRef]
- Poyé, A.; Dubois, J.-L.; Lubrano-Lavaderci, F.; D’Humières, E.; Bardon, M.; Hulin, S.; Bailly-Grandvaux, M.; Ribolzi, J.; Raffestin, D.; Santos, J.J.; et al. Dynamic model of target charging by short laser pulse interactions. Phys. Rev. E 2015, 92, 043107. [Google Scholar] [CrossRef] [PubMed]
- Krása, J.; Nassisi, V.; Klír, D. Target holder as a specific sensor for laser-induced plasma ablation. Phys. Lett. A 2021, 385, 126980. [Google Scholar] [CrossRef]
- Krása, J.; Nassisi, V.; Klír, D. Effect of grounding and isolation of the target on the emissive properties of laser-produced plasma. Phys. Plasmas 2021, 28, 092104. [Google Scholar] [CrossRef]
- Tudisco, S.; Mascali, D.; Gambino, N.; Anzalone, A.; Gammino, S.; Musumeci, F.; Scordino, A.; Spitaleri, A. Investigation of laser-produced aluminum plasma. Nucl. Instrum. Methods Phys. Res. Sect. A 2011, 653, 47–51. [Google Scholar] [CrossRef]
- Tan, T.H.; McCall, G.H.; Williams, A.H. Determination of laser intensity and hot-electron temperature from fastest ion velocity measurement on laser-produced plasma. Phys. Fluids 1984, 27, 296–301. [Google Scholar] [CrossRef]
- Krása, J.; Burian, T.; Hájková, V.; Chalupský, J.; Jelínek, Š.; Frantálová, K.; Krupka, M.; Kuglerová, Z.; Singh, S.K.; Vozda, V.; et al. Ion emission from warm dense matter produced by irradiation with a soft x-ray free-electron laser. Matter Radiat. Extremes 2024, 9, 016602. [Google Scholar] [CrossRef]
- Price, C.J.; Donnelly, T.D.; Giltrap, S.; Stuart, N.H.; Parker, S.; Patankar, S.; Lowe, H.F.; Drew, D.; Gumbrell, E.T.; Smith, R.A. An in-vacuo optical levitation trap for high-intensity laser interaction experiments with isolated microtargets. Rev. Sci. Instrum. 2015, 86, 033502. [Google Scholar] [CrossRef] [PubMed]
- Sinenian, N.; Manuel, M.J.; Frenje, J.A.; Séguin, F.H.; Li, C.K.; Petrasso, R.D. An empirical target discharging model relevant to hot-electron preheat in direct-drive implosions on OMEGA. Plasma Phys. Control. Fusion 2013, 55, 045001. [Google Scholar] [CrossRef][Green Version]
- Giuffreda, E.; Delle Side, D.; Nassisi, V.; Krása, J. Plasma production in carbon-based materials. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 2017, 406, 225. [Google Scholar] [CrossRef]
- Delle Side, D.; Caricato, A.P.; Krása, J.; Nassisi, V. On the origin of negative target currents during laser ablation of polyethylene. EPJ Web Conf. EDP Sci. 2018, 167, 04006. [Google Scholar] [CrossRef][Green Version]
- Raimbourg, J. Electromagnetic compatibility management for fast diagnostic design. Rev. Sci. Instrum. 2004, 75, 4234–4236. [Google Scholar] [CrossRef]
- Stoeckl, C.; Glebov, V.Y.; Jaanimagi, P.A.; Knauer, J.P.; Meyerhofer, D.D.; Sangster, T.C.; Storm, M.; Sublett, S.; Theobald, W.; Key, M.H.; et al. Operation of target diagnostics in a petawatt laser environment. Rev. Sci. Instrum. 2006, 77, 10F506. [Google Scholar] [CrossRef]
- Bourgade, J.L.; Marmoret, R.; Darbon, S.; Rosch, R.; Troussel, P.; Villette, B.; Glebov, V.; Shmayda, W.T.; Gomme, J.C.; Le Tonqueze, Y.; et al. Diagnostics hardening for harsh environment in laser megajoule. Rev. Sci. Instrum. 2008, 79, 10F301. [Google Scholar] [CrossRef] [PubMed]
- Eder, D.C.; Throop, A.; Brown, C.G., Jr.; Kimbrough, J.; Stowell, M.L.; White, D.A.; Song, P.; Back, N.; MacPhee, A.; Chen, H.; et al. Mitigation of Electromagnetic Pulse (EMP) Effects from Short-Pulse Lasers and Fusion Neutrons; Technical Report, LLNL-TR-411183; Lawrence Livermore National Laboratory: Livermore, CA, USA, 2009. [Google Scholar]
- Krása, J.; KlÍr, D.; Řezáč, K.; Cikhardt, J.; Velyhan, A.; Pfeifer, M.; Dostál, J.; Krůs, M.; Dudžák, R.; Buryšková, S.; et al. Target current: An appropriate parameter for characterizing the dynamics of laser-matter interaction. In Proceedings of the XXII International Symposium on High Power Laser Systems and Applications, Frascati, Italy, 9–12 October 2019; Volume 11042. [Google Scholar] [CrossRef]
- Benjamin, R.F.; McCall, G.H.; Ehler, A.W. Measurement of return current in a laser-produced plasma. Phys. Rev. Lett. 1979, 42, 890–893. [Google Scholar] [CrossRef]
- Cikhardt, J.; Krása, J.; De Marco, M.; Pfeifer, M.; Velyhan, A.; Krouský, E.; Cikhardtová, B.; Klír, D.; Rezáč, K.; Ullschmied, J.; et al. Measurement of the target current by inductive probe during laser interaction on terawatt laser system PALS. Rev. Sci. Instrum. 2014, 85, 103507. [Google Scholar] [CrossRef]
- Krupka, M.; Singh, S.; Pisarczyk, T.; Dostal, J.; Kalal, M.; Krasa, J.; Dudzak, R.; Burian, T.; Jelinek, S.; Chodukowski, T.; et al. Design of modular multi-channel electron spectrometers for application in laser matter interaction experiments at Prague Asterix Laser System. Rev. Sci. Instrum. 2021, 92, 023514. [Google Scholar] [CrossRef]
- Pisarczyk, T.; Kalal, M.; Gus’kov, S.Y.; Batani, D.; Renner, O.; Santos, J.; Dudzak, R.; Zaras-Szydłowska, A.; Chodukowski, T.; Rusiniak, Z.; et al. Hot electron retention in laser plasma created under terawatt subnanosecond irradiation of Cu targets. Plasma Phys. Control. Fusion 2020, 62, 115020. [Google Scholar] [CrossRef]
- Yi, T.; Yang, J.; Yang, M.; Wang, C.; Yang, W.; Li, T.; Liu, S.; Jiang, S.; Ding, Y.; Xiao, S. Investigation into the electromagnetic impulses from long pulse laser illuminating solid targets inside a laser facility. Photonic Sens. 2016, 6, 249–255. [Google Scholar] [CrossRef][Green Version]
- Xia, Y.; Li, D.; Zhang, S.; Wu, M.; Yang, T.; Geng, Y.; Zhu, J.; Xu, X.; Li, C.; Wang, C.; et al. Enhancing electromagnetic radiations by a pre-ablation laser during laser interaction with solid target. Phys. Plasmas 2020, 27, 032705. [Google Scholar] [CrossRef]
- Nelissen, K.; Liszi, M.; De Marco, M.; Ospina, V.; Drotár, I.; Gatti, G.; Kamperidis, C.; Volpe, L. Characterisation and modelling of ultrashort laser-driven electromagnetic pulses. Sci. Rep. 2020, 10, 3108. [Google Scholar] [CrossRef]
- Rączka, P.; Nowosielski, L.; Rosinski, M.; Makaruk, D.; Makowski, J.; Zaras-Szydłowska, A.; Tchórz, P.; Badziak, J. Measurement of the electric field strength generated in the experimental chamber by 10 TW femtosecond laser pulse interaction with a solid target. J. Instrum. 2019, 14, P04008. [Google Scholar] [CrossRef]
- Vinoth Kumar, L.; Manikanta, E.; Leela, C.; Prem Kiran, P. Spectral selective radio frequency emissions from laser induced breakdown of target materials. Appl. Phys. Lett. 2014, 105, 064102. [Google Scholar] [CrossRef]
- Ostrovskaya, G.V.; Zaĭdel’, A.N. Laser spark in gases. Sov. Phys. Usp. 1974, 16, 834–855. [Google Scholar] [CrossRef]
- Englesbe, A.; Elle, J.; Reid, R.; Lucero, A.; Pohle, H.; Domonkos, M.; Kalmykov, S.; Krushelnik, K.; Schmitt-Sody, A. Gas pressure dependence of microwave pulses generated by laser-produced filament plasmas. Opt. Lett. 2018, 43, 4953–4956. [Google Scholar] [CrossRef]
- Blair, G.; Sprangle, P. Generation of rf radiation by low-intensity laser pulse trains in air. Phys. Rev. E 2023, 108, 015203. [Google Scholar] [CrossRef]
- Link, A.; Freeman, R.R.; Schumacher, D.W.; Van Woerkom, L.D. Effects of target charging and ion emission on the energy spectrum of emitted electrons. Phys. Plasmas 2011, 18, 053107. [Google Scholar] [CrossRef]
- Cristoforetti, G.; Antonelli, L.; Atzeni, S.; Baffigi, F.; Barbato, F.; Batani, D.; Boutoux, G.; Colaitis, A.; Dostal, J.; Dudzak, R.; et al. Measurements of parametric instabilities at laser intensities relevant to strong shock generation. Phys. Plasmas 2018, 25, 012702. [Google Scholar] [CrossRef]
- Prasad, Y.B.S.R.; Barnwal, S.; Bolkhovitinov, E.A.; Naik, P.A.; Kamath, M.P.; Joshi, A.S.; Kumbhare, S.R.; Rupasov, A.A.; Gupta, P.D. Study of self-generated magnetic fields in laser produced plasmas using a three-channel polaro-interferometer. Rev. Sci. Instrum. 2011, 82, 123506. [Google Scholar] [CrossRef] [PubMed]
- Pisarczyk, T.; Gus’kov, S.Y.; Dudzak, R.; Chodukowski, T.; Dostal, J.; Demchenko, N.N.; Korneev, P.; Kalinowska, Z.; Kalal, M.; Renner, O.; et al. Space-time resolved measurements of spontaneous magnetic fields in laser-produced plasma. Phys. Plasmas 2015, 22, 102706. [Google Scholar] [CrossRef]
- Kalal, M. Complex interferometry: Its principles and applications to fully automated on-line diagnostics. Czech. J. Phys. 1991, 41, 743–748. [Google Scholar] [CrossRef]
- Bolkhovitinov, E.A.; Gospodinov, G.A.; Ivanov, K.A.; Rupasov, A.A.; Savel’ev, A.B. Three-channel polaro-interferometer for laser-produced plasma diagnostics with femtosecond time resolution. Quantum Electron. 2019, 49, 577. [Google Scholar] [CrossRef]
- Singh, S.; Versaci, R.; Laso Garcia, A.; Morejon, L.; Ferrari, A.; Molodtsova, M.; Schwengner, R.; Kumar, D.; Cowan, T. Compact high energy X-ray spectrometer based on forward Compton scattering for high intensity laser plasma experiments. Rev. Sci. Instrum. 2018, 89, 085118. [Google Scholar] [CrossRef] [PubMed]
- Haden, D.; Golovin, G.; Yan, W.; Fruhling, C.; Zhang, P.; Zhao, B.; Banerjee, S.; Umstadter, D. High energy X-ray Compton spectroscopy via iterative reconstruction. Nucl. Instrum. Methods A 2020, 951, 163032. [Google Scholar] [CrossRef]
- Singh, S.; Krupka, M.; Istokskaia, V.; Krasa, J.; Giuffrida, L.; Dudzak, R.; Dostal, J.; Burian, T.; Versaci, R.; Margarone, D.; et al. Hot electron and x-ray generation by sub-ns kJ-class laser-produced tantalum plasma. Plasma Phys. Control. Fusion 2022, 64, 105012. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krása, J.; Krupka, M.; Agarwal, S.; Nassisi, V.; Singh, S. Advanced Diagnostics of Electrons Escaping from Laser-Produced Plasma. Plasma 2024, 7, 366-385. https://doi.org/10.3390/plasma7020021
Krása J, Krupka M, Agarwal S, Nassisi V, Singh S. Advanced Diagnostics of Electrons Escaping from Laser-Produced Plasma. Plasma. 2024; 7(2):366-385. https://doi.org/10.3390/plasma7020021
Chicago/Turabian StyleKrása, Josef, Michal Krupka, Shubham Agarwal, Vincenzo Nassisi, and Sushil Singh. 2024. "Advanced Diagnostics of Electrons Escaping from Laser-Produced Plasma" Plasma 7, no. 2: 366-385. https://doi.org/10.3390/plasma7020021
APA StyleKrása, J., Krupka, M., Agarwal, S., Nassisi, V., & Singh, S. (2024). Advanced Diagnostics of Electrons Escaping from Laser-Produced Plasma. Plasma, 7(2), 366-385. https://doi.org/10.3390/plasma7020021





