The Phenomenon of a Cathode Spot in an Electrical Arc: The Current Understanding of the Mechanism of Cathode Heating and Plasma Generation
Abstract
1. Introduction
2. Experimental Data
Specific Problem Formulation to Explicitly Understand the Experiments
3. Evolution of the Spot Theory and Spot Mechanism Understanding
3.1. General Cathode Phenomena and Mathematical Description
3.2. Vaporization Model: Primary System of Equation with Limited Conditions
- Mackeown’s equation indicates a relation between E, j, s, and the cathode potential drop uc in the cathode [63].
- The equation of the current density of electrons emitted from the cathode in its general form depends on Ts and E:where D(E,εe) is the probability that an electron with energy εe will emerge from the metal, and N(εe,T) is the supply function.
- The energy balance equation takes into account the power input to the cathode spot by ion kinetic and potential energy and the dissipation powers by thermal conduction into the cathode, which is determined by a steady-state solution to the heat conduction equation [59], vaporization determined with the Dushman equation [60], the cooling effect of electron emission, and radiation.
- All variables are treated as constants over the spot area and it is assumed that the distribution of the current density in the cathode spot is uniform. The following equation of the total spot current Is is used:
4. Explosive Phenomena in Electrical Discharges
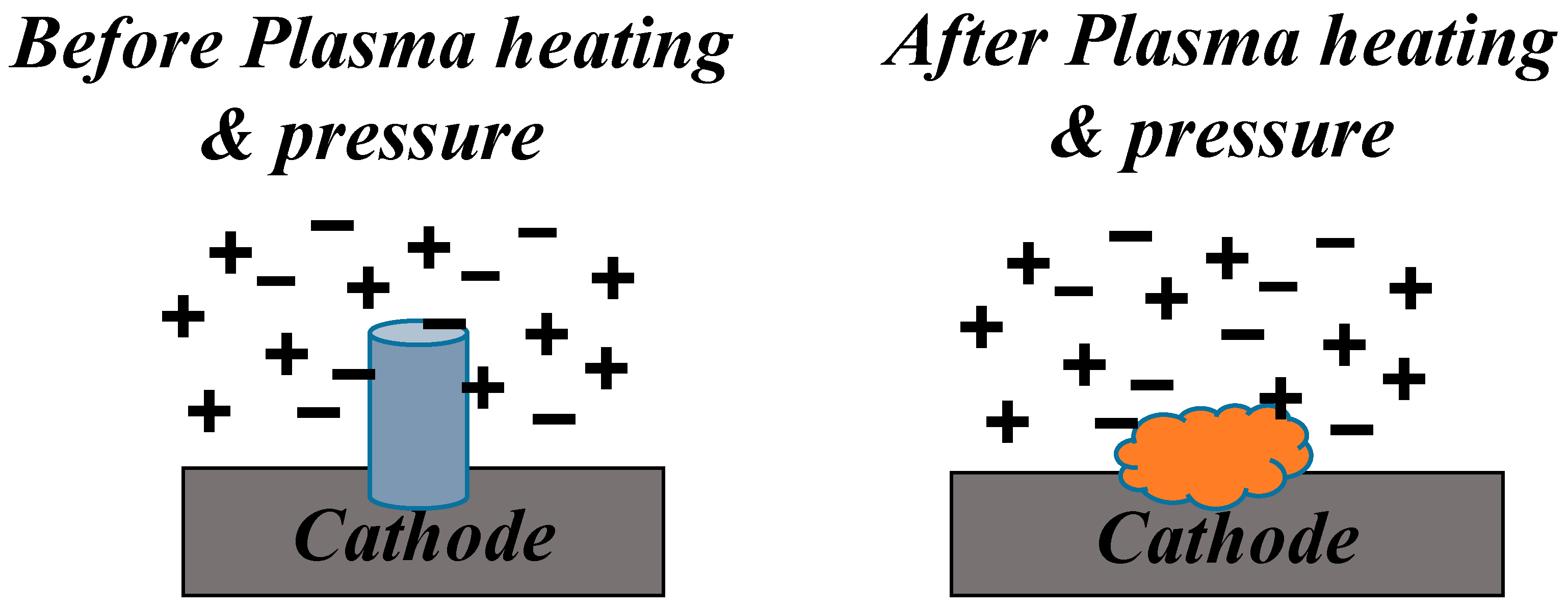
4.1. Exploding Phenomena by High-Voltage Application: Explosive Electron Emission (EEE)
4.2. Explosive Electron Emission Application in the Cathode Spot Mechanism
4.3. Further Development of the Explosive Approach and Emission Center Formation
- For simplicity, the heat conduction equation is presented here only with the main term (right side) of Joule heating as the volume source, which is expressed in the following form:where is the density of the cathode material, c is the specific heat, and ρr(T) is the resistivity of the cathode;
- The Mackeown equation for the electric field (Equation (1));
- The equation of thermionic electron emission with Schottky effect, i(t).
4.4. Fractal Approach
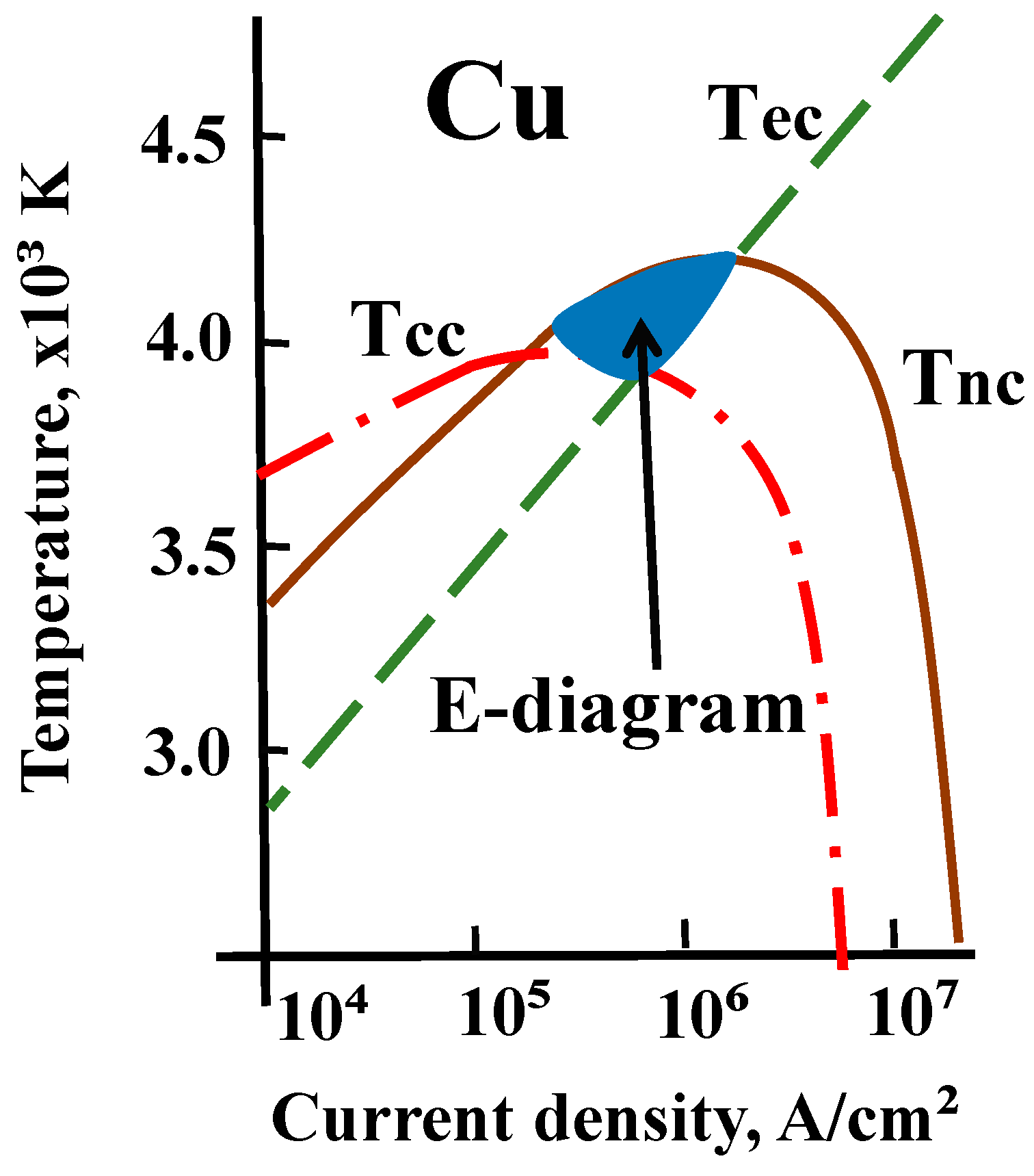
4.5. Specifics of the EC Model
5. Model of Ionization Cathode Vapor and Electron Emission
5.1. Summary Overview of Specific Previous Models
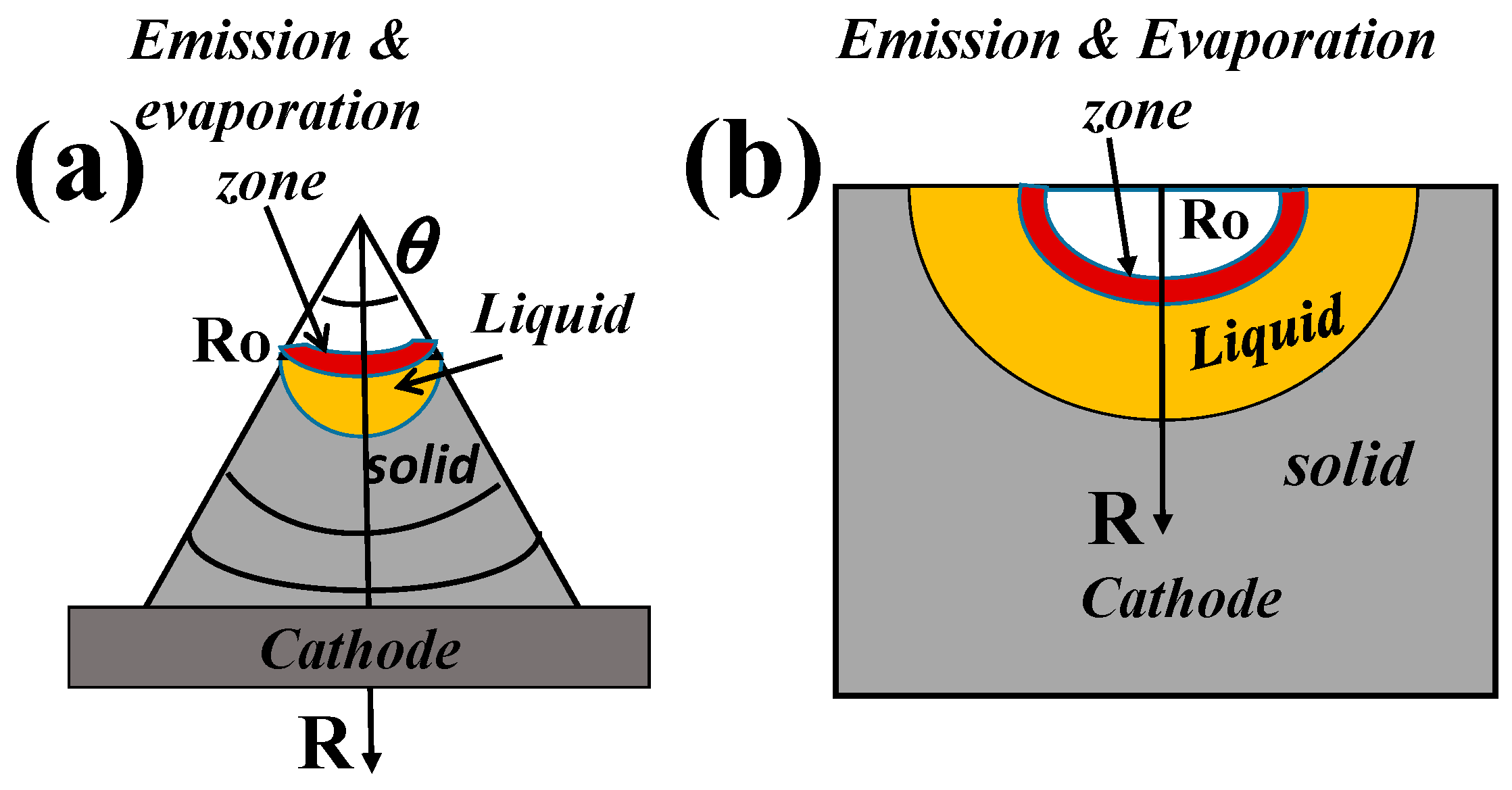
5.2. Cathode Spot Description Using a Closed Mathematical Approach: The Kinetic Model
5.3. The Kinetic Study of Cathode Vaporization and Plasma Generation
5.4. Numerical Solutions
- An observation of different spot types, as presented in Table 1, considered the heating specifics and the kinetics of the evaporating flux. The spot dynamics data, characterized by different spot velocities, lifetimes, and group spots, were understood by studying the cathode heating phenomena, taking into account the specifics of the cathode geometry and surface impurity. In particular, the mechanism of spot association was considered, which appeared to be due to an impeded plasma flow under background pressure, which occurred by filling the expanding plasma in the gap during arcing or by using external low-pressure gas [95].
- Specific experiments were carried out with a film cathode [29]. The experiments were undertaken to determine the basic features of Cu film cathode spots, in contrast to impurity films on bulk surfaces, for which information about film properties is limited. Features of moving spots were studied with the “signature” method: the spots left a clearly defined erosion trace of a certain width, in which all of the metal thin film was removed from the glass substrates with no signs of disturbance on the glass substrate. No explosion material was observed around the traced region, nor any protrusions that can initiate an explosion. A very low current per spot was measured, ranging from 0.1 to 0.4 A, when the film thickness increased from 0.017 to 0.06 µm.The calculations using the vaporization kinetic model for the film cathode [96] showed that the cathode potential drop uc for the Cu film weakly changed with the film thickness, and the calculated value well agree with the low measured value of 11 V [97]. The measured trace width coincides with the calculated spot diameter, and the measured spot velocity well agrees with the calculated value. The important theoretical result is the low power loss obtained due to heat conduction and therefore the low effective voltage of the film cathode. This model prediction allows us to explain the observed low current per spot and the low cathode potential drop, which appeared to be significantly smaller than that for a bulk cathode (15 V [29]).
- One of the cathode spot problems that should be understood is the spot nature in the Hg cathode, characterized by considerable volatility in comparison to its very low electron emissive ability at any temperature. The explosive model used the electrostatic pulling force on the liquid surface caused by microprotusions due to the Tonks instability [98]. In general, the local electric field at a protrusion tip can be enhanced by some factor over an average electric field. However, firstly, the typical microprotrusion growth time [98] (~1 ms) is large compared to the spot lifetime, for most spot types and especially for the fast spots existing in the Hg cathode. Secondly, the space charge sheath of the average field for the plasma spot is much smaller than the tip, and therefore the field cannot be enhanced [16].In the case of volatile materials, a model of a double-layer cathode plasma was developed. The layer adjacent to the cathode surface serves as a “plasma cathode”, supplying electrons to the remaining part of the discharge. The electrons are emitted from a plasma cathode produced by the thermal ionization of the evaporated material. The current continuity at the cathode surface is closed by the ion flux from the adjacent cathode plasma. The model revealed two types of time-dependent solutions with characteristic spot lifetimes of 0.1–1 µs and 100 µs corresponding to the experimentally observed two forms of Hg arc. The theoretical study allowed for the explanation of the observed arc forms: the transitional form with a cathode drop of 18 V appeared at a low current, and fundamental arc forms with a cathode drop of 8.5–10 V appeared when the arc current was sufficient for several spots to exist simultaneously [16].
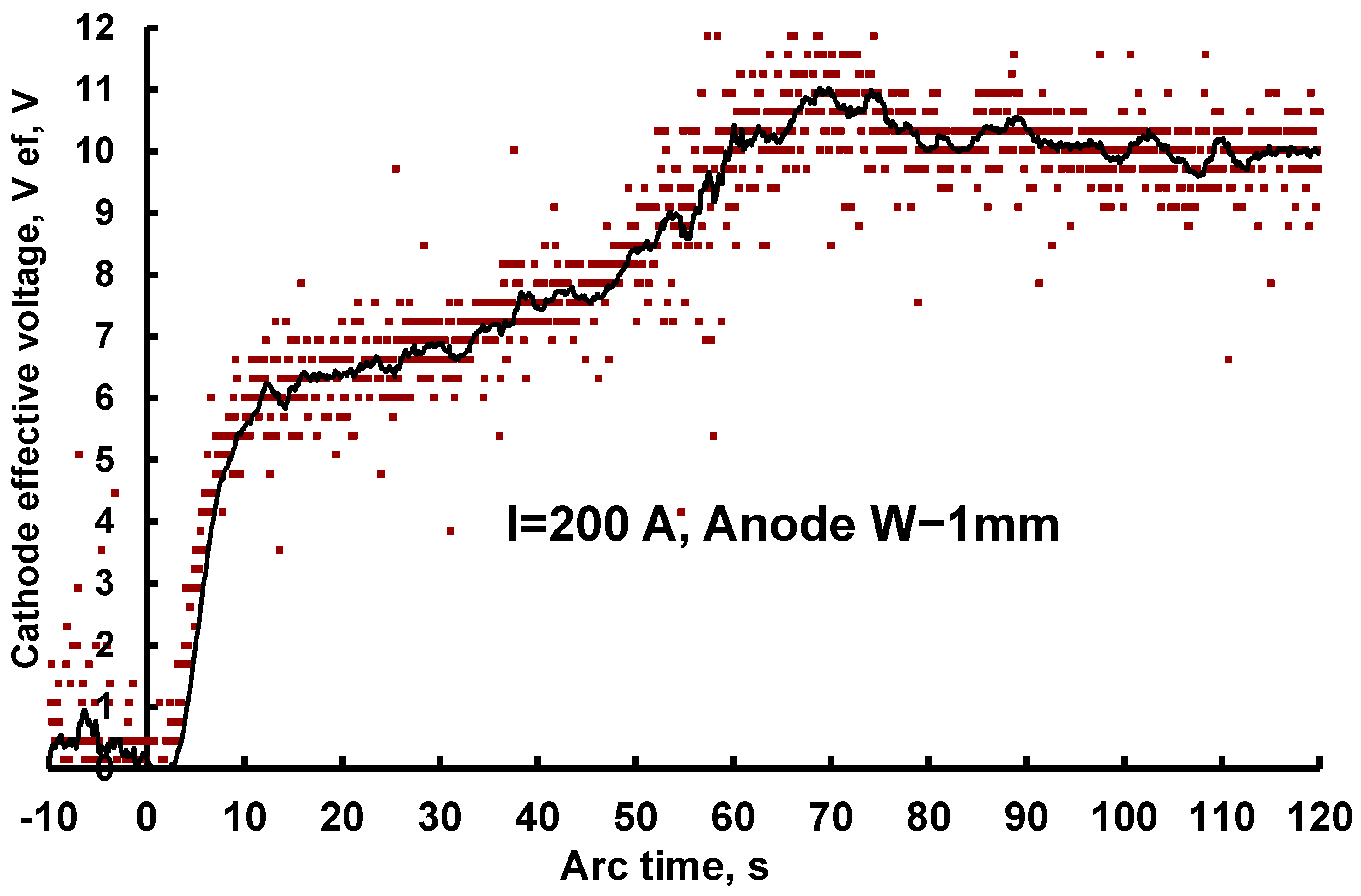
- Another well-established experimental observation is the energy losses due to cathode heat conduction per unit current, which is defined by the cathode effective voltage uef. The uef value was determined by measuring the temperature rise in the cathode with the thermocouple at the backside of a cleaned bulk disk. In the case of open cathode–anode gap configuration, arcing times varied up to half a second, and for different cathode materials and arc currents, the measuring uef was 6 V [99]. The time-dependent measurements for closed electrode gap configuration VABBA (see above) showed an interesting result [100]. At the beginning of the arc (when the anode is cold and not influencing the cathode plasma jet expansion), the traditional result was obtained as uef = 6 V. By taking into account the arc time (when the anode is hot, and the cathode plasma jets re-evaporate, increasing the gap plasma density), the measuring uef increased up to 12 V. In order to illustrate the effect of uef increase, the time dependence of the cathode effective voltage uef is presented in Figure 9, which was obtained in accordance with the methodology described previously [100] for an arc sustained between a water-cooled Cu cathode with 30 mm diameter and a cylindrical shower head W anode with a 50 mm outer diameter and 1 mm holes.It is difficult to accept that the whole cathode temperature rise, and the increase in the effective voltage shown in Figure 9, were caused by explosive phenomena. It can be assumed that, during protrusion explosions, its accumulated energy is carried away by super high-speed (2 × 106 cm/s) plasma plume at a large distance of 10−3 cm during a short time of 10−9 s, and during the same time, the micro-area in the cathode around the protrusion is cooled by some mechanism. It is reasonable to consider that disk heating occurs due to the heat flux as a consequence of energetic interactions of the cathode plasma with its surface and that the backside temperature is determined based on disk heat conduction. The model that considered such interaction was developed recently, explaining the nature of energy loss by cathode heat conduction and the cathode effective voltage in vacuum arcs [101].
- Specifics of current–voltage characteristics in high current vacuum by the presence of an axial magnetic field (AMF) were studied. The experiment showed that a high arc voltage, which may lead to an increase in the heat flux on the electrodes, characterized such a high current arc. However, the imposition of an AMF also changes the arc’s current–voltage characteristics [102]. At high currents, the voltage initially decreases as the AMF increases from zero, and then reaches a minimum at a critical value of the AMF, and slowly increases thereafter with a further increase in the magnetic field. This current–voltage behavior with a minimum voltage was previously not understandable and was explained by considering the hydrodynamics of plasma jet expansion from cathode spots as an individual flow from each spot or as the mixing of the jets depending on the presence of AMF [103]. Note that it is difficult to represent any change in the cathode jet structure, which can arise due to any individual nanosecond explosion under an AMF.The observed retrograde spot motion, the effect of spots expanding in the form of a ring, [104], and the effect of acute angle can be explained in the case when the adjacent layer to the cathode plasma interacts with the cathode surface and not when the plasma exploded and expanded as a single plume far from the cathode. Figure 10 demonstrates an example of spot expansion in a ring form on an Al cathode obtained in accordance with the methodology described previously [51]. The understanding of these experimental results has recently been detailed [105,106,107].
- The model [108] of an arc spot on a refractory cathode was developed self-consistently considering the effect of a virtual cathode in order to match the discrepancy between very large electron emissions relative to the low evaporation flux (low neutral density) for a wide range of cathode temperatures. Such an approach took into account the presence of the virtual cathode by only considering the spot development as a self-sustained subject. However, the effect of the virtual cathode cannot be due to limiting the spot current by a current Ic determined by the resistivity of the electrical circuit, when the emissivity of an EC is greater than Ic, as arbitrarily proposed in the model [79].
6. Discussion and Summary
- Consider the measured maximal spot velocity of 103–104 cm/s, which was observed experimentally (Table 1). In this case, the time of spot location in the range of a spot radius of about 10 µm was about 10−6–10−7 s, while the EC time location was in the nanosecond range, indicating a significantly larger velocity. Thus, here is a discrepancy (modeling the next explosion occurs under the plasma of the previous EC) that indicated an enhanced value observed but a lower spot velocity.
- The products of explosion may not reproduce the needed plasma parameters because of a very small protrusion size. The estimation showed that the mass of the exploding protrusion was not enough to support the plasma density in order to initiate a new EC, taking into account the high velocity of the expanding product of the explosion.
- The exploded plasma plume expanded with velocity 2 × 106 cm/s and was removed from the cathode surface at a large distance of ~10−3 cm during the explosion time of 10−9 s. Therefore, the calculating process cannot be self-sustained.
- In essence, the traditional name “cathode spot”, established are the beginning of the previous century, was renamed “EC”, to which was added the word “TON”. Thus, the new name is “ECTON”, assuming that an explosion is an elementary act in the processes occurring in the cathode spot of a vacuum discharge [79]. However, it is misunderstood and even confusing when interpreting the cathode spot mechanism. This act cannot be considered elementary because too many arbitrarily inputted parameters are taken for the simulation of an ECTON. According to the observed cathode spot dynamics, the spot is a self-sustained phenomenon in which the physical processes continuously support regenerative plasma in contrast to a singular act of an explosion. In addition, many different models were used (by reducing the electron emission upon cooling or, conversely, by reaching an enormous critical cathode temperature), indicating that this process is rather specific and not universal.
7. Conclusions
- As the electrical arc is a self-organized discharge, in order to adequately study the interrelated phenomena in the cathode region of a vacuum arc, it is necessary to use the mathematical model formulated in the closed form, i.e., without arbitrarily established parameters.
- The initial plasma conditions for a transient spot study should be coupled, as it is necessary for the self-consistent simulation of the spot development process. The initial plasma characteristics that are given as parametric independent data including micro-sized current location may yield results that are not consistent with real observable phenomena.
- At arc ignition, the spot initiation is supported by a rise in electrical power (sharp growth in the cathode potential drop with moderate current density changes), which is obtained using a closed mathematical model. In contrast, no real strong growth of current density was obtained using the approach with arbitrarily given parameters, including the constant cathode potential drop at the moment of cathode spot initiation and its development.
Funding
Conflicts of Interest
References
- Landau, L.D.; Pitaevskii, L.P.; Lifshitz, E.M. Electrodynamics of Continuous Media, 2nd ed.; Pitaevskii, L.P., Lifshitz, E.M., Eds.; Pergamon Press: Oxford, UK; New York, NY, USA, 1984. [Google Scholar]
- Meek, J.M.; Craggs, J.D. Electrical Breakdown of Gases; Clarendon Press: Oxford, UK, 1953. [Google Scholar]
- Nasser, E. Fundamentals of Gaseous Ionization and Plasma Electronics; Wiley Series in Plasma Physics; John Wiley and Sons: New York, NY, USA, 1971. [Google Scholar]
- Keidar, M.; Beilis, I.I. Plasma Engineering, 2nd ed.; Academic Press: Cambridge, MA, USA; Elsevier: Amsterdam, The Netherlands, 2018. [Google Scholar]
- Finkelnburg, W.; Maecker, H. Electrische Bogen und Thermisches Plasma; Handbuch der Physic; Springer: Berlin/Heidelberg, Germany, 1956; Volume XXII, pp. 254–444. [Google Scholar]
- Kesaev, I.G. Cathode Processes in the Mercury Arc; Consultants Bureau: New York, NY, USA, 1964. [Google Scholar]
- Lafferty, J.M. Vacuum Arcs; John Wiley and Sons: New York, NY, USA, 1980. [Google Scholar]
- Qu, J.; Zeng, M.; Dewei Zhang, D.; Yang, D.; Wu, X.; Ren, Q.; Zhang, J. A review on recent advances and challenges of ionic wind produced by corona discharges with practical applications. J. Phys. D Appl. Phys. 2022, 55, 153002. [Google Scholar] [CrossRef]
- Mavrodineanu, R. Hollow Cathode Discharges—Analytical Applications. J. Res. Natl. Bur. Stand. 1984, 89, 143–185. [Google Scholar] [CrossRef] [PubMed]
- Bogaerts, R. The glow discharge. An exciting plasma. J. Anal. At. 1999, 14, 1375–1384. [Google Scholar]
- Canton, A.; Cavazzana, R.; Grando, L.; Spolaore, M.; Zuin, M. Designing high efficiency glow discharge cleaning systems. Nucl. Mater. Energy 2019, 19, 468–472. [Google Scholar] [CrossRef]
- Mac Rae, D.R. Plasma reactors for process metallurgy applications. Pure Appl. Chem. 1988, 60, 721–725. [Google Scholar] [CrossRef]
- Karimi-Sibaki, E.; Kharicha, A.; Ludwig, A.; Wu, M.; Bohacek, J. A Parametric Study of the Vacuum Arc Remelting (VAR) Process: Effects of Arc Radius, Side-Arcing, and Gas Cooling. Metall. Mater. Trans. B 2020, 51, 222–235. [Google Scholar] [CrossRef]
- Bokova, V.M.; Sisa, O.F. Application of an Electric Arc to Produce Metal Powders. Surf. Eng. Appl. Electrochem. 2020, 56, 390–399. [Google Scholar] [CrossRef]
- Kah, P.; Latifi, H.; Suoranta, R.; Martikainen, J.; Pirinen, M. Usability of arc types in industrial welding. Int. J. Mech. Mater. Eng. 2014, 9, 15. [Google Scholar] [CrossRef]
- Beilis, I.I. Plasma and Spot Phenomena in Electrical Arcs; Springer: Cham, Switzerland, 2020; Volumes 1–2. [Google Scholar]
- Anders, A. Cathodic Arcs: From Fractal Spots to Energetic Condensation; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Slade, P.G. The Vacuum Interrupter: Theory, Design, and Application, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2021. [Google Scholar]
- Boxman, R.L.; Martin, P.J.; Sander, D.M. (Eds.) Handbook of Vacuum Arc Science and Technology; Noyes Publisher: Park Ridge, NJ, USA, 1995. [Google Scholar]
- Lugscheider, E.; Knotek, O.; Barimani, C.; Zimmermann, H. Arc PVD-coated cutting tools for modern machining applications. Surf. Coat. Technol. 1997, 91–95, 641–646. [Google Scholar] [CrossRef]
- Nikolaev, A.G.; Oks, E.M.; Savkin, K.P.; Yushkov, G.Y.; Brown, I.G. Upgraded vacuum arc ion source metal ion implantation. Rev. Sci. Instrum. 2012, 83, 02A502. [Google Scholar] [CrossRef]
- Zolotukhin, D.B.; Daniels, K.P.; Brieda, L.; Keidar, M. Onset of the magnetized arc and its effect on the momentum of a low-power two-stage pulsed magneto-plasma-dynamic thruster. Phys. Rev. 2020, 102, 021203. [Google Scholar] [CrossRef] [PubMed]
- Siemroth, P.; Wenzel, C.; Kliomes, W.; Schultrich, B.; Schulke, T. Metallization of submicron tranches and vias with high aspect ratio. Thin Solid Films 1997, 308–309, 455–459. [Google Scholar] [CrossRef]
- Monteiro, O.R. Novel metallization technique for filling 100-nm-wide trenches and vias with very high aspect ratio. J. Vac. Sci. Technol. B 1999, 17, 1094–1097. [Google Scholar] [CrossRef]
- Daalder, J.E. Components of cathode erosion in vacuum arcs. J. Phys. D Appl. Phys. 1976, 9, 2379–2395. [Google Scholar] [CrossRef]
- Witke, T.; Siemroth, P. Deposition of Droplet-Free Films by Vacuum Arc Evaporation—Results and Applications. IEEE Trans. Plasma Sci. 1999, 27, 1039–1044. [Google Scholar] [CrossRef]
- Beilis, I.I.; Boxman, R.L. Thin Film Deposition by Plasma Beam of a Vacuum Arc with Refractory Anodes. Ch. In Advances in Thin Films, Nanostructured Materials, and Coatings; Alexander, D.P., Valentine, N., Eds.; Springer: Berlin/Heidelberg, Germany, 2019; pp. 1–17. [Google Scholar]
- Kesaev, I.G. Law Governing the Cathode Drop and the Threshold Currents in an Arc Discharge in a Pure Metal. Sov. Phys.-Tech. Phys. 1964, 9, 1146–1154. [Google Scholar]
- Kesaev, I.G. Cathode Processes in Electric Arcs; NAUKA Publishers: Moscow, Russia, 1968. [Google Scholar]
- Grakov, V.E. Cathode Fall of an Arc Discharge in a Pure Metal. Sov. Phys.-Tech. Phys. 1967, 12, 286–292. [Google Scholar]
- Davis, W.D.; Miller, H.C. Analysis of the electrode products emitted by DC arcs in a vacuum ambient. J. Appl. Phys. 1969, 40, 2212–2221. [Google Scholar] [CrossRef]
- Plyutto, A.A.; Ryzhkov, V.N.; Kapin, A.T. High speed plasma streams in vacuum arcs. Sov. Phys. JETP 1965, 20, 328–337. [Google Scholar]
- Kutzner, J.; Miller, H.C. Integrated Ion Flux Emitted from the Cathode Spot Region of a Diffuse Vacuum-Arc. J. Phys. D Appl. Phys. 1992, 25, 686–693. [Google Scholar] [CrossRef]
- Tanberg, R. On the cathode of an arc drawn in vacuum. Phys. Rev. 1930, 35, 1080–1989. [Google Scholar] [CrossRef]
- Kimblin, C.W. Vacuum Arc Ion Currents and Electrode Phenomena. Proc. IEEE 1971, 59, 546–555. [Google Scholar] [CrossRef]
- Kimblin, C.W. Erosion and Ionization in Cathode Spot Regions of Vacuum Arcs. J. Appl. Phys. 1973, 44, 3074–3081. [Google Scholar] [CrossRef]
- Rakhovsky, V.I. Experimental study of the dynamics of cathode spots development. IEEE Trans. Plasma Sci. 1976, 4, 87–102. [Google Scholar] [CrossRef]
- Tuma, T.D.; Chen, C.L.; Davies, D.K. Erosion Products from the Cathode Spot Region of a Copper Vacuum Arc. J. Appl. Phys. 1978, 49, 3821–3831. [Google Scholar] [CrossRef]
- Daalder, J.E. Erosion and the origin of charged and neutral species in vacuum arcs. J. Phys. D Appl. Phys. 1975, 8, 1647–1659. [Google Scholar] [CrossRef]
- Juttner, B.; Puchkarev, V. Phenomenology. In Handbook of Vacuum Arc Science and Technology; Noyes Publications: Park Ridge, NJ, USA, 1995; pp. 73–151. [Google Scholar]
- Djakov, B.E. Cathode spot phenomena in low current vacuum arcs on arc cleaned electrode surfaces Spot Dynamics. Contrib. Plasma Phys. 1993, 33, 201–207. [Google Scholar] [CrossRef]
- Djakov, B.E.; Holmes, R. Cathode spot structure and dynamics in low-current vacuum arcs. J. Phys. D Appl. Phys. 1974, 7, 569–580. [Google Scholar] [CrossRef]
- Djakov, B.E.; Holmes, R. Cathode spot division in vacuum arcs with solid metal cathodes. J. Phys. D Appl. Phys. 1971, 4, 504–509. [Google Scholar] [CrossRef]
- Siemroth, P.; Schuke, T.; Witke, T. Investigation of cathode spots and plasma formation of vacuum arcs by high speed microscopy and spectroscopy. IEEE Trans. Plasma Sci. 1977, 25, 571–579. [Google Scholar] [CrossRef]
- Achtert, J.; Altrichter, B.; Juttner, B.; Peach, P.; Pusch, H.; Reiner, H.D.; Rohrbeck, W.; Siemroth, P.; Wolf, P. Influence of surface contaminations on cathode processes of vacuum discharges. Beitr. Plasma Phys. 1977, 17, 419–431. [Google Scholar] [CrossRef]
- Beilis, I.I.; Djakov, B.E.; Juttner, B.; Pusch, H. Structure and dynamics of high-current arc cathode spots in vacuum. J. Phys. D Appl. Phys. 1997, 30, 119–130. [Google Scholar] [CrossRef]
- Jüttner, B. Cathode spots of electric arcs. J. Phys. D Appl. Phys. 2001, 34, R103–R123. [Google Scholar] [CrossRef]
- Anders, S.; Anders, A. Emission Spectroscopy of Low-current Vacuum Arcs. J. Phys. D Appl. Phys. 1991, 24, 1986–1992. [Google Scholar] [CrossRef]
- Anders, A.; Anders, S.; Jüttner, B.; Botticher, W.; Luck, H.; Schroder, G. Pulsed dye laser diagnostics of vacuum arc cathode spots. IEEE Trans. Plasma Sci. 1992, 20, 466–472. [Google Scholar] [CrossRef]
- Anders, A.; Anders, S.; Jüttner, B. Brightness distribution and current density of vacuum arc cathode spots. J. Phys. D Appl. Phys. 1992, 25, 1591–1599. [Google Scholar] [CrossRef]
- Beilis, I.I.; Sagi, B.; Zhitomirsky, V.; Boxman, R.L. Cathode spot motion in a vacuum arc with a long roof-shaped cathode under magnetic field. J. Appl. Phys. 2015, 117, 233303. [Google Scholar] [CrossRef]
- Raizer, Y.P. Gas Discharge Physics; Springer: Berlin, Germany, 1997. [Google Scholar]
- Braginsky, S.I. Phenomena of transferring in a plasma. In Reviews of the Plasma Physics; Leontovich, M.A., Ed.; Consultants Bureau: New York, NY, USA, 1965; Volume 1. [Google Scholar]
- Artsimovich, L.A.; Sagdeev, R.Z. Plasma Physics for Physicists; Springer: Berlin, Germany, 1983. [Google Scholar]
- Shkarofsky, I.P.; Johnston, T.W.; Bachynski, M.P. The Particle Kinetics of Plasmas; Addison Wesley Publishing Company: Reading, MA, USA, 1966. [Google Scholar]
- Granowski, W.L. Der Elektrische Strom Im Gas. B.1. Allgemeine Probleme der Elektrodynamik der Gase; Akademie Ferlag: Berlin, Germany, 1955. [Google Scholar]
- McDaniel, E.W. Collision Phenomena in Ionized Gases; John Wiley: New York, NY, USA; London, UK, 1964. [Google Scholar]
- Biberman, L.M.; Vorob’ev, V.S.; Yakubov, I.T. Kinetics of Nonequilibrium Low-Temperature Plasmas; Consultants Bureau: New York, NY, USA, 1987. [Google Scholar]
- Carslaw, H.S.; Jaeger, J.C. Conduction of Heat in Solids; Clarendon Press: Oxford, UK, 1959. [Google Scholar]
- Dushman, S. Scientific Foundation of Vacuum Technique; Lafferty, J.M., Ed.; John Wiley & Sons: New York, NY, USA, 1962. [Google Scholar]
- Anisimov, S.I.; Imas, Y.A.; Romanov, S.G.; Khodyko, Y.V. Action of High Power Radiation on Metals; National Technical Information Service: Springfield, VA, USA, 1971.
- Miller, H.C. Vacuum Arcs. IEEE Trans. Plasma Sci. 2023, 51, 1585–1594. [Google Scholar] [CrossRef]
- Mackeown, S.S. The cathode drop in an electric arc. Phys. Rev. 1929, 34, 611–614. [Google Scholar] [CrossRef]
- Ecker, G. Electrode components of the arc discharge. Ergeb. Exakten Naturwiss 1961, 33, 1–104. [Google Scholar]
- Lee, T.H.; Greenwood, A. Theory for the cathode mechanism in metal vapor arcs. J. Appl. Phys. 1961, 32, 916–923. [Google Scholar] [CrossRef]
- Ecker, G. The existence diagram a useful theoretical tool in applied physics. Z. Naturforschung A 1971, 26, 935–939. [Google Scholar] [CrossRef]
- Ecker, G. Zur theorie des vakuumbogens. Beitr. Plasmaphys. 1971, 11, 405–415. [Google Scholar] [CrossRef]
- Rothstein, J. The arc spot as a steady state exploding wire phenomena. In “Exploding Wires”, Version 3; Chace, W.G., Moore, H.K., Eds.; Plenum: New York, NY, USA, 1964; pp. 115–124. [Google Scholar]
- Dyke, W.P.; Trolan, J.K.; Martin, E.E.; Barbour, J.P. The Field Emission Initiated Vacuum arc I. Experiments Arc Initiation. Phys. Rev. 1953, 91, 1043–1054. [Google Scholar] [CrossRef]
- Flynn, P.T.G. The Discharge Mechanism in the High-Vacuum Cold-Cathode Pulsed X-ray Tube. Proc. Phys. Soc. 1956, 69, 748–762. [Google Scholar] [CrossRef]
- Kassirov, G.M.; Mesyats, G.A. Breakdown mechanism of short vacuum gaps. Sov. Phys. Tech. Phys. 1964, 9, 1141–1145. [Google Scholar]
- Mesyats, G.A.; Proskurovskii, D.I. Explosive electron emission from metallic needles. Sov. Phys JETP Let. 1971, 13, 4–6. [Google Scholar] [CrossRef]
- Fursey, G.N.; Vorontsov-Vel’yaminov, P.N. Qualitative model of initiation of a vacuum arc. Sov. Phys. Tech. Phys. 1967, 12, 1370–1382. [Google Scholar]
- Bugaev, S.P.; Litvinov, E.A.; Mesyats, G.A.; Proskurovskii, D.I. Explosive Emission of Electrons. Sov. Phys. Usp. 1975, 18, 51–61. [Google Scholar] [CrossRef]
- Fursey, G.N. Field Emission and Vacuum Breakdown. IEEE Trans. Electr. Insul. 1985, E–20, 659–679. [Google Scholar] [CrossRef]
- Mesyats, G.A.; Proskurovskii, D.I. Pulsed Electrical Discharge in Vacuum; Springer: Berlin, Germany, 1989. [Google Scholar]
- Mesyats, G.A. The Role of Fast Processes in Vacuum Breakdown; Phenomena in Ionized Gases: Oxford, UK, 1971; p. 333. [Google Scholar]
- Litvinov, A.; Mesyats, G.A.; Parfenov, A.G. The nature of explosive electron emission. Sov. Phys.-Dokl. 1983, 28, 272–273. [Google Scholar]
- Mesyats, G.A. Cathode Phenomena in a Vacuum Discharge: The Breakdown, the Spark, and the Arc; Nauka: Moscow, Russia, 2000. [Google Scholar]
- Barengolts, S.A.; Mesyats, G.A. Explosive emission processes in thermonuclear facilities with magnetic plasma confinement and in linear electron positron colliders. Phys. Uspekhi 2023, 66, 704–721. [Google Scholar] [CrossRef]
- Beilis, I.I.; Garibashvily, I.D.; Mesyats, G.A.; Skvortsov, V.A.; Fortov, V.E. Electromagnetic and gasdynamic of the explosive electron emission processes from metal spikes. In Proceedings of the RXIV-th Internaional Symposium on Discharge and Electrical Insulation in Vacuum, Santa Fe, NM, USA, 17–20 September 1990; pp. 548–551. [Google Scholar]
- Uimanov, I.V. A two-dimensional nonstationary model of the initiation of an explosive center beneath the plasma of a vacuum arc cathode spot. IEEE Trans. Plasma Sci. 2003, 31, 822–826. [Google Scholar] [CrossRef]
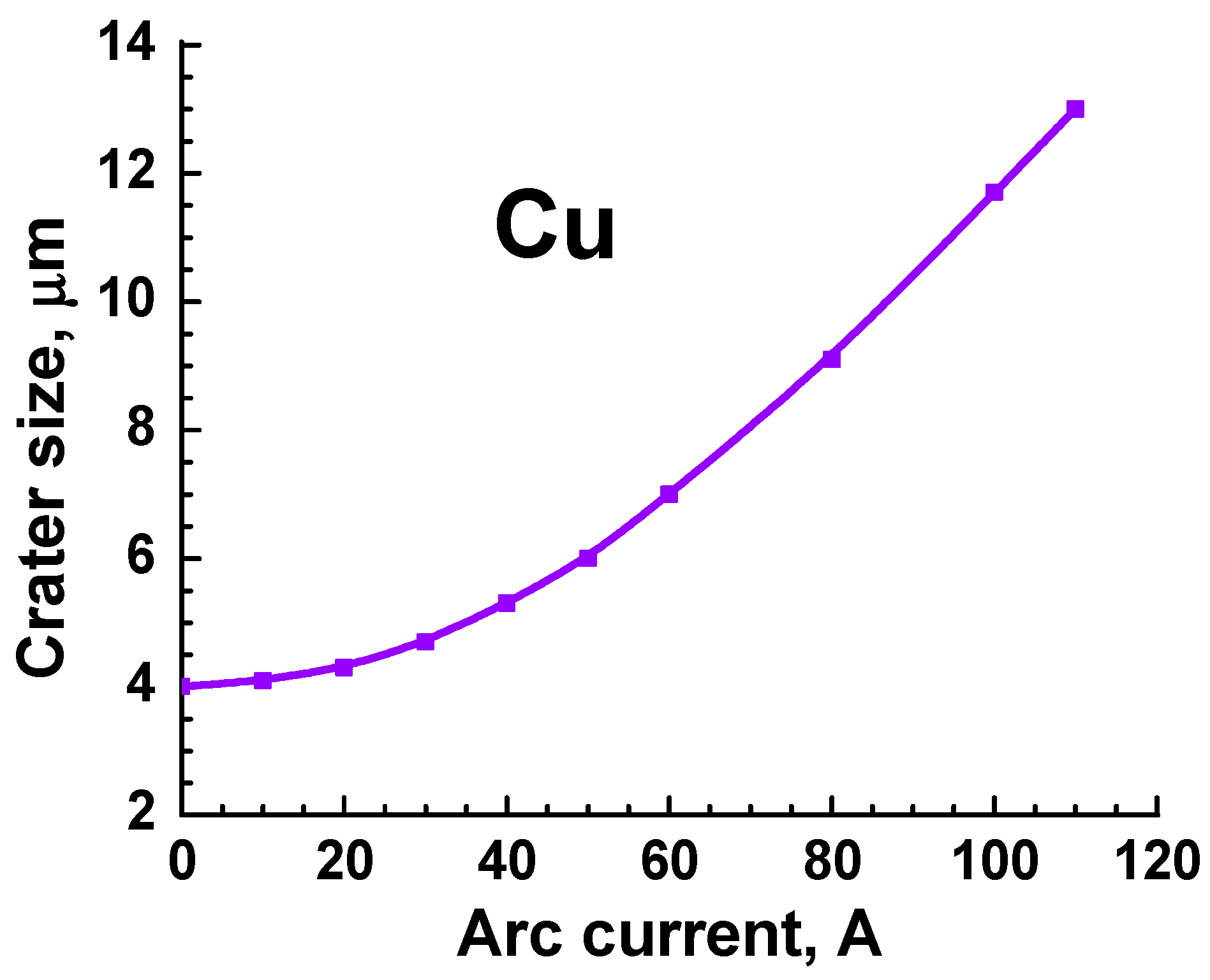
- Daalder, J. Diameter and current density of single and multiple cathode discharges in vacuum. IEEE Trans. Power Syst. 1974, PAS–93, 1747–1758. [Google Scholar] [CrossRef]
- Hitchcock, A.H.; Guile, A.E. A scanning electron microscope study of the role of copper oxide layers on arc cathode erosion rates. J. Mater. Sci. 1977, 12, 1095–1104. [Google Scholar] [CrossRef]
- Rich, J.A. Resistance heating in the arc cathode spot zone. J. Appl. Phys. 1961, 32, 1023–1031. [Google Scholar] [CrossRef]
- Beilis, I.I.; Lyubimov, G.A. Parameters of cathode region of vacuum arc. High Temp. 1975, 13, 1057–1064. [Google Scholar]
- Puchkarev, V.F.; Bochkarev, M.B. Cathode spot initiation under plasma. J. Phys. D Appl. Phys. 1994, 27, 1214–1219. [Google Scholar] [CrossRef]
- Budenstein, P.P.; Hayes, P.J. Breakdown Conduction in Al-SiO-Al Capacitors. J. Appl. Phys. 1967, 38, 2837–2851. [Google Scholar] [CrossRef]
- Klein, N.; Budenstein, P.P. Electrical Pulse Breakdown of Silicon Oxide Films. J. Appl. Phys. 1969, 40, 2728–2740. [Google Scholar] [CrossRef]
- Hancox, R. Importance of insulating inclusions in arc initiation. Br. J. Appl. Phys. 1960, 11, 468. [Google Scholar] [CrossRef]
- Minnebaev, K.F.; Rau, E.I.; Tatarintsev, A.A. Charging of dielectrics when bombarded with Ar+ ions medium energies. Solid State Phys. 2019, 61, 1090–1093. (In Russian) [Google Scholar] [CrossRef]
- Borisov, D.P.; Koval, N.N.; Kreindel, M.Y.; Litvinov, E.A.; Shchanin, P.M. Influence of surface state and voltages for breakdown of the near-electrode ionic layer in the plasma of a vacuum arc. ZhTF 1992, 62, 57–63. (In Russian) [Google Scholar]
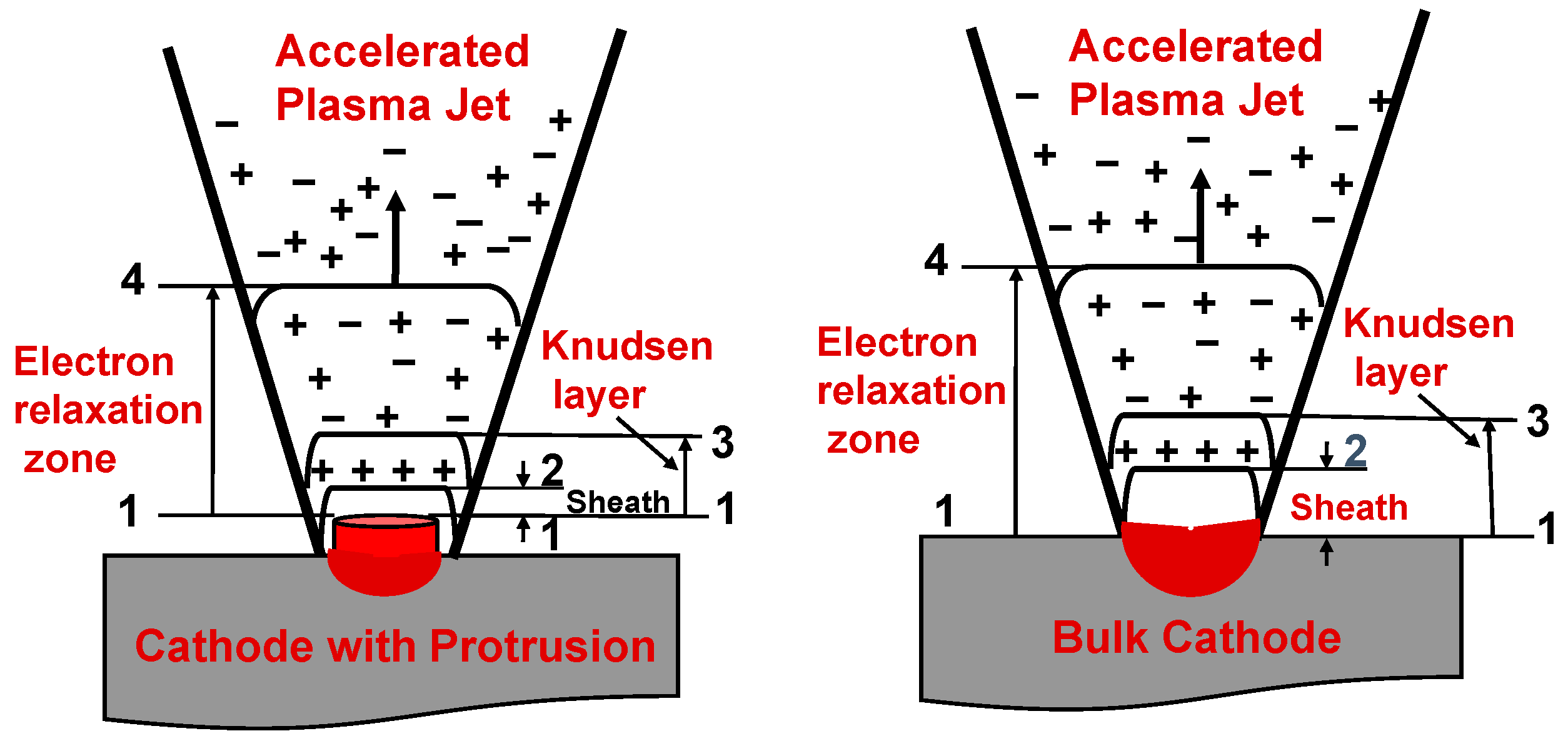
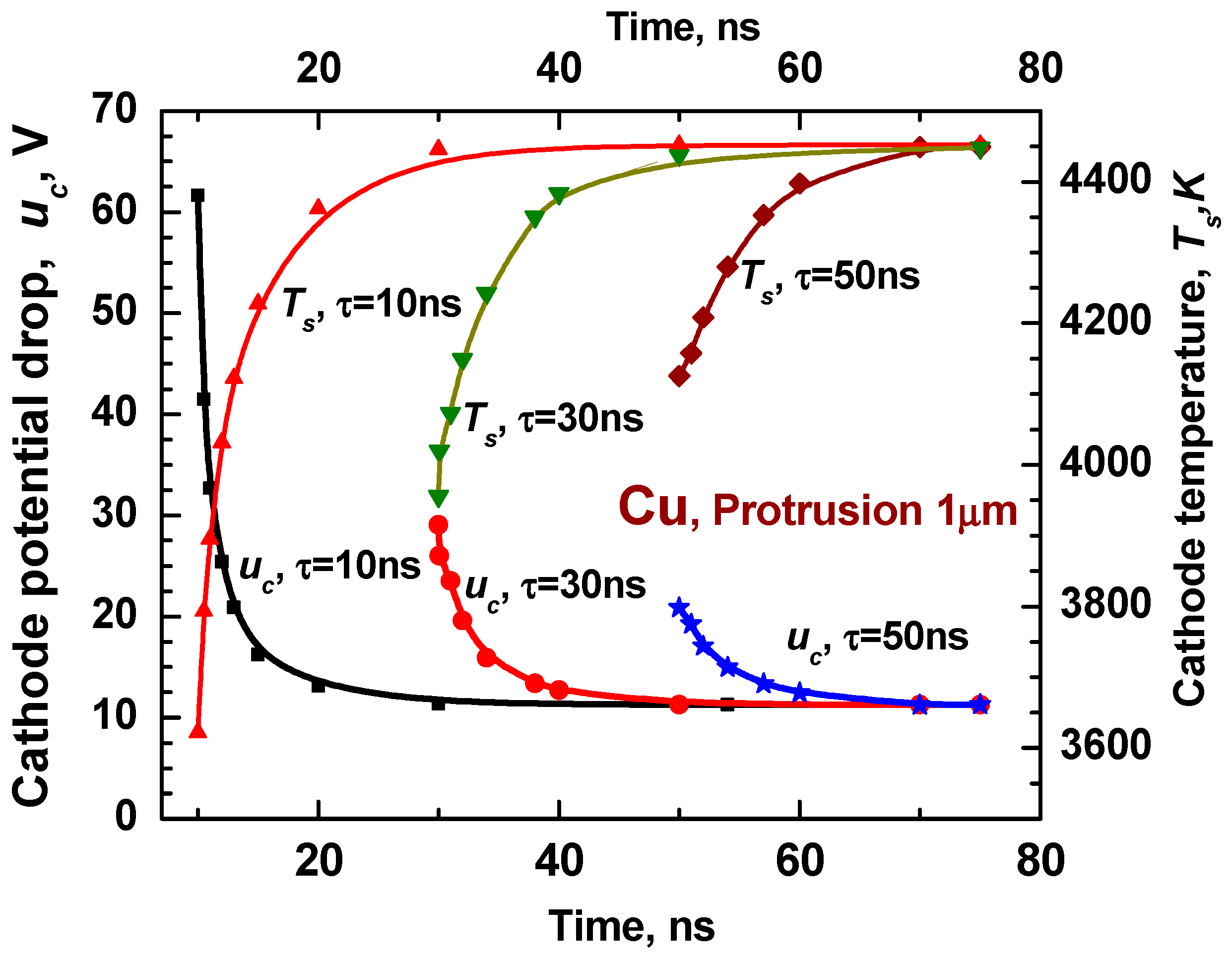
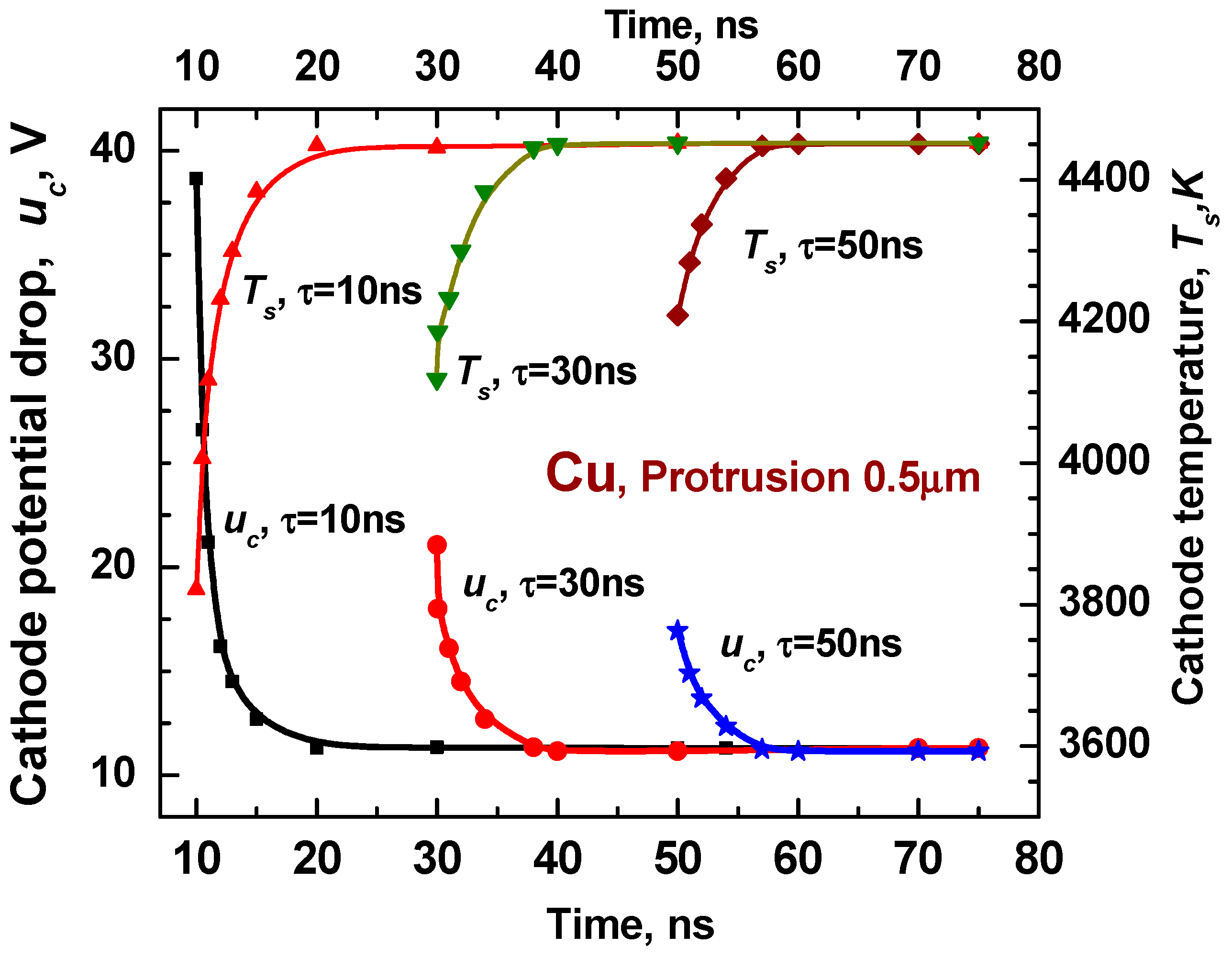
- Beilis, I.I. Continuous Transient Cathode Spot Operation on a Microprotrusion: Transient Cathode Potential Drop. IEEE Trans. Plasma Sci. 2011, 39, 1277–1283. [Google Scholar] [CrossRef]
- Beilis, I.I. Cathode Spot Development on a Bulk Cathode in a Vacuum Arc. IEEE Trans. Plasma Sci. 2013, 41 Pt II, 1979–1986. [Google Scholar] [CrossRef]
- Beilis, I.I. Vacuum Arc Cathode Spot Theory: History and Evolution of the Mechanisms. IEEE Trans. Plasma Sci. 2018, 47, 3412–3433. [Google Scholar] [CrossRef]
- Beilis, I.I. Arc Spot on Film Cathode Using Kinetic Approach of Plasma Flow. IEEE Trans. Plasma Sci. 2022, 50 Pt 2, 2714–2719. [Google Scholar] [CrossRef]
- Grakov, V. Cathode fall in vacuum arcs with deposited cathodes. Sov. Phys.-Tech. Phys. 1967, 12, 1248–1250. [Google Scholar]
- Tonks, L. A theory of liquid surface rupture by a uniform electric field. Phys. Rev. 1935, 48, 562–568. [Google Scholar] [CrossRef]
- Daalder, J.E. Energy dissipation in the cathode of a vacuum arc. J. Phys. D Appl. Phys. 1977, 10, 2225–2254. [Google Scholar] [CrossRef]
- Beilis, I.I.; Koulik, Y.; Boxman, R.L. Effective Cathode Voltage in a Vacuum Arc with a Black Body Electrode Configuration. IEEE Trans. Plasma Sci. 2013, 41, 1992–1995. [Google Scholar] [CrossRef]
- Beilis, I.I. Plasma Energy Loss by Cathode Heat Conduction in a Vacuum Arc: Cathode Effective Voltage. Plasma 2023, 6, 492–502. [Google Scholar] [CrossRef]
- Agarwal, M.S.; Holmes, R. Arcing voltage of the metal vapor vacuum arc. J. Phys. D Appl. Phys. 1984, 17, 757–767. [Google Scholar] [CrossRef]
- Keidar, M.; Schulman, M.B. Modeling the effect of an axial magnetic field on the vacuum arc. IEEE Trans. Plasma Sci. 2001, 29, 684–689. [Google Scholar] [CrossRef]
- Agarwal, M.S.; Holmes, R. Cathode spot motion in high-current vacuum arcs under self-generated azimuthal and applied axial magnetic fields. J. Phys. D Appl. Phys. 1984, 17, 743–756. [Google Scholar] [CrossRef]
- Beilis, I.I. Vacuum arc cathode spot grouping and motion in magnetic fields. IEEE Trans. Plasma Sci. 2002, 30, 2124–2132. [Google Scholar] [CrossRef]
- Beilis, I.I. Mechanism of cathode spot splitting in vacuum arcs in an oblique magnetic field. Phys. Plasmas 2015, 22, 103510. [Google Scholar] [CrossRef]
- Beilis, I.I. Vacuum arc cathode spot motion in oblique magnetic fields: An interpretation of the Robson experiment. Phys. Plasmas 2016, 23, 093501. [Google Scholar] [CrossRef]
- Beilis, I.I. Model of a steady-state arc spot on a refractory cathode in vacuum. Sov. Tech. Phys. Lett. 1988, 14, 494–495. [Google Scholar]
- Beilis, I.I. The nature of high voltage initiation of an electrical arc in a vacuum. Appl. Phys. Lett. 2010, 97, 121501. [Google Scholar]


| Characteristics | Kind of Spot Motion | Arc and Cathode Surface Conditions | Range of Parameters | ||
|---|---|---|---|---|---|
| vs, cm/s | Is, A | ts, μs | |||
| Superfast spot | Fast | Pollution surface or with contamination | 103–104 | ≤10 | 0.1–10 |
| Moderately fast | Cleaned cathode surface | ≤103 | ≤10 | <0.1–10 | |
| Film cathode | Cathode with metallic films | ≥103 | ~0.1 | 1–100 | |
| Group spot | Under a magnetic field | up to 103 | Few of ten | 1–10 | |
| Group spot | Slow | In a vacuum | ≤10 | 100–300 | 102–103 |
| Individual spots | Arc with filled gas in electrode gap | 10–100 | 10 | ≤100 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Beilis, I.I. The Phenomenon of a Cathode Spot in an Electrical Arc: The Current Understanding of the Mechanism of Cathode Heating and Plasma Generation. Plasma 2024, 7, 329-354. https://doi.org/10.3390/plasma7020019
Beilis II. The Phenomenon of a Cathode Spot in an Electrical Arc: The Current Understanding of the Mechanism of Cathode Heating and Plasma Generation. Plasma. 2024; 7(2):329-354. https://doi.org/10.3390/plasma7020019
Chicago/Turabian StyleBeilis, Isak I. 2024. "The Phenomenon of a Cathode Spot in an Electrical Arc: The Current Understanding of the Mechanism of Cathode Heating and Plasma Generation" Plasma 7, no. 2: 329-354. https://doi.org/10.3390/plasma7020019
APA StyleBeilis, I. I. (2024). The Phenomenon of a Cathode Spot in an Electrical Arc: The Current Understanding of the Mechanism of Cathode Heating and Plasma Generation. Plasma, 7(2), 329-354. https://doi.org/10.3390/plasma7020019





