3.1. Fundamentals of Energy-Dispersive X-ray Spectroscopy (EDX)
As we will see in subsequent sections, our proof of principle rests on the basis of reliable results from a conventional EDX characterization. Therefore, it is timely to review both the operating principles of the technique and the minimum requirements necessary to achieve a correct characterization using EDX.
The basic principle of operation of EDX begins when the surface of a material is “illuminated” with a beam of electrons. Said illumination beam causes the expulsion of secondary electrons from the atoms that make up the sample. The ejected electrons leave vacancies in the lower energy levels of the atom. The vacancies are occupied by electrons from upper shells, following the selection rules of quantum mechanics (
) [
12]. The energy difference between the upper level, from where the replacement electron descends, and the energy level that was vacated by irradiation with the microscope’s electron beam is precisely the energy of the X-ray emitted by the atom [
17]. Because the differences between the energy levels of each element are unique and characteristic, it is possible to identify the presence of a specific element by detecting the energy of the X-rays it emits.
It is essential to note that EDX does not provide us with information about the chemical bonds of the sample studied; it only tells us what elements are present. In other words, EDX is an elemental analysis technique, not a chemical analysis technique [
18]. Furthermore, we must consider that EDX is a semiquantitative technique because it is based on the premise that if an element is more abundant in the sample, more characteristic X-rays will be detected from that element. Consequently, the weight percentages (wt%) obtained from this technique are proportional to the number of X-rays produced by the elements that make up the sample, i.e., the greater abundance of a given element equals a greater amount of characteristic X-rays produced and, therefore, a greater wt%.
Although EDX cannot specify the existence of chemical compounds, if performed correctly, it provides a very reliable wt% [
19]. Attention must be paid to multiple requirements to perform an EDX analysis, including the technical characteristics of the equipment, the type and location of the X-ray detectors, or the accelerating voltages. However, three thumb rules are standard for all equipment and all materials whose constituent elements have an atomic number equal to or greater than 5 [
18,
20], and these are:
- (a)
The acceleration voltage for the “illumination” beam must be at least 2.5 times the K orbital energy of the heaviest element present in the sample.
- (b)
The exposure time must be sufficient for all elements present in the sample to reach 3000 counts or more.
- (c)
The wt% obtained for each element must be over three times its standard deviation. Otherwise, the element must be discarded, as its statistical representativeness is not sufficiently robust. The total wt% will only include those elements that meet the criterion that wt% > 3 * σwt%.
Furthermore, to improve the accuracy of quantitative results, the ZAF correction method has been developed. It accounts for three major effects that influence the intensity of characteristic X-rays emitted from a sample:
Atomic number (Z) effect: differences in atomic number between the sample and the standard used for calibration can influence the generation and backscattering of X-rays.
Absorption (A) effect: X-rays generated within the sample can be absorbed by the surrounding material before reaching the detector, affecting the measured intensity.
Fluorescence (F) effect: high-energy X-rays from one element can excite secondary X-rays from another element, leading to an overestimation of the second element’s concentration.
The ZAF method involves dividing the measured intensities by the intensities obtained from a known standard of similar composition to obtain k-ratios. Corrections for the Z, A, and F effects are then iteratively applied to the k-ratios using theoretical models, and calculations based on the sample composition, X-ray energies, and detector geometry. These corrected k-ratios are used to calculate the weight or atomic percentages of the elements in the sample [
21].
Using the ZAF correction method ensures the more accurate quantification of elements by compensating for these physical effects that can otherwise skew the results. This method has also spurred the development of more advanced quantification algorithms, such as AZtec Oxford’s True-Q, which are more suitable for analyzing light elements.
3.2. Foundational Elements of the Analysis Methodology
This section defines the elements that form the foundation of our proposed analysis methodology. Likewise, in
Section 3.3, we will apply this methodology to a set of thin films of silicon oxynitride (SiON), grown with different deposition conditions to modify their physical and compositional features characteristics.
Because we will start analyzing the stoichiometry of Si, N, and O compounds, let us start by selecting the compound we will use as a reference to evaluate stoichiometry. We have chosen stoichiometric silicon oxynitride, whose molecular formula is Si
2N
2O [
22]. Based on this compound, we will determine the proximity or remoteness of any other Si, N, and O material in terms of stoichiometry.
One of the pivotal aspects of our methodology is the in-depth analysis of the stoichiometric relationships between the three elements that constitute SiON. This analysis will enable us to discern how many nitrogen or oxygen atoms silicon can chemically bond with. To accomplish this, we can start with the ideal stoichiometric silicon dioxide, whose molecular formula is SiO
2 [
23]. In this compound, we observe that each Si atom can chemically bond with two O atoms. On the other hand, to understand how silicon bonds with nitrogen, we analyze the ideal stoichiometric silicon nitride, Si
3N
4 [
24]. In silicon nitride, three Si atoms bond with four N atoms. In other words, each Si atom is associated with 1.33 N atoms.
From the analysis of silicon dioxide and silicon nitride, we have determined the stoichiometric relationships between the elements of interest for SiON, concluding that Si can bond with 2 O or with 1.33 N. This relationship is critical because it indicates that when replacing oxygen with nitrogen in the SiO2 formula, we should do so in a proportion that incorporates 0.66 nitrogen for each oxygen removed. In our reference compound, Si2N2O, we found a ratio of two N atoms and one O atom for every two Si atoms, which confirms that the stoichiometric relationships observed in SiO2 and Si3N4 are maintained. Likewise, we can identify a stoichiometry line with SiO2 and Si3N4 at its ends and passing through Si2N2O. This line is represented by the equation Si3−yN4−2yOy for .
Once the stoichiometric relationships have been established, it is convenient to identify the molar proportions and of the compounds that we use to identify these relationships. These proportions are derived directly from their molecular formulas. We observe that in SiO2, there are no nitrogen atoms; therefore, , while the oxygen-to-silicon ratio is two to one, resulting in . In the case of Si3N4, we have and . Finally, the molar proportions of Si2N2O are and .
This molar ratio calculation is not limited to the molecular formulas of ideal stoichiometric compounds. It is also possible to calculate molar proportions from the weight percentages (wt%) of each constituent element of a compound, as obtained in EDX analyses. To calculate this, follow the “Interconversion between weight percentages (wt%) and empirical molecular formula” procedure in the
Appendix A: Glossary of core concepts. This procedure allows us to elucidate the empirical molecular formula from the wt%, allowing us to directly determine the molar proportions of the compounds studied using EDX.
Up to this point, we have determined the stoichiometric relationships between the SiON elements. Likewise, we have identified the molar proportions of three ideal stoichiometric compounds formed by Si, N, and O. In addition, we know how to determine the molar proportions from the wt% obtained through an EDX analysis. Therefore, the strategy we will follow next will consist of comparing the molar proportions of the compounds characterized by EDX against the molar proportions of the three ideal stoichiometric compounds formed by Si, N, and O.
The comparison between molar proportions would only be useful if it could be quantified. To assign numerical values to our comparisons, we will define the N and O deviations as the absolute value of the difference between the molar ratio of the SiON compound under study and the molar ratio of the reference stoichiometric SiON (Si
2N
2O). The expressions for calculating the deviations of N (
) and O (
) are presented in Equations (1) and (2), respectively. In these equations,
and
represent the molar proportions in the analyzed SiON compound, while the values of one and one-half correspond to the proportions
and
of the reference compound Si
2N
2O.
Let us now introduce the concept of the total deviation index (TDI), which quantifies the magnitude of the deviation of a given SiON compound with respect to the Si
2N
2O that we are using as a reference. This index offers a numerical value that allows for a direct comparison of the compound under study against the reference compound. The TDI is calculated as the sum of
plus
and is presented in Equation (3).
Suppose that a certain SiON compound under analysis is identical to Si2N2O; in that scenario, we would have and , leading to both and being zero. Consequently, the TDI is also equal to zero. Due to the absolute values in the and expressions, the minimum possible value for the TDI is zero. As we have observed, this situation occurs when the studied compound has molar proportions identical to those of the reference compound. On the other hand, any TDI value different from zero will be positive, indicating that the higher this value, the more significant the discrepancy between the molar proportions of the analyzed compound and those of the reference compound. Based on the above, we can see that the TDI behaves analogously to the magnitude of a vector.
Now, let us calculate the TDIs of the compounds at the ends of the SiON stoichiometry line, i.e., Si3N4 and SiO2. If our analyzed compound is identical to Si3N4, then , , , and , and we obtain a first maximum value for the TDI, which will be 5/6 or approximately 0.83. At the other extreme, if the SiON compound is identical to SiO2, then , , , and , so we will obtain a second value maximum for the TDI, which is 5/2 or 2.5. The previous calculations allow us to affirm that the compound we are using as a reference (Si2N2O) is closer to Si3N4 than to SiO2.
Let us proceed one step further, analyzing what happens with the TDI of the SiON compounds that lie on the Si3−yN4−2yOy stoichiometry line for . We can immediately identify that when y = 0, we have Si3N4 with TDI = 0.83. For y = 1, we find our reference compound Si2N2O, whose TDI is 0; finally, when y = 2, the compound is SiO2 with a TDI of 2.5. Therefore, we identify that when starting at y = 0, we have a TDI of 0.83 that decreases progressively as the value of y increases until it reaches its minimum value of zero when y = 1. However, if we continue increasing the value of y from 1 to 2, the TDI increases again from 0 to 2.5. The behavior of the TDI on the stoichiometry line confirms its character as a vector magnitude.
At this point, we can safely refer to the TDI as the magnitude of a vector that correctly quantifies the differences between the molar proportions of the compounds under study and the reference compound. However, more than the TDI is needed to provide complete information about the studied compound. For example, in the SiON stoichiometry line, we find a pair of TDIs with a value equal to 0.5, the first occurring when y = 1/2 (Si5/2N3O1/2) and the second when y = 4/3 (Si5/3N4/3O4/3). The amount of oxygen in Si5/3N4/3O4/3 is more than double that in Si5/2N3O1/2, so both compounds’ compositional features and physical characteristics are considerably different; however, their TDI is the same. For this reason, it is crucial to not only know the TDI, which acts as the magnitude of a vector, but also determine its direction. We will call this vector the stoichiometric deviation vector (SDV).
To determine the deviation direction (DD) of the SDV, we will compare the molar proportions of nitrogen and oxygen with respect to the silicon (
and
) of the SiON compound for evaluation against the molar proportions of N and O for Si
2N
2O. For SiO
2, the molar proportions are
and
, while in Si
3N
4, they are
and
. Therefore, to change from the reference Si
2N
2O towards Si
3N
4 or SiO
2, the molar ratios must conform to the following sets of specific conditions:
| For Si3N4 | For SiO2 |
|
|
Consequently, any compound of Si, N, and O that meets the criteria and will have an SDV directed from Si2N2O towards Si3N4, with a magnitude defined by the TDI. Similarly, those compounds that comply with and will have an SDV pointing towards SiO2 with a magnitude equal to the TDI.
There are cases where there is no specific DD, such as when or . These compositions do not agree with the stoichiometric relationships observed in SiON composites, probably indicating the presence of a heterogeneous physical mixture of Si3N4 + SiO2 or reflecting limitations in the EDX characterization.
As a conclusion to the section, we have detailed that from the information obtained by the EDX technique, it is possible to determine a deviation magnitude (TDI) and a deviation direction (DD), which together form the stoichiometric deviation vector (SDV). The need to define an SDV arises from being able to represent whether the molar proportions of the compound under analysis make it similar to Si3N4 or SiO2. All those compounds that meet one or another set of specific deviation conditions will have a defined direction. On the other hand, compounds that do not meet pre-established sets of criteria will lack such a vector, probably indicating the presence of atypical phases or mixtures, which require deeper characterization to understand their structure, composition, and physical and compositional features characteristics traits.
3.3. Application of the Analysis Methodology to Silicon Oxynitrides: A Proof of Principle
To experimentally evaluate the compositions, ten silicon oxynitride (SiON) films were grown using RF sputtering equipment. The common deposition parameters are described in the section “Deposition and Characterization Equipment and Procedures”. The specific variations for each sample are presented in
Table 1.
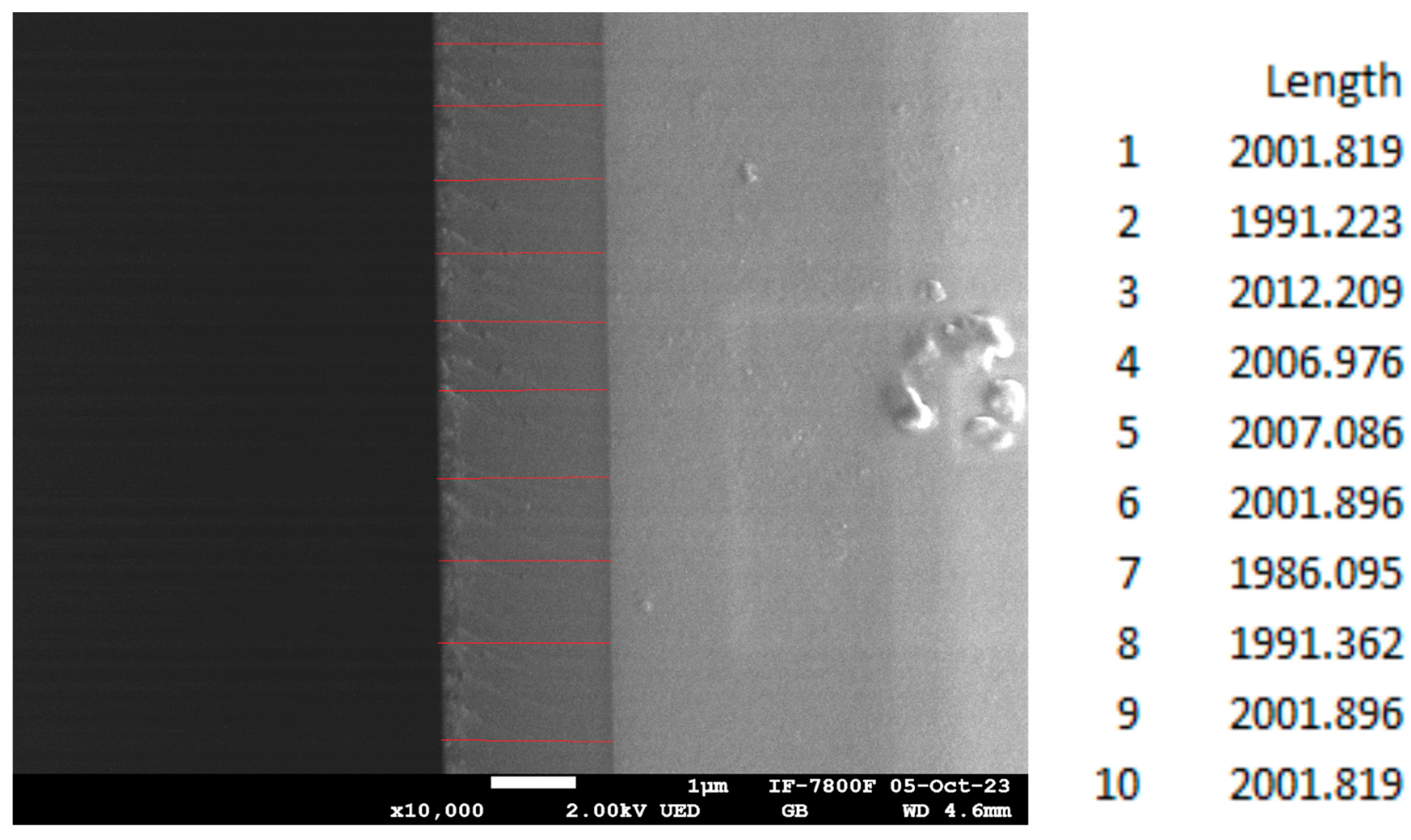
To generate an appropriate volume of interaction that provides significant X-ray signals, all thin films were grown to an estimated thickness of 2 μm, as shown in
Figure 1, corresponding to sample 9.
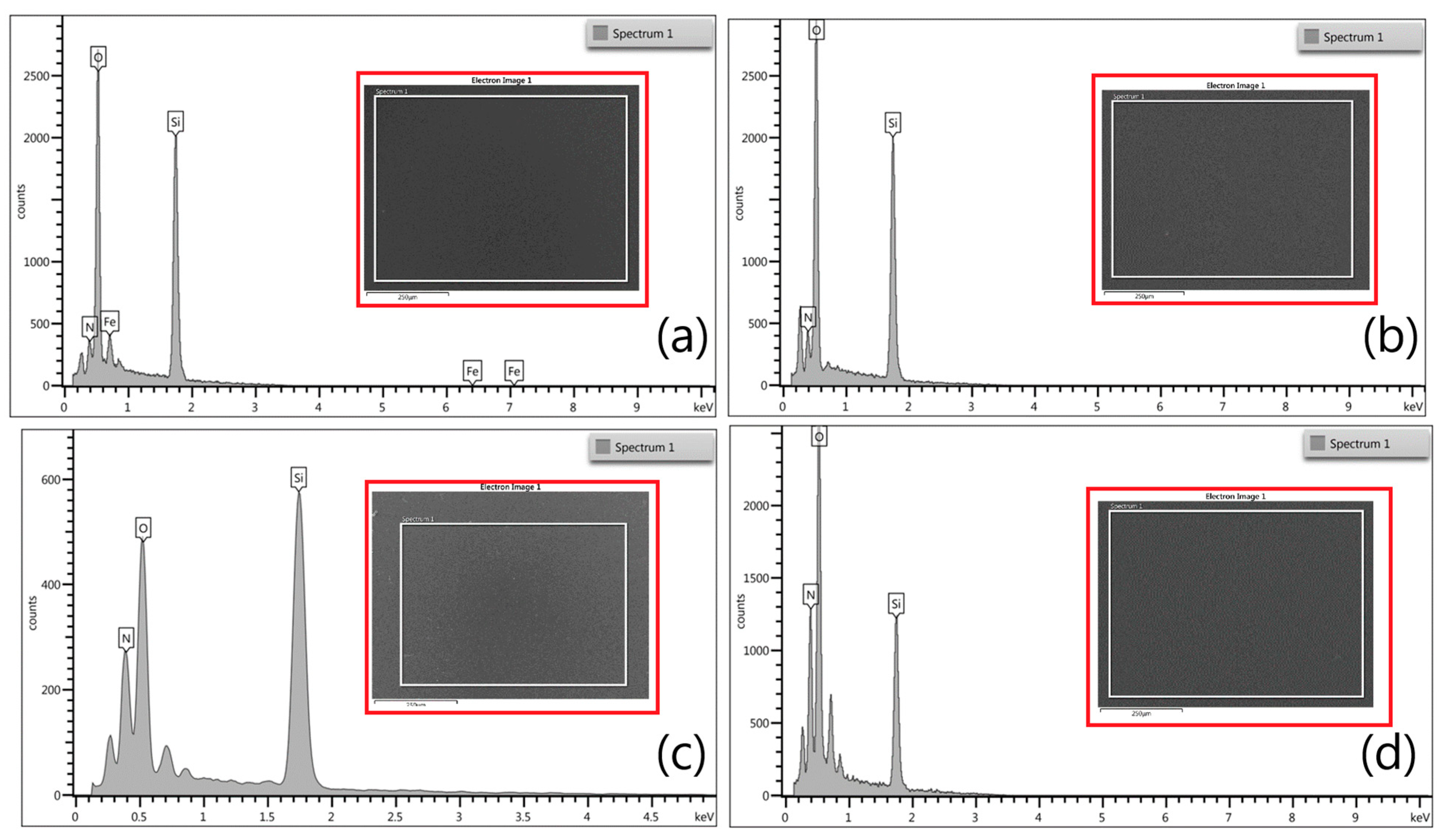
The resulting EDX spectra of samples 6, 7, 8, and 9 are shown in
Figure 2 (inset depicts the evaluated area). The presence of nitrogen, oxygen, and silicon is listed in
Table 2.
Subsequently, our SiON films’ weight percentages were analyzed to calculate the total deviation indices (TDIs). The sets of deviation direction criteria discussed in the previous section were applied to determine the presence of a stoichiometric deviation vector (SDV) and, if applicable, its direction. Our analysis indicates the formation of four compounds with similarities to SiO
2 (dioxide-like), six compounds without defined SDVs, and no compounds with SDVs directed toward Si
3N
4. These findings are summarized in
Table 2. Meanwhile,
Figure 3 illustrates samples with SDVs directed towards SiO
2 and displays the magnitude and direction of the resulting vectors.
To highlight the regions that meet the criteria for presenting an SDV either towards Si
3N
4 or SiO
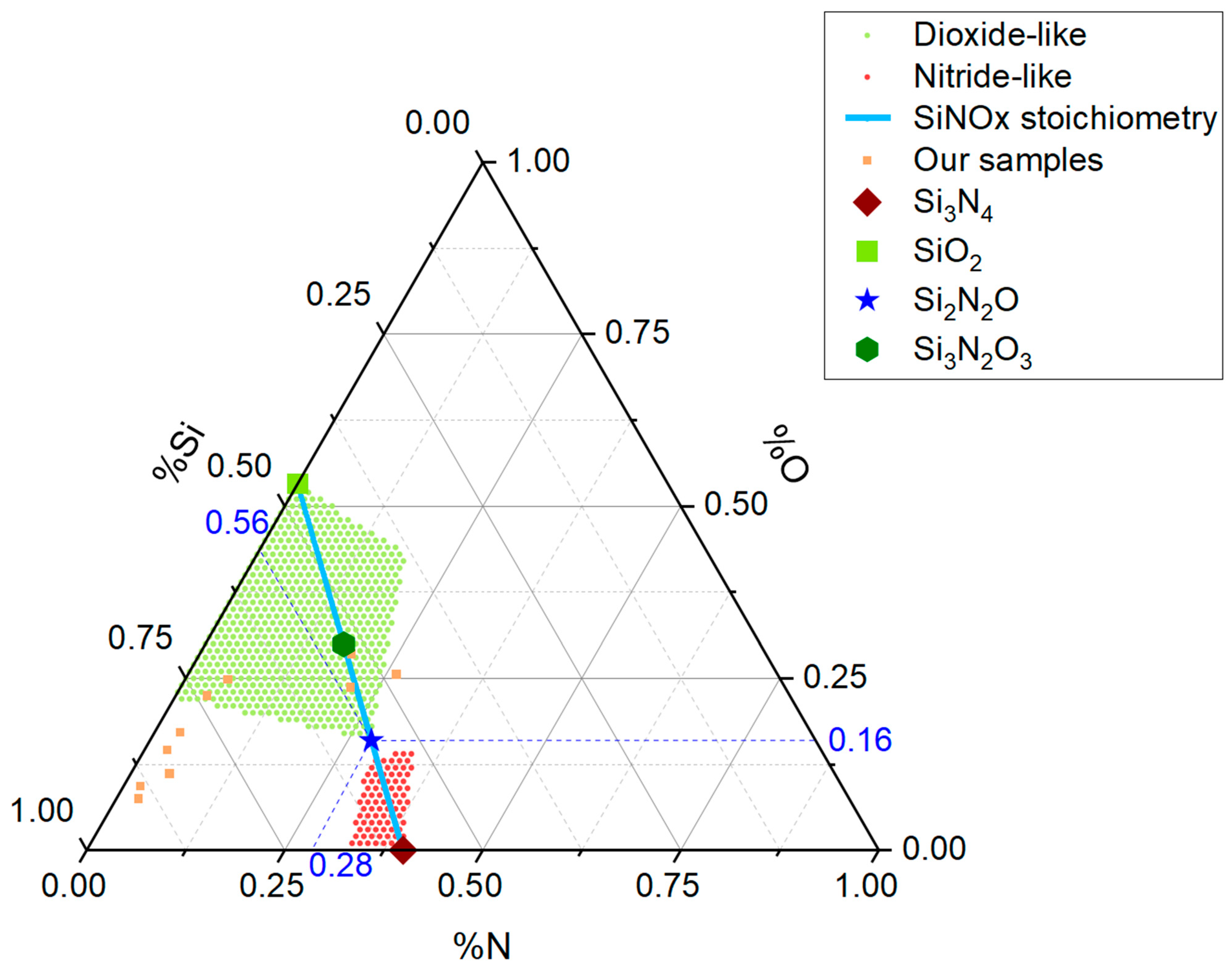
2, a ternary diagram is presented in
Figure 4. This diagram assesses the concentrations of nitrogen, oxygen, and silicon. It serves as a computational visualization tool designed to illustrate how the presence of a stoichiometric deviation vector (SDV) positions a compound within a specific region of the diagram, suggesting compositional similarity with stoichiometric compounds.
This ternary diagram was generated by discretizing the possible concentration universe of nitrogen, oxygen, and silicon, starting at [0 0 100], where the first column represents the weight percentage of N, the second of O, and the third of Si. The result was 5185 distinct combinations. Upon applying the deviation criteria and calculating the TDIs for each of the 5185 combinations, it was identified that 672 (12.96%) of the discrete concentrations exhibited an SDV towards SiO2 (dioxide-like). On the other hand, only 84 concentrations (1.62%) were oriented towards Si3N4 (nitride-like).
In
Figure 4, the position of the stoichiometric compound Si
2N
2O with percentage concentrations of 27.96% N, 15.97% O, and 56.07% Si is represented by a star. Dashed blue lines were drawn from the Si
2N
2O to each of the three concentration axes, indicating the corresponding values in blue. To determine the concentration of any component element, one must draw lines parallel to the grid and identify the position in each of the three linear axes.
Figure 4 also displays the ten samples referenced in
Table 2 and the locations of the stoichiometric compounds Si
3N
2O
3, Si
3N
4, and SiO
2. The red and green areas represent concentrations with stoichiometric deviation vectors similar to nitride and dioxide, respectively. Furthermore, the stoichiometric line, described by the formula Si
3−yN
4−2yO
y, is incorporated. The stoichiometric line connects the ideal stoichiometric compounds Si
3N
4 and SiO
2. The analysis of our samples’ positions suggests that similarity to SiO
2 does not require very strict alignment with the trajectory represented by the formula Si
3−yN
4−2yO
y.
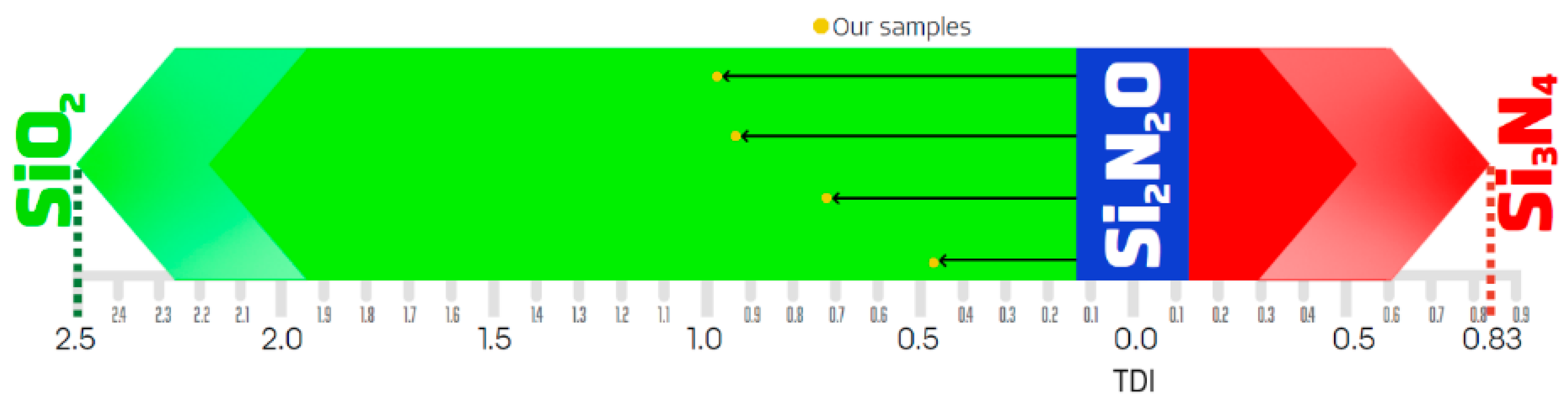
Figure 5 shows the TDIs with values less than or equal to 2.5. Within this representation, two regions are delimited for compounds with defined SDVs (regardless of their position with respect to the Si
3−yN
4−2yO
y line). The area limited by the blue line groups the concentrations classified as dioxide-like, while the red line confines the nitride-like components.
The color gradation reflects the magnitude of the TDI, varying from cool colors for lower values to warm colors for higher values. Specifically, in the dioxide-like zone, the TDIs gradually increase from 0, close to Si2N2O, until reaching 2.5 when approaching SiO2. Compounds with values close to 2.5 exhibit characteristics distinctly similar to silicon dioxide.
On the other hand, the extensive white region reflects TDI values that exceed the threshold of 2.5 with a tendency to infinity on the oxygen axis. Contrary to the compounds within the nitride- and dioxide-like zones, those located out from SiO2 or Si3N4 zones do not have a defined SDV as they do not meet the established criteria for deviation and magnitude. We estimate that Si, N, and O compounds without a defined SDV could indicate the presence of atypical structures or complex mixtures with physical and compositional feature characteristics that are substantially different from the compounds of the stoichiometric line.
It is essential to highlight that the SiON compounds found within the dioxide-like and nitride-like areas may not necessarily be ideal stoichiometric compounds or mixtures of SiO2 + Si3N4. Moreover, we know little about how the constituent elements might be bonded or whether the studied compounds might have even fallen into the regions due to the biases inherent in a conventional EDX analysis. However, our proposal is that despite not precisely knowing the compositional feature characteristics of the studied compound, it is possible to estimate whether the behavior, both compositional feature characteristics and physical characteristics, is more similar to Si2N2O, SiO2, or Si3N4 depending on the magnitude and direction of SDV for those SiON compounds that have fallen into the dioxide-like and nitride-like areas.
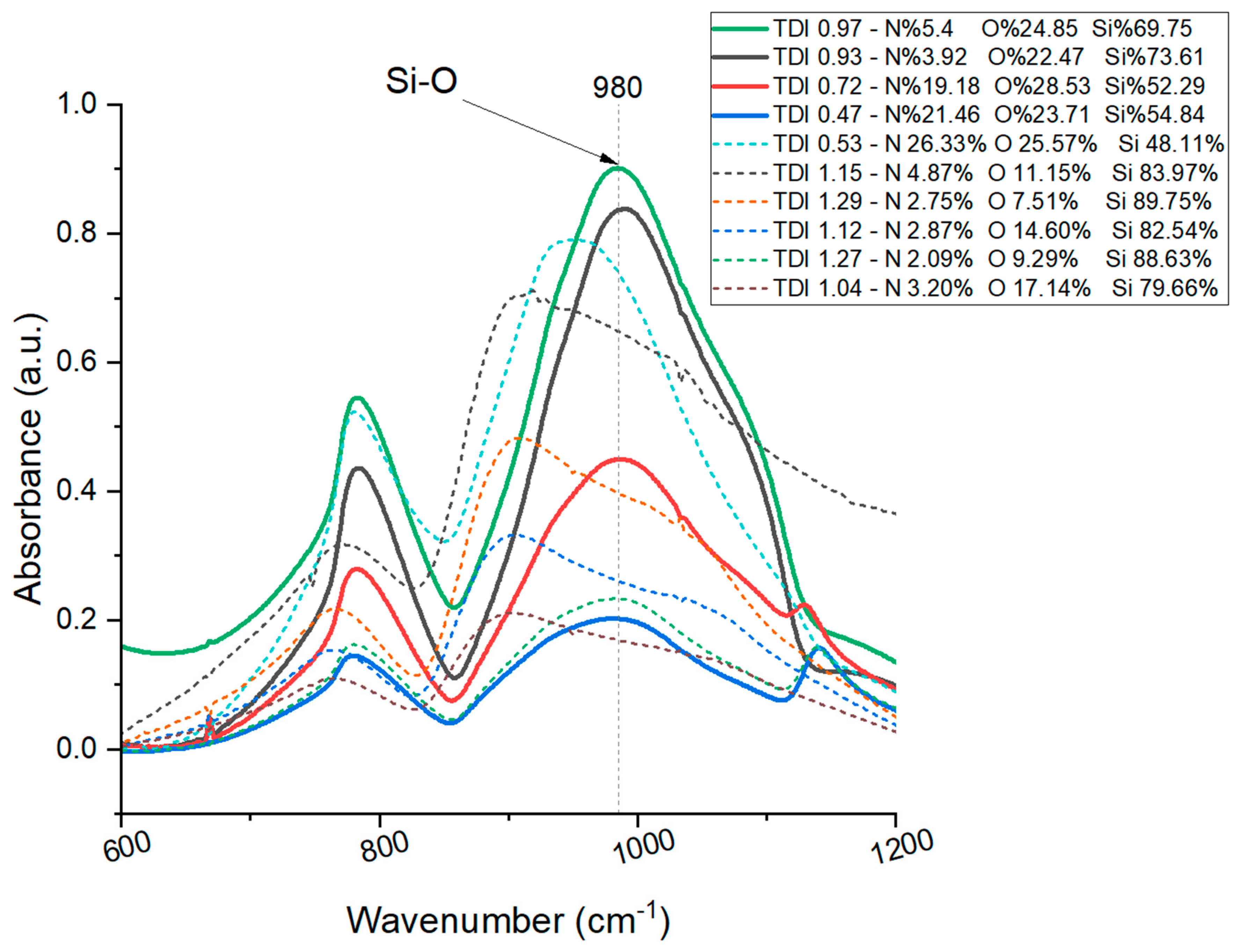
To prove the above, we performed a Fourier transform infrared spectroscopy characterization (FTIR) to validate the effectiveness of the SDV in predicting compositional features characteristics from the data obtained by EDX. Absorbance measurements were taken in three different areas for each sample, and the results were averaged to ensure a reliable representation.
Figure 6 presents the FTIR absorbance spectrum of our ten samples presented in
Table 2.
From
Figure 6, the spectra of compounds without a defined SDV (represented with dashed lines for differentiation) do not show peaks in the silicon–oxygen bond band. Conversely, for the four compounds with a determined SDV, the spectra reveal pronounced peaks at 980 cm
−1, corresponding to the absorption band of silicon–oxygen (Si-O) bonds [
25]. The observed trend is that as the TDI increases, the absorbance at 980 cm
−1 also intensifies, indicating a higher proportion of Si-O bonds characteristic of SiO
2. In contrast, as the TDI decreases, the absorbance at the peak diminishes, and the presence of nitrogen increases, suggesting a departure from the characteristics of SiO
2 and an approach to the properties of Si
2N
2O. To quantify this, we calculated the area under the curve in the FTIR spectra for compounds with a defined SDV as shown on
Table 3, which correlates with the number of bonds absorbing at that frequency.
Continuing with the evaluation of our methodology, we incorporated the calculation of the refractive index. These values are detailed in
Table 2. The refractive index of silicon oxynitrides can range from 1.44 (SiO
2) to 2.05 (Si
3N
4) [
26]. Our methodology predicts that compounds with a well-defined SDV and greater similarity to SiO
2 will have indices close to 1.44, while those with a lower oxygen proportion will approach 2.05. The refractive index listed in
Table 2 confirms this trend, showing a non-systematic oscillation in compounds without a defined SDV.
These findings are consistent with one of our initial hypotheses: it is possible to obtain insights into the compound’s physical and compositional feature characteristics by comparing molar proportions and defining the directions of the vectors.
3.4. Beyond Three Elements: Application of the Methodology to Aluminum–Silicon–Oxynitride Compounds
This section aims to demonstrate that our proof of principle is scalable to more complex materials. For this purpose, we will use the previous methodology, adding a new element: aluminum. Firstly, we present the algorithm used in the previous section as a set of six steps that can be tailored to different compounds:
Selecting a reference compound to evaluate the stoichiometry of the analyzed compounds.
Determining the stoichiometric relationships between the elements present in the reference compound.
Identifying the molar proportions and formulation of deviation equations.
Analyzing the samples under study using EDX to determine their constituent elements’ weight percentages (wt%). From the EDX wt%, we will determine the empirical molecular formula and the molar proportions of the compounds under study.
Evaluating the molar proportions of the studied compounds in the deviation equations and calculating the TDI.
Determining the SDV direction by indicating how the molar proportions change from the reference compound to the compounds at the stoichiometry lines’ ends.
By following these steps, we can easily tailor the algorithm to different compounds, making our methodology scalable and adaptable to a wide range of materials. Let us apply this algorithm now to compounds with more elements, such as aluminosilicate oxynitrides (SiAlON).
1. Selection of a reference compound.
As in the previous case, we start from a base ideal stoichiometric compound that will serve as a reference to assess the proximity or distance of the compounds to be evaluated. The ideal stoichiometry chosen as the basis for this proof of principle is Si
4Al
2O
2N
6, commonly abbreviated as SiAlON [
27].
2. Determination of stoichiometric relationships.
In order to determine the stoichiometric relationships in the four elements of SiAlON, we first need to identify other ideal stoichiometric compounds consisting of at least two SiAlON elements. We have identified six such compounds. Let us start by considering the two compounds from the previous case, SiO
2 and Si
3N
4. We will also add Si
2N
2O, which was used as a reference, to this list, thus completing the possible deviations towards silicon oxynitrides. We also need to evaluate deviations towards aluminum oxynitrides. The stoichiometric formulas that will serve as aluminum-based ideal stoichiometric compounds are AlN [
28], Al
23O
27N
5 [
29], and Al
2O
3 [
30].
For silicon oxynitrides, we can recall from the previous section that in SiO2, each Si atom can bond with two oxygen atoms. In Si3N4, the Si is associated with 1.33 N atoms; in Si2N2O, each silicon can be associated with 2 O or 1.33 N. Now, let us discuss the case of aluminum oxynitrides. In this case, aluminum is the element used to define the stoichiometric relationships. We observe that the aluminum nitrogen ratio for AlN is 1, whereas, for Al2O3, the aluminum oxygen ratio is 1.5. Finally, in Al23O27N5, 27 oxygen and 5 nitrogen atoms can be bonded for every 23 aluminum atoms, which confirms that the stoichiometric relationships observed in AlN and Al2O3 are maintained, that is, that aluminum can carry either 1 nitrogen or 1.5 oxygens.
These stoichiometric relations will help us construct stoichiometric lines originating from Si
4Al
2O
2N
6. It is important to note that we have extended beyond a one-dimensional stoichiometric deviation as in
Figure 3 for the Si
2N
2O compound; now, the deviations extend across a plane. Six trajectories, where the proportions are conserved, are summarized in
Table 4, along with the ranges in which they are valid.
3. Identification of Molar Proportions and Formulation of Deviation Equations.
The previous analysis has allowed us to elucidate that stoichiometric proportions can be formulated from either silicon or aluminum, depending on which destination we are heading toward. For instance, when the deviation is towards aluminum oxynitrides, the weight proportion of silicon wt%Si→0. Conversely, if the compound leans toward silicon oxynitrides, the weight proportion of aluminum (wt%Al) is reduced to zero. The latter leads to the formation of indeterminations.
To handle this issue, we adopted a convenient perspective that considers aluminum and silicon not as individual elements but as a combination of both, that is, the sum of the number of silicon atoms plus aluminum atoms. This approach helps us eliminate the indeterminacies that would occur depending on which compound we are heading. Therefore, in
Table 5, we present the molar proportions of oxygen and nitrogen with respect to the sum of aluminum + silicon in Si
4Al
2O
2N
6 (our reference compound).
These proportions help us quantify the deviation from the initial stoichiometry. The magnitude of the deviation is defined as the absolute value of the difference in the proportions of nitrogen or oxygen with respect to the sum of aluminum and silicon. The nitrogen and oxygen deviation equations are presented as follows in Equations (4) and (5).
where:
represents the deviation of nitrogen relative to aluminum and silicon from the stoichiometry of Si4Al2O2N6 to the concentration under analysis.
represents the deviation of oxygen relative to aluminum and silicon from the stoichiometry of Si4Al2O2N6.
is the current molar proportions of nitrogen.
is the current molar proportions of oxygen.
As in the previous analysis, the total deviation index (TDI) is the sum of the deviations and is presented in Equation (6). TDI is the magnitude of the total deviation of the composite with respect to Si
4Al
2O
2N
6:
4. EDX Analysis
In
Section 3.3, samples grown by RF sputtering were assessed to evaluate the effectiveness of the proposed methodology. The results obtained with this method showed good correspondence with those from the compositional feature characteristics characterization (FTIR). For SiAlON in this conceptual test phase, we are unable to perform multiple depositions; instead, we analyze the entire universe of compounds formed by Si, Al, O and N using the discretized values of the weight proportions of SiAlON elements that are calculated in
Section 3.5.
Subsequently, we define the fundamental molar proportions, those corresponding to the stoichiometric formulas of each ideally stoichiometric compound. Using the stoichiometric formulas in
Table 4, we determine the weight percentages. These calculations allow us to precisely know the amount of each element present in each sample. The detailed results are presented in
Table 6.
As shown in the data in
Table 6, the silicon oxynitride compounds exhibit a complete absence of aluminum. Similarly, the compounds AlN, Al
23O
27N
5, and Al
2O
3 contain no silicon, reinforcing the validity of the approach that groups aluminum and silicon as a “single element” to reduce indeterminacies in our estimations. This methodology allows us to reduce the uncertainties inherent to the composition of complex materials.
5. Evaluation of Molar Proportions and Calculation of TDI.
Unlike SiON, we are now in a plane rather than a single dimension. Therefore, we must calculate different TDIs, one for each ideal stoichiometric compound. To obtain the magnitude of the SDV, the TDI is calculated using formulas 4 to 6. The results are shown in
Table 7 and
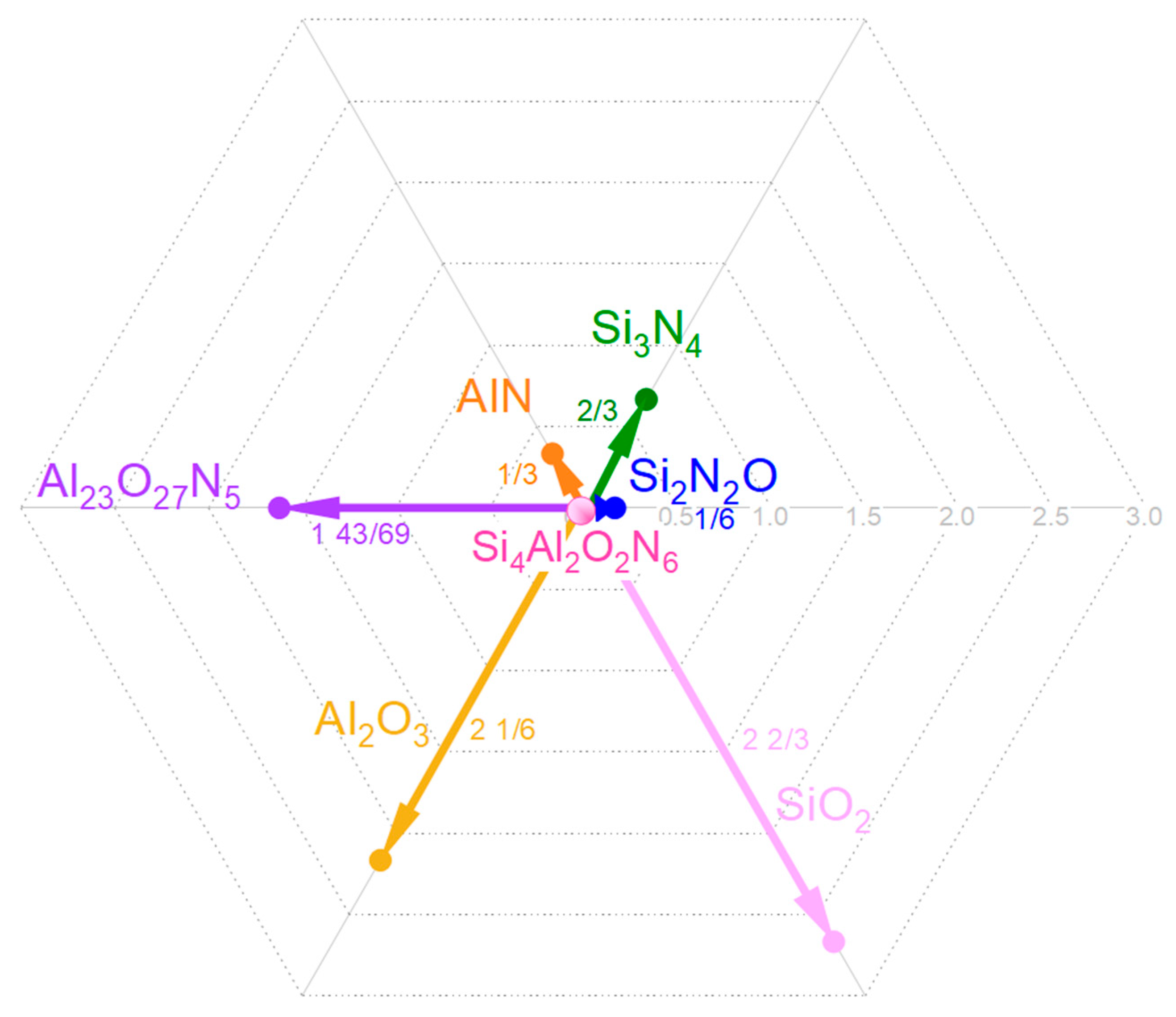
Figure 7.
Figure 7 provides a vector diagram that helps us understand how the compositions of the six compounds in the quaternary system differ from our reference composition. The vectors show the adjustments necessary to move from the reference to any of the six compounds, with the compounds containing a high weight concentration of oxygen (SiO
2, Al
23O
27N
5, and Al
2O
3) corresponding to the longest vectors, hence being further away from SiAlON’s physical and compositional feature characteristics.
6. Determination of the direction of the SDV.
To determine the direction of deviation from the reference compound to one of the six extremes, we first identify the molar proportions of all involved compounds, as summarized in
Table 8. Using these molar proportions, we can identify six compounds towards which SiAlON can deviate: SiO
2, Si
2N
2O, Si
3N
4, AlN, Al
23O
27N
5, and Al
2O
3. By formulating the inequalities based on these molar proportions, we can predict and validate the direction of deviation for each compound tested. Let us take Si
4Al
2O
2N
6 to SiO
2 as an example; to be considered an SDV, we must meet the entire set of deviation conditions.
: This condition suggests that the material favors a more silicon-rich composition, consistent with a shift toward SiO2, a silicon–oxygen compound without aluminum or nitrogen.
< 3/2: This reflects that the nitrogen content decreases relative to silicon as we move towards a stoichiometry that favors SiO2. This condition ensures that nitrogen is present in a lower relative quantity, approaching SiO2 where N = 0.
: This limitation refines the ratio of silicon to oxygen, ensuring that we are moving in that direction by reducing the silicon content but without exceeding the concentration in the SiO2 (where it acquires the value of one-half).
: This restriction does not favor a stoichiometry rich in aluminum with respect to oxygen, which is consistent with a deviation towards SiO2, where aluminum does not play a predominant role.
: Because SiO2 does not contain nitrogen, the relative presence of nitrogen relative to oxygen must also decrease, reflecting a reduction in nitrogen’s contribution to the composition.
These five constraints form the set of deviation conditions towards SiO
2. The six sets of deviation conditions from Si
4Al
2O
2N
6 to the ideal stoichiometric compounds are presented in
Table 9.
The evaluation process classifies compounds that do not meet all of the requirements for a given set of conditions as “No stoichiometric similar”. However, compounds that satisfy any criteria set’s conditions are classified as “like” the compound towards which they are directed to. As we saw in the previous section, this process can predict compound properties with limited prior information.
After establishing and justifying the deviation conditions and formulas to measure TDI, programs were developed in Mathematica, Excel, and OriginLab software. These programs are not necessary to use our methodology. However, they enable the calculation of compliance percentages with the six conditions and the identification of the location of the stoichiometric SiAlON compounds in the universe of Si, Al, O, and N compositions, and they provide visual tools to facilitate the understanding of the utility of our tool. The results are detailed in the next section.
3.5. SiAlON Graphical Representation
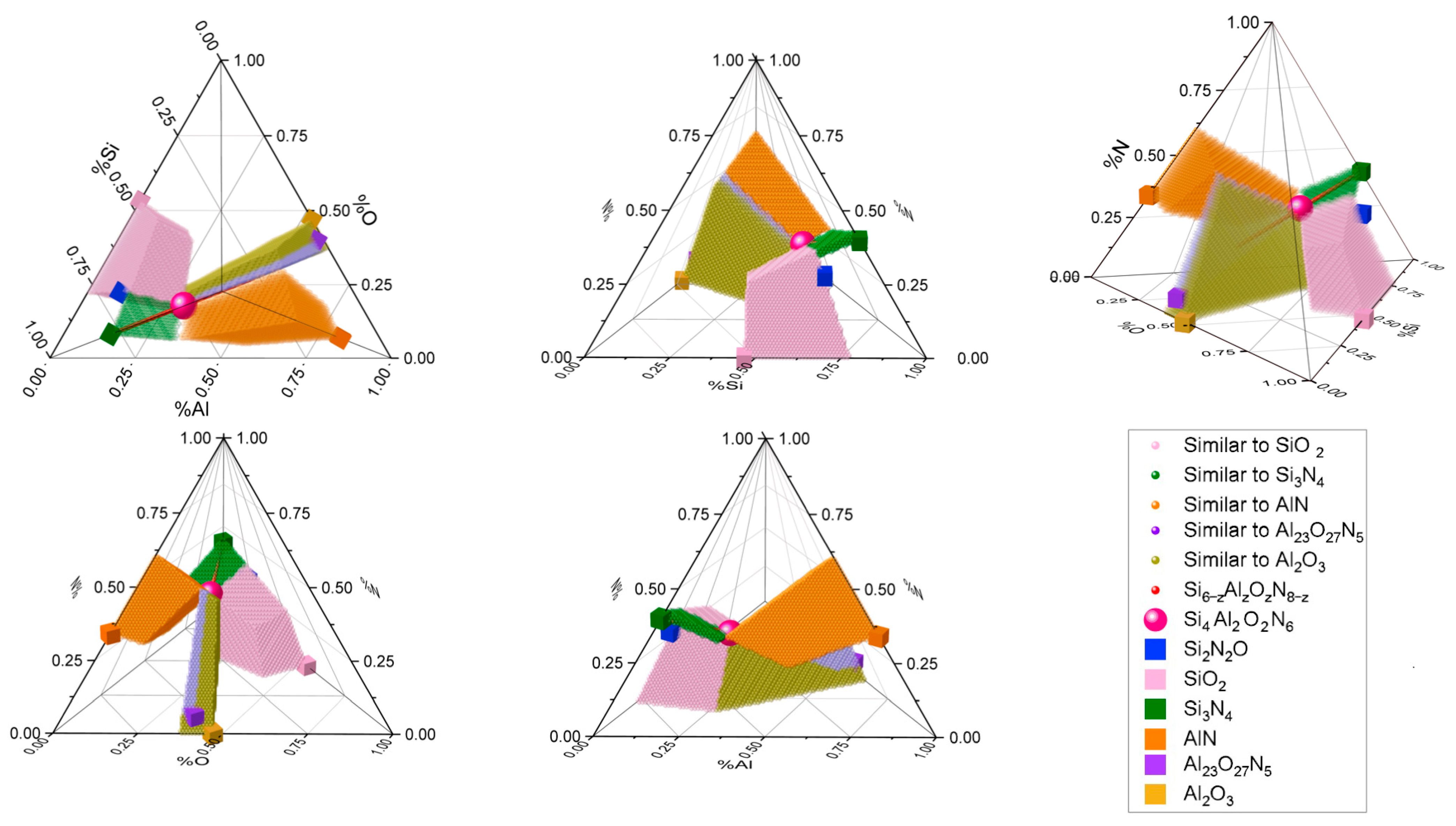
In order to gain a better understanding of the percentage of compounds that meet the similarity conditions with stoichiometric compounds, we created quaternary diagrams. These diagrams are in the shape of a tetrahedron or a pyramid with four faces, with each vertex corresponding to one of the four components—Si, Al, O, and N. Any point within the tetrahedron displays the molecular weight distribution of the constituent elements. To generate these images, we used a method that discretizes the space of possible combinations of the distribution of molecular weights of the SiAlON elements.
Methodology
Initially, we ensured that the sum of the molecular weight percentages of these elements reached 100% in each possible combination. Using Mathematica, we organized the data into an array for each combination of percentages, arranged in four columns representing each element, N, O, Al, and Si, respectively.
The described script uses a “for” loop that iterates from 0 to 101. In each iteration, a row of four columns is formed. The first column contains each integer from 0 to n − 1 repeated n − i times; the second column contains a sequence from 0 to n − 1 − i for each integer i; the third column inverts this sequence; and the fourth column calculates the complement up to 100 for the sum of the first three columns. Subsequently, all rows are combined into a table. The total number of rows (combinations) generated was 176,851, varying from [100 0 0 0] (100% nitrogen) to [0 0 0 100] (pure silicon).
Next, we evaluate each combination using the formulas and sets of criteria discussed in steps 3 and 6 of the previous section. This comprehensive analysis allows us to explore the universe of discrete weight percentages within the SiAlON system, providing a detailed understanding of its potential molecular configurations.
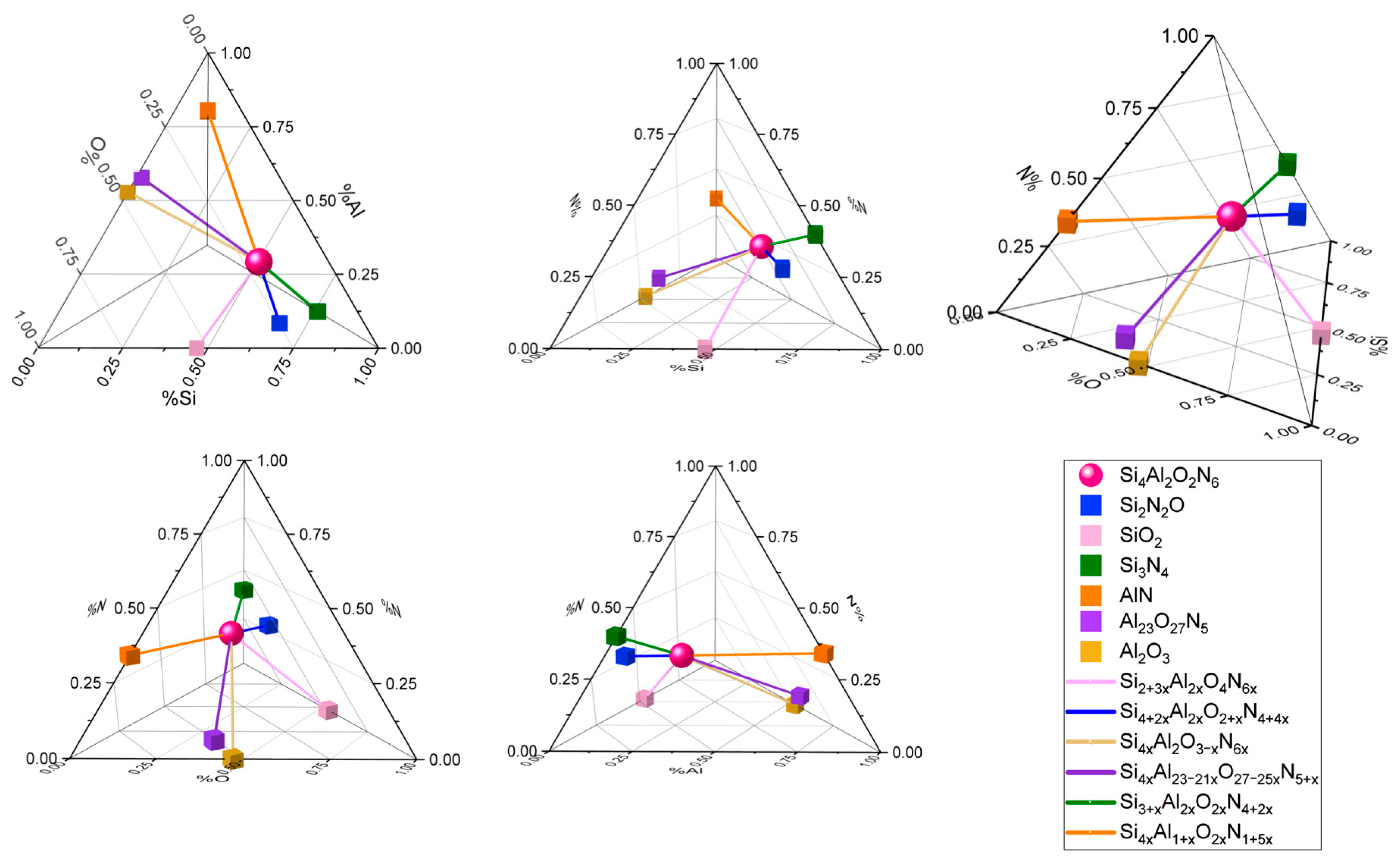
Figure 8 shows a detailed representation of the relative position of the six stoichiometric components that have been analyzed, along with the stoichiometric lines that connect them to the reference compound (Si
4Al
2O
2N
6). The stoichiometric lines correspond to the formulas indicated in
Table 4. The diagram has been presented from multiple perspectives to help visualize the position of the ideal stoichiometric compounds and the length of the stoichiometric lines. By analyzing
Figure 8, it is possible to conclude that the silicon oxynitrides are much closer to Si
4Al
2O
2N
6, which means they will exhibit properties more similar to SiAlON than the aluminum oxynitrides.
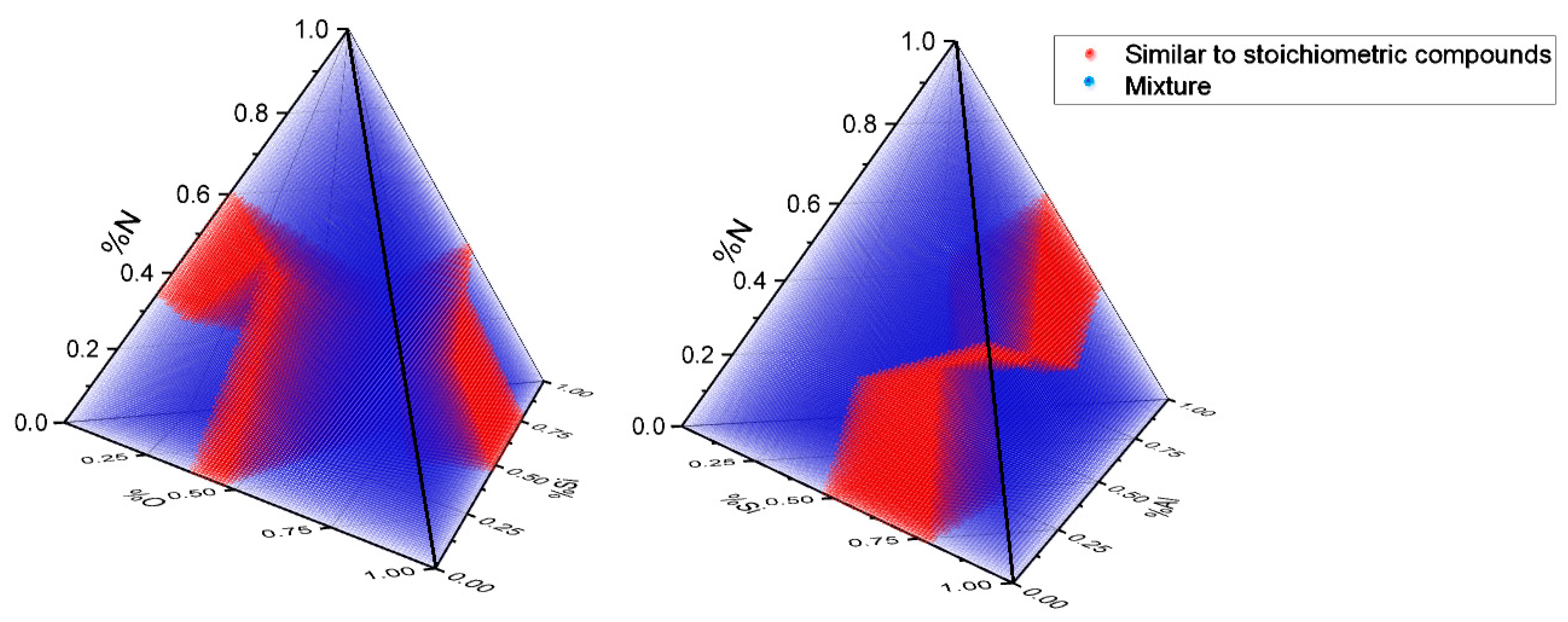
In order to provide a clear understanding of the proportion of compounds with a defined stoichiometric deviation vector, i.e., stoichiometry-like compounds (Si2N2O, SiO2, Si3N4, AlN, Al23O27N5, and Al2O3) and those lacking an SDV, evaluations were carried out based on the criteria outlined in step 6 for determining the direction of the SDV.
The calculations indicate that 35,616 combinations, equivalent to 20.14%, fulfilled at least one of the conditions for stoichiometric similarity. The remaining 141,235 combinations, representing 79.86%, did not exhibit a defined SDV and were therefore classified as non-stoichiometric-like. These findings are detailed in
Figure 9. The number of possible compounds directed toward each ideal stoichiometric compound is summarized in
Table 10.
From
Table 10, the absence of combinations like Si
2N
2O stands out, attributed to the strict deviation criteria applied (mainly
), making it practically impossible to meet. Despite generating a percentage of combinations close to the real one, it should not be overlooked that this analysis is discrete, and an infinite number of potential combinations has not been considered. Those components that were adjusted to a set of conditions are shown in
Figure 10.
The graph includes the ideal stoichiometric components to illustrate how the region of like compounds extends from the reference compound to them. The large volumes corresponding to compounds similar to SiO2, AlN, and Al2O3 are evident.
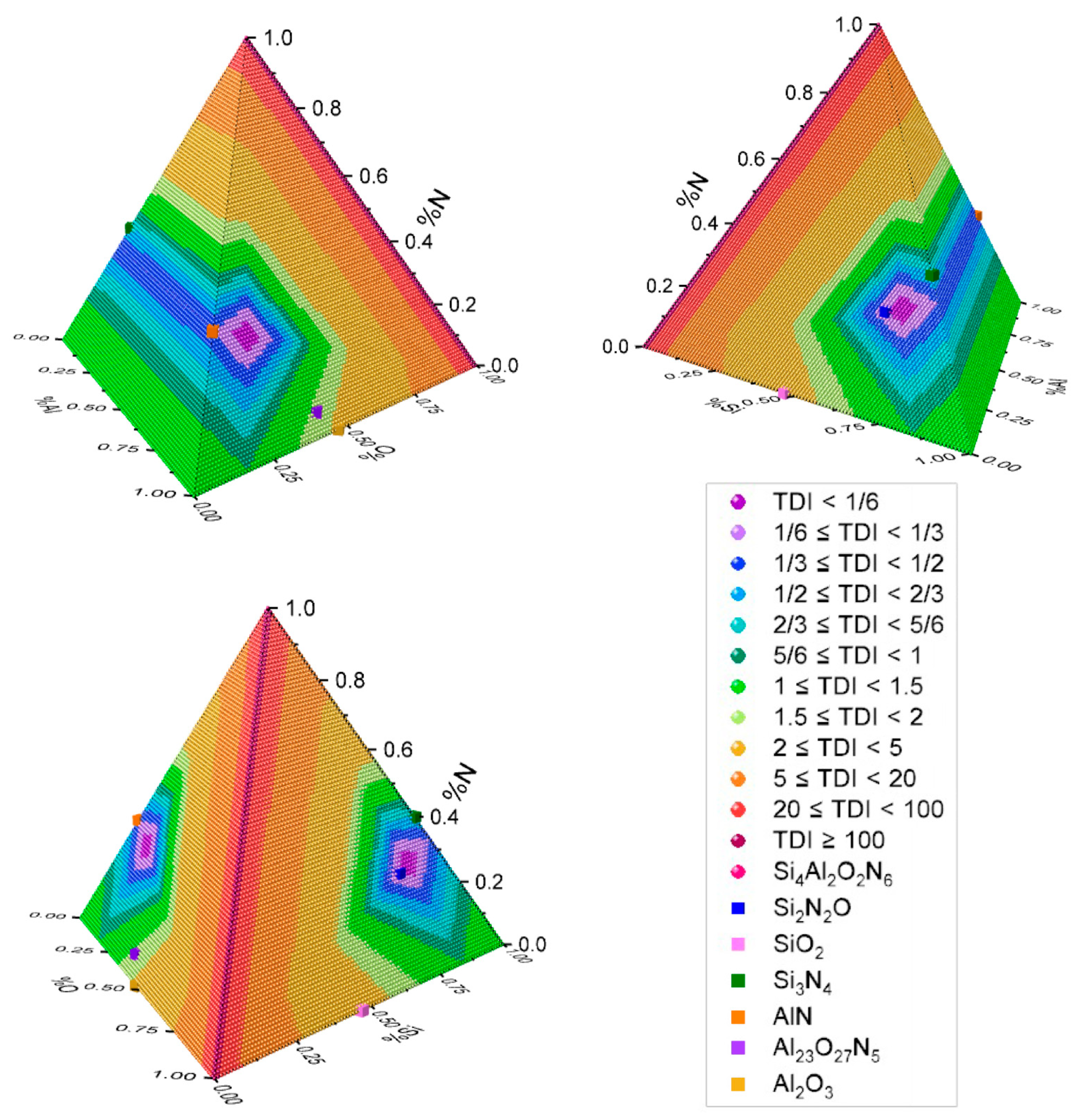
Lastly,
Figure 11 presents a diagram with TDI values on a color scale, analogous to
Figure 4 but adapted for four-element compounds. An increase in TDIs is observed as they diverge from the reference compound, consistent with the definition provided in the “Foundational Elements of the Analysis Methodology” (
Section 3.2), where the TDI evaluates how much a component deviates from the reference stoichiometry.
The TDI scale values are consistent with those in
Table 7, highlighting Si
2N
2O as the closest compound with a TDI of one-sixth and SiO
2 as the farthest with a TDI ≈ 2.667. These TDIs, combined with stoichiometric lines, illustrate the proximity between the reference compound and other compounds, which can be interpreted in terms of their physical and compositional feature characteristics. Additionally, the visual representation aids in identifying the percentage of stoichiometric compounds that meet the set of criteria for each ideal compound, thereby providing insights into their characteristics. Finally, a three-dimensional view emphasizes proportionality and placement within the universe of possible combinations.
The visualization of the behavior of SiAlON compounds under the studied conditions offers a comprehensible way to confirm the validity of our approach. It provides a practical tool for researchers in the field of materials. This multidimensional approach is a valuable tool for gaining insights into the physical and compositional feature characteristics of the compounds based on a standard EDX elemental analysis.