Investigation of Variability of Flaw Strength Distributions on Brittle SiC Ceramic
Abstract
1. Introduction
- The phenomenological and macroscopic approaches which consider specimen strengths, like the Weibull model;
- The approaches that consider the flaws as physical entities [2,19,20,21,22,23,24,25]. They are more fundamental. They are based on the flaw strength density function. The severity of a flaw is measured either using the flaw strength or flaw size. The flaw strength is defined as the critical value of the local stress operating on a volume element containing a single flaw (also referred to as elemental stress) that causes the extension of the flaw. The failure probability of a test piece is derived from the flaw strength density function using the following equation [21,22,23,24,25]:
2. Materials and Methods
2.1. Generation of Flaw Strength Data
2.2. Analysis of Flaw Strength Data
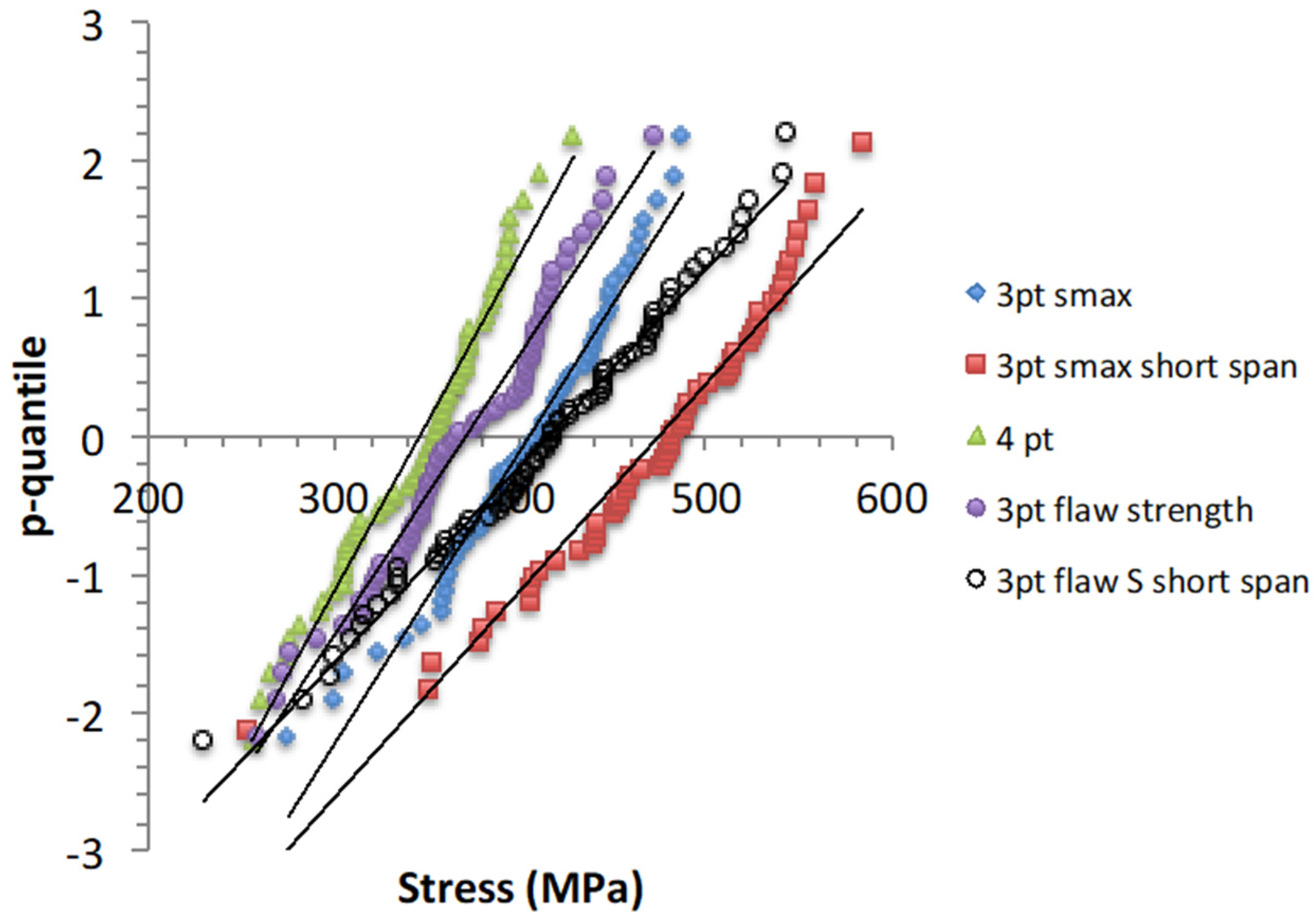
2.2.1. p-Quantile Diagrams and Normal Distribution [16]
2.2.2. Analysis Using the Normal Distribution
2.2.3. Analysis Using the Elemental Strength Model
2.2.4. Analysis Using the Weibull Model
- (a)
- Maximum Likelihood Estimation (MLE)
- (b)
- Method of moments (MM)
3. Results
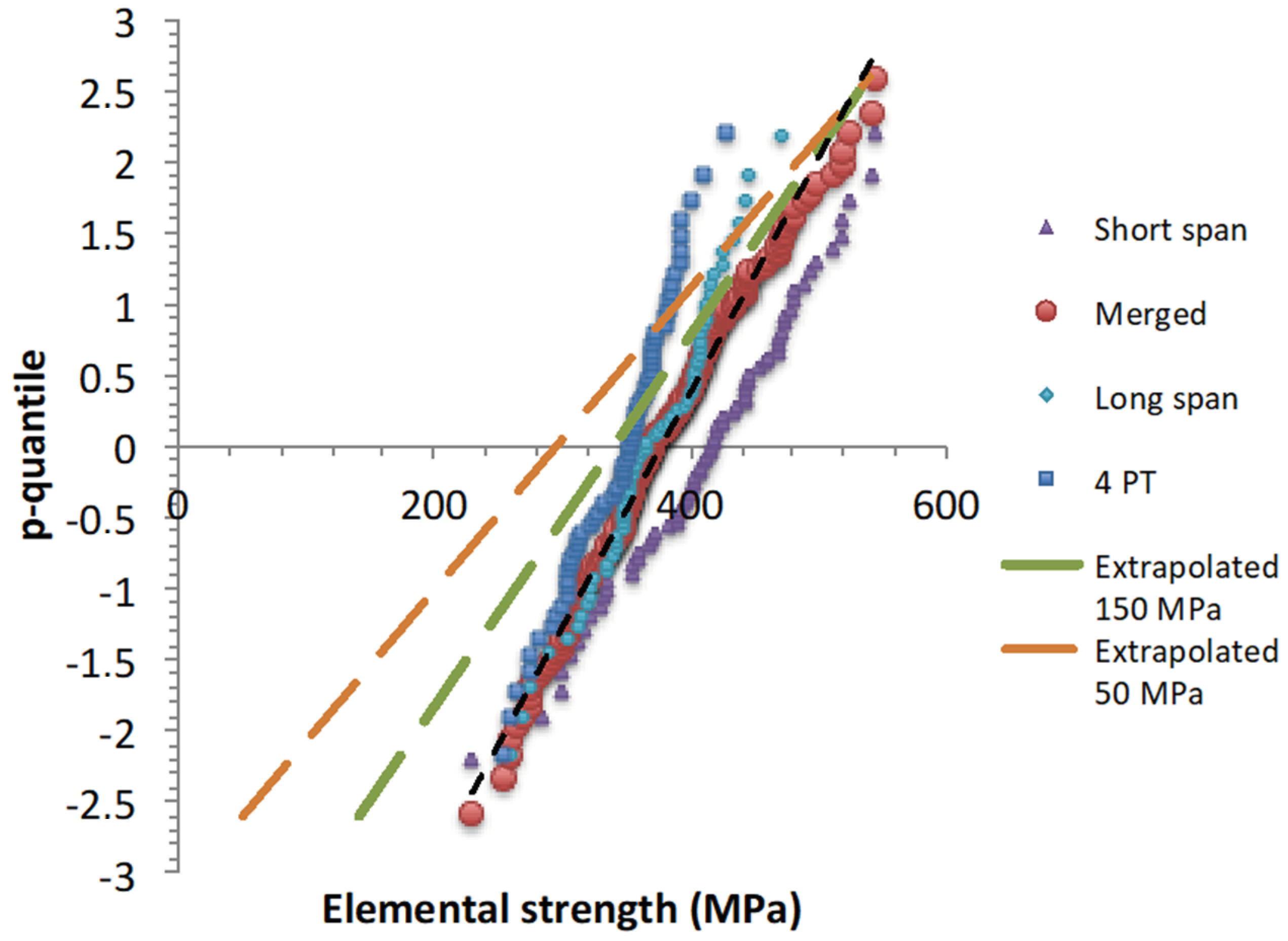
3.1. The p-Quantile Diagrams
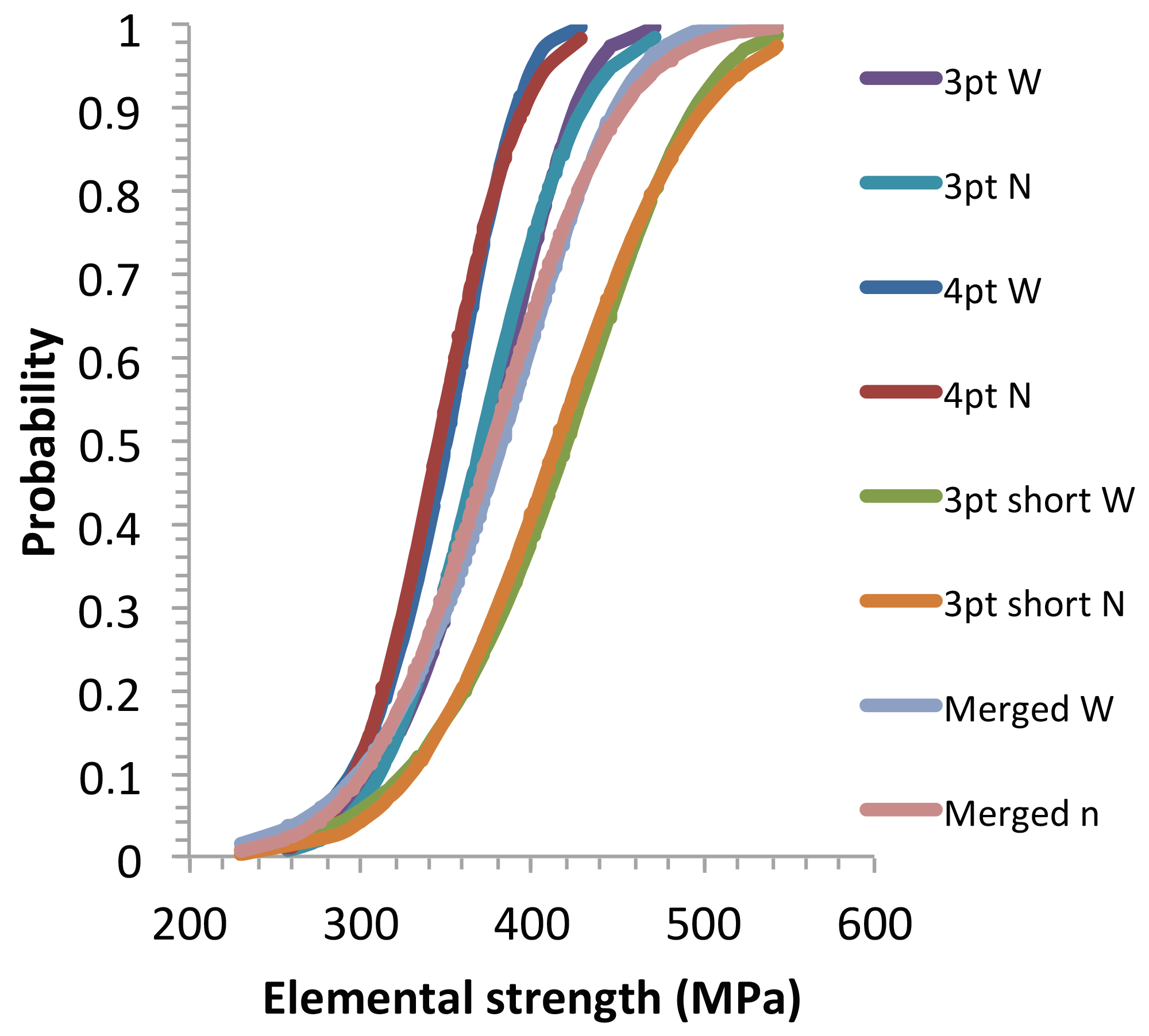
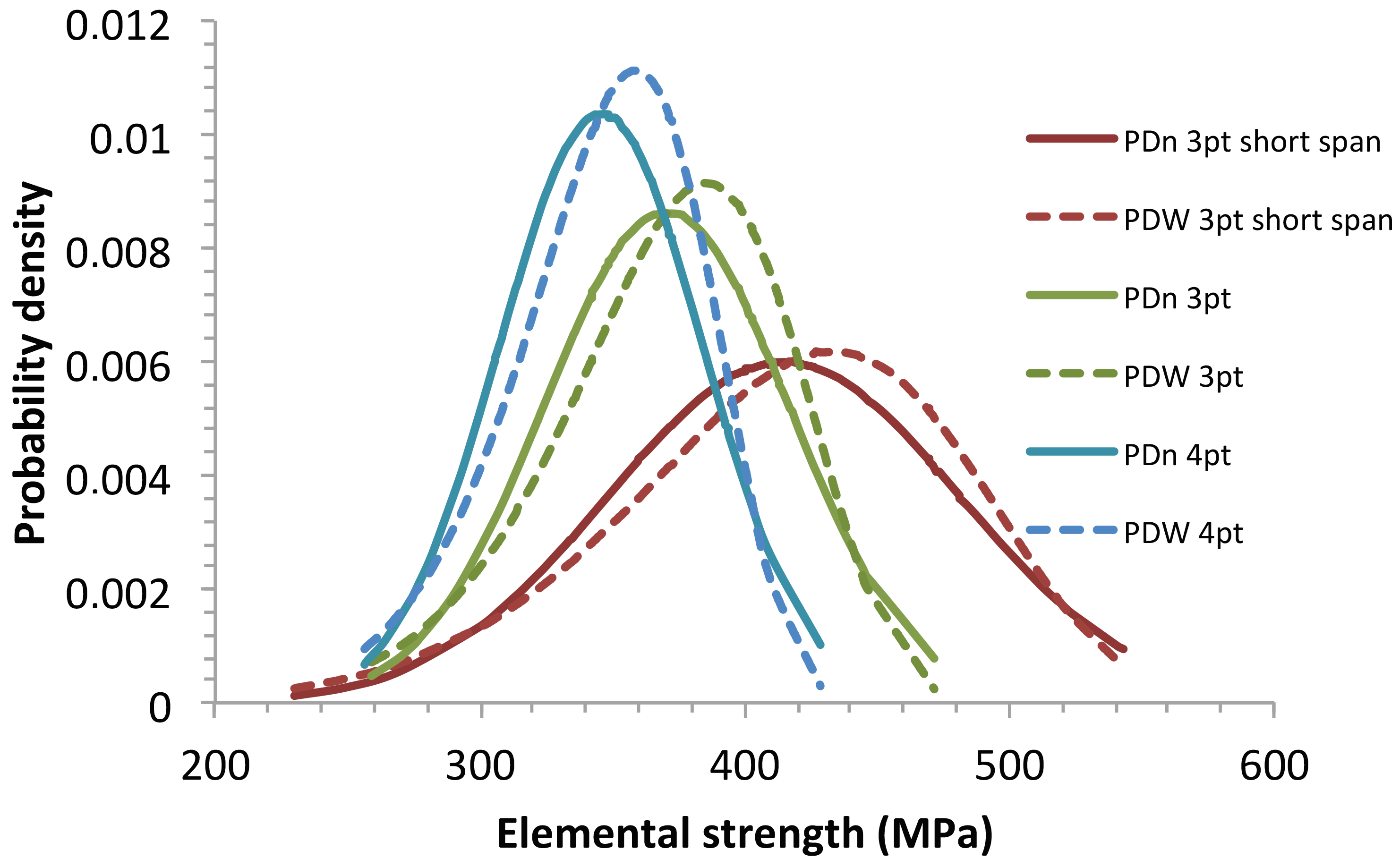
3.2. Distributions of Flaw Strengths
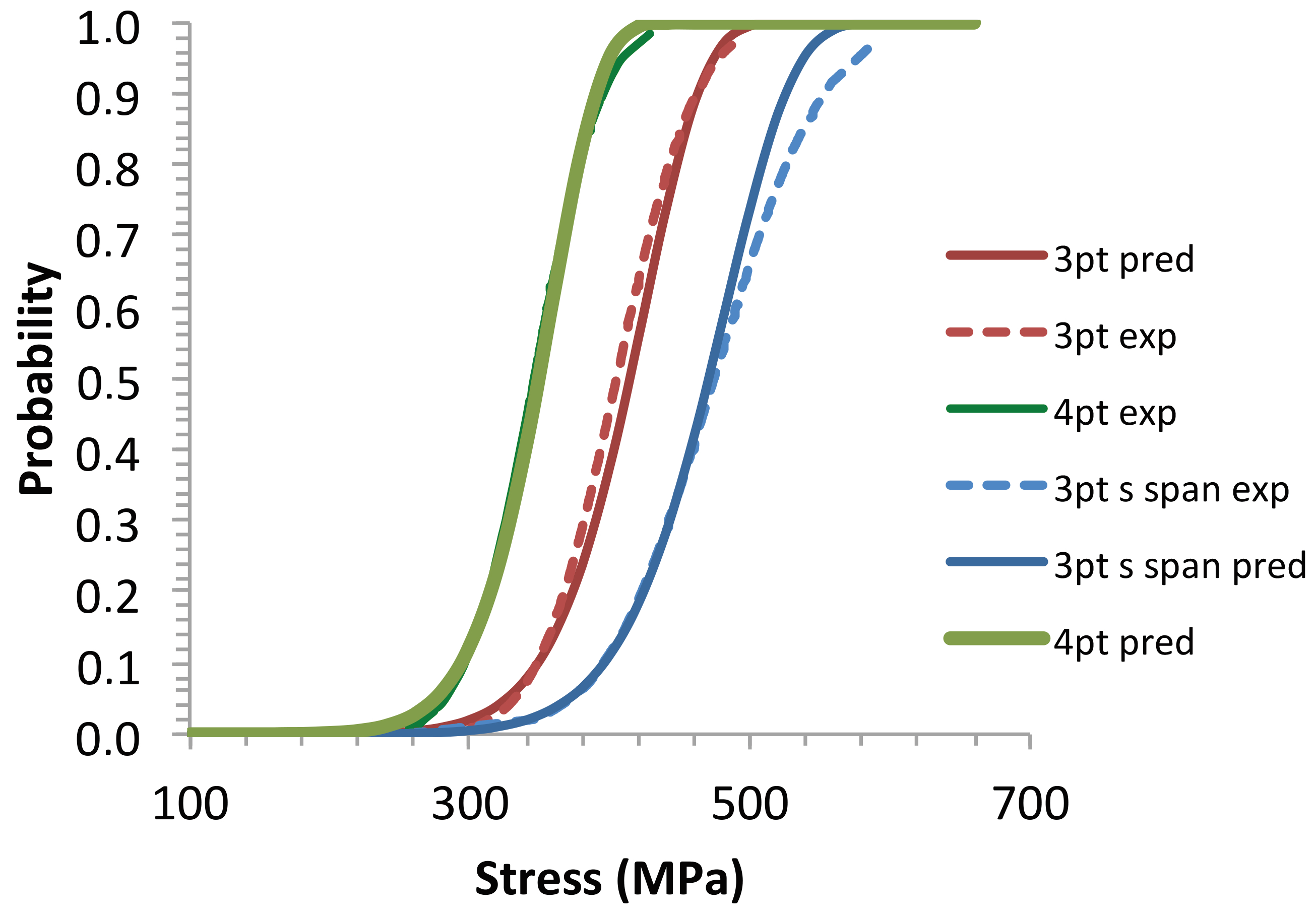
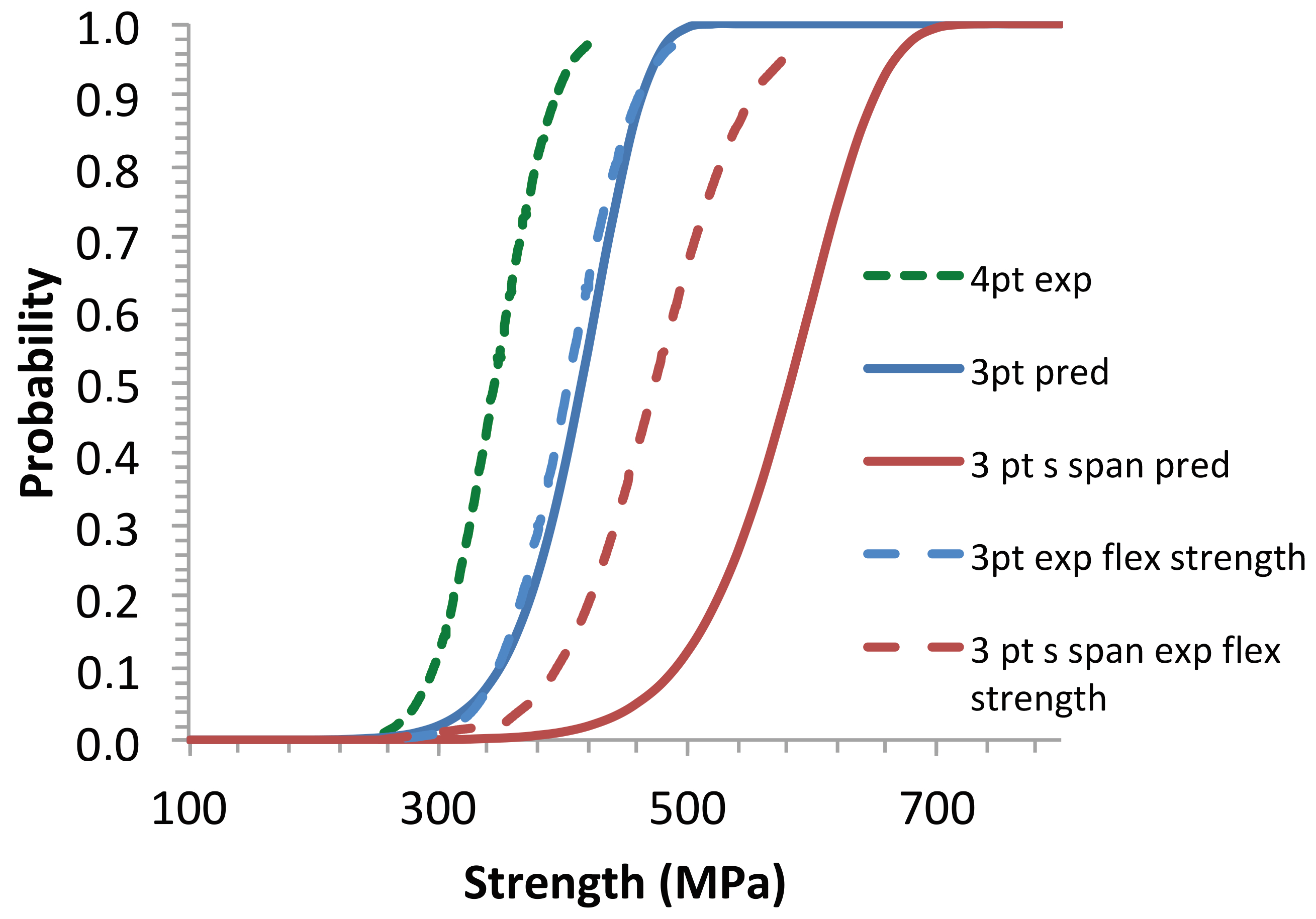
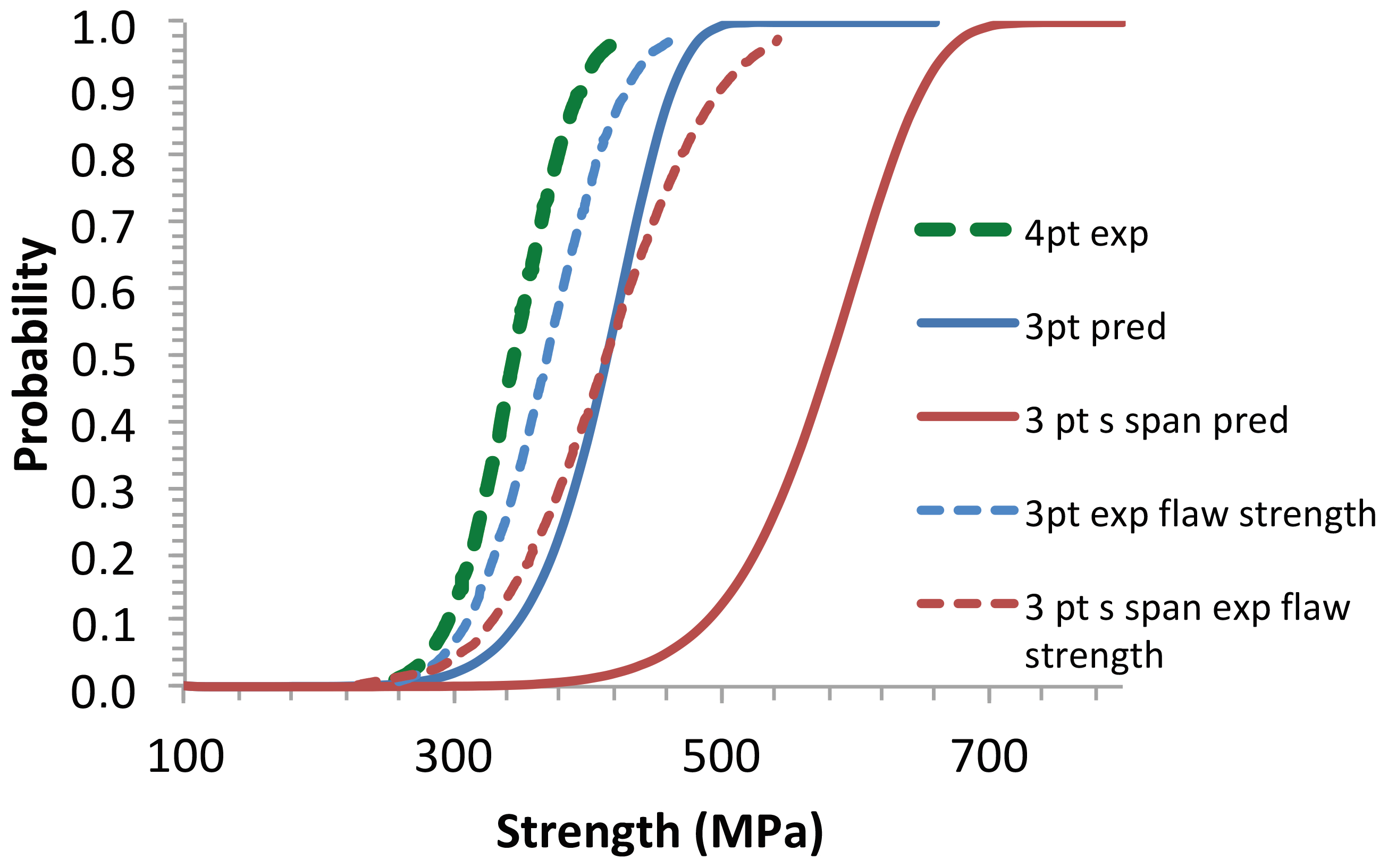
3.3. Predictions of CDFs
4. Discussion: Implications
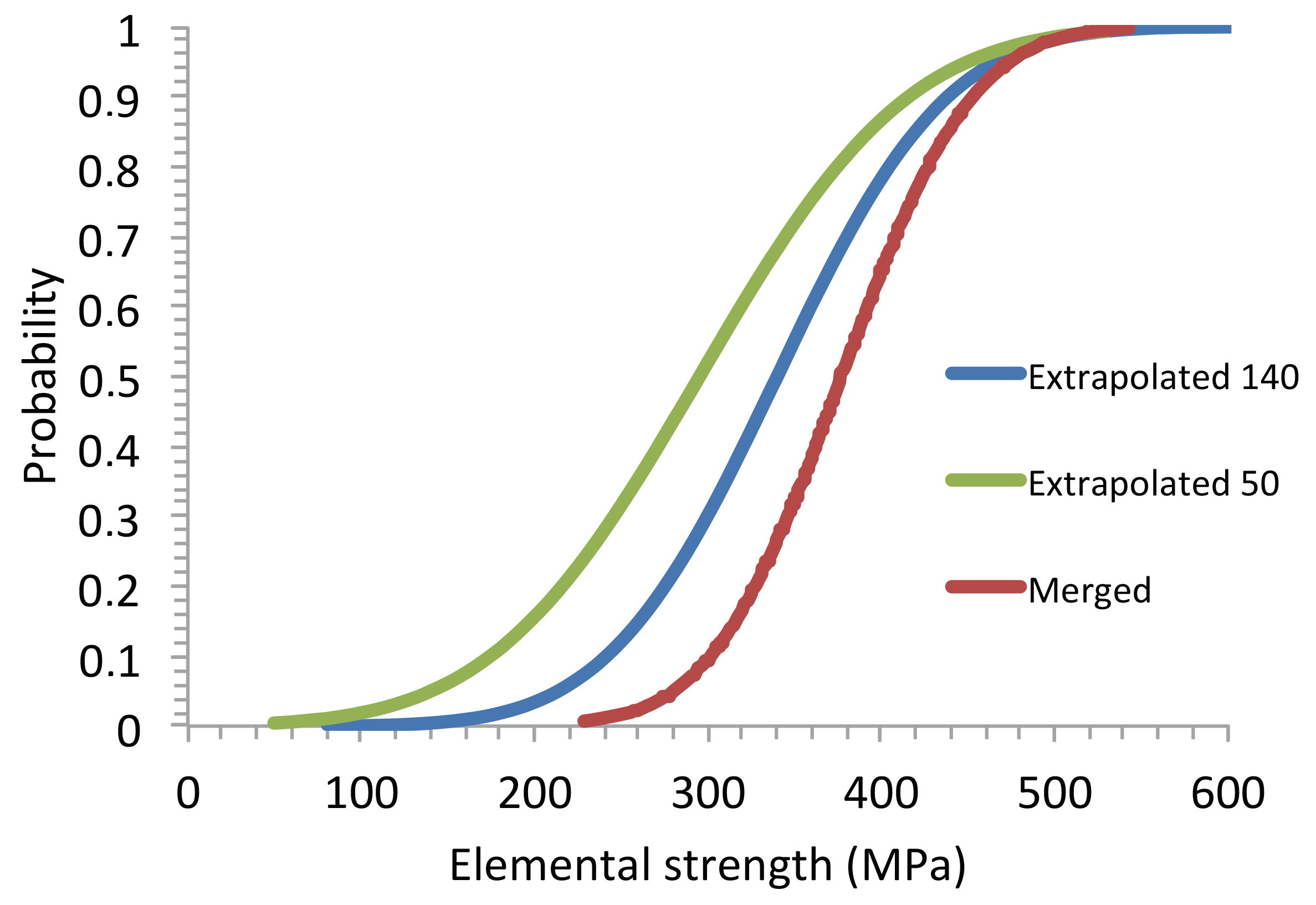
4.1. Merged Flaw Strength Distribution
4.2. Extrapolated Flaw Strength Distribution
4.3. Influence of n and Size
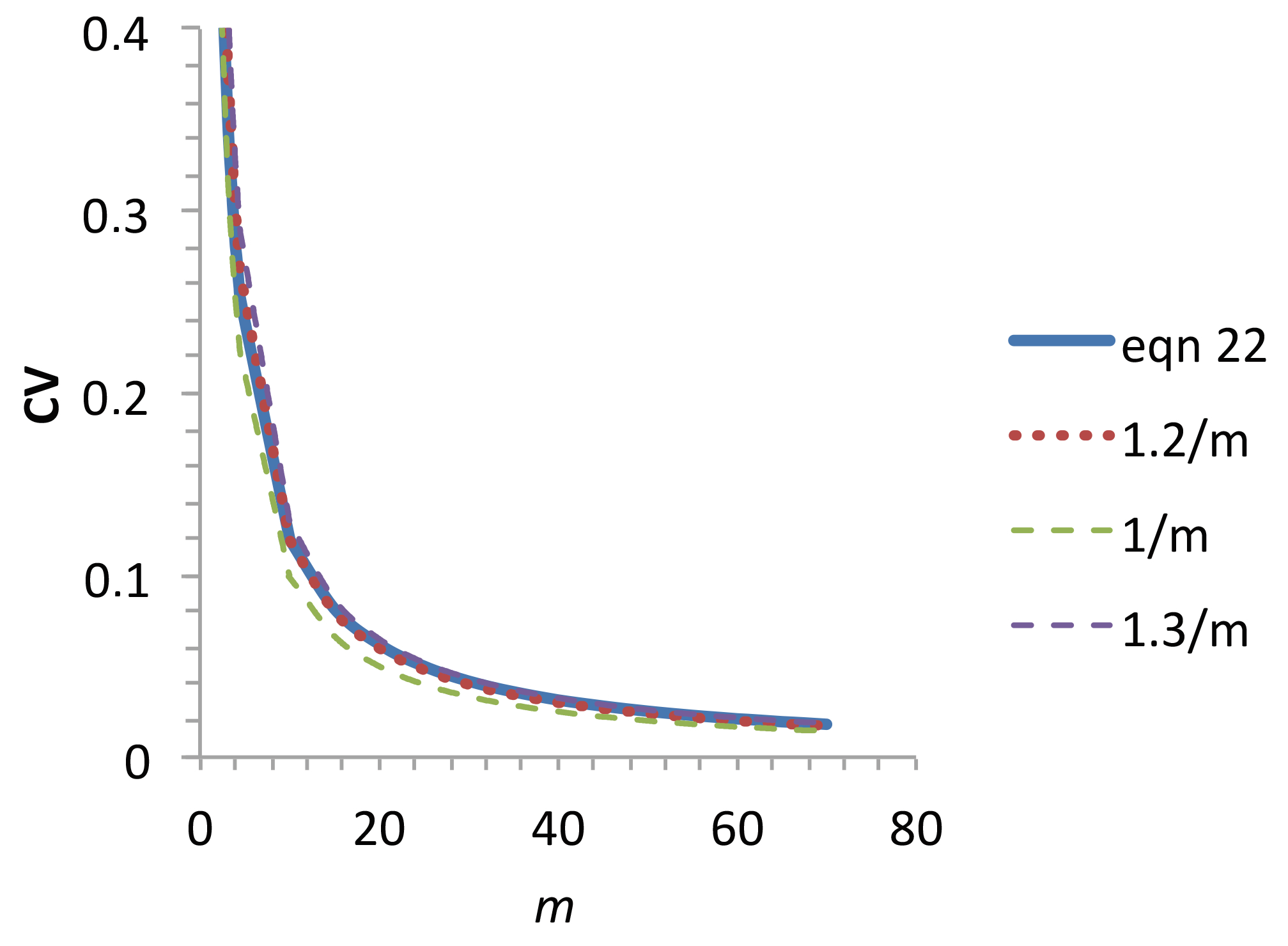
4.3.1. Influence of n on p-Quantile Maximum Values
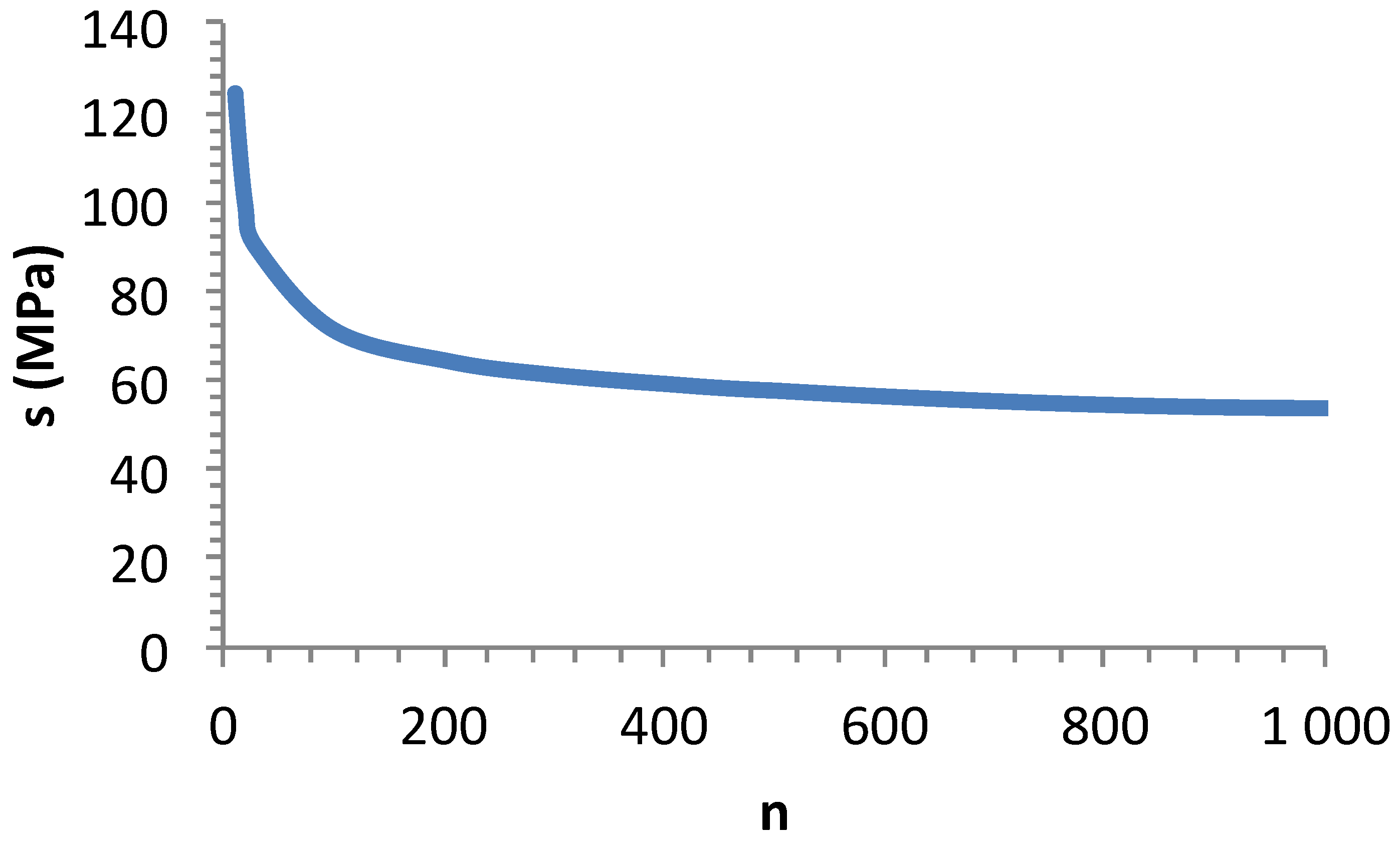
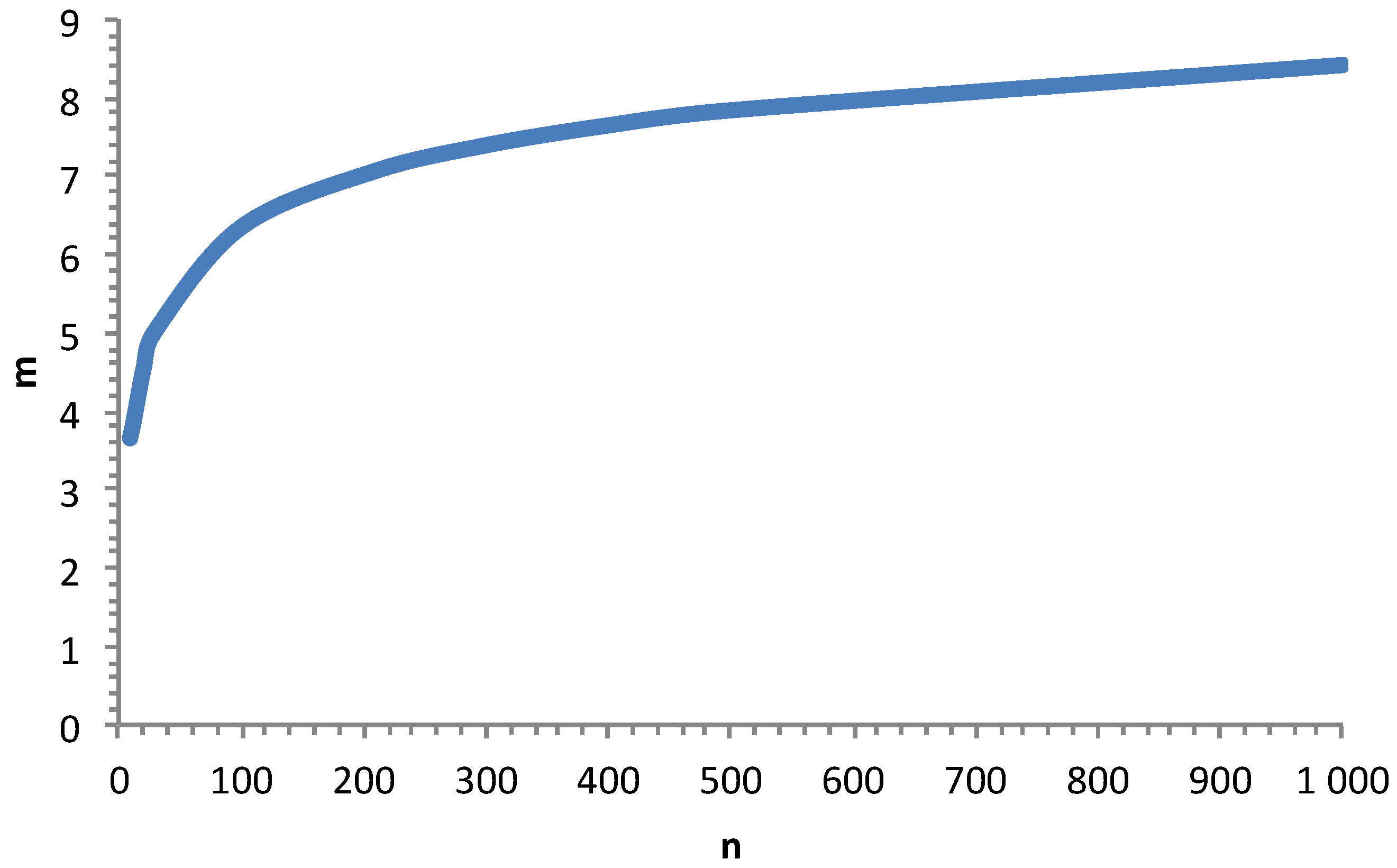
4.3.2. Influence of n and Volume on Statistical Parameters
4.4. Validity of the Uniaxial Analysis for a Short Span
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Weibull, W. A statistical distribution function of wide applicability. J. Appl. Mech. 1951, 18, 293–297. [Google Scholar] [CrossRef]
- Lamon, J. Brittle Fracture and Damage of Brittle Materials and Composites: Statistical-Probabilistic Approaches; ISTE Press–Elsevier: London, UK, 2017; ISBN 9781785481215. [Google Scholar]
- Gong, J. A new probability index for estimating Weibull modulus for ceramics with least square method. J. Mater. Sci. Lett. 2000, 19, 827–829. [Google Scholar] [CrossRef]
- Barnett, V. Probability plotting methods and order statistics. J. R. Stat. Soc. 1975, C24, 95–108. [Google Scholar] [CrossRef]
- Watson, A.S.; Smith, R.L. An examination of statistical theories for fibrous materials in the light of experimental data. J. Mater. Sci. 1985, 20, 3260–3270. [Google Scholar] [CrossRef]
- Paramonov, Y.; Andersons, J. A family of weakest link models for fiber strength distribution. Compos. Part A 2007, 38, 1227–1233. [Google Scholar] [CrossRef]
- Phani, K.K. A new modified Weibull distribution function for the evaluation of the strength of silicon carbide and alumina fibres. J. Mater. Sci. 1988, 23, 2424–2428. [Google Scholar] [CrossRef]
- Amaniampong, G.; Burgoyne, C.J. Statistical variability in the strength and failure strain of aramid and polyester yarns. J. Mater. Sci. 1994, 29, 5141–5152. [Google Scholar] [CrossRef]
- Bergman, B. On the estimation of the Weibull modulus. J. Mater. Sci. Lett. 1984, 3, 689–692. [Google Scholar] [CrossRef]
- Sullivan, J.D.; Lauzon, P.H. Experimental probability estimators for Weibull plots. J. Mater. Sci. Lett. 1986, 5, 1245–1247. [Google Scholar] [CrossRef]
- Basu, B.; Tiwari, D.; Kundu, D.; Prasad, R. Is Weibull Distribution the Most Appropriate Statistical Strength Distribution for Brittle Materials? Ceram. Int. 2009, 35, 237–246. [Google Scholar] [CrossRef]
- Lu, C.; Danzer, R.; Fischer, F.D. Fracture statistics of brittle materials: Weibull or normal distribution. Phys. Rev. E 2002, 65, 06702. [Google Scholar] [CrossRef] [PubMed]
- Peirce, F.T. Tensile Tests for Cotton Yarns—The Weakest Link. J. Text. Inst. 1926, 17, 355–368. [Google Scholar]
- Epstein, B. Application of the theory of extreme values in fracture problems. J. Am. Statist. Assoc. 1948, 43, 403–412. [Google Scholar] [CrossRef]
- R’Mili, M.; Godin, N.; Lamon, J. Flaw strength distributions and statistical parameters for ceramic fibres: The Normal distribution. Phys. Rev. E 2012, 85, 1106–1112. [Google Scholar]
- Lamon, J.; R’Mili, M. Investigation of flaw strength distributions from tensile force-strain curves of fiber tows. Compos. Part A 2021, 145, 106262. [Google Scholar] [CrossRef]
- Lissart, N.; Lamon, J. Statistical analysis of failure of SiC fibers in the presence of bimodal flaw populations. J. Mater. Sci. 1997, 32, 6107–6117. [Google Scholar] [CrossRef]
- Vu, C.-C.; Tran, H.-H. Performance analysis of methods to estimate Weibull parameters for the compressive strength of concrete. Case Stud. Constr. Mater. 2023, 19, e02330. [Google Scholar] [CrossRef]
- Freudenthal, A. Statistical Approach to Brittle Fracture. Chapter 6. In Fracture; Liebowitz, H., Ed.; Academic Press: New York, NY, USA, 1968; Volume II. [Google Scholar]
- Gumbel, E. Statistics of Extremes; Columbia University Press: New York, NY, USA, 1968. [Google Scholar]
- Jayatilaka, A.D.S.; Trustrum, K. Statistical approach to brittle fracture. J. Mater. Sci. 1977, 10, 1426–1430. [Google Scholar] [CrossRef]
- Argon, A.S.; McClintock, F.A. Mechanical Behavior of Materials; Addison-Wesley: Reading, MA, USA, 1966. [Google Scholar]
- Batdorf, S.B.; Crose, J.G. A statistical theory for the fracture of brittle structures subjected to nonuniform polyaxial stresses. J. Appl. Mech. 1974, 41, 459–464. [Google Scholar] [CrossRef]
- Lamon, J. Ceramics reliability: Statistical analysis of multiaxial failure using the Weibull approach and the Multiaxial Elemental Strengh model. J. Am. Ceram. Soc. 1990, 73, 2204–2212. [Google Scholar] [CrossRef]
- Lamon, J.; Evans, A.G. The statistical analysis of bending strengths for brittle solids: A multiaxial fracture problem. J. Am. Ceram. Soc. 1983, 66, 177–182. [Google Scholar] [CrossRef]
- Bhattacharya, P.; Bhattacharjee, R. A study on Weibull distribution for estimating the parameters. J. Appl. Quant. Methods 2010, 5, 234–241. [Google Scholar] [CrossRef]
- Bin Deng, B.; Wang, X.; Jiang, D.; Gong, J. Description of the statistical variations of the measured strength for brittle ceramics: A comparison between two-parameter Weibull distribution and normal distribution. Process. Appl. Ceram. 2020, 14, 293–302. [Google Scholar] [CrossRef]
- Lamon, J.; R’Mili, M. Investigation of Specimen Size Effects on P-Quantile Diagrams and Normal Distributions of Critical Flaw Strengths in Fiber Tows. J. Compos. Sci. 2022, 6, 171. [Google Scholar] [CrossRef]
- Lamon, J. Statistical analysis of fracture of Silicon Nitride using the short span bending technique. Int. J. Turbo Jet Engines 1988, 5, 13–22. [Google Scholar] [CrossRef]



| Length (mm) | Thickness 2d (mm) | Width b (mm) | Upper Span 2l1 (mm) | Lower Span 2l (mm) | |
|---|---|---|---|---|---|
| 3pt long span | 45 | 4 | 4 | 0 | 40 |
| 3pt short span | 20 | 4 | 4 | 0 | 10 |
| 4pt | 45 | 4 | 4 | 20 | 40 |
| p-Quantile vs. Strength | Pn vs. PW | PDW vs. PDn | |
|---|---|---|---|
| 4pt | 0.985 | 0.99 | 0.93 |
| 3pt flex strength | 0.988 | 0.997 | |
| 3pt flex strength short span | 0.97 | 0.997 | |
| 3pt flaw strength | 0.988 | 0.997 | 0.90 |
| 3pt flaw strength short span | 0.99 | 0.998 | 0.95 |
| Merged flaw strength | 0.995 | 0.998 |
| μ (MPa) | s (MPa) | m Weibull | λ | σs(1) (MPa) Weibull | Scale Factor (MPa) Weibull | S0 (MPa) Elemental Model | VE (10−9 m3) | |
|---|---|---|---|---|---|---|---|---|
| 4pt flaw strength | 345.67 | 38.46 | 10.9 (MM) 10.8 (MLE) | 10.9 | 362.06 (MM) 362.44 (MLE) | 64.9 | 57 | 7.29 |
| 3pt flexural strength | 404.67 | 44.26 | 11.1 (MM) 10.97 (MLE) | 423.73 (MM) 424,20 (MLE) | 66.03 | 1.09 | ||
| 3pt flexural strength short span | 474.27 | 61.26 | 9.3 (MM) 9.9 (MLE) | 499.23 (MM) 499.0 (MLE) | 48.42 | 0.38 | ||
| 3pt flaw strength | 370.3 | 46.2 | 9.63 (MM) 9.35 (MLE) | 389.82 (MM) 390.1 (MLE) | 46.99 | 1.42 | ||
| 3pt flaw strength short span | 414.8 | 66.55 | 7.36 (MM) 7.29 (MLE) | 442.22 (MM) 442.2 (MLE) | 24.54 | 0.57 | ||
| Merged flaw strength | 377.15 | 59.12 | 7.56 (MM) 6.64 (MLE) | 401.65 (MM) 402.93 (MLE) | 33 | |||
| extrapolated flaw strength | 340 | 76.92 | 5.08 | 369.97 | ||||
| extrapolated flaw strength | 295.28 | 94.34 | 3.5 | 328.09 |
| Smin (MPa) | Smax (MPa) | zp | n | |
|---|---|---|---|---|
| 4pt flaw strength | 256 | 429 | +/− 2.2 | 69 |
| 3pt flexural strength | +/− 2.2 | 68 | ||
| 3pt flexural strength short span | +/− 2.13 | 70 | ||
| 3pt flaw strength | 259 | 472 | +/− 2.18 | 68 |
| 3pt flaw strength short span | 230 | 544 | +/− 2.2 | 70 |
| Merged flaw strength | 230 | 544 | +/− 2.59 | 207 |
| extrapolated flaw strength | 140 | 544 | +/− 2.6 | 210 |
| extrapolated flaw strength | 50 | 544 | +/− 2.6 | 210 |
| n, V | Smin | Smax | Mean μ | Slope 1/s | s | m |
|---|---|---|---|---|---|---|
| n↗ V = cst | constant | constant | constant | ↗ | ↘ | ↗ |
| n↗ V = cst | constant | ↗ | ↗ | ↗ | ↘ | ↗ |
| n = cst V↗ | constant | ↘ | ↘ | ↗ | ↘ | indeterminate |
| V↗ n↘ | constant | ↘ | ↘ | ↘ | ↗ | ↘ |
| V ↗ | ↘ | ↘ | ↘ | ↗ | ↘ | indeterminate |
| V ↗ | ↘ | ↘ | ↘ | ↘ | ↗ | ↘ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lamon, J. Investigation of Variability of Flaw Strength Distributions on Brittle SiC Ceramic. Ceramics 2024, 7, 759-776. https://doi.org/10.3390/ceramics7020050
Lamon J. Investigation of Variability of Flaw Strength Distributions on Brittle SiC Ceramic. Ceramics. 2024; 7(2):759-776. https://doi.org/10.3390/ceramics7020050
Chicago/Turabian StyleLamon, Jacques. 2024. "Investigation of Variability of Flaw Strength Distributions on Brittle SiC Ceramic" Ceramics 7, no. 2: 759-776. https://doi.org/10.3390/ceramics7020050
APA StyleLamon, J. (2024). Investigation of Variability of Flaw Strength Distributions on Brittle SiC Ceramic. Ceramics, 7(2), 759-776. https://doi.org/10.3390/ceramics7020050






