Effects of Replacing Co2+ with Zn2+ on the Dielectric Properties of Ba [Zn1/3(Nb1/2Ta1/2)2/3]O3 Ceramics with High Dielectric Constant and High Quality Factor
Abstract
1. Introduction
2. Experimental Procedures
2.1. Synthesis of the Samples
2.2. Characterization of the Samples
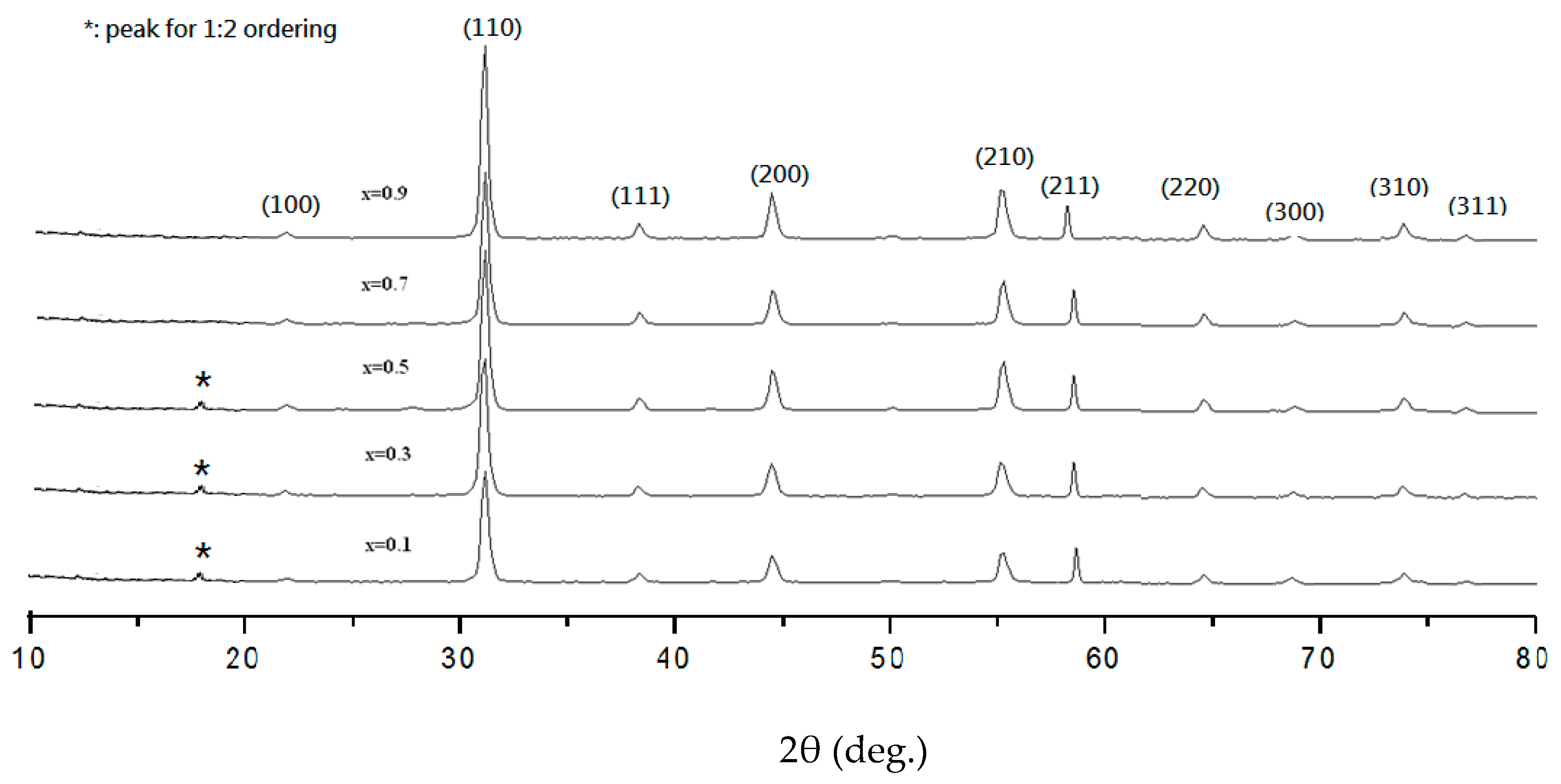
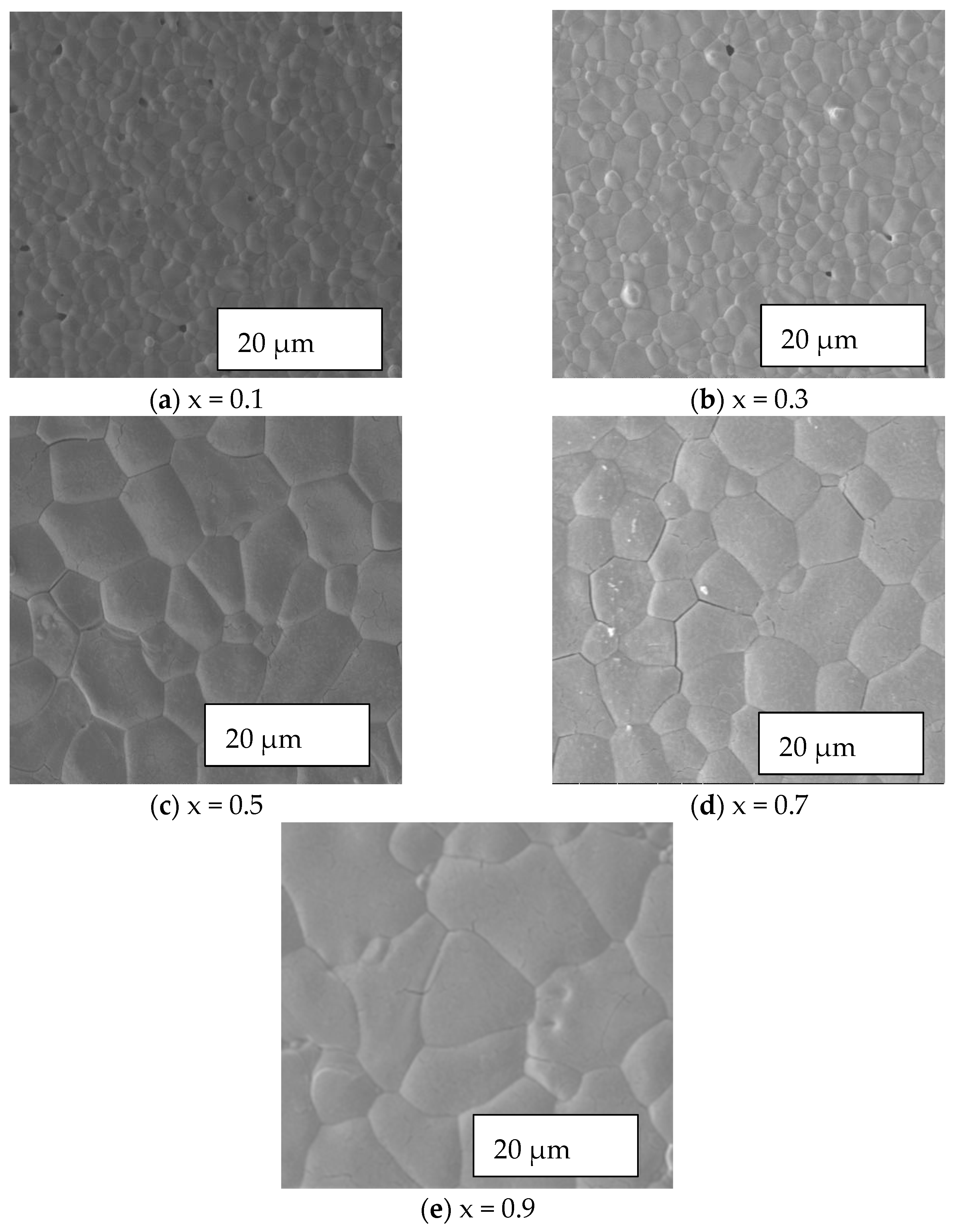
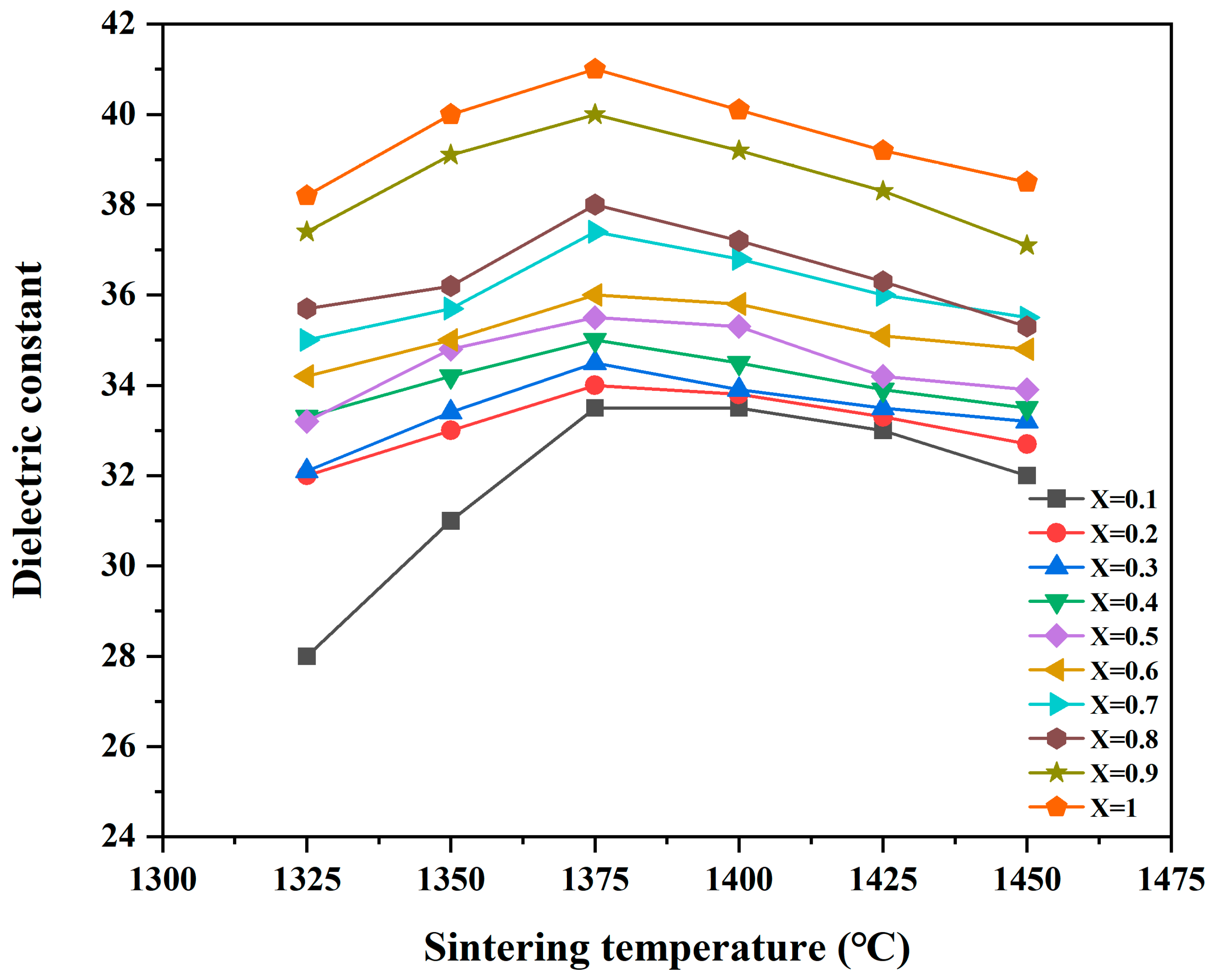
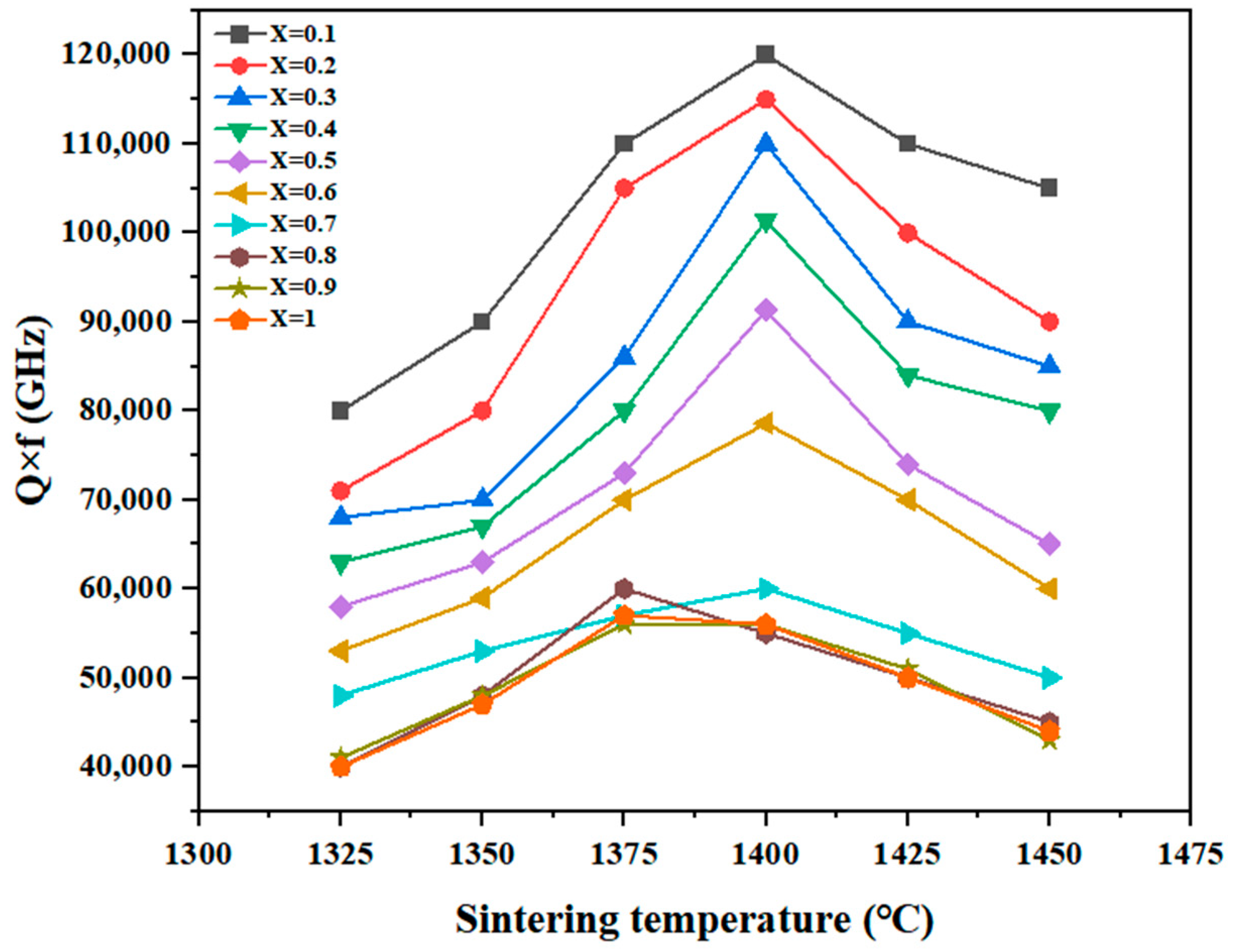
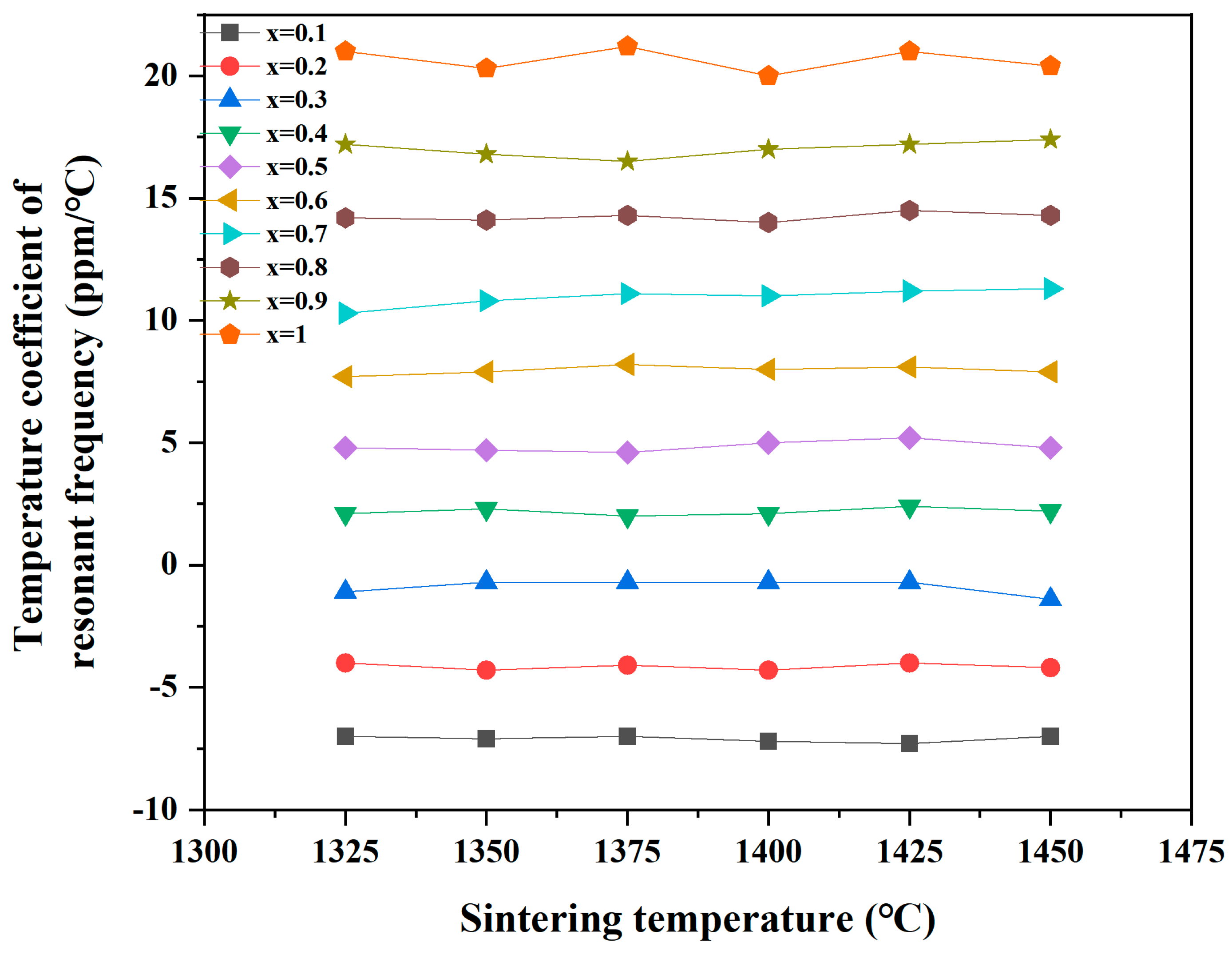
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Millet, J.M.; Roth, R.S.; Ettlinger, L.D.; Parker, H.S. Phase Equilibria and Crystal Chemistry in the Ternary Systems BaO-TiO2-Nb2. J. Solid State Chem. 1987, 67, 259–270. [Google Scholar] [CrossRef]
- Liu, F.; Cheng, L.-J.; Li, H.; Liu, S.-J. Ordering-induced domains in sub-micron-sized Ba(Zn1/3Ta2/3)O3–BaZrO3 microwave ceramics. J. Mater. Sci. Mater. Electron. 2021, 32, 26126–26136. [Google Scholar] [CrossRef]
- Siny, I.G.; Tao, R.; Katiyar, R.S.; Guo, R.; Bhalla, A.S. Raman spectroscopy of Mg-Ta order-disorder in BaMg1/3Ta2/3O3. J. Phys. Chem. Solids 1998, 59, 181–195. [Google Scholar] [CrossRef]
- Ioachim, A. Effect of the sintering temperature on the Ba(Zn1/3Ta2/3)O3 dielectric properties. J. Eur. Ceram. Soc. 2007, 27, 1117–1122. [Google Scholar] [CrossRef]
- Moussa, S.M.; Ibberson, R.M.; Bieringer, M.; Fitch, A.N.; Rosseinsky, M.R. In situ Measurement of Cation Order and Domain Growth in an Electroceramic. Chem. Mater. 2003, 15, 2527–2533. [Google Scholar] [CrossRef]
- Bensemma, N.; Trefalt, G.; Glinsek, S.; Kosec, M.; Taibi, K.; Abbaci, M. Investigation of the BaTiO3-BaMg1/3Nb2/3 system: Structural, dielectric, ferroelectric and electromechanical studies. J. Electroceram. 2013, 30, 206–212. [Google Scholar] [CrossRef]
- Kim, I.-T. Effects of non-stoichiometry and chemical inhomogeneity on the order-disorder phase formation in the complex perovskite compounds, Ba(Ni1/3Nb2/3)O3and Ba(Zn1/3Nb2/3)O3. J. Mater. Sci. 1995, 30, 514–521. [Google Scholar] [CrossRef]
- Fan, Y.; Zhou, Z.; Liang, R.; Zhou, M.; Dong, X. The effect of A-site nonstoichiometry on the microstructure, electric properties, and phase stability of NaNbO3 polycrystalline ceramics. J. Eur. Ceram. Soc. 2019, 39, 4712–4718. [Google Scholar] [CrossRef]
- Surendran, K.P.; Sebastian, M.T.; Mohanan, P.; Moreira, R.L.; Dias, A. Effect of Nonstoichiometry on the Structure and Microwave Dielectric Properties of Ba(Mg0.33Ta0.67)O3. Chem. Mater. 2005, 17, 142–151. [Google Scholar] [CrossRef]
- Storr, B.; Kodali, D.; Chakrabarty, K.; Baker, P.A.; Rangari, V.; Catledge, S.A. Single-Step Synthesis Process for High-Entropy Transition Metal Boride Powders Using Microwave Plasma. Ceramics 2021, 4, 257–264. [Google Scholar] [CrossRef]
- Yang, H. The latest process and challenges of microwave dielectric ceramics based on pseudo phase diagrams. J. Adv. Ceram. 2021, 10, 885–932. [Google Scholar] [CrossRef]
- Dernovsek, O.; Dernovsek, M.O.; Eberstein, M.; Schiller, W.A. LTCC glass-ceramic composites for microwave application. J. Eur. Ceram. Soc. 2001, 21, 1693–1697. [Google Scholar] [CrossRef]
- Liu, L.; Chen, Y.; Feng, Z.; Wu, H.; Zhang, X. Crystal structure; infrared spectra, and microwave dielectric properties of the EuNbO4 ceramic. Ceram. Int. 2021, 47, 4321–4326. [Google Scholar] [CrossRef]
- Ji, Y.; Song, K.; Zhang, S.; Lu, Z.; Wang, G.; Li, L.; Zhou, D.; Wang, D.; Reaney, I.M. Cold sintered, temperature-stable CaSnSiO5-K2MoO4 composite microwave ceramics and its prototype microstrip patch antenna. J. Eur. Ceram. Soc. 2021, 41, 424–429. [Google Scholar] [CrossRef]
- Chen, C.; Peng, Z.; Xie, L.; Bi, K.; Fu, X. Microwave dielectric properties of novel (1 − x)MgTiO3–xCa0.5Sr0.5TiO3 ceramics. J. Mater. Sci. Mater. Electron. 2020, 31, 13696–13703. [Google Scholar] [CrossRef]
- Zaman, A.; Uddin, S. Nasir Mehboob and Asad Ali, Structural investigation and improvement of microwave dielectric properties in Ca(HfxTi1−x)O3 ceramics. Phys. Scr. 2021, 96, 025701. [Google Scholar] [CrossRef]
- Bao, J.; Du, J.; Liu, L.; Wu, H.; Zhou, Y.; Yue, Z. A new type of microwave dielectric ceramic based on K2O–SrO–P2O5 composition with high quality factor and low sintering temperature. Ceram. Int. 2022, 48, 784–794. [Google Scholar] [CrossRef]
- Nomura, S.; Kaneta, K. Ba(Mg1/3Ta2/3)O3 Ceramics with Temperature-Stable High Dielectric Constant and Low Microwave Loss. Jpn. J. Appl. Phys. 1982, 21, L624. [Google Scholar] [CrossRef]
- Kawashima, S.; Nishida, M.; Ueda, I.; Ouchi, H. Dielectric properties of Ba(Zn,Ta)O-Ba(Zn,Nb)O ceramic. Proc. Ferroelectr. Mater. 1977, 1, 293. [Google Scholar]
- Desu, S.B.; O’Bryan, H.M. Microwave Loss Quality of BaZn1/3Ta2/3O3 Ceramics. J. Am. Ceram. Soc. 1985, 68, 546. [Google Scholar] [CrossRef]
- Larson, A.C.; Von Dreele, R.B. Los Alamos National Laboratory Report LAUR-86-748. 1987. Available online: https://11bm.xray.aps.anl.gov/documents/GSASManual.pdf (accessed on 25 January 2024).
- Hakki, B.W.; Coleman, P.D. A Dielectric Resonator Method of Measuring Inductive Capacities in the Millimeter Range. IRE Trans. Microw. Theory Tech. 1960, 8, 402. [Google Scholar] [CrossRef]
- Belous, A.; Ovchar, O.; Durilin, D.; Krzmanc, M.M.; Valant, M.; Suvorov, D. High-Q Microwave Dielectric Materials Based on the Spinel Mg2TiO4. J. Am. Ceram. Soc. 2006, 89, 3441. [Google Scholar] [CrossRef]
- Hannay, J.H. Constantino Grosse. DIBORIDE MICROPATTERNED SURFACES FOR CELL CULTURE. Ferroelectrics 1988, 86, 171–179. (United States Patent Application 20230133393). Available online: https://www.zhangqiaokeyan.com/patent-detail/06130502846548.html (accessed on 25 January 2024).
- Shannon, S.R.D. Dielectric polarizabilities of ions in oxides and fluorides. J. Appl. Phys. 1993, 73, 348–366. [Google Scholar] [CrossRef]
- Lichtenecker, K. Dielectric constant of natural and synthetic mixtures. Phys. Z. 1926, 27, 115. [Google Scholar]
- Chen, Y.-B. Dielectric properties and crystal structure of (Mg0.9Zn0.05Co0.05)4(Nb(1−x)Tax)2O9 ceramics. J. Alloys Compd. 2012, 541, 283–287. [Google Scholar] [CrossRef]
- Penn, S.J.; Alford, N.M.; Templeton, A.; Wang, X.; Xu, M. Michael Reece and Kevin Schrapel. Am. Ceram. Soc. 1997, 80, 1885–1888. [Google Scholar] [CrossRef]

| x= | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 |
|---|---|---|---|---|---|---|---|---|---|
| a (Å) | 5.7753 (2) | 5.7756 (2) | 5.7761 (5) | 5.7784 (1) | 5.78025 (3) | 5.78123 (1) | 5.78237 (6) | 5.78242(1) | 5.78415 (3) |
| b (Å) | 5.7753 (2) | 5.7756 (2) | 5.7761 (5) | 5.7784 (1) | 5.78025 (3) | 5.78123 (1) | 5.78237 (6) | 5.78242 (1) | 5.78415 (3) |
| c (Å) | 7.0873 (4) | 7.0874 (3) | 7.0873 (1) | 7.0873 (4) | 7.0874 (1) | 7.0872 (3) | 7.0873 (2) | 7.0871 (2) | 7.0873 (1) |
| Vm (Å 3) | 204.7612 | 204.8312 | 204.9012 | 204.9712 | 205.0412 | 205.1112 | 205.1812 | 205.2512 | 205.3212 |
| αm (theoretical) | 46.82 | 46.83 | 46.86 | 46.89 | 46.9 | 4.692 | 46.93 | 46.94 | 46.96 |
| αm (exp.) | 44.73 | 44.77 | 44.78 | 44.85 | 44.93 | 44.98 | 45.08 | 45.12 | 45.3 |
| εr (cal.) | 33.5 | 33.8 | 33.9 | 34.5 | 35.3 | 35.8 | 36.8 | 37.2 | 39.2 |
| εr (measured) | 33.31 | 33.61 | 33.71 | 34.3 | 35.09 | 35.59 | 36.58 | 36.97 | 38.95 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.-B.; Fan, Y.; Chang, S.-H.; Shen, S. Effects of Replacing Co2+ with Zn2+ on the Dielectric Properties of Ba [Zn1/3(Nb1/2Ta1/2)2/3]O3 Ceramics with High Dielectric Constant and High Quality Factor. Ceramics 2024, 7, 426-435. https://doi.org/10.3390/ceramics7010027
Chen Y-B, Fan Y, Chang S-H, Shen S. Effects of Replacing Co2+ with Zn2+ on the Dielectric Properties of Ba [Zn1/3(Nb1/2Ta1/2)2/3]O3 Ceramics with High Dielectric Constant and High Quality Factor. Ceramics. 2024; 7(1):426-435. https://doi.org/10.3390/ceramics7010027
Chicago/Turabian StyleChen, Yuan-Bin, Yu Fan, Shiuan-Ho Chang, and Shaobing Shen. 2024. "Effects of Replacing Co2+ with Zn2+ on the Dielectric Properties of Ba [Zn1/3(Nb1/2Ta1/2)2/3]O3 Ceramics with High Dielectric Constant and High Quality Factor" Ceramics 7, no. 1: 426-435. https://doi.org/10.3390/ceramics7010027
APA StyleChen, Y.-B., Fan, Y., Chang, S.-H., & Shen, S. (2024). Effects of Replacing Co2+ with Zn2+ on the Dielectric Properties of Ba [Zn1/3(Nb1/2Ta1/2)2/3]O3 Ceramics with High Dielectric Constant and High Quality Factor. Ceramics, 7(1), 426-435. https://doi.org/10.3390/ceramics7010027







