Characterization of the Dissolution of Water Microdroplets in Oil
Abstract
1. Introduction
2. Materials and Methods
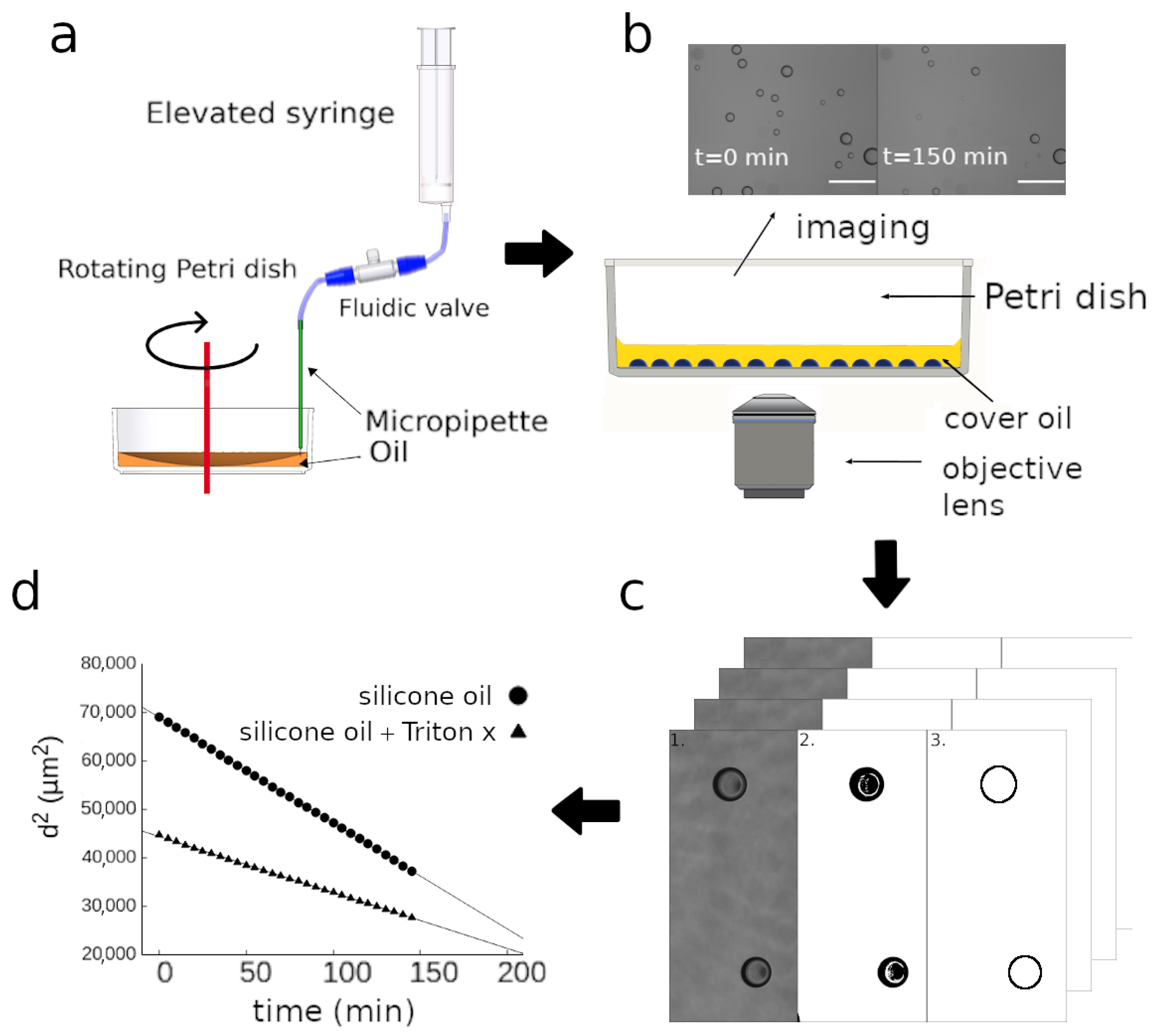
2.1. Droplet Generation
2.2. Time-Lapse Analysis
2.3. Image Analysis
2.4. Moisture Determination of Oils
3. Results
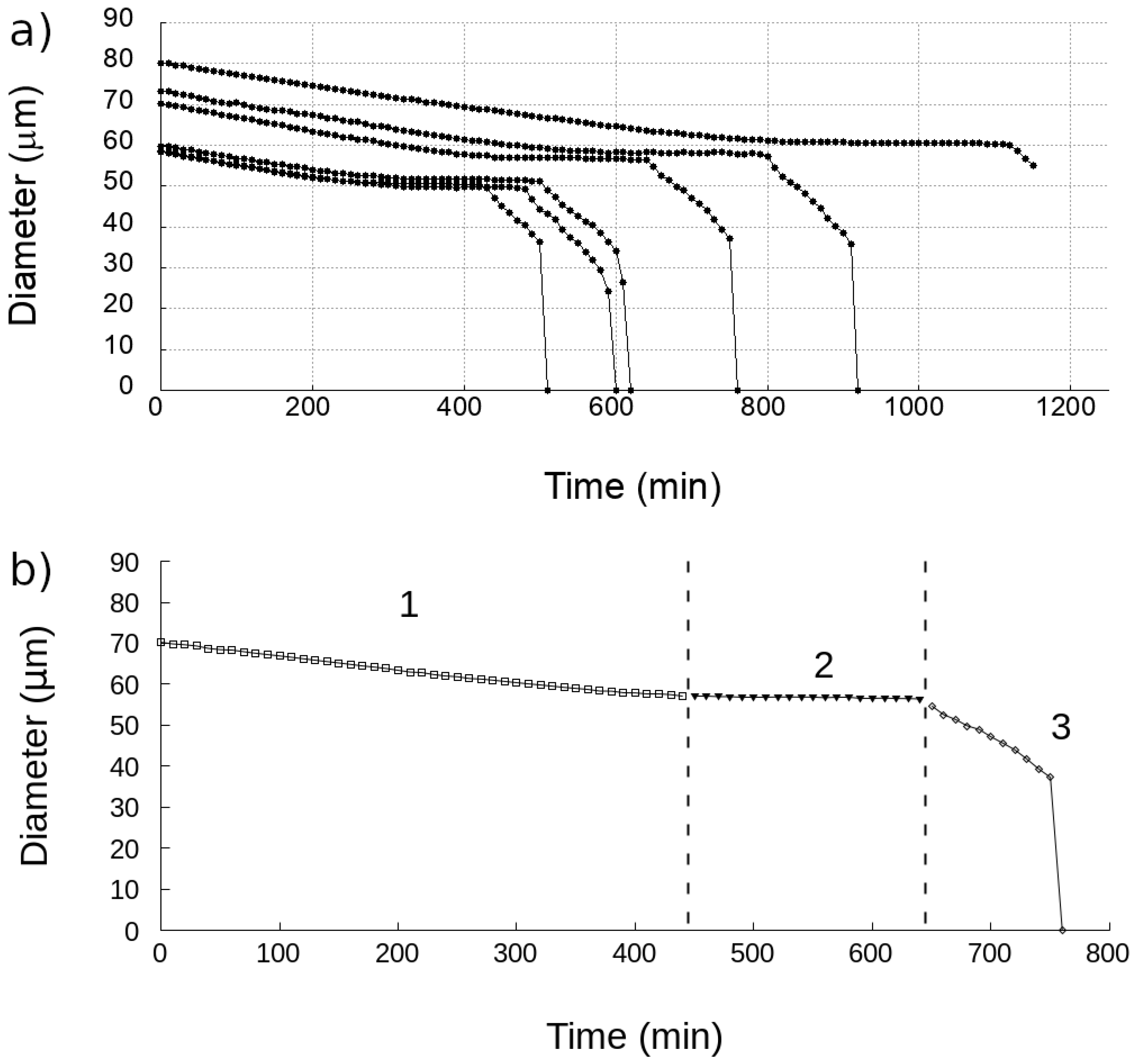
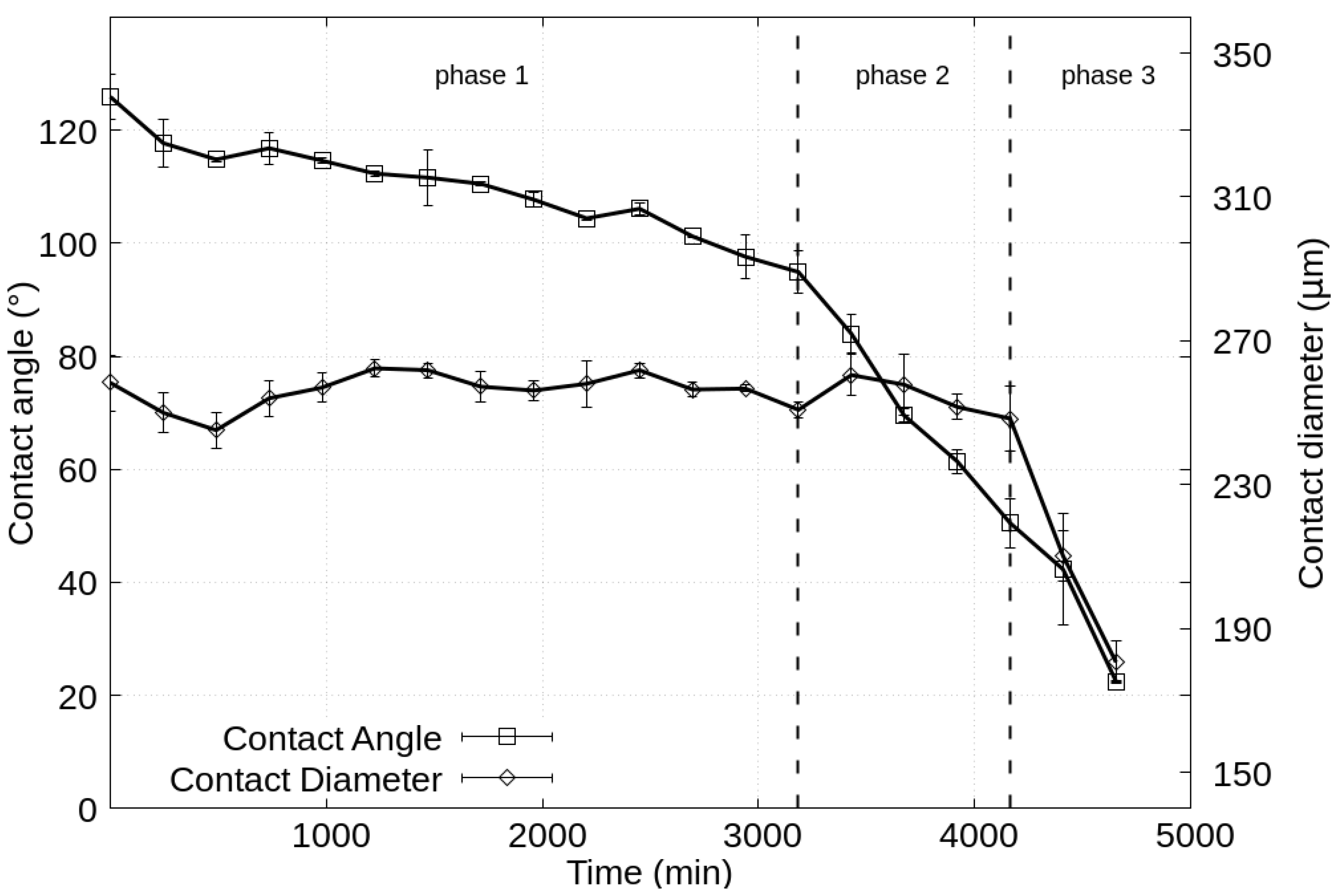
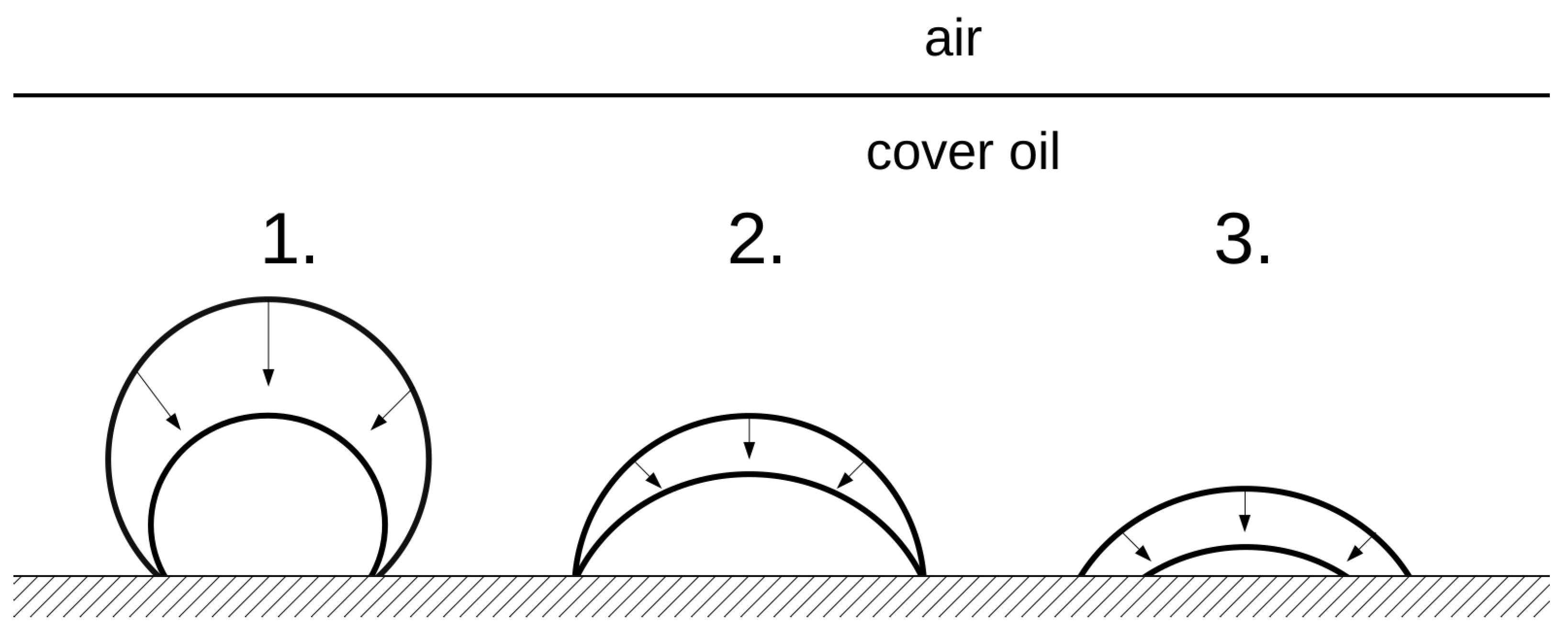
3.1. Time-Lapse Analysis of Droplets
3.2. Calculation of the Diffusion Coefficient and Saturation Density
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Griffiths, A.D.; Tawfik, D.S. Miniaturising the laboratory in emulsion droplets. Trends Biotechnol. 2006, 24, 395–402. [Google Scholar] [CrossRef]
- Tadros, T.F. Fundamental principles of emulsion rheology and their applications. Colloids Surf. A 1994, 91, 39–55. [Google Scholar] [CrossRef]
- Weitz, D.A. Perspective on droplet-based single-cell sequencing. Lab Chip 2017, 17, 2539. [Google Scholar] [CrossRef]
- Ding, Y.; Choo, J.; Demello, A.J. From single-molecule detection to next-generation sequencing: Microfluidic droplets for high-throughput nucleic acid analysis. Microfluid. Nanofluid. 2017, 21, 58. [Google Scholar] [CrossRef]
- Shembekar, N.; Chaipan, C.; Utharala, R.; Merten, C.A. Droplet-based microfluidics in drug discovery, transcriptomics and high-throughput molecular genetics. Lab Chip 2016, 16, 1314–1331. [Google Scholar] [CrossRef] [PubMed]
- Haeberle, S.; Zengerle, R. Microfluidic platforms for lab-on-a-chip applications. Lab Chip 2007, 7, 1094–1110. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Y.; Zhang, Y.X.; Liu, W.W.; Ma, Y.; Fang, Q.; Yao, B. Printing 2-dimentional droplet array for single-cell reverse transcription quantitative PCR assay with a microfluidic robot. Sci. Rep. 2015, 5, 9551. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Y.; Zhu, L.N.; Guo, R.; Cui, H.J.; Ye, S.; Fang, Q. Nanoliter-scale protein crystallization and screening with a microfluidic droplet robot. Sci. Rep. 2014, 4, 5046. [Google Scholar] [CrossRef] [PubMed]
- White, A.K.; VanInsberghe, M.; Petriv, I.; Hamidi, M.; Sikorski, D.; Marra, M.A.; Piret, J.; Aparicio, S.; Hansen, C.L. High-throughput microfluidic single-cell RT-qPCR. Proc. Natl. Acad. Sci. USA 2011, 108, 13999–14004. [Google Scholar] [CrossRef] [PubMed]
- Dalerba, P.; Kalisky, T.; Sahoo, D.; Rajendran, P.S.; Rothenberg, M.E.; Leyrat, A.A.; Sim, S.; Okamoto, J.; Johnston, D.M.; Qian, D.; et al. Single-cell dissection of transcriptional heterogeneity in human colon tumors. Nat. Biotechnol. 2011, 29, 1120–1127. [Google Scholar] [CrossRef] [PubMed]
- Murphy, S.V.; Atala, A. 3D bioprinting of tissues and organs. Nat. Biotechnol. 2014, 32, 773–785. [Google Scholar] [CrossRef] [PubMed]
- Francz, B.; Ungai-Salánki, R.; Sautner, É.; Horvath, R.; Szabó, B. Subnanoliter precision piezo pipette for single-cell isolation and droplet printing. Microfluid. Nanofluid. 2020, 24, 12. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhang, Y.X.; Cai, L.F.; Fang, Q. Sequential operation droplet array: An automated microfluidic platform for picoliter-scale liquid handling, analysis, and screening. Anal. Chem. 2013, 85, 6723–6731. [Google Scholar] [CrossRef] [PubMed]
- Mazutis, L.; Gilbert, J.; Ung, W.L.; Weitz, D.A.; Griffiths, A.D.; Heyman, J.A. Single-cell analysis and sorting using droplet-based microfluidics. Nat. Protoc. 2013, 8, 870. [Google Scholar] [CrossRef]
- Shah, R.K.; Shum, H.C.; Rowat, A.C.; Lee, D.; Agresti, J.J.; Utada, A.S.; Chu, L.Y.; Kim, J.W.; Fernandez-Nieves, A.; Martinez, C.J.; et al. Designer emulsions using microfluidics. Mater. Today 2008, 11, 18–27. [Google Scholar] [CrossRef]
- Baret, J.C. Surfactants in droplet-based microfluidics. Lab Chip 2012, 12, 422–433. [Google Scholar] [CrossRef]
- Picknett, R.; Bexon, R. The evaporation of sessile or pendant drops in still air. J. Colloid Interface Sci. 1977, 61, 336–350. [Google Scholar] [CrossRef]
- Soolaman, D.M.; Yu, H.Z. Water microdroplets on molecularly tailored surfaces: Correlation between wetting hysteresis and evaporation mode switching. J. Phys. Chem. B 2005, 109, 17967–17973. [Google Scholar] [CrossRef]
- Erbil, H.Y.; McHale, G.; Newton, M. Drop evaporation on solid surfaces: Constant contact angle mode. Langmuir 2002, 18, 2636–2641. [Google Scholar] [CrossRef]
- Epstein, P.S.; Plesset, M.S. On the stability of gas bubbles in liquid-gas solutions. J. Chem. Phys. 1950, 18, 1505–1509. [Google Scholar] [CrossRef]
- Su, J.T.; Needham, D. Mass transfer in the dissolution of a multicomponent liquid droplet in an immiscible liquid environment. Langmuir 2013, 29, 13339–13345. [Google Scholar] [CrossRef] [PubMed]
- Duncan, P.B.; Needham, D. Microdroplet Dissolution into a Second-Phase Solvent Using a Micropipet Technique: Test of the Epstein- Plesset Model for an Aniline- Water System. Langmuir 2006, 22, 4190–4197. [Google Scholar] [CrossRef] [PubMed]
- Schmitt, M.; Toor, R.; Denoyel, R.; Antoni, M. Spontaneous Microstructure Formation at Water/Paraffin Oil Interfaces. Langmuir 2017, 33, 14011–14019. [Google Scholar] [CrossRef] [PubMed]
- Rodríguez-Ruiz, I.; Hammadi, Z.; Grossier, R.; Gomez-Morales, J.; Veesler, S. Monitoring picoliter sessile microdroplet dynamics shows that size does not matter. Langmuir 2013, 29, 12628–12632. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Wang, J.; Bao, L.; Dietrich, E.; van der Veen, R.C.; Peng, S.; Friend, J.; Zandvliet, H.J.; Yeo, L.; Lohse, D. Mixed mode of dissolving immersed nanodroplets at a solid–water interface. Soft Matter 2015, 11, 1889–1900. [Google Scholar] [CrossRef]
- Velazquez, J.; Hileman, O., Jr. Studies on nucleation from solution of some soluble inorganic salts. Can. J. Chem. 1970, 48, 2896–2899. [Google Scholar] [CrossRef]
- Grossier, R.; Magnaldo, A.; Veesler, S. Ultra-fast crystallization due to confinement. J. Cryst. Growth 2010, 312, 487–489. [Google Scholar] [CrossRef]
- Bitterfield, D.L.; Utoft, A.; Needham, D. An Activity-Based Dissolution Model for Solute-Containing Microdroplets. Langmuir 2016, 32, 12749–12759. [Google Scholar] [CrossRef]
- Utoft, A.; Kinoshita, K.; Bitterfield, D.L.; Needham, D. Manipulating Single Microdroplets of NaCl Solutions: Solvent Dissolution, Microcrystallization, and Crystal Morphology. Langmuir 2018, 34, 3626–3641. [Google Scholar] [CrossRef]
- Pal, K.; Ramkrishna, D.; Nagy, Z.K. Mathematical Modeling of Emulsion Solvent Diffusion for Spherical Crystallization: How To Deconvolute Primary Crystal Size Distribution from Agglomerate Size Distribution? Ind. Eng. Chem. Res. 2020, 59, 6288–6300. [Google Scholar] [CrossRef]
- Espitalier, F.; Biscans, B.; Laguerie, C.; Deleuil, M. Spherical crystallization: Modeling of the emulsion solvent diffusion technique. KONA Powder Part. J. 1997, 15, 159–169. [Google Scholar] [CrossRef][Green Version]
- Pittermannova, A.; Ruberova, Z.; Zadražil, A.; Bremond, N.; Bibette, J.; Štěpánek, F. Microfluidic fabrication of composite hydrogel microparticles in the size range of blood cells. RSC Adv. 2016, 6, 103532–103540. [Google Scholar] [CrossRef]
- Umbanhowar, P.; Prasad, V.; Weitz, D.A. Monodisperse emulsion generation via drop break off in a coflowing stream. Langmuir 2000, 16, 347–351. [Google Scholar] [CrossRef]
- Mehta, R.; More, U.; Malek, N.; Chakraborty, M.; Parikh, P. Study of stability and thermodynamic properties of water-in-diesel nanoemulsion fuels with nano-Al additive. Appl. Nanosci. 2015, 5, 891–900. [Google Scholar] [CrossRef]
- Schindelin, J.; Arganda-Carreras, I.; Frise, E.; Kaynig, V.; Longair, M.; Pietzsch, T.; Preibisch, S.; Rueden, C.; Saalfeld, S.; Schmid, B.; et al. Fiji: An open-source platform for biological-image analysis. Nat. Methods 2012, 9, 676–682. [Google Scholar] [CrossRef] [PubMed]
- Zack, G.W.; Rogers, W.E.; Latt, S. Automatic measurement of sister chromatid exchange frequency. J. Histochem. Cytochem. 1977, 25, 741–753. [Google Scholar] [CrossRef]
- Stalder, A.F.; Kulik, G.; Sage, D.; Barbieri, L.; Hoffmann, P. A snake-based approach to accurate determination of both contact points and contact angles. Colloids Surf. A 2006, 286, 92–103. [Google Scholar] [CrossRef]
- Webster, A.; Cates, M. Stabilization of emulsions by trapped species. Langmuir 1998, 14, 2068–2079. [Google Scholar] [CrossRef]
- Nilsson, P.; Lindman, B. Water Self-Diff usion in Nonionic Surfactant Solutions. Hydration and Obstruction Effects. J. Phys. Chem. 1983, 87, 4756–4761. [Google Scholar] [CrossRef]
- Hilder, M.; van den Tempe, M. Diffusivity of water in groundnut oil and paraffi oil. J. Appl. Chem. Biotechnol. 1971, 21, 176–178. [Google Scholar] [CrossRef]

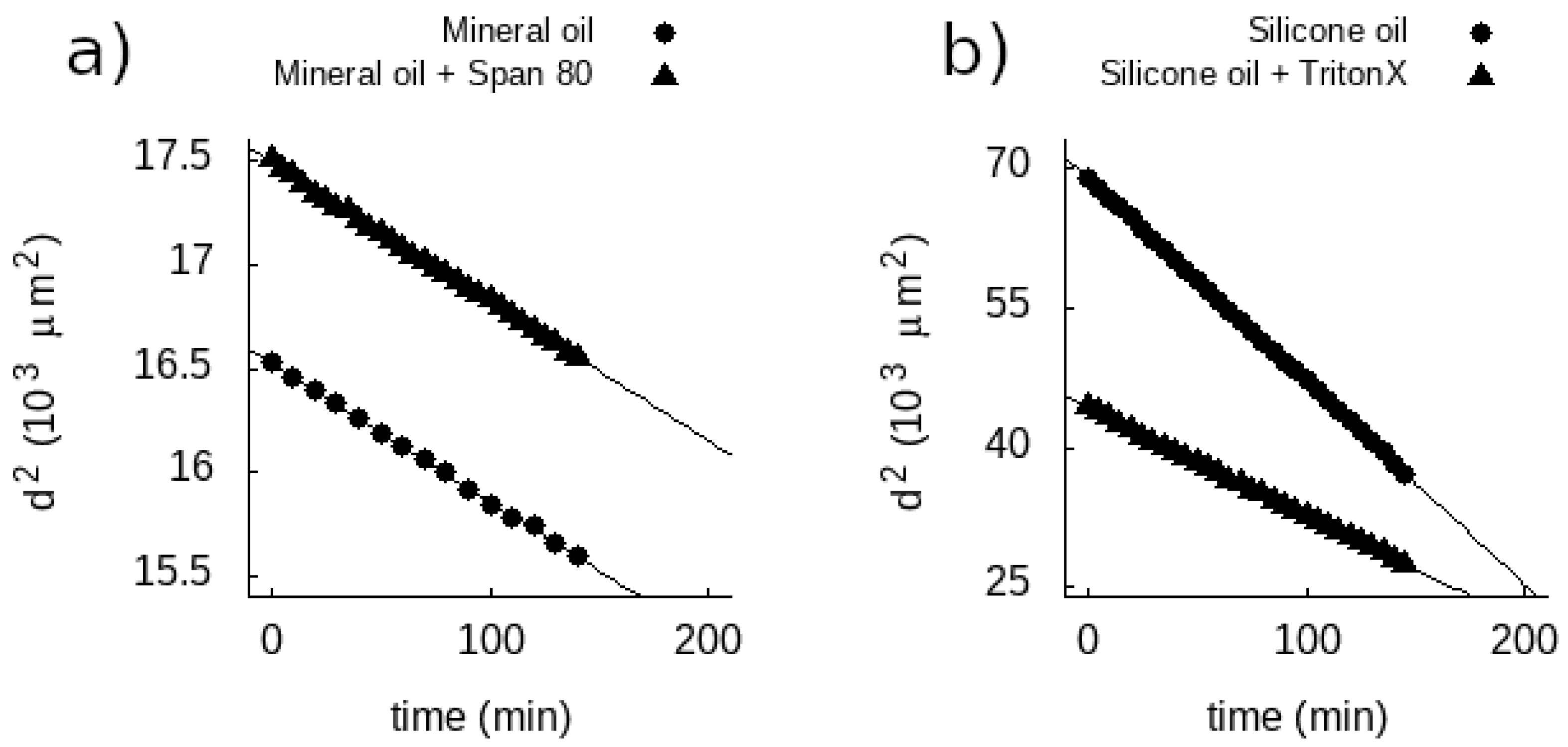
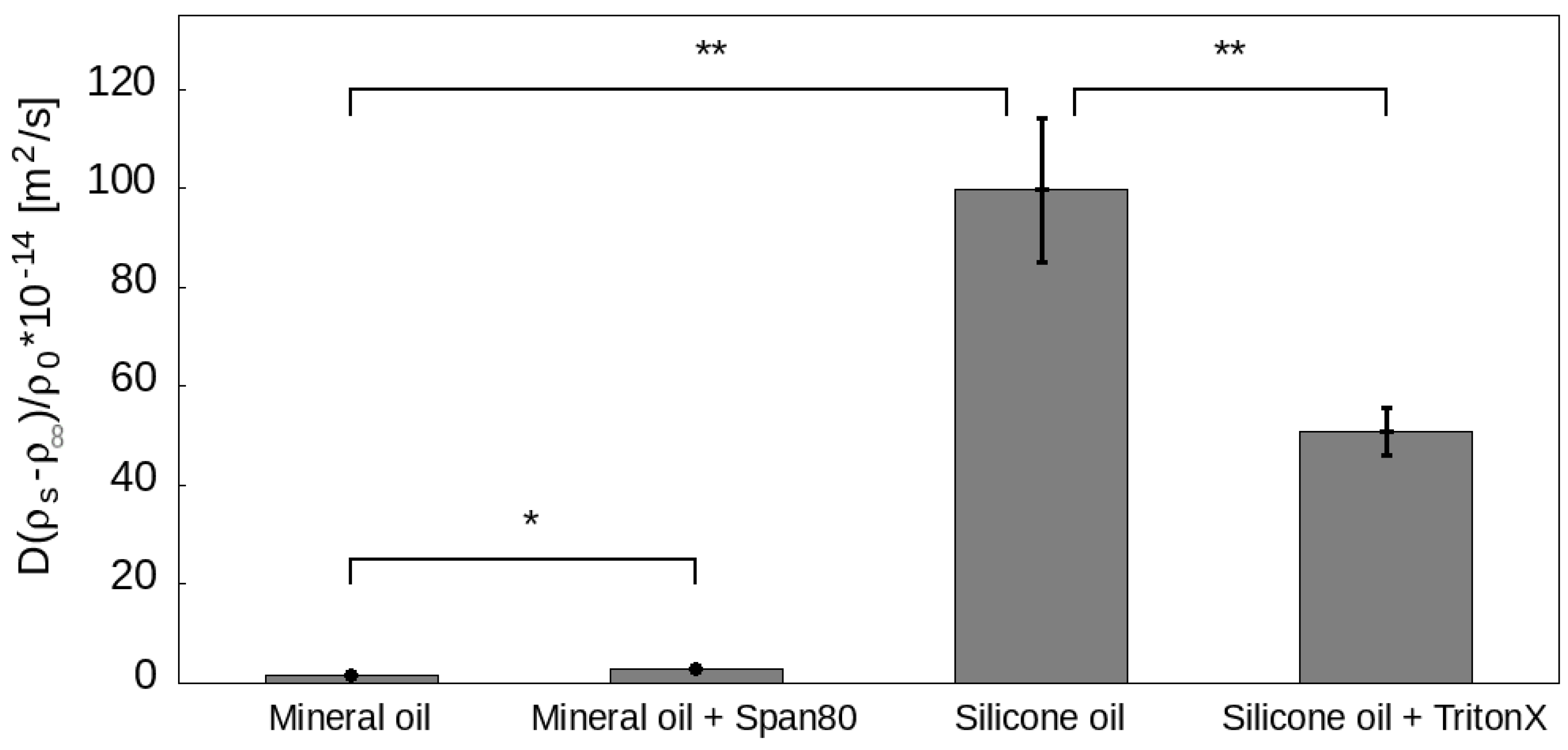
| Mineral Oil | Mineral Oil + Span80 | Silicone Oil | Silicone Oil + Triton X-100 |
|---|---|---|---|
| Mineral Oil | Saturated Mineral Oil | Silicone Oil | Saturated Silicone Oil |
|---|---|---|---|
| 341 | 480 | 609 | 2902 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gerecsei, T.; Ungai-Salánki, R.; Saftics, A.; Derényi, I.; Horvath, R.; Szabó, B. Characterization of the Dissolution of Water Microdroplets in Oil. Colloids Interfaces 2022, 6, 14. https://doi.org/10.3390/colloids6010014
Gerecsei T, Ungai-Salánki R, Saftics A, Derényi I, Horvath R, Szabó B. Characterization of the Dissolution of Water Microdroplets in Oil. Colloids and Interfaces. 2022; 6(1):14. https://doi.org/10.3390/colloids6010014
Chicago/Turabian StyleGerecsei, Tamás, Rita Ungai-Salánki, András Saftics, Imre Derényi, Robert Horvath, and Bálint Szabó. 2022. "Characterization of the Dissolution of Water Microdroplets in Oil" Colloids and Interfaces 6, no. 1: 14. https://doi.org/10.3390/colloids6010014
APA StyleGerecsei, T., Ungai-Salánki, R., Saftics, A., Derényi, I., Horvath, R., & Szabó, B. (2022). Characterization of the Dissolution of Water Microdroplets in Oil. Colloids and Interfaces, 6(1), 14. https://doi.org/10.3390/colloids6010014







