Deposition of Synthetic and Bio-Based Polycations onto Negatively Charged Solid Surfaces: Effect of the Polymer Cationicity, Ionic Strength, and the Addition of an Anionic Surfactant
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Study of the Adsorption onto Solid Surfaces
2.3. Self-Consistent Field Calculations
- Chitosan:(C)(((G)2[(C)(OH)][(OH)](G)[(O)][(Z)2[(Z)][(Z)](Z)](C)(O))n((G)2[(C)(OH)](G)[(OH)(C)[O](C)][(O)](C)(O))m)p(C)
- JR400:(C)(((G)2[(C)][(C)(O)(C)2(OH)](G)[(C)(OH)][(O)](C)(O)1((G)2[(C)(O)((C)2(O))2(C)2[(OH)](C)(N)2[(N)][(N)](N)][(C)](G)[(O)][(OH)](C)(O))1)k(C)
- PDADMAC: ((C)(N)2[(N)][(N)](N)(C))l
- SLES: (C)12((O)(C)2)2(S)2[(S)[(S)](S)
- Acetic Acid: (C)(C)[(OH)](X)2[(X)][(X)](X)
3. Results and Discussion
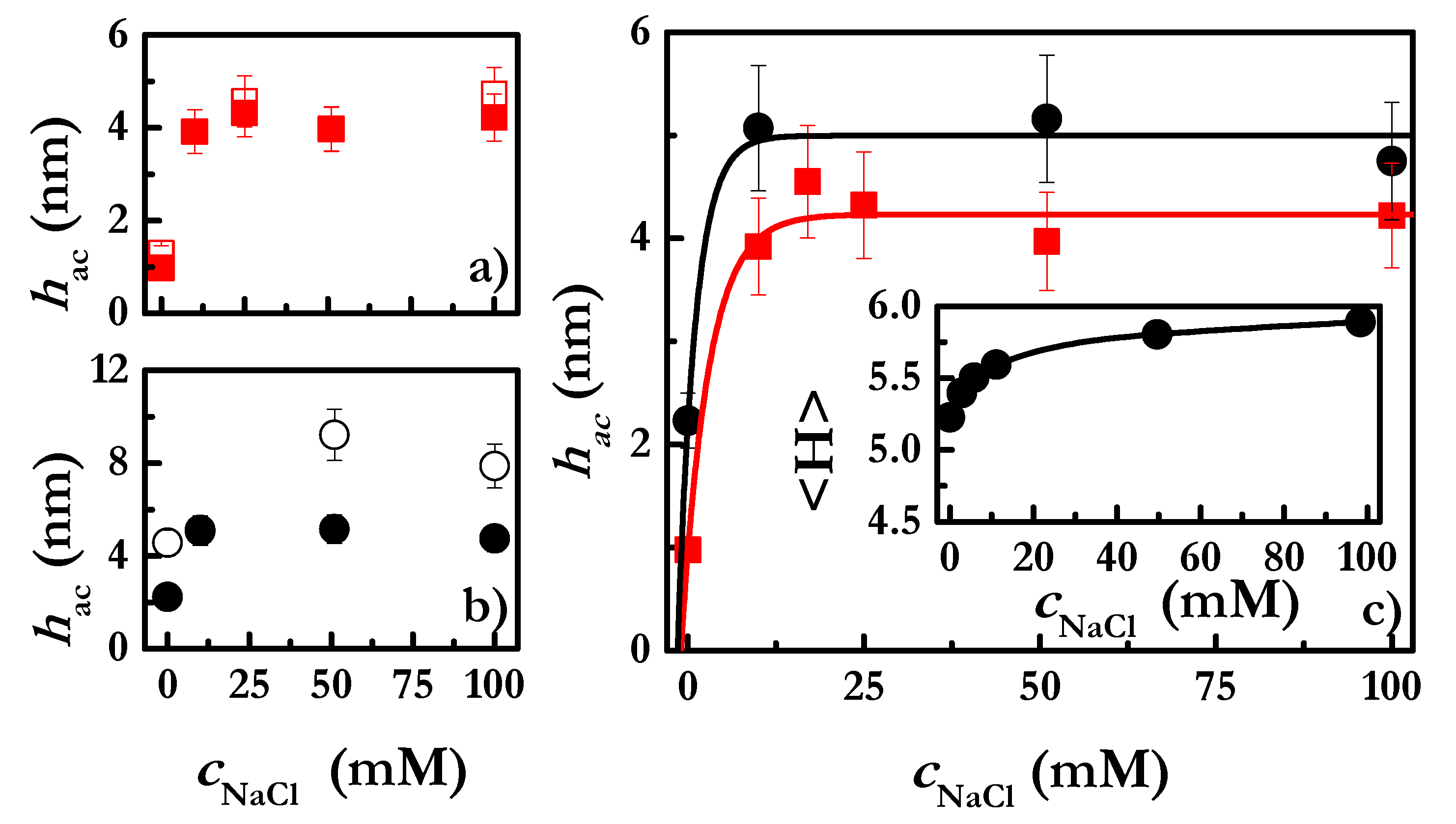
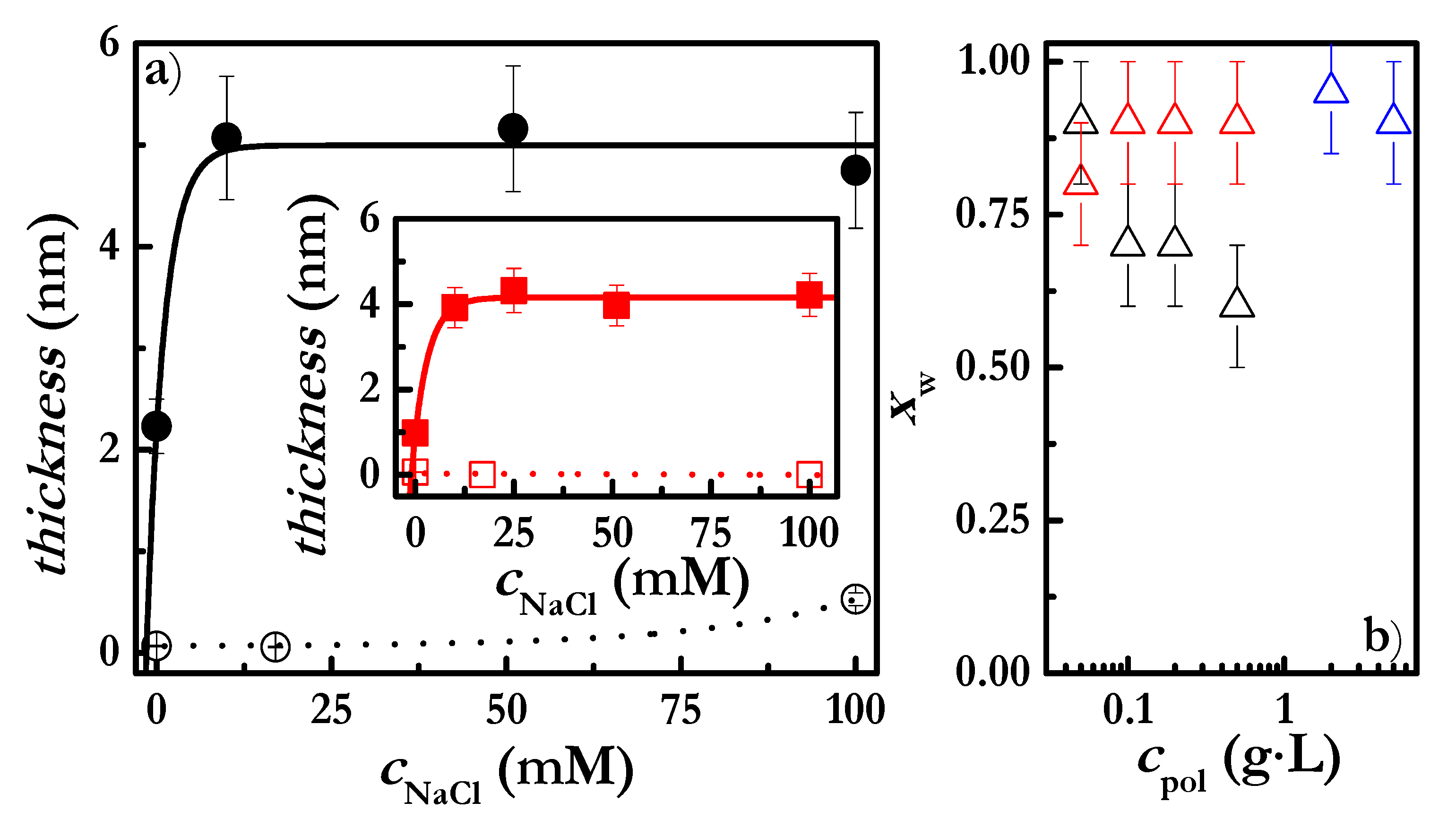
3.1. Effect of the Ionic Strength, Polymer Cationicity and Polymer Concentration on the Deposition of Polyelectrolytes
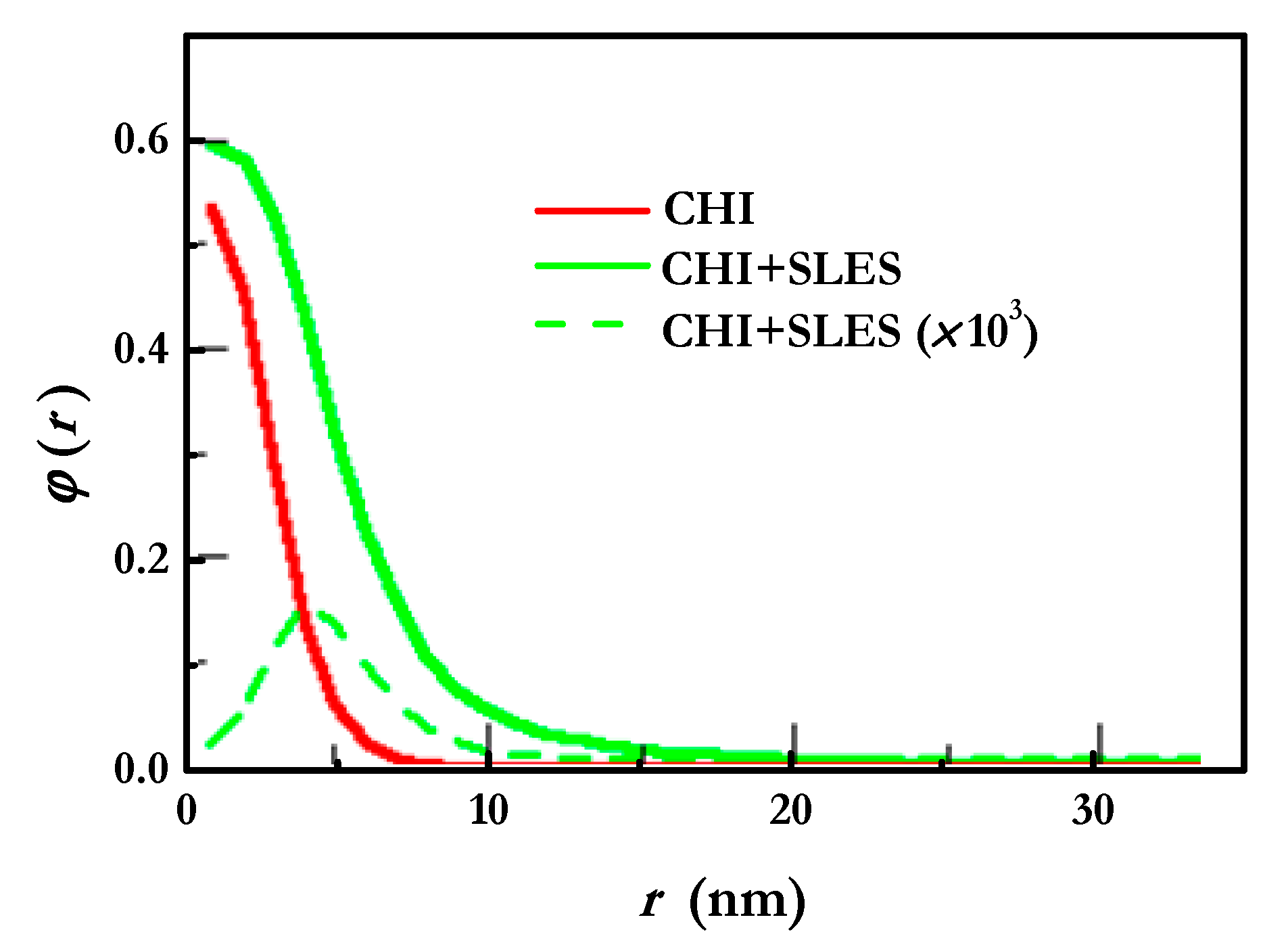
3.2. Effect of the Addition of an Oppositely Charged Surfactant on the Deposition of Polyelectrolyte Layers
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Llamas, S.; Guzmán, E.; Ortega, F.; Baghdadli, N.; Cazeneuve, C.; Rubio, R.G.; Luengo, G.S. Adsorption of polyelectrolytes and polyelectrolytes-surfactant mixtures at surfaces: A physico-chemical approach to a cosmetic challenge. Adv. Colloid Interface Sci. 2015, 222, 461–487. [Google Scholar] [CrossRef]
- Zhu, X.; Jańczewski, D.; Lee, S.; Teo, S.; Vancso, G. Cross-linked polyelectrolyte multi layers for marine antifouling applications. ACS Appl. Mat. Interfaces 2013, 5, 5961–5968. [Google Scholar] [CrossRef] [PubMed]
- Zhang, W.; Ji, X.; Ma, N.; Kong, L.; Ran, F. Wettability improvement of vanadium nitride/carbon electrode nanomaterial by electrostatic absorption of hydrophilic poly (allylamine hydrochloride). Appl. Surface Sci. 2020, 525, 146619. [Google Scholar] [CrossRef]
- Guo, J.-W.; Wang, C.-F.; Chen, S.-H.; Lai, J.-Y.; Lu, C.-H.; Chen, J.-K. Highly efficient self-cleaning of heavy polyelectrolyte coated electrospun polyacrylonitrile nanofibrous membrane for separation of oil/water emulsions with intermittent pressure. Sep. Purif. Technol. 2020, 234, 116106. [Google Scholar] [CrossRef]
- Claesson, P.M.; Dedinaite, A.; Rojas, O.J. Polyelectrolytes as adhesion modifiers. Adv. Colloid Interface Sci. 2003, 104, 53–74. [Google Scholar] [CrossRef]
- Elżbieciak-Wodka, M.; Kolasińska-Sojka, M.; Wodka, D.; Nowak, P.; Warszyński, P. Transfer of electroactive agents of different molecular size through the polyelectrolyte membranes. J. Electroanal. Chem. 2011, 661, 162–170. [Google Scholar] [CrossRef]
- Enarsson, L.-E.; Wågberg, L. Adsorption kinetics of cationic polyelectrolytes studied with stagnation point adsorption reflectometry and quartz crystal microgravimetry. Langmuir 2008, 24, 7329–7337. [Google Scholar] [CrossRef]
- Guzmán, E.; Ortega, F.; Baghdadli, N.; Luengo, G.S.; Rubio, R.G. Effect of the molecular structure on the adsorption of conditioning polyelectrolytes on solid substrates. Colloids Surf. A 2011, 375, 209–218. [Google Scholar] [CrossRef]
- Guzmán, E.; Ortega, F.; Baghdadli, N.; Cazeneuve, C.; Luengo, G.S.; Rubio, R.G. Adsorption of conditioning polymers on solid substrates with different charge density. ACS Appl. Mat. Interfaces 2011, 3, 3181–3188. [Google Scholar] [CrossRef]
- Panja, D.; Barkema, G.T.; Kolomeisky, A.B. Non-equilibrium dynamics of single polymer adsorption to solid surfaces. J. Phys. Cond. Matt. 2009, 21, 242101. [Google Scholar] [CrossRef]
- Netz, R.R.; Andelman, D. Neutral and charged polymers at interfaces. Phys. Rep. 2003, 380, 1–95. [Google Scholar] [CrossRef]
- Linse, P.; Källrot, N. Polymer adsorption from bulk solution onto planar surfaces: Effect of polymer flexibility and surface attraction in good solvent. Macromolecules 2010, 43, 2054–2068. [Google Scholar] [CrossRef]
- Guzmán, E.; Fernández-Peña, L.; Luengo, G.S.; Rubio, A.M.; Rey, A.; Léonforte, F. Self-consistent mean field calculations of polyelectrolyte-surfactant mixtures in solution and upon adsorption onto negatively charged surfaces. Polymers 2020, 12, 624. [Google Scholar] [CrossRef] [PubMed]
- Porus, M.; Maroni, P.; Borkovec, M. Structure of adsorbed polyelectrolyte monolayers investigated by combining optical reflectometry and piezoelectric techniques. Langmuir 2012, 28, 5642–5651. [Google Scholar] [CrossRef] [PubMed]
- Mészáros, R.; Varga, I.; Gilányi, T. Adsorption of poly(ethyleneimine) on silica surfaces:effect of pH on the reversibility of adsorption. Langmuir 2004, 20, 5026–5029. [Google Scholar] [CrossRef] [PubMed]
- Ballauff, M. Spherical polyelectrolyte brushes. Prog. Polym. Sci. 2007, 32, 1135–1151. [Google Scholar] [CrossRef]
- Szilagyi, I.; Trefalt, G.; Tiraferri, A.; Maroni, P.; Borkovec, M. Polyelectrolyte adsorption, interparticle forces, and colloidal aggregation. Soft Matter 2014, 10, 2479–2502. [Google Scholar] [CrossRef]
- Terada, E.; Samoshina, Y.; Nylander, T.; Lindman, B. Adsorption of cationic cellulose derivative/anionic surfactant complexes onto solid surfaces. II. Hydrophobized silica surfaces. Langmuir 2004, 20, 6692–6701. [Google Scholar] [CrossRef]
- Terada, E.; Samoshina, Y.; Nylander, T.; Lindman, B. Adsorption of cationic cellulose derivatives/anionic surfactant complexes onto solid surfaces. I. Silica surfaces. Langmuir 2004, 20, 1753–1762. [Google Scholar] [CrossRef]
- Guzmán, E.; San Miguel, V.; Peinado, C.; Ortega, F.; Rubio, R.G. Polyelectrolyte multilayers containing triblock copolymers of different charge ratio. Langmuir 2010, 26, 11494–11502. [Google Scholar] [CrossRef]
- Steitz, R.; Jaeger, W.; von Klitzing, R. Influence of charge density and ionic strength on the multilayer formation of strong polyelectrolytes. Langmuir 2001, 17, 4471–4474. [Google Scholar] [CrossRef]
- Schoeler, B.; Kumaraswamy, G.; Caruso, F. Investigation of the influence of polyelectrolyte charge density on the growth of multilayer thin films prepared by the layer-by-layer technique. Macromolecules 2002, 35, 889–897. [Google Scholar] [CrossRef]
- Zhang, X.; Sun, Y.; Gao, M.; Kong, X.; Shen, J. Effects of pH on the supramolecular structure of polymeric molecular deposition films. Macromol. Chem. Phys. 1996, 197, 509–515. [Google Scholar] [CrossRef]
- Saarinen, T.; Österberg, M.; Laine, J. Adsorption of polyelectrolyte multilayers and complexes on silica and cellulose surfaces studied by QCM-D. Colloids Surf. A 2008, 330, 134–142. [Google Scholar] [CrossRef]
- Schlenoff, J.B.; Dubas, S.T. Mechanism of polyelectrolyte multilayer growth: Charge overcompensation and distribution. Macromolecules 2001, 34, 592–598. [Google Scholar] [CrossRef]
- Hansupalak, N.; Santore, M.M. Sharp Polyelectrolyte adsorption cutoff induced by a monovalent salt. Langmuir 2003, 19, 7423–7426. [Google Scholar] [CrossRef]
- Guzmán, E.; Llamas, S.; Fernández-Peña, L.; Léonforte, F.; Baghdadli, N.; Cazeneuve, C.; Ortega, F.; Rubio, R.G.; Luengo, G.S. Effect of a natural amphoteric surfactant in the bulk and adsorption behavior of polyelectrolyte-surfactant mixtures. Colloids Surf. A 2020, 585, 124178. [Google Scholar] [CrossRef]
- Fernández-Peña, L.; Guzmán, E.; Leonforte, F.; Serrano-Pueyo, A.; Regulski, K.; Tournier-Couturier, L.; Ortega, F.; Rubio, R.G.; Luengo, G.S. Effect of molecular structure of eco-friendly glycolipid biosurfactants on the adsorption of hair-care conditioning polymers. Colloids Surf. B 2020, 185, 110578. [Google Scholar] [CrossRef]
- Nylander, T.; Samoshina, Y.; Lindman, B. Formation of polyelectrolyte–surfactant complexes on surfaces. Adv. Colloid Interface Sci. 2006, 123–126, 105–123. [Google Scholar] [CrossRef]
- von Klitzing, R. Internal structure of polyelectrolyte multilayer assemblies. Phys. Chem. Chem. Phys. 2006, 8, 5012–5033. [Google Scholar] [CrossRef]
- L’Haridon, J.; Martz, P.; Chenéble, J.C.; Campion, J.F.; Colombe, L. Ecodesign of cosmetic formulae: Methodology and application. Int. J. Cosmetic Sci. 2018, 40, 165–177. [Google Scholar] [CrossRef] [PubMed]
- Dobrynin, A.; Rubinstein, M. Theory of polyelectrolytes in solutions and at surfaces. Prog. Polym. Sci. 2005, 30, 1049–1118. [Google Scholar] [CrossRef]
- Samoshina, Y.; Nylander, T.; Claesson, P.; Schillén, K.; Iliopoulos, I.; Lindman, B. Adsorption and aggregation of cationic amphiphilic polyelectrolytes on silica. Langmuir 2005, 21, 2855–2864. [Google Scholar] [CrossRef] [PubMed]
- Cahill, B.P.; Papastavrou, G.; Koper, G.J.M.; Borkovec, M. Adsorption of poly(amido amine) (PAMAM) dendrimers on silica: importance of electrostatic three-body attraction. Langmuir 2008, 24, 465–473. [Google Scholar] [CrossRef]
- Voinova, M.V.; Rodahl, M.; Jonson, M.; Kasemo, B. Viscoelastic acoustic response of layered polymer films at fluid-solid interfaces: Continuum mechanics approach. Phys. Scr. 1999, 59, 391–396. [Google Scholar] [CrossRef]
- Azzam, R.M.A.; Bashara, N.M. Ellipsometry and Polarized Light; North-Holland Pub. Co.: Amsterdam, The Netherlands, 1977. [Google Scholar]
- Nestler, P.; Helm, C.A. Determination of refractive index and layer thickness of nm-thin films via ellipsometry. Opt. Expr. 2017, 25, 27077–27085. [Google Scholar] [CrossRef]
- Palik, E.D. Handbook of Optical Constant of Solid; Academic Press, Inc.: New York, NY, USA, 1985. [Google Scholar]
- Vörös, J. The density and refractive index of adsorbing protein layers. Biophys. J. 2004, 87, 553–561. [Google Scholar] [CrossRef]
- Halthur, T.J.; Elofsson, U. Multilayers of charged polypeptides as studied by in situ ellipsometry and quartz crystal microbalance with dissipation. Langmuir 2004, 20, 1739–1745. [Google Scholar] [CrossRef]
- Banerjee, S.; Cazeneuve, C.; Baghdadli, N.; Ringeissen, S.; Leermakers, F.A.M.; Luengo, G.S. Surfactant-polymer interactions: Molecular architecture does matter. Soft Matter. 2015, 11, 2504–2511. [Google Scholar] [CrossRef]
- Banerjee, S.; Cazeneuve, C.; Baghdadli, N.; Ringeissen, S.; Lénforte, F.; Leermakers, F.A.M.; Luengo, G.S. Modeling of polyelectrolyte adsorption from micellar solutions onto biomimetic substrates. J. Phys. Chem. B 2017, 121, 8638–8651. [Google Scholar] [CrossRef]
- Scheujtens, J.M.H.M.; Fleer, G.J. Statistical theory of the adsorption of interacting chain molecules. 1. Partition function, segment density distribution, and adsorption isotherms. J. Phys. Chem. 1979, 83, 1619–1635. [Google Scholar] [CrossRef]
- Scheutjens, J.M.H.M.; Fleer, G.J. Statistical theory of the adsorption of interacting chain molecules. 2. Train, loop, and tail size distribution. J. Phys. Chem. 1980, 84, 178–190. [Google Scholar] [CrossRef]
- Fleer, G.; Cohen-Stuart, M.A.; Scheutjens, J.; Crosgrove, T.; Vincent, B. Polymer at Interfaces; Chapman and Hall: London, UK, 1993. [Google Scholar]
- Leermakers, F.A.M.; Scheutjens, J.M.H.M. Statistical thermodynamics of association colloids: V. critical micelle concentration, micellar size and shape. J. Colloid Interface Sci. 1990, 136, 231–241. [Google Scholar] [CrossRef]
- Weininger, D. SMILES, a chemical language and information system. 1. Introduction to methodology and encoding rules. J. Chem. Inf. Comput. Sci. 1988, 28, 31–36. [Google Scholar] [CrossRef]
- Weininger, D.; Weininger, A.; Weininger, J.L. SMILES. 2. Algorithm for generation of unique SMILES notation. J. Chem. Inf. Comput. Sci. 1989, 29, 97–101. [Google Scholar] [CrossRef]
- Postmus, B.R.; Leermakers, F.A.M.; Stuart, M.A.C. Self-consistent field modeling of non-ionic surfactants at the silica−water interface: Incorporating molecular detail. Langmuir 2008, 24, 3960–3969. [Google Scholar] [CrossRef]
- Holmberg, K.; Jönsson, B.; Kronberg, B.; Lindman, B. Surfactants and Polymers in Aqueous Solution; John Wiley & Sons: Chichester, UK, 2002. [Google Scholar]
- Dobrynin, A.V.; Rubinstein, M. Effect of short-range interactions on polyelectrolyte adsorption at charged surfaces†. J. Phys. Chem. B 2003, 107, 8260–8269. [Google Scholar] [CrossRef]
- Adamczyk, Z.; Zembala, M.; Michna, A. Polyelectrolyte adsorption layers studied by streaming potential and particle deposition. J. Colloid Interface Sci. 2006, 303, 353–364. [Google Scholar] [CrossRef]
- Khan, N.; Brettmann, B. Intermolecular interactions in polyelectrolyte and surfactant complexes in solution. Polymers 2018, 11, 51. [Google Scholar] [CrossRef]
- Svensson, A.; Piculell, L.; Cabane, B.; Ilekti, P. A new approach to the phase behavior of oppositely charged polymers and surfactants. J. Phys. Chem. B 2002, 106, 1013–1018. [Google Scholar] [CrossRef]
- Varga, I.; Campbell, R.A. General physical description of the behavior of oppositely charged polyelectrolyte/surfactant mixtures at the air/water interface. Langmuir 2017, 33, 5915–5924. [Google Scholar] [CrossRef] [PubMed]
- Guzmán, E.; Fernández-Peña, L.; Ortega, F.; Rubio, R.G. Equilibrium and kinetically trapped aggregates in polyelectrolyte–oppositely charged surfactant mixtures. Curr. Opin. Colloid Interface Sci. 2020, 48, 91–108. [Google Scholar] [CrossRef]
- Llamas, S.; Guzmán, E.; Baghdadli, N.; Ortega, F.; Cazeneuve, C.; Rubio, R.G.; Luengo, G.S. Adsorption of poly(diallyldimethylammonium chloride)—sodium methyl-cocoyl-taurate complexes onto solid surfaces. Colloids Surf. A 2016, 505, 150–157. [Google Scholar] [CrossRef]
- Svensson, A.V.; Huang, L.; Johnson, E.S.; Nylander, T.; Piculell, L. Surface deposition and phase behavior of oppositely charged polyion/surfactant ion complexes. 1. Cationic guar versus cationic hydroxyethylcellulose in mixtures with anionic surfactants. ACS Appl. Mat. Interfaces 2009, 1, 2431–2442. [Google Scholar] [CrossRef]
- Clauzel, M.; Johnson, E.S.; Nylander, T.; Panandiker, R.K.; Sivik, M.R.; Piculell, L. Surface deposition and phase behavior of oppositely charged polyion–surfactant ion complexes. Delivery of silicone oil emulsions to hydrophobic and hydrophilic surfaces. ACS Appl. Mat. Interfaces 2011, 3, 2451–2462. [Google Scholar] [CrossRef]
- Santos, O.; Johnson, E.S.; Nylander, T.; Panandiker, R.K.; Sivik, M.R.; Piculell, L. Surface adsorption and phase separation of oppositely charged polyion−surfactant ion complexes: 3. Effects of polyion hydrophobicity. Langmuir 2010, 26, 9357–9367. [Google Scholar] [CrossRef]
- Llamas, S.; Fernández-Peña, L.; Akanno, A.; Guzmán, E.; Ortega, V.; Ortega, F.; Csaky, A.G.; Campbell, R.A.; Rubio, R.G. Towards understanding the behavior of polyelectrolyte–surfactant mixtures at the water/vapor interface closer to technologically-relevant conditions. Phys. Chem. Chem. Phys. 2018, 20, 1395–1407. [Google Scholar] [CrossRef]






| χ | w | G | C | O | S | N | Na | Cl | OH | Z | X | Si | ε | ν |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| w | 0 | 0 | 1.6 | −0.6 | 0 | 0.5 | 0 | 0 | −0.5 | 0.5 | 0 | 2 | 80 | 0 |
| G | 0 | 0 | 0.6 | −0.6 | 0 | 0.5 | 0 | 0 | 0.8 | 1 | 1 | 0 | 80 | 0 |
| C | 1.6 | 0.6 | 0 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 0 | 2 | 0 |
| O | −0.6 | −0.6 | 1.6 | 0 | 2 | 0 | 0 | 0 | −0.5 | 0 | 0 | 0 | 1.5 | 0 |
| S | 0 | 0 | 2 | 2 | 0 | 0 | 0 | 0 | −0.25 | 0 | 0 | 0 | 3.4 | −0.2 |
| N | 0.5 | 0.5 | 2 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 7 | 0.2 |
| Na | 0 | 0 | 2 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 6.1 | 1 |
| Cl | 0 | 0 | 2 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 6.1 | −1 |
| OH | −0.5 | 0.8 | 2 | −0.5 | −0.25 | 1 | 0 | 0 | 0 | 1 | 1 | 0 | 3.8 | 0 |
| Z | 0.5 | 1 | 2 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 7 | 0.12 |
| X | 0 | 1 | 2 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 1.5 | [cs]-dpdt |
| Si | 2 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 7 | −0.1 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hernández-Rivas, M.; Guzmán, E.; Fernández-Peña, L.; Akanno, A.; Greaves, A.; Léonforte, F.; Ortega, F.; G. Rubio, R.; Luengo, G.S. Deposition of Synthetic and Bio-Based Polycations onto Negatively Charged Solid Surfaces: Effect of the Polymer Cationicity, Ionic Strength, and the Addition of an Anionic Surfactant. Colloids Interfaces 2020, 4, 33. https://doi.org/10.3390/colloids4030033
Hernández-Rivas M, Guzmán E, Fernández-Peña L, Akanno A, Greaves A, Léonforte F, Ortega F, G. Rubio R, Luengo GS. Deposition of Synthetic and Bio-Based Polycations onto Negatively Charged Solid Surfaces: Effect of the Polymer Cationicity, Ionic Strength, and the Addition of an Anionic Surfactant. Colloids and Interfaces. 2020; 4(3):33. https://doi.org/10.3390/colloids4030033
Chicago/Turabian StyleHernández-Rivas, María, Eduardo Guzmán, Laura Fernández-Peña, Andrew Akanno, Andrew Greaves, Fabien Léonforte, Francisco Ortega, Ramón G. Rubio, and Gustavo S. Luengo. 2020. "Deposition of Synthetic and Bio-Based Polycations onto Negatively Charged Solid Surfaces: Effect of the Polymer Cationicity, Ionic Strength, and the Addition of an Anionic Surfactant" Colloids and Interfaces 4, no. 3: 33. https://doi.org/10.3390/colloids4030033
APA StyleHernández-Rivas, M., Guzmán, E., Fernández-Peña, L., Akanno, A., Greaves, A., Léonforte, F., Ortega, F., G. Rubio, R., & Luengo, G. S. (2020). Deposition of Synthetic and Bio-Based Polycations onto Negatively Charged Solid Surfaces: Effect of the Polymer Cationicity, Ionic Strength, and the Addition of an Anionic Surfactant. Colloids and Interfaces, 4(3), 33. https://doi.org/10.3390/colloids4030033










