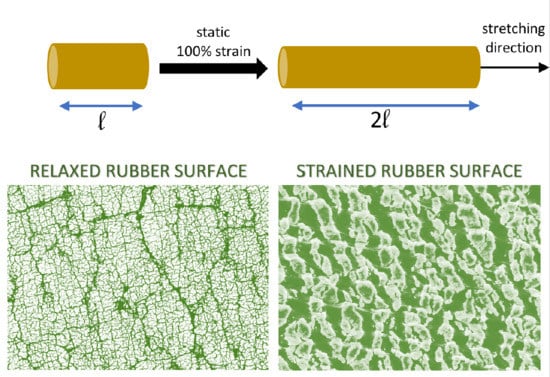
Rubber Surface Change and Static Charging under Periodic Stress
Abstract
1. Introduction
2. Materials and Methods
Characterization of Natural Rubber Surfaces
3. Results
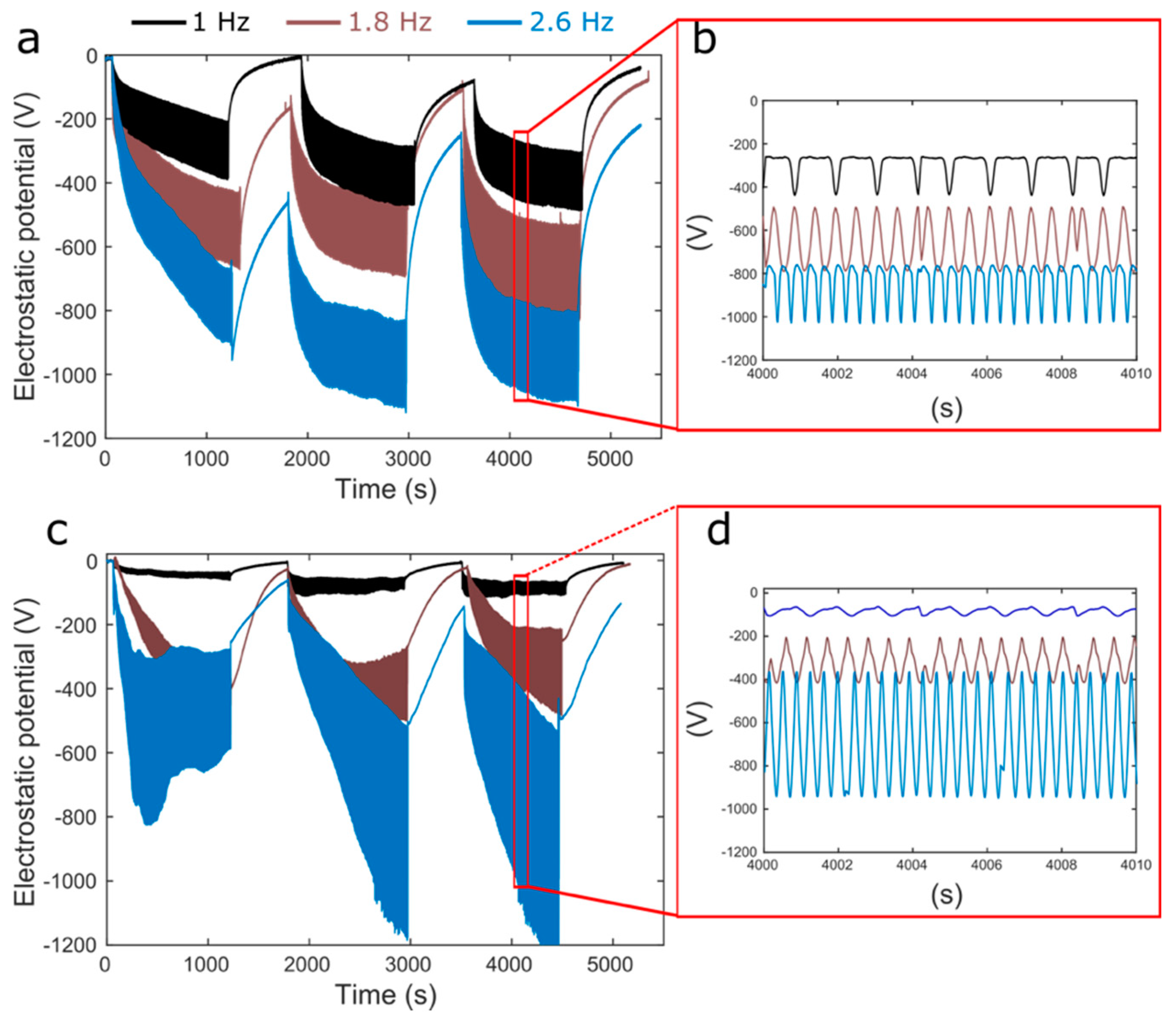
3.1. Electrostatic Potential Measurements during Rubber Stretching
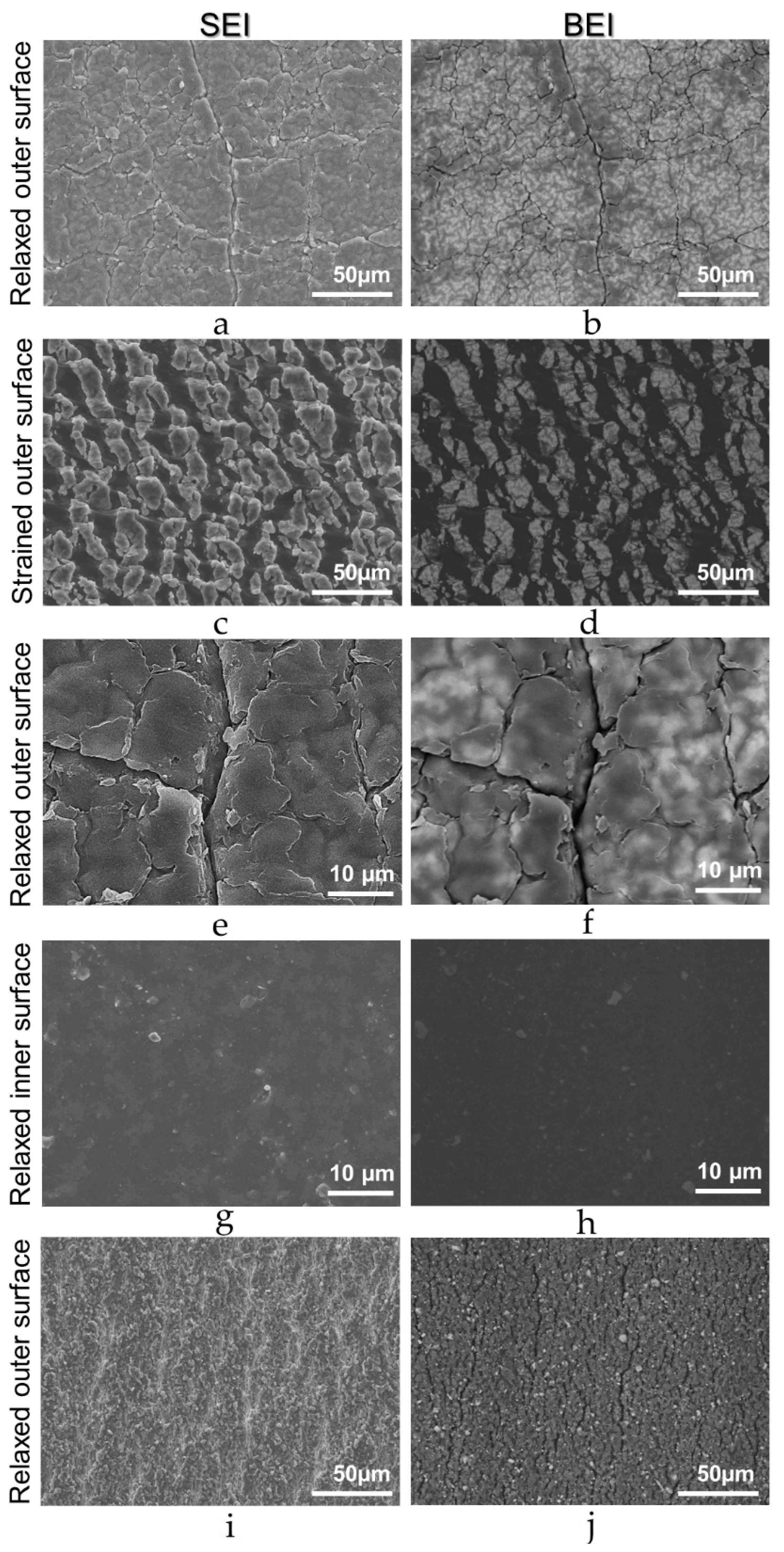
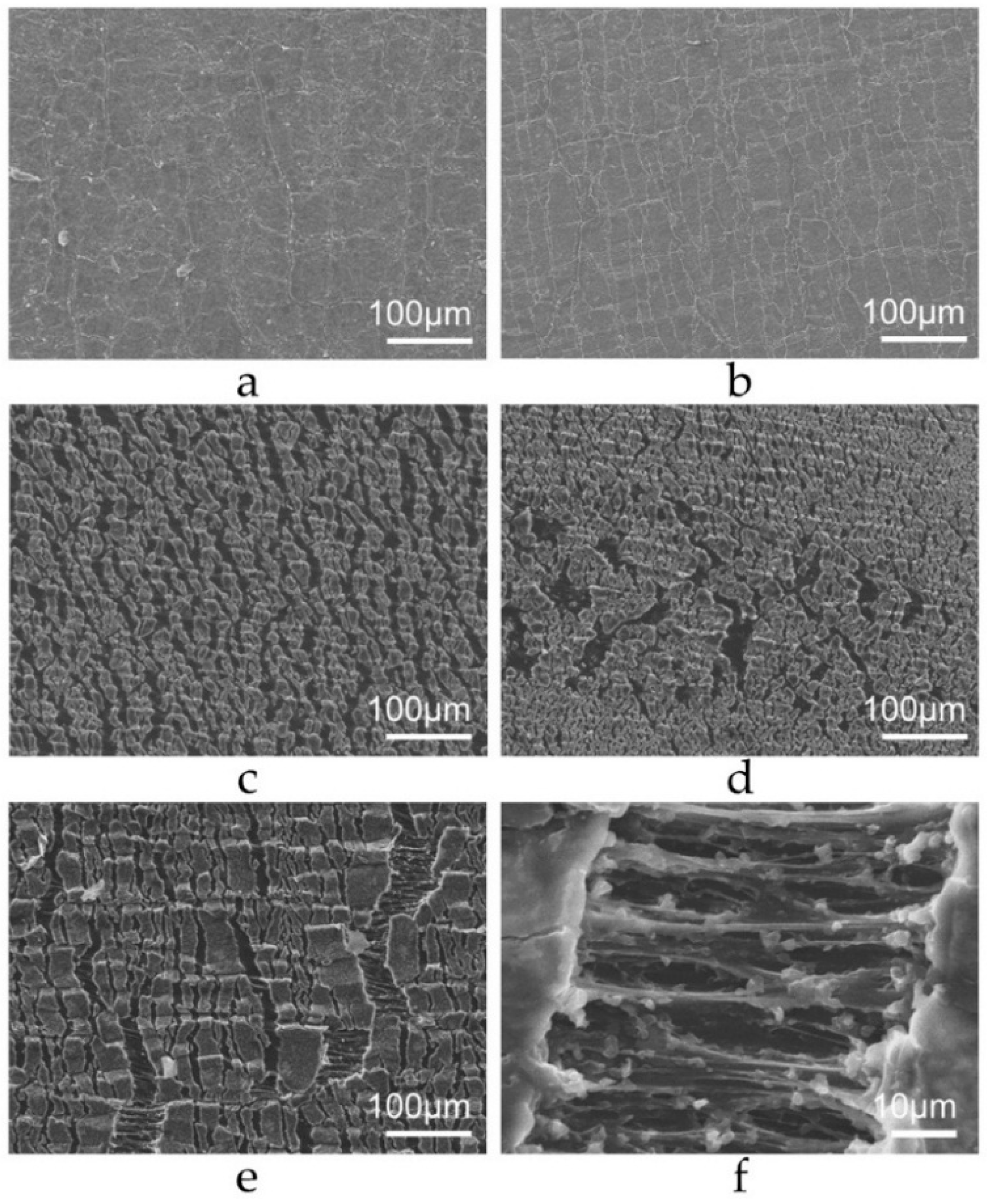
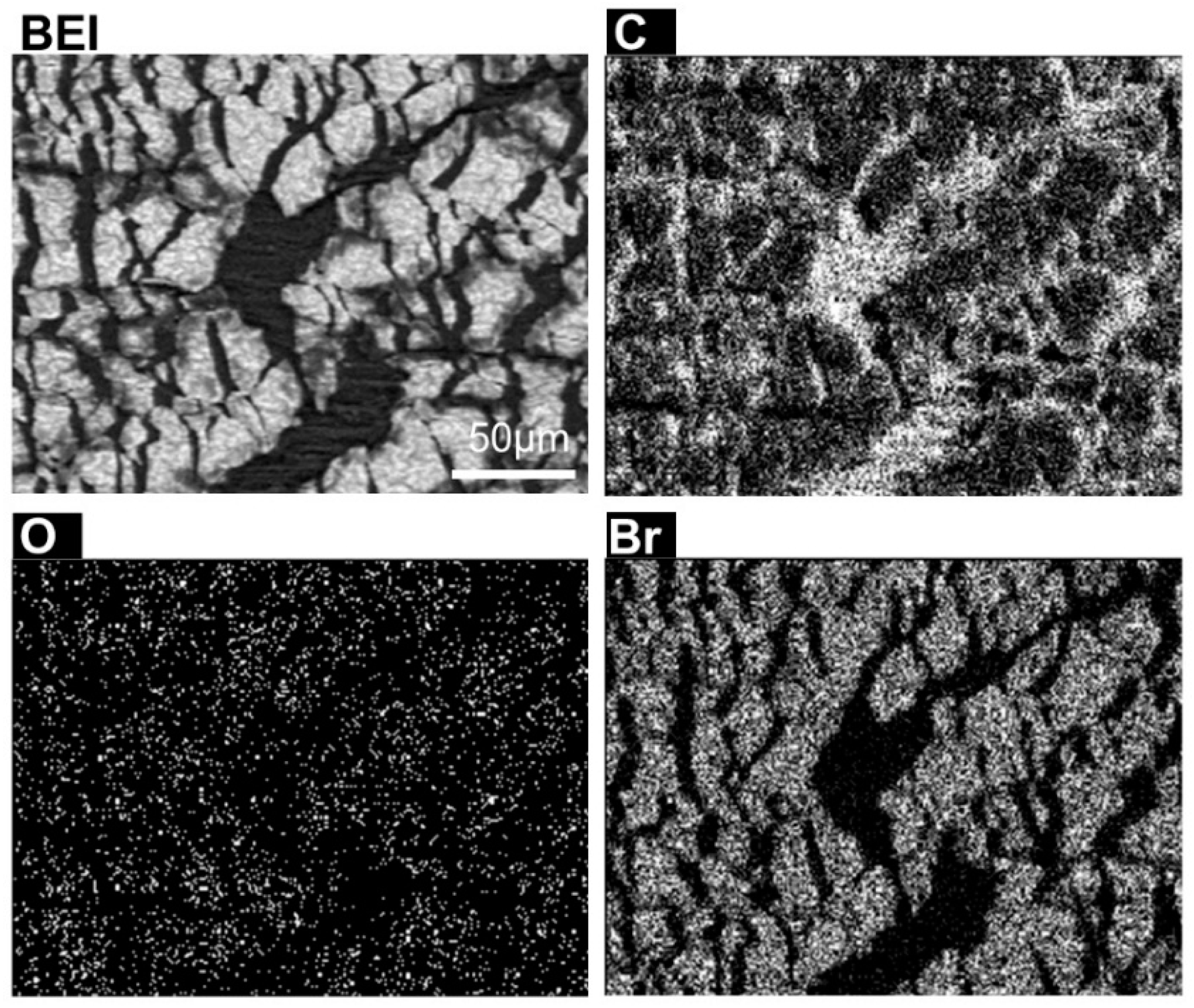
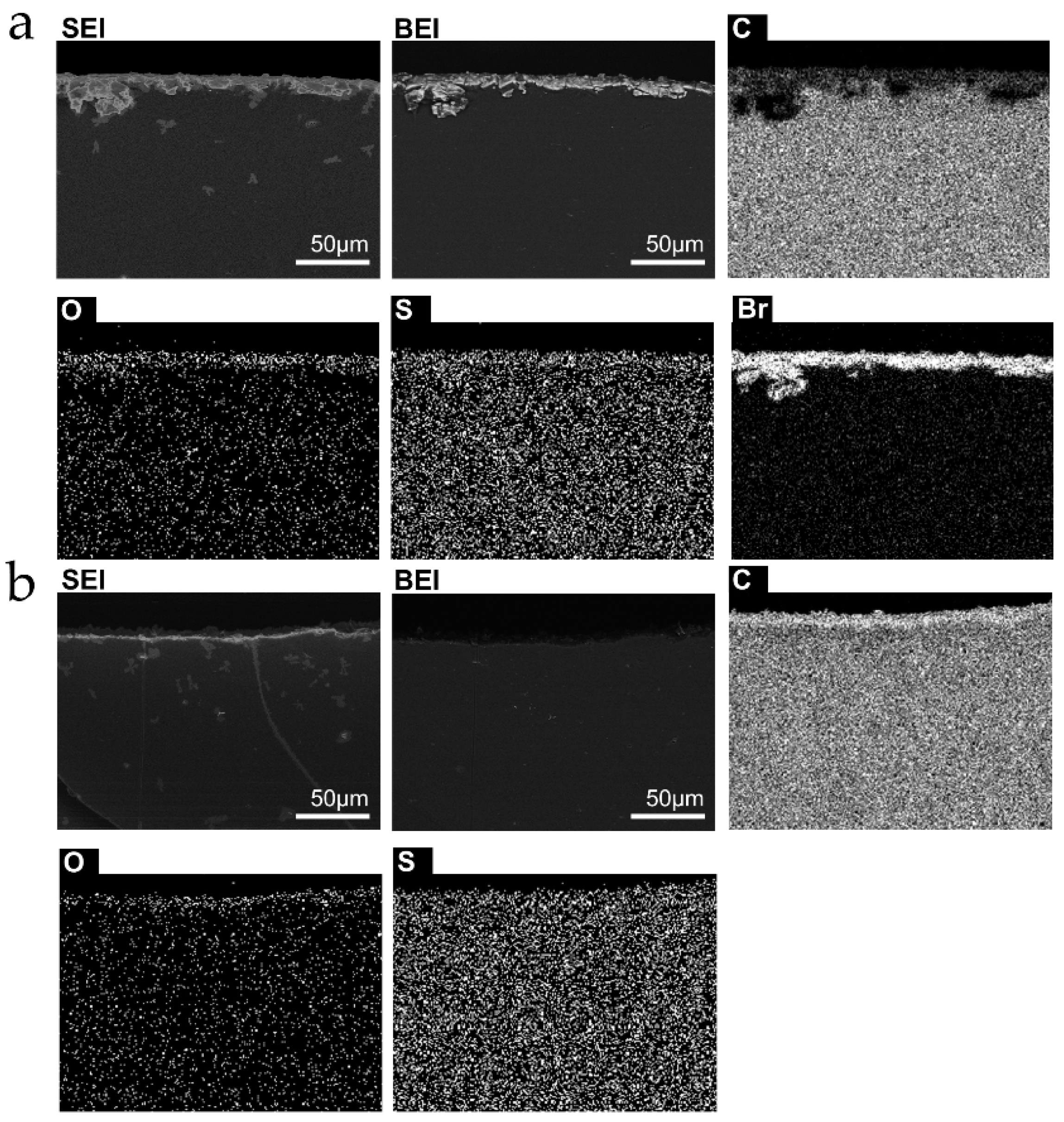
3.2. SEM Imaging of Natural Rubber Surfaces
3.2.1. Rubber Surface Heterogeneity
3.2.2. Rubber Surface Microanalysis
3.2.3. Chemical Differences between Rubber Surface and Bulk
3.2.4. Weight Gain in Stretched Rubber
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Fu, Y.; Dorfmann, L.; Xie, Y. Localized necking of a dielectric membrane. Extrem. Mech. Lett. 2018, 21, 44–48. [Google Scholar] [CrossRef]
- An, L.; Wang, F.; Cheng, S.; Lu, T.; Wang, T.J. Experimental investigation of the electromechanical phase transition in a dielectric elastomer tube. Smart Mater. Struct. 2015, 24, 035006. [Google Scholar] [CrossRef]
- Jiménez, S.M.A.; McMeeking, R.M. A constitutive law for dielectric elastomers subject to high levels of stretch during combined electrostatic and mechanical loading: Elastomer stiffening and deformation dependent dielectric permittivity. Int. J. Non-Linear Mech. 2016, 87, 125–136. [Google Scholar] [CrossRef]
- Dorfmann, L.; Ogden, R.W. Nonlinear electroelasticity: Material properties, continuum theory and applications. Proc. Math. Phys. Eng. Sci. 2017, 473, 20170311. [Google Scholar] [CrossRef] [PubMed]
- Berselli, G.; Mammano, G.S.; Dragoni, E. Design of a dielectric elastomer cylindrical actuator with quasi-constant available thrust: Modeling procedure and experimental validation. J. Mech. Des. 2014, 136, 125001. [Google Scholar] [CrossRef]
- Cao, J.; Liang, W.; Zhu, J.; Ren, Q. Control of a muscle-like soft actuator via a bioinspired approach. Bioinspir. Biomim. 2018, 13, 066005. [Google Scholar] [CrossRef] [PubMed]
- Gisby, T.A.; O’Brien, B.M.; Anderson, I.A. Self sensing feedback for dielectric elastomer actuators. Appl. Phys. Lett. 2013, 102, 193703. [Google Scholar] [CrossRef]
- Guo, J.; Xiang, C.; Rossiter, J. A soft and shape-adaptive electroadhesive composite gripper with proprioceptive and exteroceptive capabilities. Mater. Des. 2018, 156, 586–587. [Google Scholar] [CrossRef]
- Mocellini, R.R.; Bonifacich, F.G.; Lambri, F.D.; Lambri, M.A.; Zelada, G.I.; Lambri, O.A. Electric memory effects in styrene-butadiene rubber, containing electric inclusions of highly aromatic oil. J. Adv. Dielectr. 2018, 8, 1850018. [Google Scholar] [CrossRef]
- Alameh, Z.; Yang, S.; Deng, Q.; Sharma, P. Emergent magnetoelectricity in soft materials, instability, and wireless energy harvesting. Soft Matter 2018, 14, 5856–5868. [Google Scholar] [CrossRef] [PubMed]
- Dong, L.; Grissom, M.D.; Safwat, T.; Prasad, M.G.; Fisher, F.T. Resonant frequency tuning of electroactive polymer membranes via an applied bias voltage. Smart Mater. Struct. 2018, 27, 114005. [Google Scholar] [CrossRef]
- Jin, X.; Huang, Z. Random response of dielectric elastomer balloon to electrical or mechanical perturbation. J. Intell. Mater. Syst. Struct. 2016, 28, 195–203. [Google Scholar] [CrossRef]
- Helal, A.; Doumit, M.; Shaheen, R. Biaxial experimental and analytical characterization of a dielectric elastomer. Appl. Phys. A Mater. Sci. Process. 2018, 124, 2. [Google Scholar] [CrossRef]
- Burgo, T.A.L.; Batista, B.C.; Galembeck, F. Electricity on rubber surfaces: A new energy conversion effect. ACS Omega 2017, 2, 8940–8947. [Google Scholar] [CrossRef]
- Galembeck, F.; Burgo, T.A.L. Chemical Electrostatics; Springer International Publishing AG: Cham, Switzerland, 2017; ISBN 978-3-319-52374-3. [Google Scholar]
- Schein, L.B. Recent progress and continuing puzzles in electrostatics. Science 2007, 316, 1572–1573. [Google Scholar] [CrossRef] [PubMed]
- Castle, G.S.P. Contact charging between insulators. J. Electrostat. 1987, 40–41, 13–20. [Google Scholar] [CrossRef]
- Bailey, A.G. The charging of insulator surfaces. J. Electrostat. 2001, 51–52, 82–90. [Google Scholar] [CrossRef]
- Harb, A. Energy harvesting: State-of-the-art. Renew. Energy 2011, 36, 2641–2654. [Google Scholar] [CrossRef]
- Mitcheson, P.D.; Yeatman, E.M.; Rao, G.K.; Holmes, A.S.; Green, T.C. Energy harvesting from human and machine motion for wireless electronic devices. Proc. IEEE 2008, 96, 1457–1486. [Google Scholar] [CrossRef]
- Zhu, G.; Peng, B.; Chen, J.; Jing, Q.; Wang, Z.L. Triboelectric nanogenerators as a new energy technology: From fundamentals, devices, to applications. Nano Energy 2015, 14, 126–138. [Google Scholar] [CrossRef]
- Sow, M.; Widenor, R.; Kumar, A.; Lee, S.W.; Lacks, D.J.; Sankaran, R.M. Strain-induced reversal of charge transfer in contact electrification. Angew. Chem. Int. Ed. 2012, 51, 2695–2697. [Google Scholar] [CrossRef] [PubMed]
- Dogadkin, B.A.; Gul, V.E.; Morozova, N.A. The effect of electric charges formed during repeated deformations on the fatigue resistance of vulcanizates. Rubber Chem. Technol. 1960, 33, 970. [Google Scholar] [CrossRef]
- Mars, W.V.; Fatemi, A. Factors that affect the fatigue life of rubber: A literature survey. Rubber Chem. Technol. 2004, 77, 391–412. [Google Scholar] [CrossRef]
- Lake, G.J. Fatigue and fracture of elastomers. Rubber Chem. Technol. 1995, 68, 435–460. [Google Scholar] [CrossRef]
- Zhao, J.; Ghebremeskel, G.N. A review of some of the factors affecting fracture and fatigue in SBR and BR vulcanizates. Rubber Chem. Technol. 2001, 74, 409–427. [Google Scholar] [CrossRef]
- Mars, W.V.; Fatemi, A. A literature survey on fatigue analysis approaches for rubber. Int. J. Fatigue 2002, 24, 949–961. [Google Scholar] [CrossRef]
- Tee, Y.L.; Loo, M.S.; Andriyana, A. Recent advances on fatigue of rubber after the literature survey by Mars and Fatemi in 2002 and 2004. Int. J. Fatigue 2018, 110, 115–129. [Google Scholar] [CrossRef]
- Torregrosa-Coque, R.; Álvarez-García, S.; Martín-Martinez, J.M. Migration of Paraffin Wax to Sulfur Vulcanized Styrene–Butadiene Rubber (SBR) Surface: Effect of Temperature. J. Adhes. Sci. Technol. 2012, 26, 813–826. [Google Scholar] [CrossRef]
- Moyano, M.A.; Martín-Martínez, J.M. Surface treatment with UV-ozone to improve adhesion of vulcanized rubber formulated with an excess of processing oil. Int. J. Adhes. Adhes. 2014, 55, 106–113. [Google Scholar] [CrossRef]
- Costa, R.A.; Coltro, L.; Galembeck, F. A Staining Procedure for the Detection of Oxidized Sites in Polyolefins. Angew. Makromol. Chem. 1990, 180, 85–94. [Google Scholar] [CrossRef]
- Nunes, S.P.; Costa, R.A.; Barbosa, S.P.; Almeida, G.R.; Galembeck, F. Tracking Degradation and Pyrolysis of EPDM Insulators. IEEE Trans. Dielectr. Electr. Insul. 1989, 24, 99–105. [Google Scholar] [CrossRef]
- Baszkin, A.; Ter-Minassian-Saraga, L. Chemical structures of surface-oxidized and grafted polyethylene: Adsorption and wetting studies. J. Polym. Sci. Part C Polym. Symp. 1971, 34, 243–252. [Google Scholar] [CrossRef]
- Le Cam, J.-B.; Huneau, B.; Verron, E.; Gornet, L. Mechanism of fatigue crack growth in carbon black filled natural rubber. Macromolecules 2004, 37, 5011–5017. [Google Scholar] [CrossRef]
- Le Cam, J.-B.; Toussaint, E. The mechanism of fatigue crack growth in rubbers under severe loading: The effect of stress-induced crystallization. Macromolecules 2010, 43, 4708–4714. [Google Scholar] [CrossRef]
- Munoz, L.; Vanel, L.; Sanseau, O.; Sotta, P.; Long, D.; Odoni, L.; Guy, L. Fatigue crack growth dynamics in filled natural rubber. Plast. Rubber Compos. 2012, 41, 273–276. [Google Scholar] [CrossRef]
- Griffith, A.A. VI. The phenomena of rupture and flow in solids. Philos. Trans. R. Soc. 1920, 221, 163–198. [Google Scholar] [CrossRef]
- Zhou, W.; Li, X.; Lu, J.; Huang, N.; Chen, L.; Qi, Z.; Li, L.; Liang, H. Toughening mystery of natural rubber deciphered by double network incorporating hierarchical structures. Sci. Rep. 2014, 4, 7502. [Google Scholar] [CrossRef] [PubMed]
- Samaca Martinez, J.R.; Balandraud, X.; Toussaint, E.; Le Cam, J.-B.; Berghezan, D. Thermomechanical analysis of the crack tip zone in stretched crystallizable natural rubber by using infrared thermography and digital image correlation. Polymer 2014, 55, 6345–6353. [Google Scholar] [CrossRef]
- Trabelsi, S.; Albouy, P.-A.; Rault, J. Stress-induced crystallization around a crack tip in natural rubber. Macromolecules 2002, 35, 10054–10061. [Google Scholar] [CrossRef]
- Ciullo, P.A.; Hewitt, N. The Rubber Formulary; Noyes Publications/William Andrew Publishing: New York, NY, USA, 1999; pp. 4–49. ISBN 9780815519294. [Google Scholar]
- Rippel, M.M.; Lee, L.-T.; Leite, C.A.P.; Galembeck, F. Skim and cream natural rubber particles: Colloidal properties, coalescence and film formation. J. Colloid Interface Sci. 2003, 268, 330–340. [Google Scholar] [CrossRef] [PubMed]
- Roberts, A.D.; Brackley, C.A. Surface treatment of rubber to reduce friction. J. Nat. Rubber Res. 1989, 4, 1–21. [Google Scholar]
- Burgo, T.A.L.; Rezende, C.A.; Bertazzo, S.; Galembeck, A.; Galembeck, F. Electric potential decay on polyethylene: Role of atmospheric water on electric charge build-up and dissipation. J. Electrost. 2011, 69, 401–409. [Google Scholar] [CrossRef]
- Burgo, T.A.L.; Ducati, T.R.D.; Francisco, K.R.; Clinckspoor, K.J.; Galembeck, F.; Galembeck, S.E. Triboelectricity: Macroscopic charge patterns formed by self-arraying ions on polymer surfaces. Langmuir 2012, 28, 7407–7416. [Google Scholar] [CrossRef] [PubMed]
- Balestrin, L.B.S.; Duque, D.D.; Silva, D.S.; Galembeck, F. Triboelectricity in insulating polymers: Evidence for a mechanochemical mechanism. Faraday Discuss. 2014, 170, 369–383. [Google Scholar] [CrossRef] [PubMed]
- Bortot, E. Nonlinear dynamic response of soft thick-walled electro-active tubes. Smart Mater. Struct. 2018, 27, 105025. [Google Scholar] [CrossRef]


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Santos, L.P.; Campo, Y.A.S.; Da Silva, D.S.; Burgo, T.A.L.; Galembeck, F. Rubber Surface Change and Static Charging under Periodic Stress. Colloids Interfaces 2018, 2, 55. https://doi.org/10.3390/colloids2040055
Santos LP, Campo YAS, Da Silva DS, Burgo TAL, Galembeck F. Rubber Surface Change and Static Charging under Periodic Stress. Colloids and Interfaces. 2018; 2(4):55. https://doi.org/10.3390/colloids2040055
Chicago/Turabian StyleSantos, Leandra P., Yan A. S. Campo, Douglas S. Da Silva, Thiago A. L. Burgo, and Fernando Galembeck. 2018. "Rubber Surface Change and Static Charging under Periodic Stress" Colloids and Interfaces 2, no. 4: 55. https://doi.org/10.3390/colloids2040055
APA StyleSantos, L. P., Campo, Y. A. S., Da Silva, D. S., Burgo, T. A. L., & Galembeck, F. (2018). Rubber Surface Change and Static Charging under Periodic Stress. Colloids and Interfaces, 2(4), 55. https://doi.org/10.3390/colloids2040055