Dynamic Analysis of Functionally Graded Porous (FGP) Elliptic Cylindrical Shell Based on Jacobi Polynomials Ritz Method
Abstract
:1. Introduction
2. Theoretical Formulations
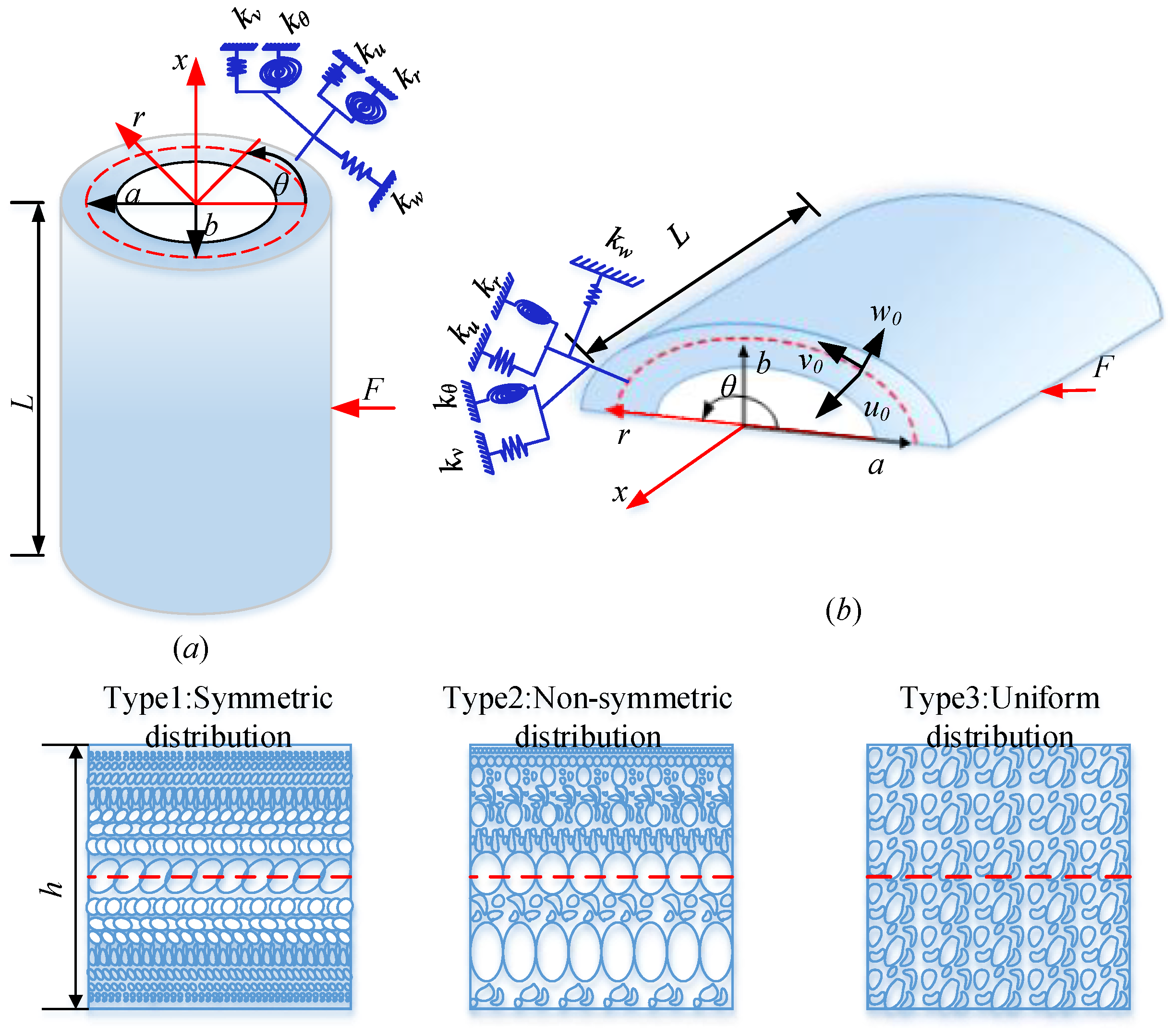
2.1. FGP Elliptic Cylindrical Shell and Panel Model
2.2. Energy Expression
2.3. Admissible Displacements and Solving Process
3. Numerical Results and Discussion
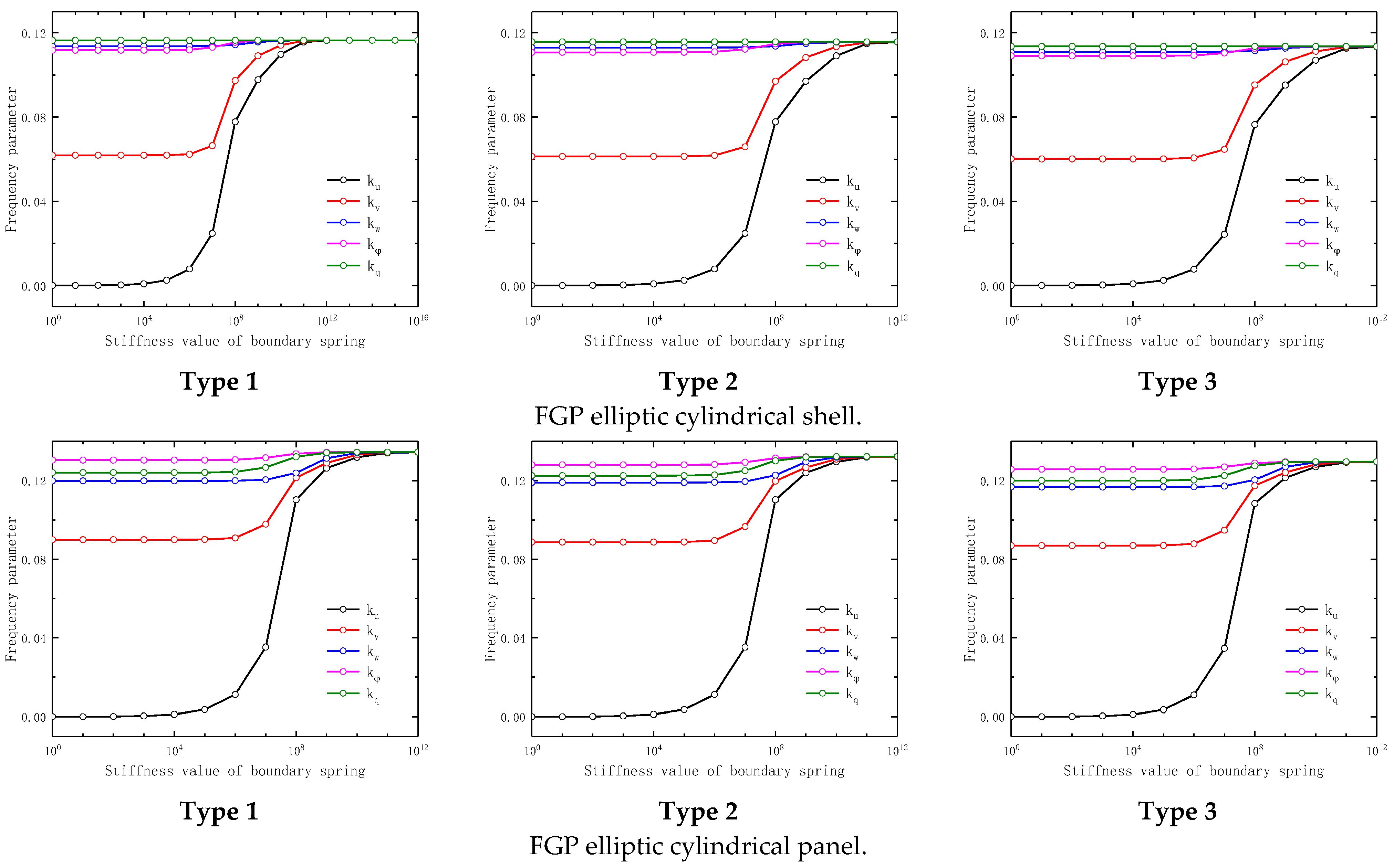
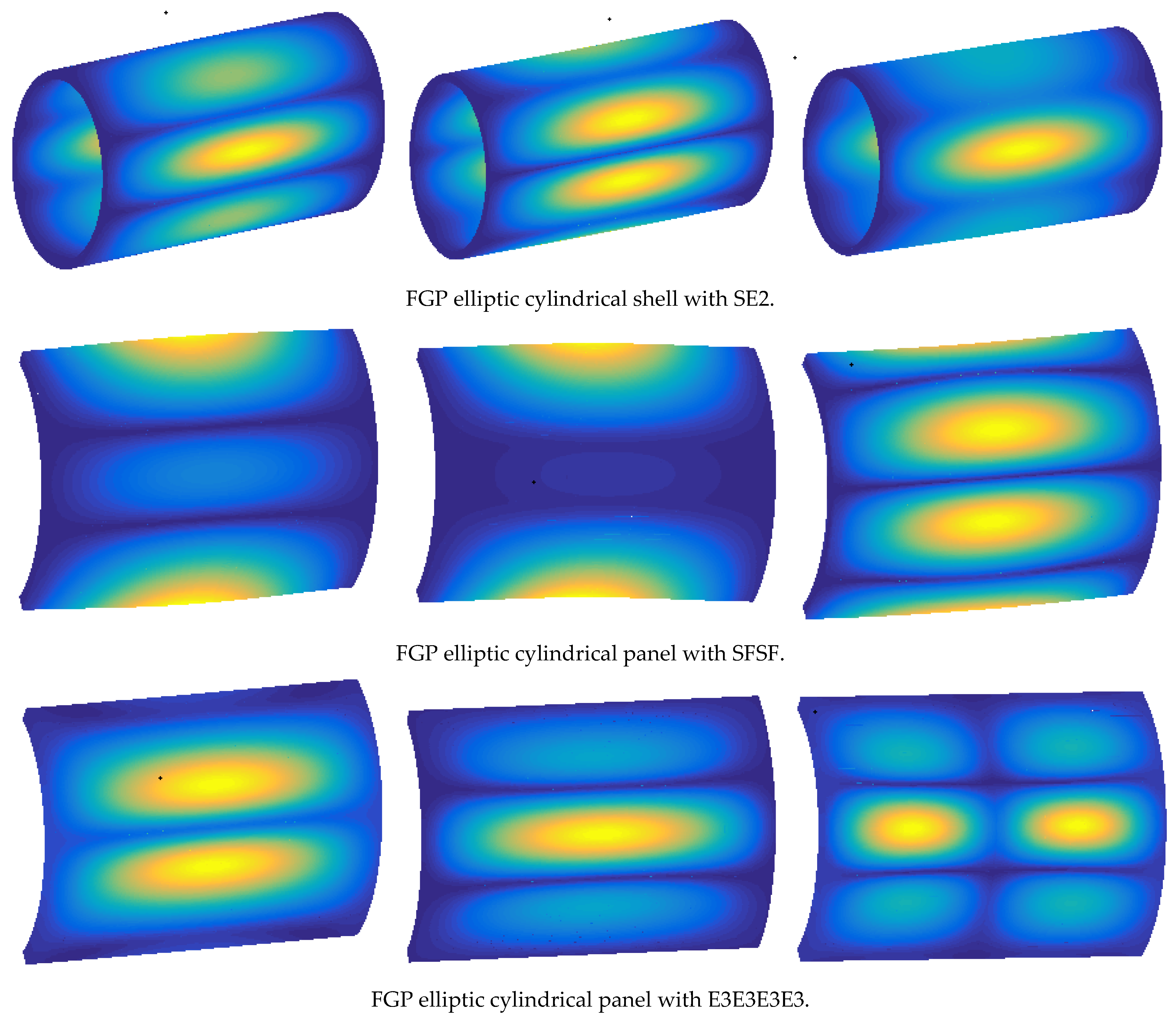
3.1. Dynamic Characteristics of FGP Elliptic Cylindrical Shell under Free Vibration
3.2. Dynamic Characteristics of FGP Elliptic Cylindrical Shell under Forced Vibration
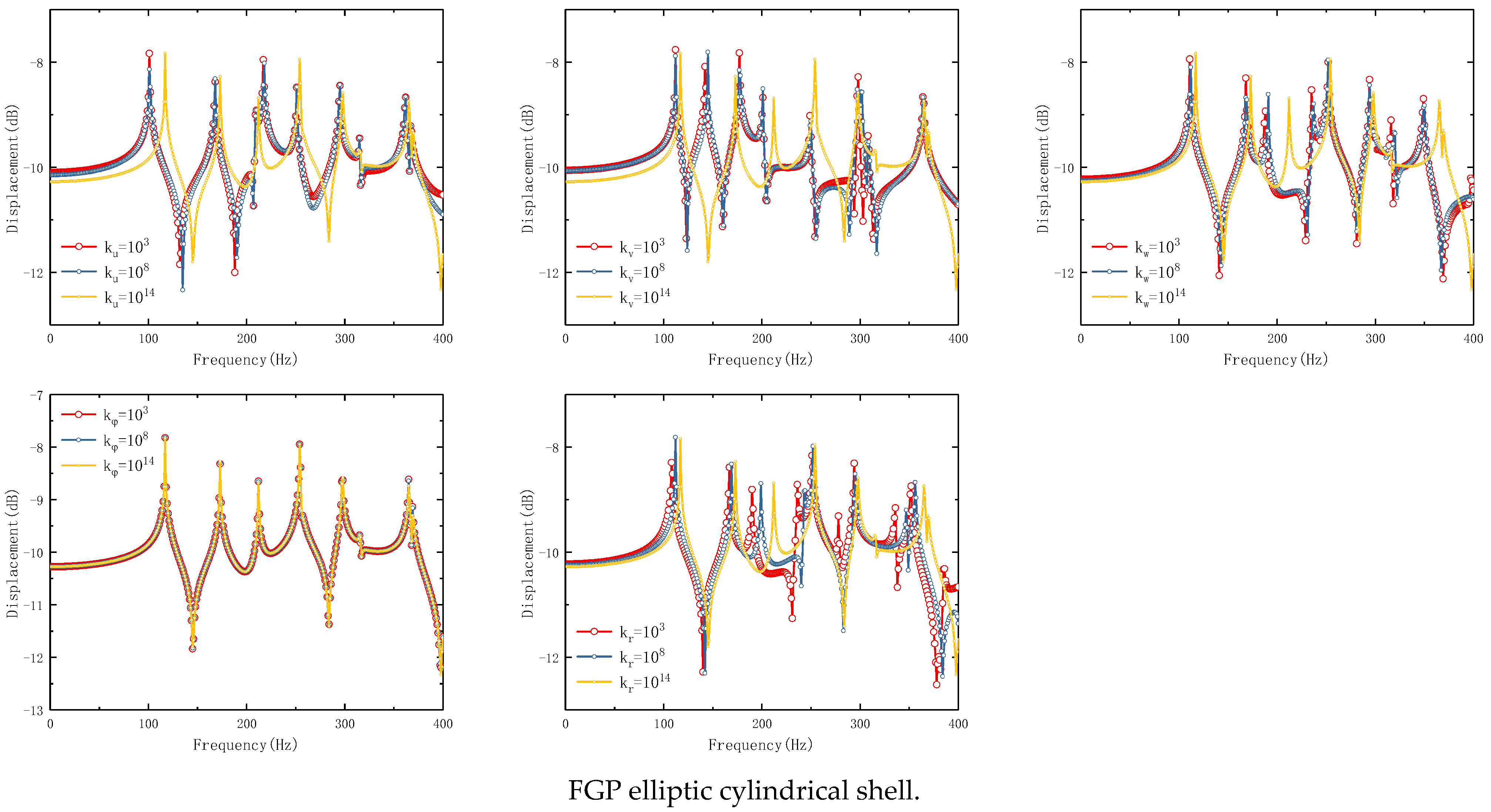
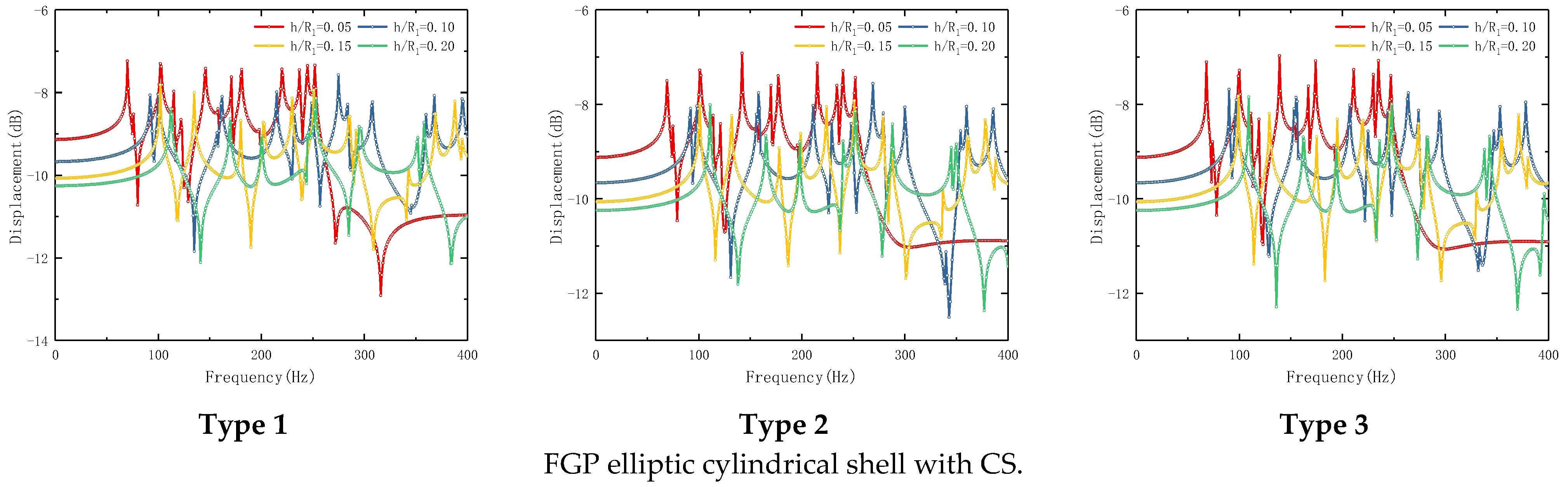
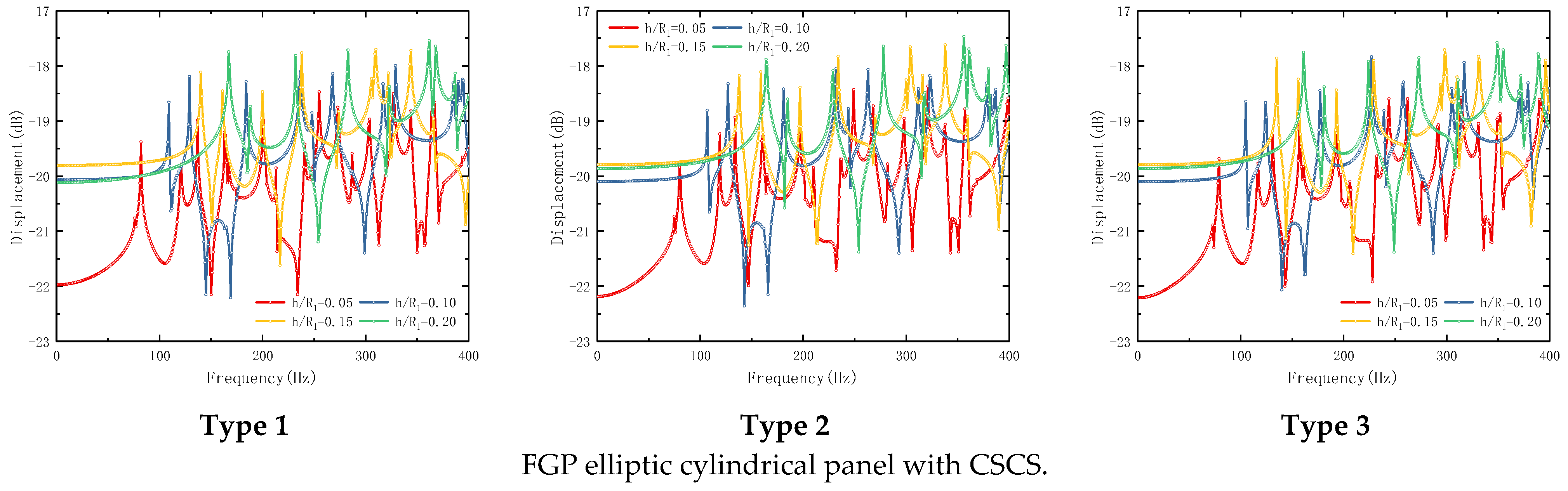
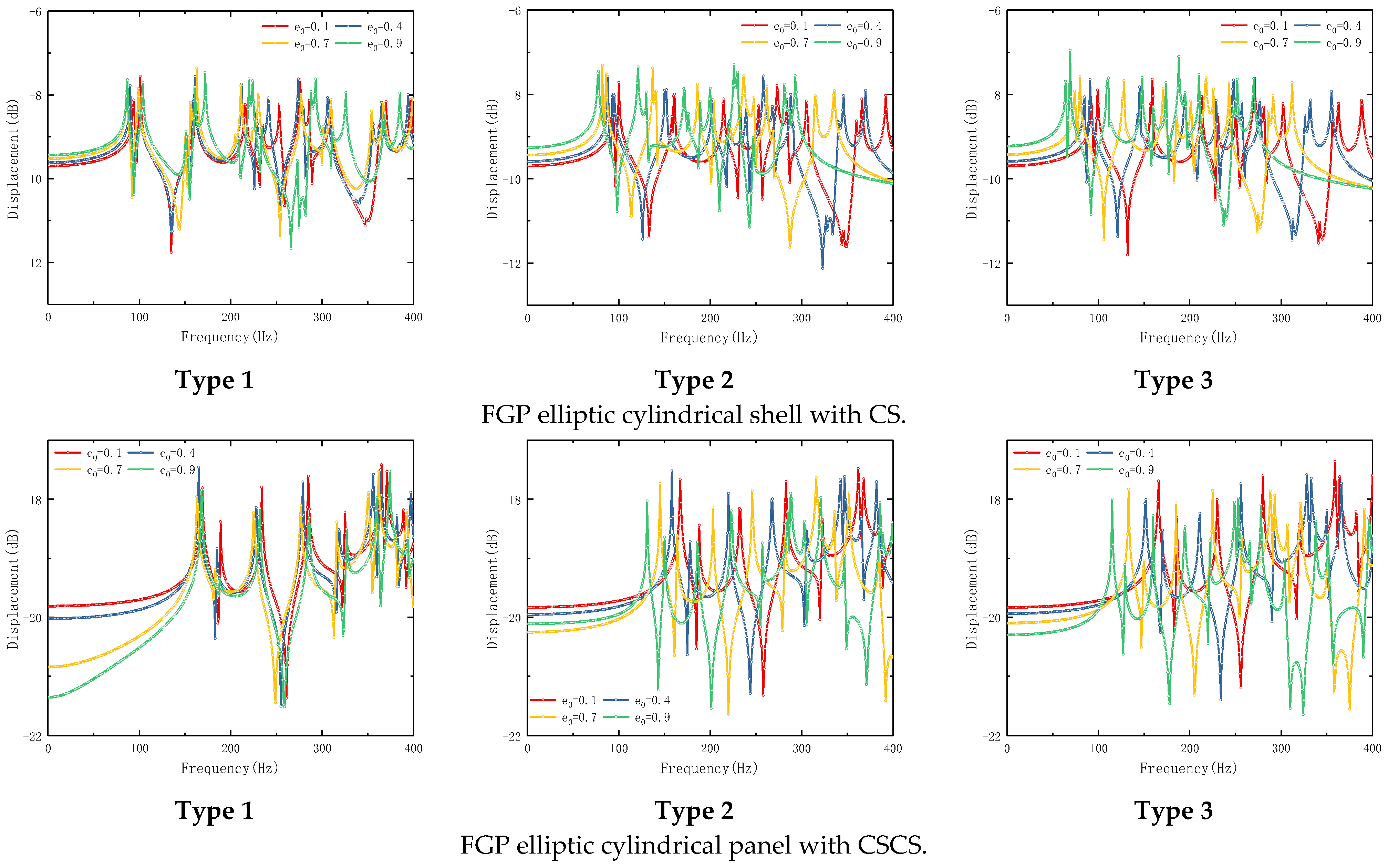
3.2.1. Steady-State Response in Frequency Domain
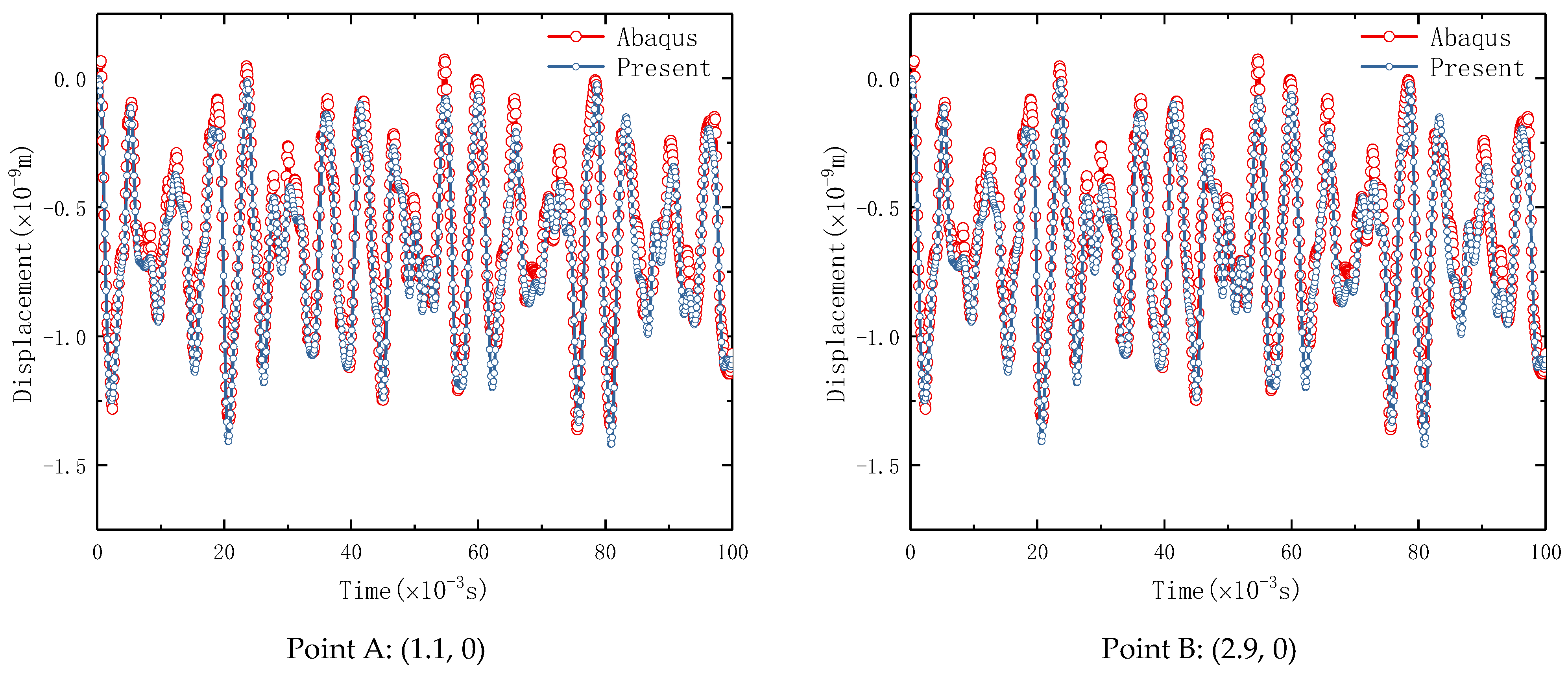
3.2.2. Transient Response in Time Domain
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Su, Z.; Jin, G.; Ye, T. Three-dimensional vibration analysis of thick functionally graded conical, cylindrical shell and annular plate structures with arbitrary elastic restraints. Compos. Struct. 2014, 118, 432–447. [Google Scholar] [CrossRef]
- Chen, D.; Yang, J.; Kitipornchai, S. Free and forced vibrations of shear deformable functionally graded porous beams. Int. J. Mech. Sci. 2016, 108–109, 14–22. [Google Scholar] [CrossRef]
- Masjedi, P.K.; Maheri, A.; Weaver, P.M. Large deflection of functionally graded porous beams based on a geometrically exact theory with a fully intrinsic formulation. Appl. Math. Model. 2019, 76, 938–957. [Google Scholar] [CrossRef]
- Żur, K.; Jankowski, P. Multiparametric Analytical Solution for the Eigenvalue Problem of FGM Porous Circular Plates. Symmetry 2019, 11, 429. [Google Scholar] [CrossRef]
- Yu, C.; Lu, J.; Li, S.; Xu, W.; Chiu, C. Dynamics sensitivity analyses of functionally graded porous (FGP) curved beams with variable curvatures and general boundary conditions. Int. J. Struct. Stab. Dyn. 2021, 21, 2150151. [Google Scholar] [CrossRef]
- Zhao, J.; Xie, F.; Wang, A.; Shuai, C.; Tang, J.; Wang, Q. A unified solution for the vibration analysis of functionally graded porous (FGP) shallow shells with general boundary conditions. Compos. Part B Eng. 2019, 156, 406–424. [Google Scholar] [CrossRef]
- Chen, D.; Yang, J.; Kitipornchai, S. Elastic buckling and static bending of shear deformable functionally graded porous beam. Compos. Struct. 2015, 133, 54–61. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Zia, M. Large amplitude nonlinear vibration analysis of functionally graded Timoshenko beams with porosities. Acta Astronaut. 2015, 116, 117–125. [Google Scholar] [CrossRef]
- Chen, D.; Kitipornchai, S.; Yang, J. Nonlinear free vibration of shear deformable sandwich beam with a functionally graded porous core. Thin-Walled Struct. 2016, 107, 39–48. [Google Scholar] [CrossRef]
- Barati, M.R. Vibration analysis of porous FG nanoshells with even and uneven porosity distributions using nonlocal strain gradient elasticity. Acta Mech. 2017, 229, 1183–1196. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, D. Free vibration of functionally graded porous cylindrical shell using a sinusoidal shear deformation theory. Aerosp. Sci. Technol. 2017, 66, 83–91. [Google Scholar] [CrossRef]
- Mirjavadi, S.S.; Afshari, B.M.; Khezel, M.; Shafiei, N.; Rabby, S.; Kordnejad, M. Nonlinear vibration and buckling of functionally graded porous nanoscaled beams. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 352. [Google Scholar] [CrossRef]
- Kim, J.; Żur, K.K.; Reddy, J.N. Bending, free vibration, and buckling of modified couples stress-based functionally graded porous micro-plates. Compos. Struct. 2019, 209, 879–888. [Google Scholar] [CrossRef]
- Akbaş, Ş.D. Forced vibration analysis of functionally graded porous deep beams. Compos. Struct. 2018, 186, 293–302. [Google Scholar] [CrossRef]
- Huang, X.L.; Dong, L.; Wei, G.Z.; Zhong, D.Y. Nonlinear free and forced vibrations of porous sigmoid functionally graded plates on nonlinear elastic foundations. Compos. Struct. 2019, 228, 111326. [Google Scholar] [CrossRef]
- Bahri, A.; Salehi, M.; Akhlaghi, M. Three-dimensional static and dynamic analyses of the functionally graded cylinder bonded to the laminated plate under general loading. Mech. Adv. Mater. Struct. 2016, 23, 1437–1453. [Google Scholar] [CrossRef]
- Zhao, J.; Choe, K.; Shuai, C.; Wang, A.; Wang, Q. Free vibration analysis of laminated composite elliptic cylinders with general boundary conditions. Compos. Part B Eng. 2019, 158, 55–66. [Google Scholar] [CrossRef]
- Javanbakht, M.; Daneshmehr, A.R.; Shakeri, M.; Nateghi, A. The dynamic analysis of the functionally graded piezoelectric (FGP) shell panel based on three-dimensional elasticity theory. Appl. Math. Model. 2012, 36, 5320–5333. [Google Scholar] [CrossRef]
- Li, H.; Wang, W.; Wang, X.; Han, Q.; Liu, J.; Qin, Z.; Xiong, J.; Guan, Z. A nonlinear analytical model of composite plate structure with an MRE function layer considering internal magnetic and temperature fields. Compos. Sci. Technol. 2020, 200, 108445. [Google Scholar] [CrossRef]
- Lee, A.S.; Kim, B.O.; Kim, Y.C. A finite element transient response analysis method of a rotor-bearing system to base shock excitations using the state-space Newmark scheme and comparisons with experiments. J. Sound Vib. 2006, 297, 595–615. [Google Scholar] [CrossRef]
- Guan, X.; Sok, K.; Wang, A.; Shuai, C.; Tang, J.; Wang, Q. A general vibration analysis of functionally graded porous structure elements of revolution with general elastic restraints. Compos. Struct. 2019, 209, 277–299. [Google Scholar] [CrossRef]
- Lu, J.; Chiu, C.; Zhang, X.; Li, S.; Meng, Z.; Xu, W. Forced vibration analyses of FGP shallow shells with variable curvature. Eur. Phys. J. Plus. 2021, 136, 1–28. [Google Scholar] [CrossRef]





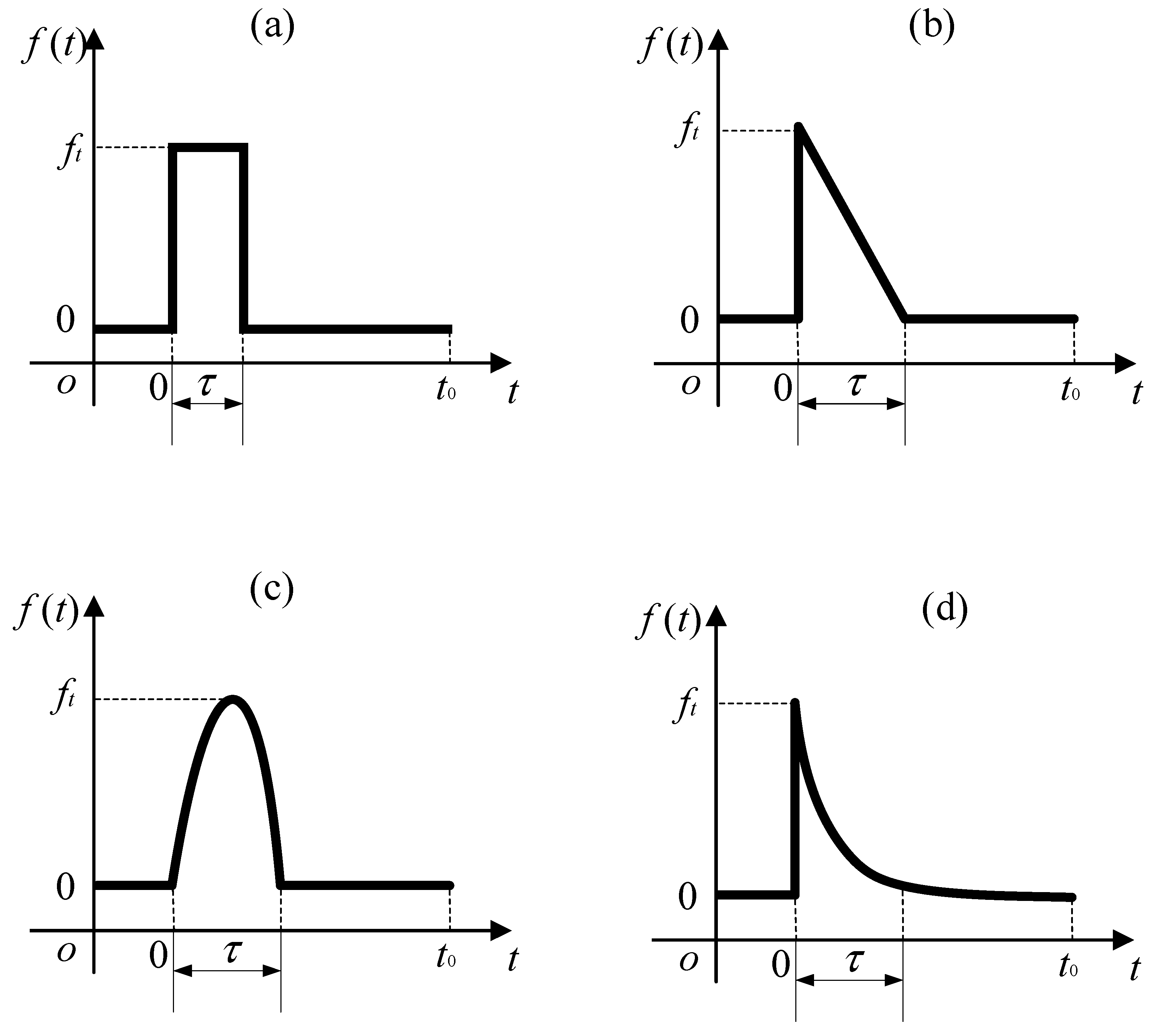



| e0 | Mode | FGP Elliptic Cylindrical Panel | FGP Elliptic Cylindrical Shell | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| CCCC | SSSS | CSCF | CC | SS | CF | ||||||||
| Guan [21] | Present | Guan [21] | Present | Guan [21] | Present | Guan [21] | Present | Guan [21] | Present | Guan [21] | Present | ||
| 0.2 | 1 | 0.569 | 0.569 | 0.497 | 0.497 | 0.241 | 0.241 | 0.423 | 0.422 | 0.386 | 0.385 | 0.171 | 0.171 |
| 2 | 0.695 | 0.695 | 0.890 | 0.590 | 0.262 | 0.262 | 0.440 | 0.440 | 0.406 | 0.406 | 0.247 | 0.246 | |
| 3 | 0.911 | 0.911 | 0.825 | 0.825 | 0.449 | 0.449 | 0.545 | 0.543 | 0.517 | 0.515 | 0.284 | 0.284 | |
| 4 | 0.938 | 0.937 | 0.844 | 0.844 | 0.517 | 0.517 | 0.613 | 0.613 | 0.594 | 0.594 | 0.432 | 0.430 | |
| 0.4 | 1 | 0.547 | 0.547 | 0.480 | 0.480 | 0.232 | 0.232 | 0.406 | 0.406 | 0.368 | 0.367 | 0.165 | 0.165 |
| 2 | 0.668 | 0.668 | 0.563 | 0.563 | 0.252 | 0.252 | 0.424 | 0.424 | 0.388 | 0.388 | 0.237 | 0.237 | |
| 3 | 0.877 | 0.877 | 0.797 | 0.797 | 0.433 | 0.432 | 0.523 | 0.521 | 0.494 | 0.492 | 0.274 | 0.274 | |
| 4 | 0.903 | 0.902 | 0.810 | 0.810 | 0.497 | 0.496 | 0.591 | 0.591 | 0.572 | 0.572 | 0.414 | 0.412 | |
| Type | e0 | FGP Elliptic Cylindrical Shell | FGP Elliptic Cylindrical Panel | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| CC | CF | CS | E1E2 | E1E3 | E2E3 | CCCC | CFCF | CSCF | E1E2E1E2 | E1E3E1E3 | E2E3E2E3 | ||
| Type 1 | 0.1 | 0.118 | 0.046 | 0.116 | 0.104 | 0.076 | 0.090 | 0.135 | 0.073 | 0.078 | 0.112 | 0.098 | 0.119 |
| 0.2 | 0.116 | 0.046 | 0.114 | 0.103 | 0.078 | 0.090 | 0.134 | 0.072 | 0.078 | 0.111 | 0.098 | 0.119 | |
| 0.3 | 0.115 | 0.046 | 0.112 | 0.101 | 0.078 | 0.089 | 0.134 | 0.072 | 0.077 | 0.111 | 0.097 | 0.118 | |
| 0.4 | 0.113 | 0.045 | 0.111 | 0.100 | 0.078 | 0.089 | 0.133 | 0.071 | 0.076 | 0.110 | 0.097 | 0.117 | |
| Type 2 | 0.1 | 0.118 | 0.046 | 0.115 | 0.104 | 0.076 | 0.090 | 0.134 | 0.073 | 0.078 | 0.112 | 0.098 | 0.119 |
| 0.2 | 0.116 | 0.045 | 0.113 | 0.102 | 0.078 | 0.089 | 0.132 | 0.071 | 0.077 | 0.110 | 0.097 | 0.117 | |
| 0.3 | 0.114 | 0.044 | 0.111 | 0.100 | 0.077 | 0.088 | 0.130 | 0.070 | 0.075 | 0.109 | 0.095 | 0.116 | |
| 0.4 | 0.112 | 0.044 | 0.109 | 0.098 | 0.076 | 0.087 | 0.127 | 0.069 | 0.074 | 0.107 | 0.094 | 0.114 | |
| Type 3 | 0.1 | 0.117 | 0.046 | 0.114 | 0.103 | 0.076 | 0.089 | 0.133 | 0.072 | 0.077 | 0.111 | 0.097 | 0.118 |
| 0.2 | 0.114 | 0.044 | 0.111 | 0.100 | 0.076 | 0.087 | 0.130 | 0.070 | 0.075 | 0.108 | 0.095 | 0.115 | |
| 0.3 | 0.110 | 0.043 | 0.108 | 0.097 | 0.075 | 0.086 | 0.126 | 0.068 | 0.073 | 0.105 | 0.093 | 0.112 | |
| 0.4 | 0.107 | 0.042 | 0.105 | 0.094 | 0.073 | 0.083 | 0.122 | 0.066 | 0.071 | 0.102 | 0.090 | 0.109 | |
| Type | a/b | FGP Elliptic Cylindrical Shell | FGP Elliptic Cylindrical Panel | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| e0 = 0.2 | e0 = 0.3 | e0 = 0.4 | e0 = 0.5 | e0 = 0.6 | e0 = 0.7 | e0 = 0.2 | e0 = 0.3 | e0 = 0.4 | e0 = 0.5 | e0 = 0.6 | e0 = 0.7 | ||
| Type 1 | 0.5 | 0.161 | 0.160 | 0.159 | 0.157 | 0.154 | 0.152 | 0.250 | 0.248 | 0.246 | 0.245 | 0.244 | 0.244 |
| 1 | 0.159 | 0.157 | 0.155 | 0.154 | 0.152 | 0.151 | 0.249 | 0.247 | 0.244 | 0.241 | 0.239 | 0.237 | |
| 1.5 | 0.139 | 0.137 | 0.135 | 0.133 | 0.132 | 0.130 | 0.194 | 0.193 | 0.192 | 0.191 | 0.191 | 0.192 | |
| 2 | 0.114 | 0.112 | 0.111 | 0.109 | 0.108 | 0.107 | 0.134 | 0.134 | 0.133 | 0.132 | 0.132 | 0.132 | |
| Type 2 | 0.5 | 0.157 | 0.154 | 0.151 | 0.147 | 0.143 | 0.137 | 0.246 | 0.242 | 0.237 | 0.232 | 0.225 | 0.219 |
| 1 | 0.157 | 0.154 | 0.151 | 0.147 | 0.143 | 0.139 | 0.247 | 0.243 | 0.238 | 0.233 | 0.227 | 0.221 | |
| 1.5 | 0.138 | 0.135 | 0.132 | 0.129 | 0.126 | 0.123 | 0.190 | 0.187 | 0.183 | 0.178 | 0.173 | 0.167 | |
| 2 | 0.113 | 0.111 | 0.109 | 0.106 | 0.104 | 0.101 | 0.132 | 0.130 | 0.127 | 0.124 | 0.121 | 0.117 | |
| Type 3 | 0.5 | 0.154 | 0.150 | 0.145 | 0.140 | 0.134 | 0.127 | 0.242 | 0.235 | 0.227 | 0.219 | 0.210 | 0.199 |
| 1 | 0.155 | 0.150 | 0.145 | 0.140 | 0.134 | 0.128 | 0.242 | 0.235 | 0.228 | 0.219 | 0.210 | 0.200 | |
| 1.5 | 0.136 | 0.132 | 0.127 | 0.123 | 0.118 | 0.112 | 0.186 | 0.181 | 0.175 | 0.169 | 0.162 | 0.154 | |
| 2 | 0.111 | 0.108 | 0.105 | 0.101 | 0.097 | 0.092 | 0.130 | 0.126 | 0.122 | 0.117 | 0.112 | 0.107 | |
| Type | L/a | FGP Elliptic Cylindrical Shell | FGP Elliptic Cylindrical Panel | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| e0 = 0.2 | e0 = 0.3 | e0 = 0.4 | e0 = 0.5 | e0 = 0.6 | e0 = 0.7 | e0 = 0.2 | e0 = 0.3 | e0 = 0.4 | e0 = 0.5 | e0 = 0.6 | e0 = 0.7 | ||
| Type 1 | 2 | 0.243 | 0.240 | 0.238 | 0.236 | 0.235 | 0.234 | 0.273 | 0.270 | 0.269 | 0.267 | 0.266 | 0.266 |
| 3 | 0.173 | 0.171 | 0.169 | 0.168 | 0.167 | 0.166 | 0.189 | 0.187 | 0.185 | 0.184 | 0.183 | 0.183 | |
| 4 | 0.138 | 0.137 | 0.136 | 0.135 | 0.134 | 0.132 | 0.153 | 0.152 | 0.151 | 0.150 | 0.149 | 0.149 | |
| 5 | 0.114 | 0.112 | 0.111 | 0.109 | 0.108 | 0.107 | 0.134 | 0.134 | 0.133 | 0.132 | 0.132 | 0.132 | |
| Type 2 | 2 | 0.239 | 0.235 | 0.230 | 0.225 | 0.220 | 0.213 | 0.269 | 0.264 | 0.259 | 0.253 | 0.247 | 0.239 |
| 3 | 0.170 | 0.167 | 0.163 | 0.160 | 0.155 | 0.150 | 0.186 | 0.183 | 0.179 | 0.175 | 0.171 | 0.166 | |
| 4 | 0.136 | 0.133 | 0.131 | 0.127 | 0.124 | 0.120 | 0.151 | 0.148 | 0.145 | 0.142 | 0.139 | 0.134 | |
| 5 | 0.113 | 0.111 | 0.109 | 0.106 | 0.104 | 0.101 | 0.132 | 0.130 | 0.127 | 0.124 | 0.121 | 0.117 | |
| Type 3 | 2 | 0.235 | 0.228 | 0.221 | 0.213 | 0.204 | 0.194 | 0.263 | 0.256 | 0.247 | 0.238 | 0.228 | 0.217 |
| 3 | 0.167 | 0.162 | 0.157 | 0.151 | 0.145 | 0.138 | 0.182 | 0.177 | 0.171 | 0.165 | 0.158 | 0.151 | |
| 4 | 0.134 | 0.130 | 0.126 | 0.121 | 0.116 | 0.110 | 0.148 | 0.144 | 0.139 | 0.134 | 0.129 | 0.122 | |
| 5 | 0.111 | 0.108 | 0.105 | 0.101 | 0.097 | 0.092 | 0.130 | 0.126 | 0.122 | 0.117 | 0.112 | 0.107 | |
| Type | θ0 | e0 | ||||||
|---|---|---|---|---|---|---|---|---|
| 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | ||
| Type 1 | π/6 | 4.998 | 4.940 | 4.882 | 4.825 | 4.768 | 4.717 | 4.675 |
| π/3 | 1.287 | 1.273 | 1.259 | 1.246 | 1.233 | 1.223 | 1.216 | |
| π/2 | 0.267 | 0.265 | 0.264 | 0.263 | 0.263 | 0.263 | 0.264 | |
| 2π/3 | 0.160 | 0.158 | 0.157 | 0.156 | 0.154 | 0.153 | 0.153 | |
| 5π/6 | 0.146 | 0.145 | 0.144 | 0.143 | 0.143 | 0.142 | 0.143 | |
| Type 2 | π/6 | 4.974 | 4.889 | 4.799 | 4.702 | 4.599 | 4.486 | 4.361 |
| π/3 | 1.280 | 1.258 | 1.235 | 1.211 | 1.184 | 1.155 | 1.124 | |
| π/2 | 0.264 | 0.260 | 0.255 | 0.250 | 0.244 | 0.237 | 0.228 | |
| 2π/3 | 0.159 | 0.156 | 0.153 | 0.150 | 0.147 | 0.143 | 0.139 | |
| 5π/6 | 0.145 | 0.142 | 0.140 | 0.137 | 0.134 | 0.130 | 0.126 | |
| Type 3 | π/6 | 4.931 | 4.800 | 4.660 | 4.509 | 4.346 | 4.165 | 3.962 |
| π/3 | 1.269 | 1.235 | 1.199 | 1.160 | 1.118 | 1.072 | 1.019 | |
| π/2 | 0.261 | 0.254 | 0.247 | 0.239 | 0.230 | 0.221 | 0.210 | |
| 2π/3 | 0.157 | 0.153 | 0.149 | 0.144 | 0.139 | 0.133 | 0.126 | |
| 5π/6 | 0.143 | 0.139 | 0.135 | 0.131 | 0.126 | 0.121 | 0.115 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, J.; Yang, Q.; Meng, Z.; Yang, K.; Xu, W.; Chiu, C. Dynamic Analysis of Functionally Graded Porous (FGP) Elliptic Cylindrical Shell Based on Jacobi Polynomials Ritz Method. J. Compos. Sci. 2023, 7, 344. https://doi.org/10.3390/jcs7080344
Lu J, Yang Q, Meng Z, Yang K, Xu W, Chiu C. Dynamic Analysis of Functionally Graded Porous (FGP) Elliptic Cylindrical Shell Based on Jacobi Polynomials Ritz Method. Journal of Composites Science. 2023; 7(8):344. https://doi.org/10.3390/jcs7080344
Chicago/Turabian StyleLu, J., Q. Yang, Z. Meng, K. Yang, W. Xu, and C. Chiu. 2023. "Dynamic Analysis of Functionally Graded Porous (FGP) Elliptic Cylindrical Shell Based on Jacobi Polynomials Ritz Method" Journal of Composites Science 7, no. 8: 344. https://doi.org/10.3390/jcs7080344
APA StyleLu, J., Yang, Q., Meng, Z., Yang, K., Xu, W., & Chiu, C. (2023). Dynamic Analysis of Functionally Graded Porous (FGP) Elliptic Cylindrical Shell Based on Jacobi Polynomials Ritz Method. Journal of Composites Science, 7(8), 344. https://doi.org/10.3390/jcs7080344





