Composite Overwrapped Pressure Vessel Design Optimization Using Numerical Method
Abstract
:1. Introduction
2. Materials and Methods
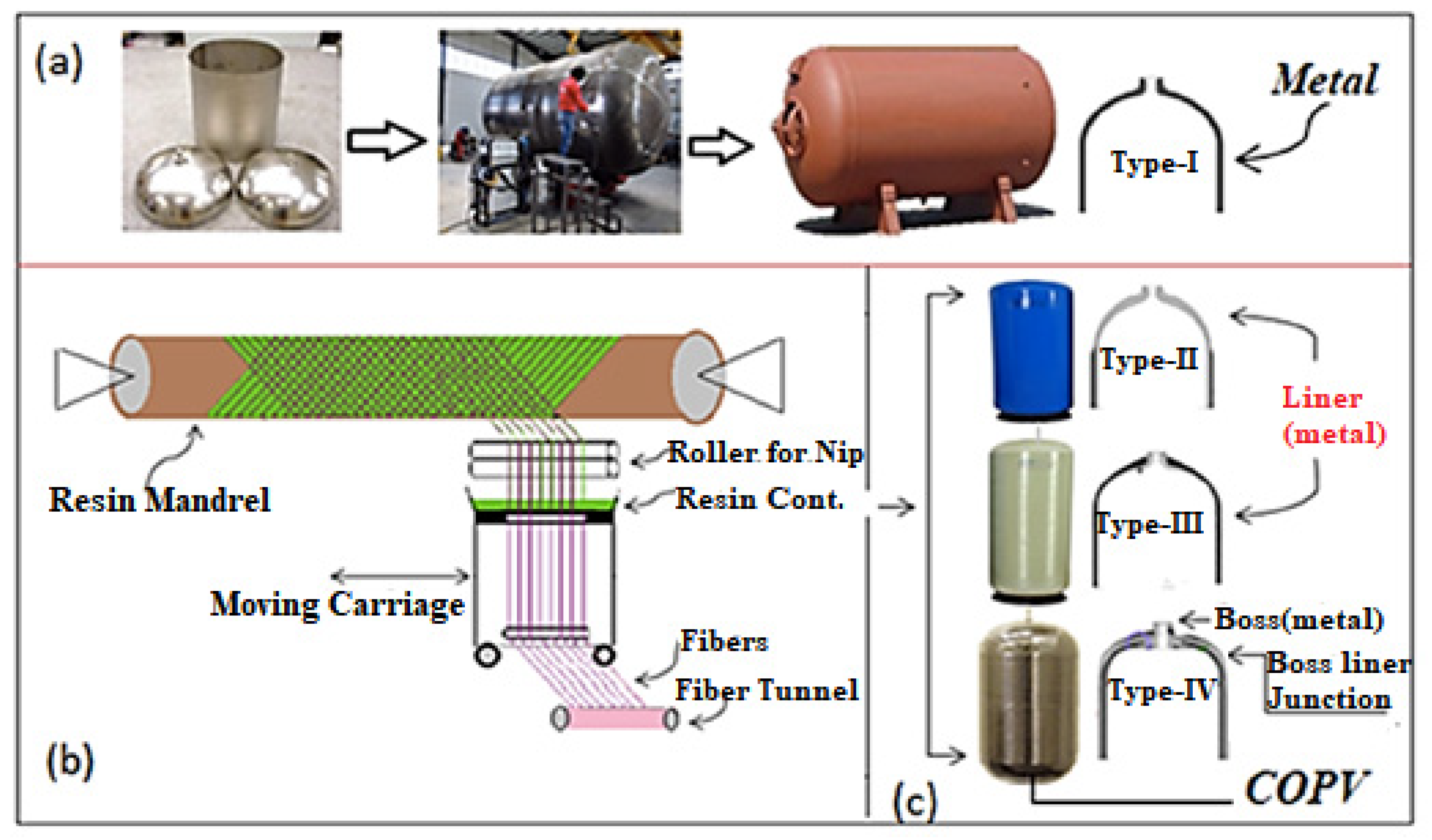
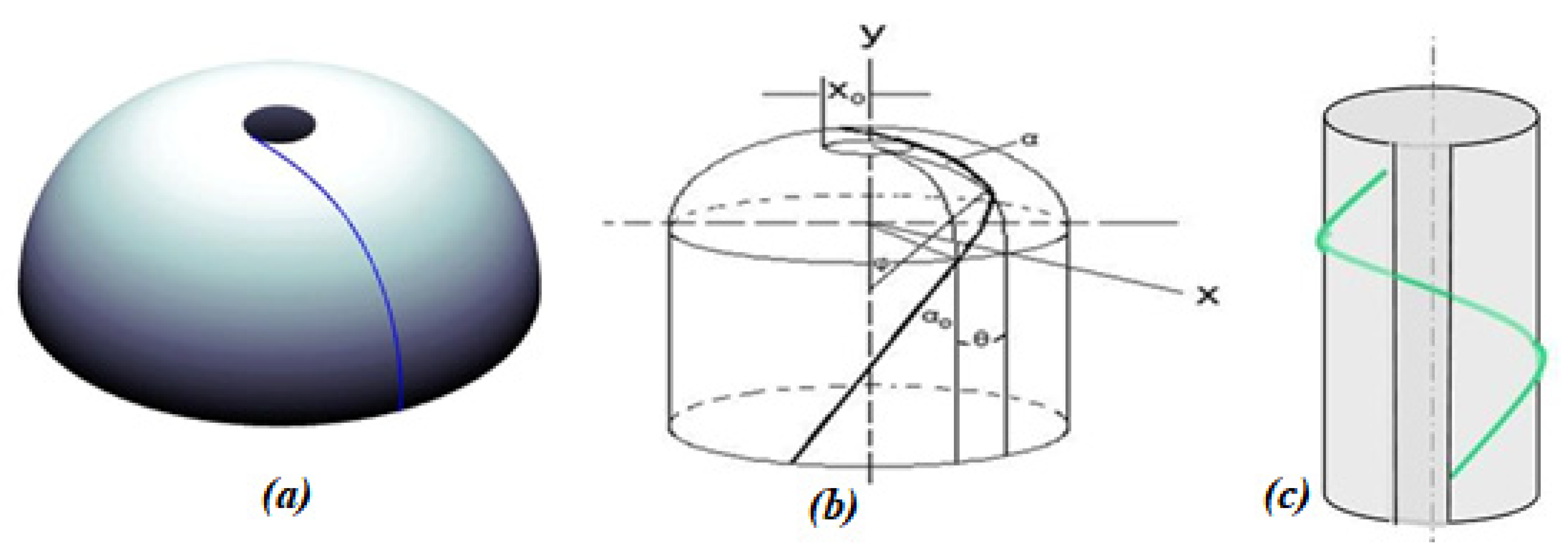
2.1. Analytical Study of the Composite Overwrapped Pressure Vessel
- ;
- ;
- .
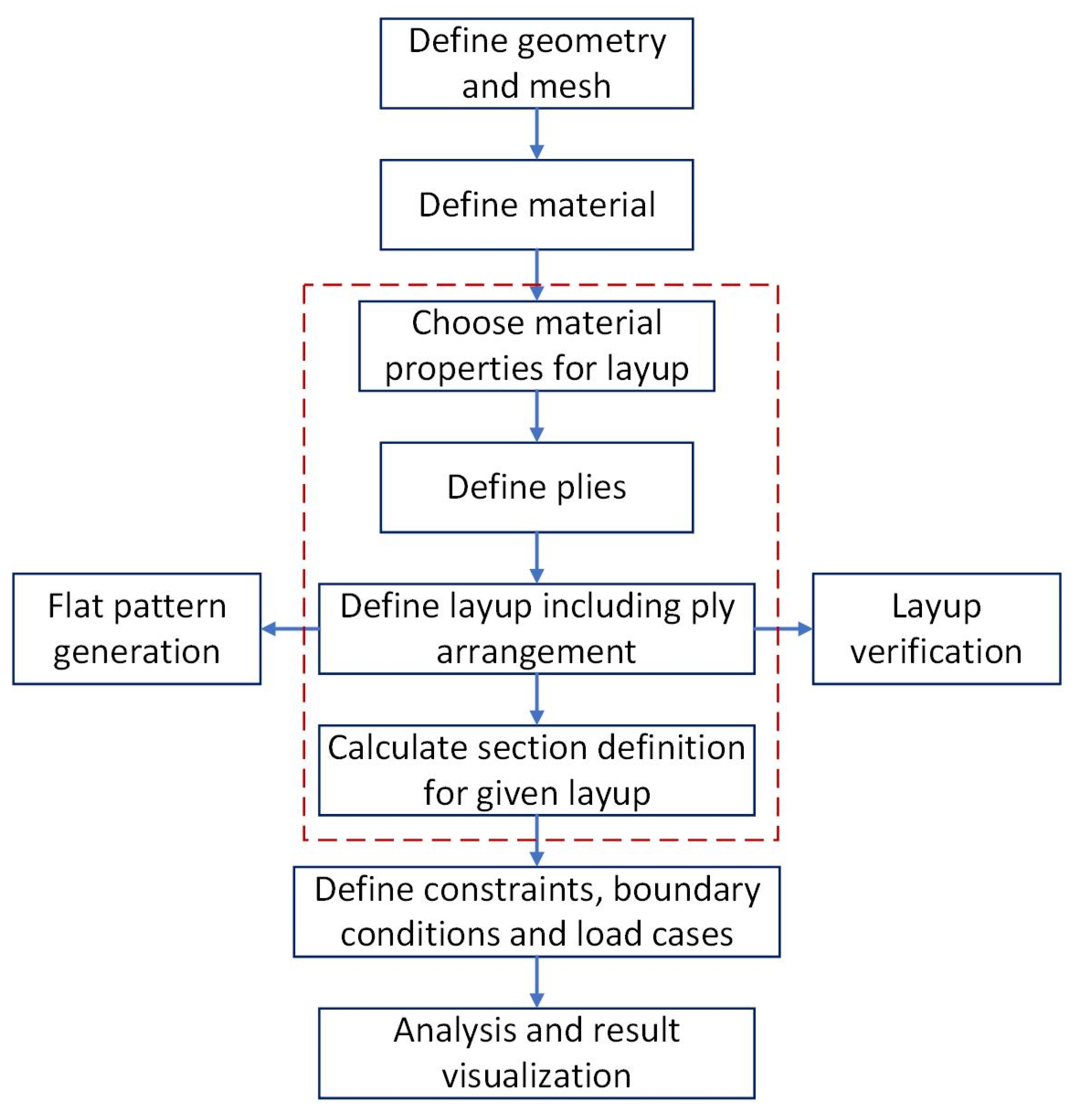
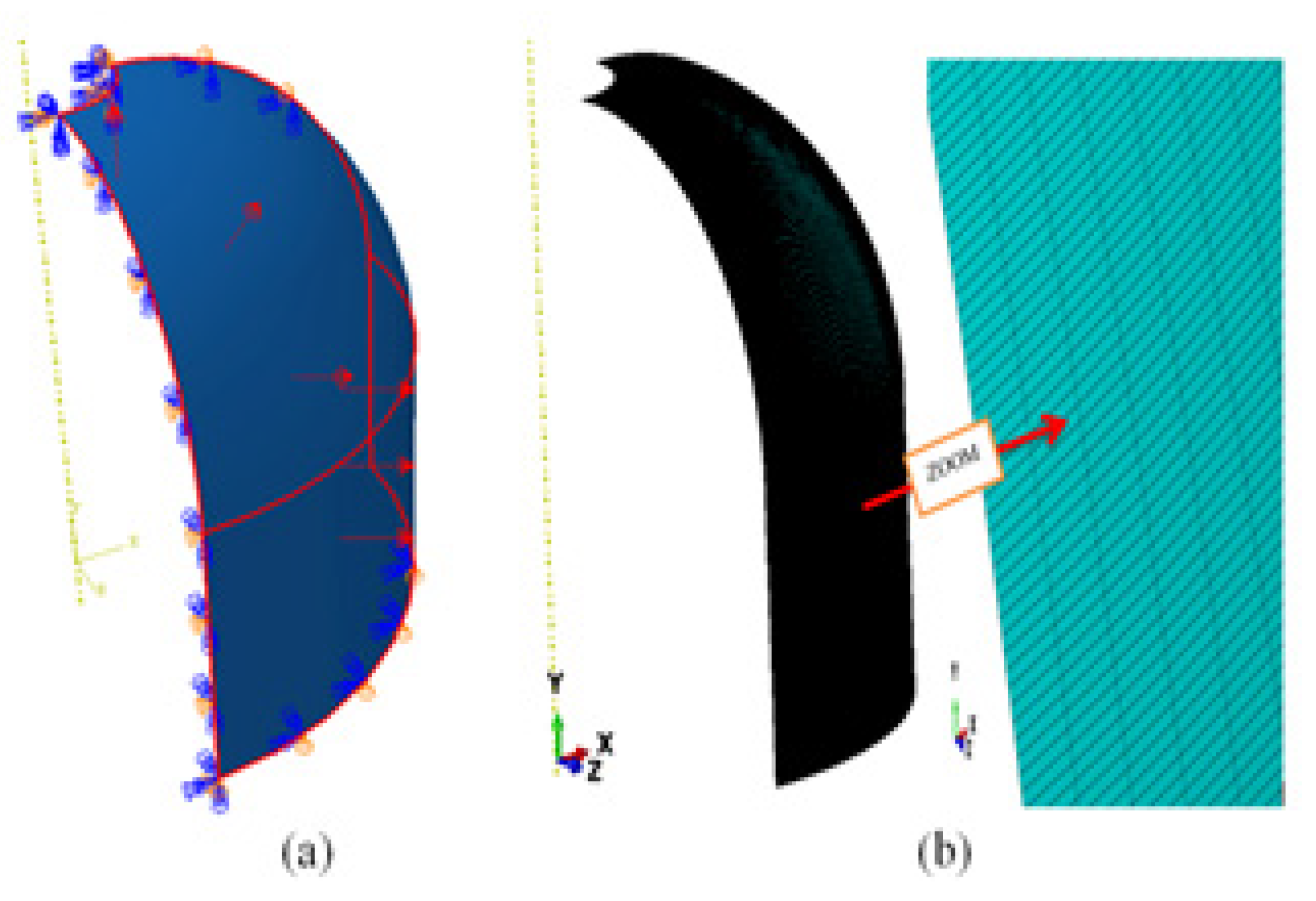
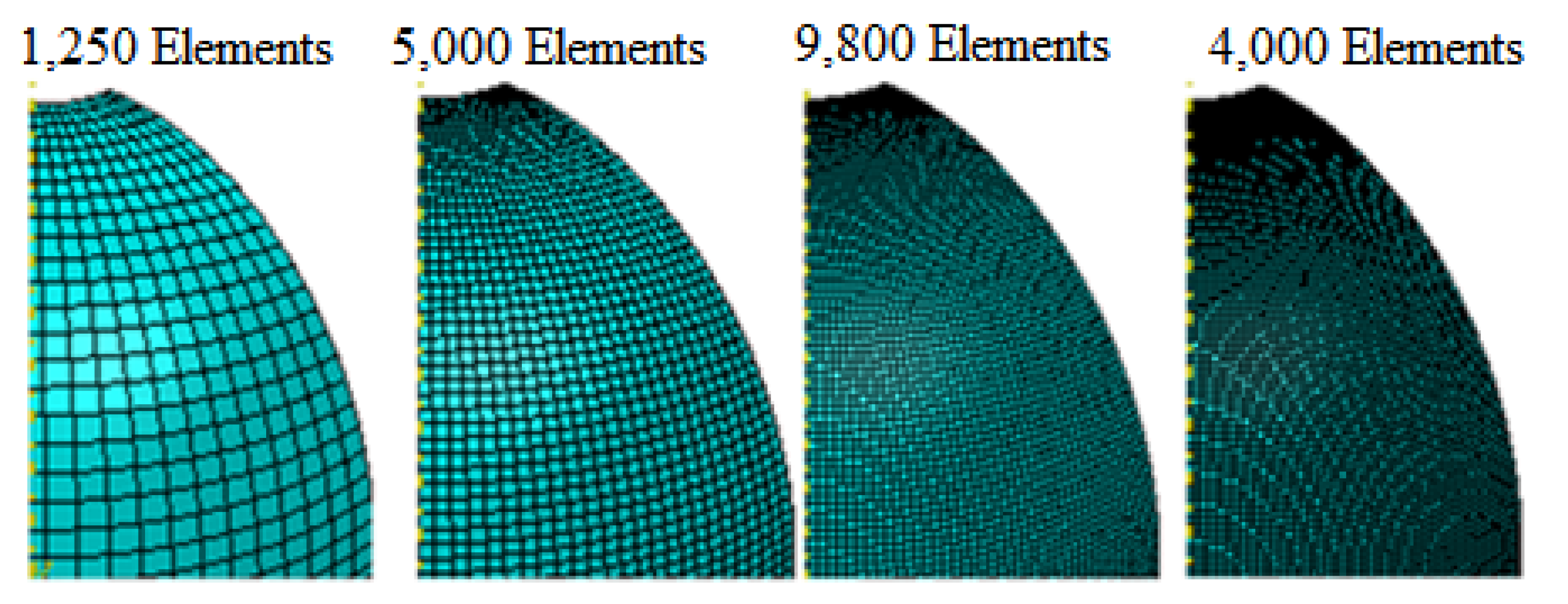
2.2. Finite Element Modeling of Composite Pressure Vessel
3. Results and Discussion
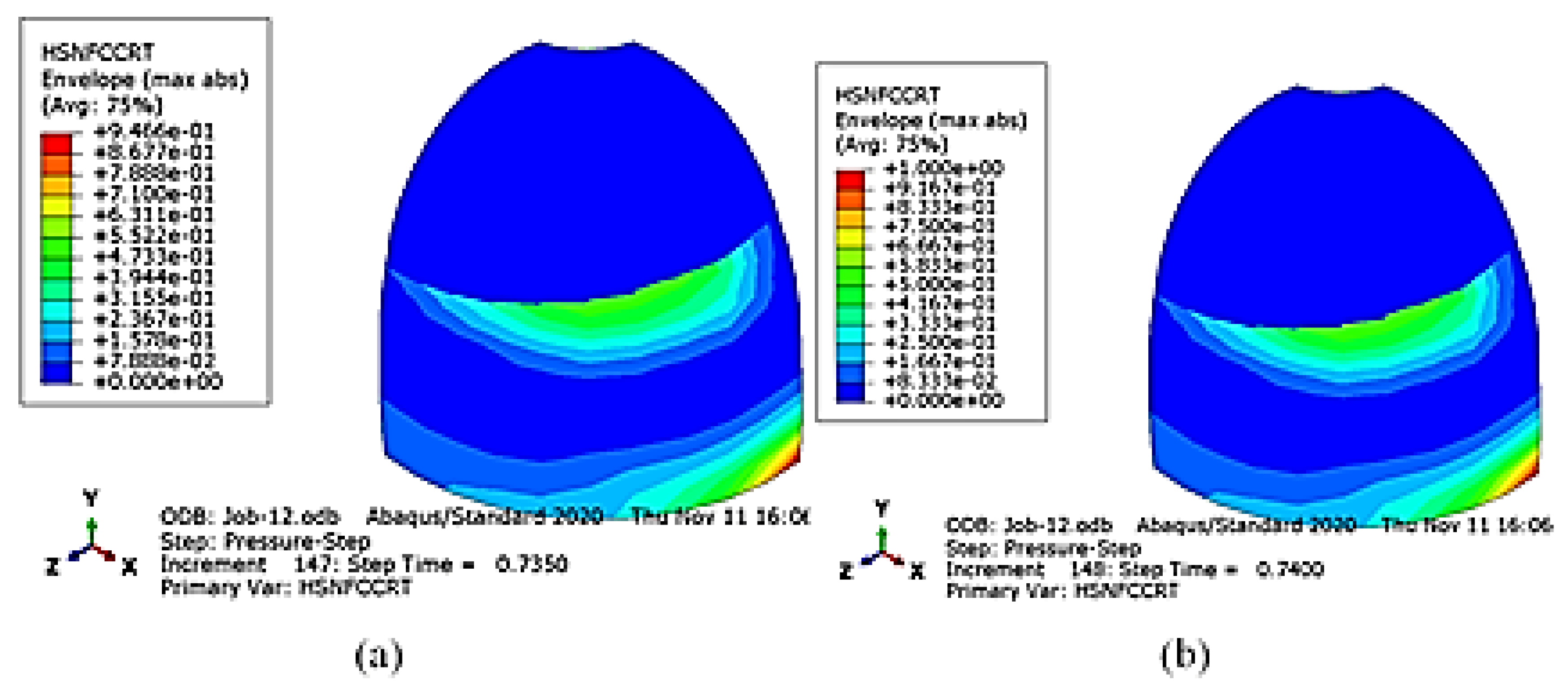
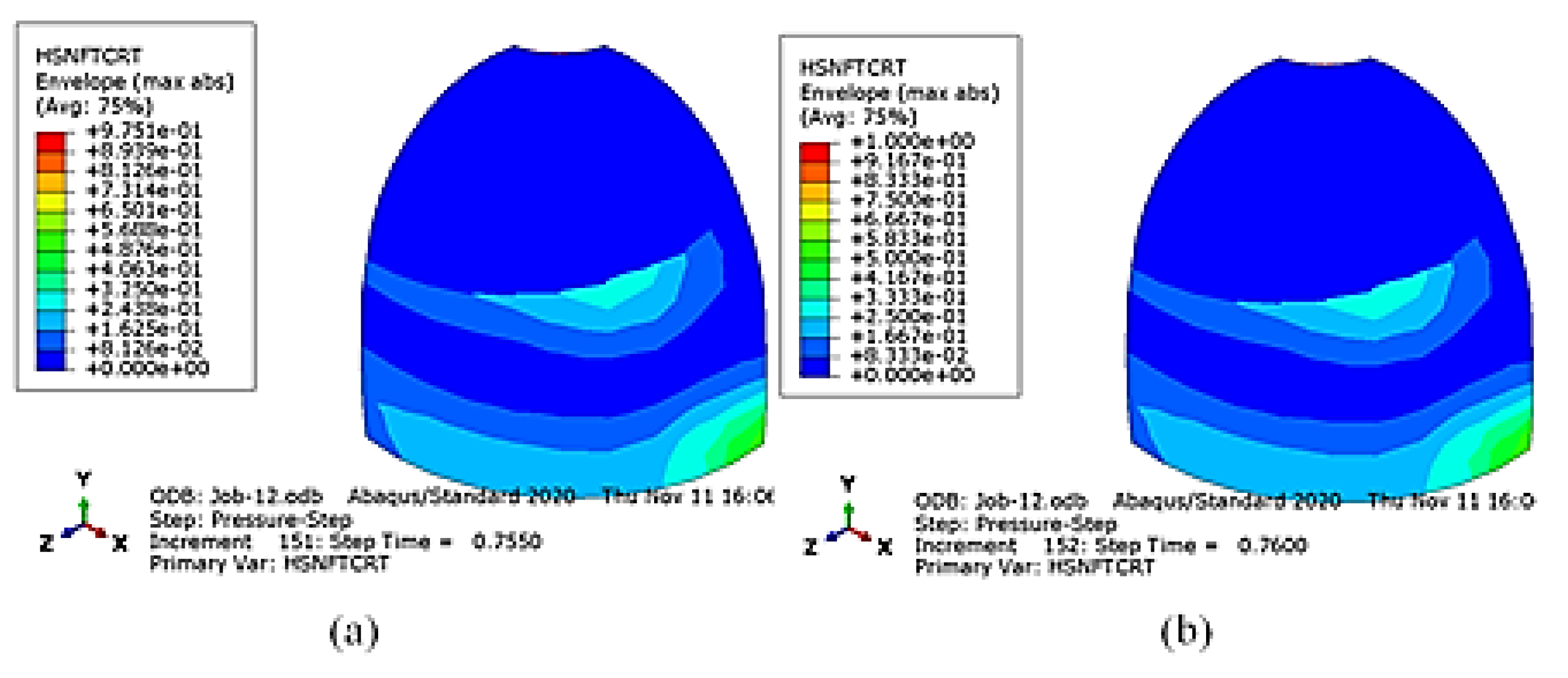
3.1. Burst Pressure Analysis
3.2. Effect of Stacking Sequence of Layers
3.3. Fiber Angle Orientation Effect on Burst Pressure
3.4. Fiber Stress–Strain Distribution in the COPV
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Andrianov, A.; Tomita, E.K.; Veras, C.A.G.; Telles, B. A Low-Cost Filament Winding Technology for University Laboratories and Startups. Polymers 2022, 14, 1066. [Google Scholar] [CrossRef]
- Li, G.; Yan, Z.; Outer, B.; Creep, R.; Load, G. Model assessment of the lifetime of a composite overwrapped pressure vessel under creep conditions. J. Phys. Conf. Ser. 2020, 1666, 012069. [Google Scholar] [CrossRef]
- Thesken, J.C.; Palko, J.L.; Eldridge, J.; Sutter, J. A Theoretical Investigation of Composite Overwrapped Pressure Vessel (COPV) Mechanics Applied to NASA Full Scale Tests; NASA Center for Aero Space Information (CASI): Hanover, MD, USA, 2009.
- Schonberg, W.P. A rupture limit equation for pre-loaded laminated composite plates. J. Compos. Sci. 2018, 2, 3. [Google Scholar] [CrossRef] [Green Version]
- Sofi, T.; Neunkirchen, S.; Schledjewski, R. Advanced manufacturing: Polymer & Composites Science Path calculation, technology and opportunities in dry fiber winding: A review. Adv. Manuf. Polym. Compos. Sci. 2018, 4, 57–72. [Google Scholar] [CrossRef]
- Regassa, Y.; Lemu, H.G.; Sirhabizu, B. Burst strength analysis of composite overwrapped pressure vessel using finite element method. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1201, 012029. [Google Scholar] [CrossRef]
- Forth, S.C.; Pat, B. Composite Overwrapped Pressure Vessels, a Primer; NASA Center for Aero Space Information: Hanover, MD, USA, 2011.
- Azeem, M.; Haji, H.; Kumar, M. Application of filament winding technology in composite pressure vessels and challenges: A Review. J. Energy Storage 2022, 49, 103468. [Google Scholar] [CrossRef]
- Jois, K.C.; Welsh, M.; Gries, T.; Sackmann, J. Numerical analysis of filament wound cylindrical composite pressure vessels accounting for variable dome contour. J. Compos. Sci. 2021, 5, 56. [Google Scholar] [CrossRef]
- Alam, S.; Yandek, G.R.; Lee, R.C.; Mabry, J.M. Design and development of a filament wound composite overwrapped pressure vessel. Compos. Part C 2020, 2, 100045. [Google Scholar] [CrossRef]
- Hastie, J.C.; Kashtalyan, M.; Guz, I.A. Analysis of filament-wound sandwich pipe under combined internal pressure and thermal load considering restrained and closed ends. Int. J. Press. Vessel. Pip. 2021, 191, 104350. [Google Scholar] [CrossRef]
- Madhavi, M.; Rao, K.V.J.; Rao, K.N. Design and analysis of filament wound composite pressure vessel with integrated-end domes. Def. Sci. J. 2009, 59, 73–81. [Google Scholar] [CrossRef] [Green Version]
- Barthelemy, H.; Weber, M.; Barbier, F. Hydrogen storage: Recent improvements and industrial perspectives. Int. J. Hydrogen Energy 2017, 42, 7254–7262. [Google Scholar] [CrossRef]
- Sahami, M.; Heidary, H. Parametric study on drilling of GFRP composite pipe produced by fi lament winding process in different backup condition. Compos. Struct. 2020, 243, 111661. [Google Scholar] [CrossRef]
- Trzepieciński, T.; Najm, S.M.; Sbayti, M.; Belhadjsalah, H.; Szpunar, M.; Lemu, H.G. New advances and future possibilities in forming technology of hybrid metal–polymer composites used in aerospace Applications. J. Compos. Sci. 2021, 5, 217. [Google Scholar] [CrossRef]
- Luca, S.; Emmanouel, A.; Costanzo, B. Robotic filament winding: An innovative technology to manufacture complex shape structural parts. Compos. Struct. 2019, 220, 699–707. [Google Scholar] [CrossRef]
- Zu, L.; Xu, H.; Jia, X.; Zhang, Q.; Wang, H.; Zhang, B. Winding path design based on mandrel profile updates of composite pressure vessels. Compos. Struct. 2020, 235, 111766. [Google Scholar] [CrossRef]
- Krysiak, P.; Błachut, A.; Kaleta, J. Theoretical and experimental analysis of inter-layer stresses in filament-wound cylindrical composite structures. Materials 2021, 14, 7037. [Google Scholar] [CrossRef]
- Bari, K.; Bollenbach, L. Spiderweb cellular structures manufactured via additive layer manufacturing for aerospace application. J. Compos. Sci. 2022, 6, 133. [Google Scholar] [CrossRef]
- Nguyen, B.N.; Simmons, K. A multiscale modeling approach to analyze filament-wound composite pressure vessels. J. Compos. Mater. 2013, 47, 2113–2123. [Google Scholar] [CrossRef]
- Canal, J.P.; Micuzzi, A.; Logarzo, H.; Terlisky, A.; Toscano, R.; Dvorkin, E. On the finite element modeling of COPVs. Comput. Struct. 2019, 220, 1–13. [Google Scholar] [CrossRef]
- Hugaas, E.; Vedvik, N.P.; Echtermeyer, A.T. Progressive fatigue failure analysis of a filament wound ring specimen with a hole. J. Compos. Sci. 2021, 5, 251. [Google Scholar] [CrossRef]
- Tarakcioglu, N.; Samanci, A.; Arikan, H.; Akdemir, A. The fatigue behavior of (±55°) 3 filament wound GRP pipes with a surface crack under internal pressure. Compos. Struct. 2007, 80, 207–211. [Google Scholar] [CrossRef]
- Hu, J.; Chen, J.; Sundararaman, S.; Chandrashekhara, K. Analysis of composite hydrogen storage cylinders subjected to localized flame impingements. Int. J. Hydrogen Energy 2008, 33, 2738–2746. [Google Scholar] [CrossRef]
- Halawa, M.; Al-Huniti, N. Optimum design of carbon/epoxy composite pressure vessels including moisture effects. J. Compos. Sci. 2019, 3, 65. [Google Scholar] [CrossRef] [Green Version]
- Alam, S.; Divekar, A. Design optimisation of composite overwrapped pressure vessel through finite element analysis. In Proceedings of the ASME IMECE2017, Tampa, FL, USA, 3–9 November 2017; Volume 9. [Google Scholar] [CrossRef]
- Kamal, A.M.; El-Sayed, T.A.; El-Butch, A.M.A. Analytical and finite element modeling of pressure vessels for seawater reverse osmosis desalination plants. Desalination 2016, 397, 126–139. [Google Scholar] [CrossRef]
- Henrietta, W.L.; Nicolaas, E.; Phillimon, M.M.; Dmitri, B. Chapter 13—Hydrogen Storage, Electrochemical Power Sources: Fundamentals, Systems, and Applications; Smolinka, T., Garche, J., Eds.; Elsevier: Amsterdam, The Netherlands, 2022; pp. 455–486. [Google Scholar] [CrossRef]
- Sulaiman, S.; Borazjani, S.; Tang, S.H. Finite element analysis of filament-wound composite pressure vessel under internal pressure. IOP Conf. Ser. Mater. Sci. Eng. 2013, 50, 012061. [Google Scholar] [CrossRef] [Green Version]
- Kartav, O.; Kangal, S.; Yücetürk, K.; Tanoğlu, M. Development and analysis of composite overwrapped pressure vessels for hydrogen storage. J. Compos. Mater. 2021, 55, 4141–4155. [Google Scholar] [CrossRef]
- Wang, H.; Fu, S.; Chen, Y.; Hua, L. Thickness-prediction method involving tow redistribution for the dome of composite hydrogen storage vessels. Polymers 2022, 14, 902. [Google Scholar] [CrossRef]
- Kang, H.; He, P.; Zhang, C.; Dai, Y.; Lv, H.; Zhang, M.; Yang, D. Stress–strain and burst failure analysis of fiber wound composite material high-pressure vessel. Polym. Polym. Compos. 2021, 29, 1291–1303. [Google Scholar] [CrossRef]
- Sulaiman, S.; Borazjani, S.; Roshanand, A.; Heydaryan, S. Failure analysis of aluminum reinforced composite vessel. Appl. Mech. Mater. 2014, 392, 178–182. [Google Scholar] [CrossRef]
- Nikbakt, S.; Kamarian, S.; Shakeri, M. A review on optimization of composite structures Part I: Laminated composites. Compos. Struct. 2017, 195, 158–185. [Google Scholar] [CrossRef]
- Kumar, S.S.; Kumari, A.S. Design and Failure analysis of Geodesic Dome of a Composite Pressure vessel. Int. J. Eng. Technol. 2012, 1, 1–8. [Google Scholar]










| Property | Units | Value | |
|---|---|---|---|
| Aluminum (AL6061) | Density | (kg/m3) | 1570 |
| Young’s modulus | GPa | 74.12 | |
| Poisson’s ratio (ν) | - | 0.3 | |
| Ultimate shear strength | GPa | 0.6 | |
| Elastic | Young’s modulus in direction 1 (E1) | GPa | 176.8 |
| Young’s modulus in direction 2 (E2) | GPa | 10.3 | |
| Poisson’s ratio in direction 12 (ν12) | - | 0.23 | |
| Shear modulus in direction 12 (G12) | MPa | 4.8 | |
| Hashin’s parameters | Tensile strength in direction 1(XT) | GPa | 3.3 |
| Compressive strength in direction 1 (XC) | GPa | 1.7 | |
| Tensile strength in direction 2 (YT) | GPa | 0.096 | |
| Compressive strength in direction 2 (YC) | GPa | 0.289 | |
| Shear strength in direction 1 (SL) | GPa | 0.096 | |
| Shear strength in direction 2 (ST) | MPa | 0.096 | |
| Damage Evolution | Longitudinal Tensile Fracture Energy (Glt) | MJ/mm2 | 984.778 |
| Longitudinal Compressive Fracture Energy (Glc) | MJ/mm2 | 277.9966 | |
| Transverse Tensile Fracture Energy (Gtt) | MJ/mm2 | 7.02575 | |
| Transverse Compressive Fracture Energy (Gtc) | MJ/mm2 | 117.694 |
| Case No. | No. of Layers | Winding Angle [°]/Ply Sequence | Calculated Burst Pressure Range (MPa) | Predicted Average Burst Pressure (MPa) |
|---|---|---|---|---|
| 1 | 13 | PP* [15, −15] s | - | - |
| 2 | 13 | PP [30, −30] s | - | - |
| 3 | 13 | PP [45, −45] s | 19.7–20.5 | 20.1 |
| 4 | 13 | PP [55, −55] s | 20.7–27.756 | 24.228 |
| 5 | 13 | PP [60, −60] s | 17.7–24.75 | 21.225 |
| 6 | 13 | HH** [75, −75] s | 13.95–29.772 | 21.861 |
| 7 | 13 | HH [89, −89] s | 13.35–15.9 | 14.175 |
| 8 | 13 | PHP [25/−25/87.5/−25/87.5/−25/87.5] s | - | - |
| 9 | 5 | PP [55, −55] s | 20.115–28.515 | 24.315 |
| 10 | 8 | PP [55, −55] s | 21–27.99 | 24.495 |
| 11 | 14 | PP [55, −55] s | 20.7–27.9 | 24.3 |
| 12 | 20 | PP [55, −55] s | 20.859–27.759 | 24.309 |
| 13 | 21 | PP [55, −55] s | 20.706–27.756 | 24.231 |
| 14 | 27 | PP [55, −55] s | 20.8566–27.756 | 24.3063 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Regassa, Y.; Gari, J.; Lemu, H.G. Composite Overwrapped Pressure Vessel Design Optimization Using Numerical Method. J. Compos. Sci. 2022, 6, 229. https://doi.org/10.3390/jcs6080229
Regassa Y, Gari J, Lemu HG. Composite Overwrapped Pressure Vessel Design Optimization Using Numerical Method. Journal of Composites Science. 2022; 6(8):229. https://doi.org/10.3390/jcs6080229
Chicago/Turabian StyleRegassa, Yohannes, Jema Gari, and Hirpa G. Lemu. 2022. "Composite Overwrapped Pressure Vessel Design Optimization Using Numerical Method" Journal of Composites Science 6, no. 8: 229. https://doi.org/10.3390/jcs6080229
APA StyleRegassa, Y., Gari, J., & Lemu, H. G. (2022). Composite Overwrapped Pressure Vessel Design Optimization Using Numerical Method. Journal of Composites Science, 6(8), 229. https://doi.org/10.3390/jcs6080229








