S-N Curve Models for Composite Materials Characterisation: An Evaluative Review

Abstract

S-N behavior has been a backbone of material fatigue life studies since the 19th century. Numerous S-N curve models have been produced but they have been arbitrarily chosen in numerous research works dominantly for composite materials. In this paper, they were critically reviewed and evaluated for capability using the following criteria: data fitting capability, efficiency of curve fitting, applicability to data sets at various stress ratios (−0.43, −1, −3, 0.1, and 10), representability of fatigue damage at failure, and satisfaction of the initial boundary condition. The S-N curve models were found to be in two categories—one for fatigue data characterization independent of stress ratio, and the other for those designed for predicting the effect of stress ratio. The models proposed by Weibull, Sendeckyj, and Kim and Zhang for fatigue data characterization appeared to have the best capabilities for experimental data obtained from Weibull for R = −1, from Sendeckyj for R = 0.1, and from Kawai and Itoh (for R = −0.43, −3, and 10). The Kim and Zhang model was found to have an advantage over the Weibull and the Sendeckyj models for representing the fatigue damage at failure. The Kohout and Vechet model was also found to have a good fitting capability but with an inherent limitation for shaping the S-N curve at some stress ratios (e.g., R = −0.43). The S-N curve models developed for predicting the effect of stress ratio were found to be relatively inferior in data fitting capability to those developed directly for fatigue data characterization.

1. Introduction

Fatigue is a phenomenon that causes the progressive damage to materials under cyclic loading. The damage physically consists of cracks and deformation. It eventually leads to the final failure of materials. There have been two different ways to approach the fatigue damage. One of the ways is to deal with cracks individually using fracture mechanics parameters such as the stress intensity factor [1]. The other way is to deal with relatively “small” cracks [2] for fatigue life in a collective manner including crack initiation at a relatively large scale of un-notched geometry with respect to the initial flaws, which requires an S-N curve model for characterization. (S and N in the acronym stand for stress and number of fatigue loading cycles at failure respectively.) Some S-N curve models have been derived from fatigue damage formulations while some others are intuitively obtained empirically or statistically using the Weibull distribution [3]. The S-N curve models have been used for both monolithic and composite materials. The difference between the two types of materials in S-N curve modelling has not significantly been found although the fatigue damage growth pattern varies dependent on material structure. Small cracks, for example, grow in polymeric matrix in the case of fiber reinforced composites but a follow-up crack propagation stage leading to the final failure (or complete separation into two pieces) is often not clearly defined [4,5,6] whereas the initiation site(s) and crack propagation traces in the case of monolithic materials are usually identifiable on the fracture surfaces [7,8,9].

An S-N curve is essential not only for characterizing fatigue test results from un-notched specimens but also for the remaining fatigue life predictions involving fatigue damage quantification and stress ratio effect. Thus, the S-N curve behavior has been a backbone of fatigue life studies since the 19th century. A historian refers to the fatigue test results published in 1837 by Albert [10] and to the term “fatigue” appeared in 1854 [11] as the first ones. Some early fatigue studies, in fact, had begun without an S-N curve model [12]. Also, the S-N fatigue study development in history has been somewhat disorderly in sequence of events, slowing down the knowledge advancement. For example, the research of stress ratio effect on fatigue life [13,14,15,16]) mostly appears earlier than S-N curve modelling [17,18,19,20] although S-N curve modelling should be a prior task to the stress ratio effect for fatigue life prediction. One would be surprised to find the fact that the stress ratio effect has still been a contemporary research topic [21,22] since the 19th century. On the other hand, the study on fatigue damage [23,24,25,26,27] for fatigue life prediction seems to be concurrent with modelling of S-N curve [20,21].

A primary reason for the slow progress in S-N fatigue life prediction studies despite the long history may be that the studies on other topics such as stress ratio effect and fatigue damage for fatigue life predictions cannot be efficiently conducted without knowing an adequate deterministic S-N curve model. Other reasons may be as follows: (a) researchers have been persistent in adhering to particular S-N curve model(s) or producing their own model(s) regardless of the benefits of existing models [20,22,24]; and (b) researchers tend to choose particular damage accumulation rules which are not necessarily valid [28]. Palmgren and Miner’s model [23,29] may be one of those chosen by many researchers even though the Palmgren and Miner damage model is not accurate as Schutz [10] in his historical review pointed out. Also, evidence for the slow progress has been found in the literature. Philippidis and Passipoularidi [30] evaluated nine selected damage models involving residual strength degradation in relation to fatigue life. They found that no model is consistent in accurately predicting the residual strength degradation behavior of different composite laminates for fatigue life. Post et al. [31] also selectively evaluated twelve fatigue life prediction models for spectrum loading applications; they summarized that most of the investigators who proposed models simply fit them to their experimental results with their predictive ability remaining uncertain. It is only recently that Eskandari and Kim [27,32] returned to the fundamentals and provided mathematical reasoning for how fatigue damage models in the past went invalid for predicting the remaining fatigue life. Also, it is true that some researchers [33] still concurrently pursue the conventional approach based on the Palmgren and Miner’s damage model [23,29] without the fundamental basis.

Some literature dealing with S-N curve models has been available since Weibull [34] discussed S-N curve models. Schutz [10] discussed the Basquin model [17] within the context of fatigue history for a period of 1838–1996. Kohout and Vechet [35] reviewed a few S-N curve models in relation to a newly proposed model. Some S-N curve models were overviewed by Nijssen [36]. Recently, Xiong and Shenoi [37] listed four S-N curve models including Basquin model as the generally accepted ones in a monograph. However, the capabilities of S-N curve models for applications at different stress ratios have been neither much known nor extensively reviewed.

In this paper, S-N curve models available since 1910 are critically reviewed and evaluated to identify their capabilities for applications at a wide range of stress ratios when subjected to the uniaxial loading for less than giga load cycles which may be more applicable for composite materials. For evaluation and critical review, the following criteria were adopted: capability of curve fitting, efficiency of curve fitting, applicability to data sets at various stress ratios, representability of fatigue damage at failure, and satisfaction of S-N curve initial boundary condition at the first load cycle.

2. Criteria for S-N Curve Model Evaluation

An S-N curve model is a line shape function preferably on a log-log or lin-log scale for representing the phenomenon of fatigue life dependent on fatigue damage. For evaluation of, and critical review on, S-N curve models, the following criteria may be considered [38]: (a) capability of curve fitting, (b) efficiency of fitting parameter determination, (c) adaptability to various stress ratios, (d) representability of fatigue damage at failure, and (e) satisfaction of the initial boundary condition of S-N curve at the first load cycle. These criteria may be useful for evaluation as will be detailed.

Capability of curve fitting may be the most important criterion because the purpose of any S-N curve, in the first instance, is to characterize the fatigue test data prior to predicting fatigue lives under various conditions. The capability is virtually for curve shaping, complying with the data trend. Once capability of curve fitting is sufficient, an efficiency of fitting parameter determination may be considered. The number of fitting parameters in a model may be judged for the efficiency. If the number of fitting parameters is two, parameters may typically be determined using the traditional least square methods for fitting data in log-log coordinates as for the Basquin model or they can be determined using the well-established statistical methods. However, more than two fitting parameters would inevitably lead to a low efficiency of determination, requiring an additional algorithm.

Adaptability to various stress ratios may be an important criterion for wider applications although most of S-N models have been developed for particular stress ratios. It is useful to deal with the complex fatigue loading involving random variation [39] and to eventually be developed in conjunction with fatigue damage for predicting the remaining fatigue life. As shown in Figure 1 for a stress amplitude (σ_a) vs mean stress (σ_mean) diagram, the stress ratios are divided into four ranges depending on loading type: R = 0–1 under tension-tension (T-T) loading, R = 0–−1 under tension-compression (T-C) loading, R = −1–−∞ under compression-tension (C-T) loading, R = −∞–1 under compression-compression (C-C) loading. The adaptability can be evaluated for capability of curve fitting by varying values of fitting parameters in S-N curve model at different stress ratios.

Representability of fatigue damage at failure applies to some of S-N curve models derived from the fatigue damage formulations. The damage accumulated during cycling (D) or damage at failure (D_f) has existed confusingly in many different forms as listed by Hwang and Han [20], Fatemi and Yang [40], Yang and Fatemi [41], Bathias and Pineau [42], and Christian [43]. A clear difference, though, between damage at failure (D_f) and damage prior to failure (D) should be made to clarify D_f [27]. Damage at failure (D_f) is directly related to an S-N curve which is a locus of failure points caused by damage. Thus, an S-N curve itself indicates damage at failure in a way. Other quantities such as residual strength and residual modulus [44] may be able to represent the damage as well. More importantly, however, if the ultimate purpose of damage D is to predict the remaining fatigue life when applied stress varies, a damage function (D), which is a function of N and applied peak stress σ_max, is required to satisfy the compatibility condition [27] to ensure that σ_max and N compatibly contribute to the damage. If an S-N curve is derived from fatigue damage and to predict the remaining fatigue life involving independent variables (i.e., N_f and σ_max), the damage at tensile failure D_fT is required to satisfy

$$\frac{\partial D_{\mathrm{fT}}}{\partial {\sigma }_{\max }}=-A$$

(1)

or, for damage at compressive failure D_fC,

$$\frac{\partial D_{\mathrm{f}C}}{\partial \left|{\sigma }_{\min }\right|}=-A$$

(2)

where A = a constant greater than zero.

Satisfaction of the initial boundary condition of S-N curve at the first load cycle is to ensure the correspondence with a static strength to cover a full range of applied stress levels in establishing a full S-N plane for fatigue damage determination. It is also a requirement for predicting the remaining fatigue life because the initial boundary condition affects the size of an S-N plane and subsequently the damage quantity [27]. The initial boundary condition, in fact, has been arbitrary as will be discussed. For example, some researchers have adopted N_f = 0 or 0.5 or 1 at σ_uT in the absence of a rigorous rational basis while some earlier researchers have ignored the initial boundary condition. Eskandari and Kim [27] recently provided a rationale for R = 0 to be N_f = 0.5 at σ_uT. However, it has not generally been settled down on other stress ratios yet. Nonetheless, it is necessary that N_f at σ_uT should be 0 < N_f < 1 or adjustable for the future development until a rational value is found while N_f = 0 should not be used not only because it is physically impossible to be a failure point but also because it does not exist on log coordinate axis.

Finally, if an inverse expression of S-N curve equation is obtainable, it would be technically useful for converting between σ_max and N_f without a numerical scheme.

3. S-N Curve Models

3.1. For Fatigue Data Characterisation

S-N curve models developed directly for characterization of fatigue experimental data subjected to a constant amplitude of loading at a constant stress ratio (R) will be discussed in this section.

Some of early S-N curve models had been intuitively formulated. The Basquin model [17] may be the first among those:

$${\sigma }_{\max }=\alpha {(N_{\mathrm{f}})}^{\beta }$$

(3)

where σ_max = applied peak stress, α, β = model fitting parameter, and N_f = number of cycle at failure.

It represents a straight line in log-log coordinates. Equation (3) has been the most frequently used model [45] even though it does not fully fit the experimental data. It is problematic in some applications although the fitting parameters are easily determined. An alternative form to Equation (3) may be in a linear-log form given by;

$${\sigma }_{\max }=\alpha (\log N_{\mathrm{f}})+\beta$$

(4)

Subramanyan [46], Mandell and Meier [47], Bond and Farrow [48], and Tamuzs et al. [49] adopted this linear-log form for various stress ratios while Gathercole et al. [50], Adam et al. [51], and Beheshty et al. [52] adopted polynomial curve fitting of experimental data for σ_max versus logN_f.

Although the fatigue limit (σ_∞) may not exist [53], Stromeyer [54] added an additional term, σ_∞, to Equation (3):

$${\sigma }_{\max }=\alpha {(N_{\mathrm{f}})}^{\beta }+{\sigma }_{\infty }$$

(5)

where σ_max = applied peak stress, α, β = model fitting parameter, and N_f = number of cycle at failure.

Palmgren [23] added one more parameter to have,

$${\sigma }_{\max }=\alpha {(N_{\mathrm{f}})}^{\beta }+{\sigma }_{\infty }$$

(6)

where σ_max = applied peak stress, σ_∞ = fatigue limit, α, β, γ = model fitting parameter, N_f = number of cycle at failure, and δ = number of stress cycles to which the balls or the grooves are subjected during one revolution of the bearing.

Weibull [18] considered an equation for modification suggested by E. Epremian in a private communication;

$$\frac{{\sigma }_{\max }-{\sigma }_{\infty }}{{\sigma }_{\mathrm{uT}}-{\sigma }_{\infty }}=1-\psi (\alpha \log N_{\mathrm{f}}+\beta )$$

(7)

where σ_max = applied peak stress, σ_uT = ultimate tensile strength, σ_∞ = fatigue limit, α, β = model fitting parameter, N_f = number of cycle at failure, and ψ = Gaussian error function, and subsequently proposed an S-N curve model;

$${\sigma }_{\max }=\left({\sigma }_{\mathrm{uT}}-{\sigma }_{\infty }\right)\exp \left[-\alpha {(\log N_{\mathrm{f}})}^{\beta }\right]+{\sigma }_{\infty }$$

(8)

including both the ultimate strength (σ_uT) and the fatigue limit (σ_∞). The two fitting parameters (α and β) in Equation (8) can be determined by taking log on both sides of,

$$\frac{{\sigma }_{\max }-{\sigma }_{\infty }}{{\sigma }_{\mathrm{uT}}-{\sigma }_{\infty }}=\exp \left[-\alpha {(\log N_{\mathrm{f}})}^{\beta }\right]$$

(9)

The Weibull model allows the initial boundary condition of the S-N curve to be N_f = 1 at σ_max = σ_uT and is efficient for determining the fitting parameters although the fatigue damage was not considered. The fatigue limit in the equation, which may not exit, is a disadvantage for curve fitting. However, the assumed fatigue limit may be regarded as being different from a fitting parameter because they can easily be estimated for fitting purposes whereas fitting parameters can be found on a trial and error basis in some cases without much idea on the initial trial value unless a known algorithm is available.

Henry and Dayton [55] assumed

$${\sigma }_{\max }=\frac{\alpha }{N_{\mathrm{f}}}+\beta$$

(10)

where σ_max = applied peak stress, α, β = model fitting parameter, and N_f = number of cycle at failure, for an S-N curve to derive a “damage equation”, which is far from being accurate for data fitting although an initial boundary condition at the first load cycle can be managed to be met.

Sendeckyj [19] proposed

$${\sigma }_{\max }=\frac{{\sigma }_{\mathrm{uT}}}{{(1-\alpha +\alpha N_{\mathrm{f}})}^{\beta }}$$

(11)

or

$$N_{\mathrm{f}}=\frac{{\left({\sigma }_{\mathrm{uT}}/{\sigma }_{\max }\right)}^{1/\beta }-1+\alpha }{\alpha }$$

(12)

where σ_max = applied peak stress, σ_uT = ultimate tensile strength α, β = model fitting parameter, and N_f = number of cycle at failure.

Based on the assumption that so called “equivalent static strength” is of two-parameter Weibull distribution. A way of determination of parameters (α and β) was suggested to follow the steps: (a) estimation of initial α and β values using a computer program, (b) transformation of fatigue data using the estimated initial values into equivalent static strength data, and (c) fitting a Weibull distribution with a solution of non-linear algebraic equation. The steps (b) and (c) are repeated with renewed estimated values until the maximum-likelihood value of the shape parameter estimate is obtained. It is noted that this procedure needs a separate computing algorithm in addition to the first estimation for fitting parameter determination. Equations (11) and (12), however, are with only two fitting parameters so that they can be rapidly found without the procedure suggested by Sendeckyj.

Hwang and Han [24] formulated by defining so called “fatigue modulus” and a strain failure criterion:

$$N_{\mathrm{f}}={\left[\alpha (1-\frac{{\sigma }_{\max }}{{\sigma }_{\mathrm{uT}}})\right]}^{1/\beta }$$

(13)

or

$${\sigma }_{\max }={\sigma }_{\mathrm{uT}}\left(1-\frac{N_{\mathrm{f}}^{\beta }}{\alpha }\right)$$

(14)

where σ_max = applied peak stress, σ_uT = ultimate tensile strength α, β = model fitting parameter, and N_f = number of cycle at failure.

The Hwang and Han model is based on the assumption that the fatigue modulus degradation rate follows a power function. Equations (13) and (14), however, do not satisfy an initial boundary condition, given that N_f should be zero for σ_max = σ_uT, which is not possible on logarithmic coordinates used for N_f.

Ellyin in 1989 [56] proposed the total strain energy input (ΔW) as

$$\Delta W={\alpha }^{\prime }{(N_{\mathrm{f}})}^{{\beta }^{\prime }}+C$$

(15)

where ΔW = total energy input, α′, β′ = model fitting parameter, N_f = number of cycle at failure, and C = constant.

Subsequently, Ellyin and El-Kadi [57] adopted and converted this equation for a constant stress ratio with C = 0. They demonstrated that Equation (15) is consequently the same form as the Basquin model Equation (3).

Kensche [58] used the maximum strain (ε_max) instead of the maximum stress for an S-N relation,

$${\epsilon }_{\max }=\beta \frac{-\ln P{(N_{\mathrm{f}})}^{\frac{1}{\alpha }}}{{[(N_{\mathrm{f}}-{\alpha }^{\prime }){\gamma }^{\prime }]}^{\gamma }}$$

(16)

where α, β, γ′ = shape and the scale parameters respectively for the Weibull distribution, and P(N_f) = probability of survival.

The parameter γ’ allows flattening or steepening of the curve, and α′ = (1 − γ’)/γ’. This equation, in the first place, is not efficient for curve fitting because of the number of fitting parameters although it may be capable of curve fitting at different stress ratios as demonstrated in [58]. Also, it is not common to use the strain vs number of cycles (N_f) for fatigue characterization, unless other aspects are dealt with (e.g., thermos-mechanical fatigue in [59]), because the strain needs to be converted into stress for an S-N curve.

Kohout and Vechet [35] proposed a model to describe an assumed geometrical shape of the S-N curve

$${\sigma }_{\max }={\sigma }_{\infty }{\left[\frac{(N_{\mathrm{f}}+\alpha )}{(N_{\mathrm{f}}+\gamma )}\right]}^{\beta }={\sigma }_{\mathrm{uT}}{\left[\frac{(1+N_{\mathrm{f}}/\alpha )}{(1+N_{\mathrm{f}}/\gamma )}\right]}^{\beta }$$

(17)

or

$${\sigma }_{\max }={\sigma }_{\infty }{\left[\frac{N_{\mathrm{f}}}{(N_{\mathrm{f}}+\gamma )}\right]}^{\beta }$$

(18)

or

$${\sigma }_{\max }={\sigma }_{\mathrm{uT}}{\left[\frac{(N_{\mathrm{f}}+\alpha )}{\alpha }\right]}^{\beta }$$

(19)

where σ_max = applied peak stress, σ_uT = ultimate tensile strength, σ_∞ = fatigue limit, α, β, γ = model fitting parameters, and N_f = number of cycle at failure.

Equation (17) is applicable for both low and high cycles, but Equation (18) is not applicable for high cycles. Equation (19) may be generally applicable without a known fatigue limit. Equations (17) and (18) extend the Basquin model to both the low-cycle and the assumed fatigue limit (σ_∞) regions while Equation (19) extends to the low-cycle region. The Kohout and Vechet model has generally three straight lines to describe the assumed S-N curve in log-log coordinates, including two asymptotes with zero slope for tensile strength and fatigue limit (σ_∞), and a tangent for the region described by the Basquin model. The points of intersection of the tangent with the two asymptotes occur at N_f = α and N_f = γ in the case of Equations (17) and (18). Thus, the parameters α and γ are numbers of cycles at which the S-N curve bends at high and low stresses respectively, and β is the slope of the tangent between the two bends (α and γ) in log-log coordinates. Kohout and Vechet model, therefore, is limited to the predetermined S-N curve. If the bend at a high stress does not exist at some stress ratios, it would not fit experimental data. Also, Equation (19) does not satisfy the initial boundary condition given that N_f should be zero to be σ_max = σ_uT.

To derive an S-N curve, Kim and Zhang [60] quantified the fatigue damage at tensile fatigue failure (D_fT), which satisfies Equation (1):

$$D_{\mathrm{fT}}=1-\frac{{\sigma }_{\max }}{{\sigma }_{\mathrm{uT}}}$$

(20)

and they found that a fatigue damage rate follows

$$\frac{\partial D_{\mathrm{fT}}}{\partial N_{\mathrm{f}}}=\alpha {({\sigma }_{\max })}^{\beta }$$

(21)

where σ_max = applied peak stress, σ_uT = ultimate tensile strength, α, β = model fitting parameters, and N_f = number of cycle at failure.

The number of cycles at failure (N_f) in this equation can be obtained by integration, yielding an S-N curve as a function of applied peak stress (σ_max):

$$N_{\mathrm{f}}=\frac{{\sigma }_{\mathrm{uT}}^{-\beta }}{\alpha (\beta -1)}\left[{\left(\frac{{\sigma }_{\max }}{{\sigma }_{\mathrm{uT}}}\right)}^{1-\beta }-1\right]+N_0$$

(22)

or

$${\sigma }_{\max }={\sigma }_{\mathrm{uT}}{\left(\frac{\alpha (\beta -1)(N_{\mathrm{f}}+N_0)}{{\sigma }_{\mathrm{uT}}^{-\beta }}+1\right)}^{\frac{1}{1-\beta }}$$

(23)

The term N₀ in the equation is to adjust the initial number of cycles for the first cycle failure point according to the quantized analysis—it is not possible to find it with the continuity concept of S-N curve [27]. For example, N₀ = 0.5 cycle at σ_max = σ_uT with R = 0. Thus, the Kim and Zhang model satisfies the initial boundary condition. The fitting parameters for S-N curve in Equations (22) and (23) are identical to those for damage rate with respect to N_f (Equation (21)), which is an advantage for understanding a relation between damage rate and S-N curve. The two parameters (α and β) in Equations (22) and (23) can be determined for a set of fatigue data, given that Equation (21) is numerically,

$$\frac{\Delta D_{\mathrm{f}(i)}}{\Delta N_{\mathrm{f}(i)}}\approx \alpha {({\sigma }_{\max (i)})}^{\beta }$$

(24)

where subscript i = 1, 2, 3,… for different stress levels (σ_max(i)) at failure, ΔD_f(i) = D_f(i-1) − D_f(i) for D_f(i-1) > D_f(i) or |1 − σ_max(i-1)/σ_uT| > |1 − σ_max(i)/σ_uT|, ΔN_f(i) = N_f(i-1) − N_f(i) for N_f(i-1) > N_f(i), and σ_max(i) = (σ_max(i) − σ_max(i-1))/2.

Two different ways may be considered for numerically calculating ΔD_f(i)/ΔN_f(i) and the corresponding σ_max(i). One of the ways is to directly use experimental data points on an S-N plane by choosing a pair of close data points for each value. The other way is to draw the best fit line manually through S-N data points for digitization (http://arohatgi.info/WebPlotDigitizer/app/) and then collect a set of digitized points. Then, log(ΔD_f(i)/ΔN_f(i)) is plotted as a function of log σ_max(i) such that β is the slope and 10^α is an intercept. It is practically efficient with, if necessary, a minimal iteration for the best fit. Figure 2 shows the sequence for plotting the best fit S-N curve.

Kassapoglou [61] statistically derived an S-N curve expression in a form same as Equation (3) for stress ratio (R) ranges, 0 ≤ R ≤ 1 and R > 1, and another S-N curve expression for R < 0 but with more fitting parameters. Thus, Kassapoglou failed to model an S-N curve with one equation for application at different stress ratios. Nonetheless, further, Kassapoglou [62] replaced α in Equation (3) with static strength to have a static strength at N_f = 1 for predicting the remaining fatigue life under spectrum loading.

Kawai and Koizumi [21] assumed an S-N curve in conjunction with a constant fatigue life (CFL) diagram for stress ratio effect research:

$$2N_{\mathrm{f}}=\frac{2}{\alpha }\frac{{\left(1-\frac{{\sigma }_{\max }^{\chi }}{{\sigma }_B}\right)}^{\beta }}{{\left(\frac{{\sigma }_{\max }^{\chi }}{{\sigma }_B}\right)}^{\gamma }}$$

(25)

where $R=\chi ={\sigma }_{\mathrm{uC}}/{\sigma }_{\mathrm{uT}}$, and ${\sigma }_{\max }^{\chi }$ = σ_max at χ.

This equation partially produces negative values for N_f as shown in Figure 3 with the values as used: α = 0.003, β = 1, γ = 8.5, and σ_B = (σ_uT + |σ_uC|)/2. Thus, the fitting capability of this model is low. It has three fitting parameters (α, β, and γ) and one conditional property parameter (σ_B). In other words, if σ_uT < |σ_uC| then σ_B = σ_uC; and if σ_uT > |σ_uC| then σ_B = σ_uT. If a chosen value in this way does not allow a good curve fitting, an alternative value σ_B between σ_uT and |σ_uC| is required. Therefore, the efficiency of curve fitting for modelling is poor because of the number of fitting parameters and conditions imposed. The authors do not suggest a method of how to determine fitting parameters. Kawai and Itoh in 2014 suggested a similar S-N curve expression [22]:

$$2N_{\mathrm{f}}=\frac{1}{\alpha }\frac{<1-\frac{{\sigma }_{\max }^{\chi }}{{\sigma }_{\mathrm{uT}}}{>}^{\beta }}{{(\frac{{\sigma }_{\max }^{\chi }}{{\sigma }_{\mathrm{uT}}})}^{\gamma }<\frac{{\sigma }_{\max }^{\chi }}{{\sigma }_{\mathrm{uT}}}-\frac{{\sigma }_{\max }^{\chi L}}{{\sigma }_{\mathrm{uT}}}{>}^{\delta }}$$

(26)

where ${\sigma }_{\max }^{\chi L}$ = peak stress level at R = −∞.

The angular brackets <> denote the singular function (if it is negative, then zero to avoid negative number of cycles). It seems that they attempted to improve Equation (25) for S-N curve fitting but it cannot be used efficiently for the same reasons.

Major S-N curve models discussed in this section are listed in

Table 1. Summary of selected S-N curve models for data characterization.

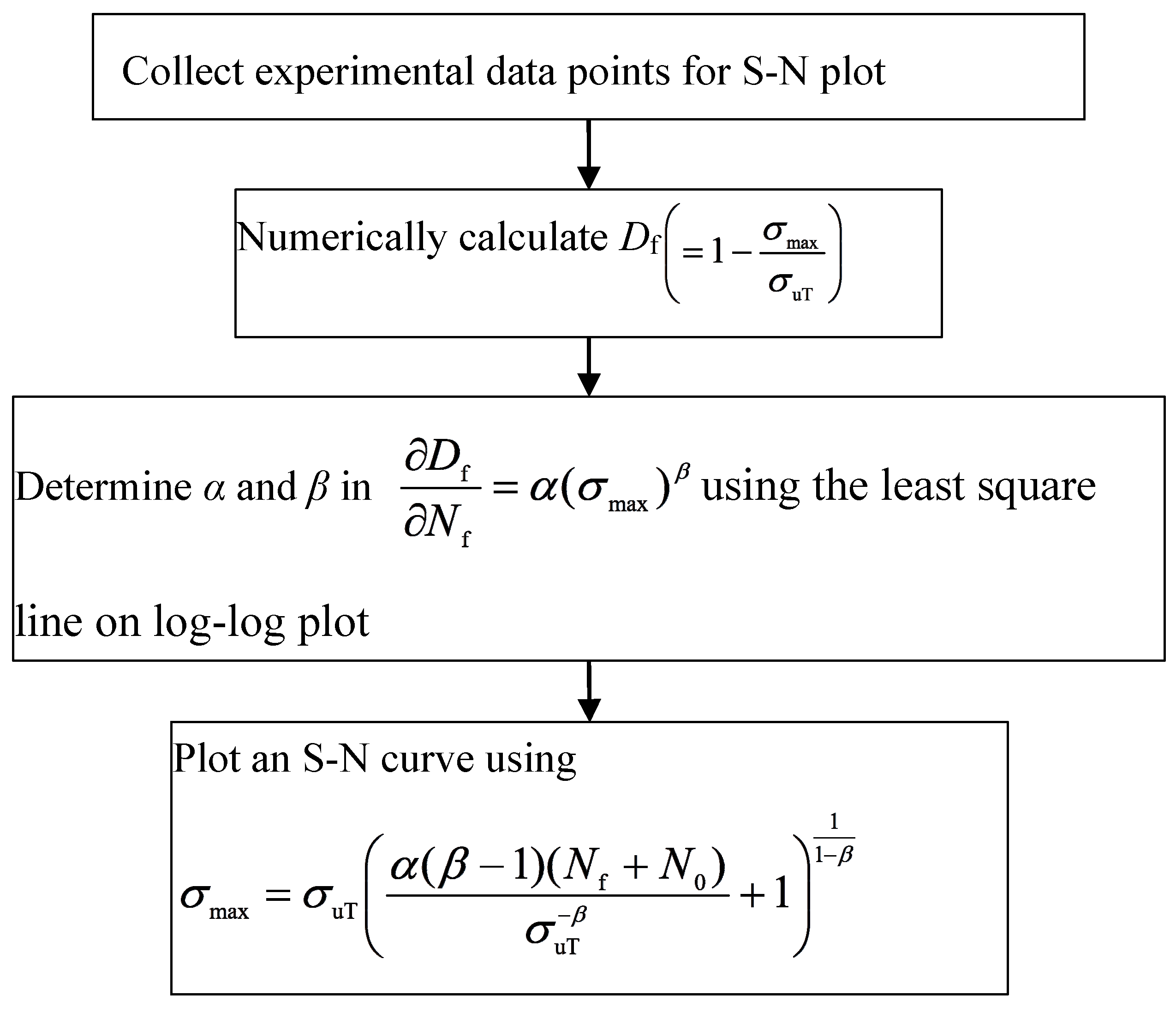
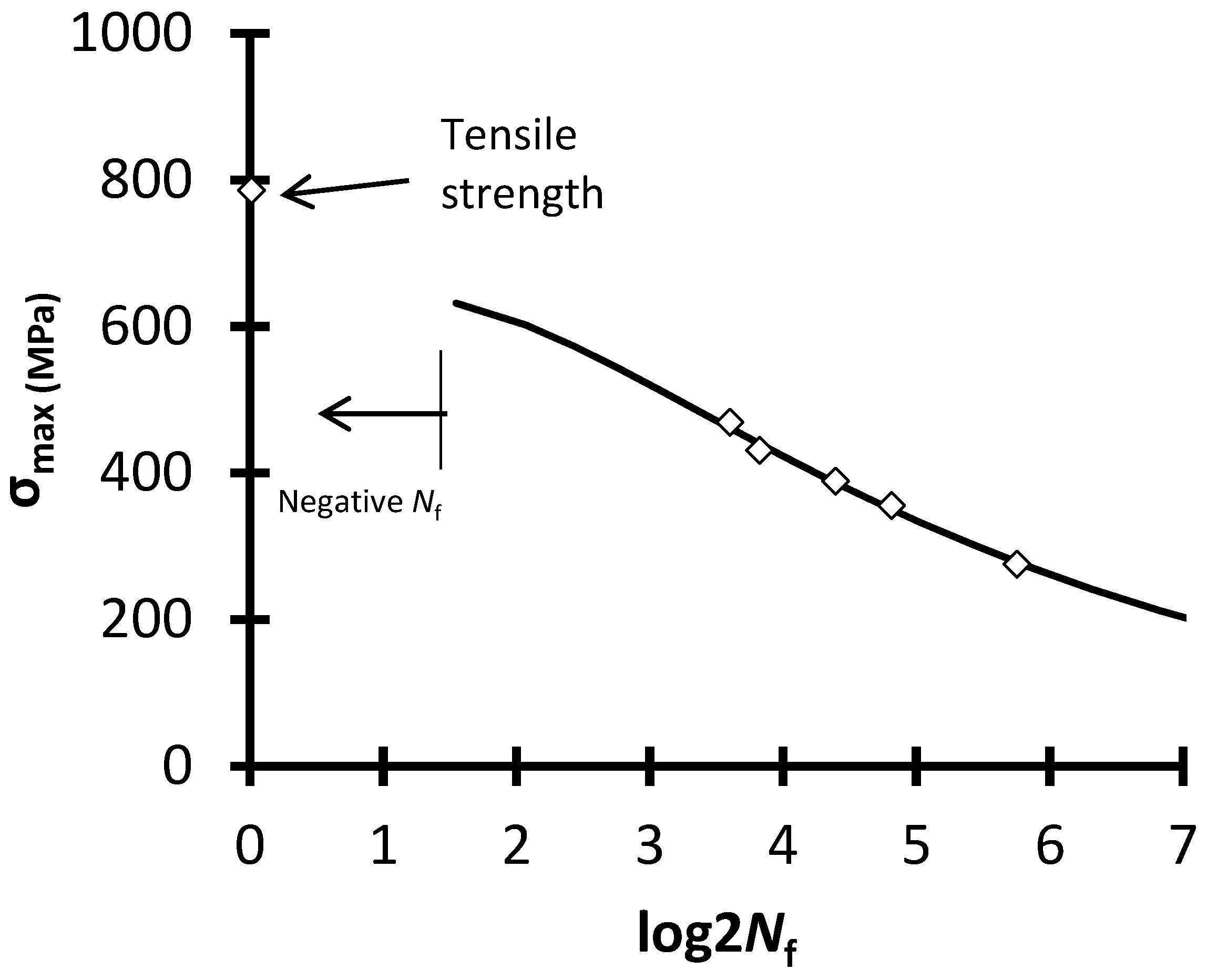
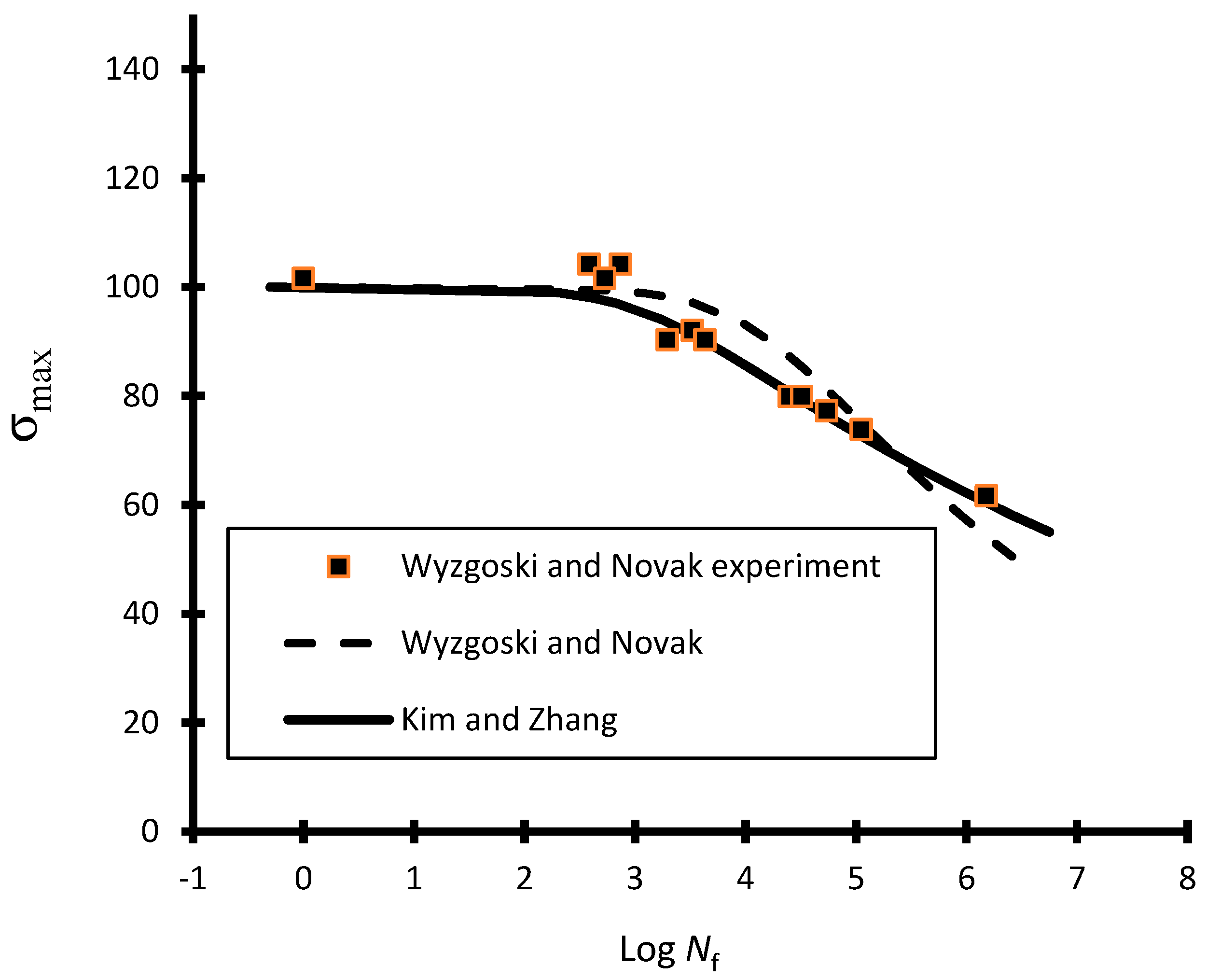
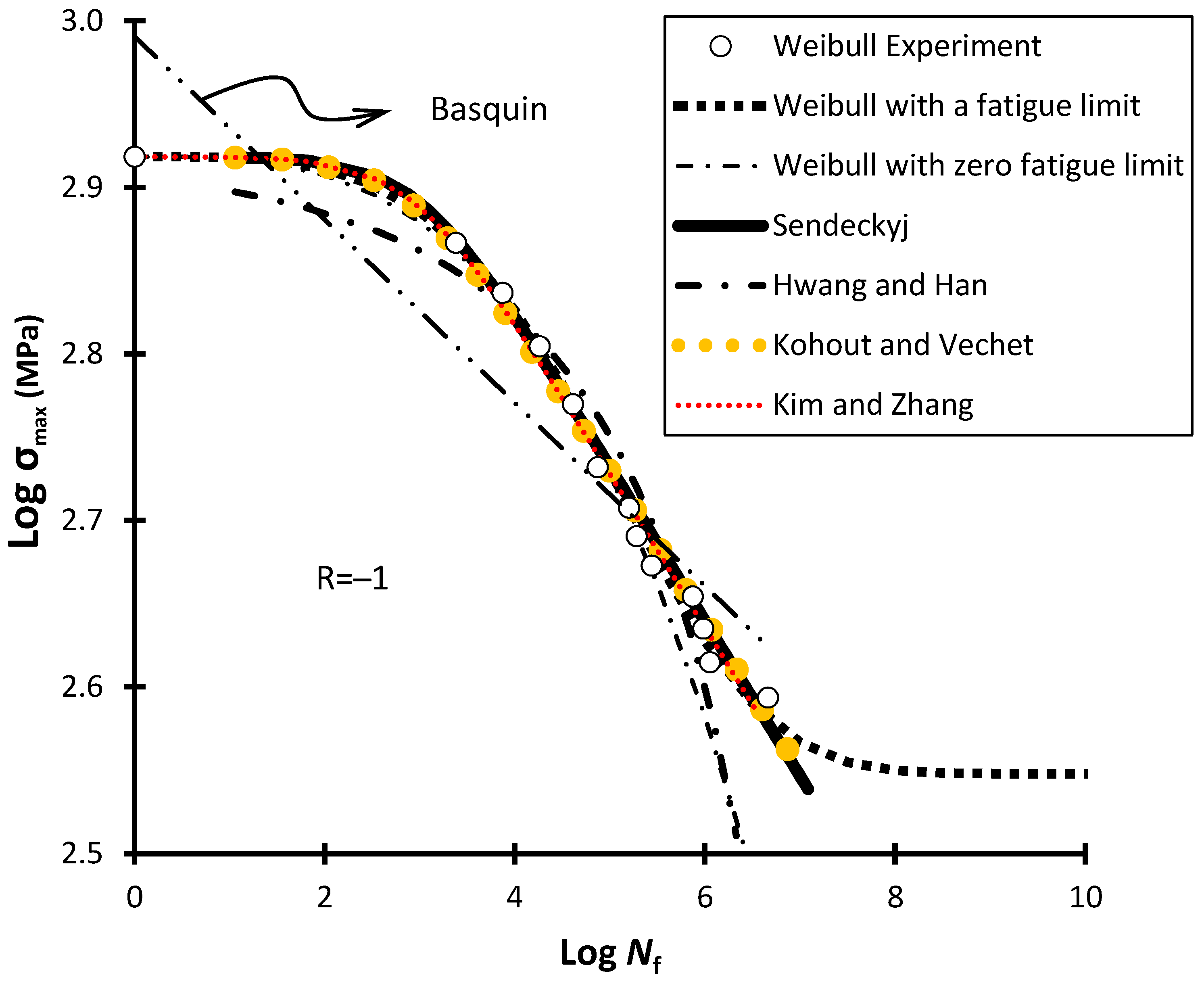
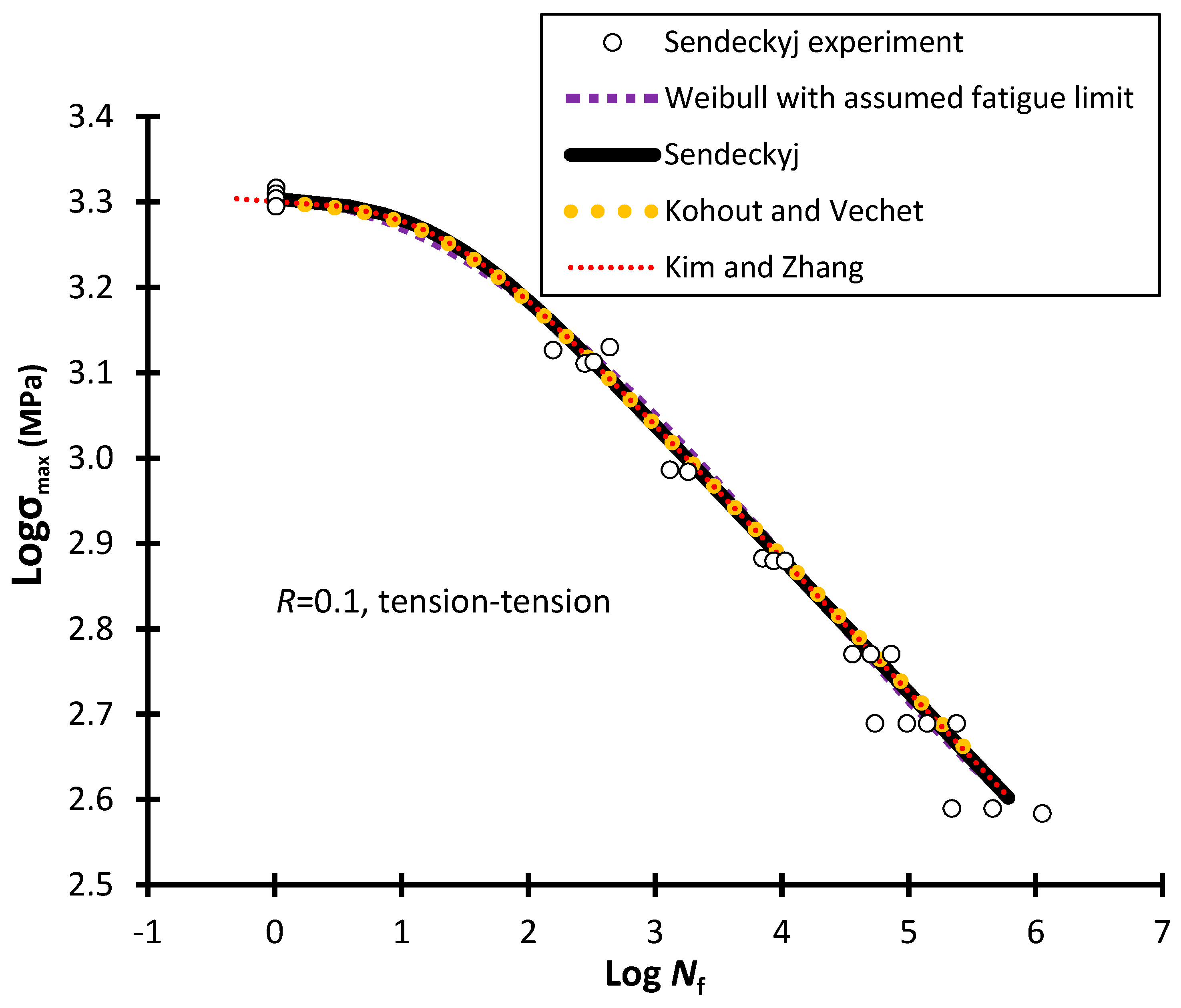
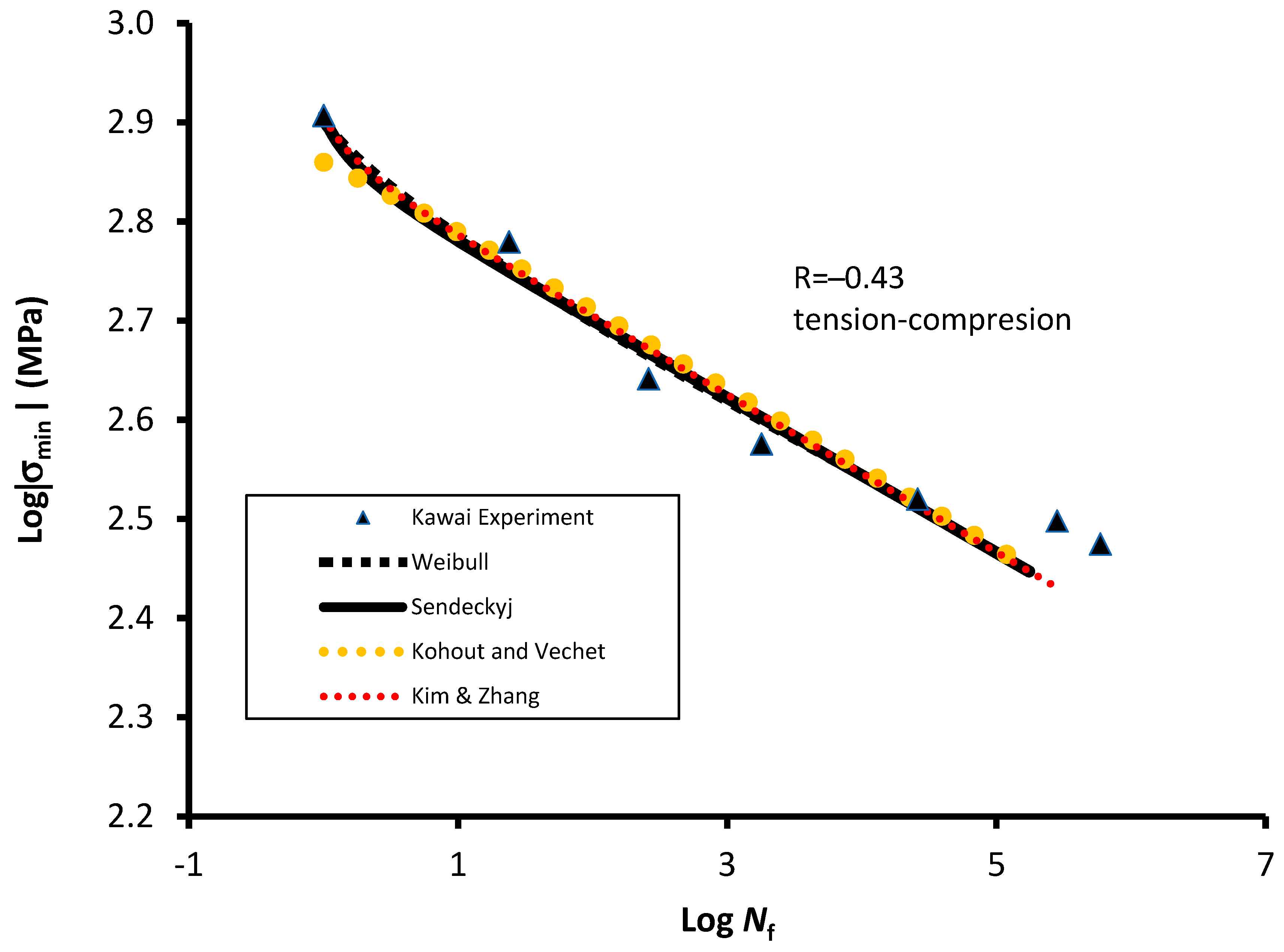
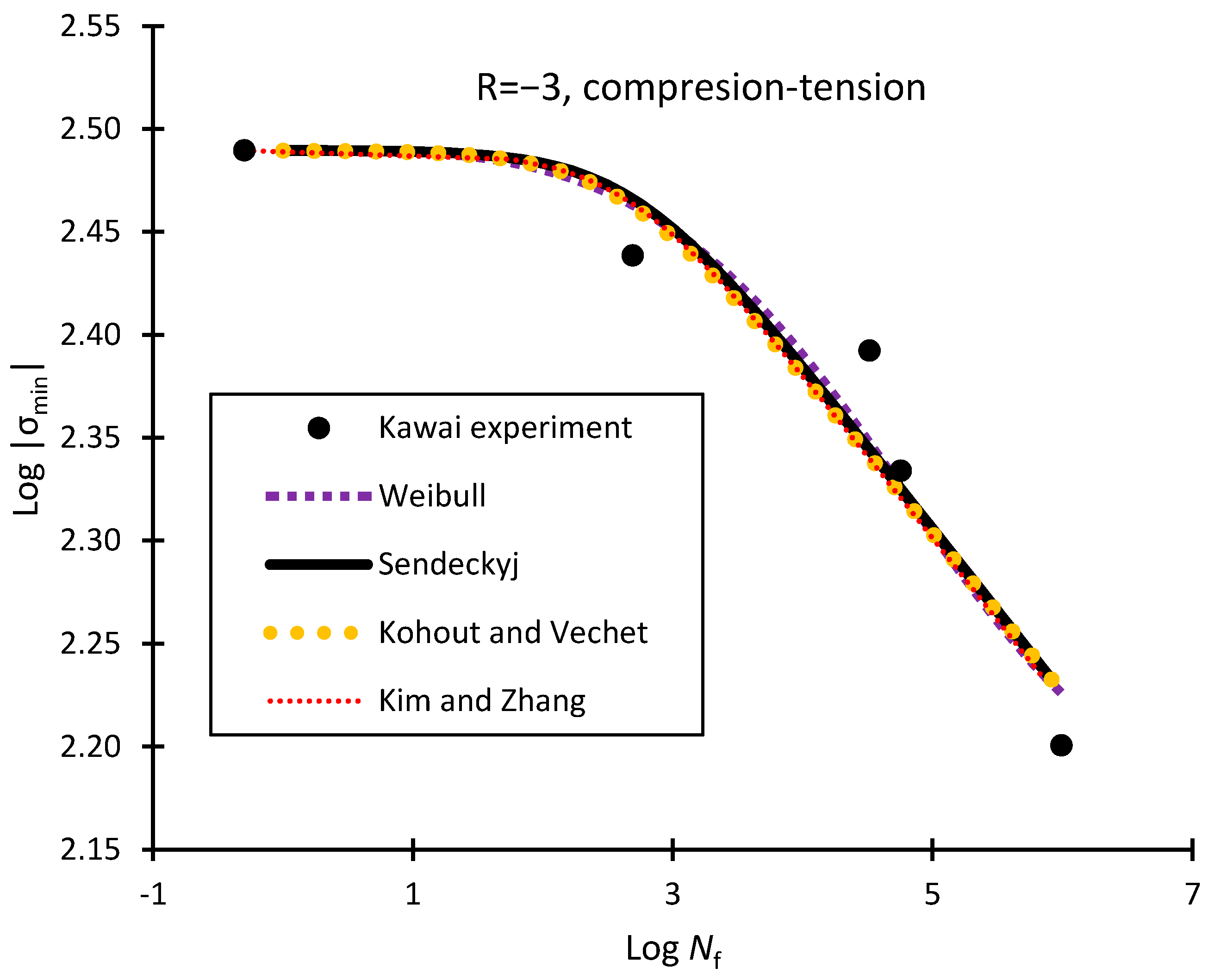
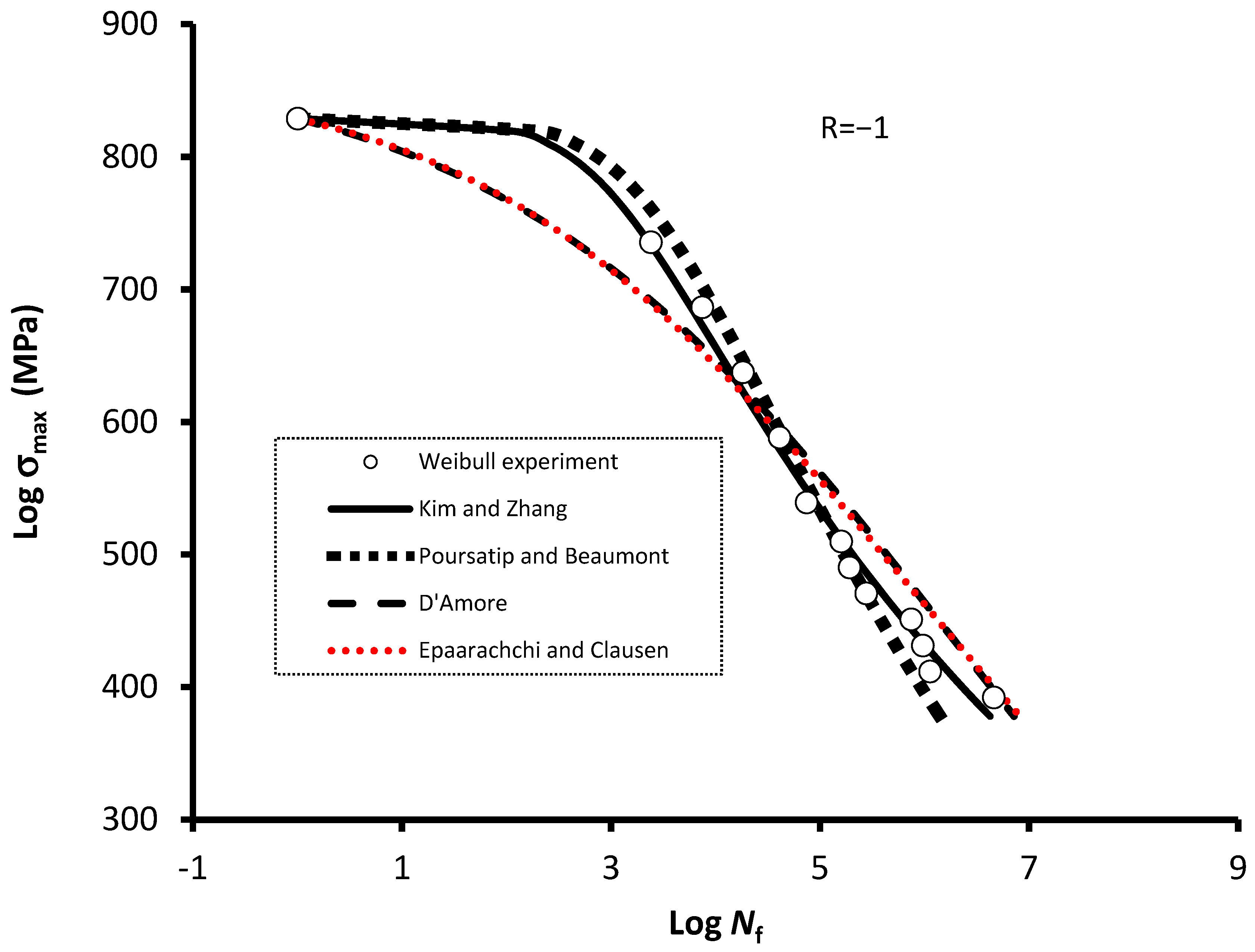
S-N Curve Models	Capability of Curve Fitting	Adaptability to Different Stress Ratios	Relation with Physical Properties			Remarks
			Boundary at Initial Nf	Damage Representation	Satisfaction of $\frac{\partial {\mathit{D}}_{\mathbf{fT}}}{\partial {\mathit{\sigma }}_{\mathbf{max}}}=-\mathit{A}$
Basquin (1910)	Poor	Poor	N/A	N/A	N/A
Stromeyer (1914)	Poor	Poor	N/A	N/A	N/A
Weibull (1952)	Good	Good	Yes	N/A	N/A	Fatigue limit is required
Henry (1955)	Poor	N/A	N/A	N/A	N/A
Marin (1962)	Poor	N/A	N/A	N/A	N/A	Same form as Basquin model.
Sendeckyj (1981)	Good	Good	Yes	N/A	N/A
Hwang and Han (1986)	Poor	Poor	No	N/A	N/A
Kohout and Vechet (2001) Equation (19)	Good	Good	No	N/A	N/A	With limited curve shaping (e.g., R = −0.43)
Kim and Zhang (2001)	Good	Good	Yes	Yes	Yes

and summarized with criteria for further discussion below.

3.2. As a Function of Stress Ratio

Some S-N curve models for stress ratio effect have been derived in relation to fatigue damage. One of reasons for this approach is that once fatigue damage is generally quantified, an S-N curve can possibly be found because S-N curve represents a special case of fatigue damage at failure as discussed above. Another reason is that the remaining fatigue life would be predictable if a valid damage function (D) is found. For these reasons, if damage is arbitrarily quantified, an S-N curve derived may not necessarily be capable of representing the fatigue data.

Poursartip et al. [25] defined a delamination damage of laminated composites as delaminated area divided by the total interfacial area, and subsequently determined the damage at failure (D_f) for a carbon fiber reinforced laminate made of 8 plies [45/90/-45/0/0/-45/90/45] with an average tensile strength of 586 MPa:

$$D_{\mathrm{f}}=2.857\left(1-\frac{{\sigma }_{\max }}{{\sigma }_{\mathrm{uT}}}\right)$$

(27)

where σ_max = applied peak stress, and σ_uT = ultimate tensile strength.

Equation (27) satisfies Equation (1) which is one of damage validity conditions. Further, they measured the stiffness reduction rate at R = 0.1 and calculated dD/dN by fitting the least square line to be

$$\frac{dD}{dN}=9.189\times {10}^{-5}{\left(\frac{\Delta \sigma }{{\sigma }_{\mathrm{uT}}}\right)}^{6.393}$$

(28)

where Δσ = applied stress range, σ_uT = ultimate tensile strength, and N = Number of cycles before failure.

(Please note that D here is not at the failure.) Equation (28) could have been more useful for characterizing damage on the S-N plane if they found a valid function of D, but it is only for the linear part of experimental stiffness reduction-cycle curves. Also, it did not consider the compatibility conditions for fatigue damage (D) [27]. Poursatip and Beaumont [26] subsequently proposed an S-N curve for stress ratio (R) effect based on an empiricism with Equation (28),

$$N_{\mathrm{f}}=3.108\times {10}^4{\left(\frac{{\sigma }_{\max }(1-R)}{{\sigma }_{\mathrm{uT}}}\right)}^{-6.393}{\left(1.222\frac{1-R}{1+R}\right)}^{\gamma }\left(1-\frac{{\sigma }_{\max }}{{\sigma }_{\mathrm{uT}}}\right)$$

(29)

where σ_max = applied peak stress, σ_uT = ultimate tensile strength, γ = fitting parameter, N_f = number of cycle at failure, and R = stress ratio.

Equation (29) can be simplified by converting R into a constant for evaluating S-N curve performance,

$$N_{\mathrm{f}}=\alpha {\left(\frac{{\sigma }_{\max }}{{\sigma }_{\mathrm{uT}}}\right)}^{-6.393}\left(\frac{{\sigma }_{\mathrm{uT}}-{\sigma }_{\max }}{{\sigma }_{\mathrm{uT}}}\right)$$

(30)

The fitting parameter α in Equation (29) does not seem to be clearly linked to the damage rate in Equation (28) although the value 6.393 in Equation (30) indicates some correspondence. Also, Equation (30) has only one fitting parameter so that it may be efficient for parameter determination if it fits data well.

Some S-N curve models simply assumed a residual strength function as the fatigue damage (D) while the prediction of the residual strength had been a single research topic by many researchers for fatigue damage [48,63,64,65,66]. D’Amore et al. [67] surmised a power law equation for a change rate of residual strength with respect to number of cycles for calculation and it was simply regarded as being identical to,

$$\frac{dD}{dN}=\alpha N^{-\beta }$$

(31)

where α, β = model fitting parameters, and N = number of cycle before failure.

The validity of Equation (31) is not known. It was followed by incorporating the stress ratio (R) with another surmise that one of the constants for the change rate of residual strength with respect to N is proportional to Δσ to have:

$$N_{\mathrm{f}}={\left[1+\frac{1}{\alpha (1-R)}(\frac{{\sigma }_{\mathrm{uT}}}{{\sigma }_{\max }}-1)\right]}^{1/\beta }$$

(32)

or

$${\sigma }_{\max }=\frac{{\sigma }_{\mathrm{uT}}}{\alpha (N_{\mathrm{f}}^{\beta }-1)(1-R)+1}$$

(33)

where σ_max = applied peak stress, σ_uT = ultimate tensile strength, N_f = number of cycle at failure, and R = stress ratio.

Taking R as a constant for S-N curve performance, we have

$$N_{\mathrm{f}}={\left[\frac{{\sigma }_{\mathrm{uT}}-{\sigma }_{\max }}{{\alpha }^{\prime }{\sigma }_{\max }}+1\right]}^{1/\beta }$$

(34)

where σ_max = applied peak stress, σ_uT = ultimate tensile strength, α′, β = model fitting parameters, and N_f = number of cycle at failure.

A value given by (σ_uT/σ_max − 1)/(1 − R) in Equation (32) was experimentally found to be constant for R = 0.1–0.7, allowing us to determine α and β ratios using data sets from different stress ratios. For a constant stress ratio, two fitting parameters need to be determined for an S-N curve. Caprino and Giorlea in 1999 [68] further experimentally verified this for R = 10 and 1.43. Equation (34) allows the initial boundary condition to be N_f = 1 at σ_uT and the fitting parameters have a relation with Equation (31) for damage.

Epaarachchi and Clausen [69] followed an approach similar to that of D’Amore et al. [67]. They used Equation (31) to propose an S-N curve model not only as a function of stress ratio but also as a function of loading frequency (and fiber orientation angle of composites):

$${\sigma }_{\mathrm{uT}}-{\sigma }_{\max }=\alpha {\left(\frac{{\sigma }_{\max }}{{\sigma }_{\mathrm{uT}}}\right)}^{0.6}{\sigma }_{\max }{(1-R)}^{1.6}\frac{1}{{\mathrm{f}}^{\beta }}(N_{\mathrm{f}}^{\beta }-1)$$

(35)

where σ_max = applied peak stress, σ_uT = ultimate tensile strength, α, β = model fitting parameters, f = loading frequency, N_f = number of cycle at failure, and R = stress ratio.

If this equation is simplified for S-N curve performance, it becomes

$$N_{\mathrm{f}}={\left(\frac{{\sigma }_{\mathrm{uT}}-{\sigma }_{\max }}{{\alpha }^{\prime }{\left({\sigma }_{\max }\right)}^{1.6}}+1\right)}^{1/\beta }$$

(36)

where α′ = model fitting parameter, which is not much different from D’Amore model Equation (34). The only difference from Equation (34) is in an exponent 1.6. Later, Epaarachchi [70] demonstrated that the model Equation (35) proposed by Epaarachchi and Clausen is not much capable of predicting S-N curve at high stress ratios unless so called “fatigue-static-fatigue curve” algorithm is used for fitting.

3.3. Associated with Stress Intensity Factor

Wyzgoski and Novak [71] attempted to relate the S-N curve with the initial and final flaw sizes using the Paris equation [72] given by

$$\frac{da}{dN}=A{\left(\Delta K\right)}^m$$

(37)

where ΔK = stress intensity factor range (=YΔσa^1/2), and A and m = constants.

They integrated this equation with limits for the final crack length (a_f) and initial crack length (a₀) assuming that geometry factor Y is constant:

$$N_{\mathrm{f}}=\frac{2}{(m-2)A{Y}^m{(\Delta \sigma )}^m}\left(\frac{1}{a_0^{\frac{(m-2)}{2}}}-\frac{1}{a_{\mathrm{f}}^{\frac{(m-2)}{2}}}\right)$$

(38)

where Δσ = stress range.

To improve the predictability of Equation (38), Wyzgoski and Novak [73] further adopted a relation between a_f and a₀ with a_f = a₀ for the first cycle fracture and a_f = t for a low stress fracture:

$$a_{\mathrm{f}}=t\exp \left[-\frac{\ln (t)-\ln (a_0)}{{\sigma }_{\mathrm{uT}}}\frac{\Delta \sigma }{1-R}\right]$$

(39)

where R = stress ratio.

Please note that they eliminated a_f in Equation (38) for Equation (39). The initial crack length (a₀) in Equation (38) was regarded as a constant independent of applied stress level. The initial crack length a₀ may not be conceptually applicable for continuous fiber reinforced composites with a multiple crack damage pattern. However, the initial crack length (a₀) may be regarded as an equivalent initial crack to the damage consisting of multiple cracks, given that the S-N curve behavior pattern may be for both single and multiple crack damages for un-notched specimens. The Wyzgoski and Novak model may be useful for providing an insight into internal damage and understanding S-N curve in relation to the stress intensity factor. At the same time, it should be noted that the stress intensity factor is limited to the tensile stress, implying that applicability of this model is not certain for stress ratios involving compressive stress, and may not be practical for S-N curve fitting due to the number of fitting parameters if A and m are treated as the fitting parameters. Figure 4 shows experimental data for injection molded PBT reinforced with 30w% short glass fibers [73] (obtained at R = 0.1 with σ_uT = 100 MPa, specimen thickness (t) = 0.0032 m) in comparison with an S-N curve plotted in dashed line using Equation (38) for: Paris equation constants A = 1.53 × 10⁻¹⁰ and m = 7.41, geometry factor Y = 1.17 for a semi-circular surface flaw [74], initial flaw size (a₀) = 0.354 mm. Also, another S-N curve plotted with Kim and Zhang model Equation (22) or (23) with α = 4.9 × 10⁻³⁵ and β = 15.028 is shown in solid line. The Wyzgoski and Novak model appear reasonable for curve fitting although it is not as accurate as the Kim and Zhang model (see below for capability).

4. Evaluation of S-N Curve Model Capability

Experimental data obtained at five different stress ratios i.e., R = −1, 0.1, −0.43, −3, and 10, which are available in the literature, will be used for S-N curve fitting evaluation. The stress ratio R = −1 was obtained from the rotating beam test which is readily implementable compared to other stress ratios to represent the loading between T-C and C-T (Figure 1). The other stress ratios: R = 0.1 may represent the tension-tension (T-T) loading for a range R = 0–1; R = −0.43 the tension-compression (T-C) loading for range R = 0–−1; R = −3 the compression-tension (C-T) loading for a range R =−1–−∞; and R = 10 the compression-compression (C-C) loading for a range R = −∞–1. When an S-N curve model involves compressive stress and failure, σ_max will be replaced with |σ_min|, and σ_uT with |σ_uC| for evaluation.

4.1. S-N Curve Models for Data Characterisation

S-N curve models with more than two fitting parameters were eliminated from evaluation because its implementation is not economical, involving unnecessary efforts, given that some models available with two parameters are sufficiently accurate for data fitting. Some other models, fitting capabilities of which are poor due to the obvious limitation for curve shaping, were also eliminated from evaluation. For example, S-N curve models represented by Equations (4) and (5) are not much different from Basquin model Equation (3). Experimental data and plots will be in log-log coordinates, which are convenient for the Kohout and Vechet model Equation (19) in comparing with other models. Equations (17) and (18) were not employed here because they contain three fitting parameters with a fatigue limit.

An experimental data set for Bofors FR 76 Steel with σ_uT = 829 MPa obtained from Weibull [18] with 111 rotating beam tests at R = −1 is plotted in Figure 5 with data points having a probability of 50% failure. Two curves from the Weibull model Equation (8) with and without fatigue limit (σ_∞) for respective two sets of fitting values—α = 21 × 10⁻⁴ and β = 3.8 with an assumed σ_∞ = 353 MPa (provided by Weibull), and α = 0.003 and β = 3.1 with an assumed σ_∞ = 0 (to see if there would be any difference)—are plotted in comparison with other S-N models to be discussed. (Please note that the plotted lines from some of models are overlapped and therefore not clearly distinguishable.) The capability of fitting with a fatigue limit (σ_∞) appears to be very good but, with σ_∞ = 0, it is relatively poor at a high N_f. The best fit with the fatigue limit by Weibull model may be regarded as the reference for comparisons with other S-N curves. Plots generated by other S-N models are shown with: α = 0.001 and β = 0.093 for Sendeckyj model Equations (11) and (12); α = 35 and β = 0.21 for Hwang and Han model Equations (13) and (14); α = 776.25 and β = −0.0895 for Kohout and Vechet model Equation (19); and α = 10^−38.44 and β = 11.809 for Kim and Zhang model Equations (22) and (23) with N₀ = 0.5. The fitting capabilities for models by Weibull, Sendeckyj, Kohout and Vechet, and Kim and Zhang appear to be practically identical to each other and may be regarded as being sufficiently good for practical data characterization. In contrast, the fitting capability of Basquin model Equation (3) is found to be poor as expected when σ_uT is included in data despite the fact that it has still been used by many researchers (e.g., refs. [75,76,77] to name a few). The fitting capability of Hwang and Han model appears to be also poor because of its limited capability—as the α value increases in Equations (13) and (14), σ_max approaches the ultimate strength at N_f = 1 but other part of the curve was found to deviate increasingly from the experimental data. A reasonable fit of Hwang and Han model can only be achieved by omitting the data points near the ultimate strength. The Basquin, and Hwang and Han models were accordingly eliminated from further comparison with other models at other stress ratios.

For T-T loading at R = 0.1, an experimental data set consisting of 28 data points obtained from Sendeckyj [19] for glass fiber reinforced epoxy composite with σ_uT = 2013 MPa is given in Figure 6. Weibull model Equation (8) was plotted with α = 0.088 and β = 1.9 for an assumed fatigue limit of 250 MPa, Sendeckyj model Equations (11) and (12) with α = 0.0485 and β = 0.157, Kohout and Vechet model Equation (19) with α = 19.953 and β = −0.155, and Kim and Zhang model Equations (22) and (23) with α = 3.13 × 10⁻²⁷ and β = 7.381 for N₀ = 0.5. The S-N curve of Sendeckyj model may be regarded as the best fit as already demonstrated in [19]. The fitting capabilities of the four models still appear to be equally good and practically identical to each other.

Figure 7 shows fatigue data obtained from Kawai and Itoh [22] for carbon fiber reinforced epoxy laminates with σ_uC = 807 MPa and σ_uT = 1887 MPa for T-C loading at R = −0.43. For comparison purposes of the models, the original experimental data point for N_f = 0.5 at σ_uC was changed to N_f = 1 because of the sensitivity of the S-N curve models to the experimental data point for the initial N_f at σ_uC also because Sendeckyj and Weibull models are designed to take N_f = 1 at σ_uC although Kohout and Vechet model Equation (19) is to take N_f = 0 at σ_uC while Kim and Zhang model is capable of taking N_f = 1 cycle at σ_uC as well, (Note N_f = 0 is not possible on logarithmic coordinate as discussed earlier.) Accordingly, N₀ in Kim and Zhang model Equations (22) and (23) was set to be 1. As a result, the model fitting parameters were found to be α = 2.5 × 10⁻¹ and β = 0.83 for Weibull model Equation (8) with an assumed σ_∞ = 0, α = 4.485 and β = 0.078 for Sendeckyj model Equations (11) and (12), α = 0.35and β = −0.08 for Kohout and Vechet model Equation (19), and α = 2.884 × 10⁻¹⁶⁰ and β = 54.388 for Kim and Zhang model Equation Equations (22) and (23). The fitting capabilities of the three models (i.e., Weibull, Sendeckyj, and Kim and Zhang) appear equally good and practically identical. However, as discussed earlier, Kohout and Vechet model Equation (19) is found to be not capable of satisfying the boundary condition at σ_uC not because of the experimental data point adjusted to be N_f = 1 at σ_uC but because of the predetermined S-N curve shape as seen near the first cycle (at log N_f = logα = log0.35 = −0.4559 for the bend) although the degree of deviation from other models is not significant in this case. If the fatigue data point more rapidly decreases at low cycles, the Kohout and Vechet S-N curve would have more tendency of deviating from the data for the same reason. Also, the points of intersection of the tangent with the two asymptotes at log N_f = logα for the bend of S-N curve is arbitrary to some extent.

For C-T loading at R = −3, Figure 8 shows fatigue experimental data obtained from Kawai and Itoh [22] for carbon fiber reinforced epoxy laminates with σ_uC = 807 MPa and σ_uT = 1887 MPa in comparison with plots from the various S-N curve models. The S-N line shape tendency is similar to those in Figure 5 and Figure 6 such that the fitting capabilities of all the models are again equally good without much sensitivity for the initial N_f at σ_uC. In the case of Kohout and Vechet model Equation (19), when α is high for the bend of S-N curve, the sensitivity to the initial experimental point N_f at σ_uC decreases as the σ_min substantially approaches the σ_uC. The model fitting parameters without adjusting the initial experimental point N_f = 0.5 at σ_uC were found to be α =3.5 × 10⁻³ and β = 3.59 for Weibull model Equation (7) with an assumed σ_∞ = 150 MPa, α = 0.001985 and β = 0.08 for Sendeckyj model Equations (11) and (12), α = 398 and β = −0.0775 for Kohout and Vechet model Equation (19), and α = 4.295 × 10⁻³⁸ and β = 13.506 with N₀ = 0.5 for Kim and Zhang model.

For C-C loading at R = 10, an experimental data set obtained from Kawai and Itoh [22] for carbon fiber reinforced epoxy laminates with σ_uC = 807 MPa, (σ_uT = 1887 MPa) is shown in Figure 9. For comparison purposes of the models, an experimental data point is adjusted again to be N_f = 1at σ_uC for the same reason in the case of R = −0.43 shown in Figure 7 for Weibull, and Secdeckyj models except Kohout and Vechet model Equation (19). Also, N₀ in Kim and Zhang model Equations (22) and (23) was set to be 1. The model fitting parameters were found to be α = 0.138 and β = 0.66 for Weibull model Equation (7) with an assumed σ_∞ = 185 MPa, α = 1.3 and β = 0.019 for Sendeckyj model Equations (11) and (12), α = 1and β = −0.02 for Kohout and Vechet model Equation (19), and α = 2.88 × 10⁻¹⁶⁰ and β = 54.388 for Kim and Zhang model with N₀ = 1. The fitting capabilities of all the models appear to be equally good and practically identical indicating that all the models can fit the approximate straight line. However, the inherent nature of Kohout and Vechet model from the predetermined S-N curve is again detectable due to the pre-determined points of intersection of the tangent with the two asymptotes at logN_f = logα = log1 = 0 for the bend of S-N curve of the model.

Table 1 lists selected S-N curve models with two or less fitting parameters for data presentation. The performances of major S-N curve models among those may be summarized as follows: (a) the three models proposed by Weibull, Sendeckyj, and Kim and Zhang have the highest fitting capabilities; (b) Kohout and Vechet model has a limited capability of S-N curve line shaping at some stress ratios although its capability, otherwise, is approximately equal to the three models; (b) Sendeckyj, and Kim and Zhang models have an advantage over Weibull model for fitting efficiency given that Weibull model needs an additional assumed fatigue limit for curve fitting; and (c) the Kim and Zhang model has an advantage over Sendeckyj model for representability of fatigue damage in which the fitting parameters serve as the damage parameters as well.

Table 2. Summary of S-N curve models for data characterization proposed by Weibull, Sendeckyj, Kohout and Vechet, and Kim and Zhang.

Experimental Data	Loading	S-N Curve Models	Fitting Parameters
			α	β	σ∞ (MPa)	N0
Weibull (1952) [18] with σuT = 829 MPa	R = −1 for T-C or C-T loading	Weibull (1952) ${\sigma }_{\max }=\left({\sigma }_{\mathrm{uT}}-{\sigma }_{\infty }\right)\exp \left[-\alpha {(\log N_{\mathrm{f}})}^{\beta }\right]+{\sigma }_{\infty }$	2.1 × 10−5	3.8	353	-
			0.003	3.1	0	-
		Sendeckyj (1981) ${\sigma }_{\max }={\sigma }_{\mathrm{uT}}/{(1-\alpha +\alpha N_{\mathrm{f}})}^{\beta }$	0.001	0.093	-	-
		Kohout and Vechet (2001) ${\sigma }_{\max }={\sigma }_{\mathrm{uT}}{\left[(N_{\mathrm{f}}+\alpha )/\alpha \right]}^{\beta }$	776.25	−0.090	-	-
		Kim and Zhang (2001) $N_{\mathrm{f}}={\sigma }_{\mathrm{uT}}^{-\beta }/[\alpha (\beta -1)]\left[{\left({\sigma }_{\max }/{\sigma }_{\mathrm{uT}}\right)}^{1-\beta }-1\right]+N_0$	10−38.44	11.81	-	0.5
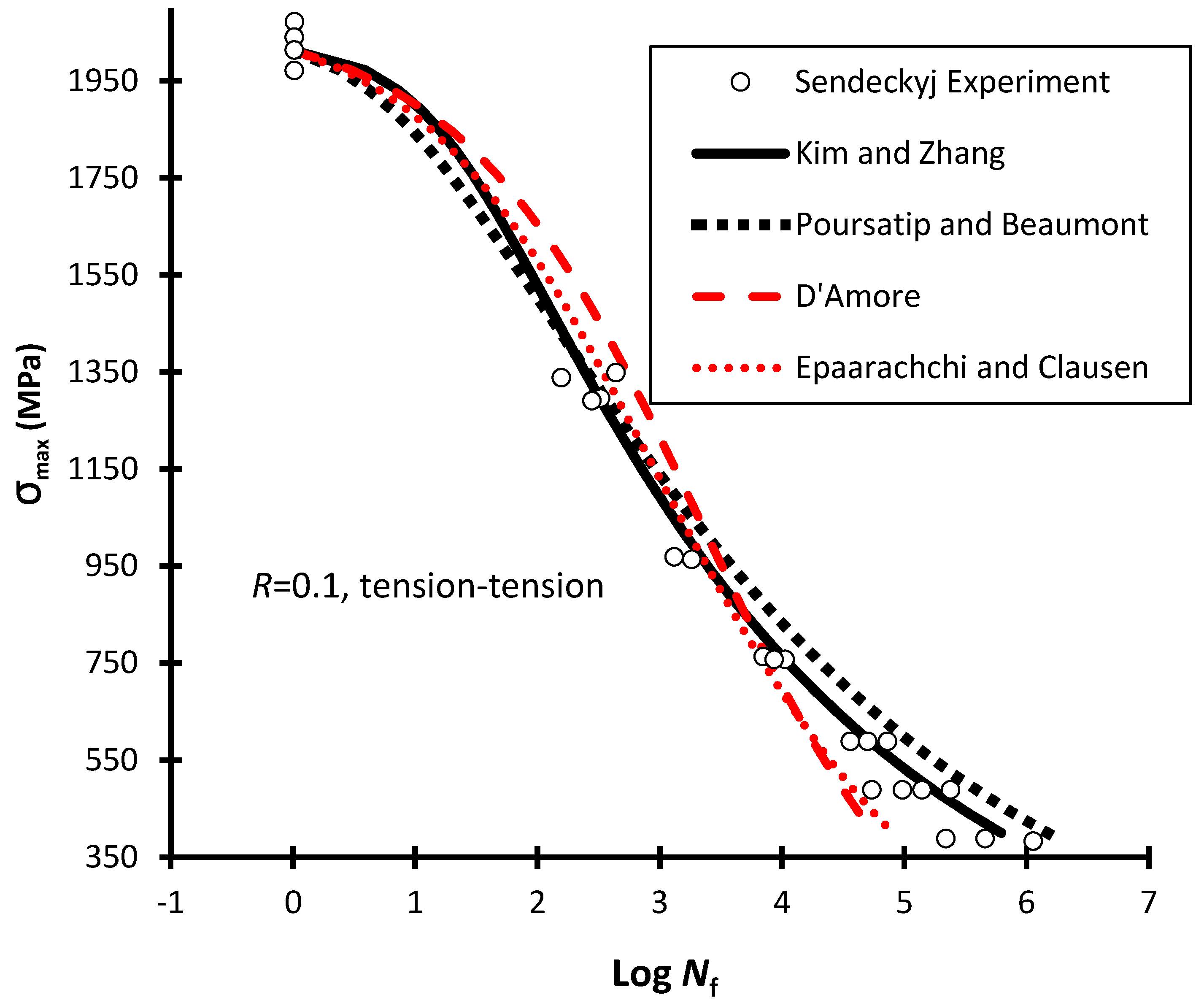
Sendeckyj (1981) [19] σuT = 2013 MPa	R = 0.1 for T-T loading	Weibull (1952)	0.088	1.9	250	-
		Sendeckyj (1981)	0.0485	0.157	-	-
		Kohout and Vechet (2001)	19.953	−0.155	-
		Kim and Zhang (2001)	3.13 × 10−27	7.381	-	0.5
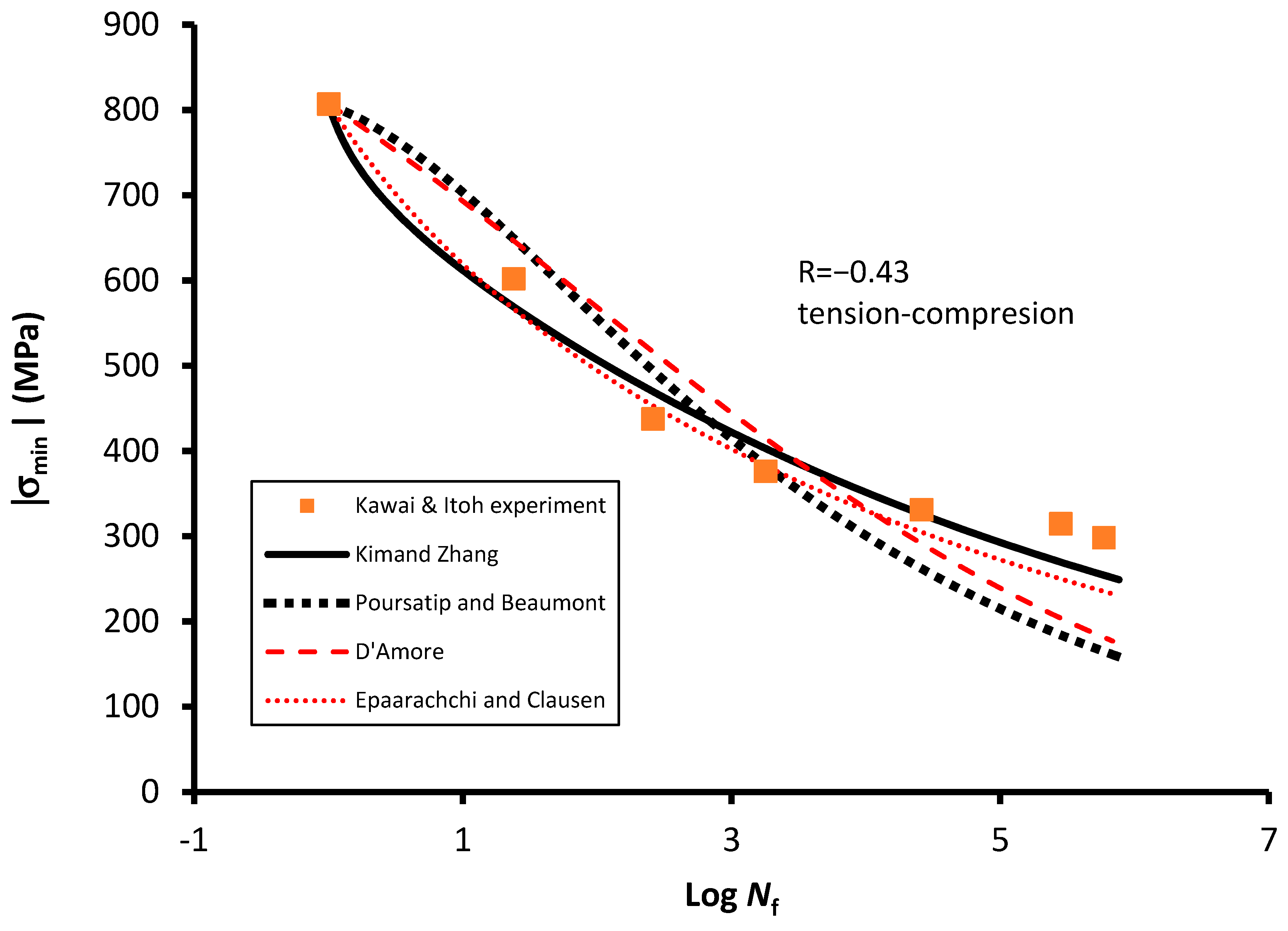
Kawai and Itoh (2014) [22] with σuC = 807 and σuT = 1887 MPa	R = −0.43 for T-C loading	Weibull	2.5 × 10−1	0.83	0	1
		Sendeckyj (1981)	4.485	0.078	-	1
		Kohout and Vechet (2001)	0.35	−0.08	-	-
		Kim and Zhang (2001)	2.88 × 10−160	54.39	-	1
Kawai and Itoh (2014) [22] with σuC = 807MPa and σuT = 1887 MPa	R = −3 for C-T loading	Weibull (1952)	3.5 × 10−3	3.59	150	-
		Sendeckyj (1981)	1.985 × 10−3	0.08	-	-
		Kohout and Vechet (2001)	398.1	−7.75 × 10−2	-	-
		Kim and Zhang (2001)	4.295 × 10−38	13.506	-	0.5
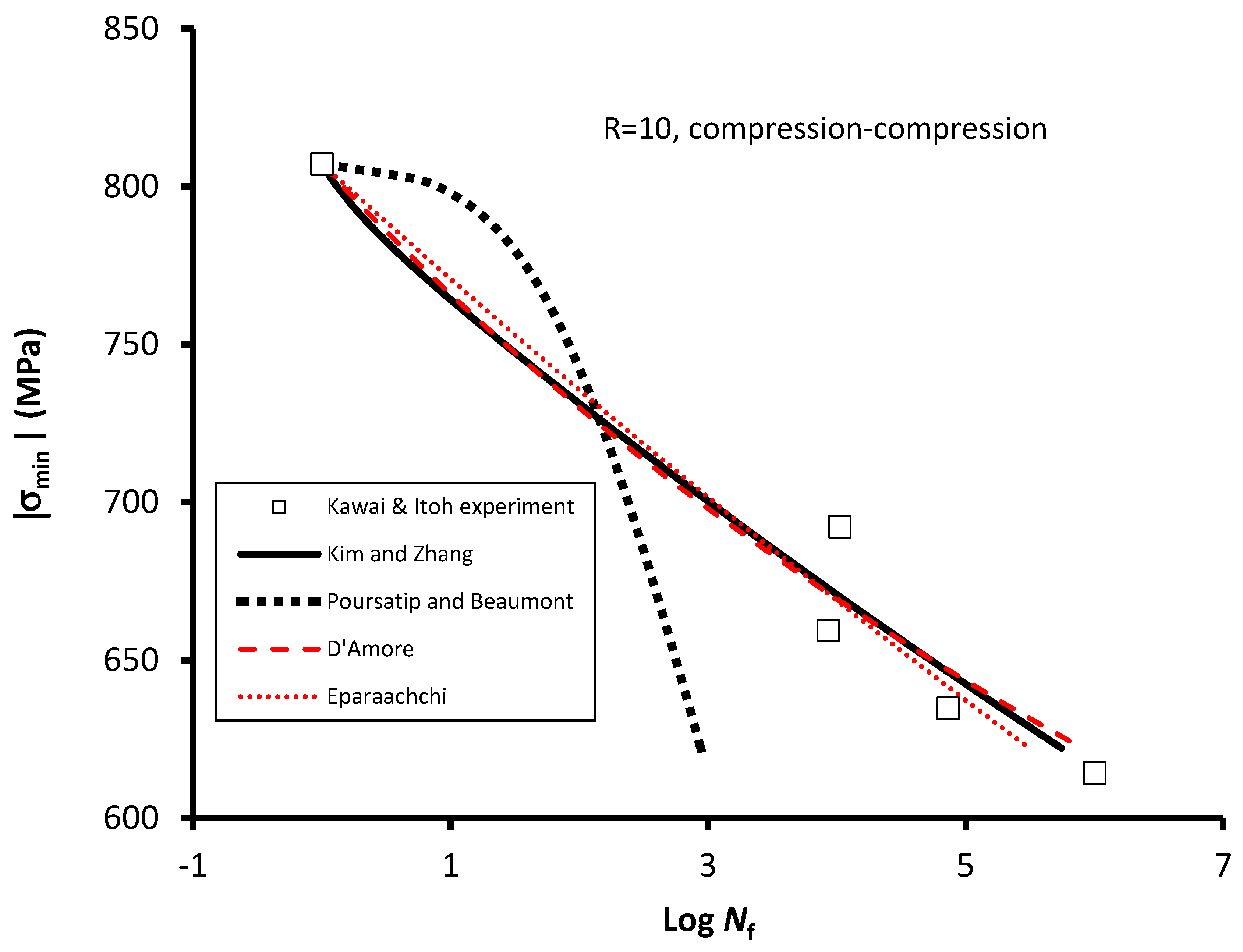
Kawai and Itoh (2014) [22] with σuC = 807 MPa and σuT = 1887 MPa	R = 10 for C-C loading	Weibull (1952)	0.138	0.66	185	1
		Sendeckyj (1981)	1.3	0.019	-	1
		Kohout and Vechet (2001)	1	−0.02	-	-
		Kim and Zhang (2001)	2.88 × 10−160	54.388	-	1

lists the four models with parameter values for different stress ratios.

Table 3. Citations of S-N curve models available in the Google Scholar as of 23 May 2018.

S-N Models	Google Scholar Citations	Citation Rates/Year
Basquin (1910)	1311	12
Weibull (1952)	14	0.21
Sendeckyj (1981)	111	3.0
Kohout and Vechet (2001)	73	4.3
Kim and Zhang (2001)	15	0.88

lists citation statistics available in the Google Scholar. A citation rate per year for Basquin model is given 12, Kohout and Vechet 4.3, Sendeckyj 3.0, Kim and Zhang 0.88, and Weibull 0.21. It brings our attention to the fact that the most incapable model has been the most frequently cited, indicating that many researchers might have compromised the fitting capability for simplicity if they had been aware of capabilities of the existing S-N curve models.

4.2. S-N Curve Models for Predicting Stress Ratio Effect

The same experimental data sets used for evaluation of S-N curve models for fatigue data characterization will be employed in this section for evaluation of S-N curve models developed for predicting the fatigue stress ratio effect. The best fit provided by the three models (Weibull, Sendeckyj, and Kim and Zhang) will be represented by the Kim and Zhang model as the best fit reference for comparisons. It is noted that D’Amore model Equations (32)–(34), and Epaarachchi and Clausen model Equations (35) and (36) adopt the initial condition N_f = 1 at σ_max = σ_uT while Poursatip and Beaumont model Equations (29) and (30) adopts N_f = 0 at σ_uC, which does not exist on logarithmic coordinate. Therefore, the same condition i.e., N_f = 1 at σ_max = σ_uT as those in Figure 7 and Figure 9 was employed for comparison purposes such that the other models and experimental data were accordingly adjusted. Experimental data and plots will be in lin-log coordinates.

An experimental data set from Weibull [18] is shown in Figure 10 in comparison with S-N curves generated from the various models for R = −1: Kim and Zhang model Equations (22) and (23) as the reference with α = 3.63 × 10⁻³⁹ and β = 11.809 for N₀ = 1; Poursatip and Beaumont model Equation (30) with α = 17,000 and an added N₀ = 1; D’Amore model Equation (34) with α′ = 0.053 and β = 0.2; and Epaarachchi and Clausen model Equation (36) with α′ = 0.0007 and β = 0.245. It is seen that all the models do not agree with the reference performed by the three models or Kim and Zhang model. The Poursatip and Beaumont model, however, appears closer to the reference fit than the other two models. It was found, at the same time, that the S-N line shape of Poursatip and Beaumont model is controlled by a single fitting parameter α which only allows it to move around the first data point (for N_f = 1 at σ_max = σ_uT) as the hinge without much controlling the shape. On the other hand, the fitting capabilities of D’Amore, and Epaarachchi and Clausen models appear relatively poor, given that radii of the curvature of the S-N curves at low cycles are too large compared to the reference fit. A possible reason for this is that the derivation may not be validly conducted as discussed earlier. Also, it would not be surprising to find that D’Amore, and Epaarachchi and Clausen models are practically identical in this case probably due to their similarities in expression and derivation [see Equations (34) and (36)]. The only difference made by the exponent 1.6 in the Epaarachchi and Clausen model Equation (36) is found to be reflected only in values of fitting parameters α′ and β.

An experimental data set from Sendeckyj [19] obtained for glass reinforced epoxy laminates with σ_uT = 2013 MPa is shown in Figure 11 in comparison with S-N curves generated from the various models for R = 0.1 under T-T loading. The fitting parameters were found as follows: α = 10^−26.505 and β = 7.3813 for Kim and Zhang model Equations (22) and (23) with N₀ = 1; α = 60 and an added N₀ = 1 for Poursatip and Beaumont model Equation (30); α′ = 0.033 and β = 0.44 for D’Amore model Equation (34); α′ = 0.00035 and β = 0.51 for Epaarachchi and Clausen model Equation (36). D’Amore, and Eparrachchi and Clausen models appear improved for fitting capabilities compared to those for R = −1 (Figure 10) probably because of the rapid decrease in experimental data at low cycles as expected from their S-N curve performances shown in Figure 10 although clear deviations from the best fit are noticed. However, the added exponent 1.6 in Equation (36) does not seem to greatly improve the D’Amore model Equation (34). A fitting capability of Poursatip and Beaumont model appear still good although a slight difference from the best fit is noticed.

Figure 12 shows experimental data from Kawai and Itoh [22] with σ_uC = 807 MPa and (σ_uT = 1887 MPa) for R = −0.43 in comparison with various models: Kim and Zhang model Equations (22) and (23) with α = 8.18 × 10⁻⁴¹ and β = 13.6 for N₀ = 1; Poursatip and Beaumont model Equation (30) with α = 29 and added N₀ = 1; D’Amore model Equation (34) with α′ = 0.3 and β = 0.19; and Epaarachchi and Clausen model Equation (36) with α′ = 0.0169 and β = 0.14. The Epaarachchi and Clausen model appear very close to the reference fit (or Kim and Zhang model) while Poursatip and Beaumont, and D’Amore models noticeably deviated from the reference fit. The difference in behavior between D’Amore model, and Epaarachchi and Clausen model indicates that the added exponent 1.6 in Equation (36) noticeably affects the S-N curve shape in this case.

An experimental data set from Kawai and Itoh [22] with σ_uC = 807 MPa, (σ_uT = 1887 MPa) for R = −3 representing C-T loading is shown in Figure 13 with S-N curves generated from the various models: Kim and Zhang model with α = 4.3 × 10⁻³⁸, β = 13.506, and N₀ = 1; Poursatip and Beaumont model Equation (30) with α = 11,000 and added N₀ = 1; for D’Amore model Equation (34) with α′ = 0.053 and β = 0.2; for the Epaarachchi and Clausen model Equation (36) with α′ = 0.001 and β = 0.25. The fitting capabilities of all models appear to be good but differences between models are not as obvious as those for R = −1 in Figure 10 because the experimental data set is relatively small compared to that for R = −1. However, the same tendencies of D’Amore, and Epaarachchi and Clausen models are seen such that the radii of the curvature of S-N curve at low cycles is still large. Also, it is noted that D’Amore, and Epaarachchi and Clausen models behave similarly again without much effect of the modified exponent 1.6 in Equation (36) on the S-N curve shape.

Figure 14 shows an experimental data set from Kawai and Itoh [22] with σ_uC = 807 MPa (σ_uT = 1887 MPa) for R = 10 with S-N curves generated from the various models: the Kim and Zhang model Equations (22) and (23) with α = 2.9 × 10⁻¹⁶⁰ and β = 54.388 for N₀ = 1; the Poursatip and Beaumont model Equation (30) with α = 719 and added N₀ = 1; the D’Amore model, Equation (34), with α′ = 0.043 and β = 0.01; the Epaarachchi and Clausen model Equation (36) with α′ = 0.0075 and β = 0.048. The fitting capabilities of the D’Amore, and Epaarachchi and Clausen models appear to be practically identical to the reference fit (or Kim and Zhang model). In contrast, the Poursatip and Beaumont model significantly deviates from the reference fit and seems to have a strong tendency of producing the concave-down S-N curve at low cycles. Judging from the earlier observations, the Poursatip and Beaumont model seems incapable of producing other shapes than the concave-down S-N curve at low cycles regardless of stress ratio. A possible reason for this may be due to some defects during derivation as discussed earlier even though Equation (27) satisfies Equation (1).

In summary, the three S-N curve models developed for predicting for stress ratio effect appear inferior to those best performed for data characterization. They are listed in

Table 4. S-N curve models developed for stress ratio effect.

S-N Curve Models	Capability of Curve Fitting and Applicability to Different Stress Ratios		Number of Fitting Parameters	Relation with Physical Properties
				Boundary at Initial Nf	Damage Representation	Satisfaction of $\frac{\partial {\mathit{D}}_{\mathbf{fT}}}{\partial {\mathit{\sigma }}_{\mathbf{max}}}=-\mathit{A}$
	R	Capability
Poursartip and Beaumont (1986) $N_{\mathrm{f}}=\alpha {\left(\frac{{\sigma }_{\max }}{{\sigma }_{\mathrm{uT}}}\right)}^{-6.393}\left(\frac{{\sigma }_{\mathrm{uT}}-{\sigma }_{\max }}{{\sigma }_{\mathrm{uT}}}\right)$	1	Good	1	Yes	Partially Yes	Yes
	0.1	Good
	−0.43	Poor
	−3	Reasonable
	10	Poor
D’Amore et al. (1996) $N_{\mathrm{f}}={\left[\frac{{\sigma }_{\mathrm{uT}}-{\sigma }_{\max }}{{\alpha }^{\prime }{\sigma }_{\max }}+1\right]}^{1/\beta }$	−1	Poor	2	Yes	N/A	N/A
	0.1	Reasonable
	−0.43	Poor
	−3	Reasonable
	10	Good
Epaarachchi and Clausen (2003) $N_{\mathrm{f}}={\left(\frac{{\sigma }_{\mathrm{uT}}-{\sigma }_{\max }}{{\alpha }^{\prime }{\left({\sigma }_{\max }\right)}^{1.6}}+1\right)}^{1/\beta }$	−1	Poor	2	Yes	N/A	N/A
	0.1	Reasonable
	−0.43	Good
	−3	Reasonable
	10	Good

and their performances are summarized.

5. Conclusions

S-N curve models proposed by Weibull, Sendeckyj, and Kim and Zhang for fatigue data characterization have been found to have the highest fitting capabilities for experimental fatigue data obtained by Weibull for R = −1, Sendeckyj for R = 0.1, and Kawai and Itoh for R = −0.43, −3, and 10. The Kohout and Vechet model was also found to have good fitting capabilities but with a limitation for shaping S-N curve at some stress ratios (e.g., R = −0.43). Also, S-N curve models developed for predicting the effect of stress ratio were found to be relatively inferior in fitting capability to those developed directly for data characterization. The Kim and Zhang model appeared to have an advantage over the Weibull and Sendeckyj models for representing the fatigue damage at failure, which may be further useful for developing applicative models.