The Development of an Assembled Truss Core Lightweight Panel and Its Method of Manufacture
Abstract
1. Introduction
2. Materials and Methods
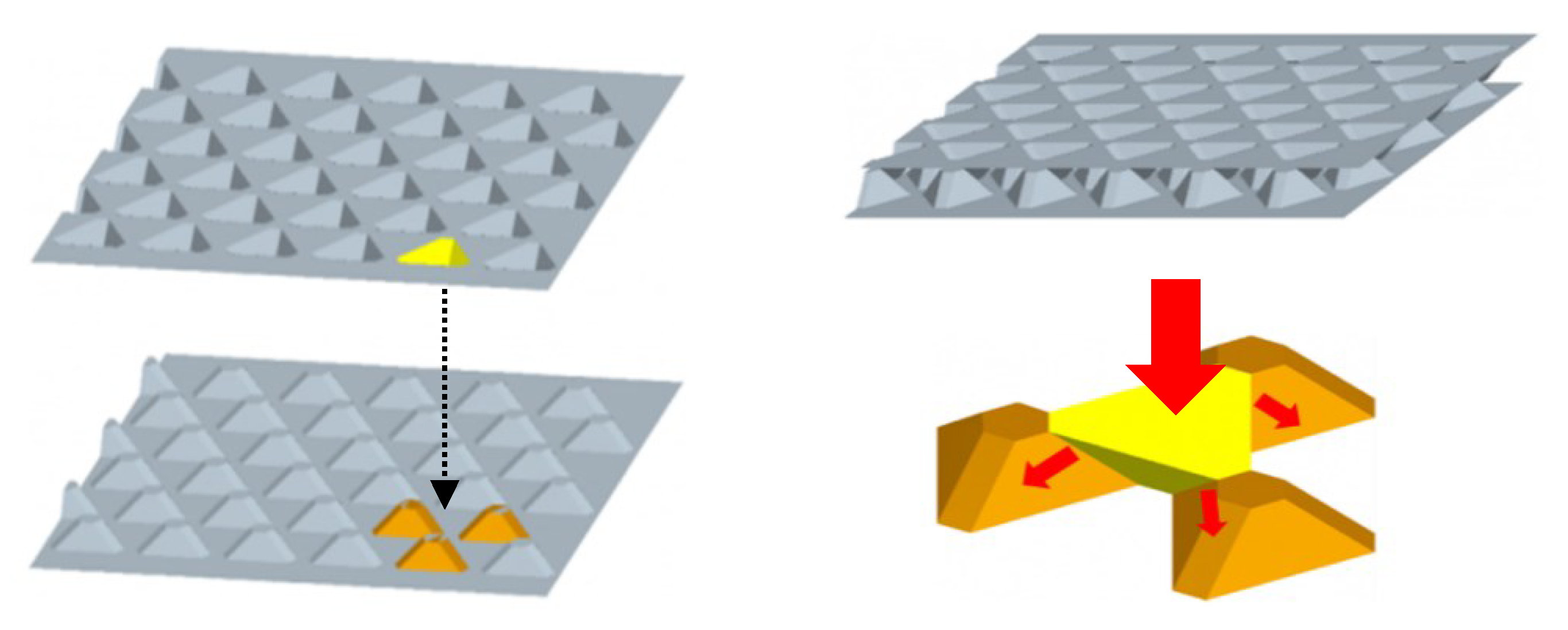
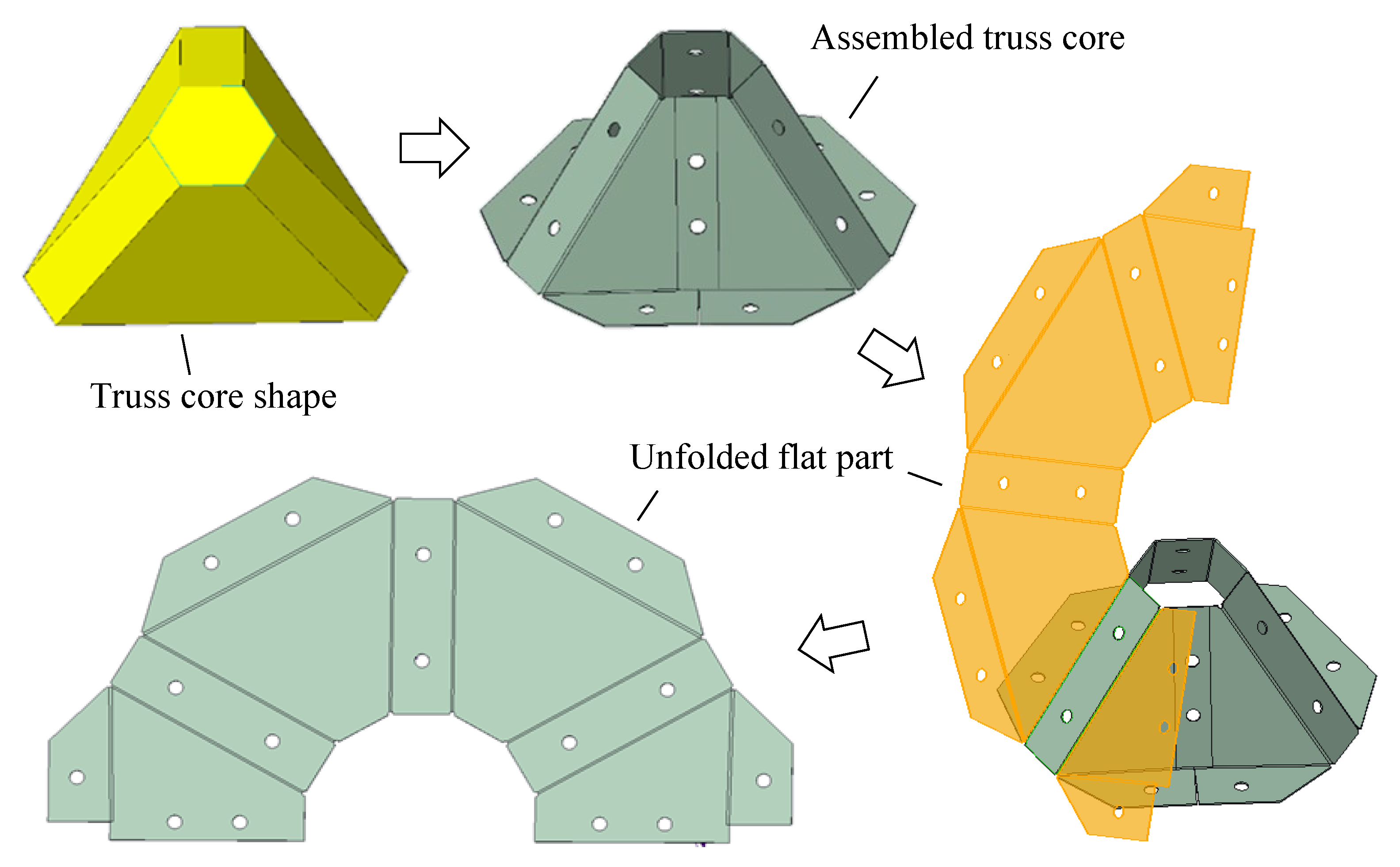
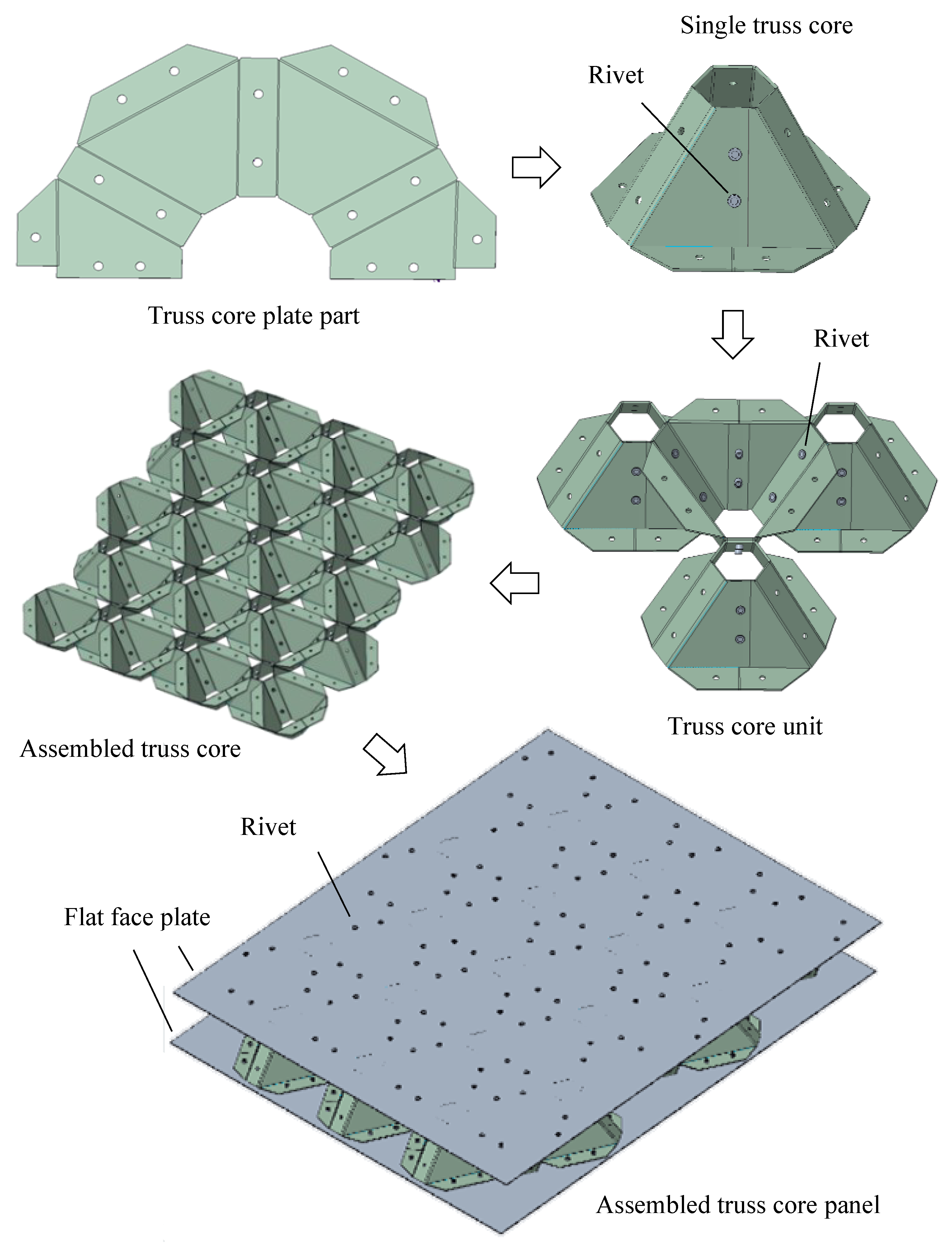
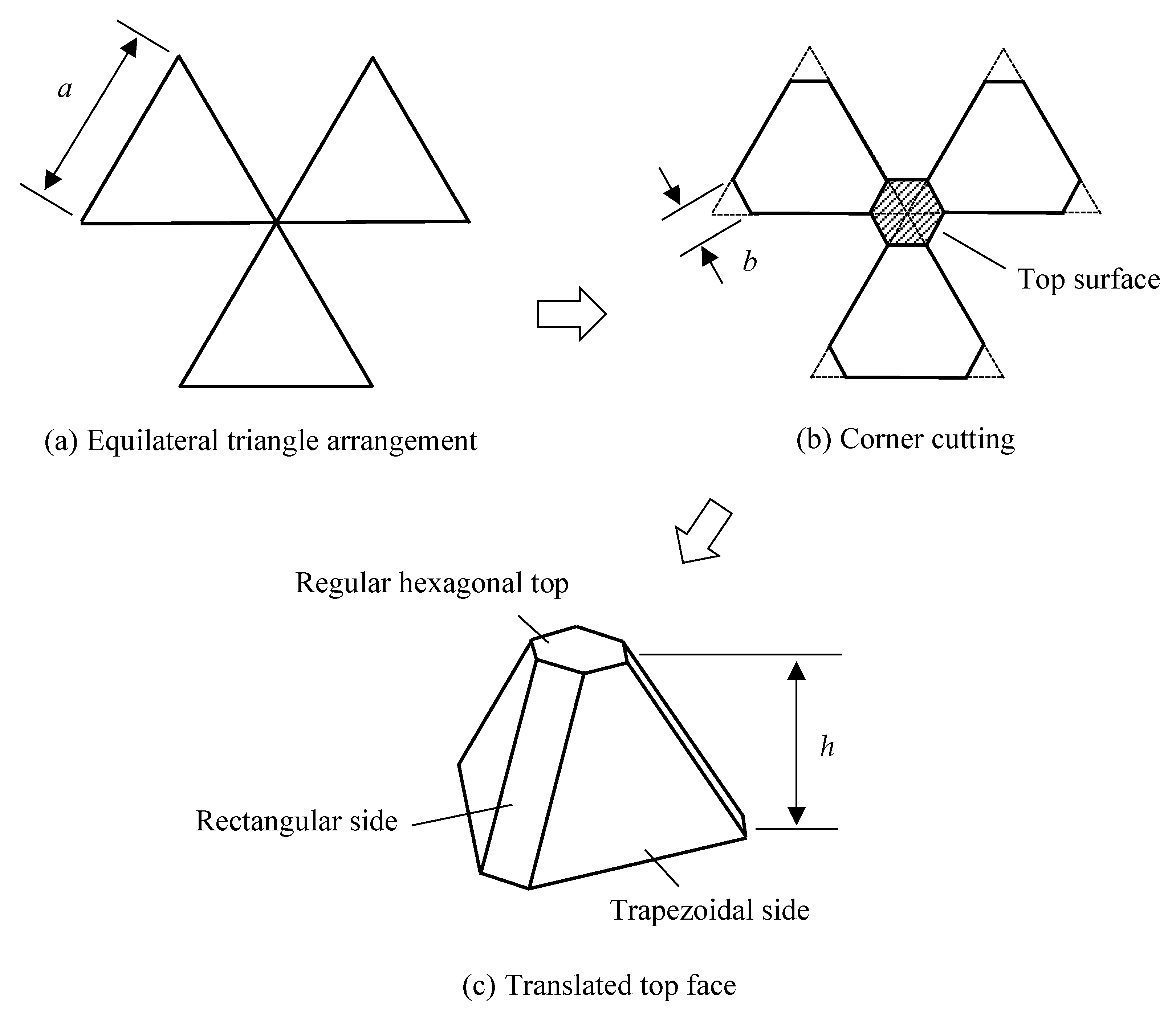
2.1. Assembled Truss Core Panel
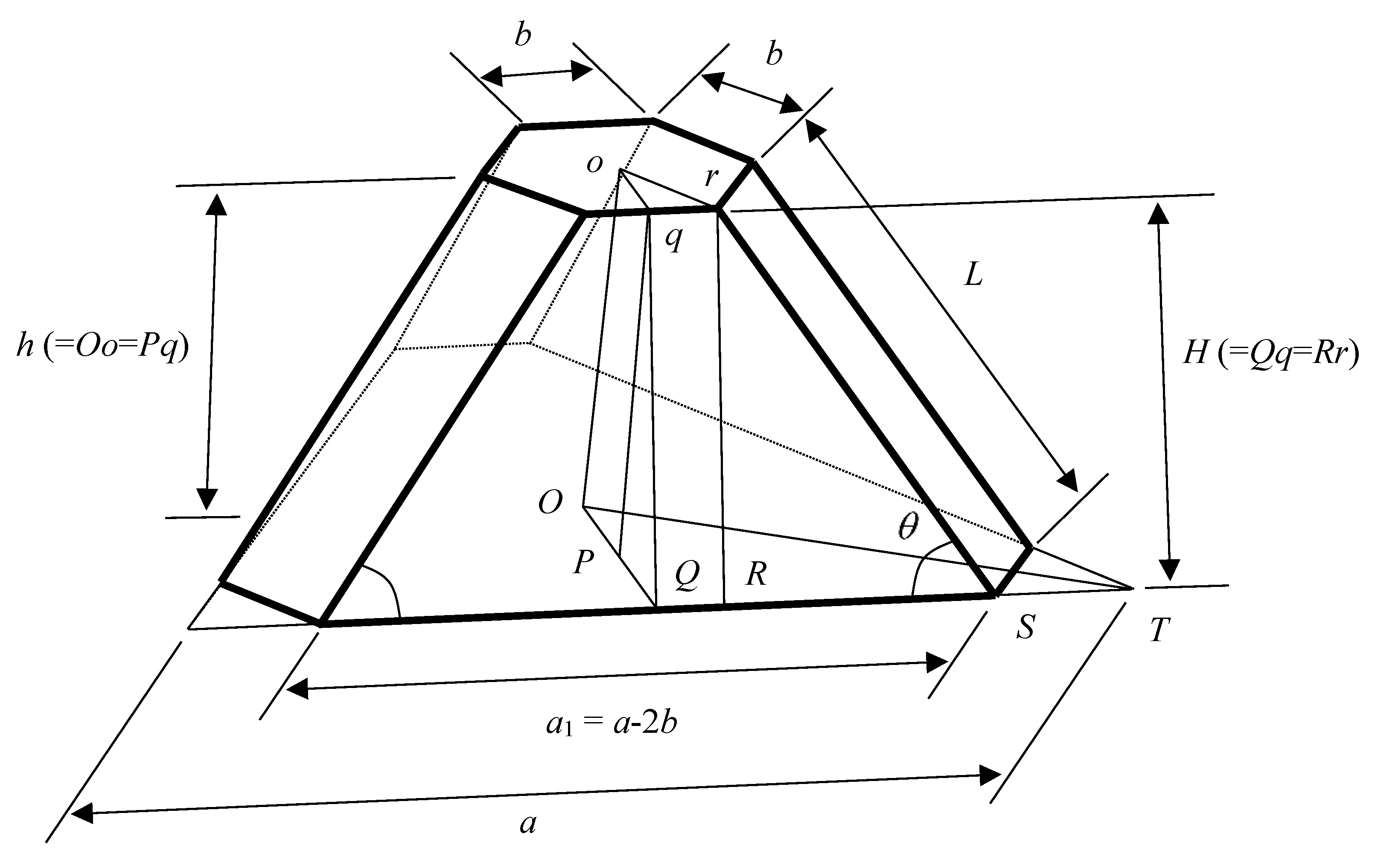
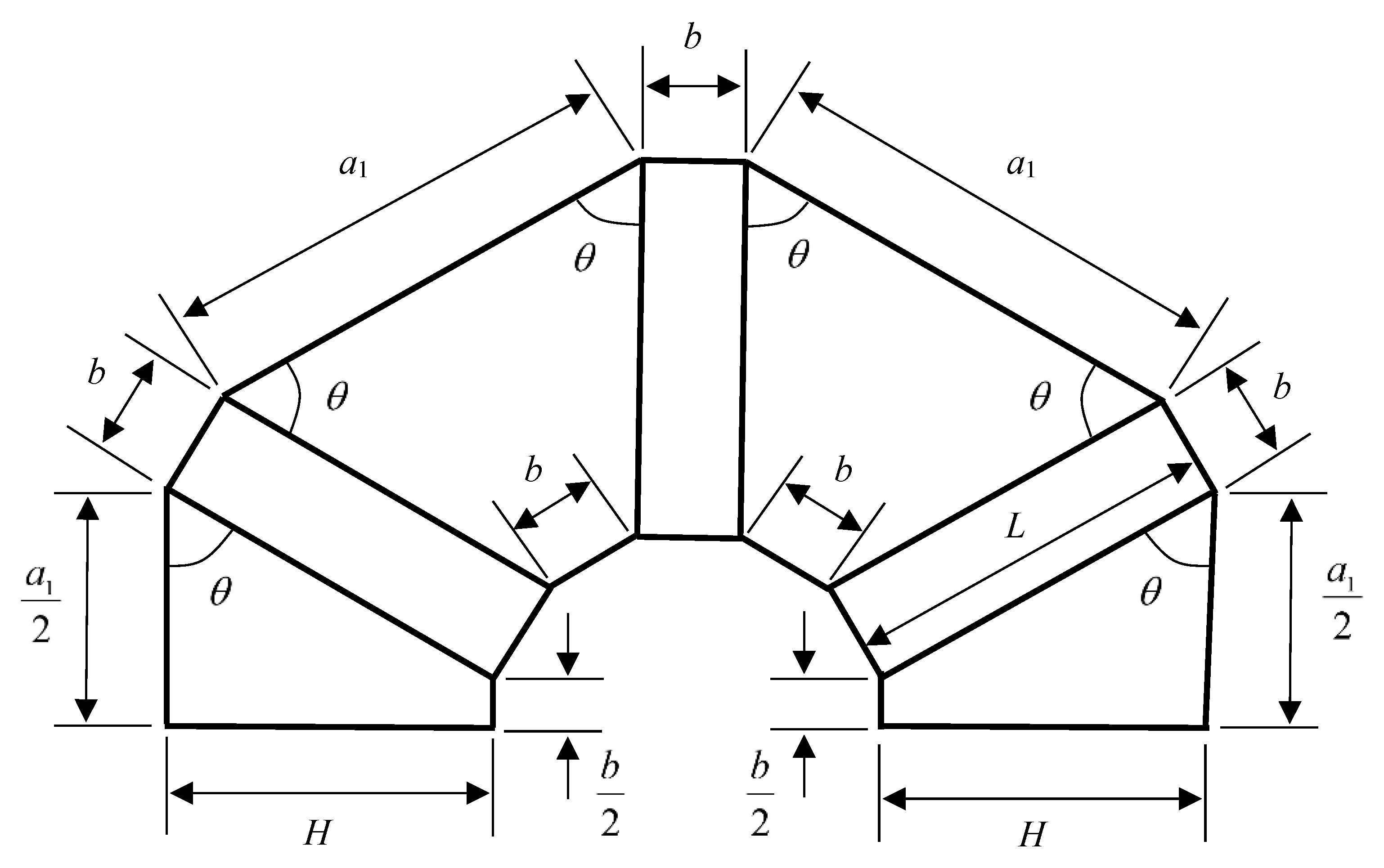
2.2. Design parameters
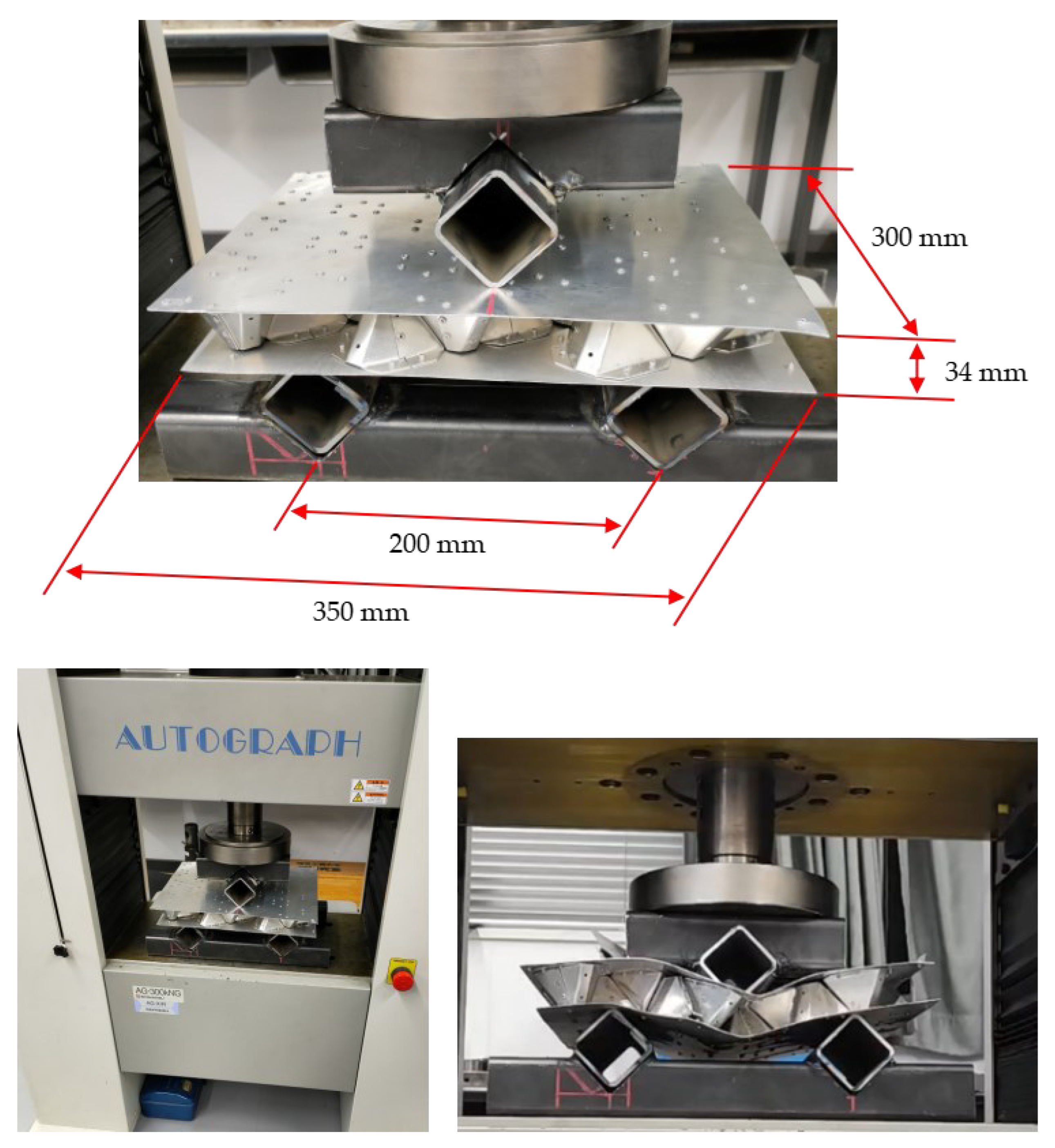
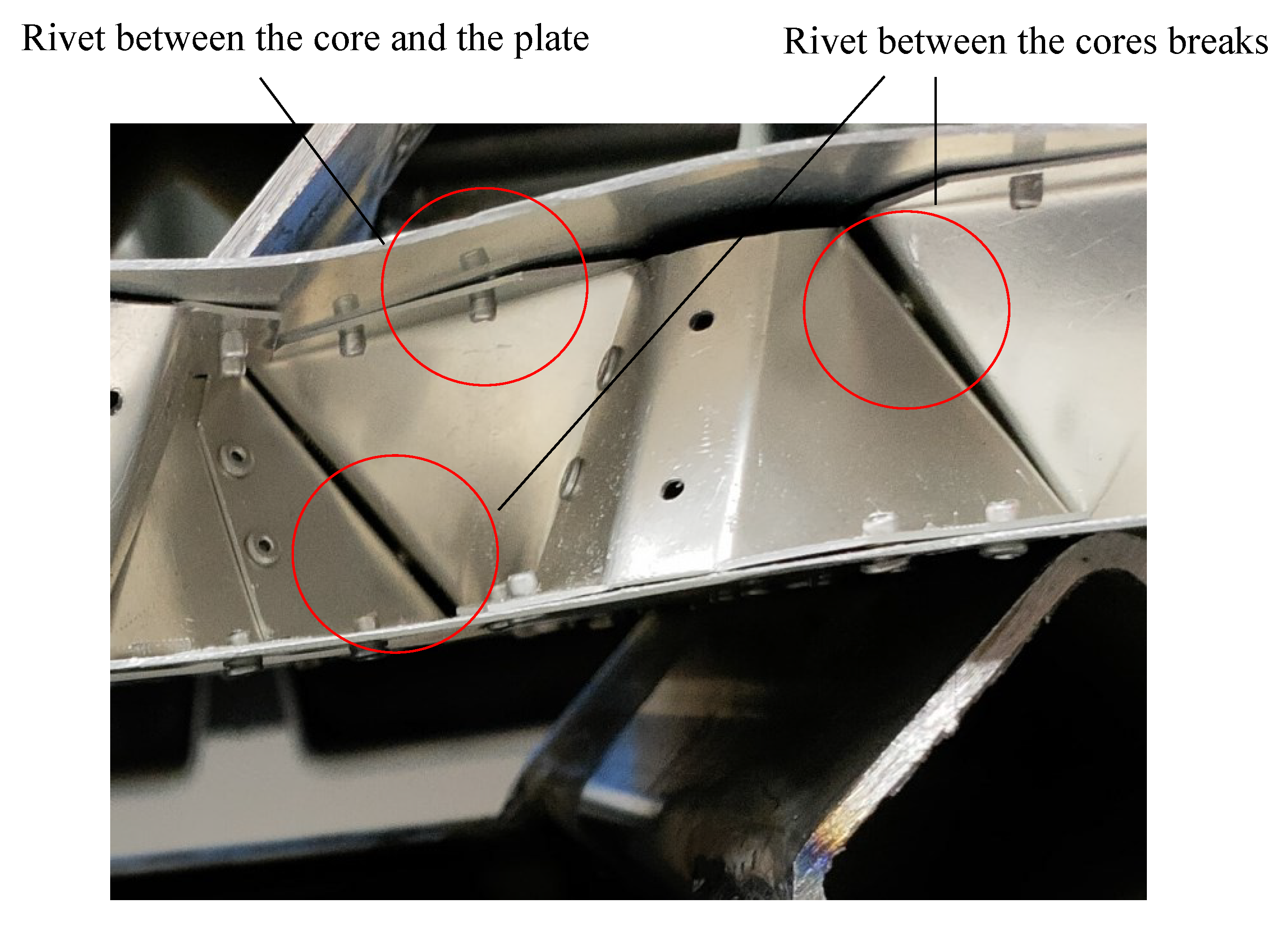
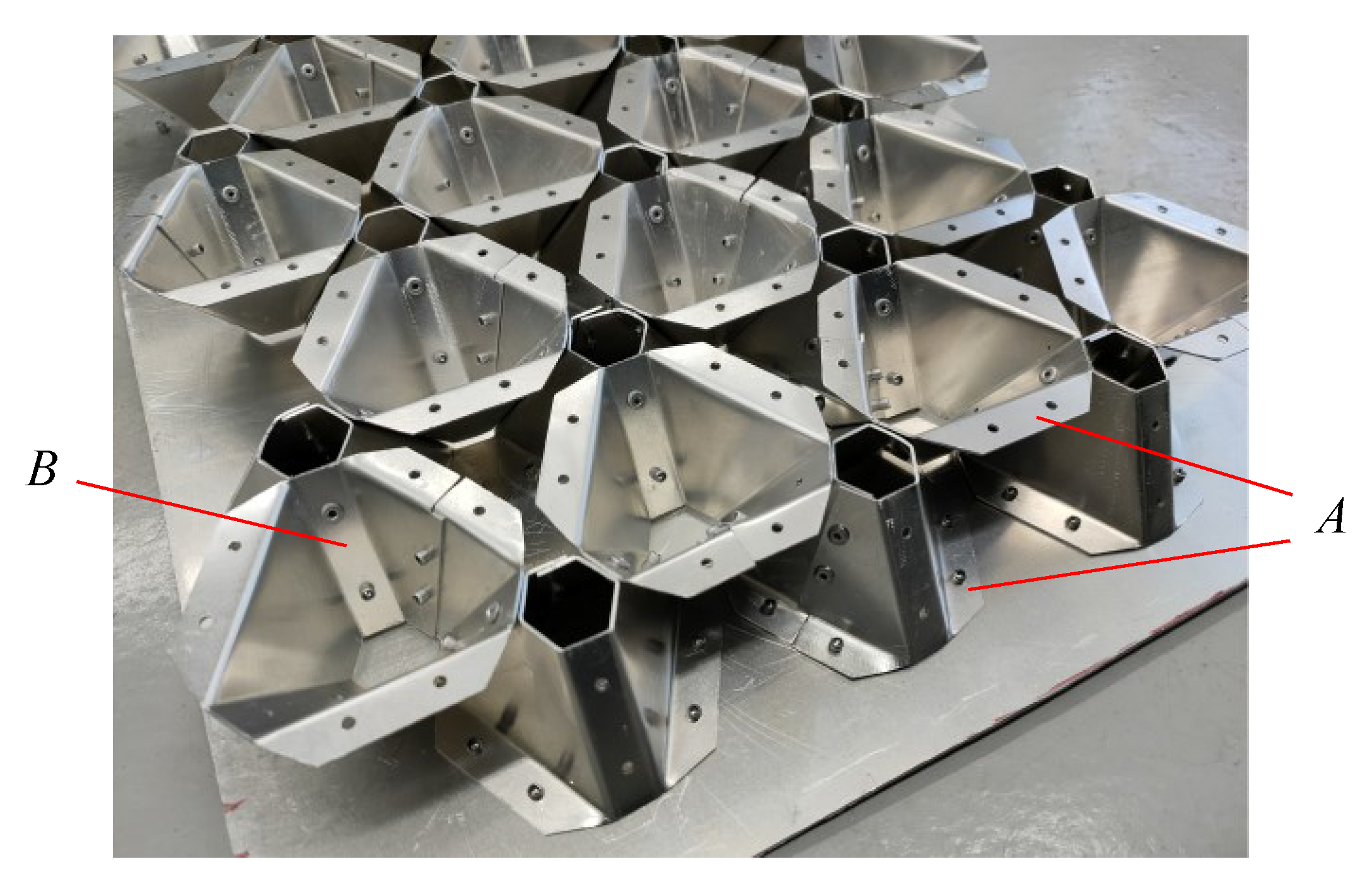
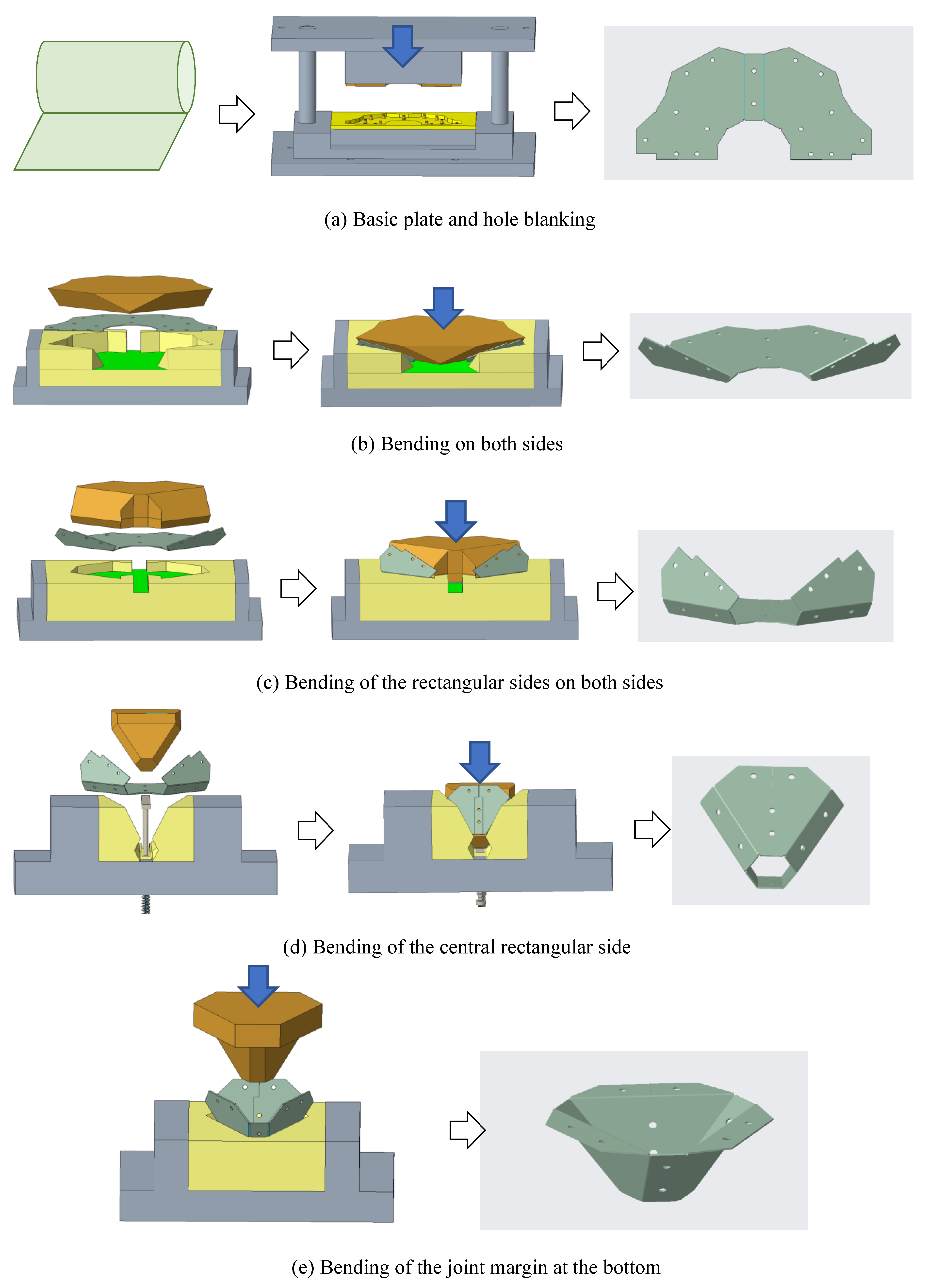
2.3. Manufacturing Test
3. Results and Discussions
3.1. Experimental Verification of Mechanical Properties
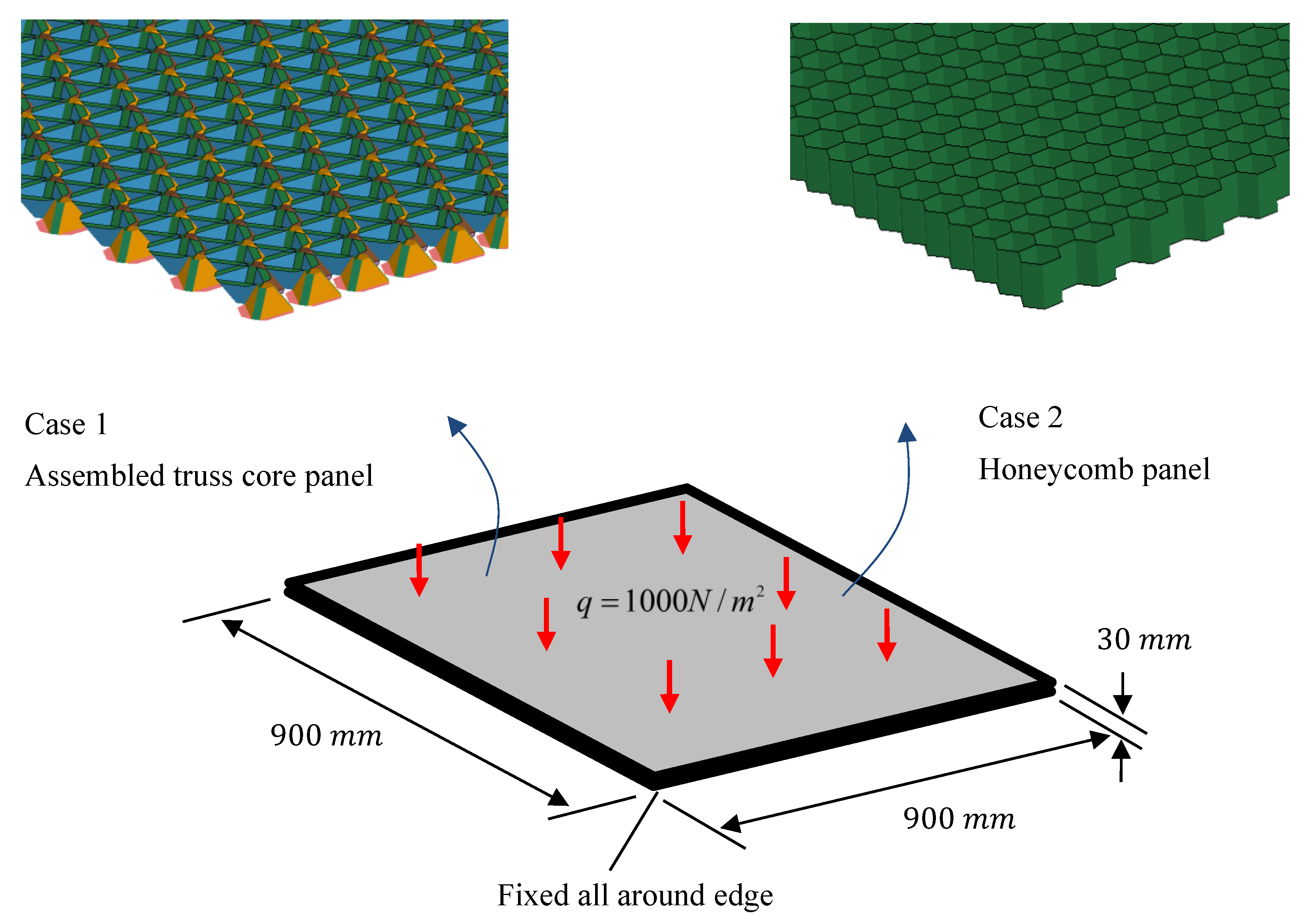
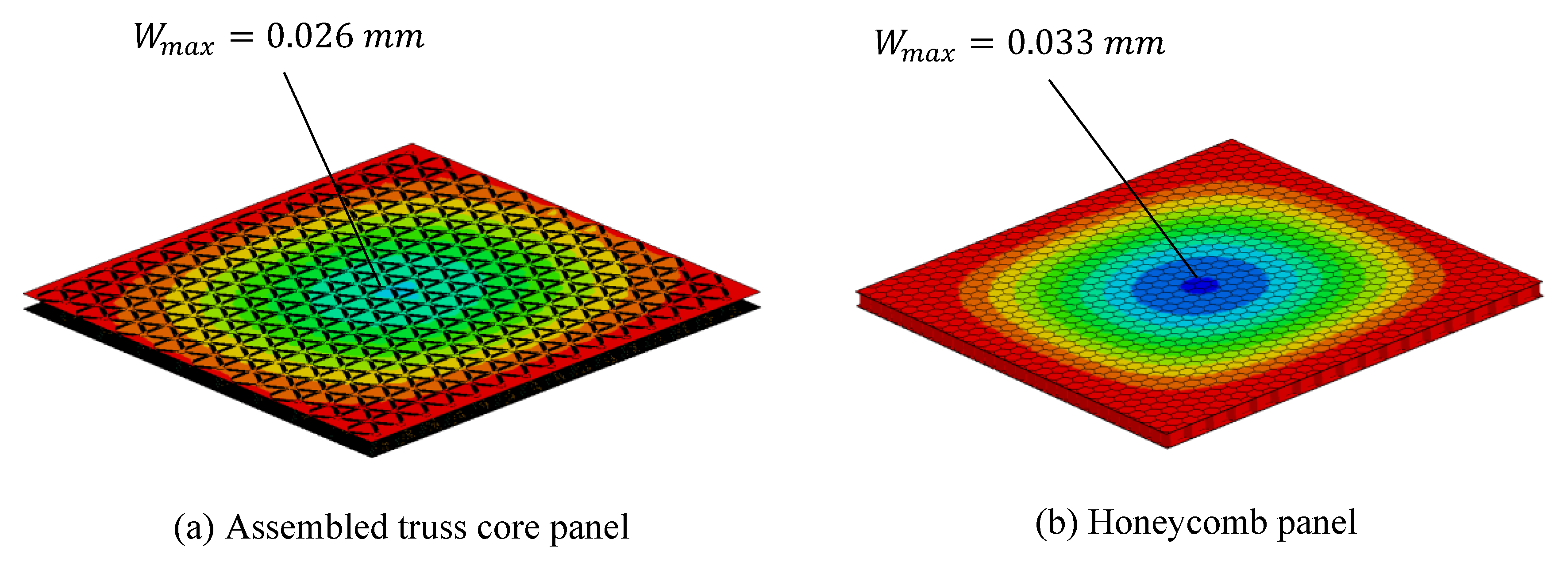
3.2. Comparison with Conventional Lightweight Panel
3.3. Comparison with Conventional Forming Method
3.4. Proposal for Practical Production
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, C.; Li, Z. A Review of Lightweight Design for Space Mirror Core Structure: Tradition and Future. Machines 2022, 10, 1066. [Google Scholar] [CrossRef]
- Mohring, H.C.; Muller, M.; Krieger, J.; Multhof, J.; Plagge, C.; Wit, J.D.; Misch, S. Intelligent lightweight structures for hybrid machine tools. Prod. Eng. 2020, 14, 583–600. [Google Scholar] [CrossRef]
- Aggogeri, F.; Borboni, A.; Merlo, A.; Pellegrini, N.; Ricatto, R. Vibration Damping Analysis of Lightweight Structures in Machine Tools. Materials 2017, 10, 297. [Google Scholar] [CrossRef] [PubMed]
- Czerwinski, F. Current Trends in Automotive Lightweighting Strategies and Materials. Materials 2021, 14, 6631. [Google Scholar] [CrossRef] [PubMed]
- Luo, W.; Zheng, Z.; Liu, F.; Han, D.; Zhang, Y. Lightweight design of truck frame. J. Phys. Conf. Ser. 2020, 1653, 012063. [Google Scholar] [CrossRef]
- Tyflopoulos, E.; Steinert, M. Topology and Parametric Optimization-Based Design Processes for Lightweight Structures. Appl. Sci. 2020, 10, 4496. [Google Scholar] [CrossRef]
- Xiong, F.; Wang, D.; Ma, Z.; Chen, S.; Lv, T.; Lu, F. Structure-material integrated multi-objective lightweight design of the front-end structure of automobile body. Struct. Multidiscip. Optim. 2018, 57, 829–847. [Google Scholar] [CrossRef]
- Meschut, G.; Janzen, V.; Olfermann, T. Innovative and Highly Productive Joining Technologies for Multi-Material Lightweight Car Body Structures. J. Mater. Eng. Perform. 2014, 23, 1515–1523. [Google Scholar] [CrossRef]
- Birk, F.; Ali, F.; Weigold, M.; Abele, E.; Schutzer, K. Lightweight hybrid CFRP design for machine tools with focus on simple manufacturing. Int. J. Adv. Manuf. Technol. 2020, 108, 3915–3924. [Google Scholar] [CrossRef]
- Yang, H.; Ng, B.C.; Yu, H.C.; Liang, H.H.; Kwok, C.C.; Lai, F.W. Mechanical properties study on sandwich hybrid metal/(carbon, glass) fiber reinforcement plastic composite sheet. Adv. Compos. Hybrid Mater. 2022, 5, 83–90. [Google Scholar] [CrossRef]
- Abhinav, S.N.; Budharaju, M.V. A Review Paper on Origin of Honeycomb Structure and its Sailing Properties. Int. J. Eng. Res. Technol. 2020, 9, 861–866. [Google Scholar]
- Birmana, V.; Kardomateas, G.A. Review of current trends in research and applications of sandwich structures. Compos. Part B Eng. 2018, 142, 221–240. [Google Scholar] [CrossRef]
- Zhang, S.; Chen, W.; Gao, D.; Xiao, L.; Han, L. Experimental Study on Dynamic Compression Mechanical Properties of Aluminum Honeycomb Structures. Appl. Sci. 2020, 10, 1188. [Google Scholar] [CrossRef]
- Galehdari, S.A.; Kadkhodayan, M.; Hadidi-Moud, S. Low velocity impact and quasi-static in-plane loading on a graded honeycomb structure; Experimental, analytical, and numerical study. Aerosp. Sci. Technol. 2015, 47, 425–433. [Google Scholar] [CrossRef]
- Wang, B.; An, P.; Jiang, H.; Zhang, Z.; Zhang, D. Honeycomb Structure Design Based on Finite Element Method. Appl. Mech. Mater. 2015, 711, 74–77. [Google Scholar] [CrossRef]
- Wang, Z.; Li, Z.; Zhou, W.; Hui, D. On the influence of structural defects for honeycomb structure. Compos. Part B Eng. 2018, 142, 183–192. [Google Scholar] [CrossRef]
- Chen, D.H.; Ozaki, S. Stress concentration due to defects in a honeycomb structure. Compos. Struct. 2009, 89, 52–59. [Google Scholar] [CrossRef]
- Qiu, C.; Guan, Z.; Guo, X.; Li, Z. Buckling of honeycomb structures under out-of-plane loads. J. Sandw. Struct. Mater. 2018, 22, 797–821. [Google Scholar] [CrossRef]
- Ishida, S. Design of cylindrical honeycomb cores: Geometric consideration. Mech. Eng. J. 2018, 5, 18-00147. [Google Scholar] [CrossRef]
- Saito, K.; Pellegrino, S.; Nojima, T. Manufacture of Arbitrary Cross-Section Composite Honeycomb Cores Based on Origami Techniques. J. Mech. Des. 2014, 136, 051011. [Google Scholar] [CrossRef]
- Nojima, T.; Saito, K. Development of newly designed ultra-light core structures. JSME Int. J. Ser. A 2006, 49, 38–42. [Google Scholar] [CrossRef]
- Saito, K.; Nojima, T. Development of Light-Weight Rigid Core Panels. J. Solid Mech. Mater. Eng. 2007, 1, 1097–1104. [Google Scholar] [CrossRef]
- Tokura, S.; Hagiwara, I. A Study for the Influence of Work Hardening on Bending Stiffness of Truss Core Panel. ASME J. Appl. Mech. 2010, 77, 031010. [Google Scholar] [CrossRef]
- Chen, J.W.; Liu, W.; Su, X.Y. Vibration and Buckling of Truss Core Sandwich Plates on An Elastic Foundation Subjected to Biaxial In-plane Loads. Comput. Mater. Contin. 2011, 24, 163–182. [Google Scholar]
- Tokura, S.; Hagiwara, I. Shape Optimization to Improve Impact Energy Absorption Ability of Truss Core Panel. J. Comput. Sci. Technol. 2011, 5, 1–12. [Google Scholar] [CrossRef]
- Tokura, S.; Hagiwara, I. Forming Process Simulation of Truss Core Panel. J. Comput. Sci. Technol. 2010, 4, 25–35. [Google Scholar] [CrossRef]
- Xia, Z.; Zhao, X.; Hagiwara, I. A Simulation Approach to Improve Forming Limitation of Truss Core Panel. Appl. Mech. Mater. 2011, 121–126, 2471–2475. [Google Scholar] [CrossRef]
- Kong, C.; Zhao, X.; Hagiwara, I. Progressive multistep press forming of a truss core panel for floor structure of electric vehicle. Int. J. Veh. Perform. 2018, 4, 200–217. [Google Scholar] [CrossRef]
- Tian, Z.; Kong, C.; Zhao, W.; Guan, J.; Zhao, X. Intermediate Model Design in the Progressive Stamping Process of a Truss Core Lightweight Panel. Appl. Sci. 2022, 12, 4002. [Google Scholar] [CrossRef]
- Deshpande, V.S.; Fleck, N.A. Collapse of truss core sandwich beams in 3-point bending. Int. J. Solids Struct. 2001, 38, 6275–6305. [Google Scholar] [CrossRef]
- Wu, Q.; Gao, Y.; Wei, X.; Mousanezhad, D.; Ma, L.; Vaziri, A.; Xiong, J. Mechanical properties and failure mechanisms of sandwich panels with ultra-lightweight three-dimensional hierarchical lattice cores. Int. J. Solids Struct. 2018, 132–133, 171–187. [Google Scholar] [CrossRef]
- Acanfora, V.; Castaldo, R.; Riccio, A. On the Effects of Core Microstructure on Energy Absorbing Capabilities of Sandwich Panels Intended for Additive Manufacturing. Materials 2022, 15, 1291. [Google Scholar] [CrossRef]
- Xiong, Z.; Guo, X.; Luo, Y.; Zhu, S. Elasto-plastic stability of single-layer reticulated shells with aluminium alloy gusset joints. Thin-Walled Struct. 2017, 15, 163–175. [Google Scholar] [CrossRef]
- Halquist, J. Ls-Dyna Keyword User’s Manual Version 971; The JRI Solutions, Limited.: Worthing, UK, 2007. [Google Scholar]
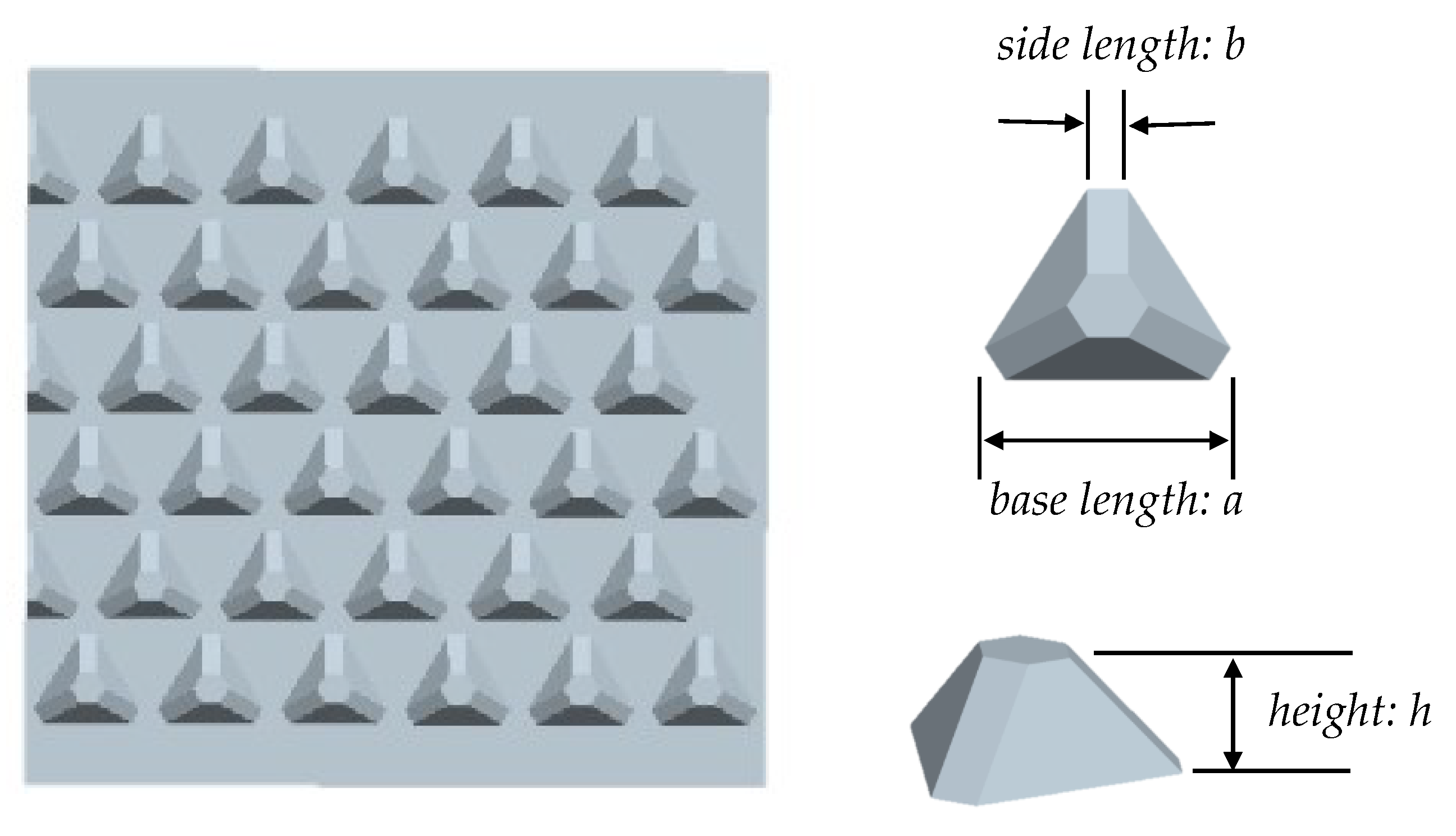





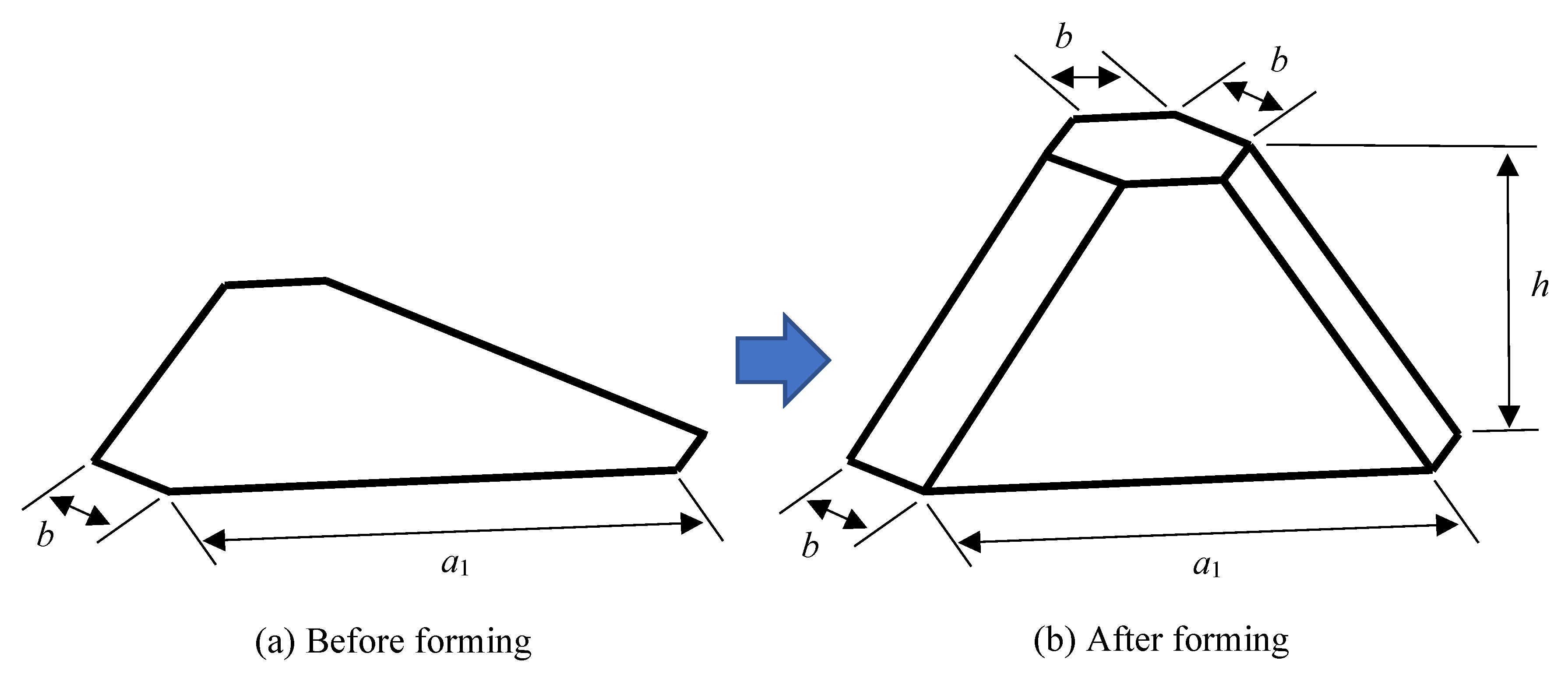
| Base triangle side length a | 70 mm |
| Corner cut side length b | 10 mm |
| Truss core height h | 33 mm |
| Plate thickness t | 1.0 mm |
| Material of face plate and truss core | Aluminum A5052 |
| Truss core panel size | 350 mm × 300 mm |
| Analysis (kN/mm) | Experiment (kN/mm) | Change | |
|---|---|---|---|
| rivets and glue | 2.01 | 1.88 | 6.91 % |
| only rivets | 0.93 | 0.87 | 9.20 % |
| Item | Parameter | |
|---|---|---|
| Material | Aluminum | A5052 |
| Young’s modulus | 70 GPa | |
| Poisson’s ratio | 0.33 | |
| Thickness | 0.8 mm | |
| Assembled truss core panel | Nodes | 142342 |
| Elements | 154385 | |
| Honeycomb core panel | Nodes | 124223 |
| Elements | 100241 | |
| Assembled Truss | Honeycomb | Change | |
|---|---|---|---|
| Maximum deflection at center point | 0.026 mm | 0.033 mm | −21.2% |
| Initial bending stiffness | 31,153.85 kN/mm | 24,545.45 kN/mm | 26.9% |
| Initial bending stiffness per unit mass | 3084.54 kN/(kgmm) | 2789.26 kN/(kgmm) | 10.6% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, Z.; Kong, C.; Guan, J.; Zhao, W.; Fukuchi, A.B.; Zhao, X. The Development of an Assembled Truss Core Lightweight Panel and Its Method of Manufacture. J. Manuf. Mater. Process. 2023, 7, 29. https://doi.org/10.3390/jmmp7010029
Tian Z, Kong C, Guan J, Zhao W, Fukuchi AB, Zhao X. The Development of an Assembled Truss Core Lightweight Panel and Its Method of Manufacture. Journal of Manufacturing and Materials Processing. 2023; 7(1):29. https://doi.org/10.3390/jmmp7010029
Chicago/Turabian StyleTian, Zhilei, Chenghai Kong, Jingchao Guan, Wei Zhao, Apollo B. Fukuchi, and Xilu Zhao. 2023. "The Development of an Assembled Truss Core Lightweight Panel and Its Method of Manufacture" Journal of Manufacturing and Materials Processing 7, no. 1: 29. https://doi.org/10.3390/jmmp7010029
APA StyleTian, Z., Kong, C., Guan, J., Zhao, W., Fukuchi, A. B., & Zhao, X. (2023). The Development of an Assembled Truss Core Lightweight Panel and Its Method of Manufacture. Journal of Manufacturing and Materials Processing, 7(1), 29. https://doi.org/10.3390/jmmp7010029







