Robust Flight-Path Angle Consensus Tracking Control for Non-Minimum Phase Unmanned Fixed-Wing Aircraft Formation in the Presence of Measurement Errors
Abstract
1. Introduction
- Regarding the consensus control of multiple non-minimum phase systems, compared with the existing approaches (such as [15,16,17,18,19,20]) that are only capable of achieving output consensus with perfect measurements, the proposed approach is the first attempt to systematically resolves the output consensus tracking and the measurement error rejection problems simultaneously. Moreover, this paper shows the application of the proposed approach to the flight-path angle consensus tracking for multiple unmanned fixed-wing aircrafts;
- The separation property of the proposed three-module control scheme allows it to be easily modified and adapted to other robust or optimal consensus tracking control problems of non-minimum phase unmanned aircraft formations or heterogeneous unmanned aircraft formations;
- As proved theoretically and verified by simulations, a single parameter in the proposed Local Measurement Error Rejection Controller determines the system robustness against measurement errors and the overall control accuracy. This property makes parameter tuning easier and more intuitive than other approaches involving multi-parameter tuning and optimization, especially for formations of large numbers of unmanned aircraft.
2. Problem Formulation
3. Control Design
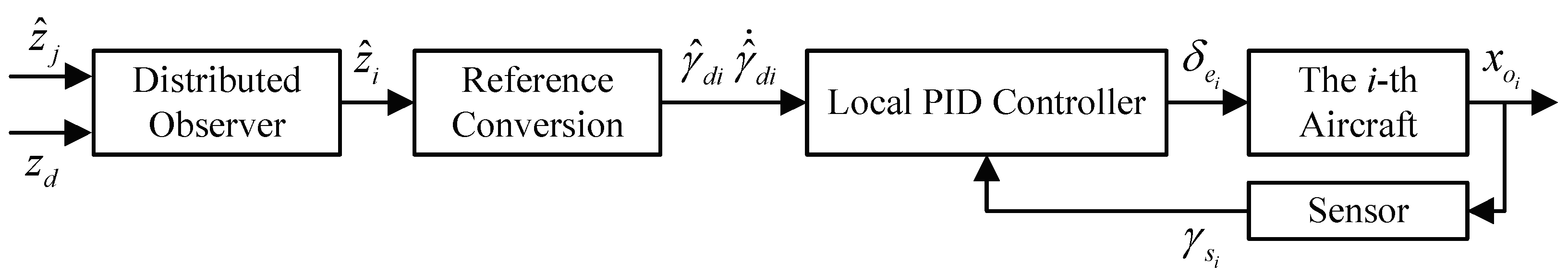
3.1. Overall Control Scheme
3.2. Design of the Distributed Observer
3.3. Design of the Casual Stable Inversion
3.4. Design of the Local Measurement Error Rejection Controller
4. Stability, Convergence, and Robustness Analysis
4.1. Analysis of the Distributed Observer
4.2. Analysis of the Casual Stable Inversion
4.3. Analysis of the Local Measurement Error Rejection Controller
- (i)
- System (15) is globally uniformly input-to-state stable if the feedback gain matrix is selected to render Hurwitz;
- (ii)
- (iii)
4.4. Analysis of the Overall Convergence Time
5. Application to Unmanned Fixed-Wing Aircraft Formation and Simulation Results
5.1. Unmanned Fixed-Wing Aircraft Model with Non-Minimum Phase Properties
5.2. Simulation Setup
5.3. Simulation Results
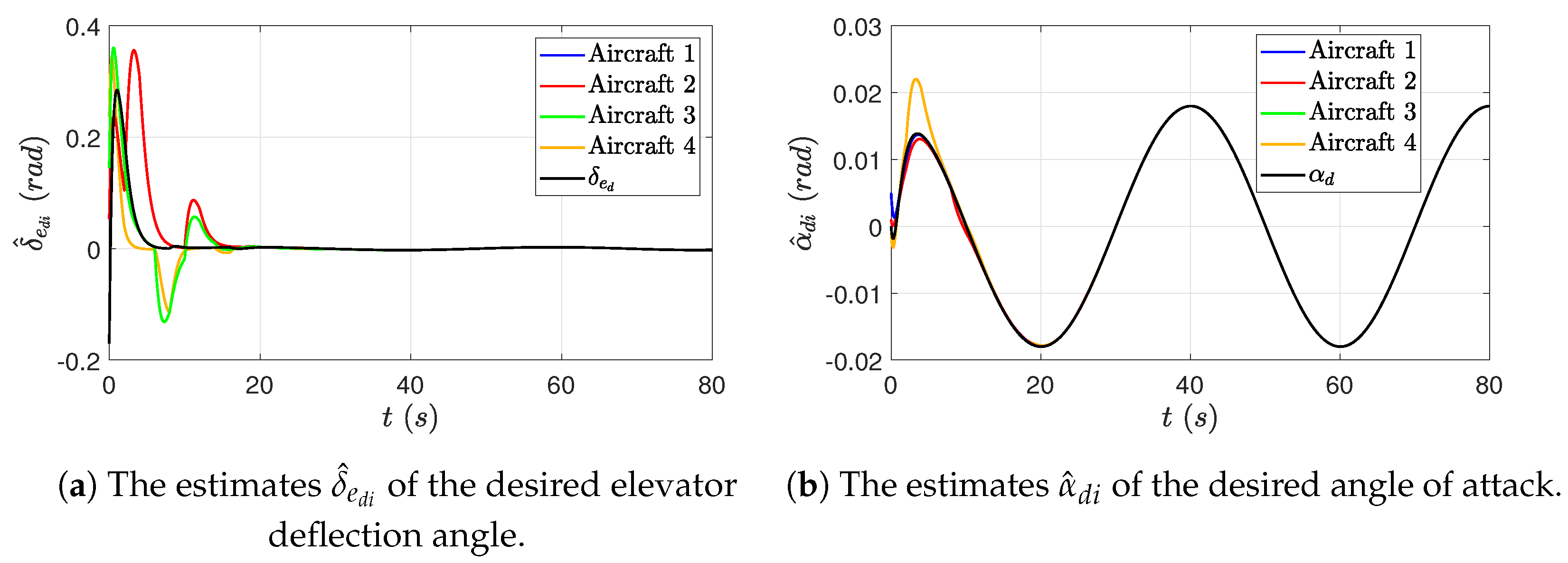
5.3.1. Simulation Results for Distributed Observer and Casual Stable Inversion
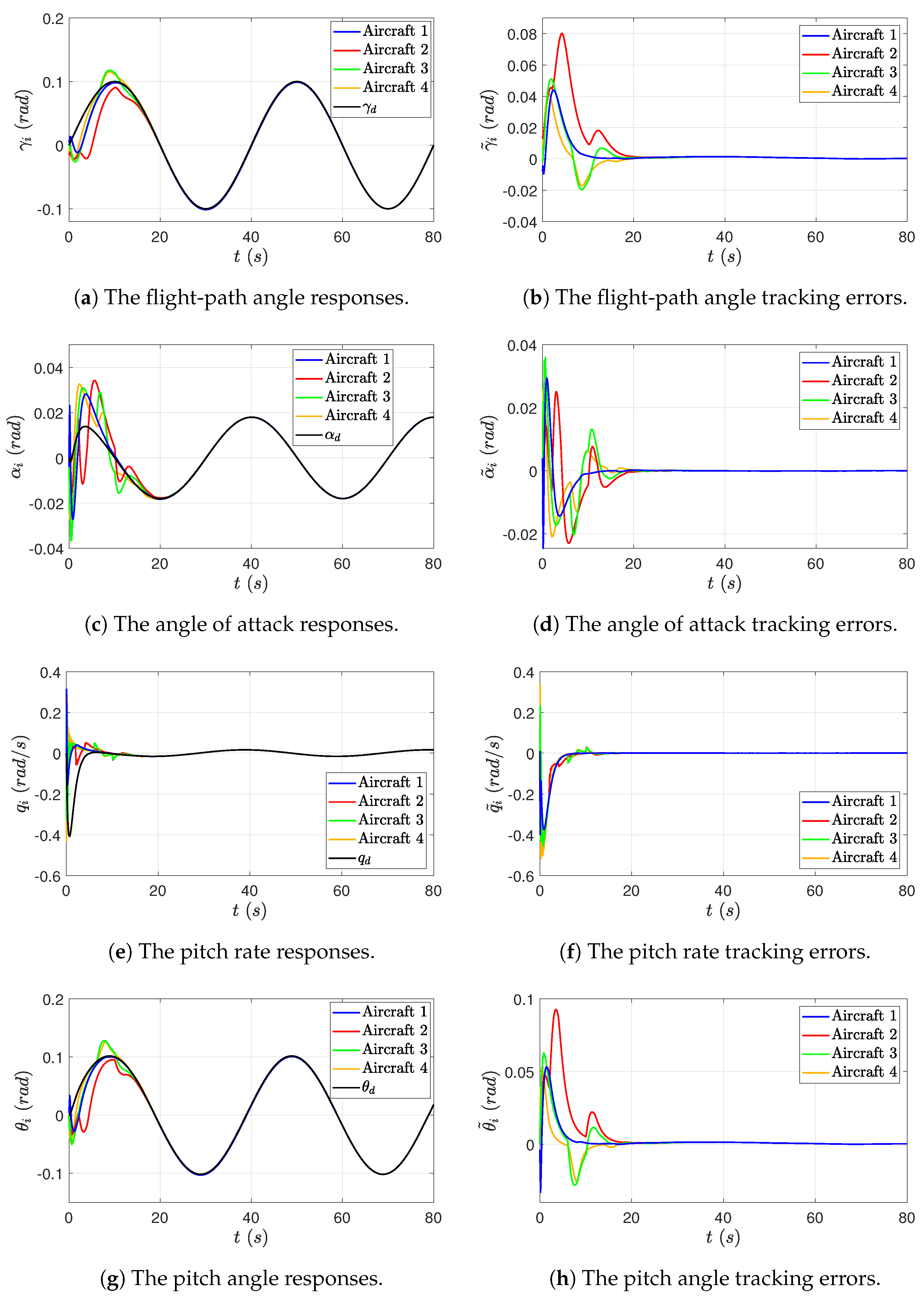
5.3.2. Simulation Results for Case 1
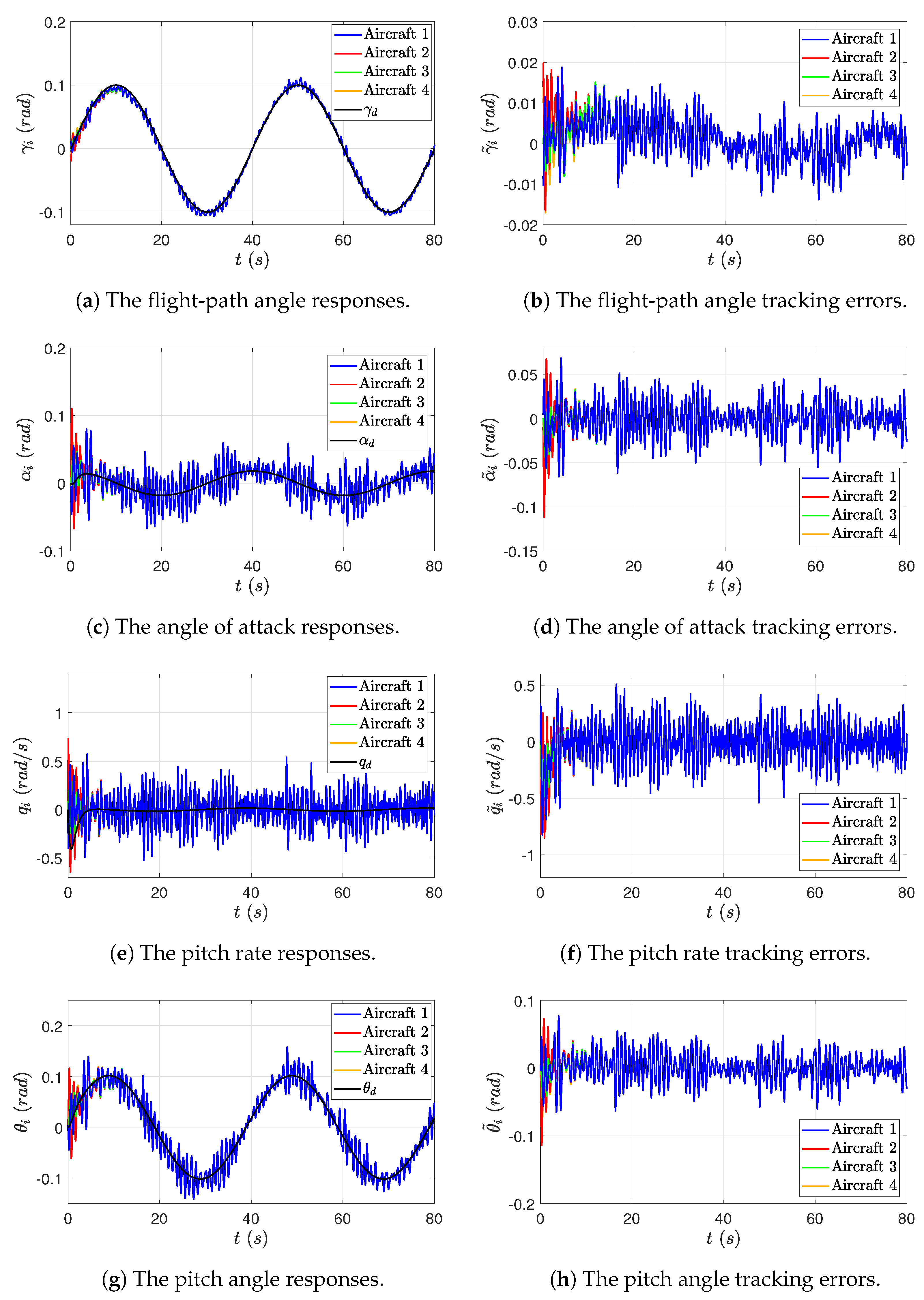
5.3.3. Simulation Results for Case 2
5.3.4. Simulation Results for Case 3
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| MEE | Measurement error estimator |
| PID | Proportional–integral–derivative |
References
- Beard, R.W.; McLain, T.W.; Nelson, D.B.; Kingston, D.; Johanson, D. Decentralized Cooperative Aerial Surveillance using Fixed-Wing Miniature UAVs. Proc. IEEE 2006, 94, 1306–1324. [Google Scholar] [CrossRef]
- Yu, Z.Q.; Zhang, Y.M.; Jiang, B.; Yu, X. Fault-Tolerant Time-Varying Elliptical Formation Control of Multiple Fixed-Wing UAVs for Cooperative Forest Fire Monitoring. J. Intell. Robot. Syst. 2021, 101, 48. [Google Scholar] [CrossRef]
- Meng, W.; He, Z.R.; Su, R.; Yadav, P.K.; Teo, R.; Xie, L.H. Decentralized Multi-UAV Flight Autonomy for Moving Convoys Search and Track. IEEE Trans. Control Syst. Technol. 2016, 25, 1480–1487. [Google Scholar] [CrossRef]
- Sun, Z.Y.; Garcia de Marina, H.; Anderson, B.D.; Yu, C.B. Collaborative Target-Tracking Control using Multiple Fixed-Wing Unmanned Aerial Vehicles with Constant Speeds. J. Guid. Control Dyn. 2021, 44, 238–250. [Google Scholar] [CrossRef]
- Breakwell, J.V. Optimal Flight-Path-Angle Transitions in Minimum-Time Airplane Climbs. J. Aircr. 1977, 14, 782–786. [Google Scholar] [CrossRef]
- de Lafontaine, J.; Lévesque, J.F.; Kron, A. Robust Guidance and Control Algorithms using Constant Flight Path Angle for Precision Landing on Mars. In Proceedings of the AIAA Guidance, Navigation, and Control Conference and Exhibit, Keystone, CO, USA, 21 August 2006; p. 6075. [Google Scholar]
- Zhu, Y.; Zhu, B.; Qin, K.Y.; Liu, H.H.T. Distributed Control for Flight-Path Angle Synchronized Tracking of Multiple Fixed-Wing Aircraft. In Proceedings of the 2016 IEEE Chinese Guidance, Navigation and Control Conference (CGNCC), Nanjing, China, 12 August 2016; pp. 312–317. [Google Scholar]
- Shi, L.; Cheng, Y.H.; Zhang, X.L.; Shao, J.L. Consensus Tracking Control of Discrete-Time Second-Order Agents over Switching Signed Digraphs with Arbitrary Antagonistic Relations. Int. J. Robust Nonlinear Control 2020, 30, 4826–4838. [Google Scholar] [CrossRef]
- Ning, B.D.; Han, Q.L.; Zuo, Z.Y. Bipartite Consensus Tracking for Second-Order Multi-Agent Systems: A Time-Varying Function-Based Preset-Time Approach. IEEE Trans. Autom. Control 2020, 66, 2739–2745. [Google Scholar] [CrossRef]
- Yoo, S.J.; Park, B.S. Connectivity-Preserving Approach for Distributed Adaptive Synchronized Tracking of Networked Uncertain Nonholonomic Mobile Robots. IEEE Trans. Cybern. 2017, 48, 2598–2608. [Google Scholar] [CrossRef]
- Wang, C.L.; Wen, C.Y.; Wang, W.; Hu, Q.L. Output-Feedback Adaptive Consensus Tracking Control for A Class of High-Order Nonlinear Multi-Agent Systems. Int. J. Robust Nonlinear Control 2017, 27, 4931–4948. [Google Scholar] [CrossRef]
- Ren, W. Consensus Tracking Under Directed Interaction Topologies: Algorithms and Experiments. IEEE Trans. Control Syst. Technol. 2010, 1, 230–237. [Google Scholar] [CrossRef]
- Parivallal, A.; Sakthivel, R.; Wang, C. Guaranteed Cost Leaderless Consensus for Uncertain Markov Jumping Multi-Agent Systems. J. Exp. Theor. Artif. Intell. 2023, 35, 257–273. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Zhu, Z.H.; Liao, F.; Gao, H.; Li, W.H.; Li, G. Finite-Time Adaptive Consensus Tracking Control Based on Barrier Function and Cascaded High-Gain Observer. Drones 2023, 7, 197. [Google Scholar] [CrossRef]
- Cao, W.J.; Liu, L.; Feng, G. Distributed Adaptive Output Consensus of Unknown Heterogeneous Non-Minimum Phase Multi-Agent Systems. IEEE/CAA J. Autom. Sin. 2023, 10, 997–1008. [Google Scholar] [CrossRef]
- Meng, T.Y.; Xie, Y.J.; Lin, Z.L. Suboptimal Output Consensus of A Group of Discrete-Time Heterogeneous Linear Non-Minimum Phase Systems. Syst. Control Lett. 2022, 161, 105134. [Google Scholar] [CrossRef]
- Shamsi, F.; Talebi, H.A.; Abdollahi, F. Output Consensus Control of Multi-Agent Systems with Nonlinear Non-Minimum Phase Dynamics. Int. J. Control 2018, 91, 785–796. [Google Scholar] [CrossRef]
- Yang, H.; Jiang, B.; Zhang, H.G. Stabilization of Non-Minimum Phase Switched Nonlinear Systems with Application to Multi-Agent Systems. Syst. Control Lett. 2012, 61, 1023–1031. [Google Scholar] [CrossRef]
- Lee, D. Robust Consensus of Linear Systems on Directed Graph with Non-Uniform Delay. IET Control Theory Appl. 2016, 10, 2574–2579. [Google Scholar] [CrossRef]
- Shamsi, F.; Talebi, H.A.; Abdollahi, F. Output Consensus Control of Nonlinear Non-Minimum Phase Multi-Agent Systems Using Output Redefinition Method. AUT J. Model. Simul. 2017, 49, 3–12. [Google Scholar]
- Li, W.H.; Zhu, Y.; Zhu, B.; Qin, K.Y.; Shi, M.J. Accurate Output Tracking Control for Nonminimum Phase Systems via An Iterative Redefinition-Based Method with Aircraft Application. In Proceedings of the 2022 34th Chinese Control and Decision Conference (CCDC), Hefei, China, 15 August 2022; pp. 4496–4502. [Google Scholar]
- Chen, B.; Chu, B.; Geng, H. Distributed Iterative Learning Control for Constrained Consensus Tracking Problem. In Proceedings of the 2020 59th IEEE Conference on Decision and Control (CDC), Jeju, Republic of Korea, 14 December 2020; pp. 3745–3750. [Google Scholar]
- Zhu, Y.; Chen, J.Y.; Zhu, B.; Qin, K.Y. Synchronised Trajectory Tracking for a Network of MIMO Non-Minimum Phase Systems with Application to Aircraft Control. IET Control Theory Appl. 2018, 12, 1543–1552. [Google Scholar] [CrossRef]
- Kodhanda, A.; Kolhe, J.P.; Zeru, T.; Talole, S.E. Robust Aircraft Control Based on UDE Theory. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2017, 231, 728–742. [Google Scholar] [CrossRef]
- Kawamura, S.; Cai, K.; Kishida, M. Distributed Output Regulation of Heterogeneous Uncertain Linear Agents. Automatica 2020, 119, 109094. [Google Scholar] [CrossRef]
- Zhang, M.R.; Saberi, A.; Stoorvogel, A.A. Synchronization for Heterogeneous Time-Varying Networks with Non-Introspective, Non-Minimum-Phase Agents in the Presence of External Disturbances with Known Frequencies. In Proceedings of the 2016 IEEE 55th Conference on Decision and Control (CDC), Las Vegas, NV, USA, 12 December 2016; pp. 5201–5206. [Google Scholar]
- Shkolnikov, I.A. Output Tracking in Causal Nonminimum-Phase Nonlinear Systems in Sliding Modes. Ph.D. Dissertation, Department of Electrical and Computer Engineering, University of Alabama in Huntsville, Huntsville, AL, USA, 22 June 2003. [Google Scholar]
- Su, Y.F.; Huang, J. Cooperative Output Regulation With Application to Multi-Agent Consensus Under Switching Network. IEEE Trans. Syst. Man Cybern. Part B Cybern. 2012, 42, 864–875. [Google Scholar]
- Gopalswamy, S.; Hedrick, J.K. Tracking Nonlinear Non-Minimum Phase Systems using Sliding Control. Int. J. Control 1993, 57, 1141–1158. [Google Scholar] [CrossRef]
- Devasia, S.; Chen, D.; Paden, B. Nonlinear Inversion-Based Output Tracking. IEEE Trans. Autom. Control 1996, 41, 930–942. [Google Scholar] [CrossRef]
- Shtessel, Y.B.; Baev, S.; Edwards, C.; Spurgeon, S. HOSM Observer for a Class of Non-Minimum Phase Causal Nonlinear MIMO Systems. IEEE Trans. Autom. Control 2010, 55, 543–548. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhu, B.; Liu, H.H.T.; Qin, K.Y. A Model-Based Approach for Measurement Noise Estimation and Compensation in Feedback Control Systems. IEEE Trans. Instrum. Meas. 2020, 69, 8112–8127. [Google Scholar] [CrossRef]
- Zhu, B.; Liu, H.H.T.; Li, Z. Robust Distributed Attitude Synchronization of Multiple Three-DOF Experimental Helicopters. IEEE Control Eng. Pract. 2015, 36, 87–99. [Google Scholar] [CrossRef]
- Khalil, H.K.; Grizzle, J.W. Nonlinear Systems, 3rd ed.; Prentice-Hall: Upper Saddle River, NJ, USA, 2002; pp. 176–177. [Google Scholar]
- Vidyasagar, M. On Undershoot and Nonminimum Phase Zeros. IEEE Trans. Autom. Control 1986, 31, 440. [Google Scholar] [CrossRef]
- Delabarra, B.A. On Undershoot in SISO Systems. IEEE Trans. Autom. Control 1994, 39, 578–581. [Google Scholar]







| Case No. | Control Approaches | Eq. | The Ultimate Bounds in the Absence of Measurement Errors | The Ultimate Bounds in the Presence of Measurement Errors |
|---|---|---|---|---|
| 1 | The proposed control | (37) | ||
| 2 | PID-based control | (80) | ||
| 3 | The proposed control under different | (37) | Not Applicable | () |
| () | ||||
| () |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Y.; Qin, K. Robust Flight-Path Angle Consensus Tracking Control for Non-Minimum Phase Unmanned Fixed-Wing Aircraft Formation in the Presence of Measurement Errors. Drones 2023, 7, 350. https://doi.org/10.3390/drones7060350
Zhu Y, Qin K. Robust Flight-Path Angle Consensus Tracking Control for Non-Minimum Phase Unmanned Fixed-Wing Aircraft Formation in the Presence of Measurement Errors. Drones. 2023; 7(6):350. https://doi.org/10.3390/drones7060350
Chicago/Turabian StyleZhu, Yang, and Kaiyu Qin. 2023. "Robust Flight-Path Angle Consensus Tracking Control for Non-Minimum Phase Unmanned Fixed-Wing Aircraft Formation in the Presence of Measurement Errors" Drones 7, no. 6: 350. https://doi.org/10.3390/drones7060350
APA StyleZhu, Y., & Qin, K. (2023). Robust Flight-Path Angle Consensus Tracking Control for Non-Minimum Phase Unmanned Fixed-Wing Aircraft Formation in the Presence of Measurement Errors. Drones, 7(6), 350. https://doi.org/10.3390/drones7060350






