Modelling Fluid Damping of Non-Conventional Vibration Modes in MEMS Resonators †
Abstract
:1. Introduction
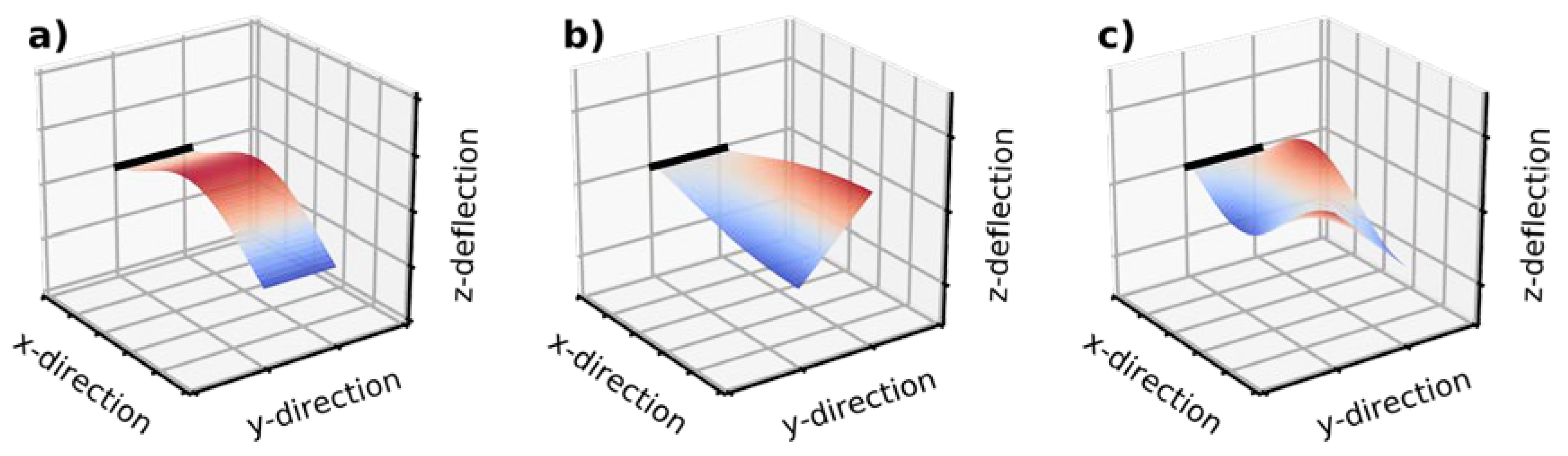
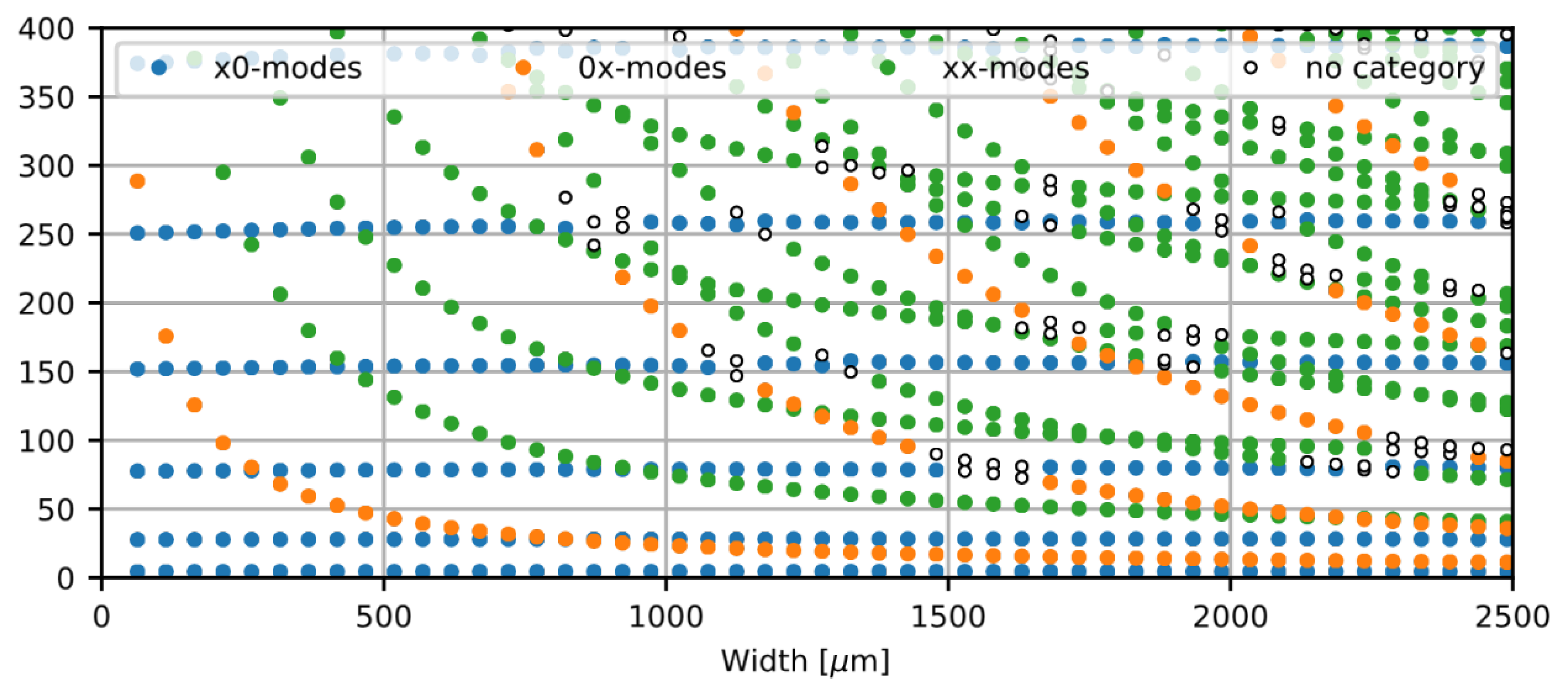
2. Results
3. Discussion
4. Conclusions
Author Contributions
References
- Van Eysden, C.A.; Sader, J.E. Frequency Response of Cantilever Beams Immersed in Viscous Fluids. In Resonant MEMS: Fundamentals, Implementation and Application; Brand, O., Ed.; Wiley-VCH: Weinheim, Germany, 2015; pp. 29–53. ISBN 978-3-527-67633-0. [Google Scholar]
- Kucera, M.; Wistrela, E.; Pfusterschmied, G.; Ruiz-Díez, V.; Manzaneque, T.; Luis Sánchez-Rojas, J.; Schalko, J.; Bittner, A.; Schmid, U. Characterization of a roof tile-shaped out-of-plane vibrational mode in aluminum-nitride-actuated self-sensing micro-resonators for liquid monitoring purposes. Appl. Phys. Lett. 2014, 104, 233501. [Google Scholar] [CrossRef]
- Logg, A.; Mardal, K.A.; Wells, G. (Eds.) Automated Solution of Differential Equations by the Finite Element Method; Springer: Berlin, Germany, 2012; ISBN 978-3-642-23098-1. [Google Scholar]
- Perkins, N.C.; Mote, C.D., Jr. Perkins and Mote, Comments on curve veering in eigenvalue problems. J. Sound Vib. 1986, 103, 451–463. [Google Scholar] [CrossRef]
- Gazzola, F.; Grunau, H.C.; Sweers, G. Polyharmonic Boundary Value Problems; Springer: Berlin, Germany, 2010; ISBN 978-3-642-12244-6. [Google Scholar]
- Tuck, E.O. Calculation of unsteady flows due to small motions of cylinders in a viscous fluid. J. Eng. Math. 1969, 3, 29–44. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Platz, D.; Pfusterschmied, G.; Schmid, U. Modelling Fluid Damping of Non-Conventional Vibration Modes in MEMS Resonators. Proceedings 2018, 2, 896. https://doi.org/10.3390/proceedings2130896
Platz D, Pfusterschmied G, Schmid U. Modelling Fluid Damping of Non-Conventional Vibration Modes in MEMS Resonators. Proceedings. 2018; 2(13):896. https://doi.org/10.3390/proceedings2130896
Chicago/Turabian StylePlatz, Daniel, Georg Pfusterschmied, and Ulrich Schmid. 2018. "Modelling Fluid Damping of Non-Conventional Vibration Modes in MEMS Resonators" Proceedings 2, no. 13: 896. https://doi.org/10.3390/proceedings2130896
APA StylePlatz, D., Pfusterschmied, G., & Schmid, U. (2018). Modelling Fluid Damping of Non-Conventional Vibration Modes in MEMS Resonators. Proceedings, 2(13), 896. https://doi.org/10.3390/proceedings2130896





