Clamping and Q-Factor Improvement in a Carbon Nanotube Resonator ^†

Abstract

This work reports the clamping effects on the performance of a carbon nanotube based nanoresonator. A direct comparison of two different nanotube-clamping geometries on the same nanotube device is presented. The nanotube was mechanically dry transferred and clamped through van-der-Waals forces onto palladium electrodes resulting in a bottom clamped configuration. A 20 nm platinum layer was then selectively deposited on the electrodes through atomic layer deposition resulting in top-bottom clamped configuration. With top clamping, a Q-factor increase of 1.5~2× has been observed accompanied by a decrease in the resonance frequency.

1. Introduction

Clamping losses have been argued to be a significant loss mechanism and especially limiting the performance of nanomechanical resonators [1,2] such as carbon nanotube (CNT) resonators, which suffer from low Q-factors (50–150) at room temperature [3]. The observed Q-factors in nanotubes and other nanoresonators are still largely unexplained and deviate from theoretical expectations [4]. While various reported experimental and theoretical work on CNTs assumed perfect clamping conditions, recently reported experimental investigations suggest clamping instability between CNT and the underlying metal contact [5,6]. Effect of different clamping geometries on carbon nanotube resonators at room temperature and low pressure to our knowledge has not been reported yet. Here, we report the effect on quality factor for the same resonator based on a single walled carbon nanotube (SWCNT) subjected to two different clamping configurations for direct comparison and investigations of clamping associated energy dissipation mechanisms. Raman spectroscopy, resonance measurements and DC transport measurements were performed for the same nanotube device to study the clamping effects.

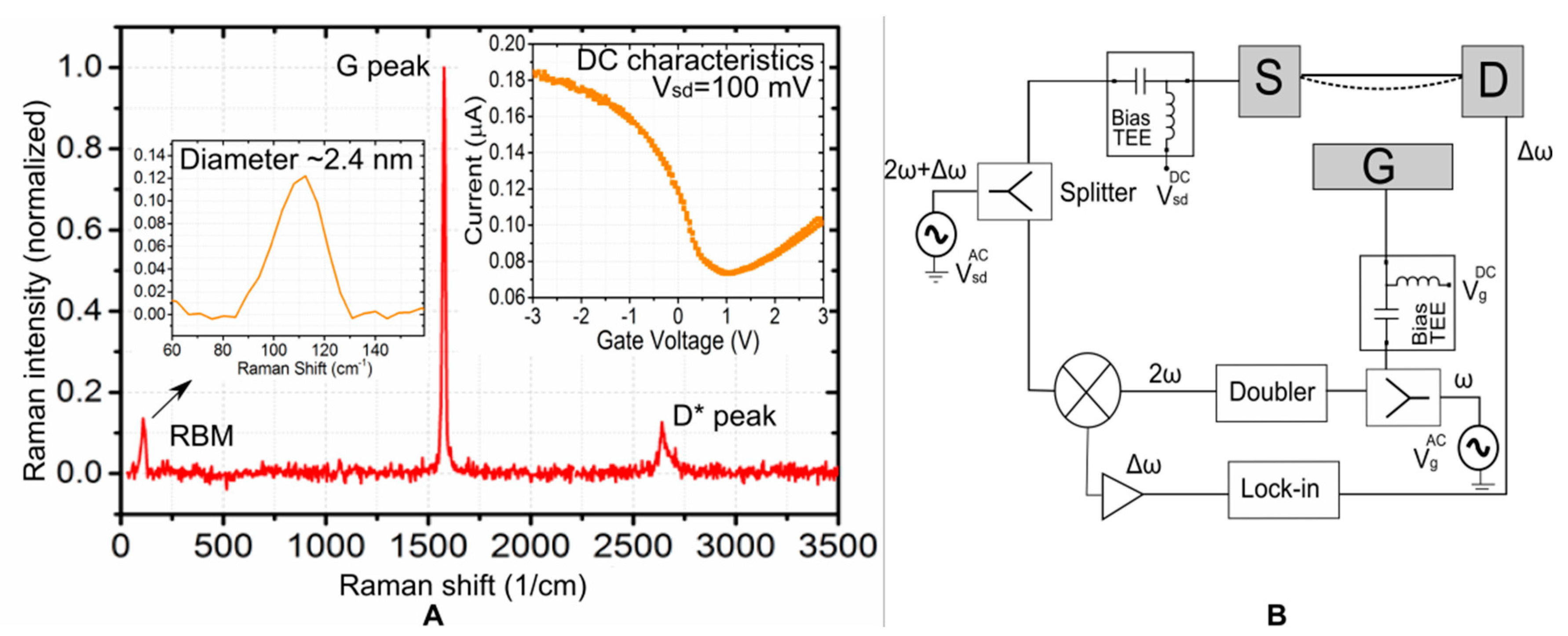
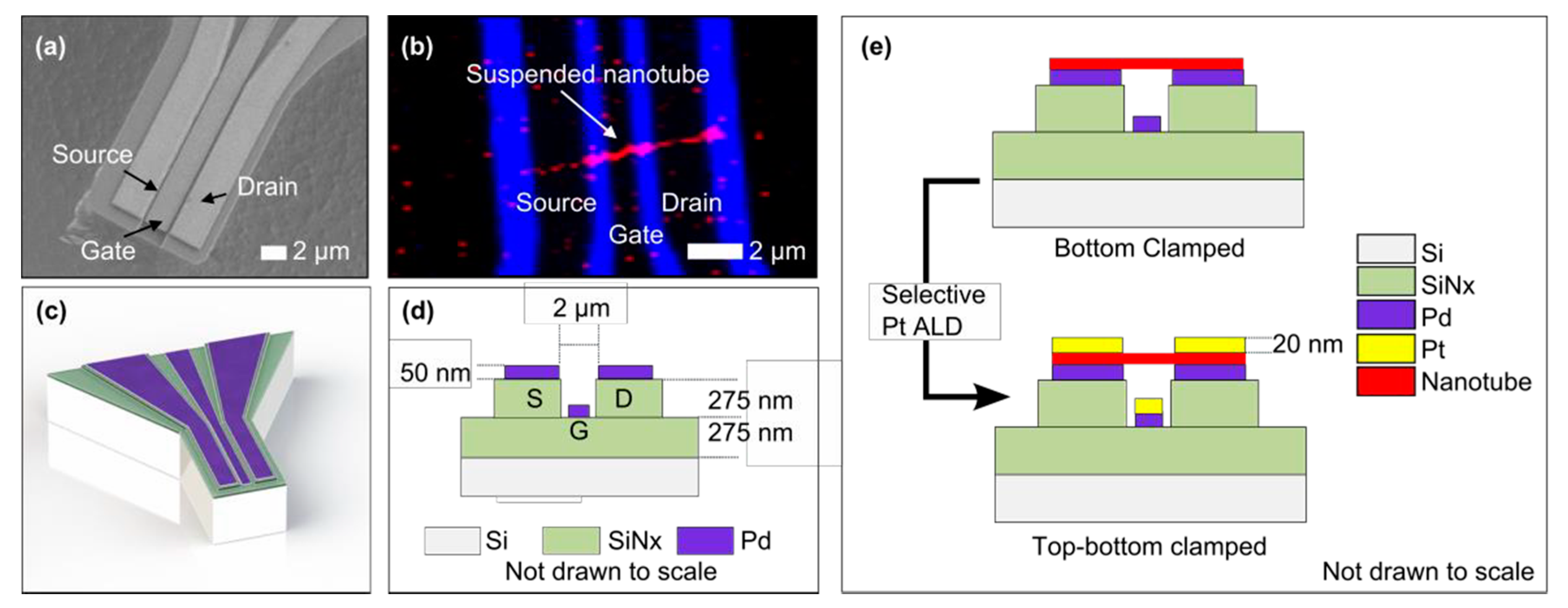
2. Fabrication and Methods

Our CNT resonator device is based on suspended field effect transistor (FET) architecture (Figure 1) with a designed channel length of 2 µm and channel-gate distance of 275 nm. FET devices were fabricated with silicon as device layer and palladium as source (S), drain (D) and gate (G) electrodes. Silicon nitride was used as a sandwiched layer and was deposited through plasma enhanced chemical vapor deposition (PECVD). This served as electrical insulation between silicon and palladium. The trench depth for gate electrode was etched through reactive ion etching (RIE) and palladium electrodes were evaporated through lift-off process [7]. The nanotubes were grown on a separate designated silicon chips and then mechanically dry-transferred [8] onto FET electrodes as reported in [5]. The nanotube is clamped down mechanically onto the palladium electrodes due to van-der-Waals forces resulting in a bottom clamped configuration. To exploit the same nanotube device for clamping effects, a 20 nm platinum layer was selectively deposited onto the metal electrodes through atomic layer deposition (ALD) [7,9]. This resulted in a top-bottom clamped configuration as shown in Figure 1e. The device was characterized (appendix A) by non-destructive Raman spectroscopy (Figure 1b) and CNT length of 2.2 µm and diameter of 2.4 nm (from radial breathing mode-RBM [10]) were obtained. The CNT-FET transfer characteristics reveals a small bandgap semiconducting (SGS) behavior. The dynamic response of CNT resonance was characterized through RF mixing technique [5] at room temperature (298 K) and vacuum (10⁻⁴ mbar).

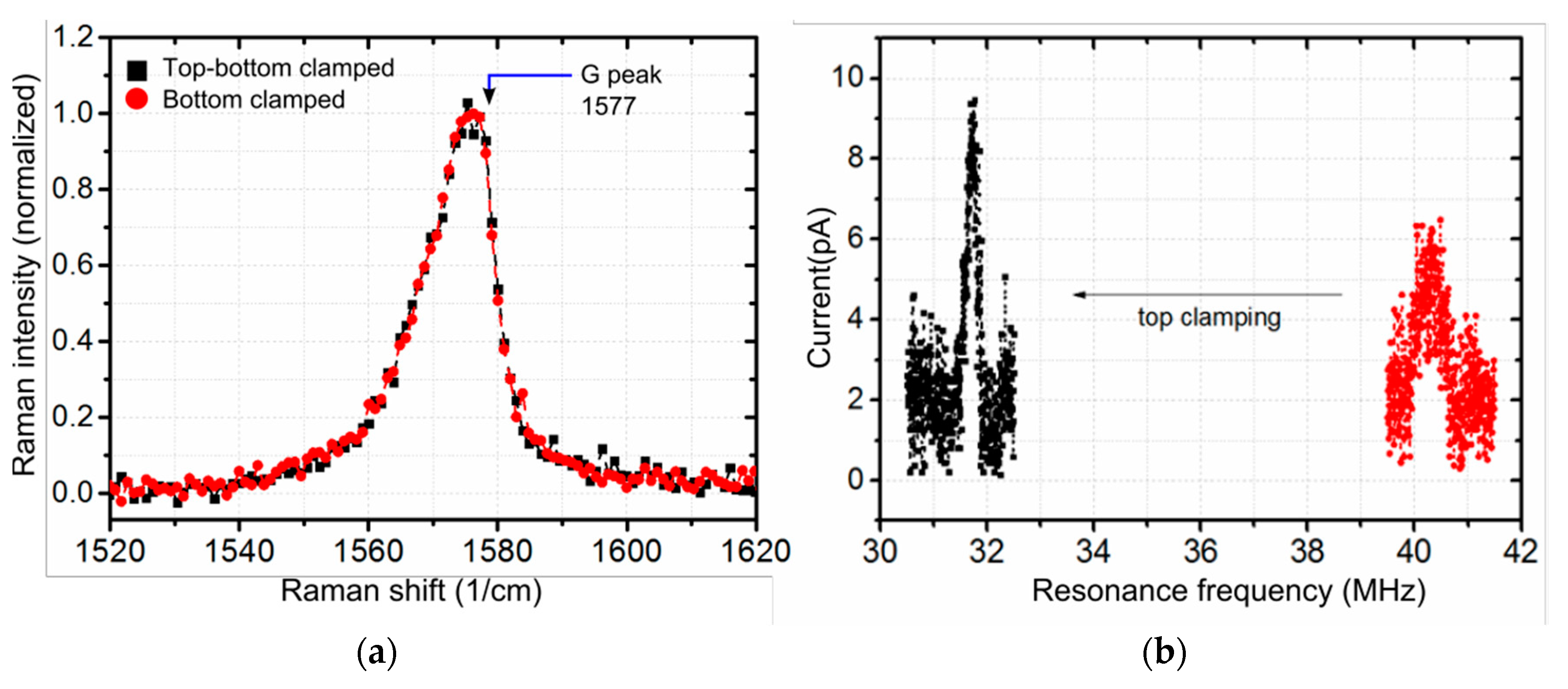
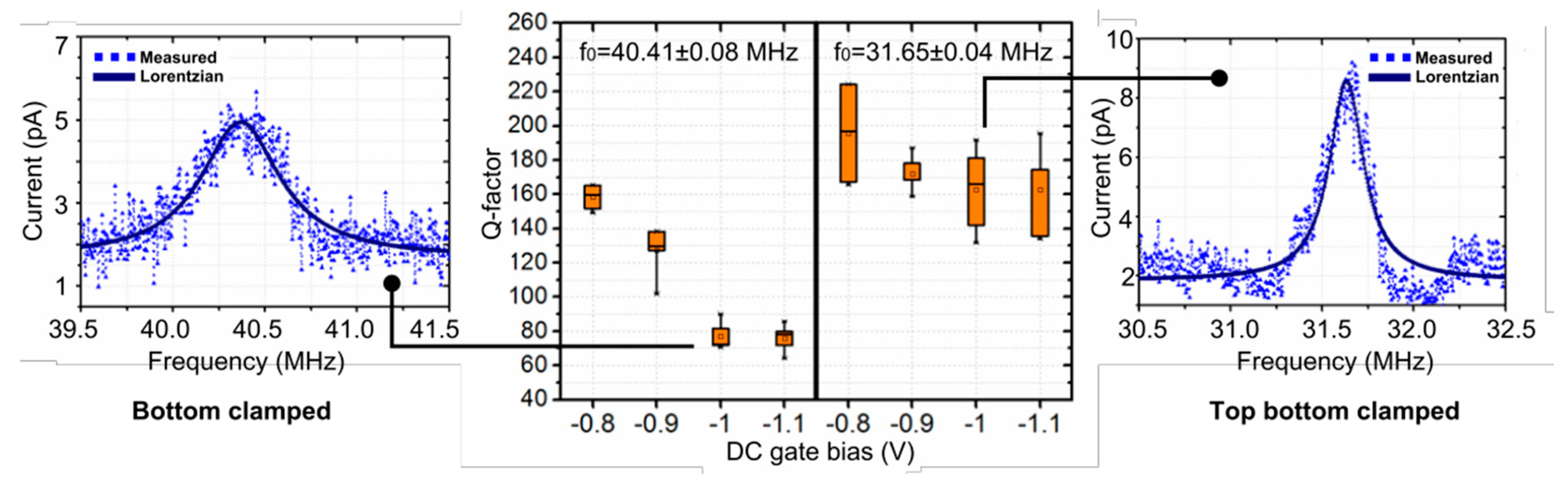
3. Experiment and Results

We investigated the Raman spectrum of the suspended nanotube before and after top clamping for a qualitative analysis as shown in Figure 2a. For both clamping configurations, Raman spectroscopy showed no significant change in G-peak position and broadening and no detectable D (defect) peak for the nanotube after the platinum ALD process suggesting no significant change in stress and structural quality. An initial resonance frequency of 40.2 MHz for bottom clamped device decreased to 31.5 MHz as a result of top clamping as shown in Figure 2b.

The direct comparison on Q-factor for top-bottom clamped vs bottom clamped device shows ~1.5–2× enhancement as shown in Figure 3 despite of resonance frequency decrease. The improvement was observed for various DC gate biases. Dependence of Q factor on DC gate bias was also visible with no significant increase in resonance frequency. The spectral broadening with increasing magnitude of DC bias could be attributed to increase in electrostatic noise; however, the off-resonance current shows no DC bias dependence. This observed dependence was attributed to amplitude dependent broadening as reported previously in carbon nanotube resonators [11].

4. Discussion and Conclusions

From the direct comparison of the same nanotube device, the observed enhancement in Q-factors suggests the influence of external mechanisms associated to clamping rather than internal mechanisms. Under the assumption of weak nature of clamping [5,6] resulting in imperfect boundary conditions, energy dissipation might arise from frictional losses due to sliding between the nanotube und the metal electrode or could be assisted though phonon-phonon interactions and edge vibrations as observed in graphene resonators [12]. The observation of amplitude dependent damping might suggest the role of geometric nonlinearities in energy dissipation mechanisms. The simultaneous increase of Q-factors and decrease in resonance frequency also requires further investigations on the effect of top metallization.