Improved Hermite–Hadamard Inequality Bounds for Riemann–Liouville Fractional Integrals via Jensen’s Inequality
Abstract
1. Introduction
2. Main Results
- If for any , there is a possibility Then, we have the following inequality for
- If , which is possible for odd n when has an equal distance and for every j, then
- is always greater than and is always less than , but there is a possibility of for any , then for the case , we have
- If n is odd, there is a possibility of single value whenever has uniform mesh with for every j and that particular value is then
- If for any , there is a possibility Then, we have the following inequality for
- If , which is possible for odd n when has an equal distance and for every j, then
- is always greater than and is always less than but there is a possibility of for any , then for the case , we have
- For the case we follow the same method used in Theorem 1 and we have
- If for any , there is a possibility for particular i, Then, we have the following inequality for
- If which is only possible for odd n when has equal distance and for every j, then
3. Application in Numerical Integration Formulas
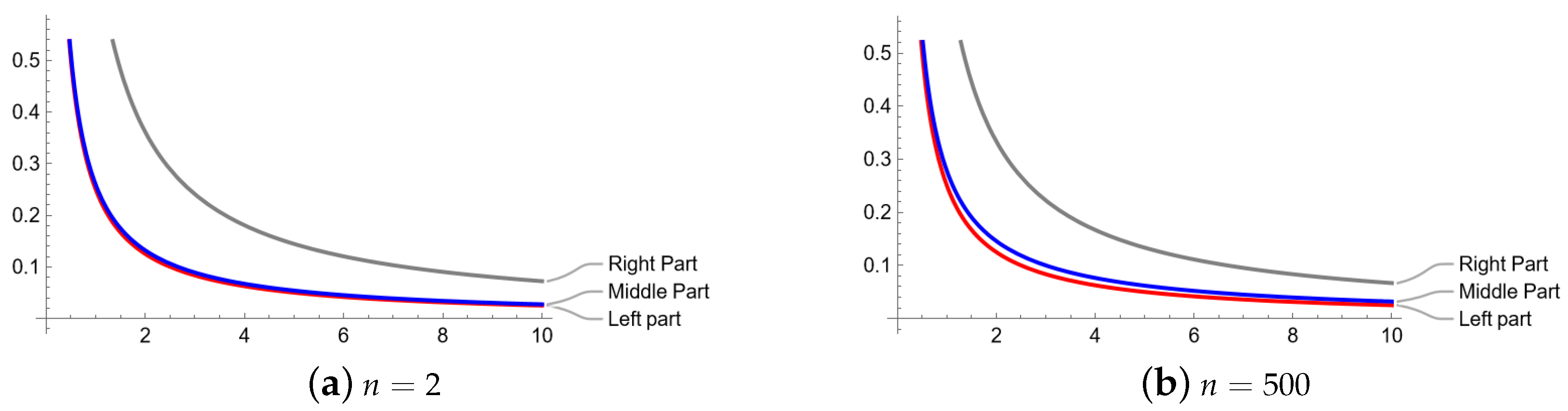
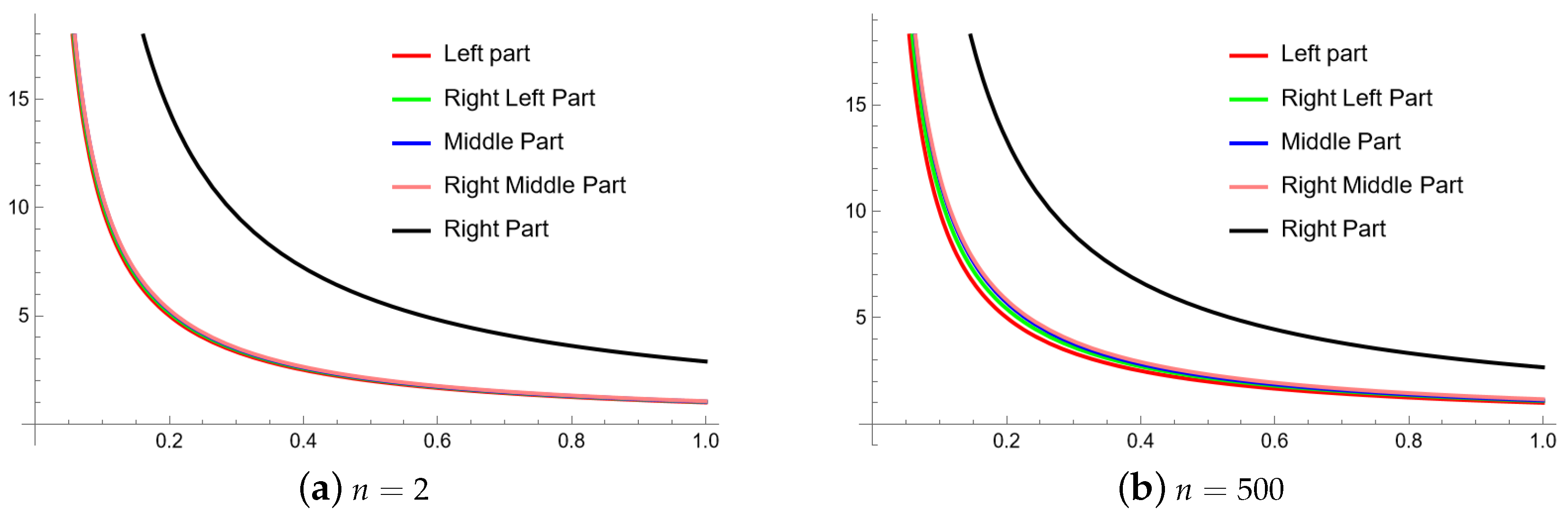
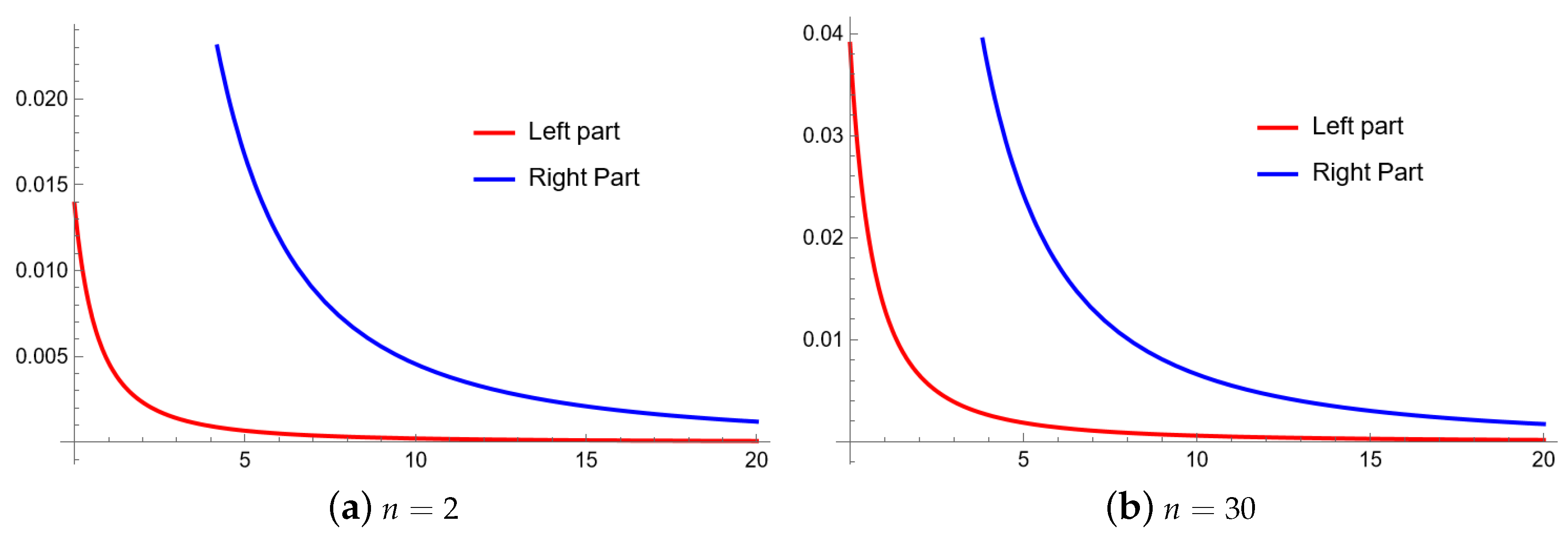
4. Numerical Examples
5. Concluding Remarks and Future Directions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ross, S.M. Introduction to Probability Models; Academic Press: Cambridge, MA, USA, 2014. [Google Scholar]
- Boyd, S.; Vandenberghe, L. Convex Optimization; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Cover, T.M. Elements of Information Theory; John Wiley & Sons: Hoboken, NJ, USA, 1999. [Google Scholar]
- Sohail, A.; Khan, M.A.; Nasr, E.A.; Ding, X. Further improvements of the Jensen inequality in the integral sense by virtue of 6-convexity along with applications. AIMS Math. 2024, 9, 11278–11303. [Google Scholar] [CrossRef]
- Pachpatte, B.G. Mathematical Inequalities; Elsevier: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Dragomir, S.S.; Agarwal, R. Two inequalities for differentiable mappings and applications to special means of real numbers and to trapezoidal formula. Appl. Math. Lett. 1998, 11, 91–95. [Google Scholar] [CrossRef]
- Kirmaci, U.S. Inequalities for differentiable mappings and applications to special means of real numbers to midpoint formula. Appl. Math. Comput. 2004, 147, 137–146. [Google Scholar] [CrossRef]
- Moradi, H.R.; Furuichi, S.; Mitroi, F.C.; Naseri, R. An extension of Jensen’s operator inequality and its application to Young inequality. Rev. Real Acad. Cienc. Exactas Fis. Nat. Ser. A Mat. 2019, 113, 605–614. [Google Scholar] [CrossRef]
- Mercer, A.M. A variant of Jensen’s inequality. J. Inequal. Pure Appl. Math. 2003, 4, 73. [Google Scholar]
- Matkovíc, A.; Pečríc, J.; Períc, I. A variant of Jensen’s inequality of Mercer’s type for operators with applications. Linear Algebra Appl. 2006, 418, 551–564. [Google Scholar] [CrossRef]
- Moradi, H.R.; Omidvar, M.E.; Khan, M.A.; Nikodem, K. Around Jensen’s inequality for strongly convex functions. Aequat. Math. 2018, 92, 25–37. [Google Scholar] [CrossRef]
- Moradi, H.R.; Furuichi, S. Improvement and generalization of some Jensen-Mercer-type inequalities. J. Math. Inequal. 2020, 14, 377–383. [Google Scholar] [CrossRef]
- Kian, M.; Moslehian, M.S. Refinements of the operator Jensen-Mercer inequality. Electron. J. Linear Algebra. 2013, 26, 742–753. [Google Scholar] [CrossRef]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; John Wiley: New York, NY, USA; London, UK, 1993. [Google Scholar]
- Oldham, K.B.; Spanier, J. The Fractional Calculus; Academic Press: New York, NY, USA; London, UK, 1974. [Google Scholar]
- Sarikaya, M.Z.; Set, E.; Yaldiz, H.; Başak, N. Hermite–Hadamard’s inequalities for fractional integrals and related fractional inequalities. Math. Comput. Model. 2013, 57, 2403–2407. [Google Scholar] [CrossRef]
- Sarikaya, M.Z.; Yildrim, H. On Hermite-Hadamard type inequalities for Riemann-Liouville fractional integrals. Miskolc Math. Notes 2016, 17, 1049–1059. [Google Scholar] [CrossRef]
- Peng, C.; Zhou, C.; Du, T.S. Riemann-Liouville fractional Simpson’s inequalities through generalized (λ1, h1, h2)-preinvexity. Ital. J. Pure Appl. Math. 2017, 38, 345–367. [Google Scholar]
- Sitthiwirattham, T.; Nonlaopon, K.; Ali, M.A.; Budak, H. Riemann-Liouville fractional Newton’s type inequalities for differentiable convex functions. Fractal Fract. 2022, 6, 175. [Google Scholar] [CrossRef]
- İşcan, x.; Wu, S. Hermite–Hadamard type inequalities for harmonically convex functions via fractional integrals. Appl. Math. Comput. 2014, 238, 237–244. [Google Scholar] [CrossRef]
- Sarikaya, M.Z.; Set, E.; Özdemir, M.E. On new inequalities of Simpson’s type for s-convex functions. Comput. Math. Appl. 2010, 60, 2191–2199. [Google Scholar] [CrossRef]
- Hyder, A.-A.; Almoneef, A.A.; Budak, H. Improvement in Some Inequalities via Jensen Mercer Inequality and Fractional Extended Riemann Liouville Integrals. Axioms 2023, 12, 886. [Google Scholar] [CrossRef]
- Öğülmxuxs, H.; Sarikaya, M.Z. Hermite–Hadamard–Mercer type inequalities for fractional integrals. Filomat 2021, 35, 2425–2436. [Google Scholar] [CrossRef]
- Kang, Q.; Butt, S.I.; Nazeer, W.; Nadeem, M.; Nasir, J.; Yang, H. New variant of Hermite–Jensen–Mercer inequalities via Riemann–Liouville fractional integral operators. J. Math. 2020, 2020, 4303727. [Google Scholar] [CrossRef]

| n | ||||
|---|---|---|---|---|
| 2 | 0.0111111 | 0.933333 | 0.944444 | |
| 4 | 0.02 | 0.88 | 0.9 | |
| 0.5 | 8 | 0.0259259 | 0.844444 | 0.87037 |
| 16 | 0.0294118 | 0.823529 | 0.852941 | |
| 32 | 0.0313131 | 0.812121 | 0.843434 | |
| 2 | 0.00793651 | 0.306878 | 0.314815 | |
| 4 | 0.0142857 | 0.285714 | 0.3 | |
| 1.5 | 8 | 0.0185185 | 0.271605 | 0.290123 |
| 16 | 0.0210084 | 0.263305 | 0.284314 | |
| 32 | 0.0223665 | 0.258778 | 0.281145 |
| n | ||||
|---|---|---|---|---|
| 0.5 | 0.0272727 | 0.836364 | 0.863636 | |
| 1.5 | 0.0194805 | 0.268398 | 0.287879 | |
| 10 | 2.5 | 0.0151515 | 0.157576 | 0.172727 |
| 3.5 | 0.0123967 | 0.11098 | 0.123377 | |
| 4.5 | 0.0104895 | 0.0854701 | 0.0959596 | |
| 0.5 | 0.0301587 | 0.819048 | 0.849206 | |
| 1.5 | 0.0215419 | 0.261527 | 0.283069 | |
| 20 | 2.5 | 0.0167548 | 0.153086 | 0.169841 |
| 3.5 | 0.0137085 | 0.107607 | 0.121315 | |
| 4.5 | 0.0115995 | 0.0827567 | 0.0943563 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, M.A.; Liu, W.; Furuichi, S.; Fečkan, M. Improved Hermite–Hadamard Inequality Bounds for Riemann–Liouville Fractional Integrals via Jensen’s Inequality. Fractal Fract. 2024, 8, 547. https://doi.org/10.3390/fractalfract8090547
Ali MA, Liu W, Furuichi S, Fečkan M. Improved Hermite–Hadamard Inequality Bounds for Riemann–Liouville Fractional Integrals via Jensen’s Inequality. Fractal and Fractional. 2024; 8(9):547. https://doi.org/10.3390/fractalfract8090547
Chicago/Turabian StyleAli, Muhammad Aamir, Wei Liu, Shigeru Furuichi, and Michal Fečkan. 2024. "Improved Hermite–Hadamard Inequality Bounds for Riemann–Liouville Fractional Integrals via Jensen’s Inequality" Fractal and Fractional 8, no. 9: 547. https://doi.org/10.3390/fractalfract8090547
APA StyleAli, M. A., Liu, W., Furuichi, S., & Fečkan, M. (2024). Improved Hermite–Hadamard Inequality Bounds for Riemann–Liouville Fractional Integrals via Jensen’s Inequality. Fractal and Fractional, 8(9), 547. https://doi.org/10.3390/fractalfract8090547







