Fractal Characteristics and Energy Evolution Analysis of Rocks under True Triaxial Unloading Conditions
Abstract
1. Introduction
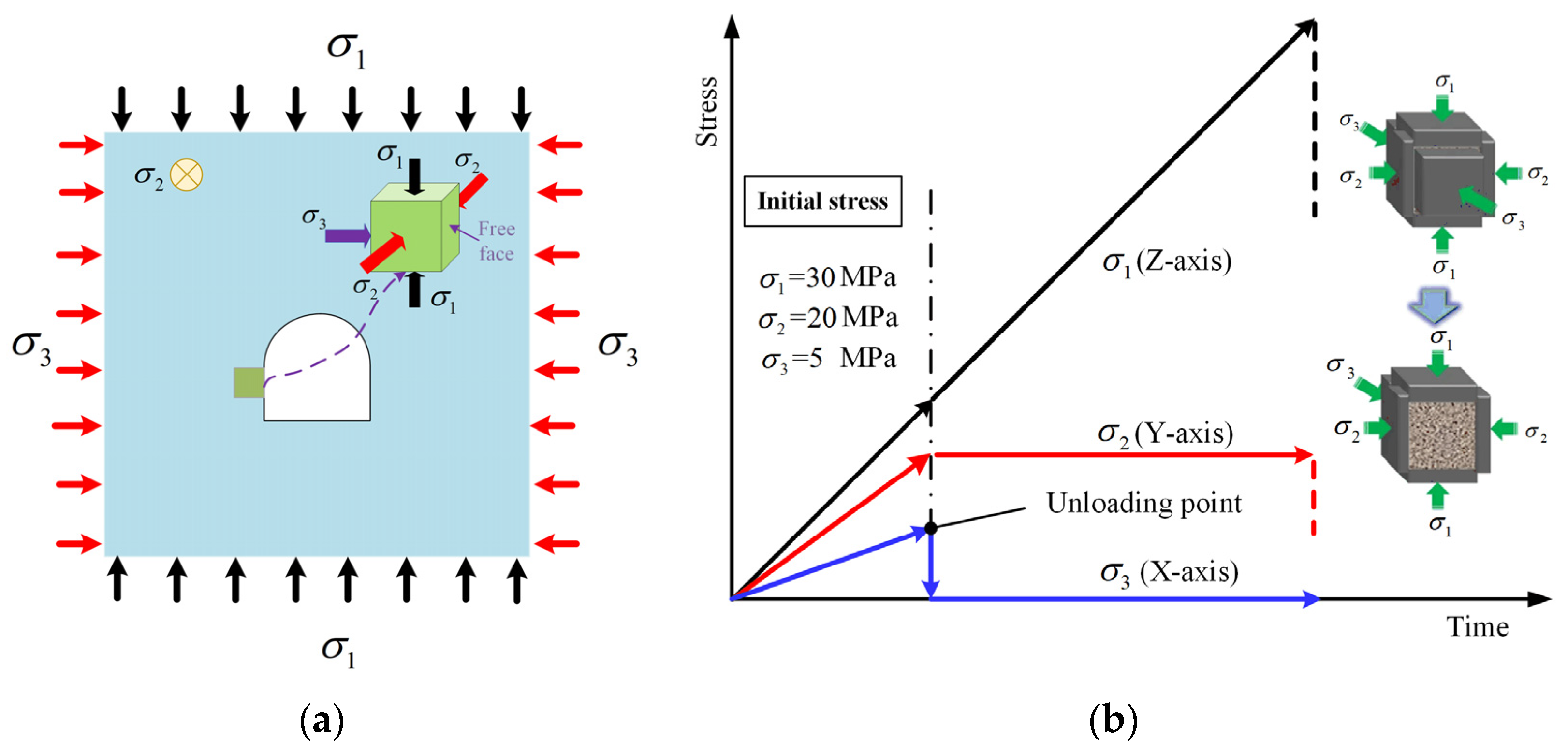
2. Experimental Materials and Program
2.1. Experimental Samples and Equipment
2.2. Experimental Program
3. Experimental Results and Analysis
3.1. Stress–Strain Curve Analysis
- Elastic stage (OA): In this stage, as the maximum principal stress increased, the relationship between stress and strain showed linear growth. In triaxial compression tests, the rock sample was initially loaded to a predetermined initial stress state. Due to the presence of initial confining pressure, the internal pore and fracture structures of the rock sample were compacted, and the development of new fractures during subsequent loading was not significant. Therefore, after reaching the initial stress and unloading, the rock sample was basically in the elastic stage.
- Yielding stage (AB): As the maximum principal stress continued to be loaded, the slope of the curve gradually decreased in a convex shape to peak stress, and the rock underwent plastic deformation. In this stage, there was a qualitative change in the development of microcracks within the rock sample, manifested by the initiation and expansion of new fractures. As stress continued to increase, the intensity and length of new fractures increased, while the overall stiffness of the rock sample gradually decreased. This resulted in a gradual decrease in the slope of the stress–strain curve.
- Softening stage (BC): After reaching peak strength, the internal structure of the specimen was damaged, causing stress to gradually decrease as strain continued to rise, resulting in a negative slope of the curve. Stress decreased to residual stress levels and then remained constant, while strain continued to increase until the specimen underwent noticeable breakdown, and the curve tended to develop along a horizontal path. Due to the low initial unloading level in this study, the single-sided transient unloading in the X direction did not directly cause rock failure. In contrast, constant loading was maintained in the Y direction, and continued loading in the Z direction resulted in rock failure. Therefore, under certain confining pressure, frictional forces were formed between the fractured rock blocks, providing residual bearing capacity.
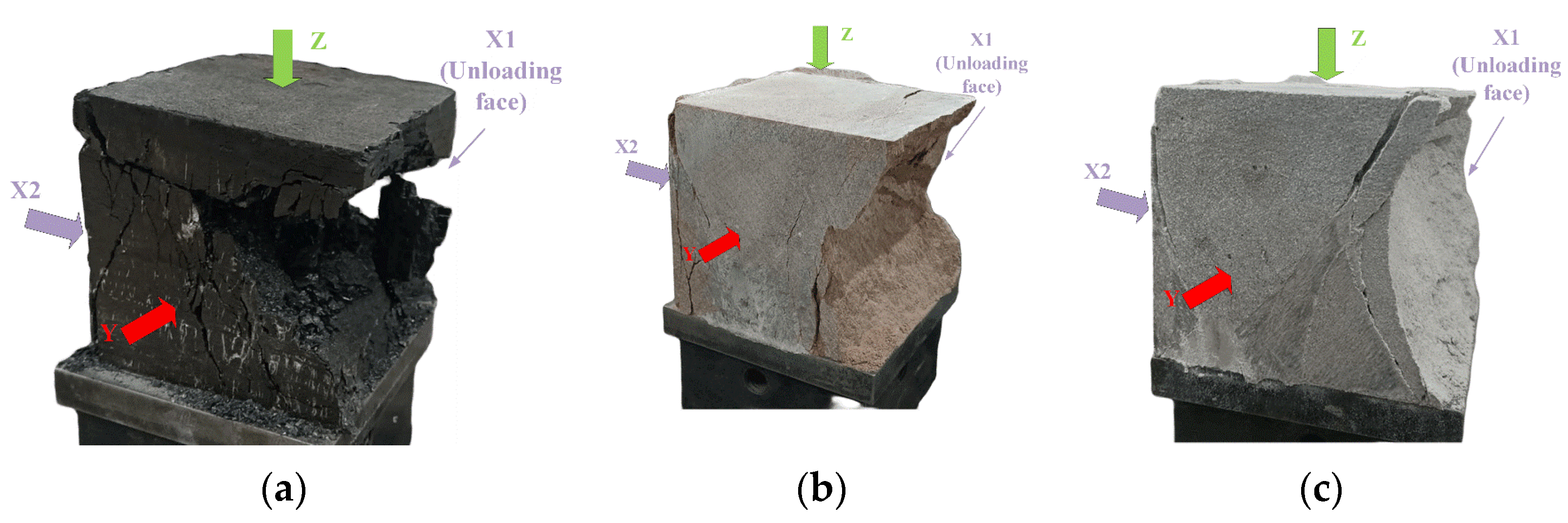
3.2. Failure Pattern Analysis
4. Numerical Simulation
4.1. Numerical Models and Schemes
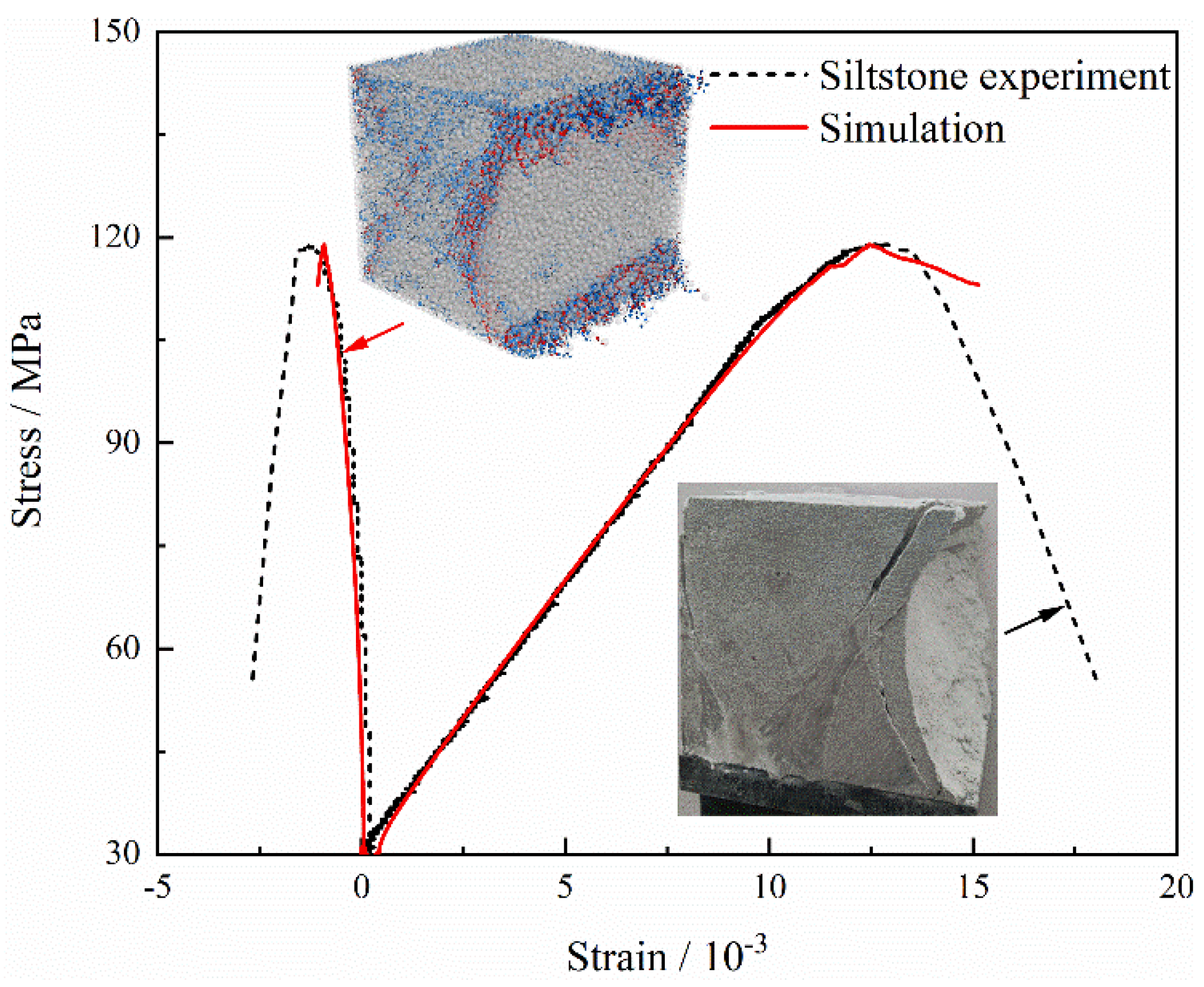
4.2. Calibration of Micro-Parameters
4.3. Energy Mechanism in PFC
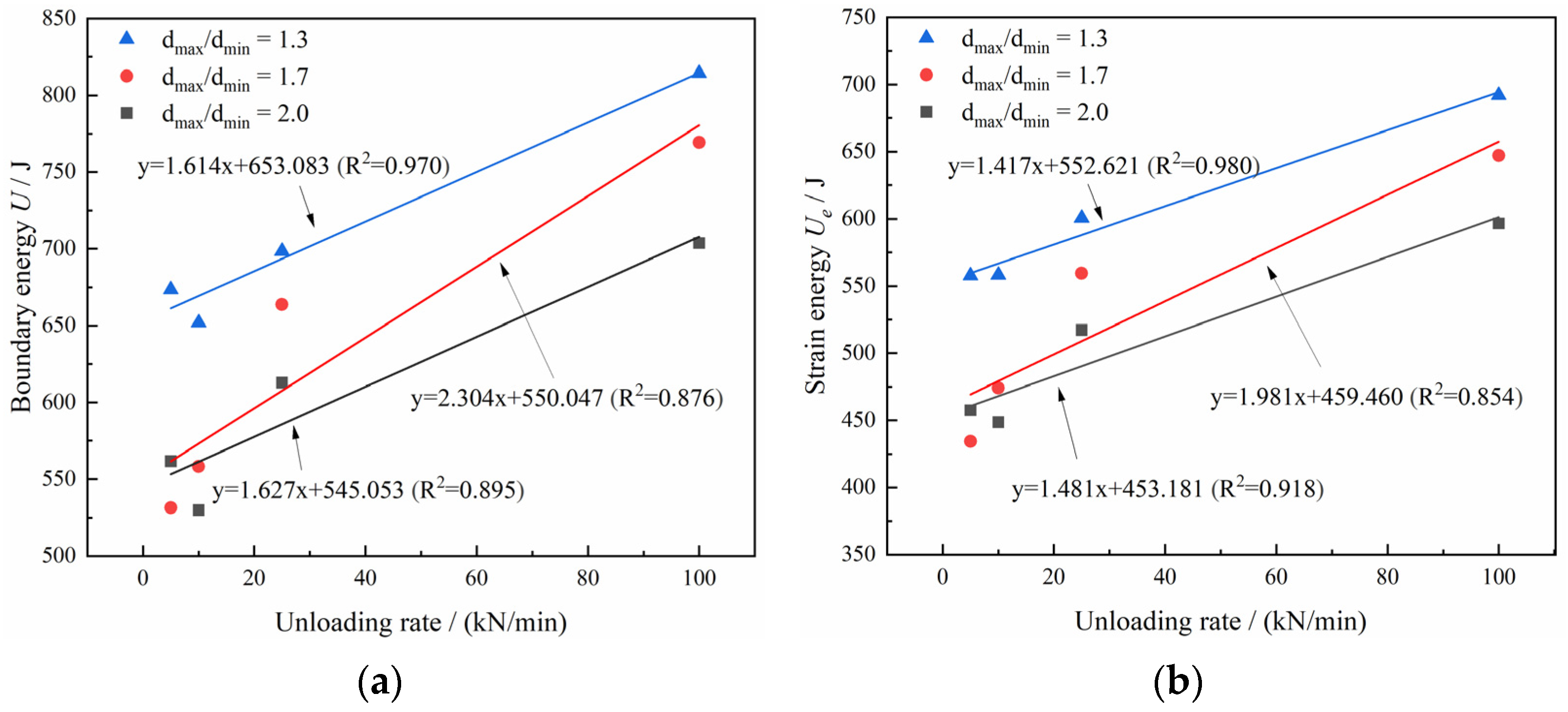
4.4. Energy Evolution Analysis
5. Discussion
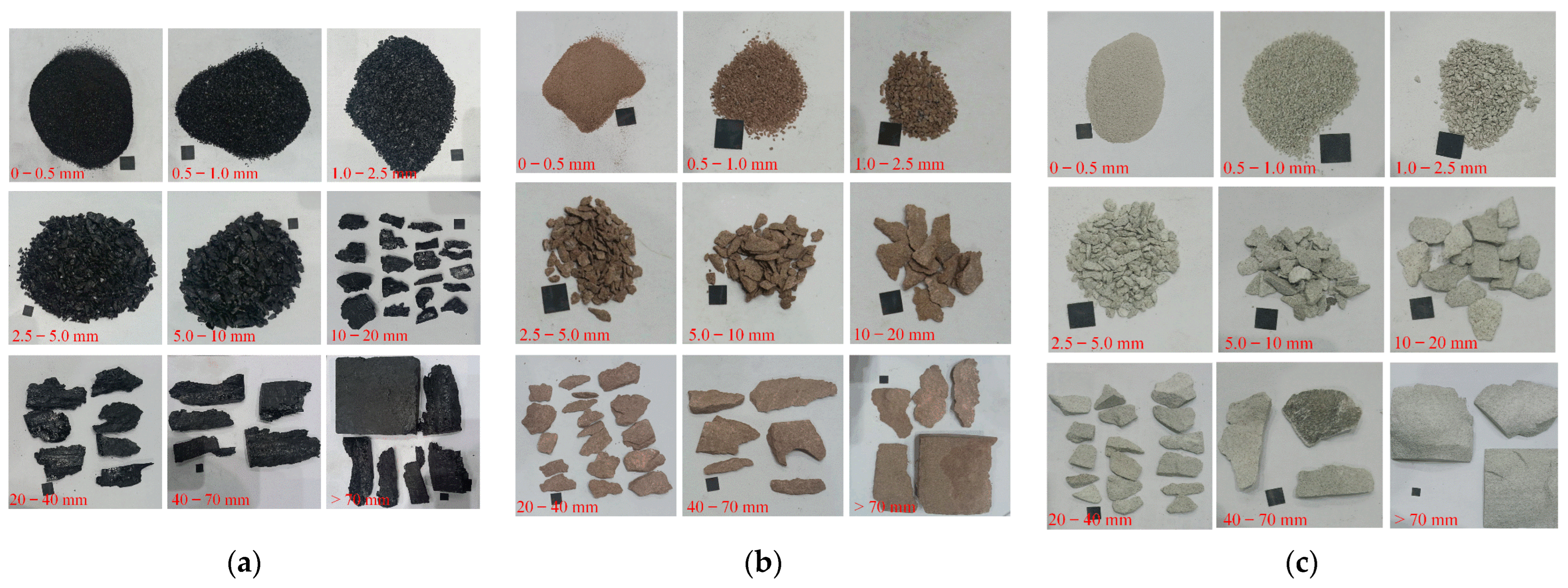
- Rocks are formed by the mutual adhesion of internal mineral particles, and the geometric and mechanical characteristics of mineral particles and adhesion, including particle size, shape, arrangement, adhesion contact relationships, adhesion fracture criteria, etc., determine the macro-mechanical properties of rock media, such as fracture characteristics. This study examined how micro-factors influence the mechanical properties and energy evolution of rocks under true triaxial unloading paths, with a focus on particle size distribution. Due to the complexity of micro-factors, further discussion is needed on spatial factors, such as mineral composition, adhesion contact relationships, and adhesion fracture criteria, not covered in this paper, as these variations in micro-factors have a significant impact on macro-mechanical properties.
- The mechanical properties and failure process of rock samples can be affected by several factors, including intermediate principal stress, unloading stress level, and unloading rate. Previous studies have indicated that as the unloading rate decreases, the peak strength of rocks and particle ejection kinetic energy decrease. This study reached a conclusion that is entirely at odds with previous findings. The discrepancy can be attributed to the unloading level. The unloading level selected for this study was below the rock’s damage stress, and single-sided unloading did not result in rock failure. The failure of the rocks occurred when the axial pressure continued to increase after unloading. Consequently, the impact of the unloading level will be the focus of the next stage of research.
6. Conclusions
- The stress–strain curves and failure modes of different types of rocks varied significantly under true triaxial unloading conditions. In this study, coal had the lowest peak strength and suffered the most severe damage, while sandstone had the highest peak strength and a more intact failure mode. The peak strength of rocks was negatively correlated with fractal dimension, meaning that rocks with lower peak strength exhibited larger final fractal dimensions of fragments and higher degrees of fragmentation.
- The unloading rate had a significant impact on the mechanical behavior and energy evolution of rocks. As the unloading rate increased, the peak strength, total energy, strain energy, and dissipation energy of rocks all showed an upward trend. It was noted that frictional energy played a key role in dissipation energy, with frictional energy positively correlated with the unloading rate, while kinetic energy was negatively correlated with the unloading rate. Additionally, under the same particle size distribution coefficient conditions, the total energy and strain energy of rocks approximately increased linearly with the increasing unloading rate.
- The heterogeneity of rocks played a crucial role in influencing the energy evolution characteristics of rock samples. As the heterogeneity of the sample increased, the total energy and strain energy stored at peak stress decreased. Additionally, the heterogeneity of rocks had a considerable influence on the distribution of dissipated energy. The frictional energy of a rock sample decreased as the grain size distribution coefficient increased, while the kinetic energy of the same sample increased as the grain size distribution coefficient increased.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, C.; Zhao, G.; Xu, W.; Meng, X.; Liu, Z.; Cheng, X.; Lin, G. Experimental Study on Failure Characteristics of Single-Sided Unloading Rock under Different Intermediate Principal Stress Conditions. Int. J. Min. Sci. Technol. 2023, 33, 275–287. [Google Scholar] [CrossRef]
- Akdag, S.; Karakus, M.; Taheri, A.; Nguyen, G.; He, M.C. Effects of Thermal Damage on Strain Burst Mechanism for Brittle Rocks Under True-Triaxial Loading Conditions. Rock Mech. Rock Eng. 2018, 51, 1657–1682. [Google Scholar] [CrossRef]
- Faradonbeh, R.S.; Taheri, A.; Sousa, L.R.; Karakus, M. Rockburst assessment in deep geotechnical conditions using true-triaxial tests and data-driven approaches. Int. J. Rock Mech. Min. 2020, 128, 104279. [Google Scholar] [CrossRef]
- He, M.C.; Zhao, F.; Cai, M.; Du, S. A Novel Experimental Technique to Simulate Pillar Burst in Laboratory. Rock Mech. Rock Eng. 2015, 48, 1833–1848. [Google Scholar] [CrossRef]
- Feng, X.T.; Xu, H.; Yang, C.; Zhang, X.; Gao, Y. Influence of Loading and Unloading Stress Paths on the Deformation and Failure Features of Jinping Marble Under True Triaxial Compression. Rock Mech. Rock Eng. 2020, 53, 3287–3301. [Google Scholar] [CrossRef]
- Li, X.; Feng, F.; Li, D.; Du, K.; Ranjith, P.G.; Rostami, J. Failure Characteristics of Granite Influenced by Sample Height-to-Width Ratios and Intermediate Principal Stress Under True-Triaxial Unloading Conditions. Rock Mech. Rock Eng. 2018, 51, 1321–1345. [Google Scholar] [CrossRef]
- Zhao, X.G.; Wang, J.; Cai, M.; Cheng, C.; Ma, L.K.; Su, R.; Zhao, F.; Li, D.J. Influence of Unloading Rate on the Strainburst Characteristics of Beishan Granite under True-Triaxial Unloading Conditions. Rock Mech. Rock Eng. 2014, 47, 467–483. [Google Scholar] [CrossRef]
- Du, K.; Tao, M.; Li, X.B.; Zhou, J. Experimental Study of Slabbing and Rockburst Induced by True-Triaxial Unloading and Local Dynamic Disturbance. Rock Mech. Rock Eng. 2016, 49, 3437–3453. [Google Scholar] [CrossRef]
- Si, X.; Gong, F. Strength-Weakening Effect and Shear-Tension Failure Mode Transformation Mechanism of Rockburst for Fine-Grained Granite under Triaxial Unloading Compression. Int. J. Rock Mech. Min. 2020, 131, 104347. [Google Scholar] [CrossRef]
- Tang, L.; Tu, S.; Tu, H.; Miao, K.; Li, W.; Zhao, H.; Ma, J.; Zhang, L. Compressive Failure Characteristics of a Coal–Rock Combination at Different Angles: Experimental Study and Fractal Analysis. Fractal Fract. 2024, 8, 240. [Google Scholar] [CrossRef]
- Ruiz-Carulla, R.; Corominas, J. Analysis of Rockfalls by Means of a Fractal Fragmentation Model. Rock Mech. Rock Eng. 2020, 53, 1433–1455. [Google Scholar] [CrossRef]
- Kruhl, J.H. Fractal-Geometry Techniques in the Quantification of Complex Rock Structures: A Special View on Scaling Regimes, Inhomogeneity and Anisotropy. J. Struct. Geol. 2013, 46, 2–21. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, E.; Li, N. Fractal Characteristics of Acoustic Emission Events Based on Single-Link Cluster Method during Uniaxial Loading of Rock. Chaos Solitons Fractals 2017, 104, 298–306. [Google Scholar] [CrossRef]
- Zhou, J.; Xu, W.; Zhao, G.; Meng, X.; Li, Y.; Wu, X.; Li, Y.; Cheng, X. Energy Evolution Law and Fractal Characteristics of Different Rock Specimen Sizes on Dynamic Compression. Geofluids 2022, 2022, 5339603. [Google Scholar] [CrossRef]
- Li, M.X.; Song, Y.H.; Zhang, G. Study on the Fractal Characteristics of Rock in the Prediction of Rockburst. RSC Adv. 2017, 7, 43073–43082. [Google Scholar] [CrossRef]
- Kong, X.; Wang, E.; Hu, S.; Shen, R.; Li, X.; Zhan, T. Fractal Characteristics and Acoustic Emission of Coal Containing Methane in Triaxial Compression Failure. J. Appl. Geophys. 2016, 124, 139–147. [Google Scholar] [CrossRef]
- Li, J.C.; Li, H.B.; Zhao, J. An Improved Equivalent Viscoelastic Medium Method for Wave Propagation across Layered Rock Masses. Int. J. Rock Mech. Min. 2015, 73, 62–69. [Google Scholar] [CrossRef]
- Xie, H.; Li, L.; Peng, R.; Ju, Y. Energy Analysis and Criteria for Structural Failure of Rocks. J. Rock Mech. Geotech. 2009, 1, 11–20. [Google Scholar] [CrossRef]
- Li, D.; Sun, Z.; Xie, T.; Li, X.; Ranjith, P.G. Energy Evolution Characteristics of Hard Rock during Triaxial Failure with Different Loading and Unloading Paths. Eng. Geol. 2017, 228, 270–281. [Google Scholar] [CrossRef]
- Zhang, L.; Cong, Y.; Meng, F.; Wang, Z.; Zhang, P.; Gao, S. Energy Evolution Analysis and Failure Criteria for Rock under Different Stress Paths. Acta Geotech. 2021, 16, 569–580. [Google Scholar] [CrossRef]
- Meng, Y.; Jing, H.; Liu, X.; Yin, Q. Effects of Initial Unloading Level on the Mechanical, Micro Failure and Energy Evolution Characteristics of Stratified Rock Mass under Triaxial Unloading Confining Pressure. Theor. Appl. Fract. Mech. 2023, 128, 104161. [Google Scholar] [CrossRef]
- Su, G.; Jiang, J.; Zhai, S.; Zhang, G. Influence of Tunnel Axis Stress on Strainburst: An Experimental Study. Rock Mech. Rock Eng. 2017, 50, 1551–1567. [Google Scholar] [CrossRef]
- Sun, F.; Guo, J.; Liu, X.; Zhang, H. Strain Rockburst Evolution Process under True Triaxial Condition with Single Face Unloading Due to Tunnel Excavation. Comput. Geotech. 2023, 159, 105465. [Google Scholar] [CrossRef]
- Gu, L.; Feng, X.T.; Kong, R.; Yang, C.; Han, Q.; Xia, Y. Excavation Stress Path Induced Fracturing Mechanism of Hard Rock in Deep Tunnel. Rock Mech. Rock Eng. 2023, 56, 1779–1806. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, S.; Zhang, X. Classification and Fractal Characteristics of Coal Rock Fragments under Uniaxial Cyclic Loading Conditions. Arab. J. Geosci. 2018, 11, 201. [Google Scholar] [CrossRef]
- Li, R.; Zhu, P.; Li, S.; Ding, C.; Lu, W.; Liu, Y. Fractal Behavior of Size Distribution and Specific Surface Area of Blasting Fragments. Appl. Sci. 2023, 13, 11832. [Google Scholar] [CrossRef]
- Pan, C.; Li, X.; Li, J.; Zhao, J. Numerical Investigation of Blast-Induced Fractures in Granite: Insights from a Hybrid LS-DYNA and UDEC Grain-Based Discrete Element Method. Geomech. Geophys. Geo-Energy Geo-Resour. 2021, 7, 49. [Google Scholar] [CrossRef]
- Wang, X.; Li, J.; Zhao, X.; Liang, Y. Propagation Characteristics and Prediction of Blast-Induced Vibration on Closely Spaced Rock Tunnels. Tunn. Undergr. Space Technol. 2022, 123, 104416. [Google Scholar] [CrossRef]
- Yan, Y.; Li, J.; Fukuda, D.; Liu, H. Experimental and Numerical Studies on Dynamic Fracturing Behavior of Roughly Jointed Rock. In Computational Particle Mechanics; Springer: Berlin/Heidelberg, Germany, 2024. [Google Scholar] [CrossRef]
- Ao, Y.; Jia, B.; Sun, C.; Liu, F. Fracture Characteristics and Energy Evolution Analysis of Pre-Cracked Granite under Uniaxial Compression Based on a 3D-Clump Model. Theor. Appl. Fract. Mech. 2023, 124, 103756. [Google Scholar] [CrossRef]
- Pan, C.; Li, X.; He, L.; Li, J. Study on the Effect of Micro-Geometric Heterogeneity on Mechanical Properties of Brittle Rock Using a Grain-Based Discrete Element Method Coupling with the Cohesive Zone Model. Int. J. Rock Mech. Min. 2021, 140, 104680. [Google Scholar] [CrossRef]
- Pan, C.; Zhao, G.; Meng, X.; Dong, C.; Gao, P. Numerical Investigation of the Influence of Mineral Mesostructure on Quasi-Static Compressive Behaviors of Granite Using a Breakable Grain-Based Model. Front. Ecol. Evol. 2023, 11, 1288870. [Google Scholar] [CrossRef]
- Blair, S.C.; Cook, N.G.W. Analysis of Compressive Fracture in Rock Using Statistical Techniques: Part II. Effect of Microscale Heterogeneity on Macroscopic Deformation. Int. J. Rock Mech. Min. 1998, 35, 849–861. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D.L. A Discrete Numerical Model for Granular Assemblies. Geotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Yuan, W.; Cheng, Y.; Min, M.; Wang, X. Study on Acoustic Emission Characteristics during Shear Deformation of Rock Structural Planes Based on Particle Flow Code. Comput. Part. Mech. 2024, 11, 105–118. [Google Scholar] [CrossRef]





| Particle Parameters | Value | Parallel Bond Parameters | Value |
|---|---|---|---|
| Density (kg·m−3) | 3178 | Parallel bond modulus (GPa) | 2.0 |
| Young’s modulus (GPa) | 6.3 | Parallel bond tensile strength (MPa) | 26.6 ± 4 |
| Friction coefficient | 0.3 | Parallel bond shear strength (MPa) | 16.6 ± 2 |
| Minimum particle radius (mm) | 1.1 | Parallel bond stiffness ratio | 1.5 |
| Maximum particle radius (mm) | 1.9 | ||
| Ratio of normal to shear stiffness | 0.8 |
| dmax/dmin = 1.3 | dmax/dmin = 1.7 | dmax/dmin = 2.0 | |
|---|---|---|---|
| 5 kN/min | 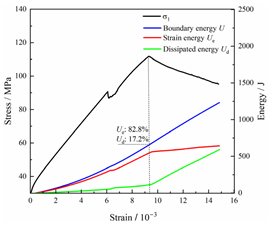 | 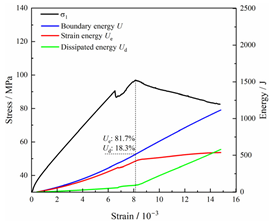 | 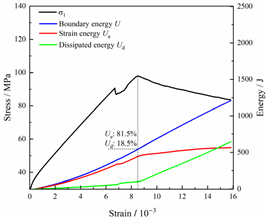 |
| 10 kN/min | 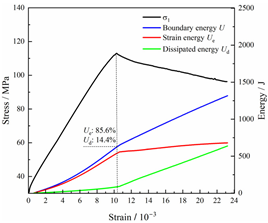 | 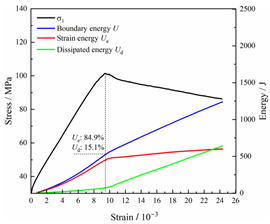 |  |
| 25 kN/min | 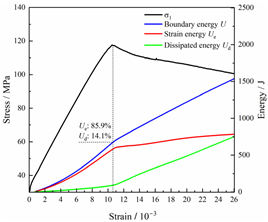 | 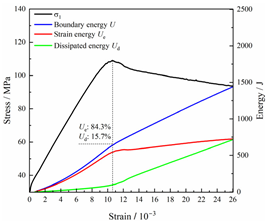 | 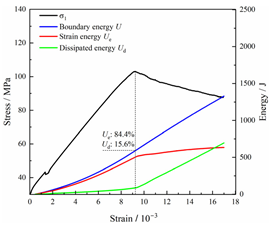 |
| 100 kN/min | 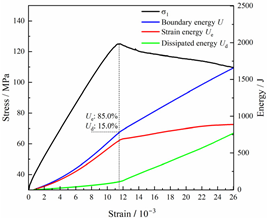 |  | 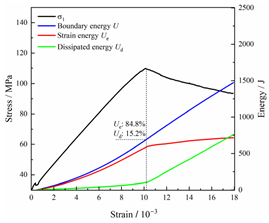 |
| Transient unloading | 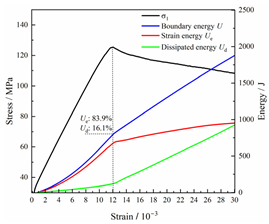 |  | 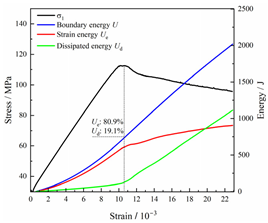 |
| dmax/dmin = 1.3 | dmax/dmin = 1.7 | dmax/dmin = 2.0 | |
|---|---|---|---|
| 5 kN/min |  | 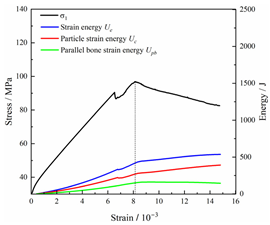 |  |
| 10 kN/min | 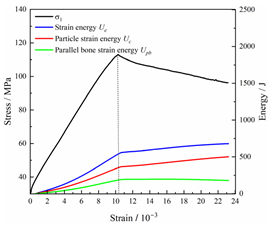 | 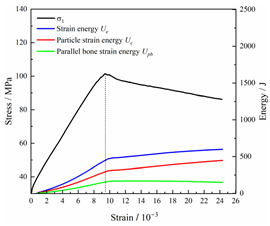 |  |
| 25 kN/min | 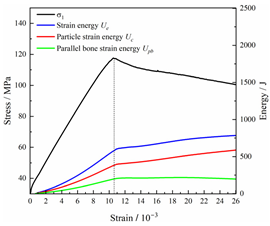 | 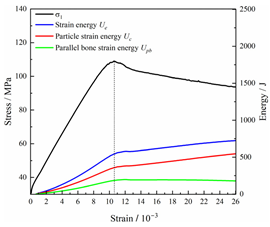 | 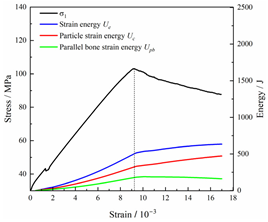 |
| 100 kN/min | 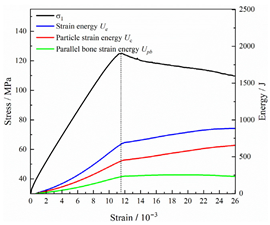 | 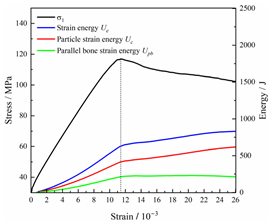 |  |
| Transient unloading | 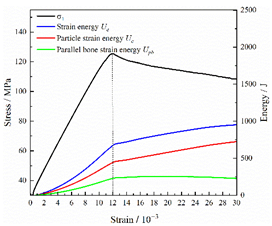 |  | 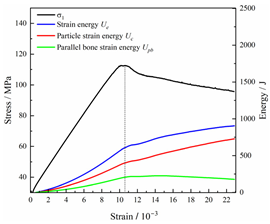 |
| dmax/dmin = 1.3 | dmax/dmin = 1.7 | dmax/dmin = 2.0 | |
|---|---|---|---|
| 5 kN/min |  |  | 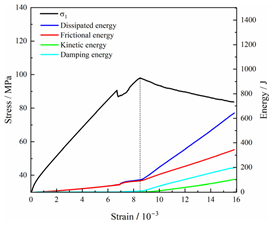 |
| 10 kN/min |  | 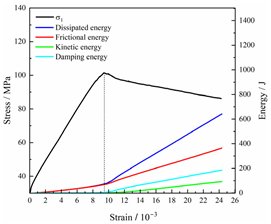 | 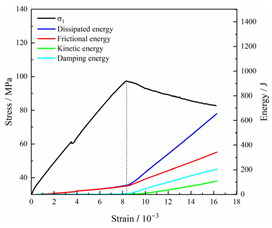 |
| 25 kN/min | 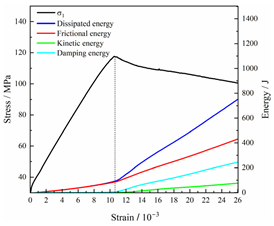 | 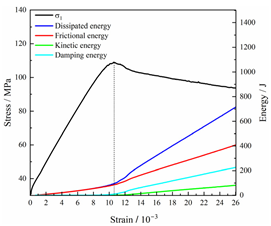 |  |
| 100 kN/min | 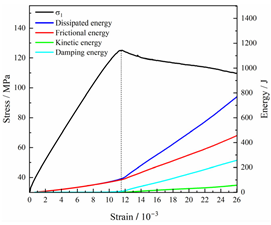 |  | 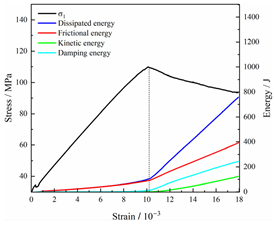 |
| Transient unloading | 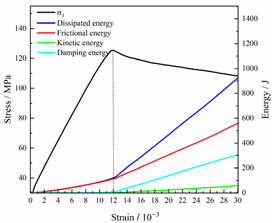 | 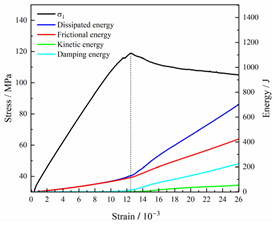 |  |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pan, C.; Liu, C.; Zhao, G.; Yuan, W.; Wang, X.; Meng, X. Fractal Characteristics and Energy Evolution Analysis of Rocks under True Triaxial Unloading Conditions. Fractal Fract. 2024, 8, 387. https://doi.org/10.3390/fractalfract8070387
Pan C, Liu C, Zhao G, Yuan W, Wang X, Meng X. Fractal Characteristics and Energy Evolution Analysis of Rocks under True Triaxial Unloading Conditions. Fractal and Fractional. 2024; 8(7):387. https://doi.org/10.3390/fractalfract8070387
Chicago/Turabian StylePan, Cheng, Chongyan Liu, Guangming Zhao, Wei Yuan, Xiao Wang, and Xiangrui Meng. 2024. "Fractal Characteristics and Energy Evolution Analysis of Rocks under True Triaxial Unloading Conditions" Fractal and Fractional 8, no. 7: 387. https://doi.org/10.3390/fractalfract8070387
APA StylePan, C., Liu, C., Zhao, G., Yuan, W., Wang, X., & Meng, X. (2024). Fractal Characteristics and Energy Evolution Analysis of Rocks under True Triaxial Unloading Conditions. Fractal and Fractional, 8(7), 387. https://doi.org/10.3390/fractalfract8070387







