Multifractal Properties of Human Chromosome Sequences
Abstract
1. Introduction
2. Theoretical Background
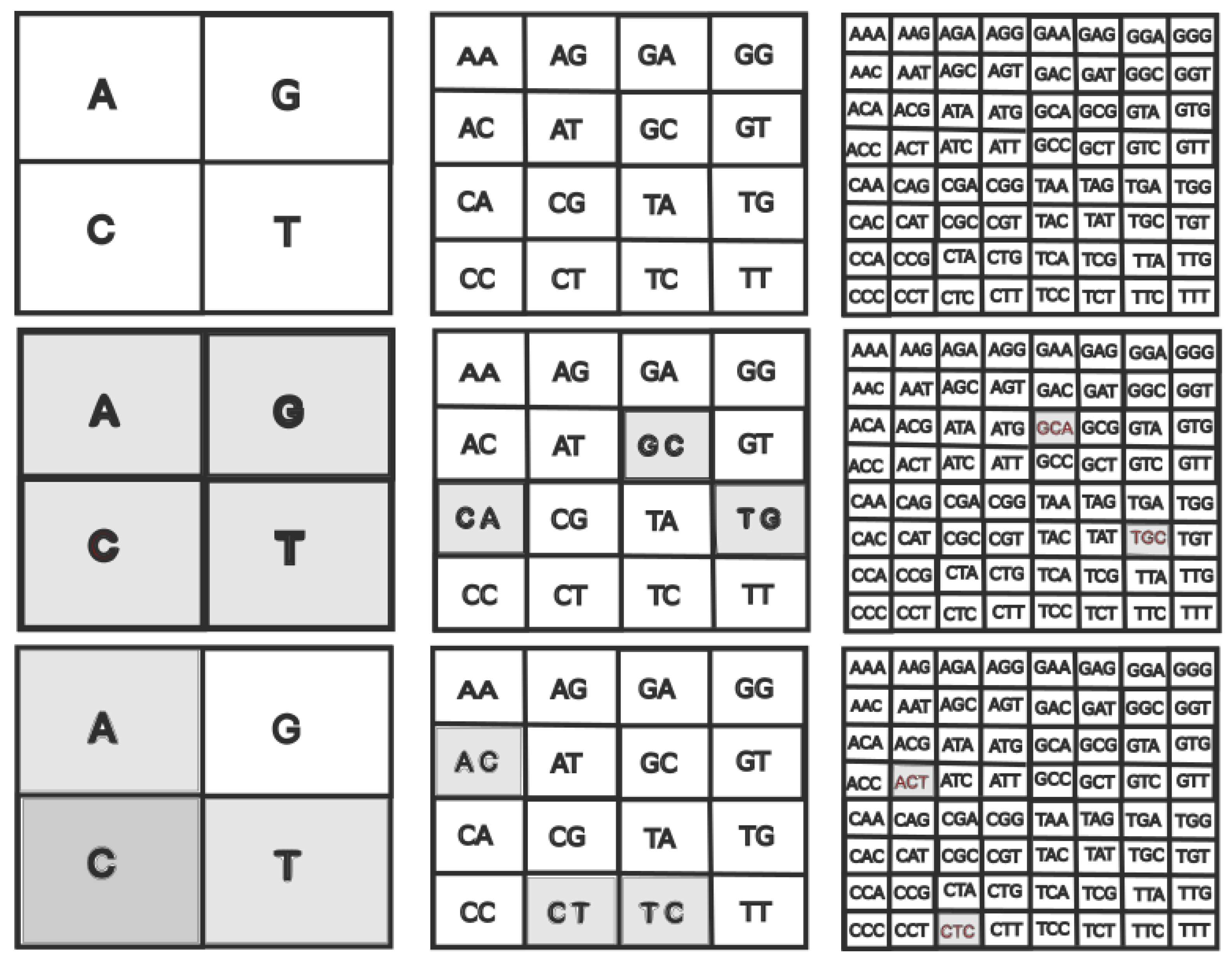
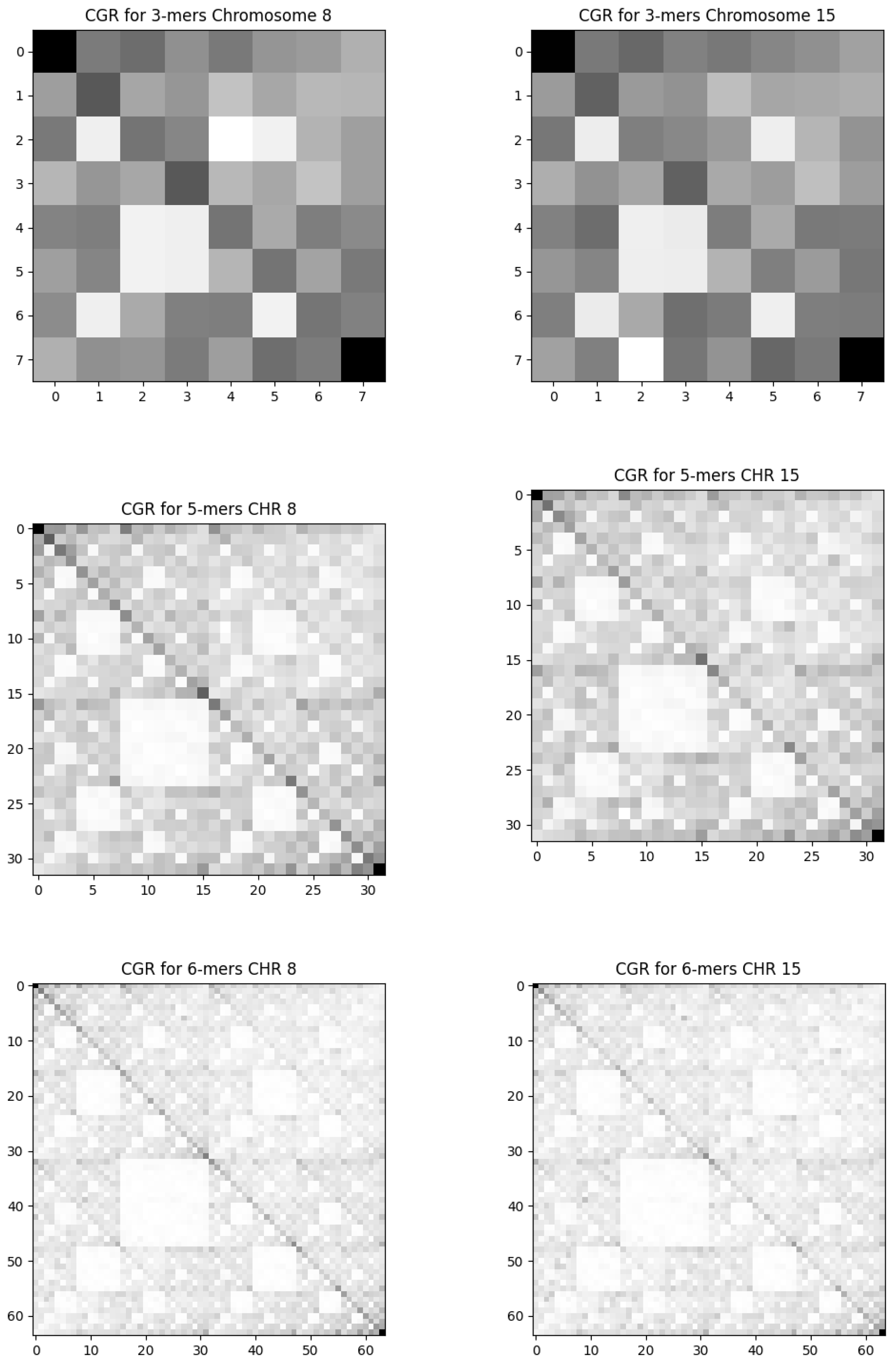
2.1. Chaos Game Representation
Global Distance
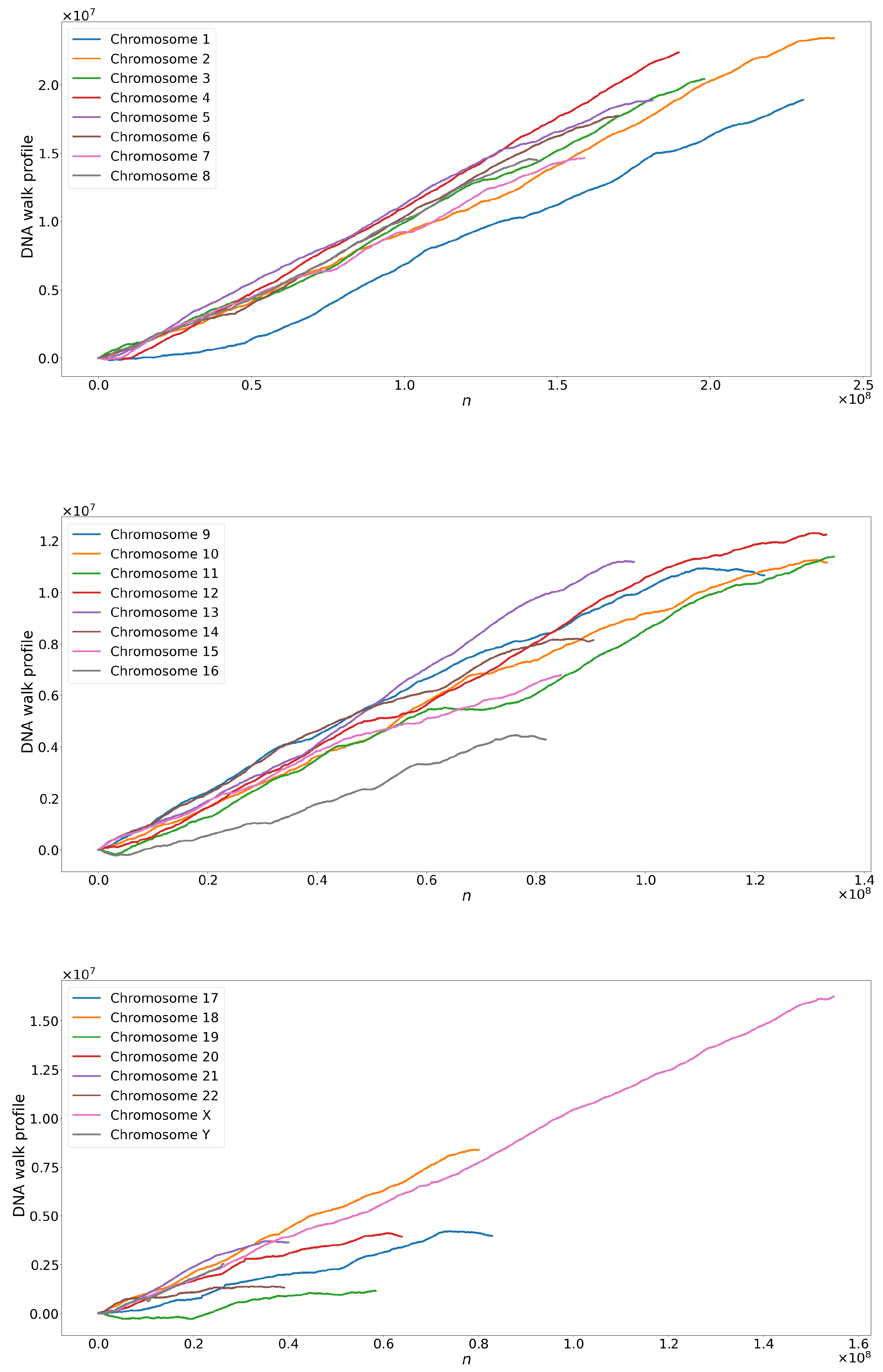
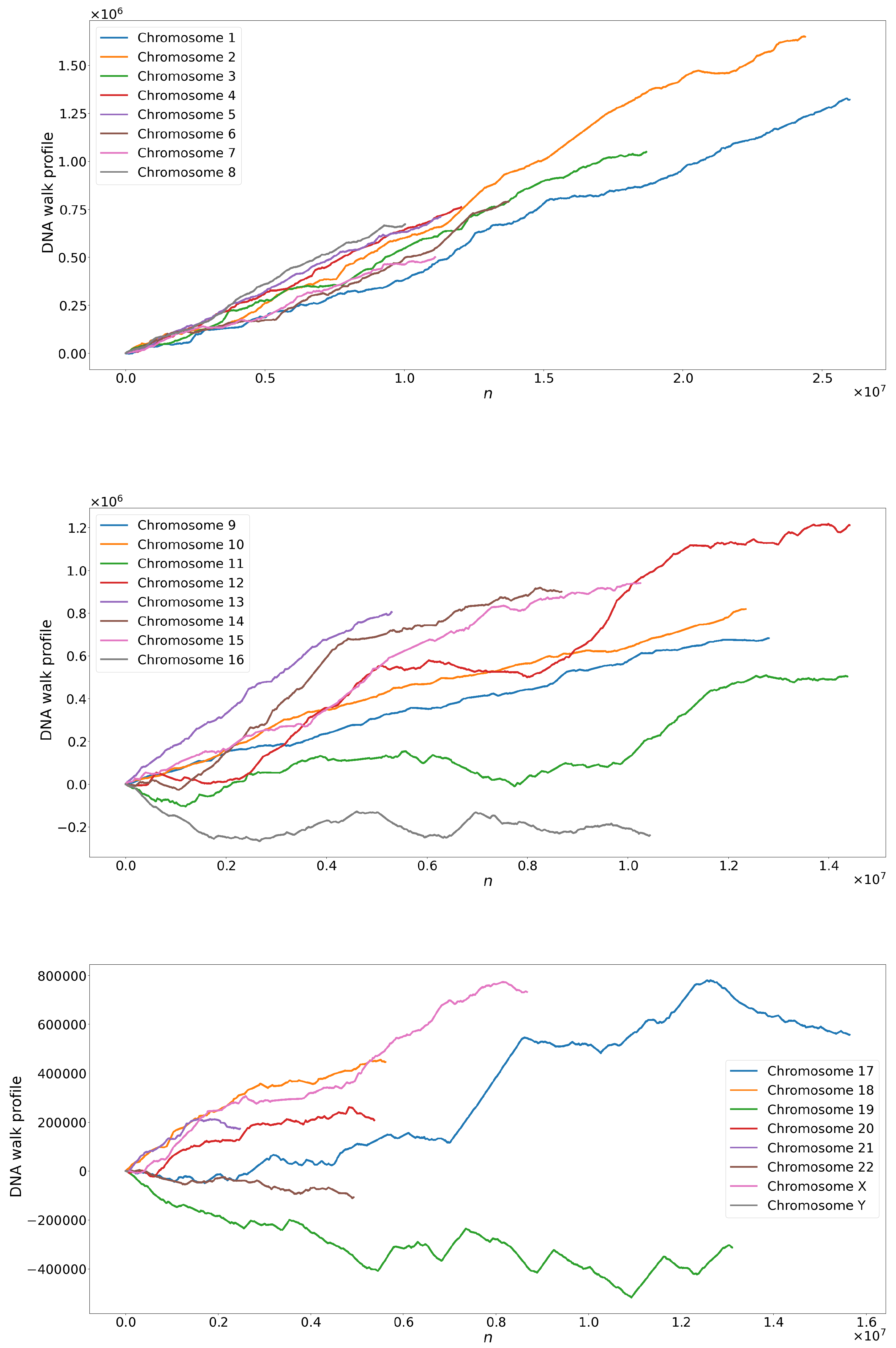
2.2. Times Series and Fractal Theory
2.3. Ordinal Patterns
Complexity–Entropy Plane
2.4. Multifractal Detrended Fluctuation Analysis
- The profile follows the calculationwhere the data’s average is denoted by .
- The profile is divided into nonoverlapping segments of equal length s, summing up segments. Because s might not always divide N, there is a chance that some of the profile will stay unsegmented. The residual segment must not be discarded; thus, the procedure is reiterated starting from the end. Finally, we obtain segments, and each one is subjected to a comprehensive calculation of the local variance using the least squares fit.
- The calculation of the variance for the segments follows from the least squares fitfor each segment v, , andfor each segment . Here, represents the polynomial fit within segment i and is determined according to the trend observed in the time series. Various polynomial orders can be utilized in the fitting process.
- Using an arbitrary polynomial, we calculated , which represents the variance in segment v of size s. The average of all segments is represented by the fluctuation function of q-th order.We return the standard DFA method for . We are interested in the fluctuation function for various values of q on each length scale s. Steps 2 through 4 are repeated, changing s,
- increases for high values of s if the series exhibits a long-range power law correlation, simulating a power lawHere, is the generalized Hurst exponent.
3. Results and Discussion
3.1. Chaos Game Representation
3.2. Time Series Analysis
3.3. Complexity Entropy
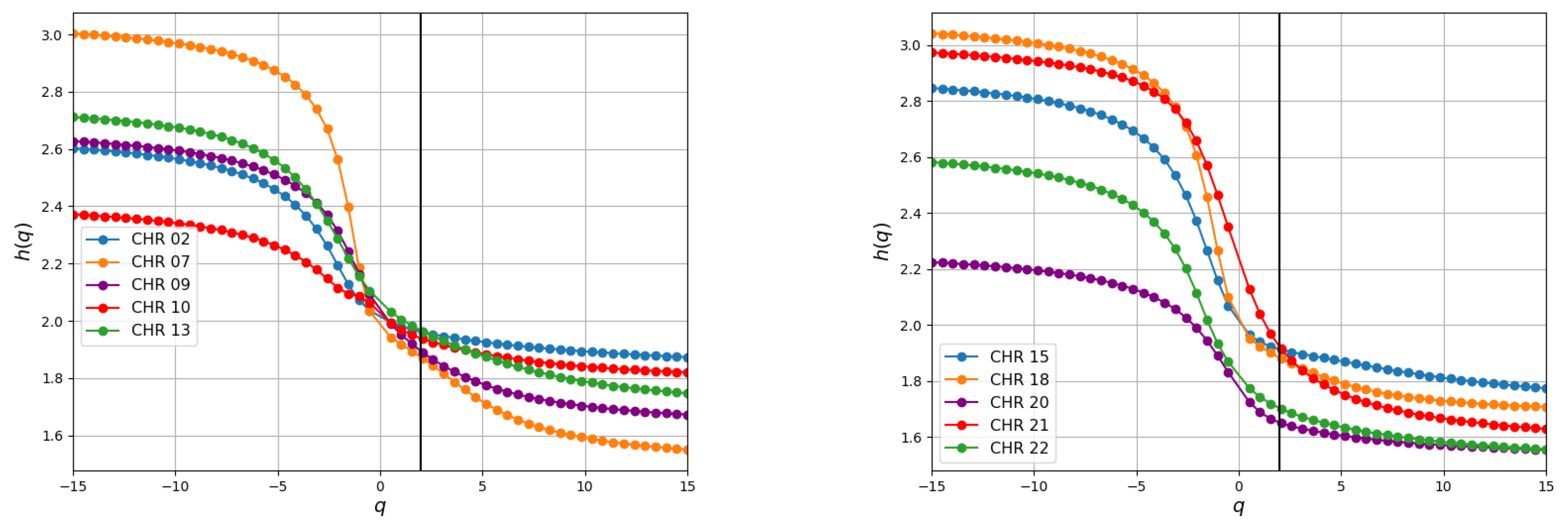
3.4. MF-DFA
4. Conclusions
Supplementary Materials
Funding
Conflicts of Interest
References
- Savolainen, V.; Cowan, R.S.; Vogler, A.P.; Roderick, G.K.; Lane, R. Towards writing the encyclopaedia of life: An introduction to DNA barcoding. Philos. Trans. R. Soc. B Biol. Sci. 2005, 360, 1805–1811. [Google Scholar] [CrossRef] [PubMed]
- Friedberg, E.C. DNA damage and repair. Nature 2003, 421, 436–440. [Google Scholar] [CrossRef]
- Snustad, D.P.; Simmons, M.J. Principles of Genetics; John Wiley & Sons: Hoboken, NJ, USA, 2015. [Google Scholar]
- Nurk, S.; Koren, S.; Rhie, A.; Rautiainen, M.; Bzikadze, A.V.; Mikheenko, A.; Vollger, M.R.; Altemose, N.; Uralsky, L.; Gershman, A.; et al. The complete sequence of a human genome. Science 2022, 376, 44–53. [Google Scholar] [CrossRef] [PubMed]
- Pareek, C.S.; Smoczynski, R.; Tretyn, A. Sequencing technologies and genome sequencing. J. Appl. Genet. 2011, 52, 413–435. [Google Scholar] [CrossRef] [PubMed]
- Voss, R.F. Evolution of long-range fractal correlations and 1/f noise in DNA base sequences. Phys. Rev. Lett. 1992, 68, 3805. [Google Scholar] [CrossRef] [PubMed]
- Buldyrev, S.V.; Goldberger, A.L.; Havlin, S.; Peng, C.K.; Simons, M.; Stanley, H.E. Generalized Lévy-walk model for DNA nucleotide sequences. Phys. Rev. E 1993, 47, 4514. [Google Scholar] [CrossRef] [PubMed]
- Yu, Z.G.; Wang, B. A time series model of CDS sequences in complete genome. Chaos Solitons Fractals 2001, 12, 519–526. [Google Scholar] [CrossRef]
- Arneodo, A.; Bacry, E.; Graves, P.; Muzy, J.F. Characterizing long-range correlations in DNA sequences from wavelet analysis. Phys. Rev. Lett. 1995, 74, 3293. [Google Scholar] [CrossRef]
- Correia, J.; Silva, R.; Anselmo, D.; da Silva, J. Bayesian inference of length distributions of human DNA. Chaos Solitons Fractals 2022, 160, 112244. [Google Scholar] [CrossRef]
- Costa, M.; Silva, R.; Anselmo, D.; Silva, J. Analysis of human DNA through power-law statistics. Phys. Rev. E 2019, 99, 022112. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. On the geometry of homogeneous turbulence, with stress on the fractal dimension of the iso-surfaces of scalars. J. Fluid Mech. 1975, 72, 401–416. [Google Scholar] [CrossRef]
- Ghil, M.; Benzi, R.; Parisi, G. (Eds.) Turbulence and Predictability in Geophysical Fluid Dynamics and Climate Dynamics; North-Holland Publ. Co.: Amsterdam, The Netherlands; New York, NY, USA, 1985; p. 449. [Google Scholar]
- Mandelbrot, B. Multifractals and 1/ƒ Noise: Wild Self-Affinity in Physics (1963–1976); Springer: New York, NY, USA, 1999. [Google Scholar]
- Olsen, L. A Multifractal Formalism. Adv. Math. 1995, 116, 82–196. [Google Scholar] [CrossRef]
- Achour, R.; Li, Z.; Selmi, B.; Wang, T. A multifractal formalism for new general fractal measures. Chaos Solitons Fractals 2024, 181, 114655. [Google Scholar] [CrossRef]
- Hattab, J.; Selmi, B.; Verma, S. Mixed multifractal spectra of homogeneous moran measures. Fractals 2024, 32, 2440003. [Google Scholar] [CrossRef]
- Selmi, B. A Review on Multifractal Analysis of Hewitt–Stromberg Measures. J. Geom. Anal. 2022, 32, 1–44. [Google Scholar] [CrossRef]
- Georgii, H.O. Gibbs Measures and Phase Transitions; De Gruyter: Berlin, Germany; New York, NY, USA, 2011. [Google Scholar] [CrossRef]
- Fathi, B.N. Analyse multifractale de mesures. C. R. Acad. Sci. Paris Sér. I Math. 1994, 319, 807–810. [Google Scholar]
- Ben Nasr, F.; Bhouri, I.; Heurteaux, Y. The Validity of the Multifractal Formalism: Results and Examples. Adv. Math. 2002, 165, 264–284. [Google Scholar] [CrossRef]
- Jeffrey, H.J. Chaos game representation of gene structure. Nucleic Acids Res. 1990, 18, 2163–2170. [Google Scholar] [CrossRef] [PubMed]
- Cigan, B. Chaos Game Representation of a Genetic Sequence. Available online: https://towardsdatascience.com/chaos-game-representation-of-a-genetic-sequence-4681f1a67e14 (accessed on 25 February 2023).
- Basu, S.; Pan, A.; Dutta, C.; Das, J. Chaos game representation of proteins. J. Mol. Graph. Model. 1997, 15, 279–289. [Google Scholar] [CrossRef]
- Rosso, O.A.; Larrondo, H.; Martin, M.T.; Plastino, A.; Fuentes, M.A. Distinguishing noise from chaos. Phys. Rev. Lett. 2007, 99, 154102. [Google Scholar] [CrossRef]
- Machado, J.T.; Costa, A.C.; Quelhas, M.D. Shannon, Rényi and Tsallis entropy analysis of DNA using phase plane. Nonlinear Anal. Real World Appl. 2011, 12, 3135–3144. [Google Scholar] [CrossRef]
- Zunino, L.; Zanin, M.; Tabak, B.M.; Pérez, D.G.; Rosso, O.A. Complexity-entropy causality plane: A useful approach to quantify the stock market inefficiency. Phys. A Stat. Mech. Its Appl. 2010, 389, 1891–1901. [Google Scholar] [CrossRef]
- Ribeiro, H.V.; Zunino, L.; Mendes, R.S.; Lenzi, E.K. Complexity–entropy causality plane: A useful approach for distinguishing songs. Phys. A Stat. Mech. Its Appl. 2012, 391, 2421–2428. [Google Scholar] [CrossRef]
- Ribeiro, H.V.; Zunino, L.; Lenzi, E.K.; Santoro, P.A.; Mendes, R.S. Complexity-entropy causality plane as a complexity measure for two-dimensional patterns. PLoS ONE 2012, 7, e40689. [Google Scholar] [CrossRef]
- Kantelhardt, J.W.; Zschiegner, S.A.; Koscielny-Bunde, E.; Havlin, S.; Bunde, A.; Stanley, H.E. Multifractal detrended fluctuation analysis of nonstationary time series. Phys. A Stat. Mech. Its Appl. 2002, 316, 87–114. [Google Scholar] [CrossRef]
- Mandelbrot, B.B.; Ness, J.W.V. Fractional Brownian Motions, Fractional Noises and Applications. SIAM Rev. 1968, 10, 422–437. [Google Scholar] [CrossRef]
- Mandelbrot, B.B.; Mandelbrot, B.B. The Fractal Geometry of Nature; WH freeman: New York, NY, USA, 1982; Volume 1. [Google Scholar]
- Wang, W.; Liu, K.; Qin, Z. Multifractal analysis on the return series of stock markets using MF-DFA method. In International Conference on Informatics and Semiotics in Organisations; Springer: Berlin/Heidelberg, Germany, 2014; pp. 107–115. [Google Scholar]
- Rego, C.; Frota, H.; Gusmão, M. Multifractality of Brazilian rivers. J. Hydrol. 2013, 495, 208–215. [Google Scholar] [CrossRef]
- Jader da Silva, J.; Stpŏsíc, B.; Stŏíc, T. Multifractality and complexity of the brazilian agribusiness commodities. Braz. J. Biom. 2016, 34, 258–278. [Google Scholar]
- de Benicio, R.B.; Stošić, T.; De Figueirêdo, P.; Stošić, B.D. Multifractal behavior of wild-land and forest fire time series in Brazil. Phys. A Stat. Mech. Its Appl. 2013, 392, 6367–6374. [Google Scholar] [CrossRef]
- Klug, W.S.; Cummings, M.R.; Spencer, C. Concepts of Genetics, 7th ed.; Pearson Education, Inc.: London, UK, 2003. [Google Scholar]
- Gilbert, W. The exon theory of genes. In Cold Spring Harbor Symposia on Quantitative Biology; Cold Spring Harbor Laboratory Press: Cambridge, MA, USA, 1987. [Google Scholar]
- Rogozin, I.B.; Carmel, L.; Csuros, M.; Koonin, E.V. Origin and evolution of spliceosomal introns. Biol. Direct 2012, 7, 1–28. [Google Scholar] [CrossRef]
- Carroll, S.B.; Doebley, J.; Griffiths, A.J.; Wessler, S.R. Introduction to Genetic Analysis; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Alberts, B. Molecular Biology of the Cell; Garland Science: New York, NY, USA, 2017. [Google Scholar]
- Chow, L.T.; Gelinas, R.E.; Broker, T.R.; Roberts, R.J. An amazing sequence arrangement at the 5 ends of adenovirus 2 messenger RNA. Cell 1977, 12, 1–8. [Google Scholar] [CrossRef] [PubMed]
- De Conti, L.; Baralle, M.; Buratti, E. Exon and intron definition in pre–mRNA splicing. Wiley Interdiscip. Rev. RNA 2013, 4, 49–60. [Google Scholar] [CrossRef]
- National Library of Medicine. Available online: https://www.ncbi.nlm.nih.gov/ (accessed on 23 February 2023).
- Almeida, J.S.; Carrico, J.A.; Maretzek, A.; Noble, P.A.; Fletcher, M. Analysis of genomic sequences by Chaos Game Representation. Bioinformatics 2001, 17, 429–437. [Google Scholar] [CrossRef] [PubMed]
- Deschavanne, P.J.; Giron, A.; Vilain, J.; Fagot, G.; Fertil, B. Genomic signature: Characterization and classification of species assessed by chaos game representation of sequences. Mol. Biol. Evol. 1999, 16, 1391–1399. [Google Scholar] [CrossRef] [PubMed]
- Pandit, A.; Dasanna, A.K.; Sinha, S. Multifractal analysis of HIV-1 genomes. Mol. Phylogenet. Evol. 2012, 62, 756–763. [Google Scholar] [CrossRef] [PubMed]
- Löchel, H.F.; Heider, D. Chaos game representation and its applications in bioinformatics. Comput. Struct. Biotechnol. J. 2021, 19, 6263–6271. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Hill, K.; Singh, S.; Kari, L. The spectrum of genomic signatures: From dinucleotides to chaos game representation. Gene 2005, 346, 173–185. [Google Scholar] [CrossRef] [PubMed]
- Hao, B.L.; Lee, H.C.; Zhang, S.Y. Fractals related to long DNA sequences and complete genomes. Chaos Solitons Fractals 2000, 11, 825–836. [Google Scholar] [CrossRef]
- Ouadfeul, S.A. Multifractal Analysis of SARS-CoV-2 Coronavirus genomes using the wavelet transform. bioRxiv 2020. [Google Scholar] [CrossRef]
- Bandt, C.; Pompe, B. Permutation entropy: A natural complexity measure for time series. Phys. Rev. Lett. 2002, 88, 174102. [Google Scholar] [CrossRef]
- Leyva, I.; Martínez, J.H.; Masoller, C.; Rosso, O.A.; Zanin, M. 20 years of ordinal patterns: Perspectives and challenges. Europhys. Lett. 2022, 138, 31001. [Google Scholar] [CrossRef]
- Unakafov, A.M.; Keller, K. Conditional entropy of ordinal patterns. Phys. D Nonlinear Phenom. 2014, 269, 94–102. [Google Scholar] [CrossRef]
- Pessa, A.A.; Ribeiro, H.V. ordpy: A Python package for data analysis with permutation entropy and ordinal network methods. Chaos Interdiscip. J. Nonlinear Sci. 2021, 31, 063110. [Google Scholar] [CrossRef]
- Zanin, M.; Olivares, F. Ordinal patterns-based methodologies for distinguishing chaos from noise in discrete time series. Commun. Phys. 2021, 4, 190. [Google Scholar] [CrossRef]
- Lopez-Ruiz, R.; Mancini, H.L.; Calbet, X. A statistical measure of complexity. Phys. Lett. A 1995, 209, 321–326. [Google Scholar] [CrossRef]
- Kantelhardt, J.W. Fractal and multifractal time series. arXiv 2008, arXiv:0804.0747. [Google Scholar]
- Halsey, T.C.; Jensen, M.H.; Kadanoff, L.P.; Procaccia, I.; Shraiman, B.I. Fractal measures and their singularities: The characterization of strange sets. Phys. Rev. A 1986, 33, 1141. [Google Scholar] [CrossRef] [PubMed]
- Kurths, J.; Herzel, H. An attractor in a solar time series. Phys. D Nonlinear Phenom. 1987, 25, 165–172. [Google Scholar] [CrossRef]
- Goldman, N. Nucleotide, dinucleotide and trinucleotide frequencies explain patterns observed in chaos game representations of DNA sequences. Nucleic Acids Res. 1993, 21, 2487–2491. [Google Scholar] [CrossRef]
- Li, M.; Chen, S.S. The tendency to recreate ancestral CG dinucleotides in the human genome. BMC Evol. Biol. 2011, 11, 1–9. [Google Scholar] [CrossRef]
- Rosas, A.; Nogueira, E., Jr.; Fontanari, J.F. Multifractal analysis of DNA walks and trails. Phys. Rev. E 2002, 66, 061906. [Google Scholar] [CrossRef] [PubMed]





| CHR | H | h | B | |
|---|---|---|---|---|
| 01 | 0.92 | 0.81 | 0.98 | 2.16 |
| 02 | 0.96 | 0.73 | 0.85 | 3.72 |
| 03 | 0.91 | 0.69 | 0.80 | 2.48 |
| 04 | 0.92 | 1.39 | 1.48 | 3.93 |
| 05 | 0.92 | 1.46 | 1.55 | 5.73 |
| 06 | 0.95 | 0.85 | 0.99 | 5.60 |
| 07 | 0.86 | 1.45 | 1.60 | 2.26 |
| 08 | 0.94 | 1.20 | 1.30 | 6.64 |
| 09 | 0.98 | 0.96 | 1.08 | 1.76 |
| 10 | 0.93 | 0.55 | 0.65 | 1.95 |
| 11 | 0.85 | 1.02 | 1.20 | 2.64 |
| 12 | 0.90 | 1.46 | 1.62 | 6.04 |
| 13 | 0.96 | 0.96 | 1.12 | 1.95 |
| 14 | 0.93 | 1.60 | 1.75 | 4.64 |
| 15 | 0.91 | 1.07 | 1.23 | 3.92 |
| 16 | 0.92 | 0.49 | 0.59 | 3.92 |
| 17 | 0.85 | 1.10 | 1.20 | 2.20 |
| 18 | 0.88 | 1.33 | 1.44 | 3.64 |
| 19 | 0.95 | 0.58 | 0.69 | 5.90 |
| 20 | 0.65 | 0.67 | 0.76 | 2.45 |
| 21 | 0.91 | 1.34 | 1.48 | 1.50 |
| 22 | 0.70 | 1.02 | 1.16 | 3.14 |
| X | 0.91 | 0.98 | 1.11 | 5.53 |
| Y | 0.76 | 0.57 | 0.70 | 0.52 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Correia, J.P.; Silva, R.; Anselmo, D.H.A.L.; Vasconcelos, M.S.; da Silva, L.R. Multifractal Properties of Human Chromosome Sequences. Fractal Fract. 2024, 8, 312. https://doi.org/10.3390/fractalfract8060312
Correia JP, Silva R, Anselmo DHAL, Vasconcelos MS, da Silva LR. Multifractal Properties of Human Chromosome Sequences. Fractal and Fractional. 2024; 8(6):312. https://doi.org/10.3390/fractalfract8060312
Chicago/Turabian StyleCorreia, J. P., R. Silva, D. H. A. L. Anselmo, M. S. Vasconcelos, and L. R. da Silva. 2024. "Multifractal Properties of Human Chromosome Sequences" Fractal and Fractional 8, no. 6: 312. https://doi.org/10.3390/fractalfract8060312
APA StyleCorreia, J. P., Silva, R., Anselmo, D. H. A. L., Vasconcelos, M. S., & da Silva, L. R. (2024). Multifractal Properties of Human Chromosome Sequences. Fractal and Fractional, 8(6), 312. https://doi.org/10.3390/fractalfract8060312






