Glass Beads Test with True Triaxial Stress Path Achieved by Conventional Triaxial Apparatus
Abstract
1. Introduction
2. Materials and Methods
2.1. Test Materials
2.2. A Novel Method for Stress Paths
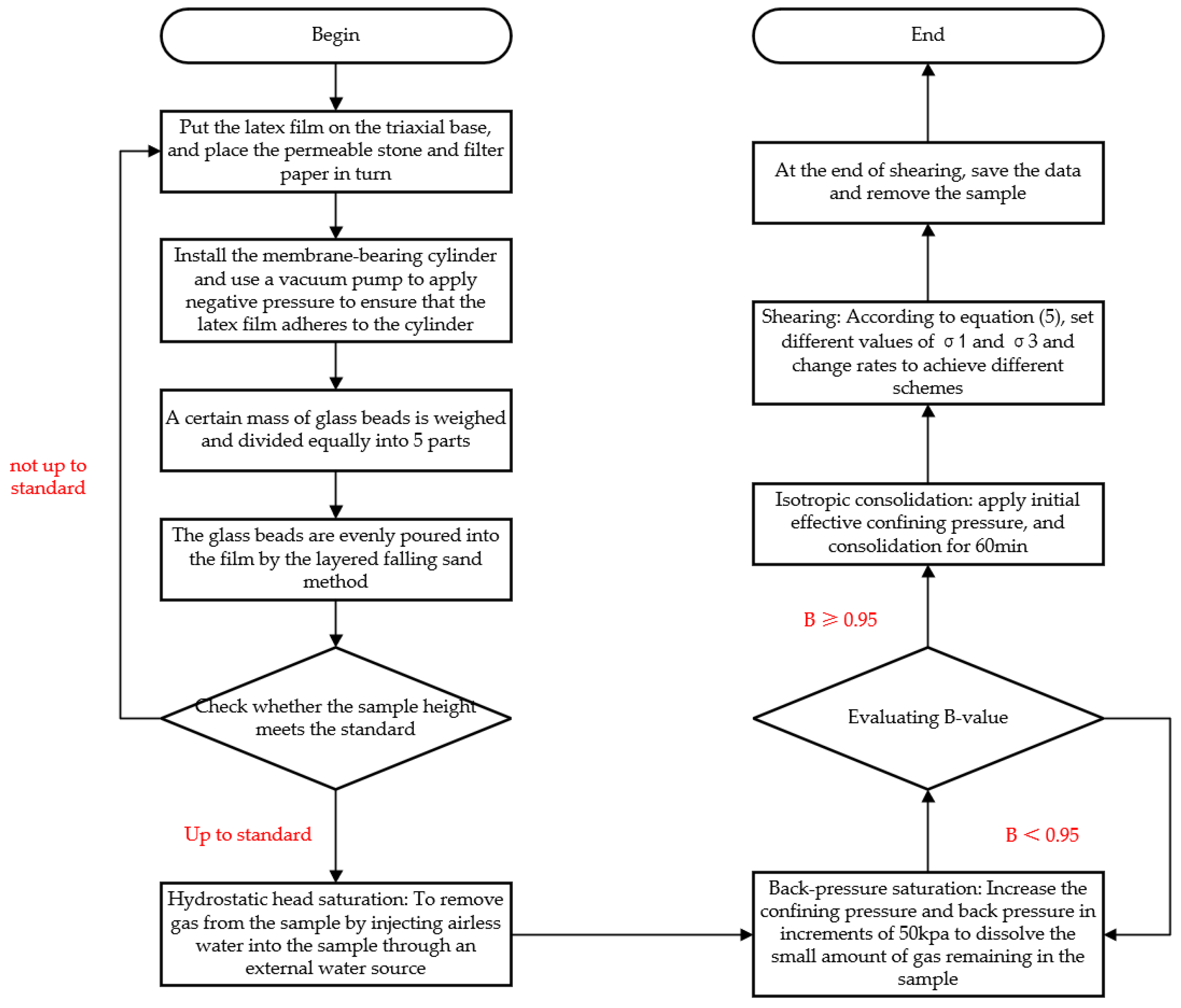
2.3. Test Scheme and Procedure
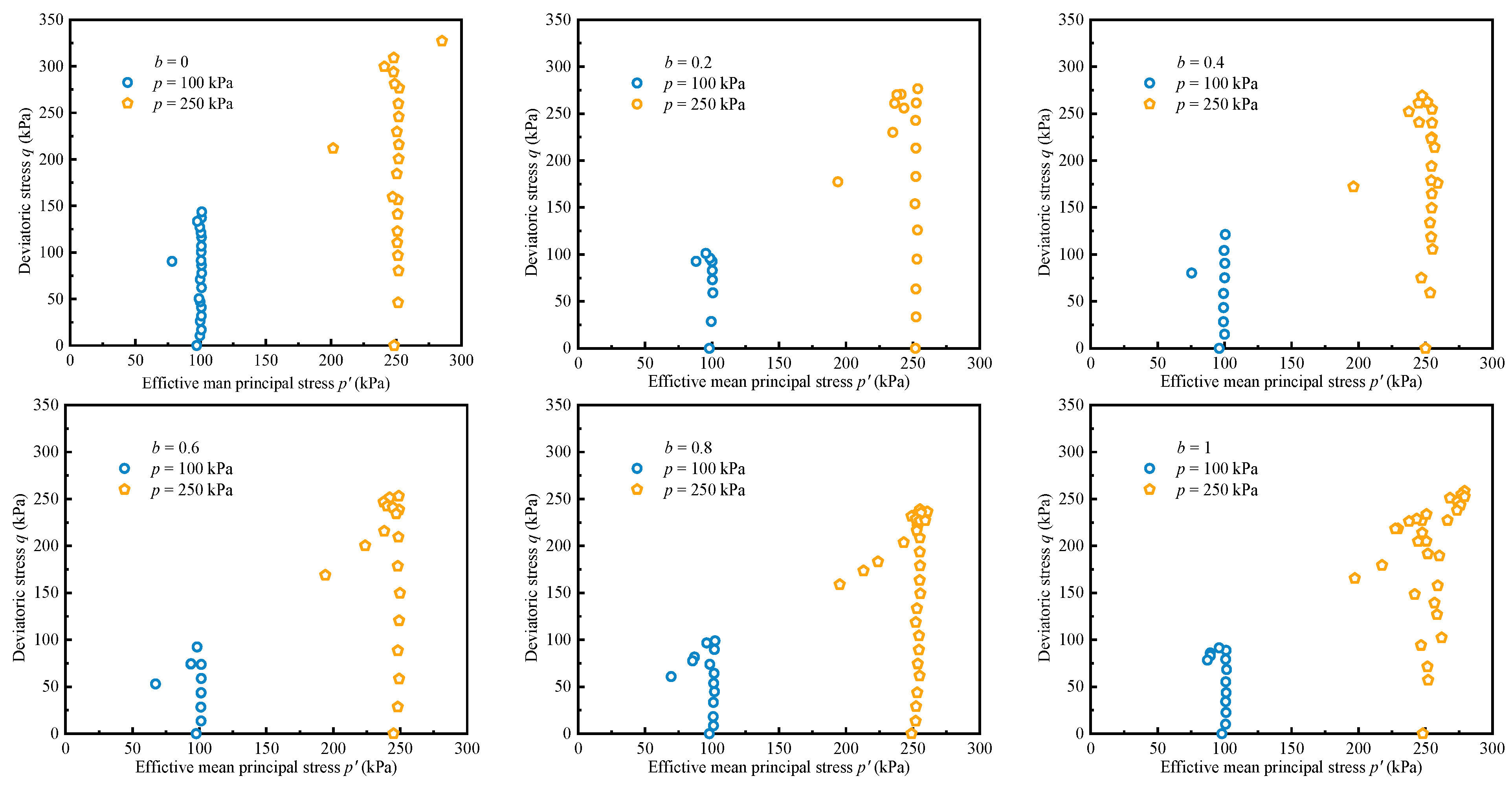
3. Results and Discussion
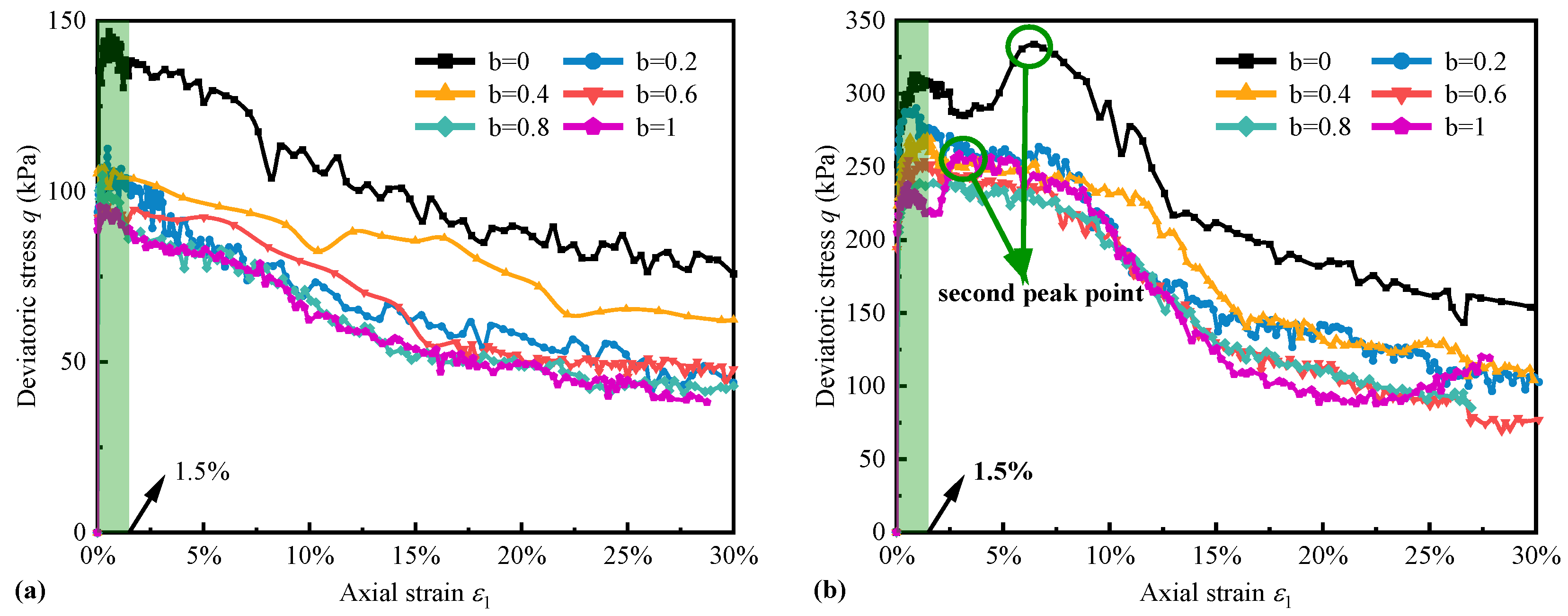
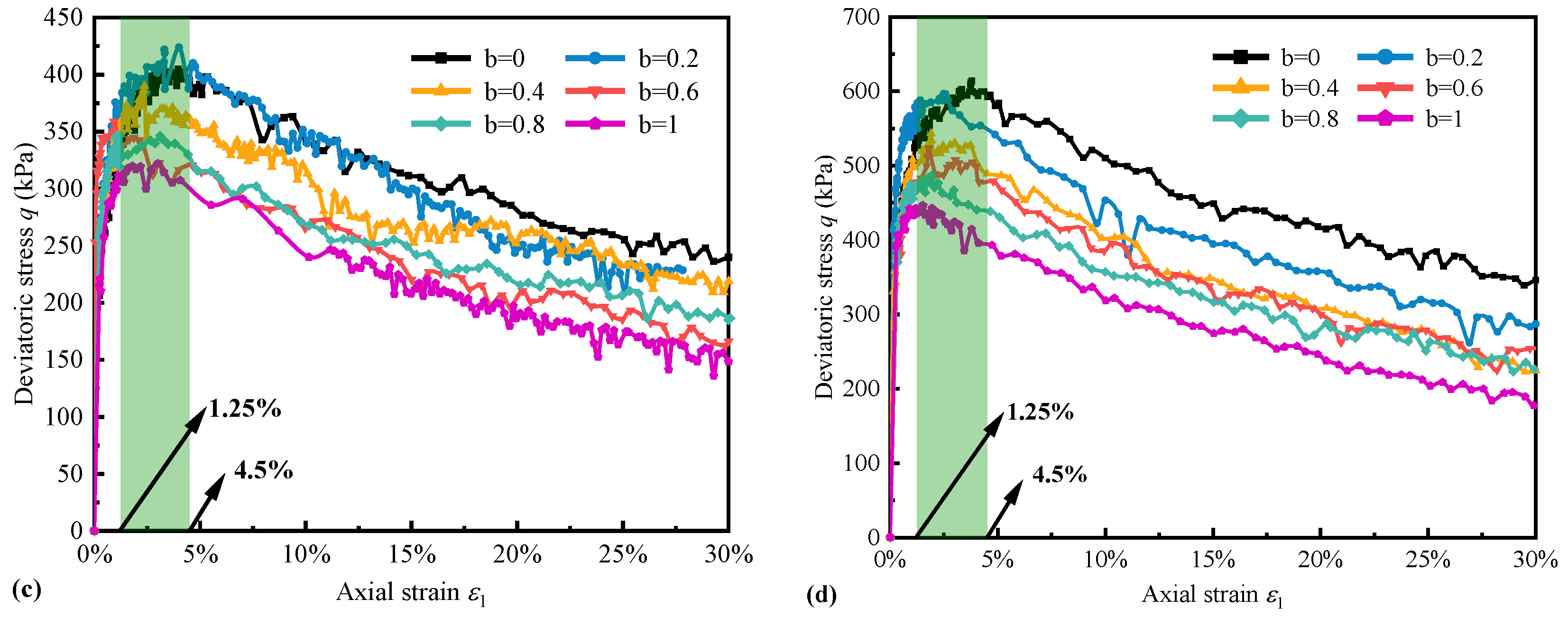
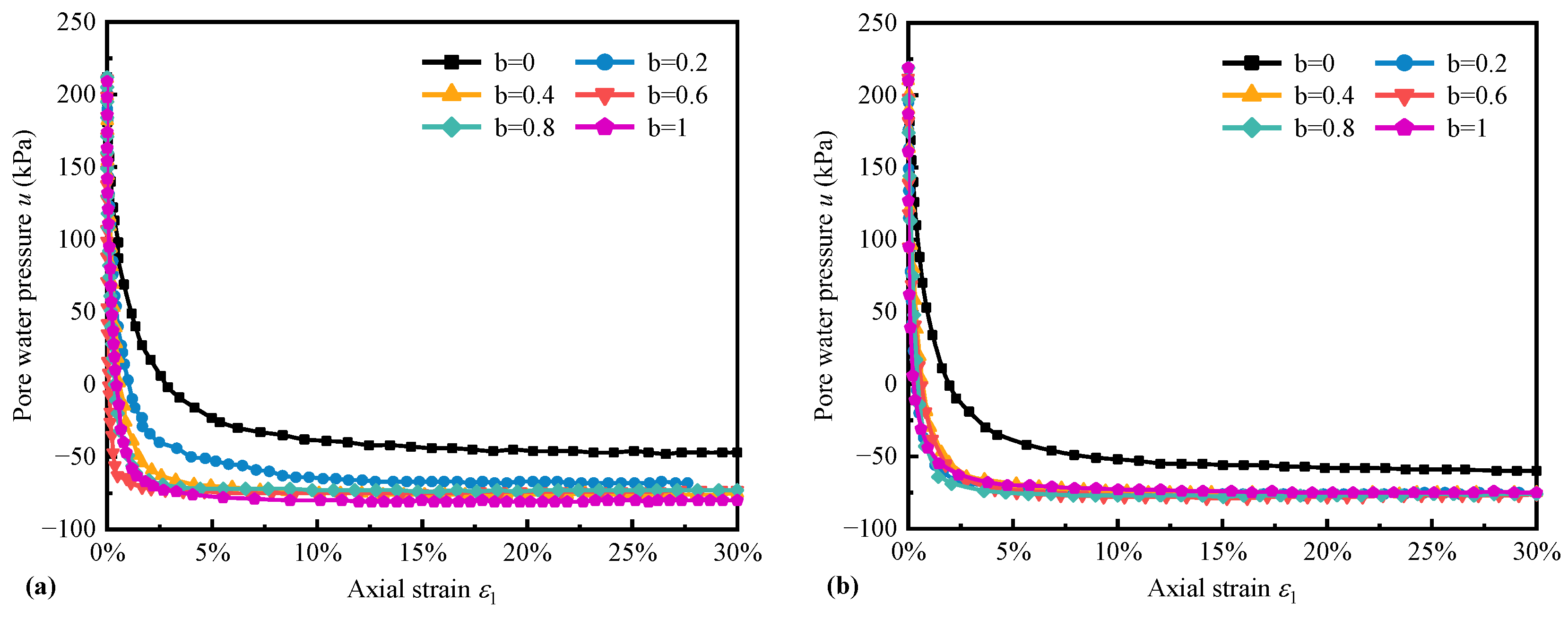
3.1. Strain–Stress Relationship
3.2. Dilatancy Characteristics
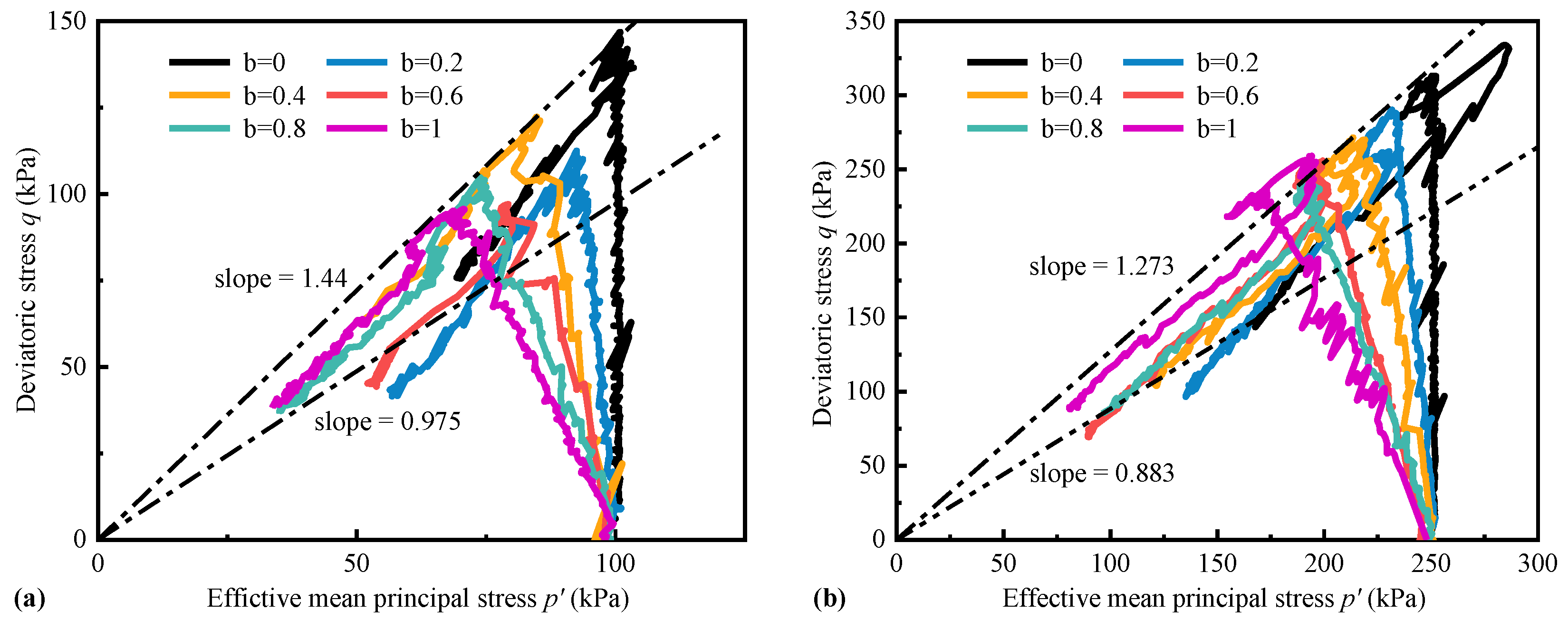
3.3. Strength Characteristics
4. Conclusions
- (1)
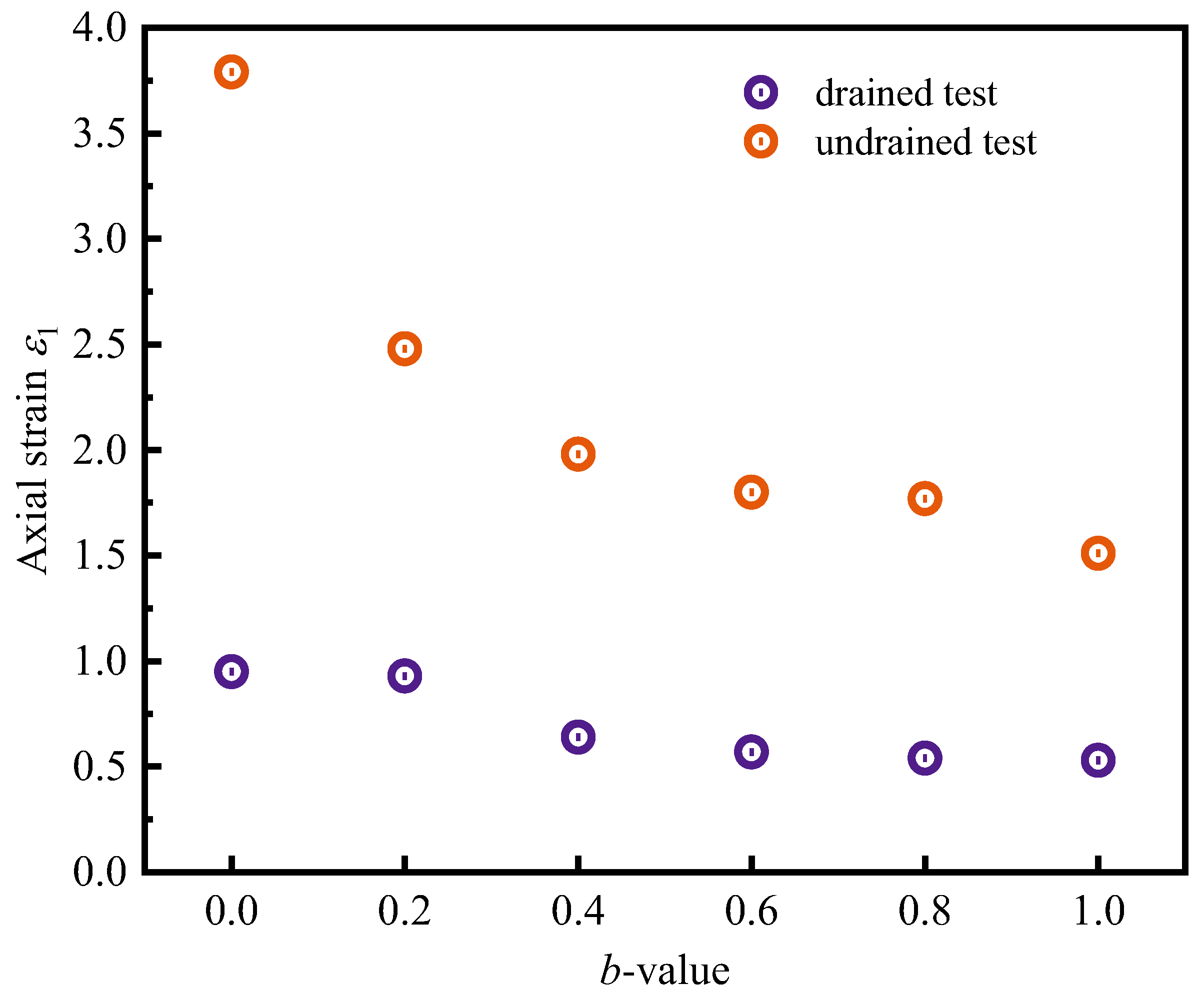
- The specimens exhibited varying degrees of strain softening, dilatancy, and shear band formation phenomena across different b-values under two distinct confining pressures and drained conditions. The initial modulus increased with rising p. Except for b = 0, the initial modulus decreased with increasing b-value, thus verifying the effectiveness of this novel method in illustrating stress paths.
- (2)
- The peak strength of the undrained test was greater than that of the drained test, while the peak strength decreased with increasing b-value. The stress–strain curves of the drained tests showed two notable peaks, particularly at b = 0 and b = 1, and the second peak was significantly higher than the first. Furthermore, the peak friction angle and peak stress ratio of the drained test at p = 250 kPa were higher than those of the undrained test. Under drained conditions, the specimens exhibited the highest compressive performance at b = 1. The disparities between drained and undrained tests indicated an intensified water sensitivity of glass beads.
- (3)
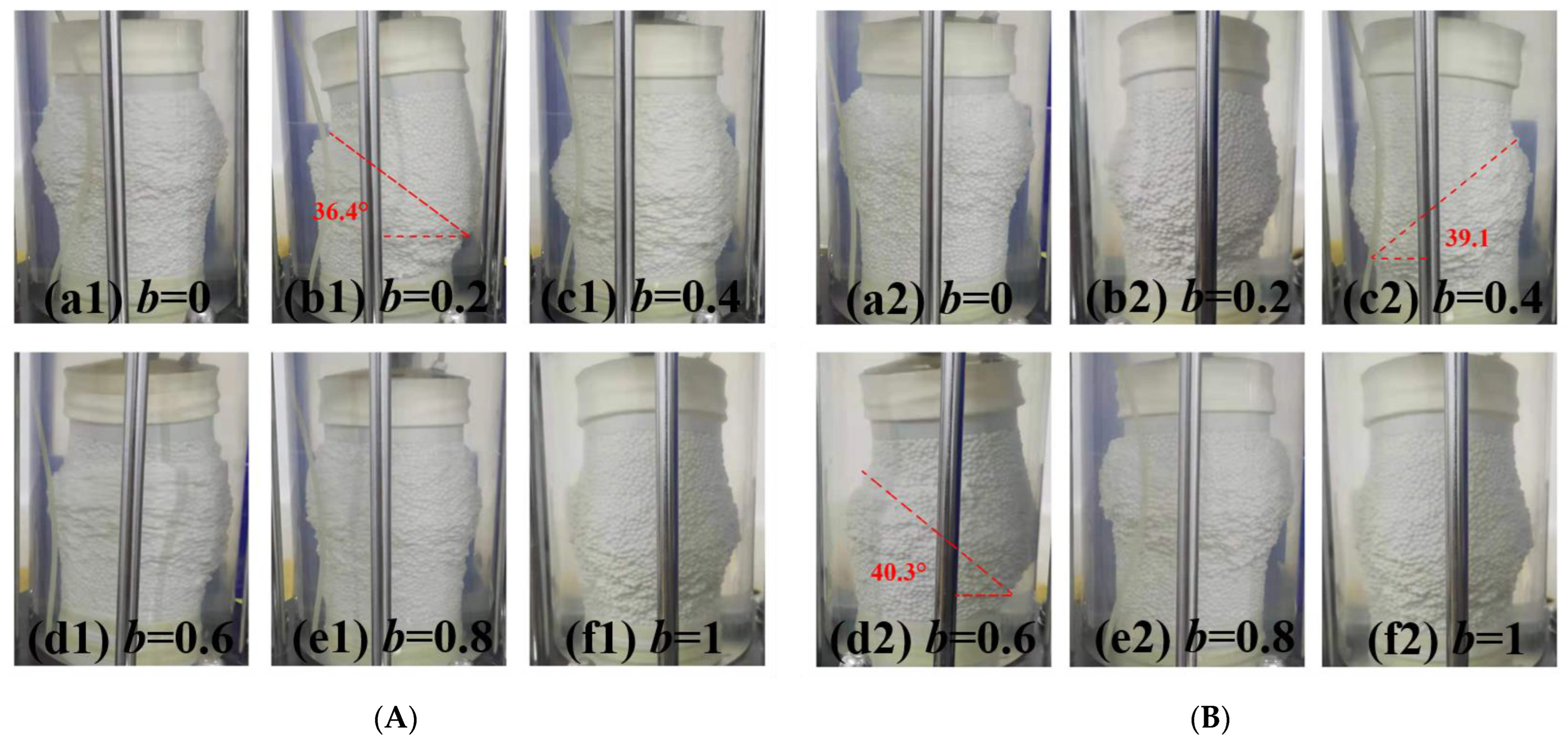
- At an axial strain of 30%, the majority of specimens displayed “bulging” deformations, with the position of this deformation correlated to the b-value. Shear bands were observed at specific b-values, all inclined at angles less than 45°. The application of the novel method generated remarkable shear bands in axisymmetric specimens at b-values ranging from 0.2 to 0.6, which exhibited a substantial deviation from conventional triaxial tests.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yin, F.J.; Cai, G.Q.; Su, Y.L.; Shan, Y.P.; Li, J. Rate-dependent experimental study on unsaturated coarse-grained soil controlled by matrix suction. Chin. J. Geotech. Eng. 2023, 45, 24–28. [Google Scholar]
- Fu, Z.; Chen, S.; Han, H. Experimental investigations on the residual strain behavior of a rockfill material subjected to dynamic loading. J. Mater. Civ. Eng. 2017, 29, 04016278. [Google Scholar] [CrossRef]
- Chen, B.; Li, Y.Q.; Liu, X.F. Study on Stick-slip Behaviour of Granular Materials in Triaxial Test. J. Disaster Prev. Mitig. Eng. 2023, 43, 568–575. [Google Scholar]
- Liu, J.; Zhou, W.; Ji, X.; Wei, G.; Yuan, S.; Li, X. Dilatancy Analysis of Granular Materials Based on Mesoscopic Topological Evolutions. Chin. J. Mech. 2022, 54, 707–718. [Google Scholar]
- Maosong, H.; Xuefeng, L.; Cangqin, J. A Double Yield Surface Constitutive Model for Sand Based on State-dependent Critical State Theory. Chin. J. Geotech. Eng. 2010, 32, 1764–1771. [Google Scholar]
- Beibing, D.; Juny, Y.; Cuiying, Z. An Experimental Study on the Effect of Inter-particle Friction on Shear Behavior of Granular Materials. Chin. J. Mech. 2013, 45, 375–383. [Google Scholar]
- Marschi, N.D.; Chan, C.K.; Seed, H.B. Evaluation of properties of rockfill materials. ASCE J. Soil Mech. Found. Div. 1972, 98, 95–114. [Google Scholar] [CrossRef]
- Ning, F.; Liu, J.; Kong, X. Critical state and grading evolution of rockfill material under different triaxial compression tests. Int. J. Geomech. 2020, 20, 04019154. [Google Scholar] [CrossRef]
- Marsal, R.J. Large scale testing of rockfill materials. ASCE J. Soil Mech. Found. Div. 1967, 93, 27–43. [Google Scholar] [CrossRef]
- Lee, K.L.; Farhoomand, I. Compressibility and crushing of granular soil in anisotropic triaxial compression. Can. Geotech. J. 1967, 4, 68–86. [Google Scholar] [CrossRef]
- Varadarajan, A.; Sharma, K.G.; Venkatachalam, K. Testing and modeling two rockfill materials. J. Geotech. Geoenviron. Eng. 2003, 129, 206–218. [Google Scholar] [CrossRef]
- Xiao, Y.; Liu, H. Elastoplastic constitutive model for rockfill materials considering particle breakage. Int. J. Geomech. 2017, 17, 04016041. [Google Scholar] [CrossRef]
- Xiao, Y.; Liu, H.; Desai, C.S. Effect of intermediate principal-stress ratio on particle breakage of rockfill material. J. Geotech. Geoenviron. Eng. 2016, 142, 06015017. [Google Scholar] [CrossRef]
- Xiao, Y.; Meng, M.; Daouadji, A.; Chen, Q.; Wu, Z.; Jiang, X. Effects of particle size on crushing and deformation behaviors of rockfill materials. Geosci. Front. 2020, 11, 375–388. [Google Scholar] [CrossRef]
- Jia, Y.; Xu, B.; Chi, S.; Xiang, B.; Xiao, D.; Zhou, Y. Particle breakage of rockfill material during triaxial tests under complex stress paths. Int. J. Geomech. 2019, 19, 04019124. [Google Scholar] [CrossRef]
- Guo, X.L.; Hu, H.; Bao, C.G. Experimental Studies of the Effects of Grain Breakage on the Dilatancy and Shear Strength of Rock Fill. Chin. J. Geotech. Eng. 1997, 19, 86–91. [Google Scholar]
- Liu, H.; Qin, H.; Gao, Y.; Zhou, Y. Experimental Study on Particle Breakage of Rockfill and Coarse Aggregates. Rock Soil Mech. 2005, 26, 562–566. [Google Scholar]
- Chi, S.; Jia, Y. Rown’s Stress-dilatancy Model Modified for Energy Dissipation of Particle Breakage. Chin. J. Geotech. Eng. 2005, 27, 31–34. [Google Scholar]
- Liu, E.L.; Tan, Y.L.; Chen, S.S.; Li, G.Y. Investigation on Critical State of Rockfill Materials. J. Hydraul. Eng. 2012, 43, 505–511+519. [Google Scholar]
- Oda, M.; Konishi, J.; Nemat-Nasser, S. Experimental micromechanical evaluation of the strength of granular materials: Effects of particle rolling. Mech. Mater. 1982, 1, 269–283. [Google Scholar] [CrossRef]
- Bésuelle, P.; Desrues, J.; Raynaud, S. Experimental characterization of the localization phenomenon inside a Vosges sandstone in a triaxial cell. Int. J. Rock Mech. Min. Sci. 2000, 37, 1223–1237. [Google Scholar] [CrossRef]
- Cheng, Z.; Ding, H.; Wu, L. Experimental Study on Mechanical Behavior of Granular Material. Chin. J. Geotech. Eng. 2007, 29, 1151–1158. [Google Scholar]
- Kong, D.; Zhang, B.; Sun, X. Triaxial Tests on Particle Breakage Strain of Artificial Rockfill Materials. Chin. J. Geotech. Eng. 2009, 31, 464–469. [Google Scholar]
- Takei, M.; Kusakabe, O.; Hayashi, T. Time-dependent behavior of crushable materials in one-dimensional compression tests. Soils Found. 2001, 41, 97–121. [Google Scholar] [CrossRef]
- Zhao, N.; Zuo, Y.; Wang, Z.; Yu, S. Grading scale method for coarse-grained soils based on fractal theory. Rock Soil Mech. 2016, 37, 3513–3519. [Google Scholar]
- Chen, Z.; Li, G.; Wei, K.; Wu, L.; Zhu, Y. Ultimate state and probability of particle breakage for rockfill materials based on fractal theory. Chin. J. Geotech. Eng. 2021, 43, 1192–1200. [Google Scholar]
- Dai, B.B.; Yang, J.; Zhou, C.Y. Observed effects of interparticle friction and particle size on shear behavior of granular materials. Int. J. Geomech. 2016, 16, 04015011. [Google Scholar] [CrossRef]
- Liu, L. Micromechanics of Granular Assemblies of Elastic-perfectly Plastic Sphered During Qusai-Static Deformation. Chin. J. Geotech. Eng. 2007, 29, 524–530. [Google Scholar]
- Ibsen, L.B.; Prasstrup, U. The Danish rigid boundary true triaxial apparatus for soil testing. Geotech. Test. J. 2002, 25, 254–265. [Google Scholar] [CrossRef]
- Rodriguez, N.M.; Lade, P.V. True triaxial tests on cross-anisotropic deposits of fine Nevada sand. Int. J. Geomech. 2013, 13, 779–793. [Google Scholar] [CrossRef]
- Abelev, A.V.; Lade, P.V. Effects of cross anisotropy on three-dimensional behavior of sand. I: Stress-strain behavior and shear banding. J. Eng. Mech. 2003, 129, 160–166. [Google Scholar] [CrossRef]
- Haruyama, M. Anisotropic deformation-strength characteristics of an assembly of spherical particles under three-dimensional stresses. Soils Found. 1981, 21, 41–55. [Google Scholar] [CrossRef] [PubMed]
- Li, K.; Li, X.; Chen, Q. Laboratory Analyses of Noncoaxiality and Anisotropy of Spherical Granular Media under True Triaxial State. Int. J. Geomech. 2023, 23, 04023150. [Google Scholar] [CrossRef]
- Mahmud Sazzad, M.D.; Suzuki, K.; Modaressi-Farahmand-Razavi, A. Macro-micro responses of granular materials under different b values using DEM. Int. J. Geomech. 2012, 12, 220–228. [Google Scholar] [CrossRef]
- Zhou, W.; Xie, T.T.; Ma, G.; Chang, X.L. Stress and Deformation Analysis of Rockfill in True Triaxial Stress Conditions Based on PFC. Rock Soil Mech. 2012, 33, 3006–3012+3080. [Google Scholar]
- Li, X.F.; Ma, Z.G. Method for Achieving Stress or Strain Path in Three-Dimensional Space with Pseudo-Triaxial Apparatus. CN114235571B, 9 May 2023. [Google Scholar]
- Ma, Z.; Li, X. Aeolian Sand Test with True Triaxial Stress Path Achieved by Pseudo-Triaxial Apparatus. Sustainability 2023, 15, 8328. [Google Scholar] [CrossRef]
- Min, Z.; Chengshun, X. True Triaxial Experimental Research on Shear Behaviors of Sand under Different Intermediate Principal Stresses and Different Stress Paths. J. Hydraul. Eng. 2015, 46, 1072–1079. [Google Scholar]
- Xu, W.D.; Li, X.F.; Yang, W.W.; Jia, H.J. Triaxial test on glass beads simulating coarse-grained soil. Res. Cold Arid Reg. 2022, 14, 274–280. [Google Scholar] [CrossRef]
- Hettler, A.; Vardoulakis, I. Behaviour of dry sand tested in a large triaxial apparatus. Geotechnique 1984, 34, 183–197. [Google Scholar] [CrossRef]
- Peric, D.; Runesson, K.; Sture, S. Evaluation of plastic bifurcation for plane strain versus axisymmetry. J. Eng. Mech. 1992, 118, 512–524. [Google Scholar] [CrossRef]
- Zhou, Y.; Pan, J.; Cheng, Z.L. Strength and Dilation of Sandy Gravel Material Based on Large-scale Ture Triaxial Tests. Chin. J. Rock Mech. Eng. 2017, 36, 2818–2825. [Google Scholar]
- Zuo, Y.; Jiang, J.; Pan, J.; Zhao, N.; Zhou, Y. Deformation Characteristics of Coarse Granular Materials in Large-scale True Triaxial Tests. Chin. J. Geotech. Eng. 2019, 41, 37–40. [Google Scholar]
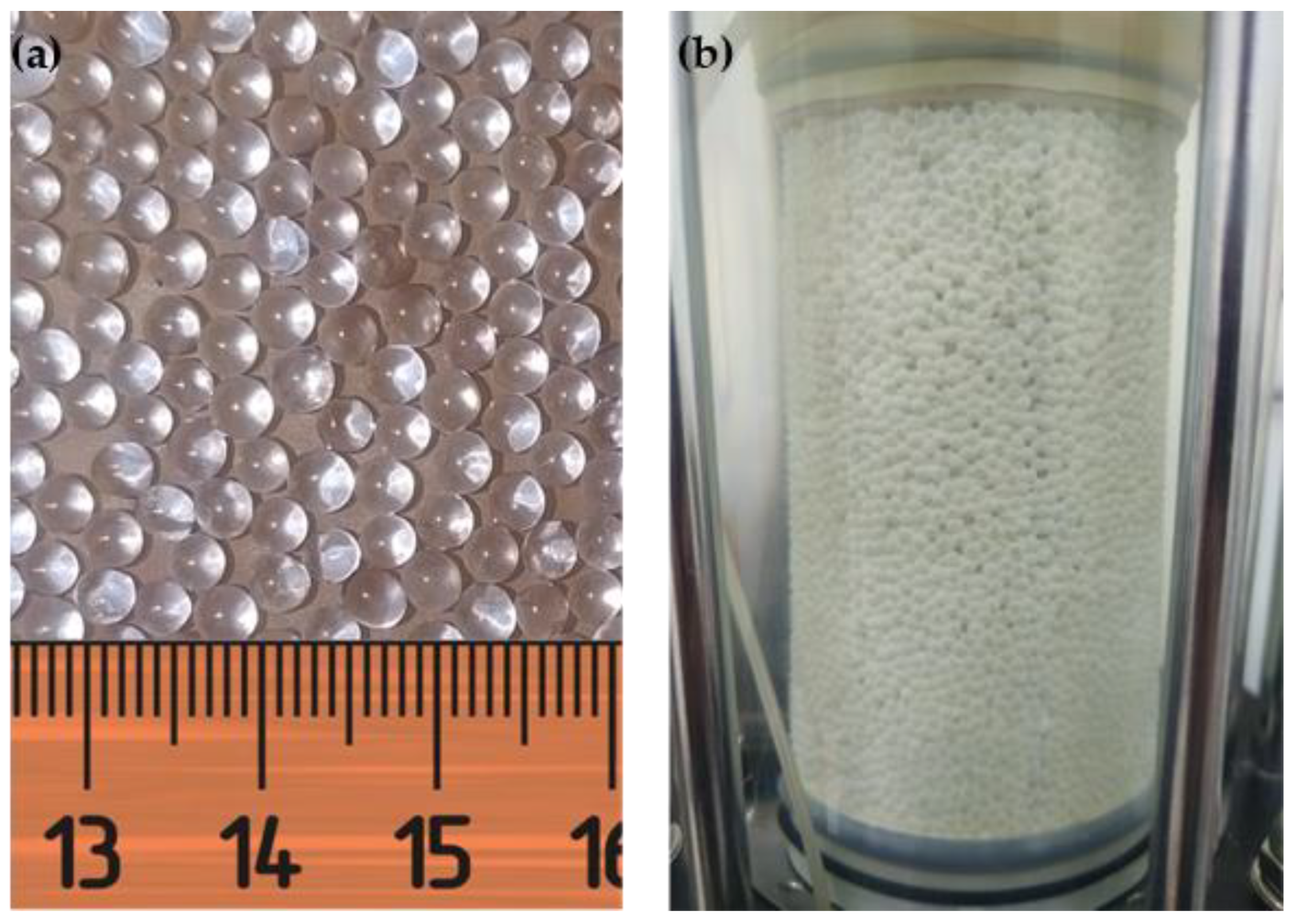




| Specific Gravity (kg/dm) | Young’s Modulus (GPa) | Mohs Hardness | Void Ratio (In Theory) | |
|---|---|---|---|---|
| Max | Min | |||
| 2.5 | 63 | ≥6 | 0.91 | 0.34 |
| Drained Condition | b-Values | p (kPa) |
|---|---|---|
| drained undrained | 0 | 100 250 |
| 0.2 | ||
| 0.4 | ||
| 0.6 | ||
| 0.8 | ||
| 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Ma, J.; Lv, L. Glass Beads Test with True Triaxial Stress Path Achieved by Conventional Triaxial Apparatus. Fractal Fract. 2024, 8, 193. https://doi.org/10.3390/fractalfract8040193
Li X, Ma J, Lv L. Glass Beads Test with True Triaxial Stress Path Achieved by Conventional Triaxial Apparatus. Fractal and Fractional. 2024; 8(4):193. https://doi.org/10.3390/fractalfract8040193
Chicago/Turabian StyleLi, Xuefeng, Jiahui Ma, and Longlong Lv. 2024. "Glass Beads Test with True Triaxial Stress Path Achieved by Conventional Triaxial Apparatus" Fractal and Fractional 8, no. 4: 193. https://doi.org/10.3390/fractalfract8040193
APA StyleLi, X., Ma, J., & Lv, L. (2024). Glass Beads Test with True Triaxial Stress Path Achieved by Conventional Triaxial Apparatus. Fractal and Fractional, 8(4), 193. https://doi.org/10.3390/fractalfract8040193







