Novel Controller Design for Finite-Time Synchronization of Fractional-Order Nonidentical Complex Dynamical Networks under Uncertain Parameters
Abstract
1. Introduction
- (1)
- A new criterion for FOCNUP synchronization is proposed using fractional-order theory and inequality theory.
- (2)
- A new controller is designed to achieve the FTS of FOCNUPs.
- (3)
- Two general controllers are proposed and compared with the new controller proposed, and corresponding numerical simulations are performed to highlight the advantages of our proposed new controller.
2. Preliminaries
3. Main Results
- (i)
- (ii)
- (iii)
- in which the estimate setting time (EST) can be estimated as:
- (i)
- (ii)
- (i)
- (ii)
- (iii)
4. Numerical Simulation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Shahal, S.; Wurzberg, A.; Sibony, I.; Duadi, H.; Shniderman, E.; Weymouth, D.; Davidson, N.; Fridman, M. Synchronization of complex human networks. Nat. Commun. 2020, 11, 3854. [Google Scholar] [CrossRef]
- Luarn, P.; Yang, J.C.; Chiu, Y.P. The network effect on information dissemination on social network sites. Comput. Hum. Behav. 2014, 37, 1–8. [Google Scholar] [CrossRef]
- Zhou, Q.; Wei, D.Q. Collective dynamics of neuronal network under synapse and field coupling. Nonlinear Dyn. 2021, 105, 753–765. [Google Scholar] [CrossRef]
- Liu, F.Y.; Yang, Y.Q.; Chang, Q. Synchronization of fractional-order delayed neural networks with reaction-diffusion terms: Distributed delayed impulsive control. Commun. Nonlinear Sci. Numer. Simul. 2023, 124, 107303. [Google Scholar] [CrossRef]
- Yu, J.; Hu, C.; Jiang, H.J.; Fan, X.L. Projective synchronization for fractional neural networks. Neural Netw. 2014, 49, 87–95. [Google Scholar] [CrossRef] [PubMed]
- Majidabad, S.S.; Shandiz, H.T.; Hajizadeh, A. Nonlinear fractional-order power system stabilizer for multi-machine power systems based on sliding mode technique. Int. J. Robust Nonlinear Control 2015, 25, 1548–1568. [Google Scholar] [CrossRef]
- Chen, W.; Zhou, B. Controllability of flow-conservation transportation networks with fractional-order dynamics. Complexity 2021, 2021, 8524984. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Tao, B.B.; Xiao, M.; Sun, Q.S.; Cao, J.D. Hopf bifurcation analysis of a delayed fractional-order genetic regulatory network model. Neurocomputing 2018, 275, 677–686. [Google Scholar] [CrossRef]
- Sun, H.; Duan, J.; Xu, T. Analytical solutions of fractional order chemical engineering equations by a new fractional sub-equation method. Chem. Eng. Sci. 2020, 214, 115399. [Google Scholar]
- Das, M.; Samanta, G.P. A delayed fractional order food chain model with fear effect and prey refuge. Math. Comput. Simul. 2020, 178, 218–245. [Google Scholar] [CrossRef]
- Gibson, J.; Beierlein, M.; Connors, B. Two networks of electrically coupled inhibitory neurons in neocortex. Nature 1999, 402, 75–79. [Google Scholar] [CrossRef]
- Creo, S.; Lancia, M.R.; Vernole, P. Convergence of fractional diffusion processes in extension domains. J. Evol. Eq. 2020, 20, 109–139. [Google Scholar] [CrossRef]
- Jiang, Y.W.; Zhang, B.; Shu, X.J.; Wei, Z.H. Fractional-order autonomous circuits with order larger than one. J. Adv. Res. 2020, 25, 217–225. [Google Scholar] [CrossRef]
- Fan, Y.; Huang, X.; Wang, Z.; Li, Y. Nonlinear dynamics and chaos in a simplified memristor-based fractional-order neural network with discontinuous memductance function. Nonlinear Dyn. 2018, 93, 611–627. [Google Scholar] [CrossRef]
- Shukla, M.K.; Sharma, B.B. Secure communication and image encryption scheme based on synchronisation of fractional order chaotic systems using backstepping. Int. J. Simul. Process. Model. 2018, 13, 473–485. [Google Scholar] [CrossRef]
- Li, H.; Zhang, L.; Hu, C.; Jiang, Y.; Teng, Z. Dynamical analysis of a fractional-order predator-prey model incorporating a prey refuge. J. Appl. Math. Comput. 2017, 54, 435–449. [Google Scholar] [CrossRef]
- Povstenko, Y.Z. Fractional radial heat conduction in an infinite medium with a cylindrical cavity and associated thermal stresses. Mech. Res. Commun. 2010, 37, 436–440. [Google Scholar] [CrossRef]
- Huang, J.; Shen, T. Well-posedness and dynamics of the stochastic fractional magneto-hydrodynamic equations. Nonlinear Anal. Theory Methods Appl. 2016, 133, 102–133. [Google Scholar] [CrossRef]
- Ding, Z.; Chen, C.; Wen, S.; Li, S.; Wang, L. Lag projective synchronization of nonidentical fractional delayed memristive neural networks. Neurocomputing 2022, 469, 138–150. [Google Scholar] [CrossRef]
- Yang, L.X.; Jiang, J. Adaptive synchronization of drive-response fractional-order complex dynamical networks with uncertain parameters. Commun. Nonlinear Sci. Numer. Simul. 2014, 19, 1496–1506. [Google Scholar] [CrossRef]
- Roohi, M.; Zhang, C.; Chen, Y. Adaptive model-free synchronization of different fractional-order neural networks with an application in cryptography. Nonlinear Dyn. 2020, 100, 3979–4001. [Google Scholar] [CrossRef]
- Prakash, M.; Balasubramaniam, P.; Lakshmanan, S. Synchronization of Markovian jumping inertial neural networks and its applications in image encryption. Neural Netw. 2016, 83, 86–93. [Google Scholar] [CrossRef]
- Syed, A.M.; Hymavathi, M.; Senan, S.; Shekher, V.; Arik, S. Global asymptotic synchronization of impulsive fractional-order complex-valued memristor-based neural networks with time varying delays. Commun. Nonlinear Sci. Numer. Simul. 2019, 78, 104869. [Google Scholar] [CrossRef]
- Wang, F.; Yang, Y.Q. Quasi-synchronization for fractional-order delayed dynamical networks with heterogeneous nodes. Appl. Math. Comput. 2018, 339, 1–14. [Google Scholar] [CrossRef]
- Kao, Y.G.; Li, Y.; Park, J.H.; Chen, X.Y. Mittag-Leffler synchronization of delayed fractional memristor neural networks via adaptive control. IEEE Trans. Neural Netw. Learn. Syst. 2021, 32, 2279–2284. [Google Scholar] [CrossRef]
- Li, L.; Chen, W.S.; Wu, X.J. Global exponential stability and synchronization for novel complex-valued neural networks with proportional delays and inhibitory factors. IEEE Trans. Cybern. 2021, 51, 2142–2152. [Google Scholar] [CrossRef]
- Jia, Y.; Wu, H. Global synchronization in finite time for fractional-order coupling complex dynamical networks with discontinuous dynamic nodes. Neurocomputing 2019, 358, 20–32. [Google Scholar] [CrossRef]
- Yang, S.; Hu, C.; Yu, J.; Jiang, H. Finite-time cluster synchronization in complex-variable networks with fractional-order and nonlinear coupling. Neural Netw. 2021, 135, 212–224. [Google Scholar] [CrossRef]
- Li, Y.; Kao, Y.G.; Wang, C.H.; Xia, H.W. Finite-time synchronization of delayed fractional-order heterogeneous complex networks. Neurocomputing 2020, 384, 368–375. [Google Scholar] [CrossRef]
- Xiao, J.; Wu, L.; Wu, A.L.; Zeng, Z.G.; Zhang, Z. Novel controller design for finite-time synchronization of fractional-order memristive neural networks. Neurocomputing 2022, 512, 494–502. [Google Scholar] [CrossRef]
- Li, H.L.; Hu, C.; Zhang, L.; Jiang, H.J.; Cao, J.D. Complete and finite-time synchronization of fractional-order fuzzy neural networks via nonlinear feedback control. Fuzzy Sets Syst. 2022, 443, 50–69. [Google Scholar] [CrossRef]
- Li, H.L.; Hu, C.; Zhang, L.; Jiang, H.J.; Cao, J.D. Non-separation method-based robust finite-time synchronization of uncertain fractional-order quaternion-valued neural networks. Appl. Math. Comput. 2021, 409, 126377. [Google Scholar] [CrossRef]
- Xu, Y.; Li, Y.; Li, W. Adaptive finite-time synchronization control for fractional-order complex-valued dynamical networks with multiple weights. Commun. Nonlinear Sci. Numer. Simul. 2020, 85, 105239. [Google Scholar] [CrossRef]
- Arslan, E.; Narayanan, G.; Syed, A.M.; Arik, S.; Saroha, S. Controller design for finite-time and fixed-time stabilization of fractional-order memristive complex-valued BAM neural networks with uncertain parameters and time-varying delays. Neural Netw. 2020, 130, 60–74. [Google Scholar] [CrossRef]
- Du, F.F.; Lu, J.G.; Zhang, Q.H. Delay-dependent finite-time synchronization criterion of fractional-order delayed complex networks. Commun. Nonlinear Sci. Numer. Simul. 2023, 119, 107072. [Google Scholar] [CrossRef]
- Zhang, S.; Yu, Y.; Wang, H. Mittag-Leffler stability of fractional-order hopfield neural networks. Nonlinear Anal. Hybrid Syst. 2015, 16, 104–121. [Google Scholar] [CrossRef]
- Xu, S.; Lam, J.; Ho, D.W.; Zou, Y. Global robust exponential stability analysis for interval recurrent neural networks. Phys. Lett. A 2004, 325, 124–133. [Google Scholar] [CrossRef]
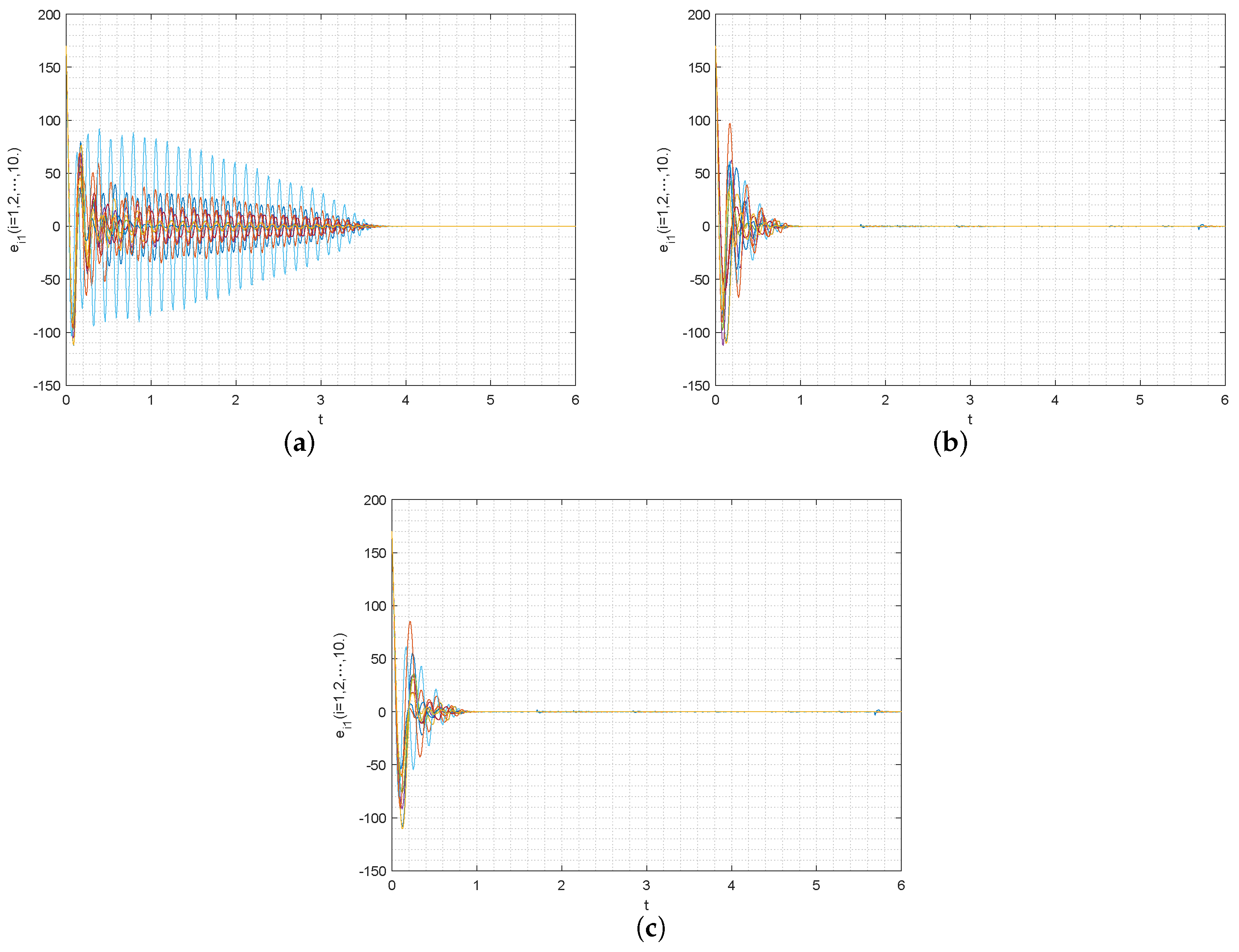

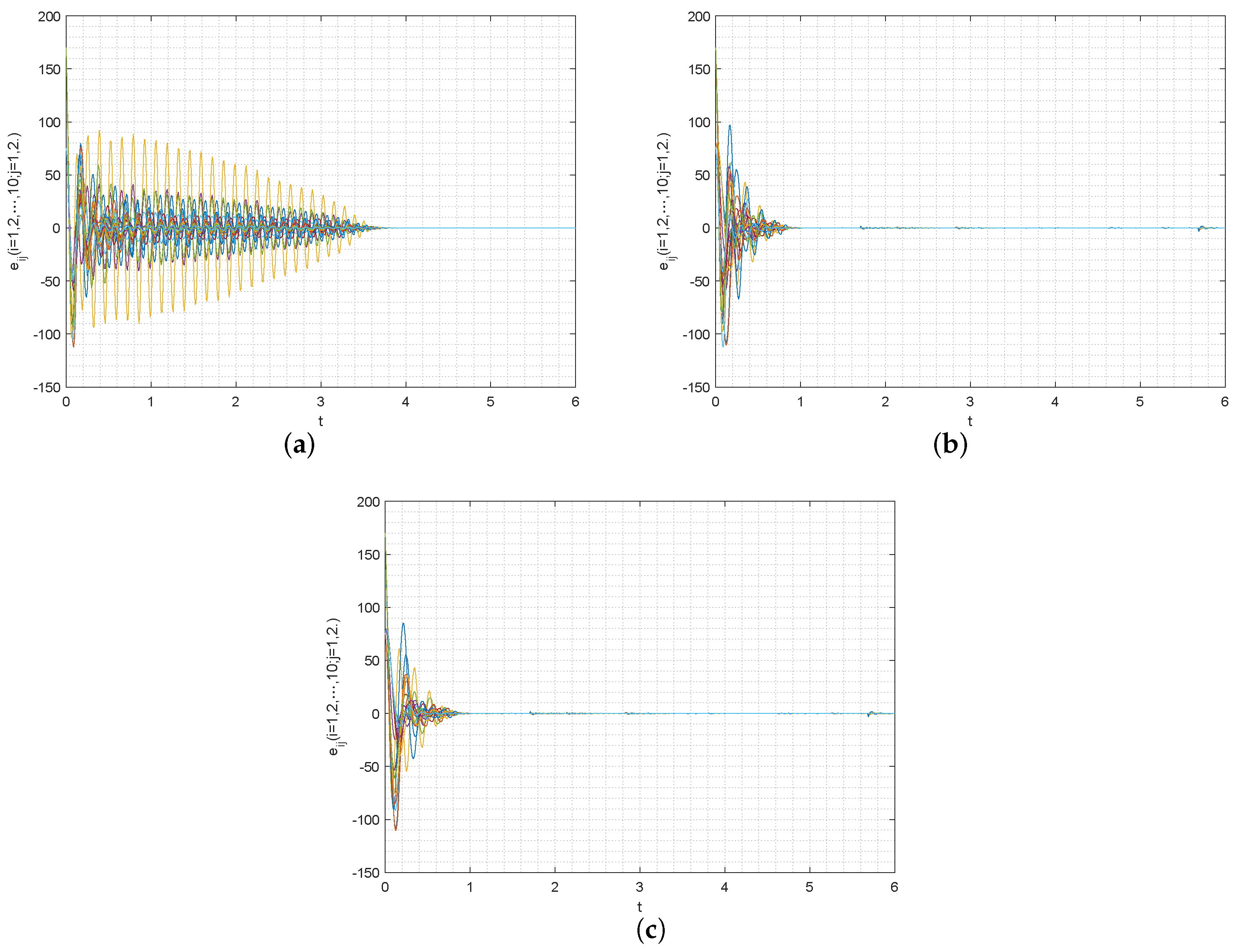
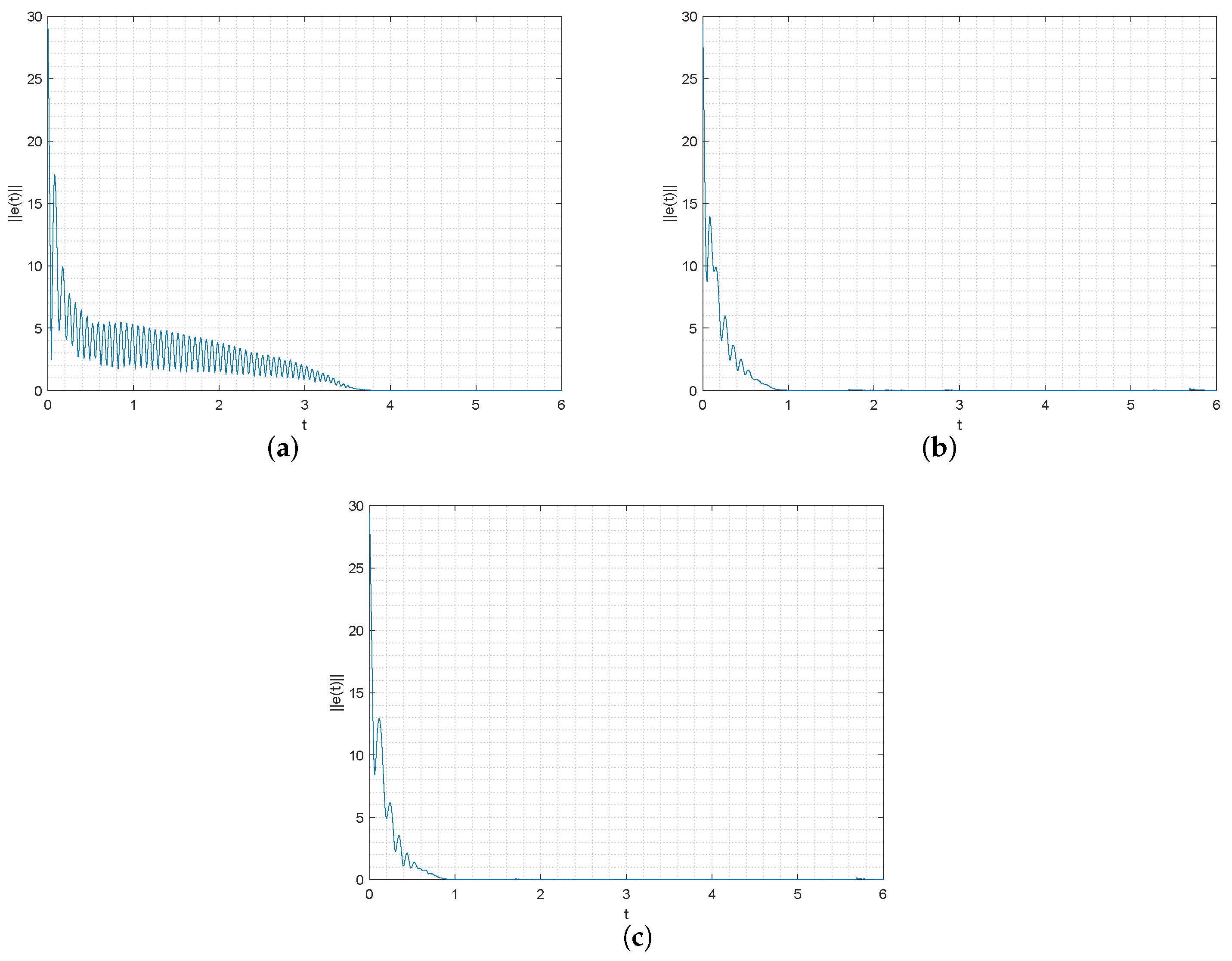
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, X.; Wang, Y.; Li, T.; Kang, R.; Zhao, Y. Novel Controller Design for Finite-Time Synchronization of Fractional-Order Nonidentical Complex Dynamical Networks under Uncertain Parameters. Fractal Fract. 2024, 8, 155. https://doi.org/10.3390/fractalfract8030155
He X, Wang Y, Li T, Kang R, Zhao Y. Novel Controller Design for Finite-Time Synchronization of Fractional-Order Nonidentical Complex Dynamical Networks under Uncertain Parameters. Fractal and Fractional. 2024; 8(3):155. https://doi.org/10.3390/fractalfract8030155
Chicago/Turabian StyleHe, Xiliang, Yu Wang, Tianzeng Li, Rong Kang, and Yu Zhao. 2024. "Novel Controller Design for Finite-Time Synchronization of Fractional-Order Nonidentical Complex Dynamical Networks under Uncertain Parameters" Fractal and Fractional 8, no. 3: 155. https://doi.org/10.3390/fractalfract8030155
APA StyleHe, X., Wang, Y., Li, T., Kang, R., & Zhao, Y. (2024). Novel Controller Design for Finite-Time Synchronization of Fractional-Order Nonidentical Complex Dynamical Networks under Uncertain Parameters. Fractal and Fractional, 8(3), 155. https://doi.org/10.3390/fractalfract8030155




