A Note on Fractional Third-Order Partial Differential Equations and the Generalized Laplace Transform Decomposition Method
Abstract
1. Intordection
2. Definitions and Ideas
- If we put , and , we obtain double Laplace transform
- If we put and replace s with , we obtain Laplace–Yang Transform
- At and replacing by v, respectively, we obtain double Sumudu Transform
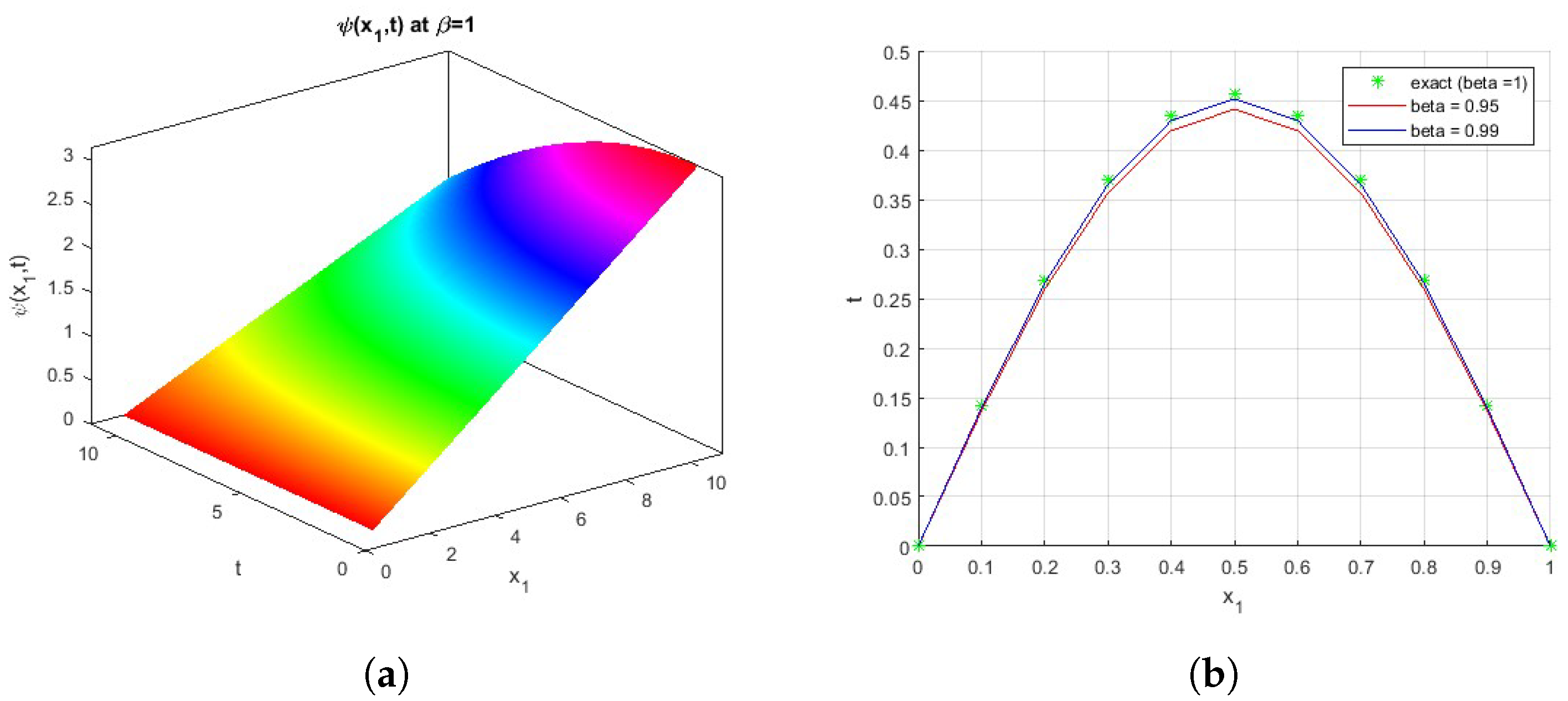
3. n + 1-Dimensional Fractional Dispersive KDV Equation and MGLTDM
| Exact | The Method | Error | The Method | Error |
|---|---|---|---|---|
| β = 1 | β = 0.95 | β = 0.99 | ||
| 0 | 0 | 0 | 0 | 0 |
| 0.1412 | 0.1363 | 0.0049 | 0.1396 | 0.0016 |
| 0.2686 | 0.2592 | 0.0094 | 0.2655 | 0.0031 |
| 0.3697 | 0.3568 | 0.0129 | 0.3654 | 0.0043 |
| 0.4346 | 0.4194 | 0.0152 | 0.4296 | 0.0050 |
| 0.4570 | 0.4410 | 0.0159 | 0.4517 | 0.0053 |
| 0.4346 | 0.4194 | 0.0152 | 0.4296 | 0.0050 |
| 0.3697 | 0.3568 | 0.0129 | 0.3654 | 0.0043 |
| 0.2686 | 0.2592 | 0.0094 | 0.2655 | 0.0031 |
| 0.1412 | 0.1363 | 0.0049 | 0.1396 | 0.0016 |
| 0.0000 | 0.0000 | 0.0000 | 0.0000 | 0.0000 |
| Exact | The Method | Error | The Method | Error |
|---|---|---|---|---|
| β = 1 | β = 0.95 | β = 0.99 | ||
| 0 | 0 | 0 | 0 | 0 |
| 0.1672 | 0.1693 | 0.0021 | 0.1676 | 0.0004 |
| 0.3277 | 0.3318 | 0.0041 | 0.3286 | 0.0009 |
| 0.4751 | 0.4811 | 0.0059 | 0.4764 | 0.0013 |
| 0.6036 | 0.6112 | 0.0075 | 0.6052 | 0.0016 |
| 0.7081 | 0.7169 | 0.0088 | 0.7100 | 0.0019 |
| 0.7843 | 0.7941 | 0.0098 | 0.7864 | 0.0021 |
| 0.8292 | 0.8396 | 0.0104 | 0.8314 | 0.0022 |
| 0.8411 | 0.8516 | 0.0105 | 0.8433 | 0.0022 |
| 0.8195 | 0.8297 | 0.0102 | 0.8216 | 0.0022 |
| 0.7651 | 0.7747 | 0.0096 | 0.7672 | 0.0020 |
| Exact | The Method | Error | The Method | Error |
|---|---|---|---|---|
| β = 1 | β = 0.95 | β = 0.99 | ||
| 0 | 0 | 0 | 0 | 0 |
| 0.1248 | 0.1298 | 0.0050 | 0.1257 | 0.0009 |
| 0.2496 | 0.2595 | 0.0099 | 0.2514 | 0.0018 |
| 0.3744 | 0.3893 | 0.0149 | 0.3771 | 0.0027 |
| 0.4992 | 0.5190 | 0.0198 | 0.5028 | 0.0036 |
| 0.6240 | 0.6488 | 0.0248 | 0.6285 | 0.0045 |
| 0.7488 | 0.7785 | 0.0297 | 0.7542 | 0.0054 |
| 0.8736 | 0.9083 | 0.0347 | 0.8799 | 0.0063 |
| 0.9984 | 1.0380 | 0.0396 | 1.0056 | 0.0072 |
| 1.1232 | 1.1678 | 0.0446 | 1.1313 | 0.0081 |
| 1.2480 | 1.2975 | 0.0495 | 1.2571 | 0.0091 |
| Exact | The Method | Error | The Method | Error |
|---|---|---|---|---|
| β = 1 | β = 0.95 | β = 0.99 | ||
| 0 | 0 | 0 | 0 | 0 |
| 0.0148 | 0.0163 | 0.0015 | 0.0153 | 0.0005 |
| 0.0536 | 0.0578 | 0.0042 | 0.0550 | 0.0014 |
| 0.0973 | 0.1036 | 0.0064 | 0.0994 | 0.0021 |
| 0.1161 | 0.1226 | 0.0065 | 0.1182 | 0.0021 |
| 0.0774 | 0.0812 | 0.0038 | 0.0787 | 0.0012 |
| −0.0458 | −0.0478 | 0.0020 | −0.0465 | 0.0007 |
| −0.2667 | −0.2768 | 0.0102 | −0.2700 | 0.0034 |
| −0.5775 | −0.5970 | 0.0195 | −0.5840 | 0.0065 |
| −0.9475 | −0.9759 | 0.0283 | −0.9569 | 0.0094 |
| −1.3242 | −1.3590 | 0.0349 | −1.3357 | 0.0116 |
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Korteweg, D.J.; de Vries, G. On the change of form of long waves advancing in a rectangular canal, and on a new type of long stationary waves. Philos. Mag. 1895, 39, 422–443. [Google Scholar] [CrossRef]
- Kenig, C.E.; Ponce, G.; Vega, L. A bilinear estimate with applications to the KdV equation. J. Am. Math. Soc. 1996, 9, 573–603. [Google Scholar] [CrossRef]
- Veeresha, P.; Prakasha, D.G.; Singh, J. Solution for fractional forced KdV equation using fractional natural decomposition method. AIMS Math. 2020, 5, 798–810. [Google Scholar] [CrossRef]
- Kelil, A.S.; Appadu, A.R. On the Numerical Solution of 1D and 2D KdV Equations Using Variational Homotopy Perturbation and Finite Difference Methods. Mathematics 2022, 10, 4443. [Google Scholar] [CrossRef]
- Areshi, M.; El-Tantawy, S.A.; Alotaibi, B.M.; Zaland, S. Study of Fuzzy Fractional Third-Order Dispersive KdV the equation in a Plasma under Atangana-Baleanu Derivative. J. Funct. 2022, 2022, 7922001. [Google Scholar] [CrossRef]
- Shah, R.; Khan, H.; Arif, M.; Kumam, P. Application of Laplace–Adomian Decomposition Method for the Analytical Solution of Third-Order Dispersive Fractional Partial Differential Equations. Entropy 2019, 21, 335. [Google Scholar] [CrossRef]
- Djidjeli, K.; Price, W.G.; Twizell, E.H.; Wang, Y. Numerical methods for the solution of the third-and fifth-order dispersive Korteweg-de Vries equations. J. Comput. Appl. Math. 1995, 58, 307–336. [Google Scholar] [CrossRef]
- Prakash, A.; Kumar, M. Numerical method for fractional dispersive partial differential equations. Commun. Numer. Anal. 2017, 1, 1–18. [Google Scholar] [CrossRef]
- Akdi, M.; Sedra, M.B. Numerical KDV Equation by the Adomian Decomposition Method. Am. J. Mod. Phys. 2013, 2, 111–115. [Google Scholar] [CrossRef]
- Soliman, A.A.; Abdou, M.A. The decomposition method for solving the coupled modified KdV equations. Math. Comput. 2008, 47, 1035–1041. [Google Scholar] [CrossRef]
- Kanth, A.R.; Aruna, K. Solution of fractional third-order dispersive partial differential equations. Egypt. J. Basic Appl. Sci. 2015, 2, 190–199. [Google Scholar]
- Sultana, T.; Khan, A.; Khandelwal, P. A new non-polynomial spline method for solution of linear and non-linear third order dispersive equations. Adv. Differ. Equ. 2018, 2018, 316. [Google Scholar] [CrossRef]
- Pandey, R.K.; Mishra, H.K. Homotopy analysis Sumudu transform method for time-fractional third order dispersive partial differential equation. Adv. Comput. Math. 2017, 43, 365–383. [Google Scholar] [CrossRef]
- Nuruddeen, R.I.; Akbar, Y.; Kim, H.J. On the application of Gα integral transform to nonlinear dynamical models with non-integer order derivatives. AIMS Math. 2022, 7, 17859–17878. [Google Scholar] [CrossRef]
- Kim, H.J.; Sattaso, S.; Nonlaopon, K.; Kaewnimit, K. An application of generalized Laplace transform in PDEs. Adv. Dyn. Syst. Appl. 2019, 14, 257–265. [Google Scholar] [CrossRef]
- Prasertsang, P.; Sattaso, S.; Nonlaopon, K.; Kim, H.J. Analytical study for certain ordinary differential equations with variable coefficients via Gα-transform. Eur. J. Pure Appl. Math. 2021, 14, 1184–1199. [Google Scholar] [CrossRef]
- Sattaso, S.; Nonlaopon, K.; Kim, H.; Al-Omari, S. Certain Solutions of Abel’s Integral Equations on Distribution Spaces via Distributional Gα-Transform. Symmetry 2023, 15, 53. [Google Scholar] [CrossRef]
- Eltayeb, H.; Alhefthi, R.K. Solution of Fractional Third-Order Dispersive Partial Differential Equations and Symmetric KdV via Sumudu–Generalized Laplace Transform Decomposition. Symmetry 2023, 15, 1540. [Google Scholar] [CrossRef]
- Sattaso, S.; Nonlaopon, K.; Kim, H. Further Properties of Laplace–Type Integral Transform. Dyn. Syst. Appl. 2019, 28, 195–215. [Google Scholar]
- Ghandehari, M.A.M.; Ranjbar, M. A numerical method for solving a fractional partial differential equation through converting it into an NLP problem. Comput. Math. Appl. 2013, 65, 975–982. [Google Scholar] [CrossRef]
- Bayrak, M.A.; Demir, A. A new approach for space-time fractional partial differential equations by residual power series method. Appl. Math. Comput. 2018, 336, 215–230. [Google Scholar]
- Thabet, H.; Kendre, S. Analytical solutions for conformable space-time fractional partial differential equations via fractional differential transform. Chaos Solitons Fractals 2018, 109, 238–245. [Google Scholar] [CrossRef]
- Eltayeb, H.; Bachar, I.; Abdalla, Y.T. A note on time-fractional Navier–Stokes equation and multi-Laplace transform decomposition method. Adv. Differ. Equ. 2020, 2020, 519. [Google Scholar] [CrossRef]
- Eltayeb, H.; Mesloub, S. The New G-Double-Laplace Transforms and One-Dimensional Coupled Sine-Gordon Equations. Axioms 2024, 13, 385. [Google Scholar] [CrossRef]
- Eltayeb, H. Analytic Solution of the Time-Fractional Partial Differential Equation Using a Multi-G-Laplace Transform Method. Fractal Fract. 2024, 8, 435. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Eltayeb, H.; Elgezouli, D.E. A Note on Fractional Third-Order Partial Differential Equations and the Generalized Laplace Transform Decomposition Method. Fractal Fract. 2024, 8, 602. https://doi.org/10.3390/fractalfract8100602
Eltayeb H, Elgezouli DE. A Note on Fractional Third-Order Partial Differential Equations and the Generalized Laplace Transform Decomposition Method. Fractal and Fractional. 2024; 8(10):602. https://doi.org/10.3390/fractalfract8100602
Chicago/Turabian StyleEltayeb, Hassan, and Diaa Eldin Elgezouli. 2024. "A Note on Fractional Third-Order Partial Differential Equations and the Generalized Laplace Transform Decomposition Method" Fractal and Fractional 8, no. 10: 602. https://doi.org/10.3390/fractalfract8100602
APA StyleEltayeb, H., & Elgezouli, D. E. (2024). A Note on Fractional Third-Order Partial Differential Equations and the Generalized Laplace Transform Decomposition Method. Fractal and Fractional, 8(10), 602. https://doi.org/10.3390/fractalfract8100602






