The Finite Volume Element Method for Time Fractional Generalized Burgers’ Equation
Abstract
1. Introduction
2. Fully Discrete FVE Scheme
3. Some Lemmas
4. Convergence Analysis
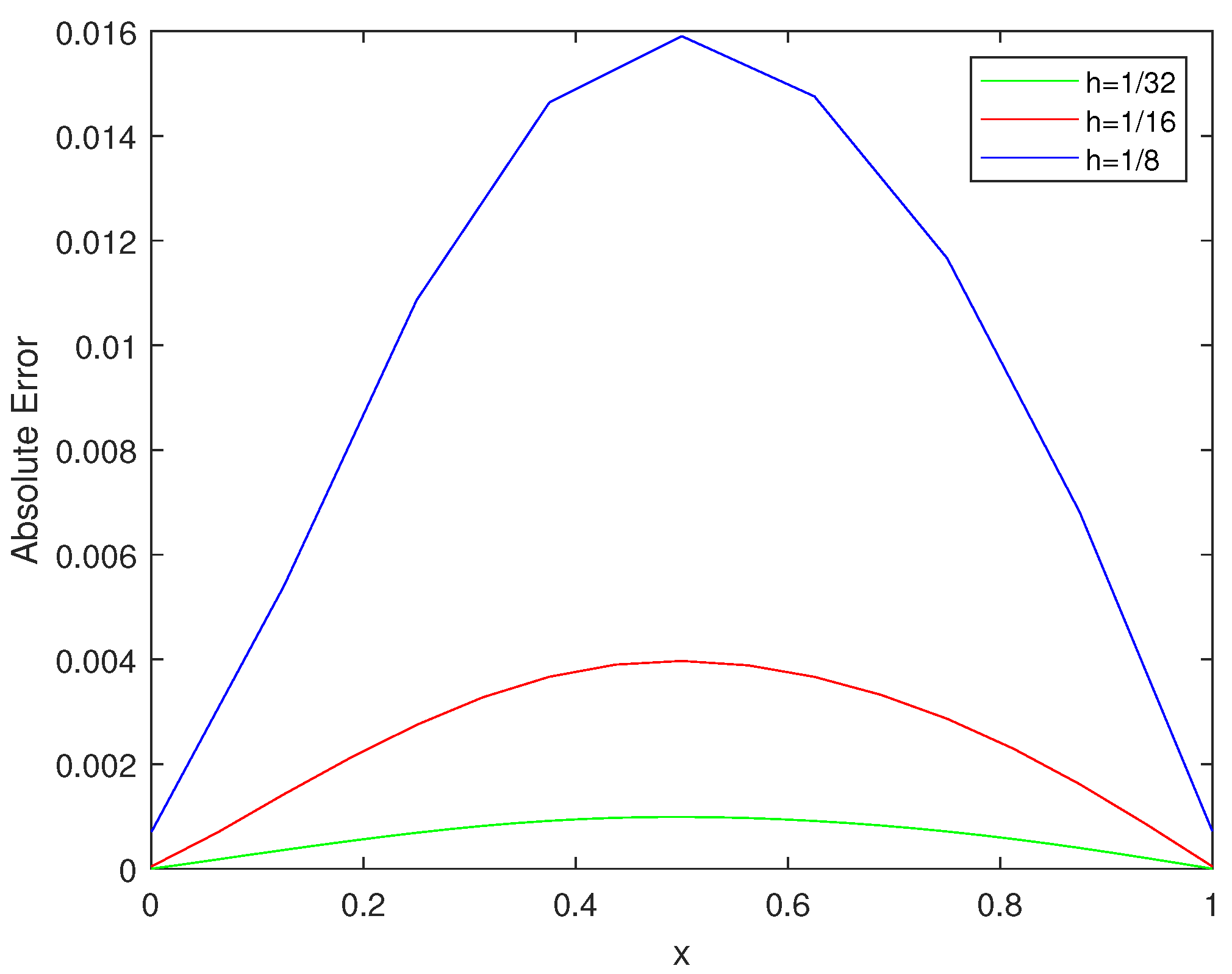
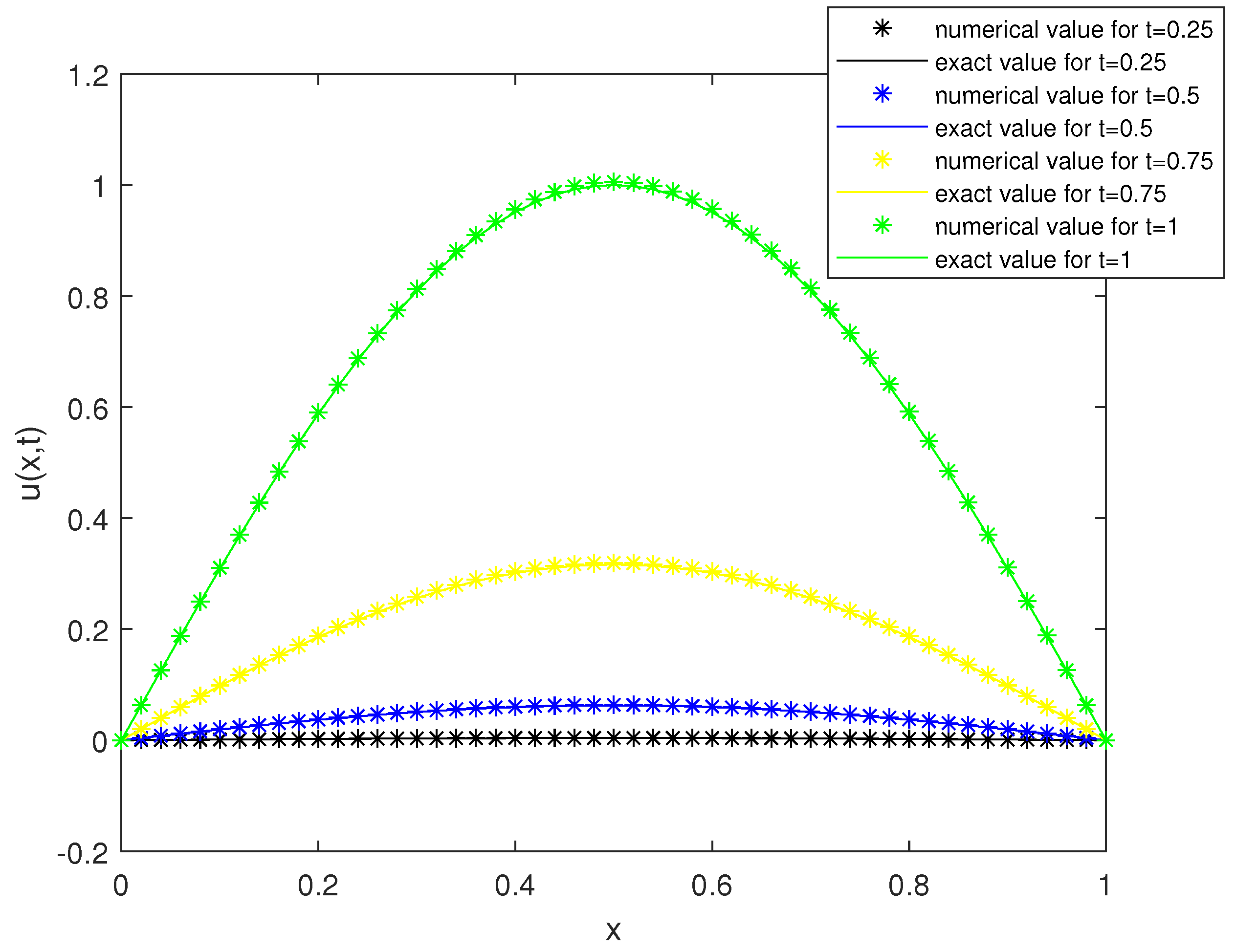
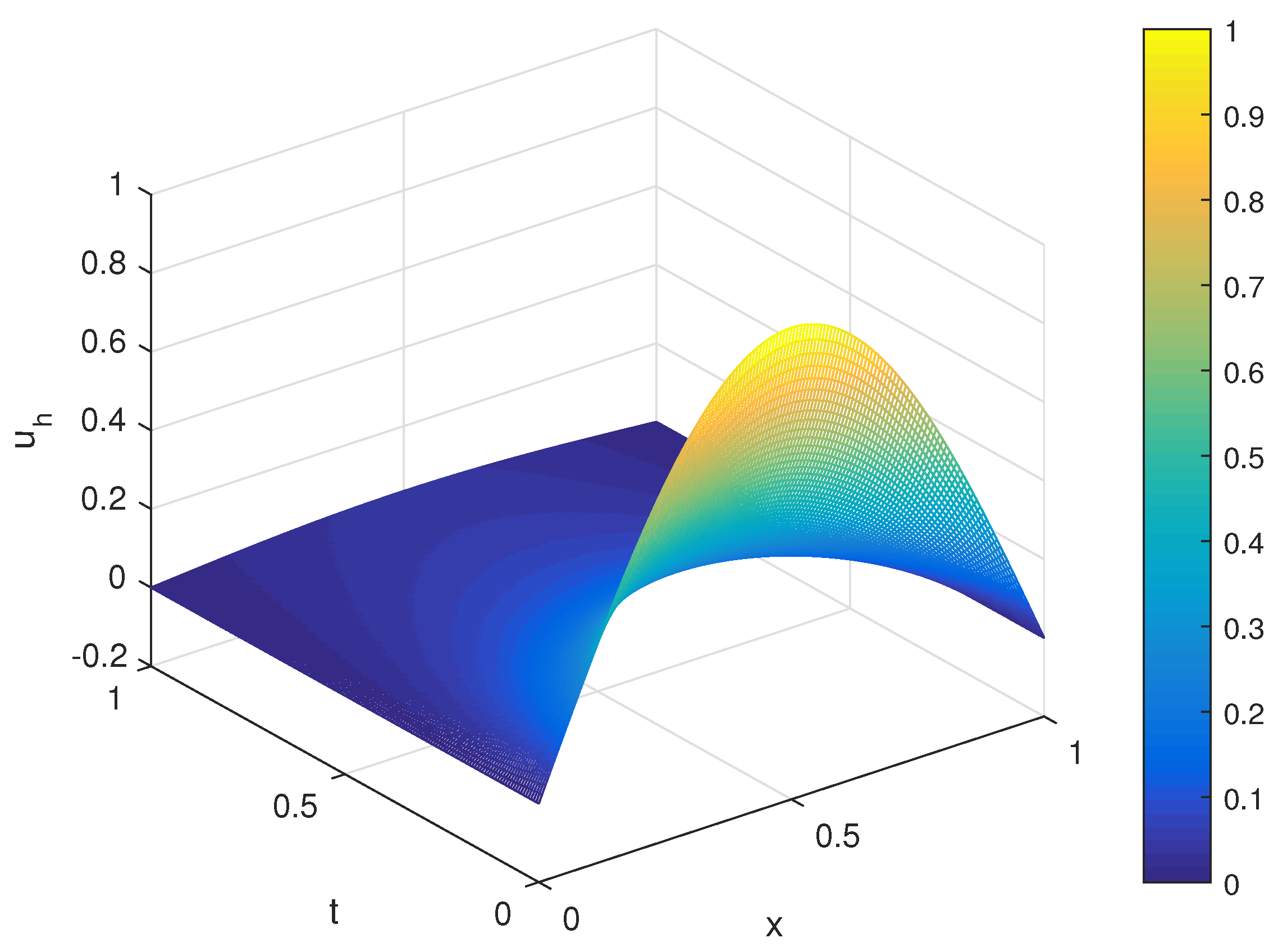
5. Numerical Examples
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Öziş, T.; Aksan, E.N.; Özdeş, A. A finite element approach for solution of Burgers’ equation. Appl. Math. Comput. 2003, 139, 417–428. [Google Scholar] [CrossRef]
- Dhawan, S.; Kapoor, S.; Kumar, S.; Rawat, S. Contemporary review of techniques for the solution of nonlinear Burgers equation. J. Comput. Sci. 2012, 3, 405–419. [Google Scholar] [CrossRef]
- Khater, A.H.; Temsah, R.S.; Hassan, M.M. A Chebyshev spectral collocation method for solving Burgers’-type equations. J. Comput. Appl. Math. 2008, 222, 333–350. [Google Scholar] [CrossRef]
- Oz, F.; Vuppala, R.K.; Kara, K.; Gaitan, F. Solving Burgers’ equation with quantum computing. Quantum Inf. Process. 2022, 21, 30. [Google Scholar] [CrossRef]
- Fu, F.; Li, J.; Lin, J.; Guan, Y.; Gao, F.; Zhang, C.; Chen, L. Moving least squares particle hydrodynamics method for Burgers’ equation. Appl. Math. Comput. 2019, 356, 362–378. [Google Scholar] [CrossRef]
- Seadawy, A.R.; Iqbal, M.; Lu, D. Propagation of long-wave with dissipation and dispersion in nonlinear media via generalized Kadomtsive–Petviashvili modified equal width-Burgers equation. Indian J. Phys. 2020, 94, 675–687. [Google Scholar] [CrossRef]
- Jafari, H.; Babaei, A.; Banihashemi, S. A novel approach for solving an inverse reaction-diffusion-convection problem. J. Optim. Theory Appl. 2019, 183, 688–704. [Google Scholar] [CrossRef]
- Nikan, O.; Jafari, H.; Golbabai, A. Numerical analysis of the fractional evolution model for heat flow in materials with memory. Alex. Eng. J. 2020, 59, 2627–2637. [Google Scholar] [CrossRef]
- Mitkowski, W. Approximation of fractional diffusion-wave equation. Acta Mech. Autom. 2011, 5, 65–68. [Google Scholar]
- Momani, S. Non-perturbative analytical solutions of the space-and time-fractional Burgers equations. Chaos Solitons Fractals 2006, 28, 930–937. [Google Scholar] [CrossRef]
- Uddin, M.H.; Arefin, M.A.; Akbar, M.A.; Inc, M. New explicit solutions to the fractional-order Burgers’ equation. Math. Probl. Eng. 2021, 2021, 6698028. [Google Scholar] [CrossRef]
- Esen, A.; Bulut, F.; Oruç, Ö. A unified approach for the numerical solution of time fractional Burgers’ type equations. Eur. Phys. J. Plus 2016, 131, 116. [Google Scholar] [CrossRef]
- Wang, X.; Wang, L. Traveling wave solutions of conformable time fractional Burgers type equations. Aims Math 2021, 6, 7266–7284. [Google Scholar] [CrossRef]
- Abdelwahed, H.G.; El-Shewy, E.K.; Mahmoud, A.A. On the time fractional modulation for electron acoustic shock waves. Chin. Phys. Lett. 2017, 34, 035202. [Google Scholar] [CrossRef]
- Garra, R. Fractional-calculus model for temperature and pressure waves in fluid-saturated porous rocks. Phys. Rev. E 2011, 84, 036605. [Google Scholar] [CrossRef]
- Keller, J.J. Propagation of simple non linear waves in gas filled tubes with friction. Z. FüR Angew. Math. Phys. ZAMP 1981, 32, 170–181. [Google Scholar] [CrossRef]
- Sugimoto, N. Burgers equation with a fractional derivative; Hereditary effects on non-linear acoustic waves. J. Fluid Mech. 1991, 225, 631–653. [Google Scholar] [CrossRef]
- Li, D.; Zhang, C.; Ran, M. A linear finite difference scheme for generalized time fractional Burgers equation. Appl. Math. Model. 2016, 40, 6069–6081. [Google Scholar] [CrossRef]
- Qiu, W.; Chen, H.; Zheng, X. An implicit difference scheme and algorithm implementation for the one-dimensional time-fractional Burgers equations. Math. Comput. Simul. 2019, 166, 298–314. [Google Scholar] [CrossRef]
- Esen, A.; Tasbozan, O. Numerical solution of time fractional Burgers equation by cubic B-spline finite elements. Mediterr. J. Math. 2016, 13, 1325–1337. [Google Scholar] [CrossRef]
- Hussein, A.J. A weak Galerkin finite element method for solving time-fractional coupled Burgers’ equations in two dimensions. Appl. Numer. Math. 2020, 156, 265–275. [Google Scholar] [CrossRef]
- Wang, H.; Xu, D.; Zhou, J.; Guo, J. Weak Galerkin finite element method for a class of time fractional generalized Burgers’ equation. Numer. Methods Partial. Differ. Equ. 2021, 37, 732–749. [Google Scholar] [CrossRef]
- Zhang, Z.Y.; Guo, L.L. An alternative technique for the symmetry reduction of time-fractional partial differential equation. Math. Methods Appl. Sci. 2021, 44, 14957–14962. [Google Scholar] [CrossRef]
- Kurt, A.; Çenesiz, Y.; Tasbozan, O. On the solution of Burgers’ equation with the new fractional derivative. Open Phys. 2015, 13, 355–360. [Google Scholar] [CrossRef]
- Cao, W.; Xu, Q.; Zheng, Z. Solution of two-dimensional time-fractional Burgers equation with high and low Reynolds numbers. Adv. Differ. Equ. 2017, 2017, 338. [Google Scholar] [CrossRef]
- Liu, J.G.; Zhang, J. A new approximate method to the time fractional damped Burger equation. AIMS Math. 2023, 8, 13317–13324. [Google Scholar] [CrossRef]
- Li, R.; Chen, Z.; Wu, W. Generalized Difference Methods for Differential Equations: Numerical Analysis of Finite Volume Methods; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Wang, T. A mixed finite volume element method based on rectangular mesh for biharmonic equations. J. Comput. Appl. Math. 2004, 172, 117–130. [Google Scholar] [CrossRef]
- Zhang, T.; Zhong, H.; Zhao, J. A full discrete two-grid finite-volume method for a nonlinear parabolic problem. Int. J. Comput. Math. 2011, 88, 1644–1663. [Google Scholar] [CrossRef]
- Guo, Y.; Shi, Y.; Li, Y. A fifth-order finite volume weighted compact scheme for solving one-dimensional Burgers’ equation. Appl. Math. Comput. 2016, 281, 172–185. [Google Scholar] [CrossRef]
- Yang, Q. The upwind finite volume element method for two-dimensional Burgers equation. In Abstract and Applied Analysis; Hindawi Press: London, UK, 2013. [Google Scholar]
- Liu, F.; Zhuang, P.; Turner, I.; Burrage, K.; Anh, V. A new fractional finite volume method for solving the fractional diffusion equation. Appl. Math. Model. 2014, 38, 3871–3878. [Google Scholar] [CrossRef]
- Hejazi, H.; Moroney, T.; Liu, F. Stability and convergence of a finite volume method for the space fractional advection—Dispersion equation. J. Comput. Appl. Math. 2014, 255, 684–697. [Google Scholar] [CrossRef]
- Sayev, K.; Arjang, F. Finite volume element method and its stability analysis for analyzing the behavior of sub-diffusion problems. Appl. Math. Comput. 2016, 290, 224–239. [Google Scholar]
- Li, J.; Liu, F.; Feng, L.; Turner, I. A novel finite volume method for the Riesz space distributed-order advection—Diffusion equation. Appl. Math. Model. 2017, 46, 536–553. [Google Scholar] [CrossRef]
- Fu, H.; Sun, Y.; Wang, H.; Zheng, X. Stability and convergence of a Crank–Nicolson finite volume method for space fractional diffusion equations. Appl. Numer. Math. 2019, 139, 38–51. [Google Scholar] [CrossRef]
- Fu, H.; Liu, H.; Wang, H. A finite volume method for two-dimensional Riemann-Liouville space-fractional diffusion equation and its efficient implementation. J. Comput. Phys. 2019, 388, 316–334. [Google Scholar] [CrossRef]
- Wang, T.; Jiang, Z.; Zhu, A.; Yin, Z. A Mixed Finite Volume Element Method for Time-Fractional Damping Beam Vibration Problem. Fractal Fract. 2022, 6, 523. [Google Scholar] [CrossRef]
- Sun, Z.; Wu, X. A fully discrete difference scheme for a diffusion-wave system. Appl. Numer. Math. 2006, 56, 193–209. [Google Scholar] [CrossRef]
- Zhang, T.; Guo, Q. The finite difference/finite volume method for solving the fractional diffusion equation. J. Comput. Phys. 2018, 375, 120–134. [Google Scholar] [CrossRef]
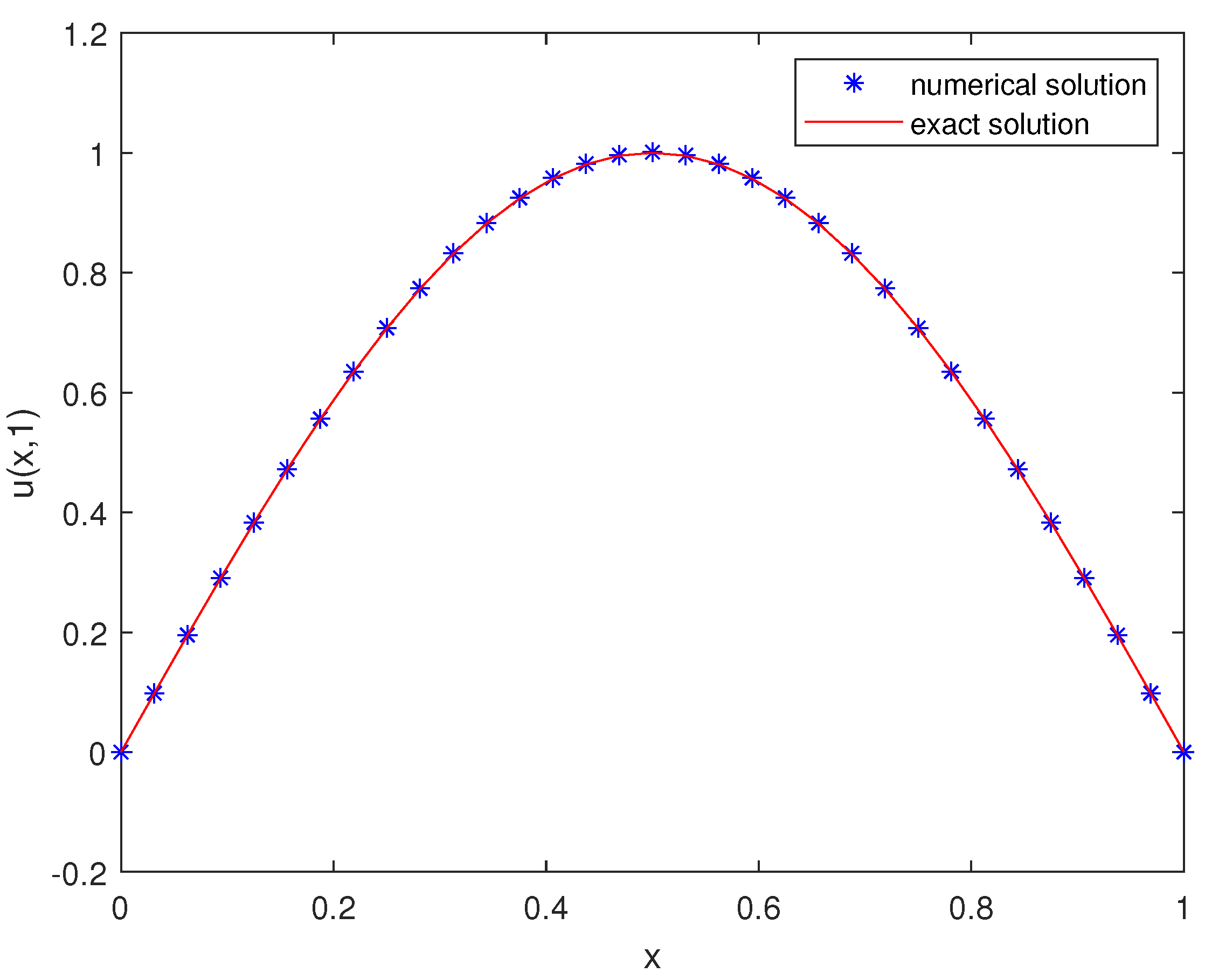
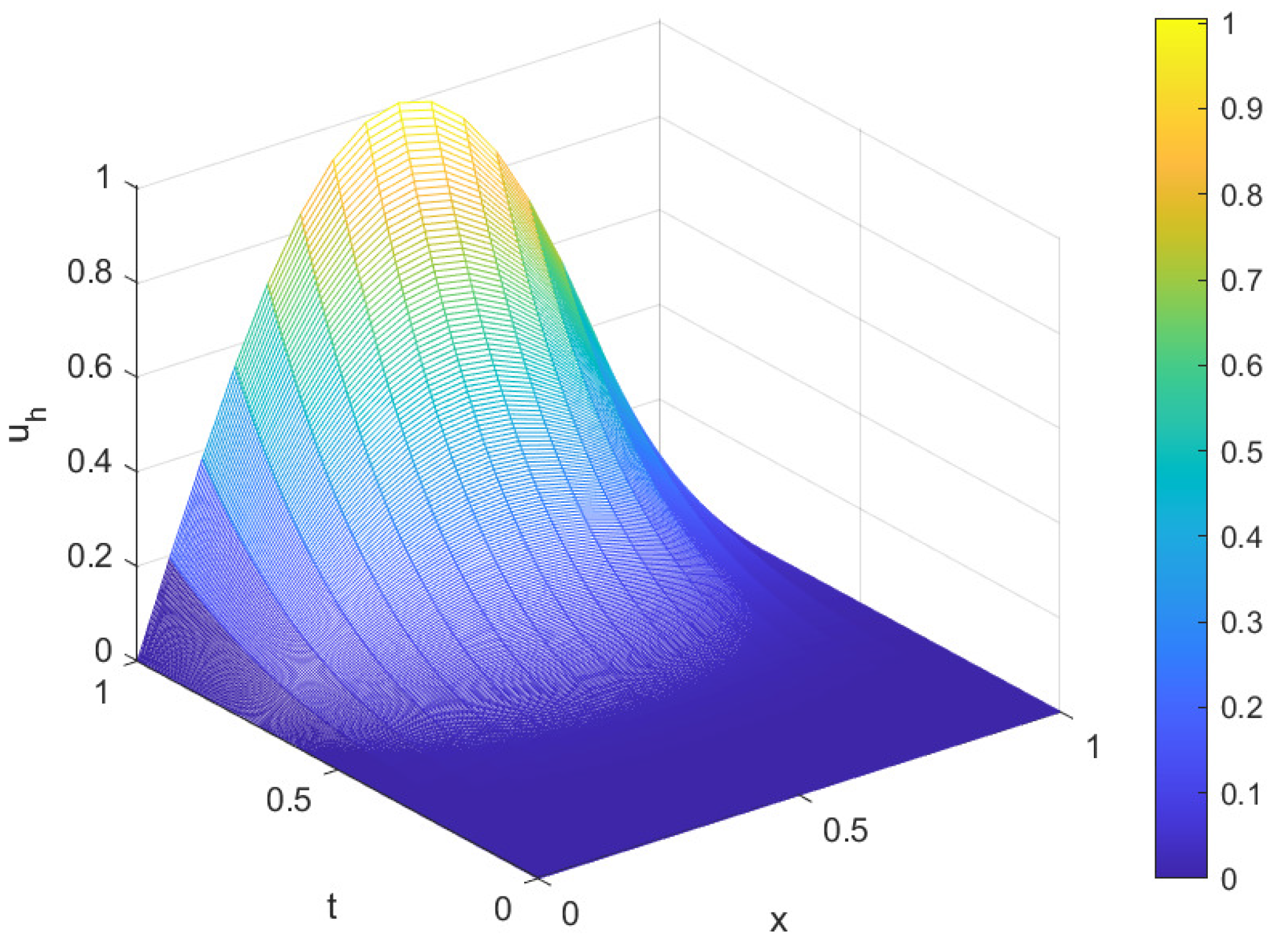
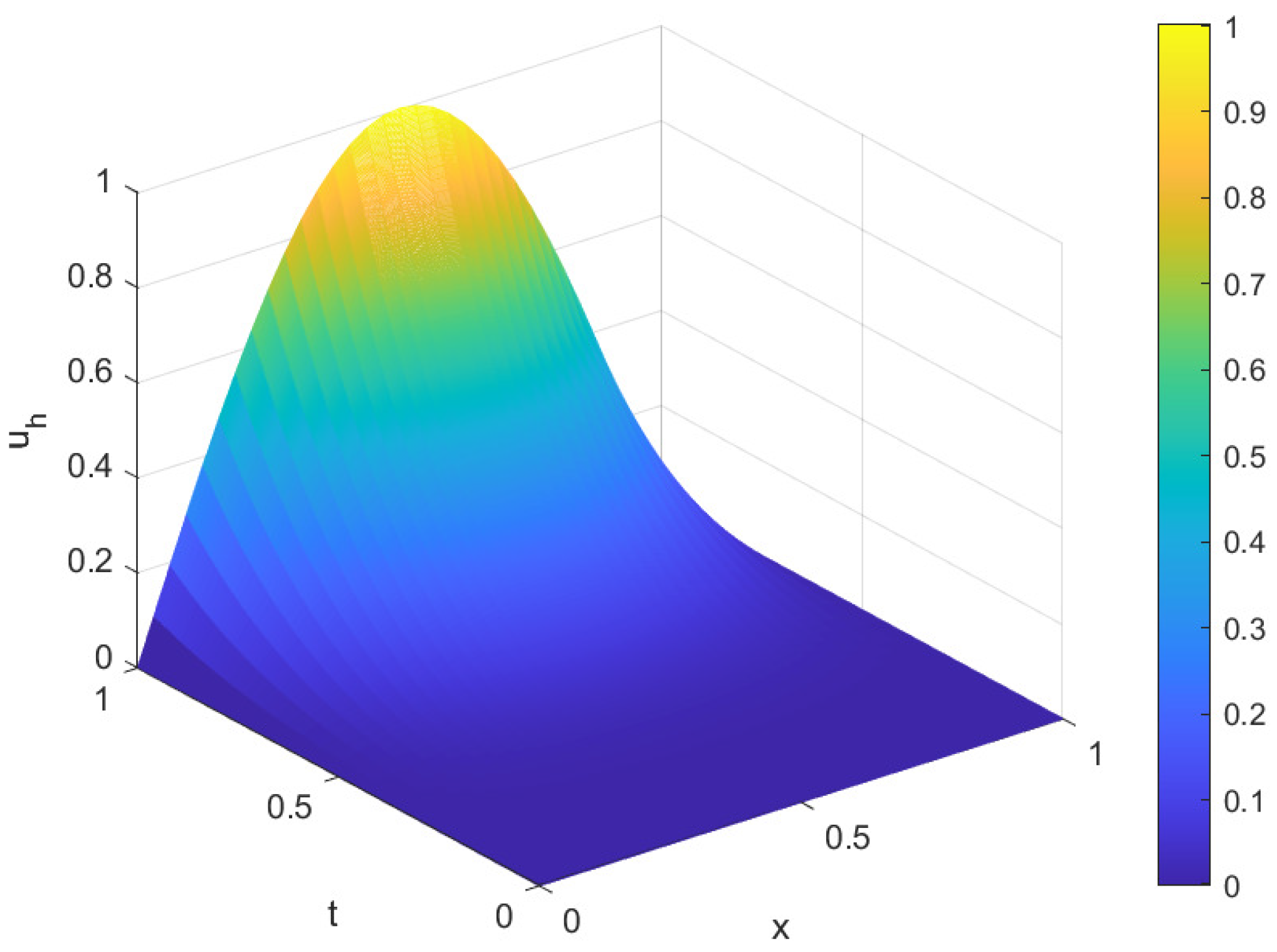
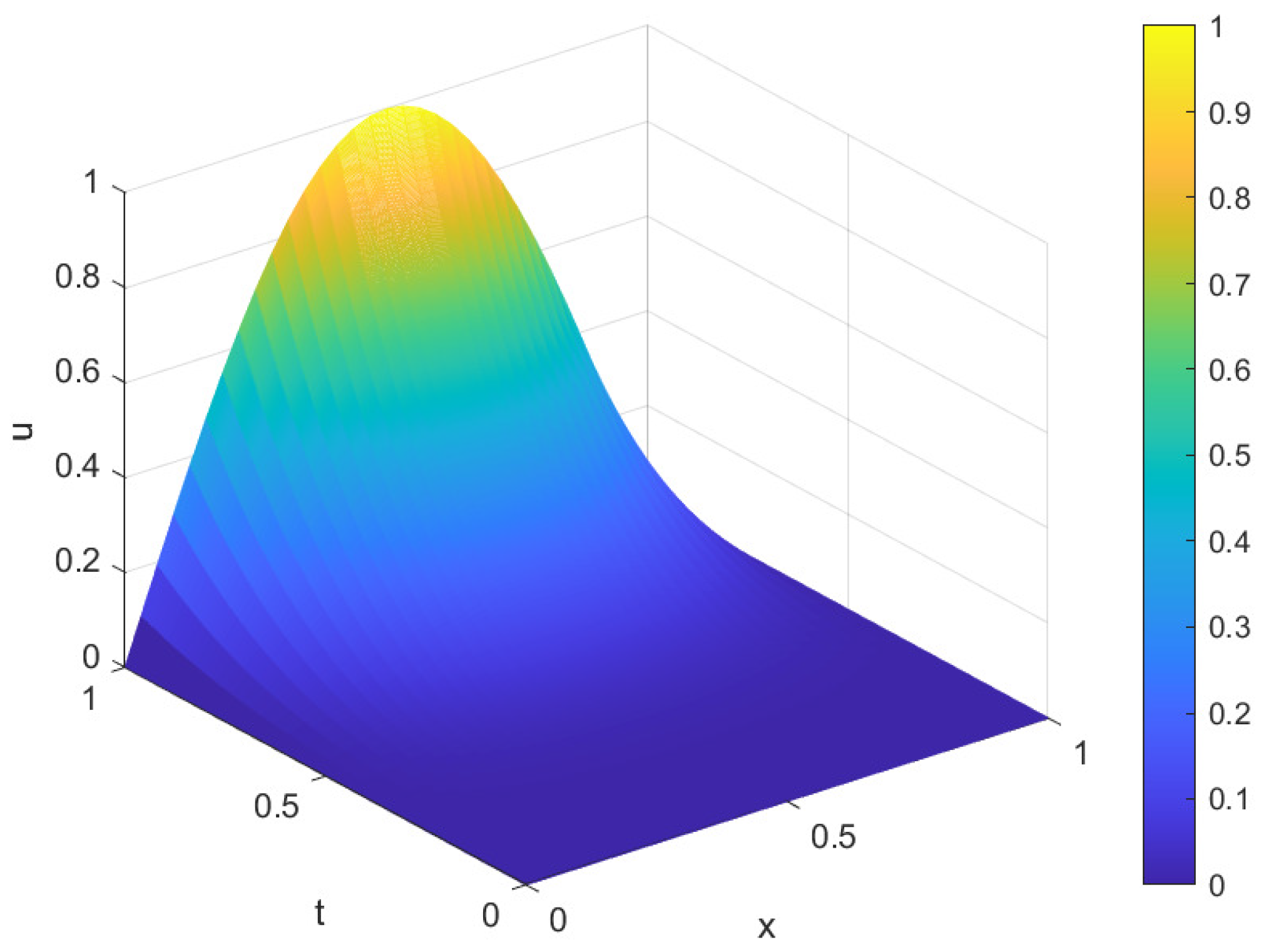

| Order | Order | ||||
|---|---|---|---|---|---|
| 1/10 | 0.0374 | - | 0.0311 | - | |
| 1/20 | 0.0185 | 1.0155 | 0.0158 | 0.9770 | |
| 1/40 | 0.0090 | 1.0395 | 0.0079 | 1.0000 | |
| 1/80 | 0.0044 | 1.0324 | 0.0040 | 0.9819 |
| h | Order | Order | |||
|---|---|---|---|---|---|
| 1/8 | 0.0121 | - | 0.0113 | - | |
| 1/16 | 0.0029 | 2.0609 | 0.0028 | 2.0128 | |
| 1/32 | 1.9992 | 1.9972 | |||
| 1/64 | 2.0162 | 2.0007 |
| h | Order | Order | |||
|---|---|---|---|---|---|
| 1/8 | 0.0299 | - | 0.0300 | - | |
| 1/16 | 0.0109 | 1.4558 | 0.0107 | 1.0310 | |
| 1/32 | 0.0039 | 1.4828 | 0.0038 | 1.0352 | |
| 1/64 | 0.0014 | 1.4780 | 0.0013 | 1.0726 |
| h | Order | Order | |||
|---|---|---|---|---|---|
| 1/25 | - | - | |||
| 1/30 | 0.7693 | 0.8220 | |||
| 1/50 | 0.8829 | 0.9325 | |||
| 1/75 | 1.2218 | 1.2626 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Yang, Q. The Finite Volume Element Method for Time Fractional Generalized Burgers’ Equation. Fractal Fract. 2024, 8, 53. https://doi.org/10.3390/fractalfract8010053
Zhang J, Yang Q. The Finite Volume Element Method for Time Fractional Generalized Burgers’ Equation. Fractal and Fractional. 2024; 8(1):53. https://doi.org/10.3390/fractalfract8010053
Chicago/Turabian StyleZhang, Jingru, and Qing Yang. 2024. "The Finite Volume Element Method for Time Fractional Generalized Burgers’ Equation" Fractal and Fractional 8, no. 1: 53. https://doi.org/10.3390/fractalfract8010053
APA StyleZhang, J., & Yang, Q. (2024). The Finite Volume Element Method for Time Fractional Generalized Burgers’ Equation. Fractal and Fractional, 8(1), 53. https://doi.org/10.3390/fractalfract8010053







