Fractional Optimal Control Model and Bifurcation Analysis of Human Syncytial Respiratory Virus Transmission Dynamics
Abstract
1. Introduction
2. Model Formulation
- •
- The total population is assumed to be constant.
- •
- The infection is seasonal.
3. Why the Caputo Operator? Reasons and Motivation
4. Analysis of RSV Model
4.1. Invariant Property
4.2. Non-Negativity Property
4.3. Existence and Uniqueness
4.4. Disease-Free Equilibrium
4.5. Endemic Equilibrium State
4.6. Basic Reproduction Number
4.7. Local Stability of the Disease-Free Equilibrium
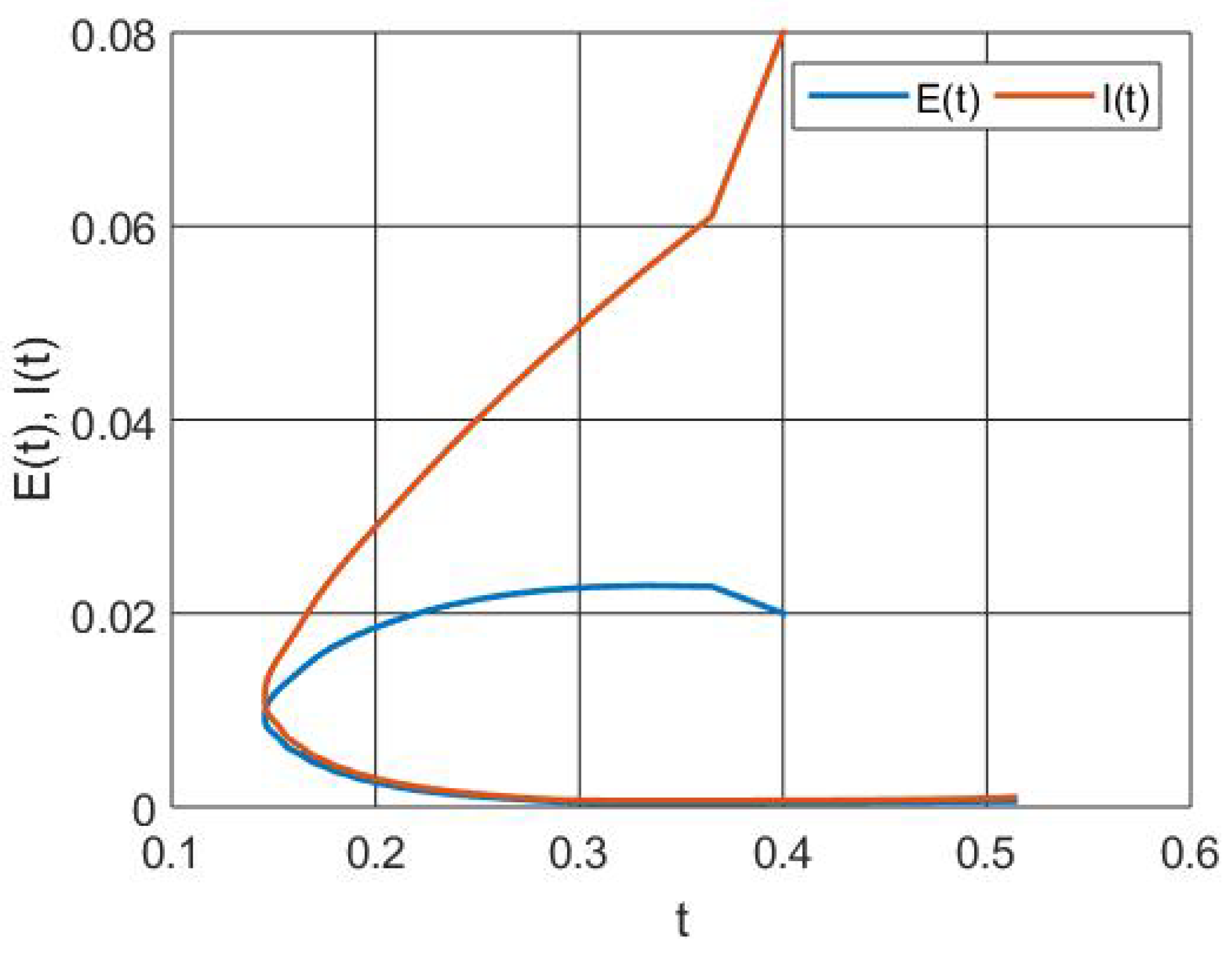
4.8. Bifurcation Analysis
4.9. Stability of Endemic Equilibrium
5. Fractional Optimal Control Problem
5.1. Objective Functional
5.2. Hamiltonian Function
5.3. Adjoint System
5.4. Optimal Control
5.5. Optimality System
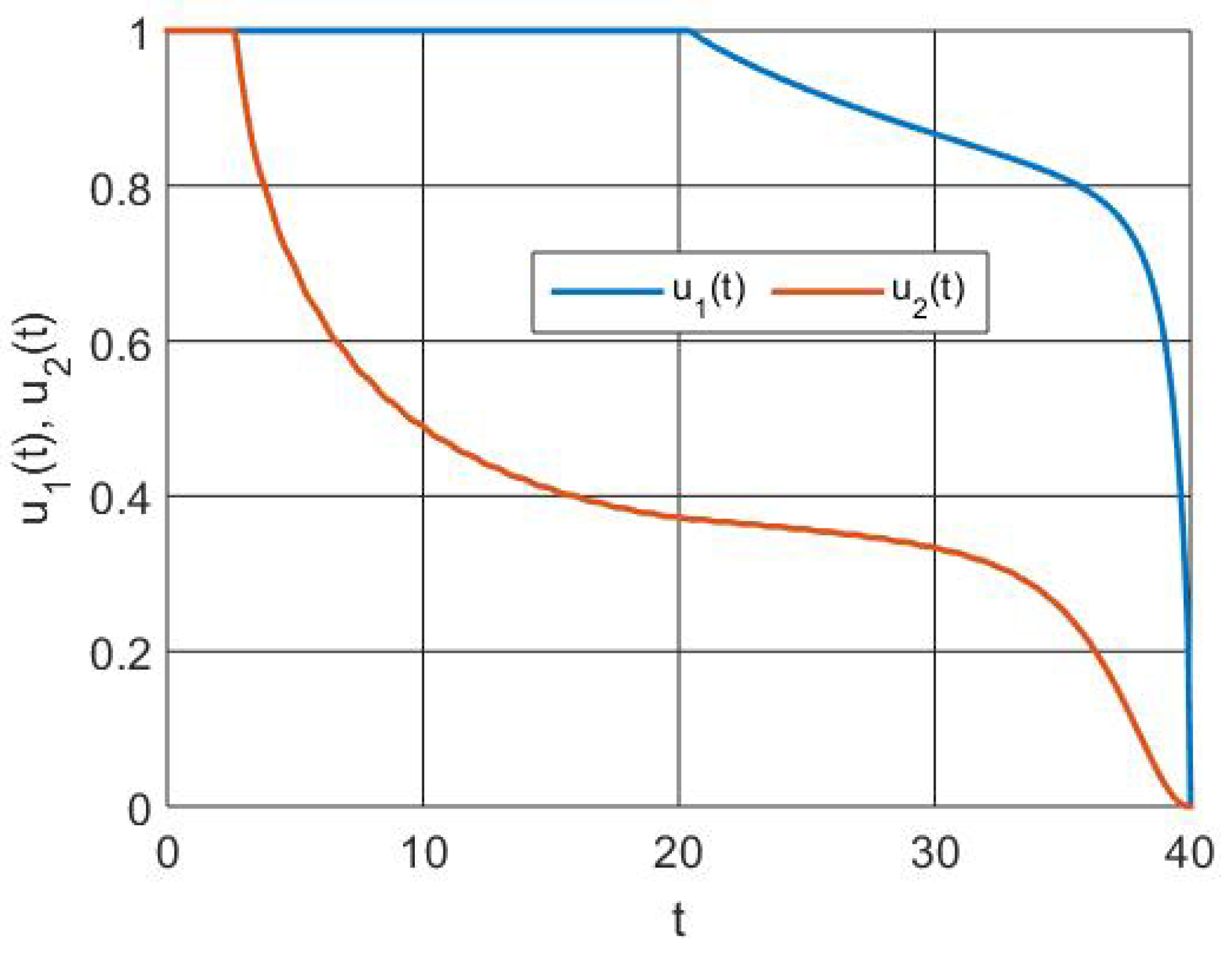
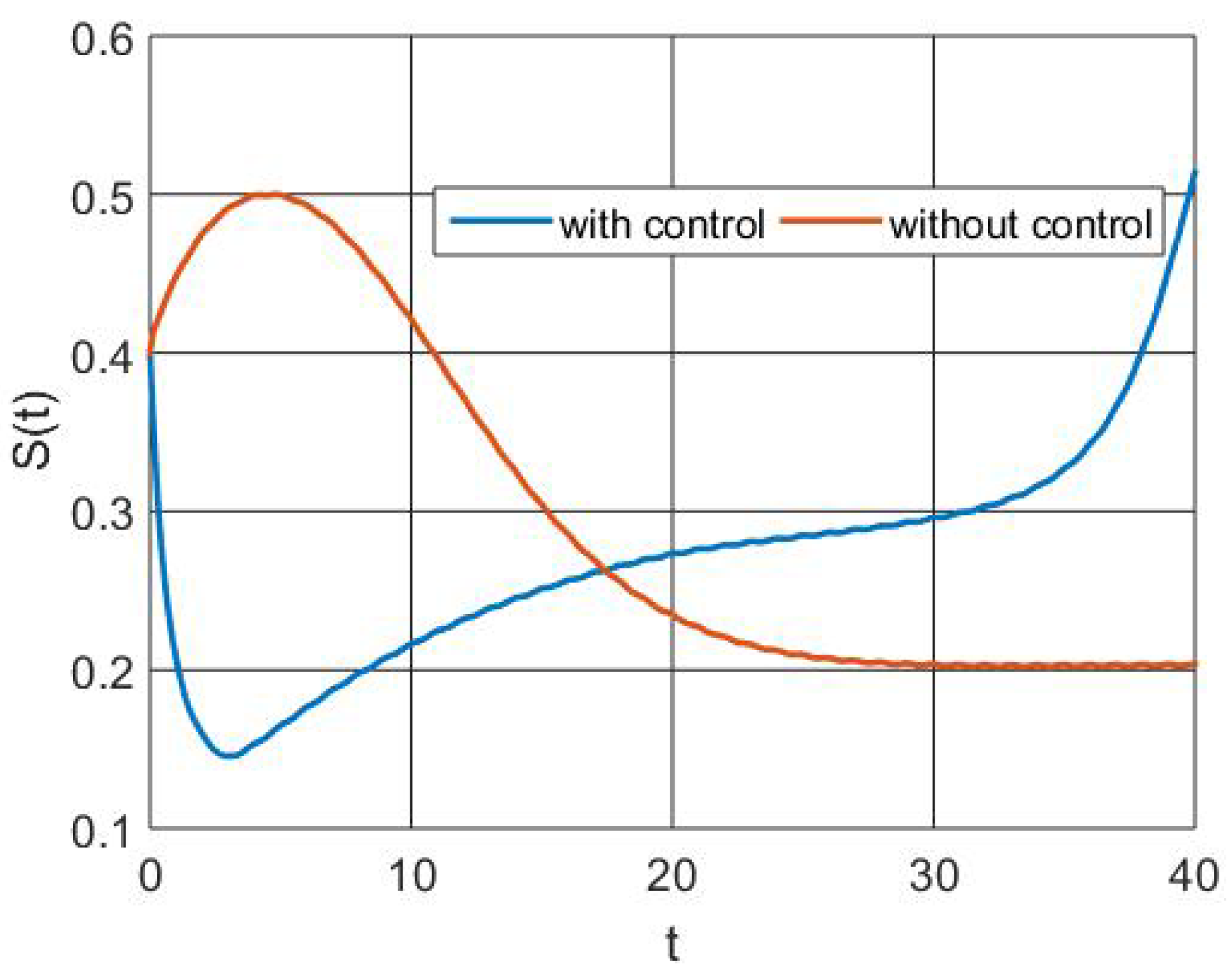
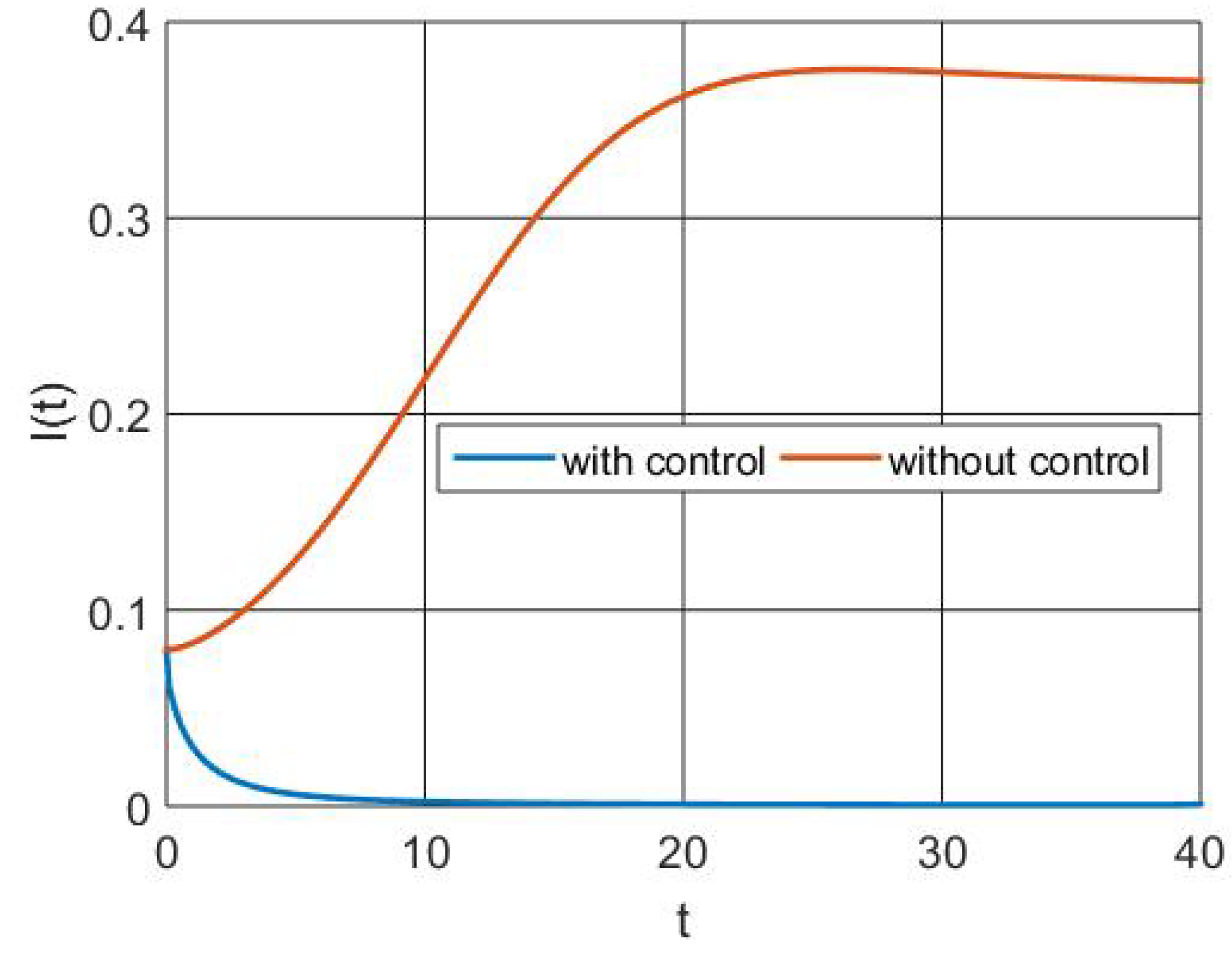
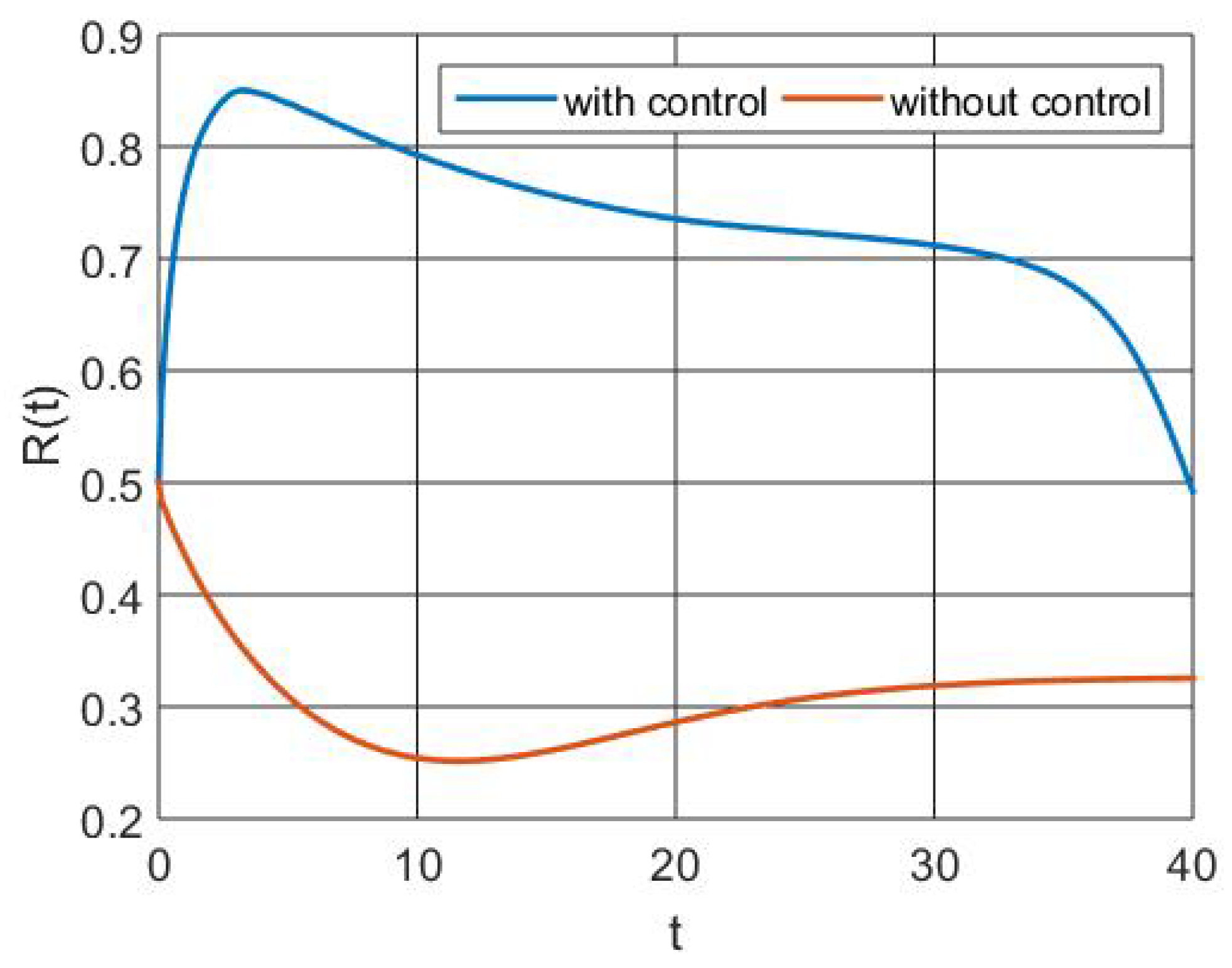
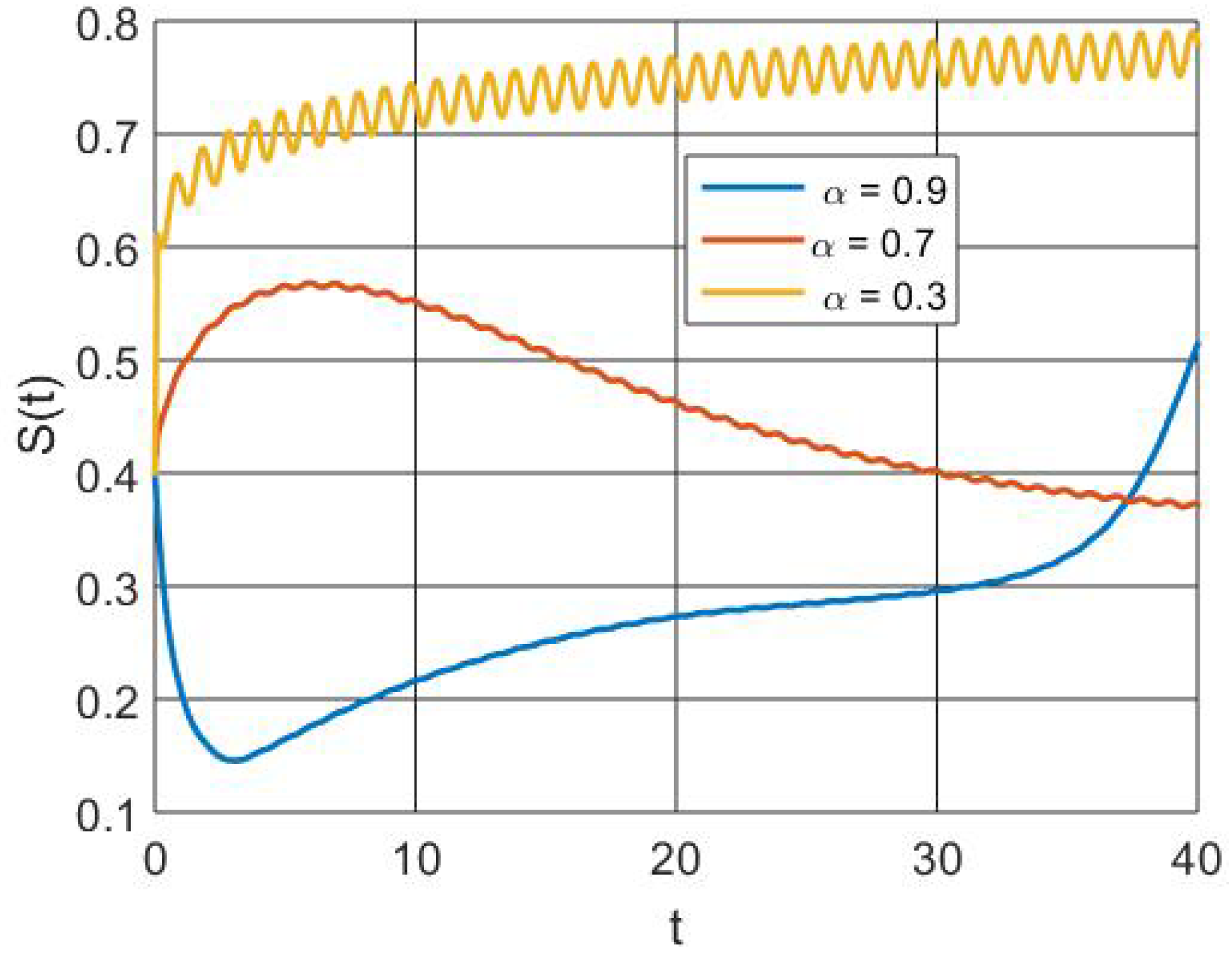
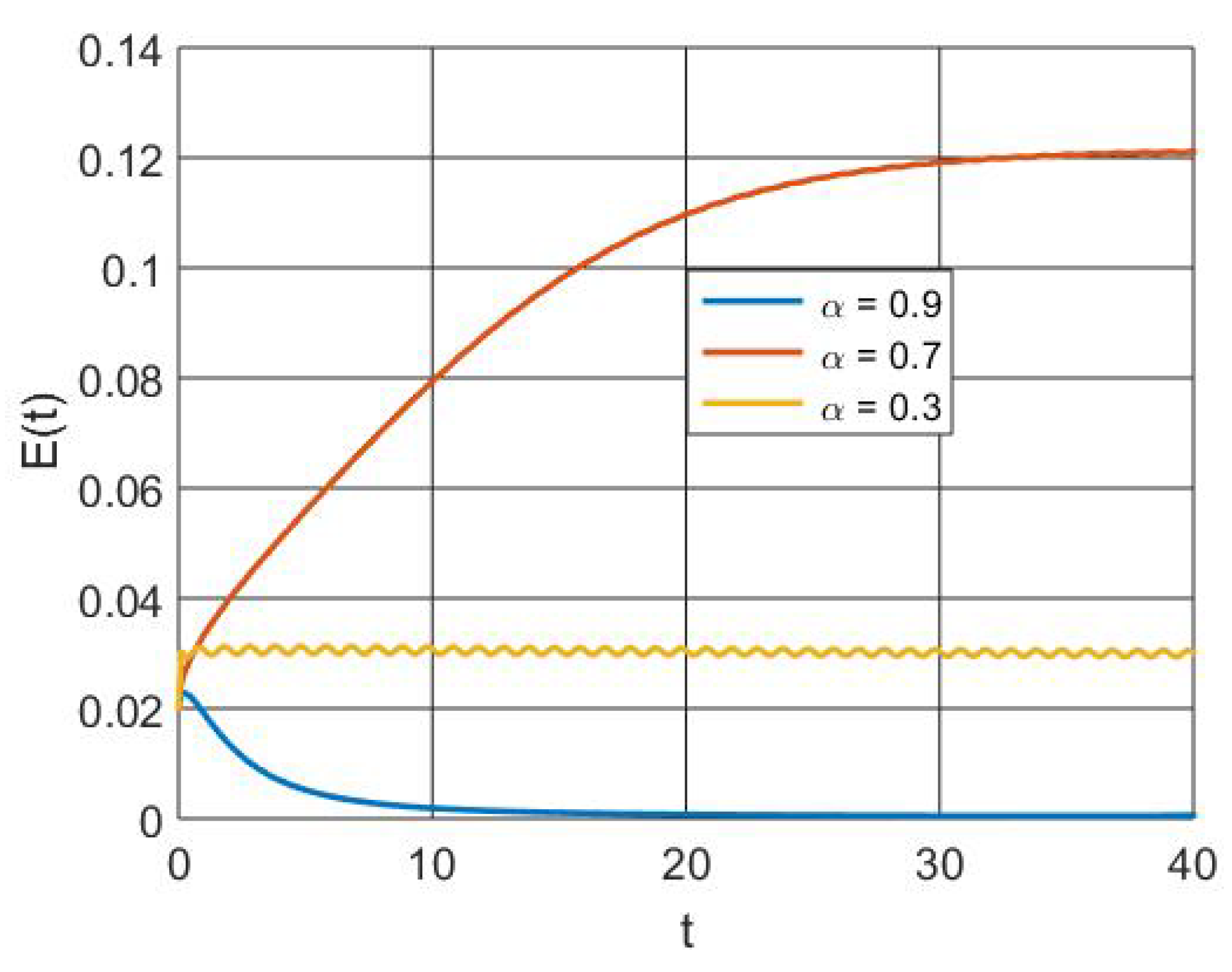
6. Numerical Simulation
7. Results and Discussion
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Lang, J.C. Use of mathematical modelling to assess respiratory syncytial virus epidemiology and interventions: A literature review. J. Math. Biol. 2022, 84, 26. [Google Scholar] [CrossRef]
- Beauchemin, C.A.; Kim, Y.I.; Yu, Q.; Ciaramella, G.; DeVincenzo, J.P. Uncovering critical properties of the human respiratory syncytial virus by combining in vitro assays and in silico analyses. PLoS ONE 2019, 14, e0214708. [Google Scholar] [CrossRef] [PubMed]
- Sungchasit, R.; Tang, I.M.; Pongsumpun, P. Mathematical Modeling: Global Stability Analysis of Super Spreading Transmission of Respiratory Syncytial Virus (RSV) Disease. Computation 2022, 10, 120. [Google Scholar] [CrossRef]
- Hodgson, D. Mathematical Modelling and Cost-Effectiveness of Future RSV Intervention Strategies. Ph.D. Thesis, UCL (University College London), London, UK, 2020. [Google Scholar]
- Mezei, A.; Cohen, J.; Renwick, M.J.; Atwell, J.; Portnoy, A. Mathematical modelling of respiratory syncytial virus (RSV) in low-and middle-income countries: A systematic review. Epidemics 2021, 35, 100444. [Google Scholar] [CrossRef] [PubMed]
- Kombe, I.K.; Agoti, C.N.; Munywoki, P.K.; Baguelin, M.; Nokes, D.J.; Medley, G.F. Integrating epidemiological and genetic data with different sampling intensities into a dynamic model of respiratory syncytial virus transmission. Sci. Rep. 2021, 11, 1463. [Google Scholar] [CrossRef] [PubMed]
- Kaslow, R.A.; Bell, D.M. Epidemiology and Control: From Principles to Pandemics. In Viral Infections of Humans: Epidemiology and Control; Springer: Berlin/Heidelberg, Germany, 2022; pp. 1–80. [Google Scholar]
- Pitzer, V.E.; Viboud, C.; Alonso, W.J.; Wilcox, T.; Metcalf, C.J.; Steiner, C.A.; Haynes, A.K.; Grenfell, B.T. Environmental drivers of the spatiotemporal dynamics of respiratory syncytial virus in the United States. PLoS Pathog. 2015, 11, e1004591. [Google Scholar] [CrossRef]
- Boukhouima, A.; Lotfi, E.M.; Mahrouf, M.; Rosa, S.; Torres, D.F.; Yousfi, N. Stability analysis and optimal control of a fractional HIV-AIDS epidemic model with memory and general incidence rate. Eur. Phys. J. Plus 2021, 136, 1–20. [Google Scholar] [CrossRef]
- Luo, D. Bifurcation Theory and Methods of Dynamical Systems; World Scientific: Singapore, 1997; Volume 15. [Google Scholar]
- Castillo-Chavez, C.; Song, B. Dynamical models of tuberculosis and their applications. Math. Biosci. Eng. 2004, 1, 361–404. [Google Scholar] [CrossRef]
- Huang, C.; Wang, J.; Chen, X.; Cao, J. Bifurcations in a fractional-order BAM neural network with four different delays. Neural Netw. 2021, 141, 344–354. [Google Scholar] [CrossRef]
- Hou, H.S.; Zhang, H. Stability and hopf bifurcation of fractional complex–valued BAM neural networks with multiple time delays. Appl. Math. Comput. 2023, 450, 127986. [Google Scholar] [CrossRef]
- Saha, S.; Samanta, G.; Nieto, J.J. Epidemic model of COVID-19 outbreak by inducing behavioural response in population. Nonlinear Dyn. 2020, 102, 455–487. [Google Scholar] [CrossRef] [PubMed]
- Saha, S.; Samanta, G.; Nieto, J.J. Impact of optimal vaccination and social distancing on COVID-19 pandemic. Math. Comput. Simul. 2022, 200, 285–314. [Google Scholar] [CrossRef] [PubMed]
- Mua, D.; Xub, C.; Liua, Z.; Panga, Y. Further Insight Into Bifurcation and Hybrid Control Tactics of a Chlorine Dioxide–Iodine–Malonic Acid Chemical Reaction Model Incorporating Delays. MATCH Commun. Math. Comput. Chem. 2023, 89, 529–566. [Google Scholar] [CrossRef]
- Giordano, G.; Blanchini, F.; Bruno, R.; Colaneri, P.; Di Filippo, A.; Di Matteo, A.; Colaneri, M. Modelling the COVID-19 epidemic and implementation of population-wide interventions in Italy. Nat. Med. 2020, 26, 855–860. [Google Scholar] [CrossRef] [PubMed]
- Boccaletti, S.; Mindlin, G.; Ditto, W.; Atangana, A. Closing editorial: Forecasting of epidemic spreading: Lessons learned from the current covid-19 pandemic. Chaos Solitons Fractals 2020, 139, 110278. [Google Scholar] [CrossRef]
- Ndaïrou, F.; Area, I.; Nieto, J.J.; Torres, D.F.M. Mathematical modeling of COVID-19 transmission dynamics with a case study of Wuhan. Chaos Solitons Fractals 2020, 135, 109846. [Google Scholar] [CrossRef]
- Lima, L.S. Dynamics based on analysis of public data for spreading of disease. Sci. Rep. 2021, 11, 12177. [Google Scholar] [CrossRef]
- Kar, T.; Nandi, S.K.; Jana, S.; Mandal, M. Stability and bifurcation analysis of an epidemic model with the effect of media. Chaos Solitons Fractals 2019, 120, 188–199. [Google Scholar] [CrossRef]
- Qureshi, S.; Yusuf, A. Fractional derivatives applied to MSEIR problems: Comparative study with real world data. Eur. Phys. J. Plus 2019, 134, 171. [Google Scholar] [CrossRef]
- Lima, L.d.S. Fractional stochastic differential equation approach for spreading of diseases. Entropy 2022, 24, 719. [Google Scholar] [CrossRef]
- Al-Hoceima, E. 1ére Journée sur l’Intelligence Artificielle & Mathématiques Appliquées 04 Mai 2023 ENSA Al-Hoceima. Available online: https://www.researchgate.net/profile/Younes-Abouelhanoune/publication/370659883_JIAMA'23_Book_Abstracts/links/6470cc8d6a3c4c6efbe1f2ce/JIAMA23-Book-Abstracts.pdf (accessed on 17 November 2023).
- Soulaimani, S.; Kaddar, A. Analysis and Optimal Control of a Fractional Order SEIR Epidemic Model with General Incidence and Vaccination. IEEE Access 2023, 11, 81995–82002. [Google Scholar] [CrossRef]
- Padder, A.; Almutairi, L.; Qureshi, S.; Soomro, A.; Afroz, A.; Hincal, E.; Tassaddiq, A. Dynamical Analysis of Generalized Tumor Model with Caputo Fractional-Order Derivative. Fractal Fract. 2023, 7, 258. [Google Scholar] [CrossRef]
- Nisar, K.S.; Farman, M.; Abdel-Aty, M.; Cao, J. A review on epidemic models in sight of fractional calculus. Alex. Eng. J. 2023, 75, 81–113. [Google Scholar] [CrossRef]
- Qureshi, S.; Jan, R. Modeling of measles epidemic with optimized fractional order under Caputo differential operator. Chaos Solitons Fractals 2021, 145, 110766. [Google Scholar] [CrossRef]
- Boukhouima, A.; Hattaf, K.; Lotfi, E.M.; Mahrouf, M.; Torres, D.F.; Yousfi, N. Lyapunov functions for fractional-order systems in biology: Methods and applications. Chaos Solitons Fractals 2020, 140, 110224. [Google Scholar] [CrossRef]
- Sidi Ammi, M.R.; Tahiri, M.; Tilioua, M.; Zeb, A.; Khan, I.; Andualem, M. Global analysis of a time fractional order spatio-temporal SIR model. Sci. Rep. 2022, 12, 5751. [Google Scholar] [CrossRef]
- Ahmad, B.; Nieto, J.J.; Alsaedi, A.; El-Shahed, M. A study of nonlinear Langevin equation involving two fractional orders in different intervals. Nonlinear Anal. Real World Appl. 2012, 13, 599–606. [Google Scholar] [CrossRef]
- Jajarmi, A.; Baleanu, D.; Sajjadi, S.S.; Nieto, J.J. Analysis and some applications of a regularized ψ–Hilfer fractional derivative. J. Comput. Appl. Math. 2022, 415, 114476. [Google Scholar] [CrossRef]
- Rosa, S.; Torres, D.F. Optimal control of a fractional order epidemic model with application to human respiratory syncytial virus infection. Chaos Solitons Fractals 2018, 117, 142–149. [Google Scholar] [CrossRef]
- Rosa, S.; Torres, D.F. Numerical Fractional Optimal Control of Respiratory Syncytial Virus Infection in Octave/MATLAB. Mathematics 2023, 11, 1511. [Google Scholar] [CrossRef]
- Ahmad, H.; Khan, M.; Ahmad, I.; Omri, M.; Alotaibi, M. A meshless method for numerical solutions of linear and nonlinear time-fractional Black–Scholes models. AIMS Math. 2023, 8, 19677–19698. [Google Scholar] [CrossRef]
- Alquran, M.; Sulaiman, T.; Yusuf, A.; Alshomrani, A.; Baleanu, D. Nonautonomous lump-periodic and analytical solutions to the (3+1)-dimensional generalized Kadomtsev–Petviashvili equation. Nonlinear Dyn. 2023, 111, 11429–11436. [Google Scholar] [CrossRef]
- Latif, S.; Sabir, Z.; Raja, M.; Altamirano, G.; Núñez, R.; Gago, D.; Sadat, R.; Ali, M. IoT technology enabled stochastic computing paradigm for numerical simulation of heterogeneous mosquito model. Multimed. Tools Appl. 2023, 82, 18851–18866. [Google Scholar] [CrossRef]
- Qayyum, M.; Ahmad, E.; Tauseef Saeed, S.; Ahmad, H.; Askar, S. Homotopy perturbation method-based soliton solutions of the time-fractional (2+1)-dimensional Wu–Zhang system describing long dispersive gravity water waves in the ocean. Front. Phys. 2023, 11, 1178154. [Google Scholar] [CrossRef]
- Sabir, Z.; Guirao, J. A soft computing scaled conjugate gradient procedure for the fractional order Majnun and Layla romantic story. Mathematics 2023, 11, 835. [Google Scholar] [CrossRef]
- Souayeh, B.; Sabir, Z. Designing Hyperbolic Tangent Sigmoid Function for Solving the Williamson Nanofluid Model. Fractal Fract. 2023, 7, 350. [Google Scholar] [CrossRef]
- Zarin, R.; Khan, M.; Khan, A.; Yusuf, A. Deterministic and fractional analysis of a newly developed dengue epidemic model. Waves Random Complex Media 2023. [Google Scholar] [CrossRef]
- Zil-E-Huma; Butt, A.; Raza, N.; Ahmad, H.; Ozsahin, D.; Tchier, F. Different solitary wave solutions and bilinear form for modified mixed-KDV equation. Optik 2023, 287, 171031. [Google Scholar] [CrossRef]
- Hashemi, M.; Mirzazadeh, M.; Ahmad, H. A reduction technique to solve the (2 + 1)-dimensional KdV equations with time local fractional derivatives. Opt. Quantum Electron. 2023, 55, 721. [Google Scholar] [CrossRef]
- Sulaiman, T.A.; Yusuf, A.; Alshomrani, A.S.; Baleanu, D. Wave solutions to the more general (2 + 1)-dimensional Boussinesq equation arising in ocean engineering. Int. J. Mod. Phys. B 2023, 2350214. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Academic Press: San Diego, CA, USA, 1998. [Google Scholar]
- Yaro, D.; Apeanti, W.O.; Akuamoah, S.W.; Lu, D. Analysis and optimal control of fractional-order transmission of a respiratory epidemic model. Int. J. Appl. Comput. Math. 2019, 5, 1–21. [Google Scholar] [CrossRef] [PubMed]
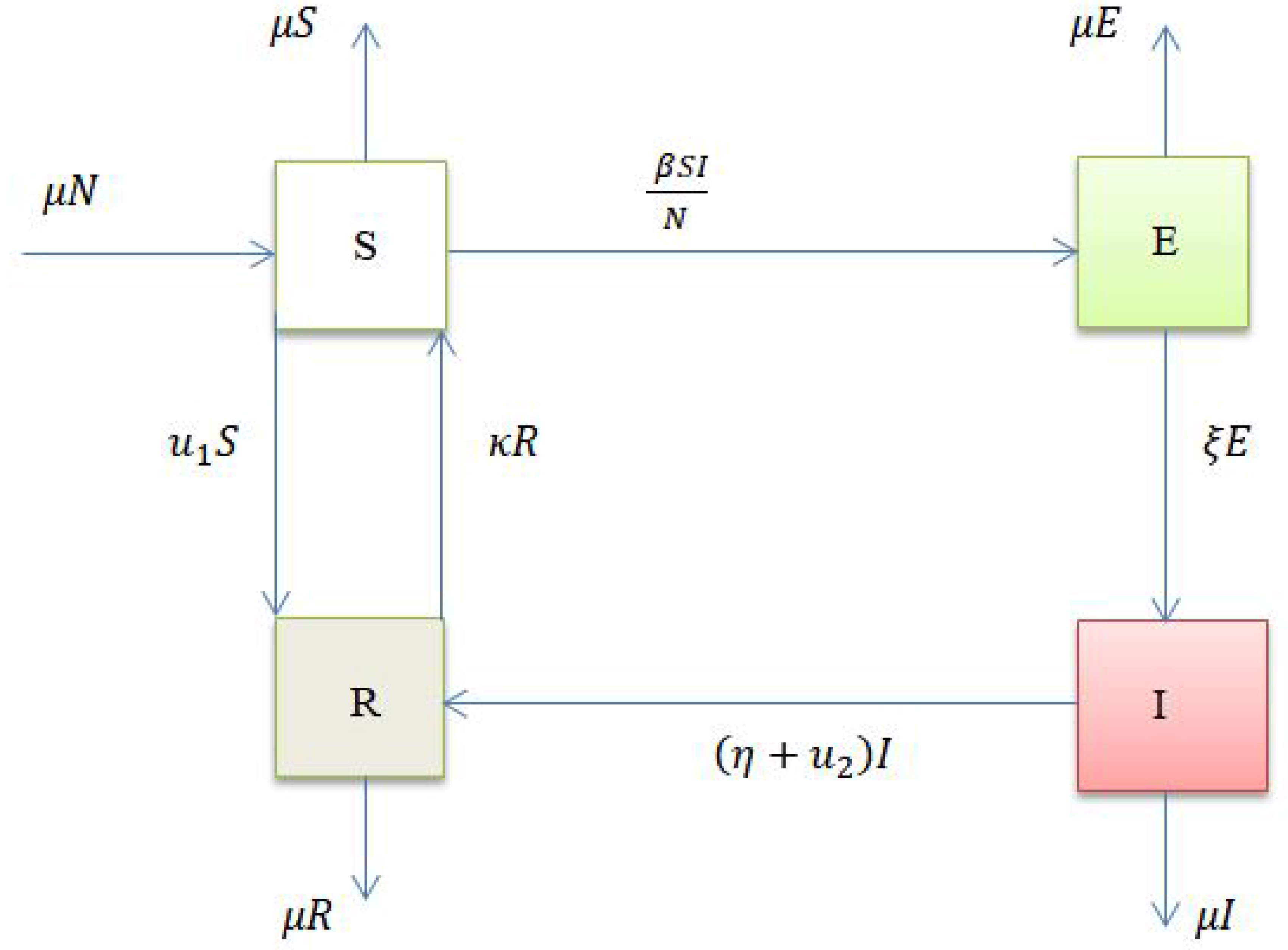





| Variable | Description |
|---|---|
| S(t) | Number of susceptible individuals at time t. |
| E(t) | Number of exposed individuals at time t. |
| I(t) | Number of infected individuals at time t. |
| R(t) | Number of recovered individuals at time t. |
| N(t) | Total population size at time t. |
| Parameter | Description |
|---|---|
| Naturally induced death rate. | |
| RSV transmission rate due to effective contact. | |
| Immunity loss rate. | |
| Progression rate from E to I. | |
| Recovery rate. | |
| Vaccination control function. | |
| Treatment control function. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Awadalla, M.; Alahmadi, J.; Cheneke, K.R.; Qureshi, S. Fractional Optimal Control Model and Bifurcation Analysis of Human Syncytial Respiratory Virus Transmission Dynamics. Fractal Fract. 2024, 8, 44. https://doi.org/10.3390/fractalfract8010044
Awadalla M, Alahmadi J, Cheneke KR, Qureshi S. Fractional Optimal Control Model and Bifurcation Analysis of Human Syncytial Respiratory Virus Transmission Dynamics. Fractal and Fractional. 2024; 8(1):44. https://doi.org/10.3390/fractalfract8010044
Chicago/Turabian StyleAwadalla, Muath, Jihan Alahmadi, Kumama Regassa Cheneke, and Sania Qureshi. 2024. "Fractional Optimal Control Model and Bifurcation Analysis of Human Syncytial Respiratory Virus Transmission Dynamics" Fractal and Fractional 8, no. 1: 44. https://doi.org/10.3390/fractalfract8010044
APA StyleAwadalla, M., Alahmadi, J., Cheneke, K. R., & Qureshi, S. (2024). Fractional Optimal Control Model and Bifurcation Analysis of Human Syncytial Respiratory Virus Transmission Dynamics. Fractal and Fractional, 8(1), 44. https://doi.org/10.3390/fractalfract8010044







