Backstepping Control with a Fractional-Order Command Filter and Disturbance Observer for Unmanned Surface Vehicles
Abstract
:1. Introduction
- (1)
- The fractional-order finite-time command filter is proposed, which is proved to be finite-time stable. It can track the derivatives of the intermediate control well without adding additional compensation signals, and the direct derivation issue is avoided when designing a controller.
- (2)
- A fractional-order finite-time disturbance observer is designed to compensate for unknown environmental disturbances and model uncertainty, which can improve the transient and steady-state performances of the USVs system.
- (3)
- The controller in the paper can ensure the globally asymptotical stability of the closed-loop system. Thus, good control performance can be achieved. In addition, using the fractional-order command filter and disturbance observer can reduce chattering caused by the finite-time differentiator, which facilitates the application in practice.
2. Preliminaries and Problem Formulation
2.1. Fractional Calculus
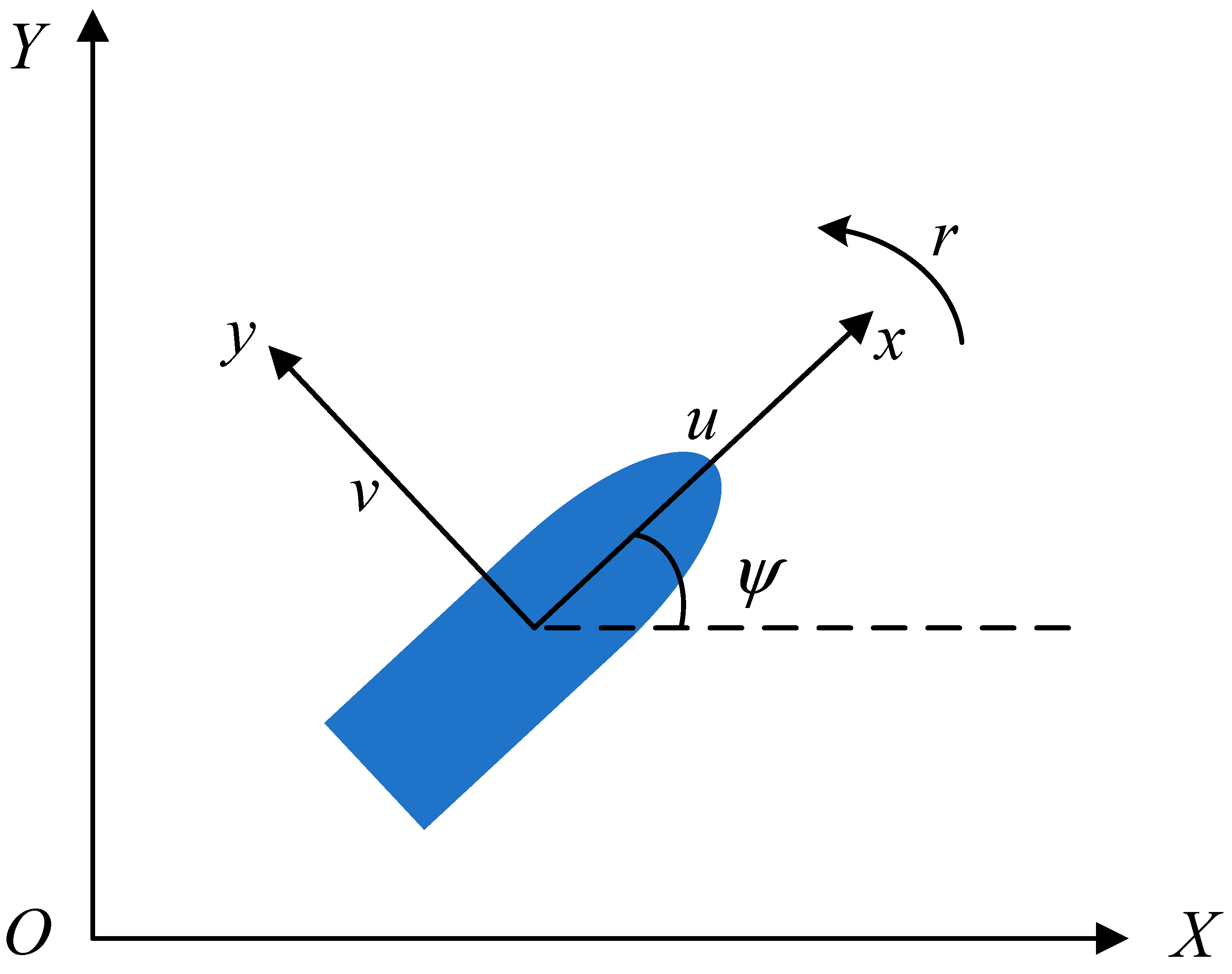
2.2. Problem Formulation
2.3. Control Objective
3. Results Analysis
3.1. Fractional-Order Finite-Time Command Filter
3.2. Fractional-Order Finite-Time Disturbance Observer
3.3. Controller Design
3.4. Stability Analysis
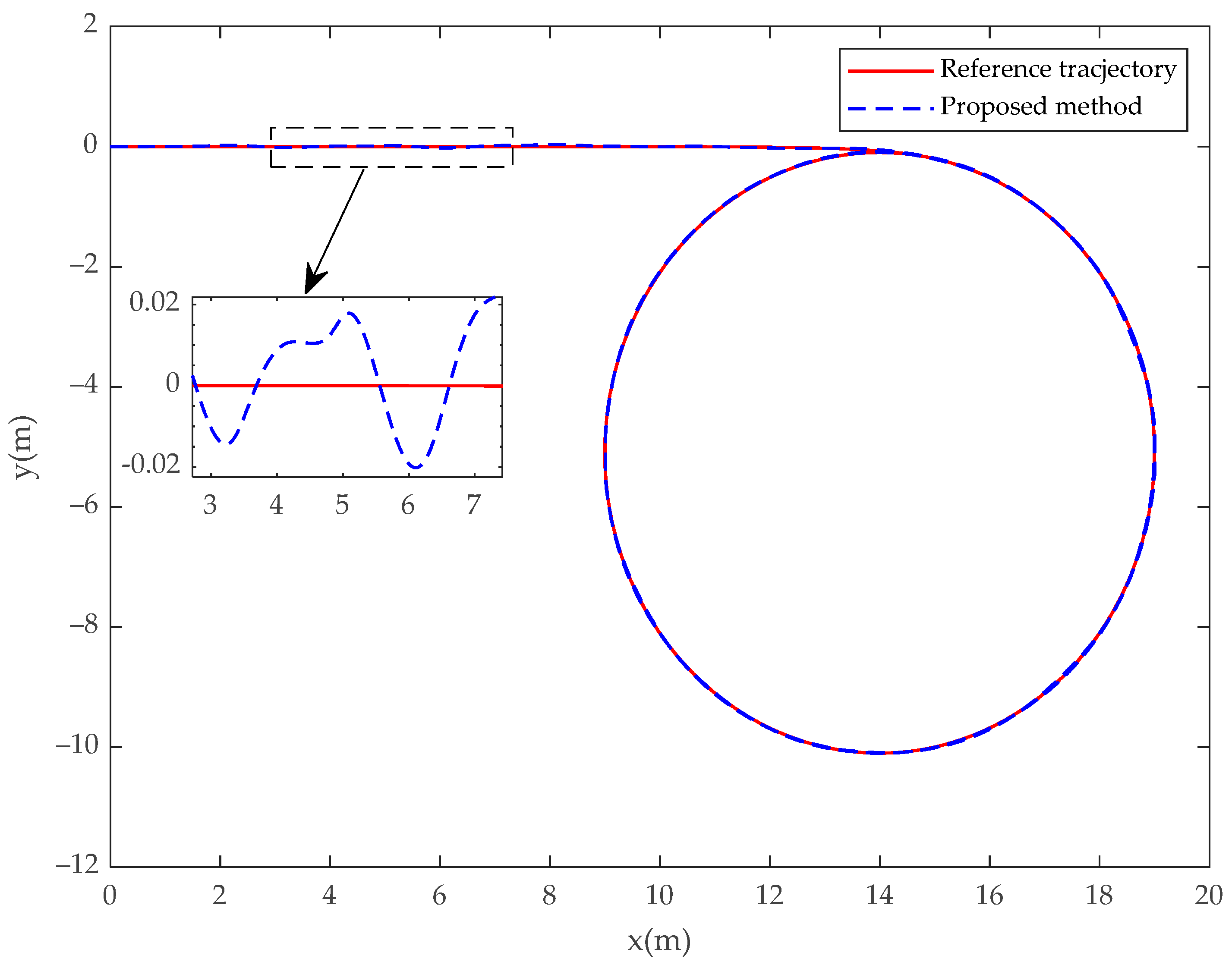
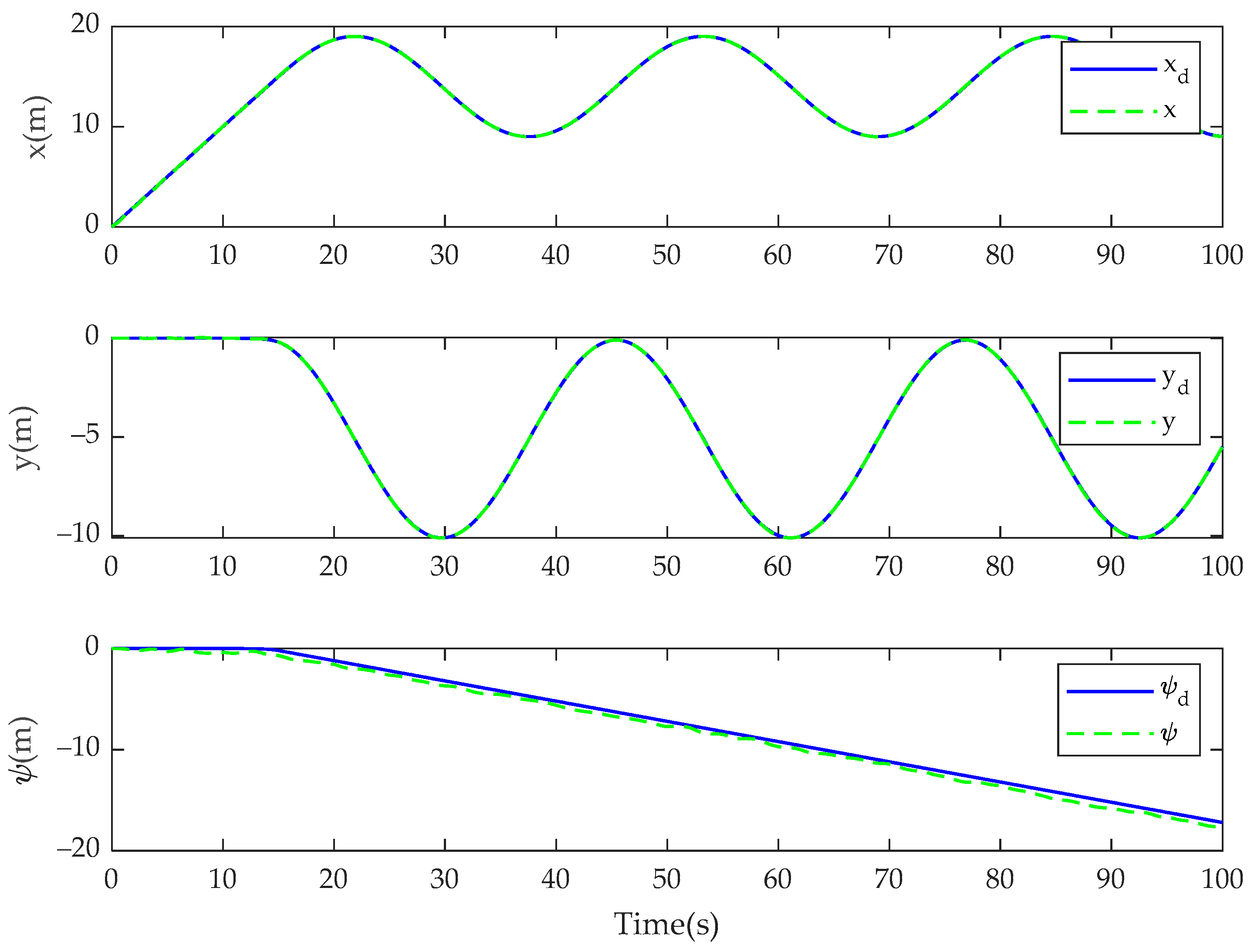
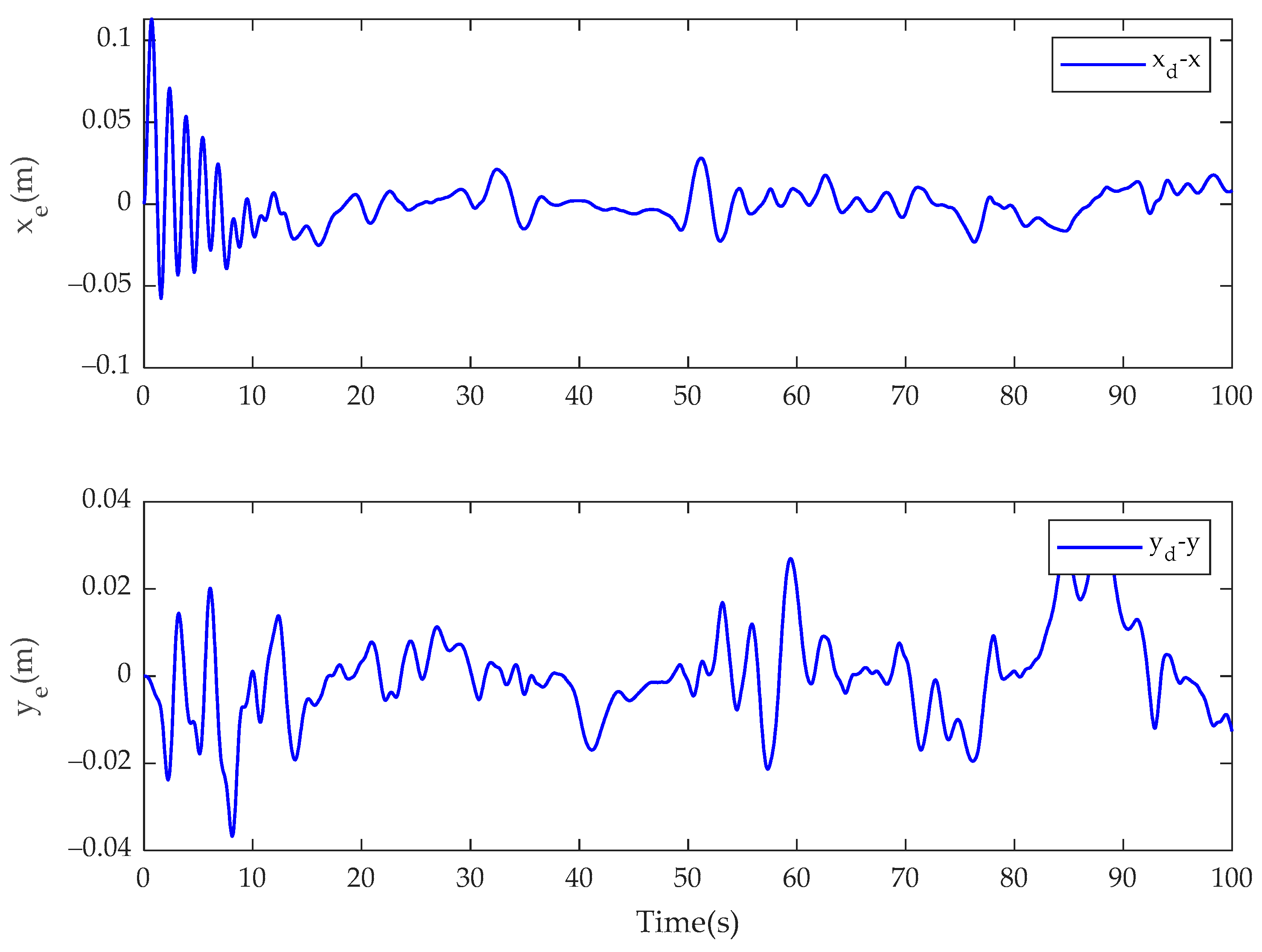
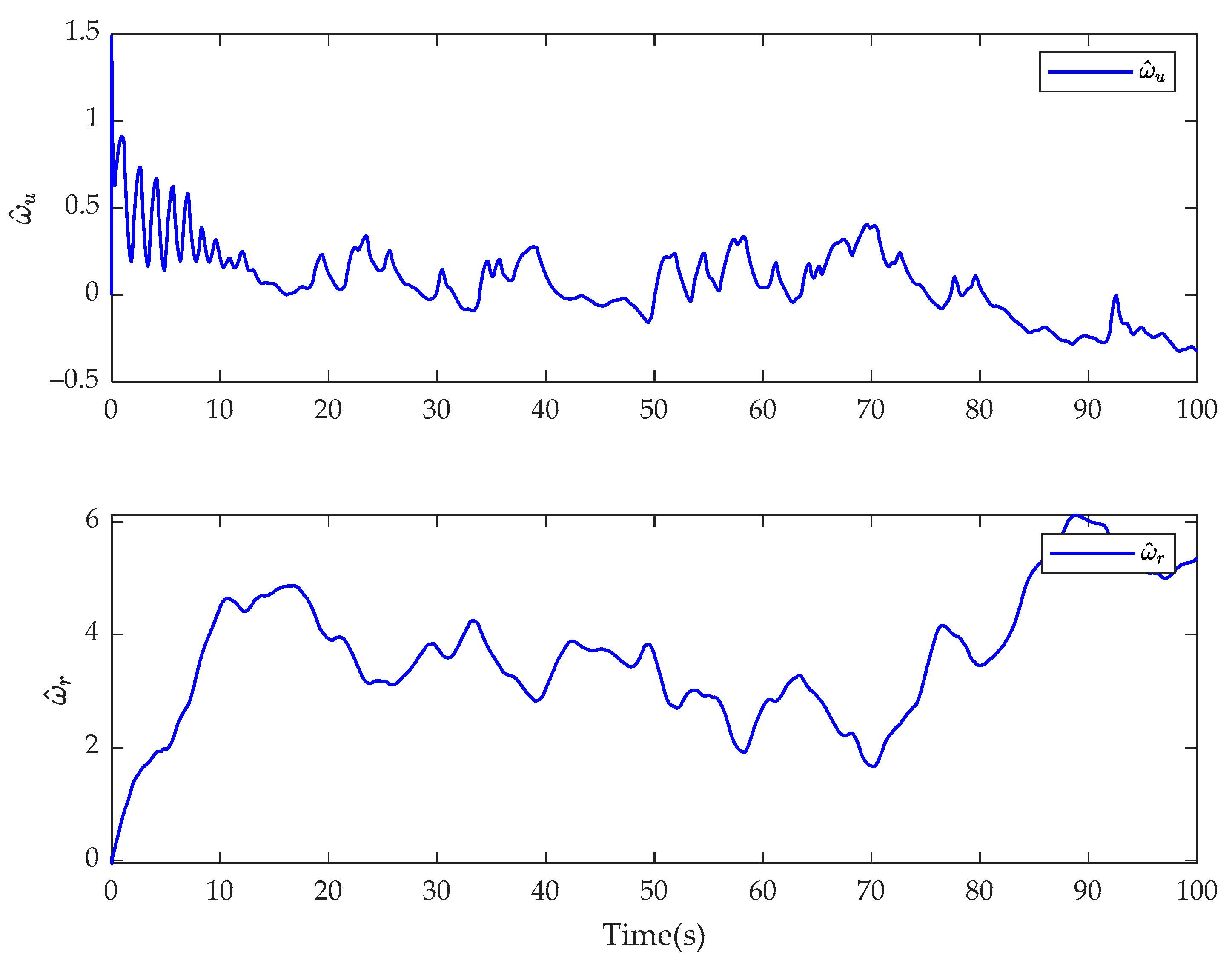
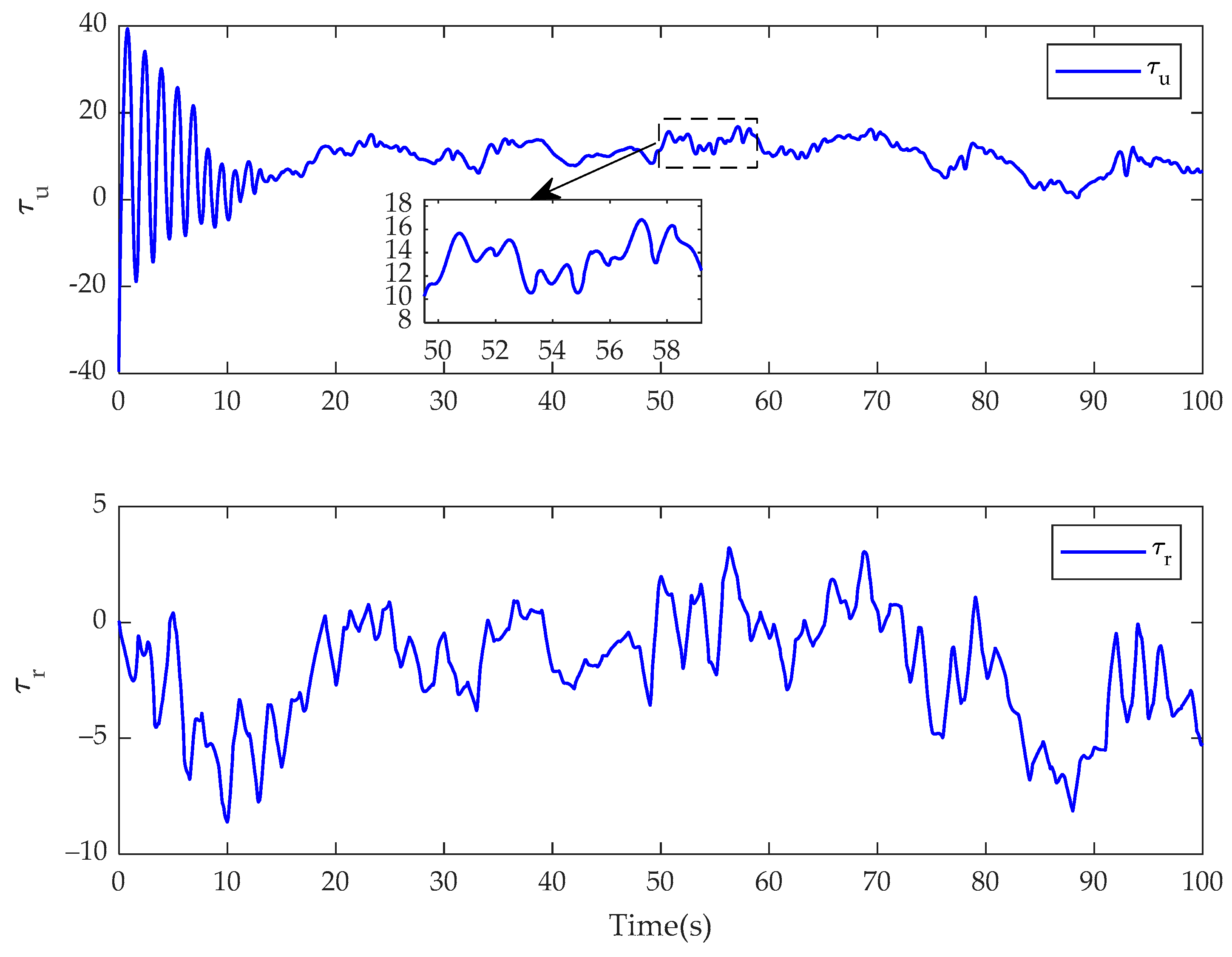
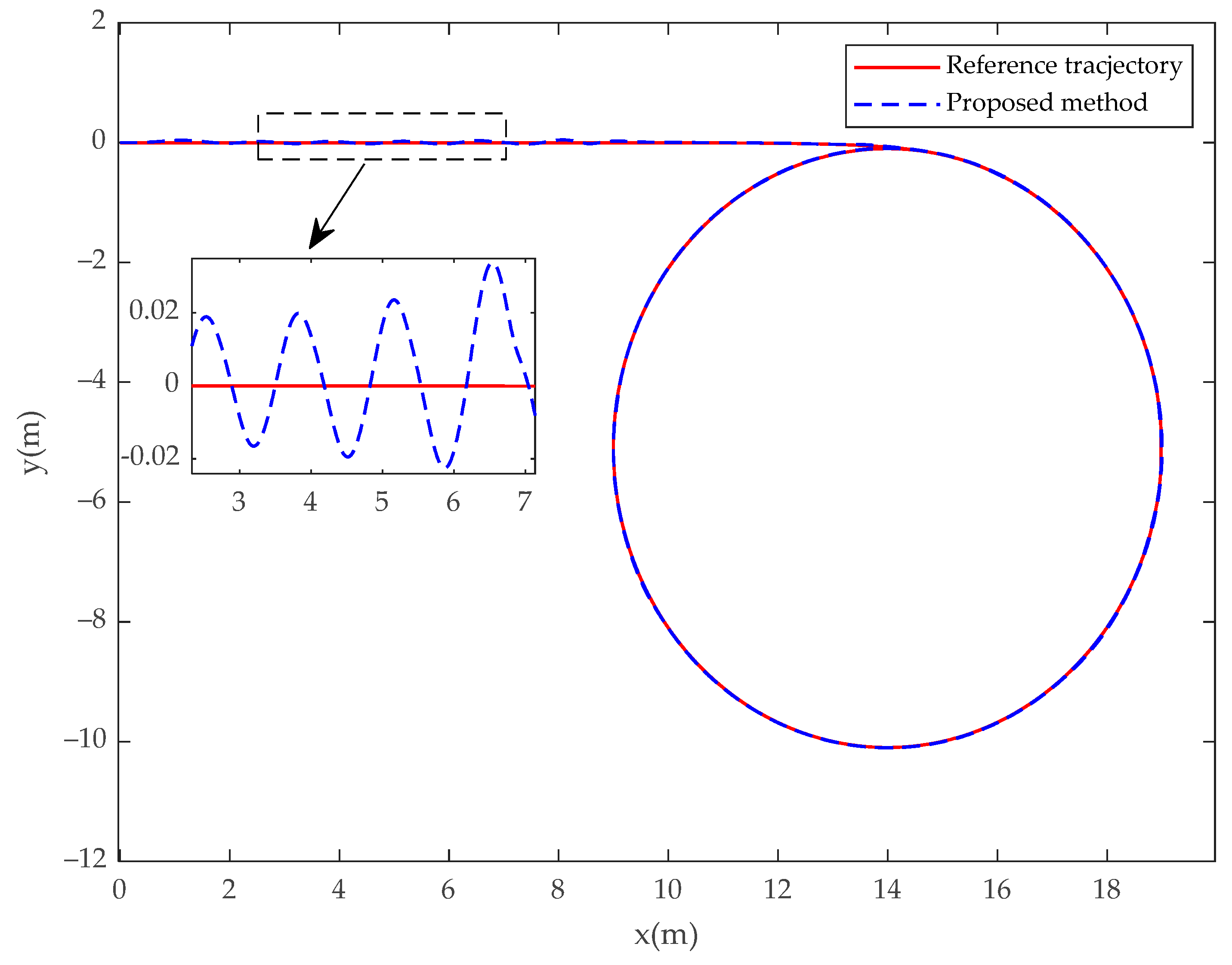
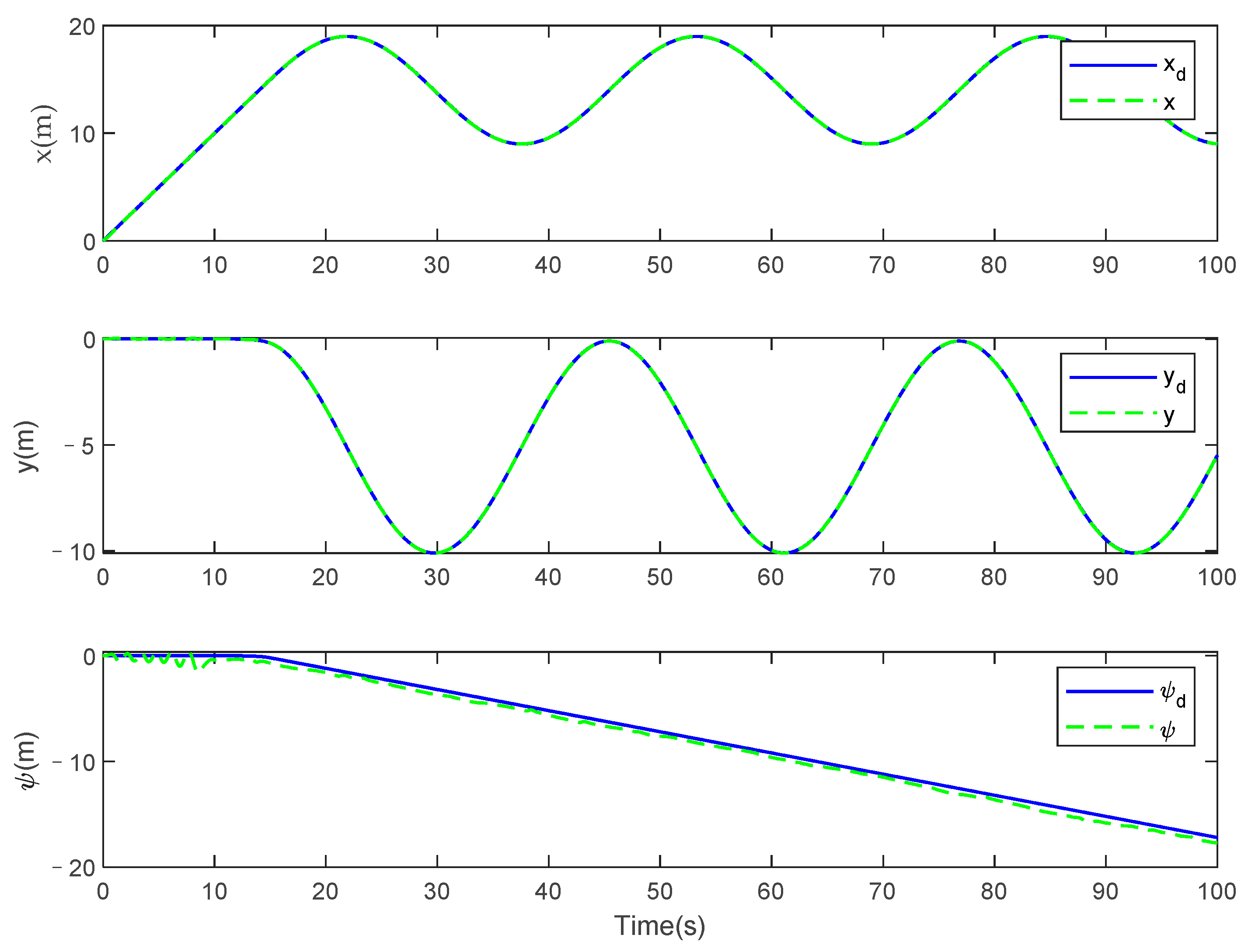
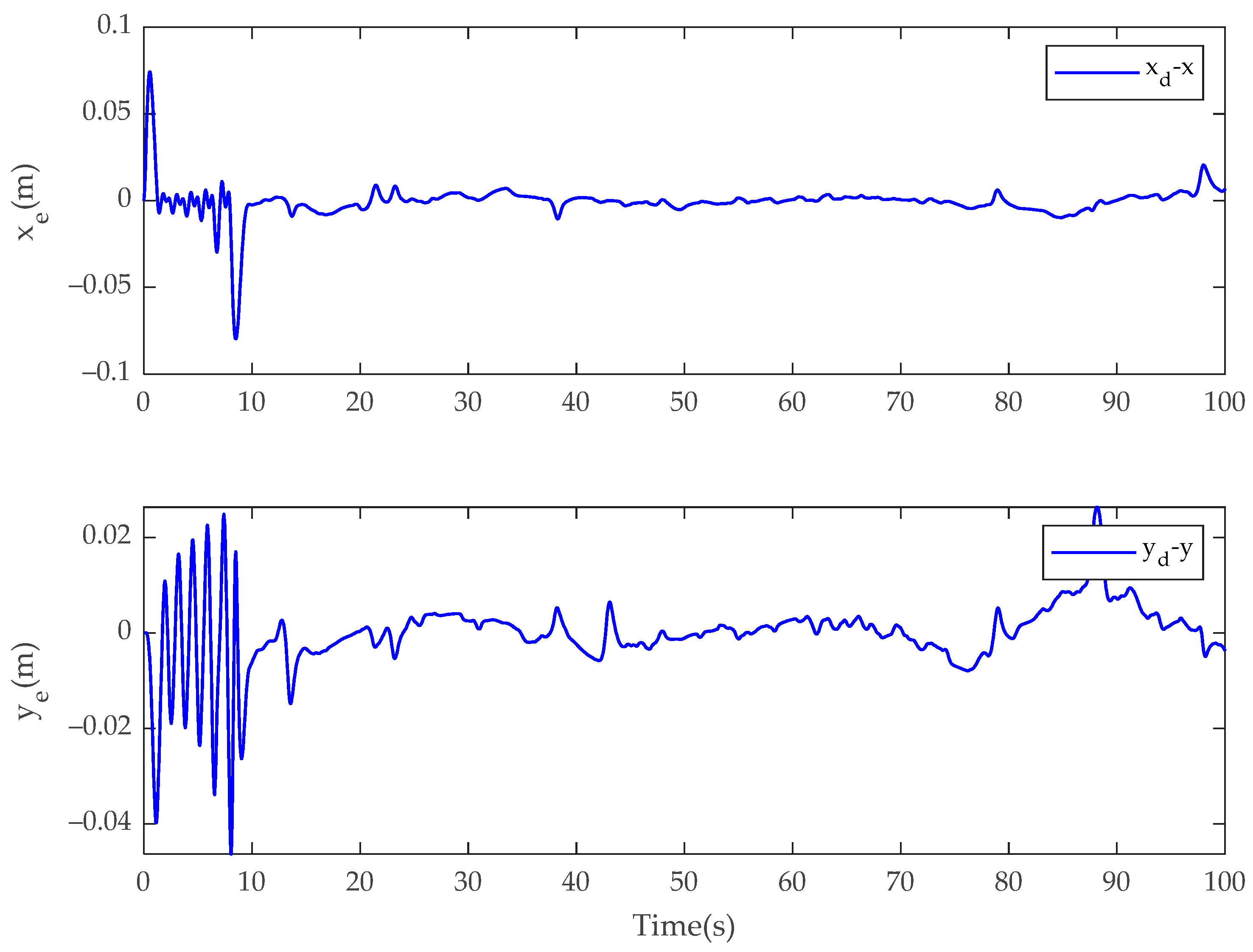
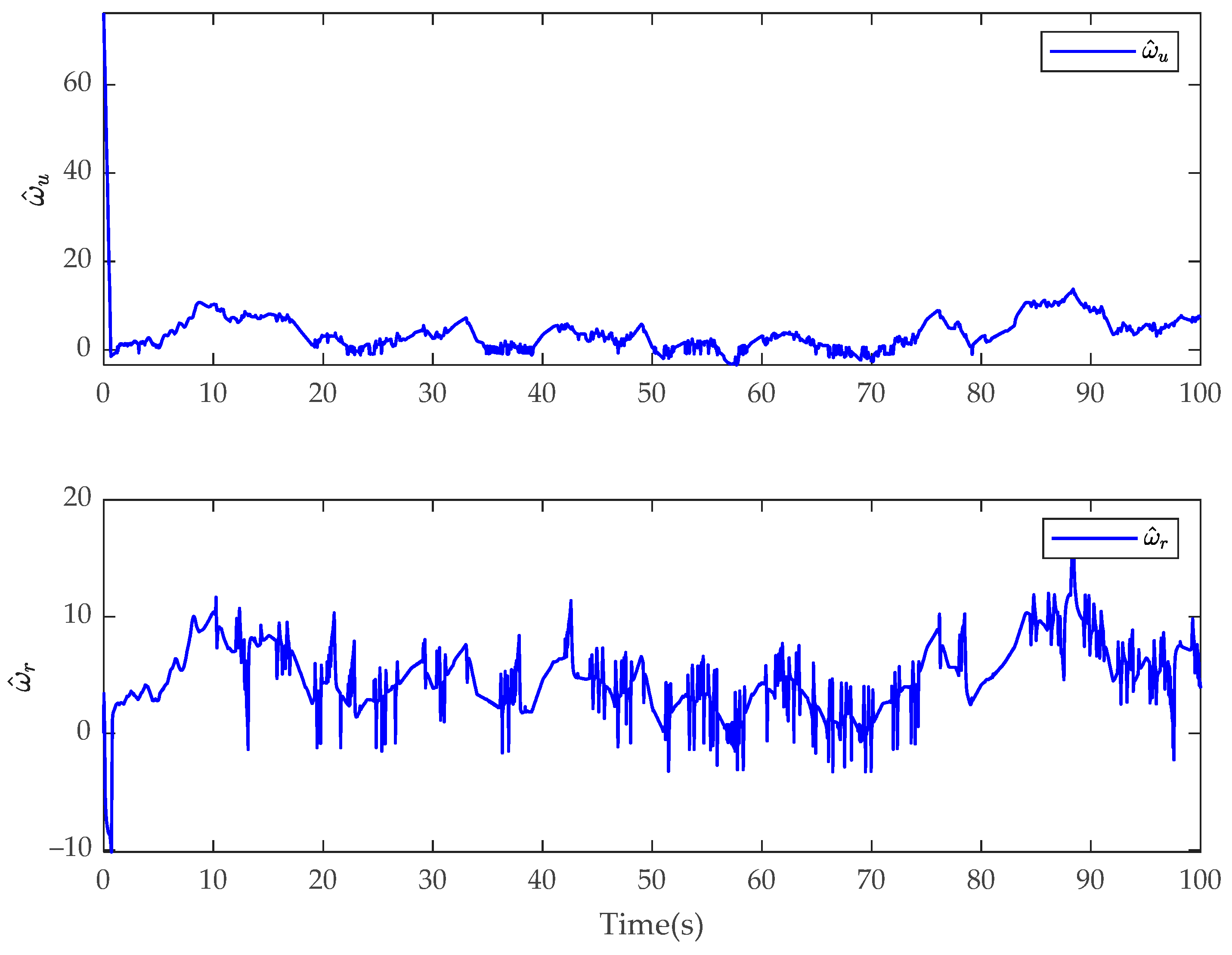
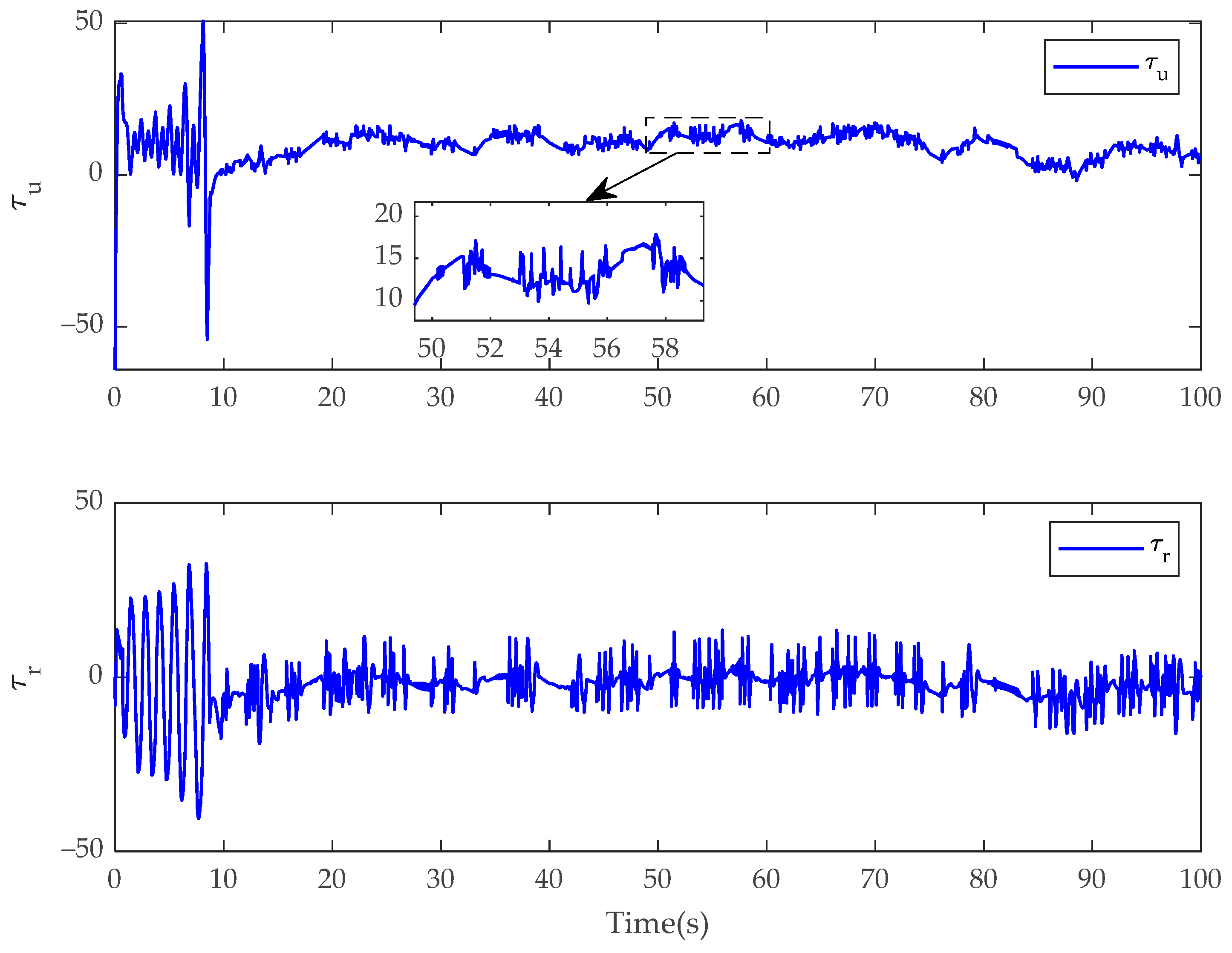
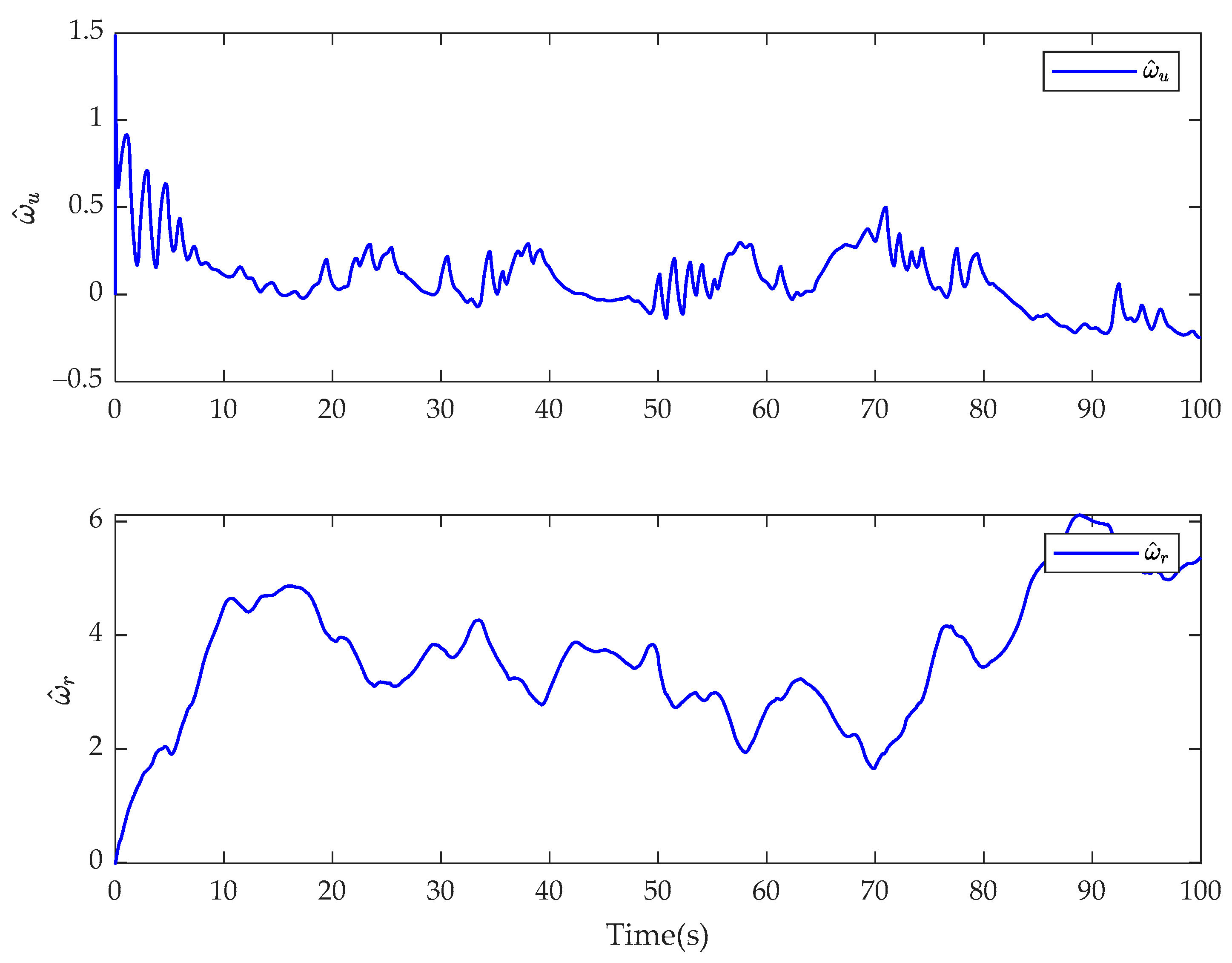
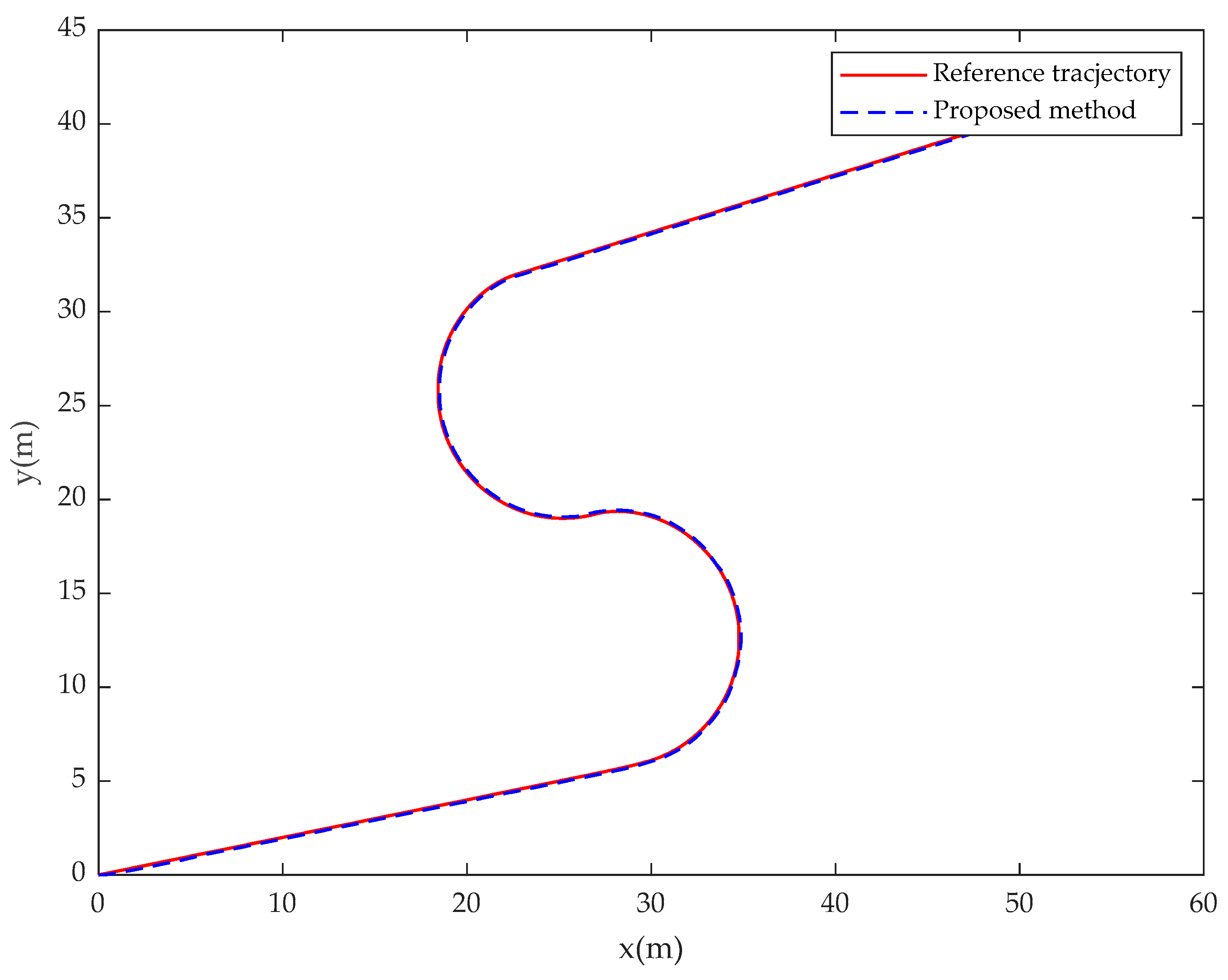
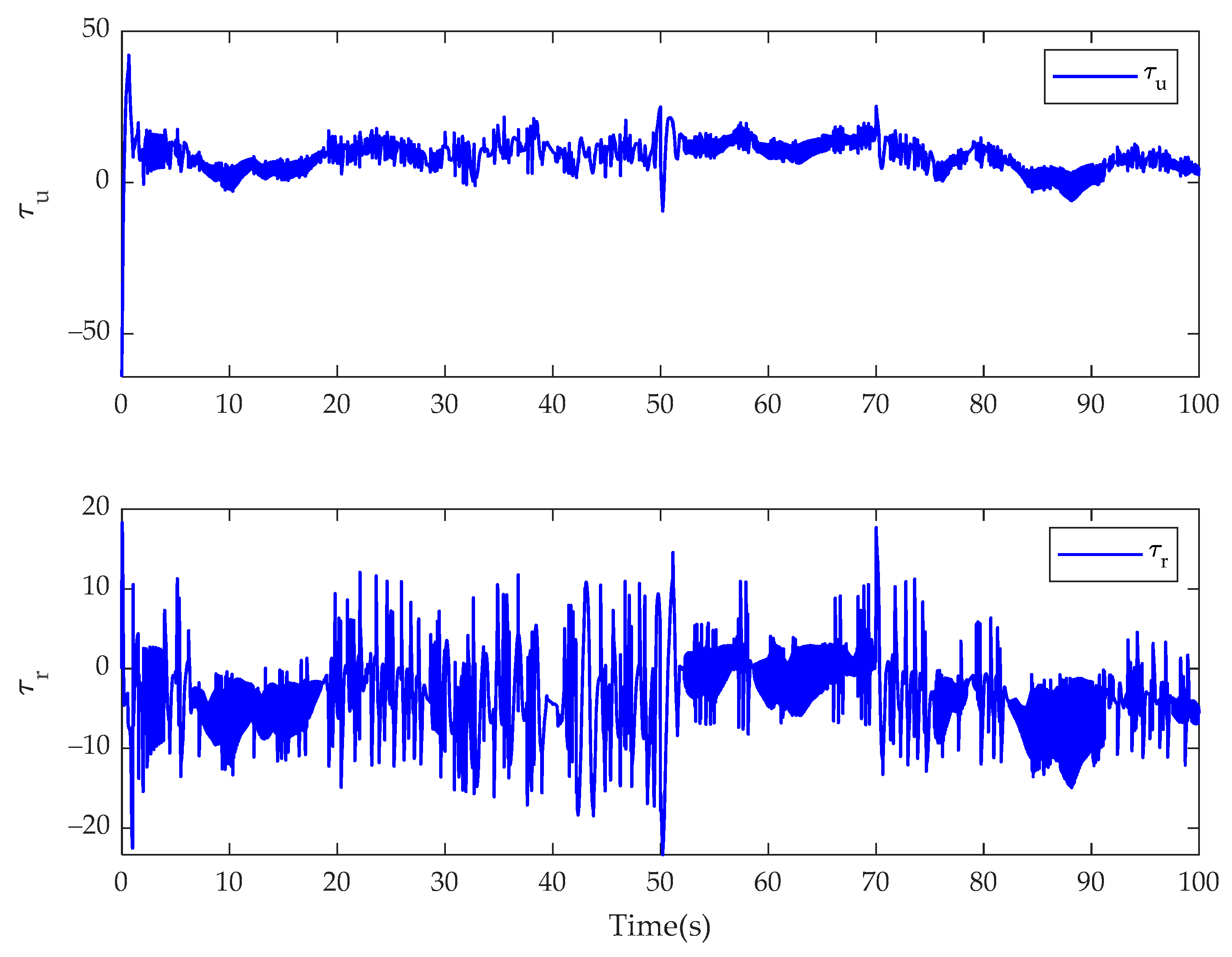
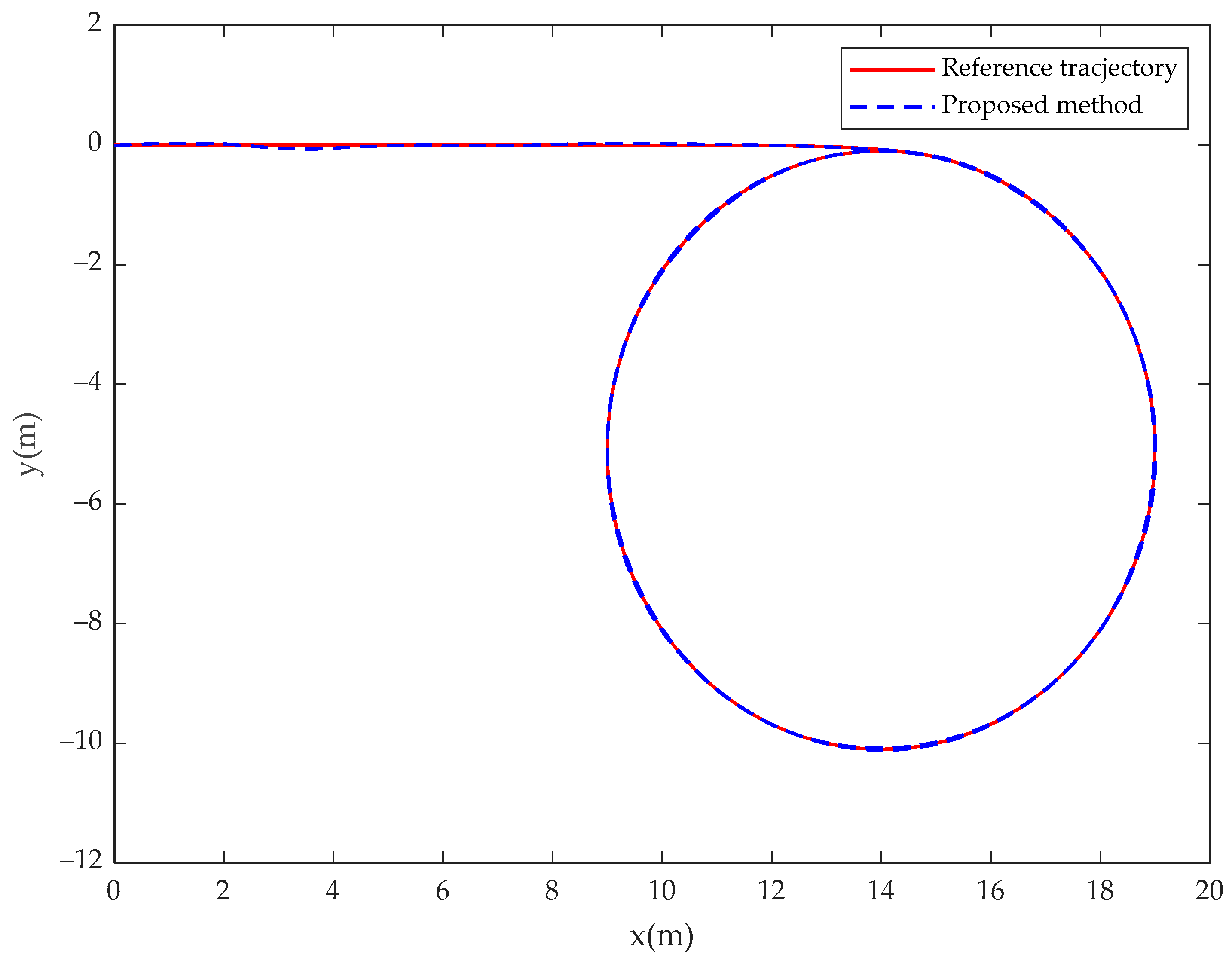
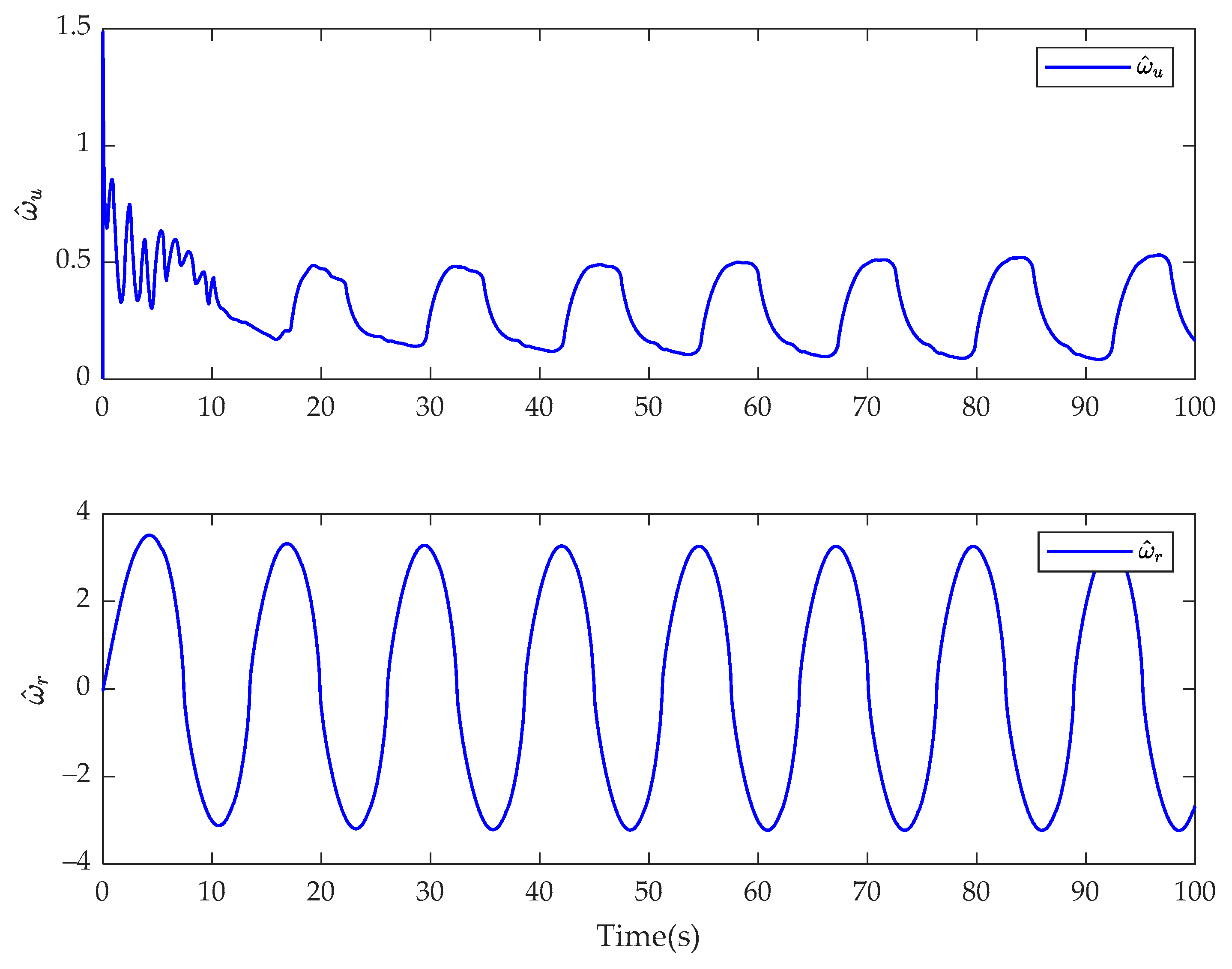
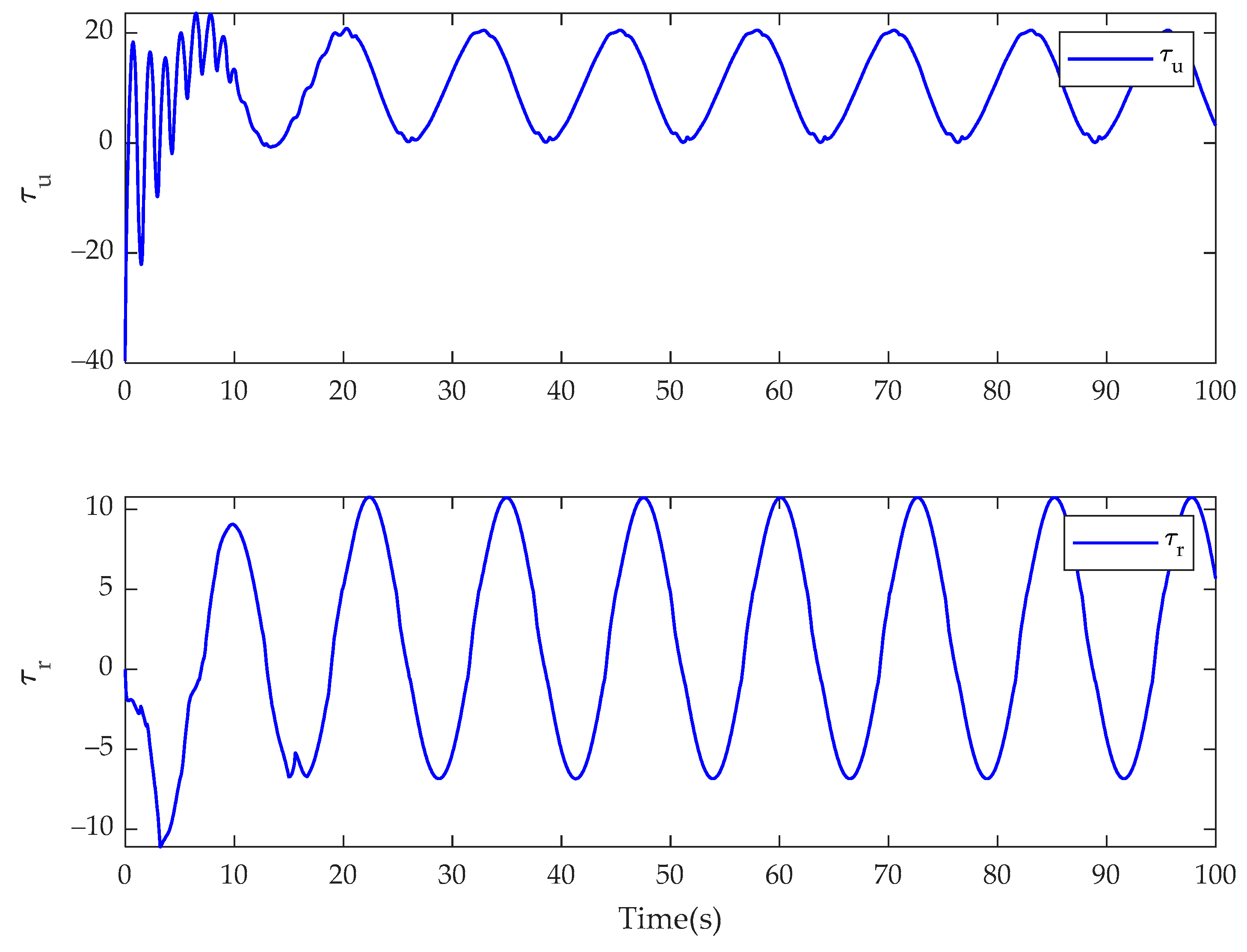
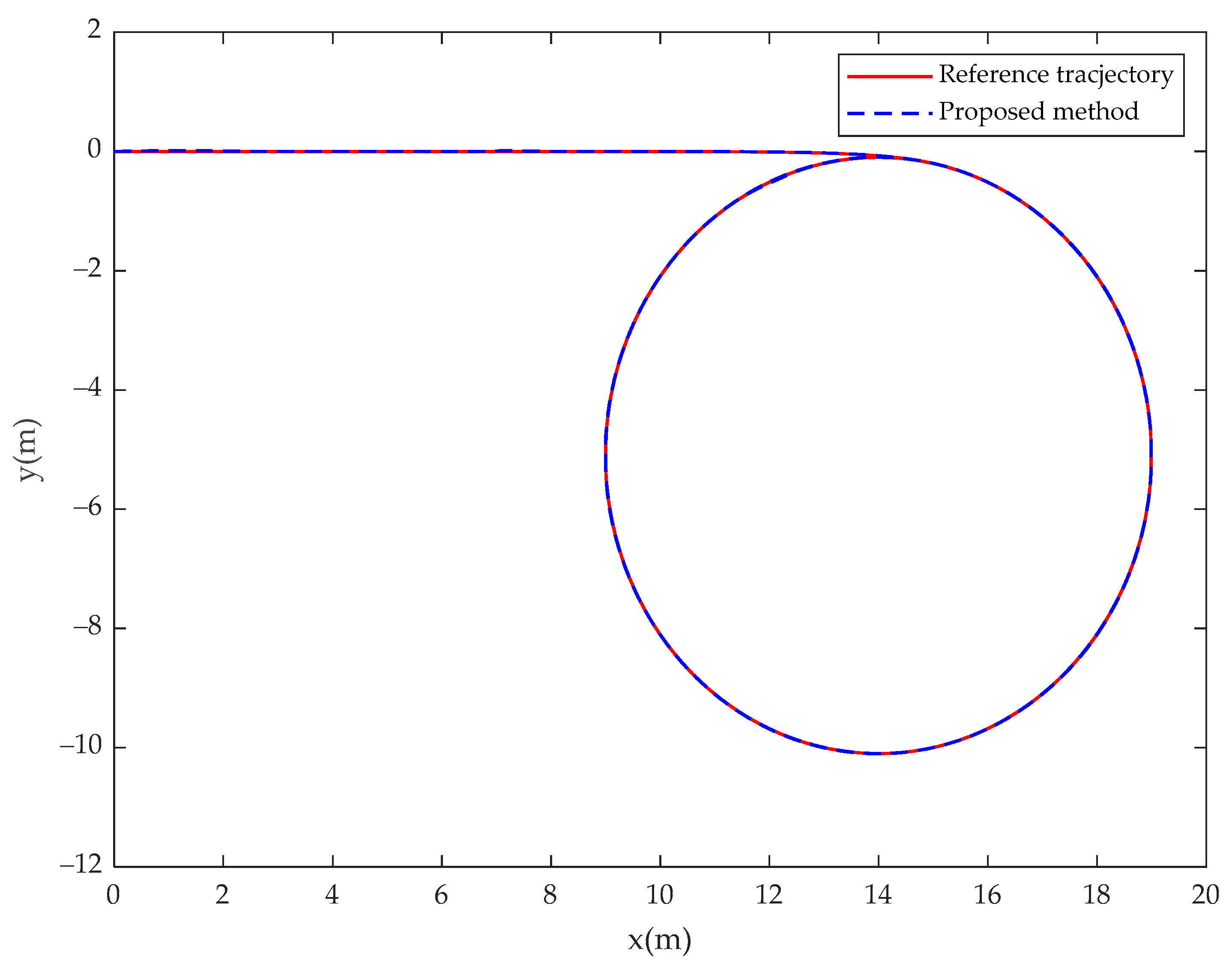
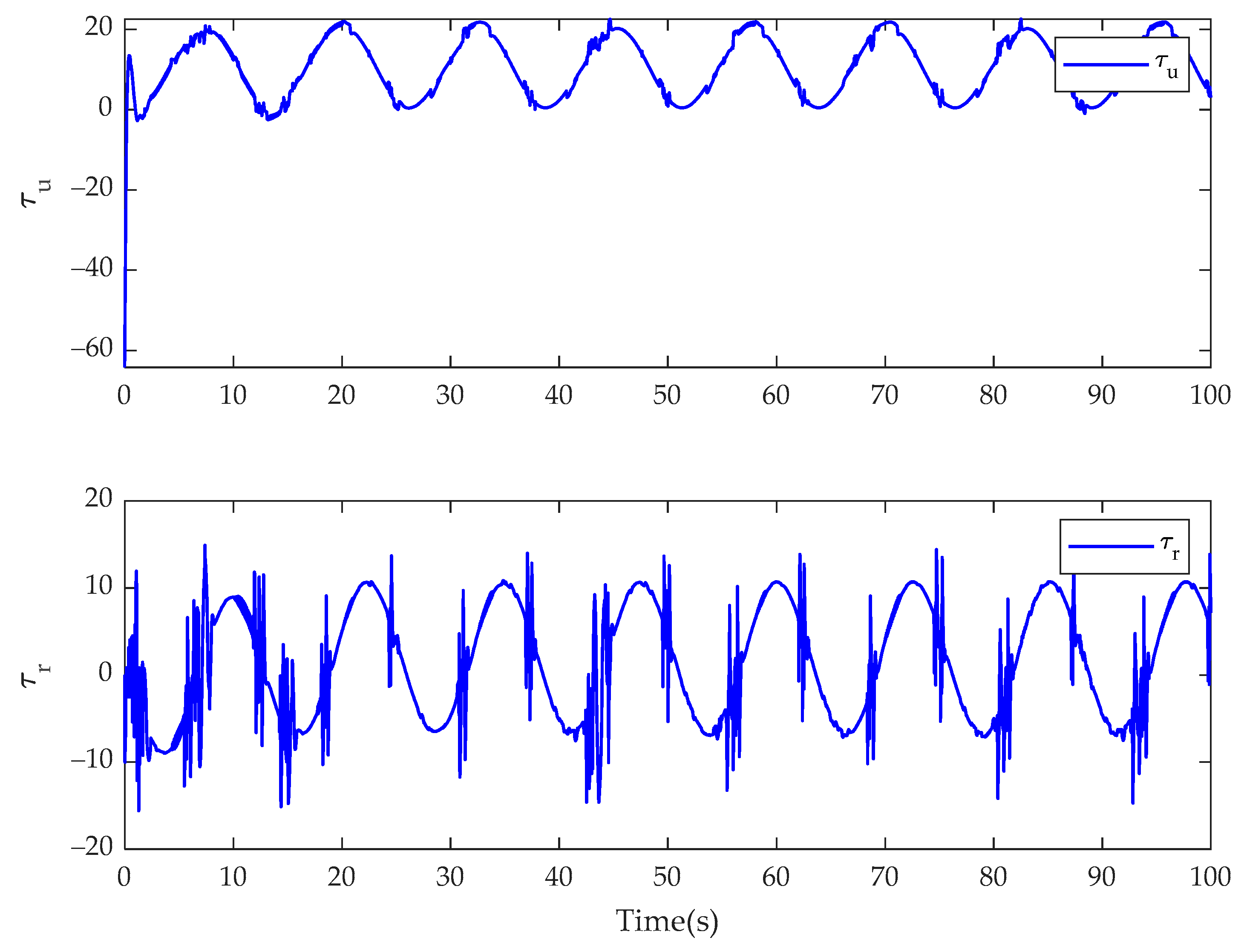
4. Simulation Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lv, C.X.; Yu, H.S.; Chen, J.; Zhao, N.; Chi, J.R. Trajectory tracking control for unmanned surface vessel with input saturation and disturbances via robust state error IDA-PBC approach. J. Frankl. Inst. 2022, 359, 1899–1924. [Google Scholar] [CrossRef]
- Guo, X.H.; Narthsirinth, N.; Zhang, W.D.; Hu, Y.Z. Unmanned surface vehicles (USVs) scheduling method by a bi-level mission planning and path control. Comput. Oper. Res. 2024, 162, 106472. [Google Scholar] [CrossRef]
- Li, J.P.; Fan, Y.S.; Liu, J.X. Adaptive NN formation tracking control for the multiple underactuated USVs with prescribed performance and input saturations. Ocean Eng. 2023, 290, 116274. [Google Scholar] [CrossRef]
- Lim, C.S.; Lee, S.S.; Levi, E. Continuous-Control-Set Model Predictive Current Control of Asymmetrical Six- Phase Drives Considering System Nonidealities. IEEE Trans. Ind. Electron. 2023, 70, 7615–7626. [Google Scholar] [CrossRef]
- Zhang, X.F.; Chen, S.N.; Zhang, J.X. Adaptive sliding mode consensus control based on neural network for singular fractional order multi-agent systems. Appl. Math. Comput. 2022, 434, 127442. [Google Scholar] [CrossRef]
- Reis, J.; Xie, W.; Cabecinhas, D.; Silvestre, C. Nonlinear Backstepping Controller for an Underactuated ASV With Model Parametric Uncertainty: Design and Experimental Validation. IEEE Trans. Intell. Veh. 2023, 8, 2514–2526. [Google Scholar] [CrossRef]
- Wang, Z.K.; Zhang, L.J.; Zhu, Z.Y. Game-based distributed optimal formation tracking control of underactuated AUVs based on reinforcement learning. Ocean Eng. 2023, 287, 115879. [Google Scholar] [CrossRef]
- Liu, H.; Pan, Y.; Cao, J.; Wang, H.; Zhou, Y. Adaptive neural network backstepping control of fractional-order nonlinear systems with actuator faults. IEEE Trans. Neural Netw. Learn. Syst. 2020, 31, 5166–5177. [Google Scholar] [CrossRef]
- Habibi, H.; Nohooji, H.R.; Howard, I. Backstepping Nussbaum gain dynamic surface control for a class of input and state constrained systems with actuator faults. Inf. Sci. 2019, 482, 27–46. [Google Scholar] [CrossRef]
- Alsaadi, F.E.; Zhang, X.L.; Alassafi, M.O.; Alotaibi, R.M.; Ahmad, A.M.; Cao, J.D. Fuzzy Command Filter Backstepping Control for Incommensurate Fractional-Order Systems via Composite Learning. Int. J. Fuzzy Syst. 2022, 24, 3293–3307. [Google Scholar] [CrossRef]
- Sheng, N.; Zhang, D.; Zhang, Q.C. Fuzzy Command Filtered Backstepping Control for Nonlinear System With Nonlinear Faults. IEEE Access 2021, 9, 60409–60418. [Google Scholar] [CrossRef]
- Cui, G.Z.; Yu, J.P.; Shi, P. Observer-based finite-time adaptive fuzzy control with prescribed performance for nonstrict-feedback nonlinear systems. IEEE Trans. Fuzzy Syst. 2020, 30, 767–778. [Google Scholar] [CrossRef]
- Lv, C.X.; Yu, H.S.; Chi, J.R.; Xu, T.; Zang, H.C.; Jiang, H.L.; Zhang, Z.W. A hybrid coordination controller for speed and heading control of underactuated unmanned surface vehicles system. Ocean Eng. 2019, 176, 222–230. [Google Scholar] [CrossRef]
- Peng, Z.H.; Wang, D.; Wang, J. Data-Driven Adaptive Disturbance Observers for Model-Free Trajectory Tracking Control of Maritime Autonomous Surface Ships. IEEE Trans. Neural Netw. Learn. Syst. 2021, 32, 5584–5594. [Google Scholar] [CrossRef] [PubMed]
- Chen, Z.; Zhang, Y.G.; Nie, Y.; Tang, J.Z.; Zhu, S.Q. Adaptive Sliding Mode Control Design for Nonlinear Unmanned Surface Vessel Using RBFNN and Disturbance-Observer. IEEE Access 2020, 8, 45457–45467. [Google Scholar] [CrossRef]
- Chen, J.; Hu, X.Y.; Lv, C.X.; Zhang, Z.Y.; Ma, R.N. Adaptive event-triggered fuzzy tracking control for underactuated surface vehicles under external disturbances. Ocean Eng. 2023, 283, 115026. [Google Scholar] [CrossRef]
- Deng, Y.; Peng, Y.; Qu, D.; Han, T.; Zhan, X.S. Neuro-adaptive containment control of unmanned surface vehicles with disturbance observer and collision-frees. ISA Trans. 2022, 129, 150–156. [Google Scholar] [CrossRef]
- Zhang, X.F.; Chen, Y.Q. Admissibility and robust stabilization of continuous linear singular fractional order systems with the fractional order α: The 0 < α < 1 case. ISA Trans. 2018, 82, 42–50. [Google Scholar]
- Zhang, X.F.; Driss, D.; Liu, D.Y. Applications of fractional operator in image processing and stability of control systems. Fractal Fract. 2023, 7, 359. [Google Scholar] [CrossRef]
- Zhang, X.F.; Lin, C.; Chen, Y.Q.; Boutat, D. A unified framework of stability theorems for LTI fractional order systems with 0 < alpha < 2. IEEE Trans. Circuits Syst. II 2020, 67, 3237–3241. [Google Scholar]
- Sabouri, J.; Effati, S.; Pakdaman, M. A neural network approach for solving a class of fractional optimal control problems. Neural Process. Lett. 2017, 45, 59–74. [Google Scholar] [CrossRef]
- Acharya, D.S.; Mishra, S.K.; Swain, S.K.; Ghosh, S. Real-Time Implementation of Fractional-Order PID Controller for Magnetic Levitation Plant With Time Delay. IEEE Trans. Instrum. Meas. 2022, 71, 1–11. [Google Scholar] [CrossRef]
- Zhang, D.D.; Li, F.; Ma, R.; Zhao, G.F.; Huangfu, Y.G. An Unknown Input Nonlinear Observer Based Fractional Order PID Control of Fuel Cell Air Supply System. IEEE. Trans. Ind. Appl. 2020, 56, 5523–5532. [Google Scholar]
- Ren, H.-P.; Wang, X.; Fan, J.-T.; Kaynak, O. Fractional order sliding mode control of a pneumatic position servo control. J. Frankl. Inst. 2019, 356, 6160–6174. [Google Scholar] [CrossRef]
- Liang, B.Y.; Zheng, S.Q.; Ahn, C.K.; Liu, F. Adaptive Fuzzy Control for Fractional-Order Interconnected Systems With Unknown Control Directions. IEEE Trans. Fuzzy Syst. 2022, 30, 75–87. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations, 1st ed.; Academic Press: San Diego, CA, USA, 1999; pp. 41–106. [Google Scholar]
- Di, Y.; Zhang, J.X.; Zhang, X.F. Alternate admissibility LMI criteria for descriptor fractional order systems with 0 < α < 2. Fractal Fract. 2023, 7, 577. [Google Scholar]
- Li, C.P.; Deng, W.H. Remarks on fractional derivates. Appl. Math. Comput. 2007, 187, 777–784. [Google Scholar]
- Demirci, E.; Ozalp, N. A method for solving differential equations of fractional order. Comput. Appl. Math. 2012, 236, 2754–2762. [Google Scholar] [CrossRef]
- Li, S.L.; Zhu, Y.K.; Bai, J.G.; Guo, G. Dynamic obstacle avoidance of unmanned ship based on event-triggered adaptive nonlinear model predictive control. Ocean Eng. 2023, 286, 115626. [Google Scholar] [CrossRef]
- Fossen, T.I. Handbook of Marine Craft Hydrodynamics and Motion Control, 2nd ed.; John Wiley& Sons: New York, NY, USA, 2011; pp. 133–142. [Google Scholar]
- Levant, A. Robust exact differentiation via sliding mode technique. Automatica 1998, 34, 379–384. [Google Scholar] [CrossRef]
- Shtessel, Y.; Edwards, C.; Fridman, L.; Levant, A. Sliding Mode Control and Observation, 1st ed.; Birkhauser Springer: New York, NY, USA, 2014; pp. 159–160. [Google Scholar]
- Levant, A. Higher-order sliding modes, differentiation and output-feedback control. Int. J. Control 2003, 76, 924–941. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, R.; Chen, J.; Lv, C.; Yang, Z.; Hu, X. Backstepping Control with a Fractional-Order Command Filter and Disturbance Observer for Unmanned Surface Vehicles. Fractal Fract. 2024, 8, 23. https://doi.org/10.3390/fractalfract8010023
Ma R, Chen J, Lv C, Yang Z, Hu X. Backstepping Control with a Fractional-Order Command Filter and Disturbance Observer for Unmanned Surface Vehicles. Fractal and Fractional. 2024; 8(1):23. https://doi.org/10.3390/fractalfract8010023
Chicago/Turabian StyleMa, Runan, Jian Chen, Chengxing Lv, Zhibo Yang, and Xiangyu Hu. 2024. "Backstepping Control with a Fractional-Order Command Filter and Disturbance Observer for Unmanned Surface Vehicles" Fractal and Fractional 8, no. 1: 23. https://doi.org/10.3390/fractalfract8010023
APA StyleMa, R., Chen, J., Lv, C., Yang, Z., & Hu, X. (2024). Backstepping Control with a Fractional-Order Command Filter and Disturbance Observer for Unmanned Surface Vehicles. Fractal and Fractional, 8(1), 23. https://doi.org/10.3390/fractalfract8010023








