Analytical Solutions for a Generalized Nonlinear Local Fractional Bratu-Type Equation in a Fractal Environment
Abstract
:1. Introduction
2. Preliminaries
2.1. Local Fractal Derivative
2.2. Local Fractal Integral
2.3. Properties of Local Fractional Derivatives
- (i)
- (ii)
- (iii)
2.4. Mittag-Leffler Function
3. Adomian Decomposition Method (ADM)
4. Solution of Generalized Local Fractional Bratu Equation
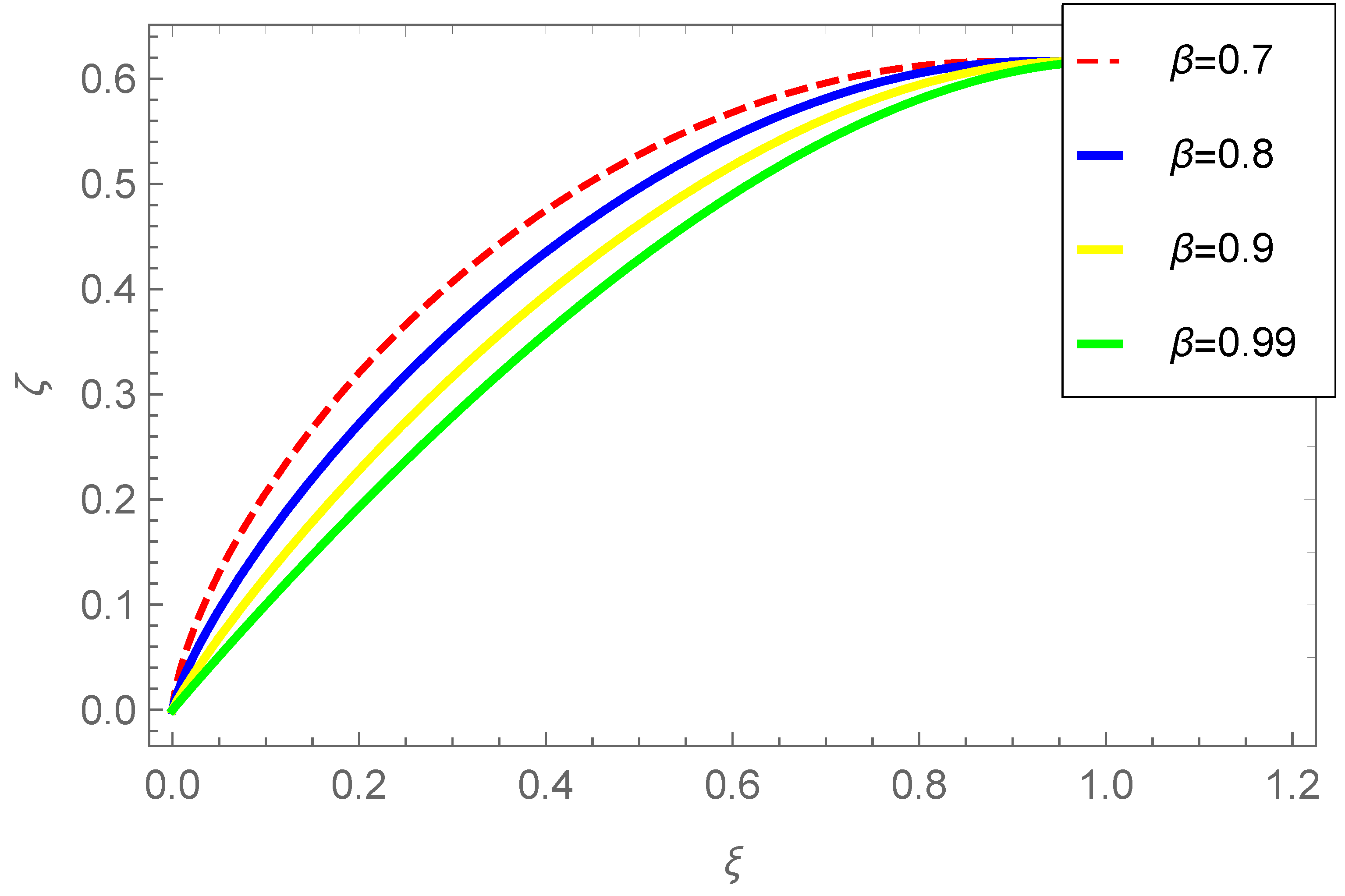
5. Particular Cases
- Special Case (i):
- Special Case (ii):
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| time co-ordinate | |
| space co-ordinate | |
| constant | |
| ℘ | constant |
References
- Miao, T.; Yu, B.; Duan, Y.; Fang, Q. A Fractal Analysis of Permeability for Fractured Rocks. Int. J. Heat Mass Transf. 2015, 81, 75–80. [Google Scholar] [CrossRef]
- Althobaiti, S.; Dubey, R.S.; Prasad, J.G. Solution of local fractional generalized fokker-planck equation using local fractional Mohand adomian decomposition method. Fractals 2022, 30, 2240028. [Google Scholar] [CrossRef]
- Li, L.; Yu, B. Fractal Analysis of the Effective Thermal Conductivity of Biological Media Embedded with Randomly Distributed Vascular Trees. Int. J. Heat Mass Transf. 2013, 67, 74–80. [Google Scholar] [CrossRef]
- Yang, X.J. Fractional Functional Analysis and Its Applications; Asian Academic: Hong Kong, China, 2011. [Google Scholar]
- Yang, X.J. Local Fractional Calculus and Its Applications; World Science Publisher: New York, NY, USA, 2012. [Google Scholar]
- Jafari, H.; Goswami, P.; Dubey, R.S.; Prasad, J.G. Solution of the local fractional generalized KDV equation using homotopy analysis method. Fractals 2021, 29, 2140014. [Google Scholar] [CrossRef]
- Wang, K.L. Exact travelling wave solution for the local fractional Camassa-Holm-Kadomtsev-Petviashvili equation. Alex. Eng. J. 2023, 63, 371–376. [Google Scholar] [CrossRef]
- Oldham, K.; Spanier, J. The Fractional Calculus: Theory and Applications of Differentiation and Integration to Arbitrary Order; Vol. 11 of Mathematics in Science and Engineering; Academic Press: New York, NY, USA, 1974. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; vol. 198 of Mathematics in Science and Engineering; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Jafari, H.; Goswami, P.; Dubey, R.S.; Sharma, S.; Chaudhary, A. Fractional SIZR model of Zombie infection. Int. J. Math. Comput. Eng. 2023, 1, 91–104. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; North-Holland Mathematics Studies, 204; Elsevier Science B.V.: Amsterdam, The Netherlands, 2006; p. xvi+523. [Google Scholar]
- Barbosa, R.S.; Tenreiro Machado, J.; Ferreira, I.M. PID controller tuning using fractional calculus concepts. Fract. Calc. Appl. Anal. 2004, 7, 119–134. [Google Scholar]
- Duarte, F.B.; Tenreiro Machado, J. Chaotic phenomena and fractional order dynamics in the trajectory control of redundant manipulators. Nonlinear Dyn. 2002, 29, 315–342. [Google Scholar] [CrossRef]
- Yang, X.J.; Srivastava, H.M. An Asymptotic Perturbation Solution for a Linear Oscillator of Free Damped Vibrations in Fractal Medium Described by Local Fractional Derivatives. Commun. Nonlinear Sci. Numer. Simul. 2015, 29, 499–504. [Google Scholar] [CrossRef]
- Yang, X.J.; Baleanu, D.; Khan, Y.; Mohyud-Din, S.T. Local Fractional Variational Iteration Method for Diffusion and Wave Equation on Cantor Sets. Rom. J. Phys. 2014, 59, 36–48. [Google Scholar]
- Yang, X.J.; Tenreiro Machado, J.A.; Baleanu, D.; Cattani, C. On exact traveling-wave solutions for local fractional Korteweg-de Vries equation. Chaos Interdiscip. J. Nonlinear Sci. 2016, 26, 110–118. [Google Scholar] [CrossRef] [PubMed]
- Kolwankar, K.M.; Gangal, A.D. Local fractional Fokker-Planck equation. Phys. Rev. Lett. 1998, 80, 214–217. [Google Scholar] [CrossRef]
- Jafari, H.; Jassim, H.K.; Tchier, F.; Baleanu, D. On the approximate solutions of local fractional differential equations with local fractional operators. Entropy 2016, 18, 150. [Google Scholar] [CrossRef]
- Zhang, Y.; Baleanu, D.; Yang, X.J. New solutions of the transport equations in porous media within local fractional derivative. Proc. Rom. Acad. Ser. A 2016, 17, 230–236. [Google Scholar]
- Wazwaz, A.M. Adomain Decomposition Method for a Reliable Treatment of the Bratu-type equations. Appl. Math. Comput. 2019, 166, 652–663. [Google Scholar]
- Yao, S.; Li, W.; Wang, K. Analytical solution for non-linear local fractional Bratu-type equation in a fractal space. Therm. Sci. 2020, 24, 3941–3947. [Google Scholar] [CrossRef]
- Wang, K.L. New perspective to the fractal Konopelchenko–Dubrovsky equations with M-truncated fractional derivative. Int. J. Geom. Methods Mod. Phys. 2023, 20, 2350072. [Google Scholar] [CrossRef]
- Yang, Y.J.; Hua, L.Q. Variational Iteration Transform Method for Fractional Differential Equations with Local Fractional Derivative. Abstr. Appl. Anal. 2014, 2014, 760957. [Google Scholar] [CrossRef]
- Wei, C.F.; Wang, K.L. Fractal soliton solutions for the fractal-fractional shallow water wave equation arising in ocean engineering. Alex. Eng. J. 2023, 65, 859–865. [Google Scholar] [CrossRef]
- Wang, K.L. A novel perspective to the local fractional Zakharov–Kuznetsov-modified equal width dynamical model on Cantor sets. Math. Methods Appl. Sci. 2023, 46, 622–630. [Google Scholar] [CrossRef]
- Kumar, S. A New Fractional Modeling Arising in Engineering Sciences and Its Analytical Approximate Solution. Alex. Eng. J. 2013, 52, 813–819. [Google Scholar] [CrossRef]
- Malyk, I.V.; Gorbatenko, M.; Chaudhary, A.; Sharma, S.; Dubey, R.S. Numerical Solution of Nonlinear Fractional Diffusion Equation in Framework of the Yang–Abdel–Cattani Derivative Operator. Fractal Fract. 2021, 5, 64. [Google Scholar] [CrossRef]
- Shah, N.A.; Hamed, Y.S.; Abualnaja, K.M.; Chung, J.D.; Shah, R.; Khan, A. A Comparative Analysis of Fractional Order Kaup–Kupershmidt Equation within Different Operators. Symmetry 2022, 14, 986. [Google Scholar] [CrossRef]
- Wang, K.J.; Wang, K.L. Variational Principles for Fractal Whitham-Broer-Kaup Equations in Shallow Water. Fractals 2020, 29, 2150028. [Google Scholar] [CrossRef]
- Yang, X.J. Fractal Vector Analysis: A Local Fractional Calculus Point of View; Academic Press: New York, NY, USA, 2021. [Google Scholar]
- Yang, X.J.; Tenreiro Machado, J.A. A New Fractal Nonlinear Burgers’ Equation Arising in the Acoustic Signals Propagation. Math. Methods Appl. Sci. 2019, 42, 7539–7544. [Google Scholar] [CrossRef]
- Kumar, D.; Singh, J.; Baleanu, D. A Hybrid Computational Approach for Klein-Gordon Equations on Cantor Sets. Nonlinear Dyn. 2017, 87, 511–517. [Google Scholar] [CrossRef]
- Wazwaz, A.M. A Reliable Modification of Adomian Decomposition Method. Appl. Math. Comput. 1999, 102, 77–86. [Google Scholar] [CrossRef]
- Adomian, G. A Review of the Decomposition Method in Applied Mathematics. J. Math. Anal. Appl. 1988, 135, 501–544. [Google Scholar] [CrossRef]
- Wang, K.L.; Yao, S.W.; Liu, Y.P.; Zhang, L.N. A Fractal Variational Principle for the Telegraph Equation with Fractal Derivatives. Fractals 2020, 28, 2050058. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alhamzi, G.; Dubey, R.S.; Alkahtani, B.S.T.; Saini, G.L. Analytical Solutions for a Generalized Nonlinear Local Fractional Bratu-Type Equation in a Fractal Environment. Fractal Fract. 2024, 8, 15. https://doi.org/10.3390/fractalfract8010015
Alhamzi G, Dubey RS, Alkahtani BST, Saini GL. Analytical Solutions for a Generalized Nonlinear Local Fractional Bratu-Type Equation in a Fractal Environment. Fractal and Fractional. 2024; 8(1):15. https://doi.org/10.3390/fractalfract8010015
Chicago/Turabian StyleAlhamzi, Ghaliah, Ravi Shanker Dubey, Badr Saad T. Alkahtani, and G. L. Saini. 2024. "Analytical Solutions for a Generalized Nonlinear Local Fractional Bratu-Type Equation in a Fractal Environment" Fractal and Fractional 8, no. 1: 15. https://doi.org/10.3390/fractalfract8010015
APA StyleAlhamzi, G., Dubey, R. S., Alkahtani, B. S. T., & Saini, G. L. (2024). Analytical Solutions for a Generalized Nonlinear Local Fractional Bratu-Type Equation in a Fractal Environment. Fractal and Fractional, 8(1), 15. https://doi.org/10.3390/fractalfract8010015






