Distinctive Shape Functions of Fractional Differential Quadrature for Solving Two-Dimensional Space Fractional Diffusion Problems
Abstract
:1. Introduction
2. Formulation of the Problem
2.1. The One-Dimensional Riesz Space Fractional Advection Equation
2.2. The One-Dimensional Riesz Space Fractional Advection–Dispersion Equation
2.3. The 2-D Fractional Diffusion Equation with Source Term [42]
3. Method of Solution
3.1. Caputo’s Fractional Derivative
3.2. Lagrange Interpolation Polynomial (PDQM)
3.3. Discrete Singular Convolution (DSCDQM)
3.4. Block Marching Technique with Differential Quadrature Discretization
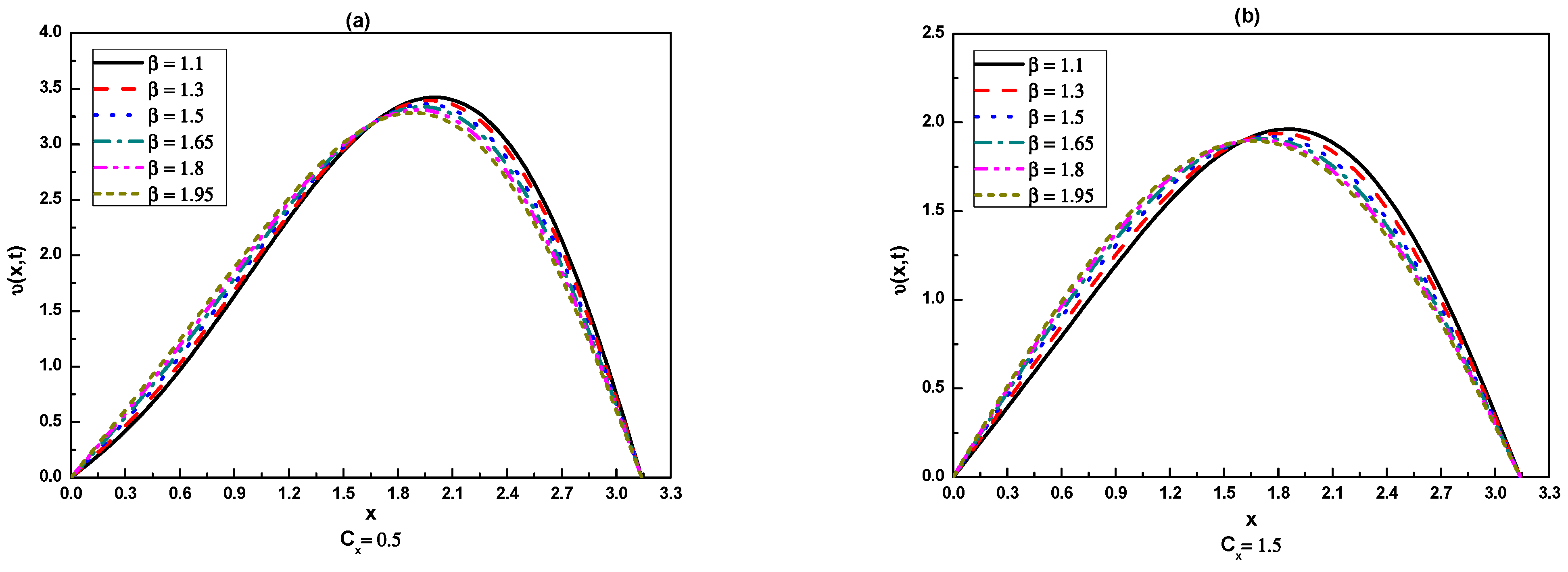
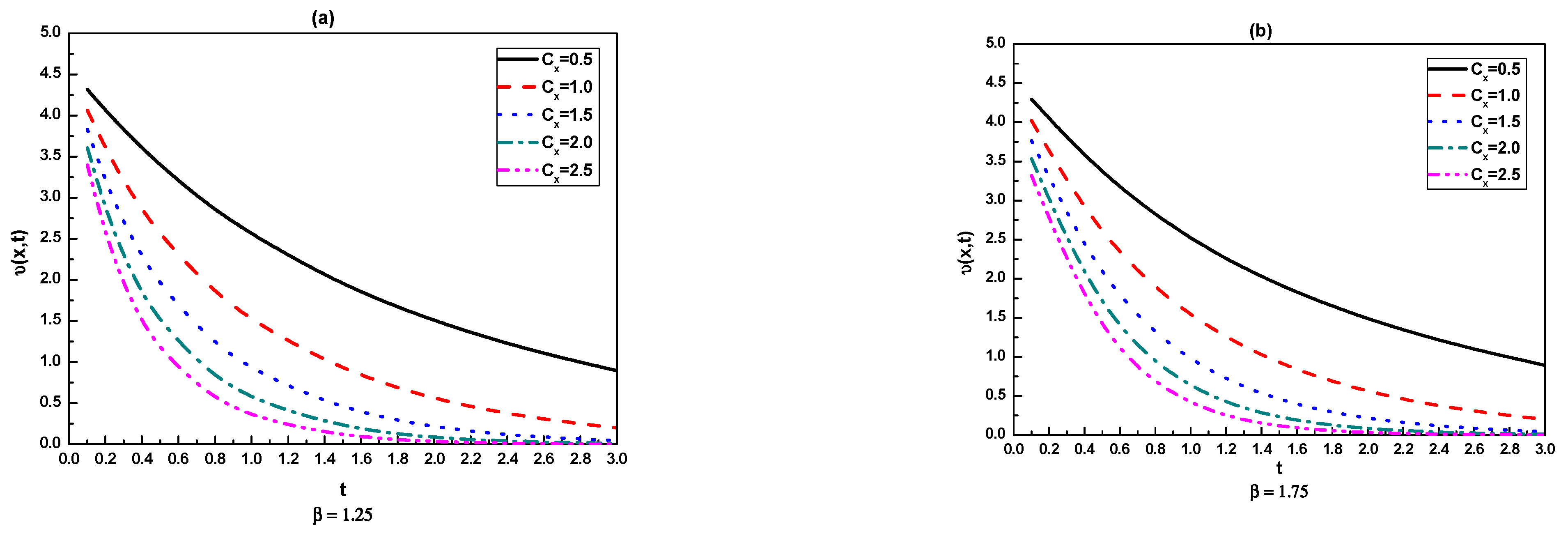
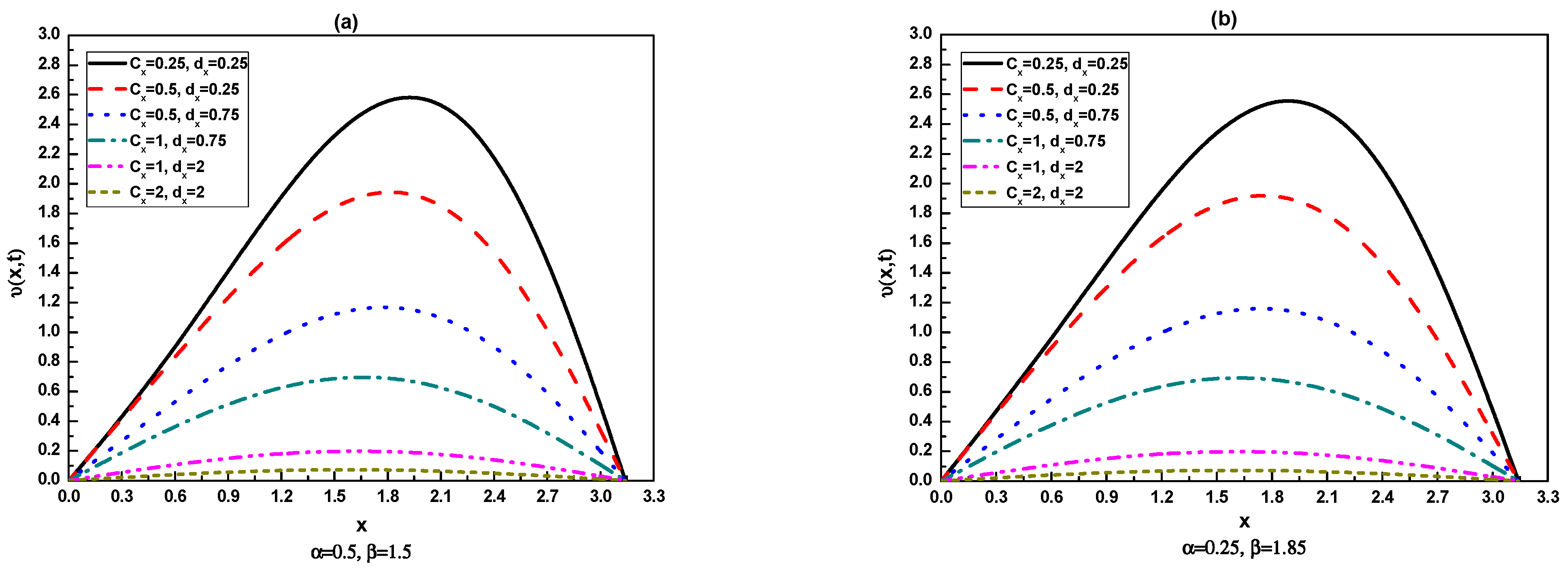
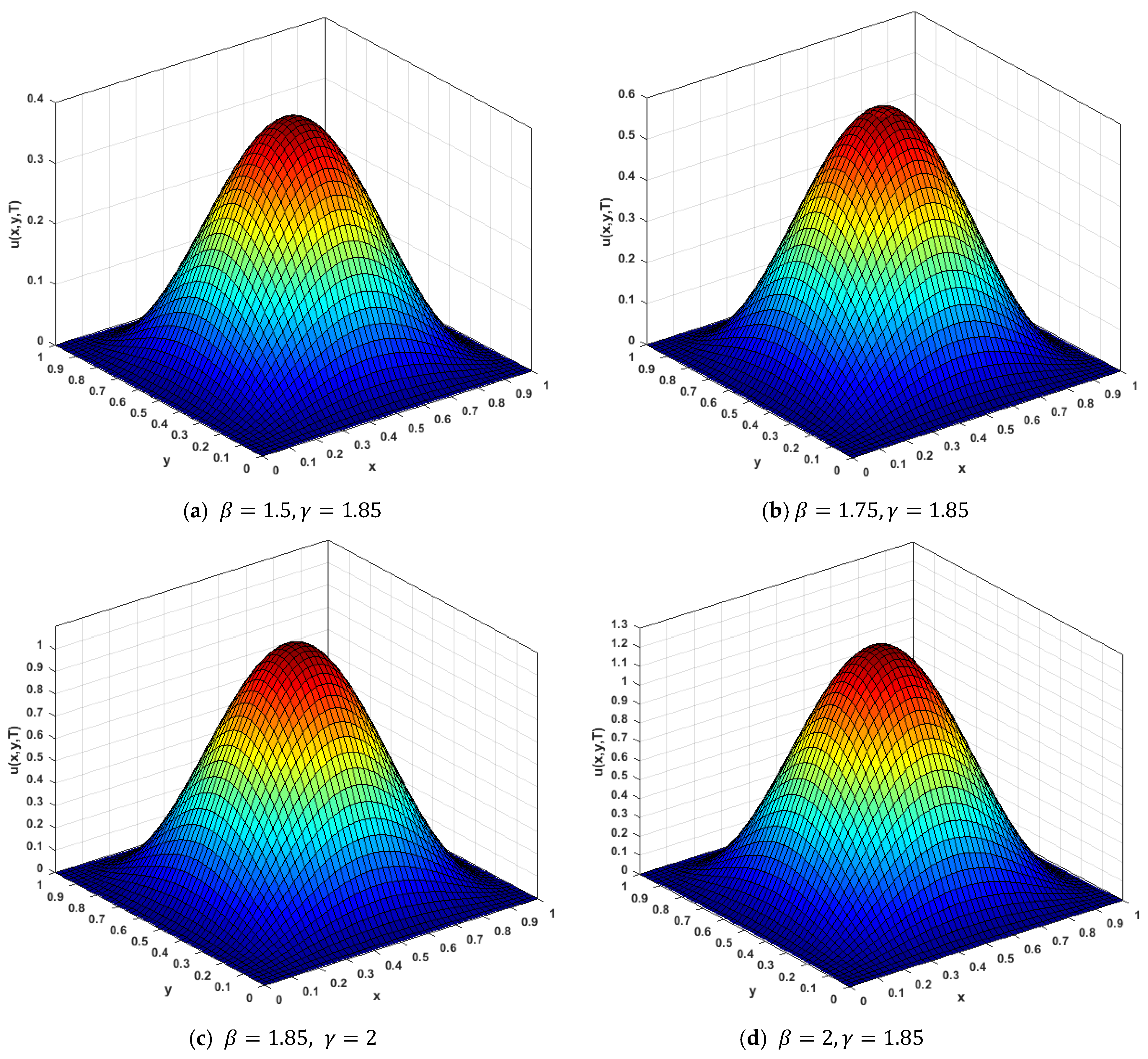
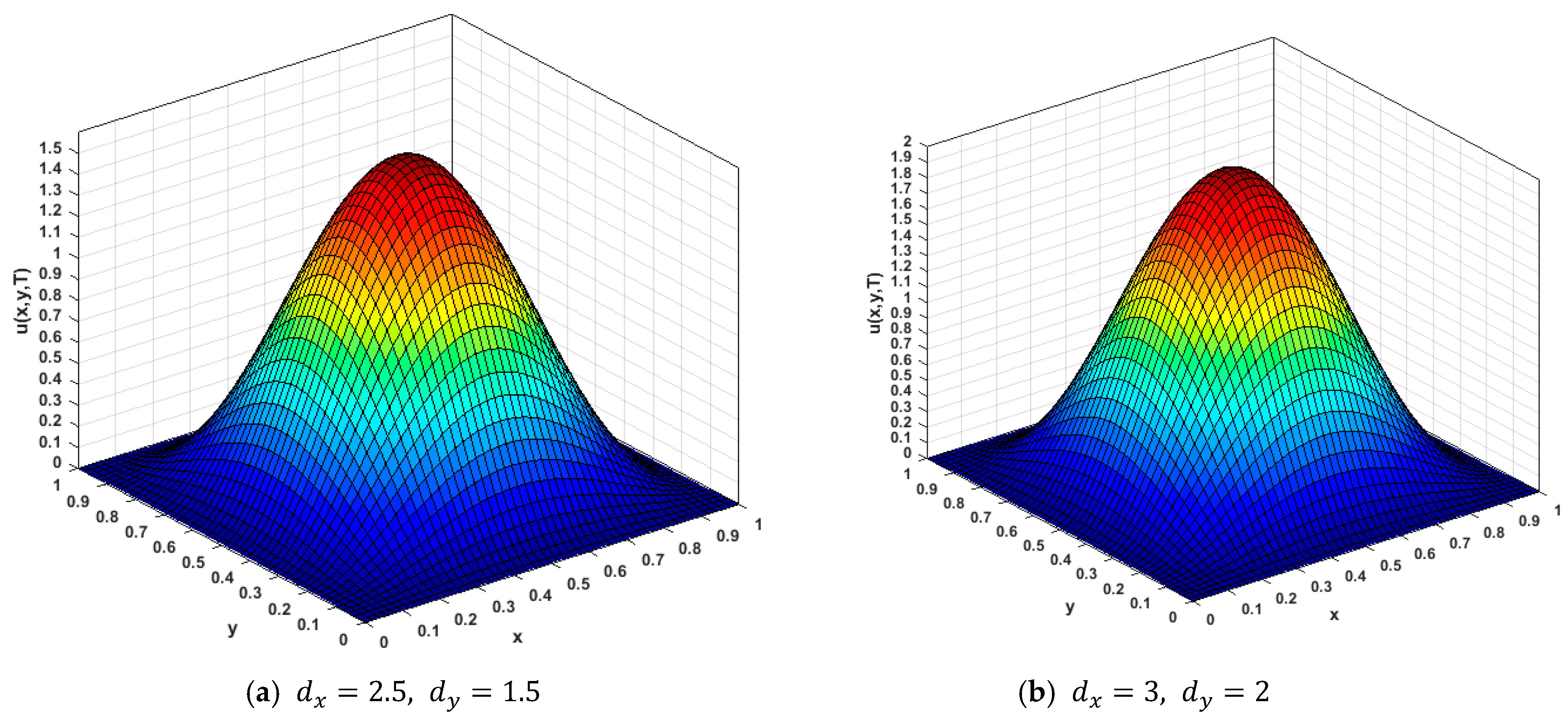
4. Numerical Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, K.; Wang, H. A Fast Characteristic Finite Difference Method for Fractional Advection–Diffusion Equations. Adv. Water Resour. 2011, 34, 810–816. [Google Scholar] [CrossRef]
- Fomin, S.; Chugunov, V.; Hashida, T. Application of Fractional Differential Equations for Modeling the Anomalous Diffusion of Contaminant from Fracture into Porous Rock Matrix with Bordering Alteration Zone. Transp. Porous Media 2010, 81, 187–205. [Google Scholar]
- Maamri, N.; Trigeassou, J.-C. A Comparative Analysis of Two Algorithms for the Simulation of Fractional Differential Equations. Int. J. Dyn. Control 2020, 8, 302–311. [Google Scholar] [CrossRef]
- Metzler, R.; Klafter, J. The Random Walk’s Guide to Anomalous Diffusion: A Fractional Dynamics Approach. Phys. Rep. 2000, 339, 1–77. [Google Scholar]
- Mirza, I.A.; Akram, M.S.; Shah, N.A.; Imtiaz, W.; Chung, J.D. Analytical Solutions to the Advection-Diffusion Equation with Atangana-Baleanu Time-Fractional Derivative and a Concentrated Loading. Alex. Eng. J. 2021, 60, 1199–1208. [Google Scholar]
- Khan, H.; Kumam, P.; Khan, Q.; Tchier, F.; Sitthithakerngkiet, K.; Dassios, I. A New Modified Analytical Approach for the Solution of Time-Fractional Convection–Diffusion Equations with Variable Coefficients. Front. Phys. 2022, 412, 900502. [Google Scholar]
- Attar, M.A.; Roshani, M.; Hosseinzadeh, K.; Ganji, D.D. Analytical Solution of Fractional Differential Equations by Akbari–Ganji’s Method. Partial Differ. Equ. Appl. Math. 2022, 6, 100450. [Google Scholar]
- Shah, R.; Khan, H.; Mustafa, S.; Kumam, P.; Arif, M. Analytical Solutions of Fractional-Order Diffusion Equations by Natural Transform Decomposition Method. Entropy 2019, 21, 557. [Google Scholar] [PubMed]
- Gu, X.-M.; Huang, T.-Z.; Ji, C.-C.; Carpentieri, B.; Alikhanov, A.A. Fast Iterative Method with a Second-Order Implicit Difference Scheme for Time-Space Fractional Convection–Diffusion Equation. J. Sci. Comput. 2017, 72, 957–985. [Google Scholar]
- Aboelenen, T. A Direct Discontinuous Galerkin Method for Fractional Convection-Diffusion and Schrödinger-Type Equations. Eur. Phys. J. Plus 2018, 133, 316. [Google Scholar]
- Parvizi, M.; Eslahchi, M.R.; Dehghan, M. Numerical Solution of Fractional Advection-Diffusion Equation with a Nonlinear Source Term. Numer. Algorithms 2015, 68, 601–629. [Google Scholar]
- Singh, J.; Swroop, R.; Kumar, D. A Computational Approach for Fractional Convection-Diffusion Equation via Integral Transforms. Ain Shams Eng. J. 2018, 9, 1019–1028. [Google Scholar]
- Bi, Y.; Jiang, Z. The Finite Volume Element Method for the Two-Dimensional Space-Fractional Convection–Diffusion Equation. Adv. Differ. Equ. 2021, 2021, 379. [Google Scholar]
- Ding, H.; Zhang, Y. New Numerical Methods for the Riesz Space Fractional Partial Differential Equations. Comput. Math. Appl. 2012, 63, 1135–1146. [Google Scholar]
- Anley, E.F.; Zheng, Z. Finite Difference Approximation Method for a Space Fractional Convection–Diffusion Equation with Variable Coefficients. Symmetry 2020, 12, 485. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, J.; Zhang, X. Semi-Discretized Numerical Solution for Time Fractional Convection–Diffusion Equation by RBF-FD. Appl. Math. Lett. 2022, 128, 107880. [Google Scholar]
- Saadeh, R. Numerical Solutions of Fractional Convection-Diffusion Equation Using Finite-Difference and Finite-Volume Schemes. J. Math. Comput. Sci. 2021, 11, 7872–7891. [Google Scholar]
- Bu, W.; Tang, Y.; Yang, J. Galerkin Finite Element Method for Two-Dimensional Riesz Space Fractional Diffusion Equations. J. Comput. Phys. 2014, 276, 26–38. [Google Scholar] [CrossRef]
- Valizadeh, S.; Malek, A.; Borhanifar, A. Compact ADI Method for Solving Two-Dimensional Riesz Space Fractional Diffusion Equation. arXiv 2018, arXiv:1802.02015. [Google Scholar]
- Chen, H.; Lv, W.; Zhang, T. A Kronecker Product Splitting Preconditioner for Two-Dimensional Space-Fractional Diffusion Equations. J. Comput. Phys. 2018, 360, 1–14. [Google Scholar]
- Li, J.; Liu, F.; Feng, L.; Turner, I. A Novel Finite Volume Method for the Riesz Space Distributed-Order Diffusion Equation. Comput. Math. Appl. 2017, 74, 772–783. [Google Scholar] [CrossRef]
- Zhuang, P.; Liu, F. Implicit Difference Approximation for the Time Fractional Diffusion Equation. J. Appl. Math. Comput. 2006, 22, 87–99. [Google Scholar] [CrossRef]
- Pingyang, Q.; Xiaodan, Z. A Numerical Method for the Space-Time Fractional Convection-Diffusion Equation. Math. Numer. Sin. 2008, 30, 305. [Google Scholar]
- Povstenko, Y.Z. Solutions to Time-Fractional Diffusion-Wave Equation in Cylindrical Coordinates. Adv. Differ. Equ. 2011, 2011, 930297. [Google Scholar] [CrossRef]
- Zhang, F.; Jiang, X. Analytical Solutions for a Time-Fractional Axisymmetric Diffusion–Wave Equation with a Source Term. Nonlinear Anal. Real World Appl. 2011, 12, 1841–1849. [Google Scholar] [CrossRef]
- Bu, W.; Liu, X.; Tang, Y.; Yang, J. Finite Element Multigrid Method for Multi-Term Time Fractional Advection Diffusion Equations. Int. J. Model. Simul. Sci. Comput. 2015, 6, 1540001. [Google Scholar] [CrossRef]
- Povstenko, Y.; Kyrylych, T. Two Approaches to Obtaining the Space-Time Fractional Advection-Diffusion Equation. Entropy 2017, 19, 297. [Google Scholar] [CrossRef]
- Mohyud-Din, S.T.; Akram, T.; Abbas, M.; Ismail, A.I.; Ali, N.H.M. A Fully Implicit Finite Difference Scheme Based on Extended Cubic B-Splines for Time Fractional Advection–Diffusion Equation. Adv. Differ. Equ. 2018, 2018, 109. [Google Scholar] [CrossRef]
- Marin, M.; Öchsner, A. The Effect of a Dipolar Structure on the Hölder Stability in Green–Naghdi Thermoelasticity. Contin. Mech. Thermodyn. 2017, 29, 1365–1374. [Google Scholar] [CrossRef]
- Lin, X.; Ng, M.K.; Sun, H.-W. A Multigrid Method for Linear Systems Arising from Time-Dependent Two-Dimensional Space-Fractional Diffusion Equations. J. Comput. Phys. 2017, 336, 69–86. [Google Scholar] [CrossRef]
- Tuan, N.H.; Aghdam, Y.E.; Jafari, H.; Mesgarani, H. A Novel Numerical Manner for Two-dimensional Space Fractional Diffusion Equation Arising in Transport Phenomena. Numer. Methods Partial Differ. Equ. 2021, 37, 1397–1406. [Google Scholar] [CrossRef]
- Devshali, P.; Arora, G. Solution of Two-Dimensional Fractional Diffusion Equation by a Novel Hybrid D (TQ) Method. Nonlinear Eng. 2022, 11, 135–142. [Google Scholar] [CrossRef]
- Jannelli, A.; Speciale, M.P. Exact and Numerical Solutions of Two-Dimensional Time-Fractional Diffusion–Reaction Equations through the Lie Symmetries. Nonlinear Dyn. 2021, 105, 2375–2385. [Google Scholar] [CrossRef]
- Zureigat, H.; Al-Smadi, M.; Al-Khateeb, A.; Al-Omari, S.; Alhazmi, S.E. Fourth-Order Numerical Solutions for a Fuzzy Time-Fractional Convection–Diffusion Equation under Caputo Generalized Hukuhara Derivative. Fractal Fract. 2023, 7, 47. [Google Scholar] [CrossRef]
- Mustafa, A.; Salama, R.S.; Mohamed, M. Semi-Analytical Analysis of Drug Diffusion through a Thin Membrane Using the Differential Quadrature Method. Mathematics 2023, 11, 2998. [Google Scholar] [CrossRef]
- Mustafa, A.; Salama, R.S.; Mohamed, M. Analysis of Generalized Nonlinear Quadrature for Novel Fractional-Order Chaotic Systems Using Sinc Shape Function. Mathematics 2023, 11, 1932. [Google Scholar] [CrossRef]
- Ragb, O.; Mohamed, M.; Matbuly, M.S. Vibration Analysis of Magneto-Electro-Thermo NanoBeam Resting on Nonlinear Elastic Foundation Using Sinc and Discrete Singular Convolution Differential Quadrature Method. Mod. Appl. Sci. 2019, 13, 49. [Google Scholar] [CrossRef]
- Shu, C. Differential Quadrature and Its Application in Engineering; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012; ISBN 1447104072. [Google Scholar]
- Civalek, Ö. Free Vibration of Carbon Nanotubes Reinforced (CNTR) and Functionally Graded Shells and Plates Based on FSDT via Discrete Singular Convolution Method. Compos. Part B Eng. 2017, 111, 45–59. [Google Scholar] [CrossRef]
- Ding, X.-L.; Jiang, Y.-L. Analytical Solutions for the Multi-Term Time–Space Fractional Advection–Diffusion Equations with Mixed Boundary Conditions. Nonlinear Anal. Real World Appl. 2013, 14, 1026–1033. [Google Scholar] [CrossRef]
- Zhang, H.; Jia, J.; Jiang, X. An Optimal Error Estimate for the Two-Dimensional Nonlinear Time Fractional Advection–Diffusion Equation with Smooth and Non-Smooth Solutions. Comput. Math. Appl. 2020, 79, 2819–2831. [Google Scholar] [CrossRef]
- Anley, E.F.; Zheng, Z. Finite Difference Method for Two-Sided Two Dimensional Space Fractional Convection-Diffusion Problem with Source Term. Mathematics 2020, 8, 1878. [Google Scholar] [CrossRef]
- Dong, G.; Guo, Z.; Yao, W. Numerical Methods for Time-Fractional Convection-Diffusion Problems with High-Order Accuracy. Open Math. 2021, 19, 782–802. [Google Scholar] [CrossRef]
- Yang, Q.; Liu, F.; Turner, I. Numerical Methods for Fractional Partial Differential Equations with Riesz Space Fractional Derivatives. Appl. Math. Model. 2010, 34, 200–218. [Google Scholar] [CrossRef]
- Zhang, Y.; Meerschaert, M.M.; Neupauer, R.M. Backward Fractional Advection Dispersion Model for Contaminant Source Prediction. Water Resour. Res. 2016, 52, 2462–2473. [Google Scholar] [CrossRef]
- Zaslavsky, G.M. Chaos, Fractional Kinetics, and Anomalous Transport. Phys. Rep. 2002, 371, 461–580. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Tadjeran, C. Finite Difference Approximations for Two-Sided Space-Fractional Partial Differential Equations. Appl. Numer. Math. 2006, 56, 80–90. [Google Scholar] [CrossRef]
- Saichev, A.I.; Zaslavsky, G.M. Fractional Kinetic Equations: Solutions and Applications. Chaos Interdiscip. J. Nonlinear Sci. 1997, 7, 753–764. [Google Scholar] [CrossRef] [PubMed]
- Caputo, M. Linear Models of Dissipation Whose Q Is Almost Frequency Independent—II. Geophys. J. Int. 1967, 13, 529–539. [Google Scholar] [CrossRef]
- Weilbeer, M. Efficient Numerical Methods for Fractional Differential Equations and Their Analytical Background; Papierflieger: Clausthal-Zellerfeld, Germany, 2006. [Google Scholar]
- Shao, Z.; Wei, G.W.; Zhao, S. DSC Time-Domain Solution of Maxwell’s Equations. J. Comput. Phys. 2003, 189, 427–453. [Google Scholar] [CrossRef]
- Wan, D.C.; Zhou, Y.C.; Wei, G.W. Numerical Solution of Incompressible Flows by Discrete Singular Convolution. Int. J. Numer. Methods Fluids 2002, 38, 789–810. [Google Scholar] [CrossRef]
- Civalek, Ö.; Kiracioglu, O. Free Vibration Analysis of Timoshenko Beams by DSC Method. Int. J. Numer. Methods Biomed. Eng. 2010, 26, 1890–1898. [Google Scholar] [CrossRef]
- Zhang, L.; Xiang, Y.; Wei, G.W. Local Adaptive Differential Quadrature for Free Vibration Analysis of Cylindrical Shells with Various Boundary Conditions. Int. J. Mech. Sci. 2006, 48, 1126–1138. [Google Scholar] [CrossRef]
- Ragb, O.; Mohamed, M.; Matbuly, M.S.; Civalek, O. An Accurate Numerical Approach for Studying Perovskite Solar Cells. Int. J. Energy Res. 2021, 45, 16456–16477. [Google Scholar] [CrossRef]
- Shu, C.; Yao, Q.; Yeo, K.S. Block-Marching in Time with DQ Discretization: An Efficient Method for Time-Dependent Problems. Comput. Methods Appl. Mech. Eng. 2002, 191, 4587–4597. [Google Scholar] [CrossRef]
- Shu, C.; Richards, B.E. Application of Generalized Differential Quadrature to Solve Two-dimensional Incompressible Navier-Stokes Equations. Int. J. Numer. Methods Fluids 1992, 15, 791–798. [Google Scholar] [CrossRef]
- Wei, G.W. Discrete Singular Convolution for the Solution of the Fokker–Planck Equation. J. Chem. Phys. 1999, 110, 8930–8942. [Google Scholar] [CrossRef]
- Zhang, F.; Gao, X.; Xie, Z. Difference Numerical Solutions for Time-Space Fractional Advection Diffusion Equation. Bound. Value Probl. 2019, 2019, 14. [Google Scholar] [CrossRef]
- Chen, M.; Deng, W. A Second-Order Numerical Method for Two-Dimensional Two-Sided Space Fractional Convection Diffusion Equation. Appl. Math. Model. 2014, 38, 3244–3259. [Google Scholar] [CrossRef]



| T | Uniform | CPU (s) | Non-Uniform | CPU (s) | ||
|---|---|---|---|---|---|---|
| 1 | 3 | 4 × 4 | 0.0020 | 0.04 | 0.0009 | 0.03 |
| 7 × 7 | 8.1287 × 10−5 | 0.05 | 7.4412 × 10−5 | 0.04 | ||
| 11 × 11 | 3.5473 × 10−5 | 0.08 | 2.2825 × 10−5 | 0.07 | ||
| 15 × 15 | 1.9741 × 10−5 | 0.11 | 6.3590 × 10−6 | 0.1 | ||
| 20 × 20 | 7.1438 × 10−6 | 0.14 | 5.0111 × 10−6 | 0.12 | ||
| 30 × 30 | 7.0035 × 10−6 | 0.16 | 5.0023 × 10−6 | 0.15 | ||
| 2 | 5 | 4 × 4 | 0.00859 | 0.05 | 0.00687 | 0.04 |
| 7 × 7 | 1.8517 × 10−4 | 0.06 | 0.5667 × 10−4 | 0.05 | ||
| 11 × 11 | 8.2024 × 10−5 | 0.11 | 4.3356 × 10−5 | 0.11 | ||
| 15 × 15 | 4.8793 × 10−5 | 0.15 | 2.4123 × 10−5 | 0.14 | ||
| 20 × 20 | 0.9991 × 10−5 | 0.16 | 7.0087 × 10−6 | 0.16 | ||
| 30 × 30 | 9.0028 × 10−6 | 0.18 | 1.0885 × 10−6 | 0.17 | ||
| 3 | 7 | 4 × 4 | 0.0587 | 0.065 | 0.0098 | 0.06 |
| 7 × 7 | 0.00247 | 0.07 | 0.00082 | 0.068 | ||
| 11 × 11 | 9.2018 × 10−4 | 0.12 | 5.4402 × 10−4 | 0.11 | ||
| 15 × 15 | 5.0011 × 10−4 | 0.16 | 1.9321 × 10−4 | 0.15 | ||
| 20 × 20 | 9.0023 × 10−5 | 0.17 | 4.0185 × 10−5 | 0.165 | ||
| 30 × 30 | 7.1616 × 10−5 | 0.20 | 2.8874 × 10−5 | 0.19 | ||
| Earlier numerical solutions [13,42,43,59] | 10 × 10 | 2.217 × 10−2 | ||||
| 20 × 20 | 5.759 × 10−3 | |||||
| 40 × 40 | 1.481 × 10−3 | |||||
| 80 × 80 | 3.727 × 10−4 | |||||
| 2M+1 | DSCDQM-DLK | DSCDQM-RSK | ||||
|---|---|---|---|---|---|---|
| 7 × 7 | 3 | 5.0055 | 8.3321 | 5.0714 | 4.6247 | 4.5932 |
| 5 | 4.8790 | 8.3198 | 5.0678 | 4.6005 | 4.5714 | |
| 7 | 4.6211 | 8.3025 | 5.0652 | 4.5932 | 4.5602 | |
| 9 | 4.5929 | 8.2577 | 5.0400 | 4.5929 | 4.5600 | |
| 11 | 4.5929 | 8.2577 | 5.0400 | 4.5929 | 4.5600 | |
| 9 × 9 | 3 | 4.9657 | 8.2470 | 5.0666 | 4.6211 | 4.5928 |
| 5 | 4.8001 | 8.2231 | 5.0641 | 4.5937 | 4.5710 | |
| 7 | 4.6111 | 8.2133 | 5.0352 | 4.5930 | 4.5599 | |
| 9 | 4.5929 | 8.2055 | 5.0344 | 4.5929 | 4.5599 | |
| 11 | 4.5929 | 8.2055 | 5.0344 | 4.5929 | 4.5599 | |
| 11 × 11 | 3 | 4.9611 | 8.2466 | 5.0660 | 4.6209 | 4.5925 |
| 5 | 4.7974 | 8.2225 | 5.0638 | 4.5932 | 4.5605 | |
| 7 | 4.6000 | 8.2055 | 5.0343 | 4.5929 | 4.5599 | |
| 9 | 4.5929 | 8.2055 | 5.0344 | 4.5929 | 4.5599 | |
| 11 | 4.5929 | 8.2055 | 5.0344 | 4.5929 | 4.5599 | |
| Earlier numerical solutions [13,42,43,59] | 4.5929 | |||||
| Exact [40,41,44] | 4.5929 | |||||
| 2M+1 | DSCDQM-DLK | DSCDQM-RSK | |||||
|---|---|---|---|---|---|---|---|
| 7 × 7 | 3 | 4.8322 | 8.1111 | 5.0512 | 4.6200 | 4.5911 | |
| 5 | 4.7595 | 8.0923 | 5.0439 | 4.5935 | 4.5706 | ||
| 7 | 4.6101 | 8.0899 | 5.0399 | 4.5931 | 4.5592 | ||
| 9 | 4.5929 | 8.0033 | 5.0296 | 4.5929 | 4.5588 | ||
| 11 | 4.5929 | 8.0033 | 5.0296 | 4.5929 | 4.5288 | ||
| 9 × 9 | 3 | 4.8225 | 8.1109 | 5.0510 | 4.6199 | 4.5910 | |
| 5 | 4.7577 | 8.0915 | 5.0435 | 4.5931 | 4.5705 | ||
| 7 | 4.6099 | 8.0885 | 5.0395 | 4.5930 | 4.5590 | ||
| 9 | 4.5929 | 8.0033 | 5.0296 | 4.5929 | 4.5588 | ||
| 11 | 4.5929 | 8.0033 | 5.0296 | 4.5929 | 4.5288 | ||
| 11 × 11 | 3 | 4.8200 | 8.1107 | 5.0508 | 4.6180 | 4.5908 | |
| 5 | 4.7510 | 8.0908 | 5.0433 | 4.5930 | 4.5702 | ||
| 7 | 4.6000 | 8.0033 | 5.0390 | 4.5929 | 4.5588 | ||
| 9 | 4.5929 | 8.0033 | 5.0296 | 4.5929 | 4.5588 | ||
| 11 | 4.5929 | 8.0033 | 5.0296 | 4.5929 | 4.5288 | ||
| Earlier numerical solutions [13,42,43,59] | 4.5929 | ||||||
| Exact [40,41,44] | 4.5929 | ||||||
| T | DSCDQM-DLK | CPU (s) | DSCDQM-RSK | CPU (s) | ||
|---|---|---|---|---|---|---|
| 1 | 3 | 4 × 4 | 4.2547 × 10−4 | 0.03 | 4.0005 × 10−4 | 0.03 |
| 7 × 7 | 3.00591 × 10−4 | 0.04 | 2.8741 × 10−4 | 0.03 | ||
| 11 × 11 | 9.5479 × 10−5 | 0.07 | 9.0214 × 10−5 | 0.06 | ||
| 15 × 15 | 9.01125 × 10−5 | 0.9 | 9.8881 × 10−6 | 0.08 | ||
| 20 × 20 | 9.7841 × 10−6 | 0.10 | 8.9125 × 10−6 | 0.09 | ||
| 30 × 30 | 7.8747 × 10−6 | 0.13 | 7.0012 × 10−6 | 0.12 | ||
| 2 | 5 | 4 × 4 | 0.0087 | 0.04 | 0.0032 | 0.04 |
| 7 × 7 | 3.2147 × 10−4 | 0.05 | 2.1313 × 10−4 | 0.06 | ||
| 11 × 11 | 1.0054 × 10−4 | 0.10 | 0.9881 × 10−4 | 0.09 | ||
| 15 × 15 | 2.1524 × 10−5 | 0.13 | 1.9651 × 10−5 | 0.11 | ||
| 20 × 20 | 0.3942 × 10−5 | 0.14 | 0.2089 × 10−5 | 0.13 | ||
| 30 × 30 | 9.3530 × 10−6 | 0.16 | 8.91248 × 10−6 | 0.16 | ||
| 3 | 7 | 4 × 4 | 0.0974 | 0.06 | 0.0441 | 0.05 |
| 7 × 7 | 0.0009 | 0.07 | 9.9874 × 10−4 | 0.07 | ||
| 11 × 11 | 3.2525 × 10−4 | 0.11 | 2.9745 × 10−4 | 0.10 | ||
| 15 × 15 | 9.0114 × 10−5 | 0.14 | 8.7815 × 10−5 | 0.12 | ||
| 20 × 20 | 8.6478 × 10−5 | 0.15 | 8.0345 × 10−5 | 0.14 | ||
| 30 × 30 | 9.0014 × 10−6 | 0.18 | 7.9992 × 10−6 | 0.17 | ||
| Earlier numerical solutions [13,42,43,59] | 10 × 10 | 2.217 × 10−2 | ||||
| 20 × 20 | 5.759 × 10−3 | |||||
| 40 × 40 | 1.481 × 10−3 | |||||
| 80 × 80 | 3.727 × 10−4 | |||||
| T | DSCDQM-DLK | DSCDQM-RSK | Exact [40,41,44] | Error Norms | |
|---|---|---|---|---|---|
| 0.5 | 1 | 2.466317 | 2.466317 | 2.466317 | 10−6 |
| 1.5 | 1.890399 | 1.890398 | 1.890398 | 10−6 | |
| 2 | 1.471863 | 1.471863 | 1.471863 | 10−6 | |
| 2.5 | 1.146013 | 1.1460129 | 1.1460129 | 10−7 | |
| 3 | 0.8925198 | 0.8925198 | 0.8925198 | 10−7 | |
| 1 | 1 | 1.471863 | 1.471863 | 1.471863 | 10−6 |
| 1.5 | 0.89252 | 0.892519 | 0.892519 | 10−6 | |
| 2 | 0.541341 | 0.541341 | 0.541341 | 10−6 | |
| 2.5 | 0.32834 | 0.3283399 | 0.3283399 | 10−7 | |
| 3 | 0.1991483 | 0.1991482 | 0.1991482 | 10−7 | |
| 1.5 | 1 | 0.89252 | 0.892519 | 0.892519 | 10−6 |
| 1.5 | 0.421597 | 0.421596 | 0.421596 | 10−6 | |
| 2 | 0.1991482 | 0.1991482 | 0.199148 | 10−6 | |
| 2.5 | 0.094071 | 0.0940709 | 0.09407098 | 10−7 | |
| 3 | 0.044436 | 0.0444359 | 0.04443598 | 10−7 | |
| 2 | 1 | 0.541341 | 0.541341 | 0.541341 | 10−6 |
| 1.5 | 0.1991483 | 0.1991482 | 0.1991482 | 10−6 | |
| 2 | 0.0732626 | 0.0732625 | 0.0732625 | 10−6 | |
| 2.5 | 0.026952 | 0.0269517 | 0.0269517 | 10−7 | |
| 3 | 0.009915 | 0.00991500 | 0.00991500 | 10−7 |
| T | Uniform | CPU (s) | Non-Uniform | CPU (s) | ||
|---|---|---|---|---|---|---|
| 1 | 3 | 4 × 4 | 0.0035 | 0.05 | 0.0011 | 0.04 |
| 7 × 7 | 3.9743 × 10−4 | 0.06 | 1.8222 × 10−4 | 0.05 | ||
| 11 × 11 | 1.7810 × 10−4 | 0.12 | 5.2824 × 10−5 | 0.10 | ||
| 15 × 15 | 1.0461 × 10−4 | 0.15 | 2.3020 × 10−5 | 0.12 | ||
| 20 × 20 | 6.4410 × 10−5 | 0.17 | 1.0636 × 10−5 | 0.13 | ||
| 30 × 30 | 3.3502 × 10−5 | 0.19 | 3.7374 × 10−6 | 0.18 | ||
| 2 | 5 | 4 × 4 | 0.0871 | 0.05 | 0.0197 | 0.05 |
| 7 × 7 | 5.5308 × 10−4 | 0.07 | 3.7420 × 10−4 | 0.06 | ||
| 11 × 11 | 4.0382 × 10−4 | 0.13 | 1.2287 × 10−4 | 0.12 | ||
| 15 × 15 | 2.4131 × 10−4 | 0.16 | 5.4123 × 10−5 | 0.15 | ||
| 20 × 20 | 1.5008 × 10−4 | 0.18 | 2.5138 × 10−5 | 0.17 | ||
| 30 × 30 | 7.8785 × 10−4 | 0.20 | 8.8650 × 10−6 | 0.19 | ||
| 3 | 7 | 4 × 4 | 0.9243 | 0.078 | 0.1893 | 0.06 |
| 7 × 7 | 0.0085 | 0.089 | 4.8959 × 10−4 | 0.08 | ||
| 11 × 11 | 6.3737 × 10−4 | 0.14 | 1.9956 × 10−4 | 0.11 | ||
| 15 × 15 | 3.8886 × 10−4 | 0.17 | 8.8831 × 10−5 | 0.16 | ||
| 20 × 20 | 2.4417 × 10−4 | 0.20 | 4.1470 × 10−5 | 0.19 | ||
| 30 × 30 | 1.2855 × 10−4 | 0.22 | 1.4676 × 10−5 | 0.21 | ||
| Earlier numerical solutions [13,42,43,59] | 10 × 10 | 1.70 × 10−2 | ||||
| 20 × 20 | 4.4 × 10−3 | |||||
| 40 × 40 | 1.1 × 10−3 | |||||
| 80 × 80 | 2.7919 × 10−4 | |||||
| 160 × 160 | 6.9932 × 10−4 | |||||
| 2M+1 | DSCDQM-DLK | DSCDQM-RSK | |||||
|---|---|---|---|---|---|---|---|
| 7 × 7 | 3 | 1.364173 | 7.369612 | 1.49798649 | 0.59077 | 0.6206803 | |
| 5 | 0.855820 | 5.031103 | 1.22468775 | 0.55218 | 0.6065857 | ||
| 7 | 0.6120001 | 4.324652 | 0.94795129 | 0.544277 | 0.601601 | ||
| 9 | 0.5489179 | 2.588603 | 0.77950301 | 0.5432457 | 0.5976476 | ||
| 11 | 0.54315976 | 2.573993 | 0.77520586 | 0.5431598 | 0.593952 | ||
| 9 × 9 | 3 | 0.7522591 | 5.3551083 | 1.31063075 | 0.5710912 | 0.6148362 | |
| 5 | 0.6465491 | 4.8875784 | 1.20578029 | 0.5515822 | 0.602117 | ||
| 7 | 0.5586295 | 3.869154 | 0.88263461 | 0.5440192 | 0.5930067 | ||
| 9 | 0.54401919 | 2.0033313 | 0.73653151 | 0.5431598 | 0.585358 | ||
| 11 | 0.54315976 | 2.0033313 | 0.73653151 | 0.5431598 | 0.585358 | ||
| 11 × 11 | 3 | 0.6885753 | 4.0367427 | 1.13874475 | 0.5520119 | 0.6004837 | |
| 5 | 0.63236859 | 3.0483982 | 1.05022346 | 0.5446208 | 0.5882798 | ||
| 7 | 0.58793606 | 2.5714146 | 0.85951594 | 0.5438473 | 0.576935 | ||
| 9 | 0.54324570 | 1.7059686 | 0.67637141 | 0.5431598 | 0.5689426 | ||
| 11 | 0.54315976 | 1.7059686 | 0.67637141 | 0.5431598 | 0.5689426 | ||
| Earlier numerical solutions [13,42,43,59] | 0.54316 | ||||||
| Exact [40,41,44] | 0.54316 | ||||||
| 2M+1 | DSCDQM-DLK | DSCDQM-RSK | ||||
|---|---|---|---|---|---|---|
| 7 × 7 | 3 | 0.81388021 | 3.4265474 | 0.93420041 | 0.5566528 | 0.6196490 |
| 5 | 0.57238038 | 2.6719679 | 0.90669865 | 0.5511525 | 0.6013432 | |
| 7 | 0.54917577 | 2.60837 | 0.86200829 | 0.5435895 | 0.5957569 | |
| 9 | 0.54315976 | 2.5739929 | 0.77520586 | 0.5431598 | 0.5939521 | |
| 11 | 0.54315976 | 2.5739929 | 0.77520586 | 0.5431598 | 0.5939521 | |
| 9 × 9 | 3 | 0.69957602 | 3.1291846 | 0.8508357 | 0.5517541 | 0.5962725 |
| 5 | 0.54745691 | 2.1202138 | 0.82934995 | 0.5508946 | 0.5930067 | |
| 7 | 0.54487862 | 2.0643509 | 0.76317384 | 0.5433316 | 0.5854437 | |
| 9 | 0.54315976 | 2.0033313 | 0.73653151 | 0.5431598 | 0.5853578 | |
| 11 | 0.54315976 | 2.0033313 | 0.73653151 | 0.5431598 | 0.5853578 | |
| 11 × 11 | 3 | 0.60417929 | 3.0956669 | 0.76747099 | 0.5508946 | 0.5778807 |
| 5 | 0.54573805 | 2.0153634 | 0.75114182 | 0.5442770 | 0.5757322 | |
| 7 | 0.54315976 | 1.7472212 | 0.68668457 | 0.5431598 | 0.5692005 | |
| 9 | 0.54315976 | 1.7059686 | 0.67637141 | 0.5431598 | 0.5689427 | |
| 11 | 0.54315976 | 1.7059686 | 0.67637141 | 0.5431598 | 0.5689427 | |
| Earlier numerical solutions [13,42,43,59] | 0.54316 | |||||
| Exact [40,41,44] | 0.54316 | |||||
| T | DSCDQM-DLK | CPU (s) | DSCDQM-RSK | CPU (s) | ||
|---|---|---|---|---|---|---|
| 1 | 3 | 4 × 4 | 4.8206 × 10−4 | 0.035 | 5.1248 × 10−4 | 0.03 |
| 7 × 7 | 3.0167 × 10−4 | 0.05 | 2.5357 × 10−4 | 0.036 | ||
| 11 × 11 | 1.5084 × 10−4 | 0.08 | 1.3345 × 10−4 | 0.065 | ||
| 15 × 15 | 9.3066 × 10−5 | 0.10 | 8.4774 × 10−5 | 0.09 | ||
| 20 × 20 | 5.9753 × 10−5 | 0.11 | 5.5136 × 10−5 | 0.097 | ||
| 30 × 30 | 3.1624 × 10−5 | 0.15 | 3.0054 × 10−5 | 0.13 | ||
| 2 | 5 | 4 × 4 | 0.0144 | 0.042 | 0.0036 | 0.038 |
| 7 × 7 | 6.9981 × 10−4 | 0.055 | 5.6238 × 10−4 | 0.062 | ||
| 11 × 11 | 3.4394 × 10−4 | 0.10 | 3.0571 × 10−4 | 0.093 | ||
| 15 × 15 | 2.1524 × 10−4 | 0.13 | 1.9651 × 10−4 | 0.11 | ||
| 20 × 20 | 1.3942 × 10−4 | 0.15 | 1.2879 × 10−4 | 0.135 | ||
| 30 × 30 | 7.3970 × 10−5 | 0.17 | 7.0371 × 10−5 | 0.16 | ||
| 3 | 7 | 4 × 4 | 0.2326 | 0.063 | 0.0578 | 0.055 |
| 7 × 7 | 0.0027 | 0.072 | 6.5842 × 10−4 | 0.068 | ||
| 11 × 11 | 5.4871 × 10−4 | 0.105 | 4.8945 × 10−4 | 0.09 | ||
| 15 × 15 | 3.4771 × 10−4 | 0.12 | 3.1815 × 10−4 | 0.11 | ||
| 20 × 20 | 2.2711 × 10−4 | 0.14 | 2.1005 × 10−4 | 0.13 | ||
| 30 × 30 | 1.2252 × 10−4 | 0.16 | 1.1680 × 10−4 | 0.155 | ||
| Earlier numerical solutions [13,42,43,59] | 10 × 10 | 1.75 × 10−2 | ||||
| 20 × 20 | 4.5 × 10−3 | |||||
| 40 × 40 | 1.1 × 10−3 | |||||
| 80 × 80 | 2.8337 × 10−4 | |||||
| 160 × 160 | 7.0902 × 10−5 | |||||
| () | T | DSCDQM-DLK | DSCDQM-RSK | Exact [40,41,44] | Error Norms |
|---|---|---|---|---|---|
| (0.5, 1) | 1 | 0.892502 | 0.892501 | 0.892501 | 10−6 |
| 1.5 | 0.421598 | 0.421597 | 0.4215967 | 10−6 | |
| 2 | 0.1991484 | 0.1991483 | 0.1991483 | 10−6 | |
| 2.5 | 0.094072 | 0.09407098 | 0.094071 | 10−7 | |
| 3 | 0.044437 | 0.044436 | 0.044436 | 10−7 | |
| (1, 1.5) | 1 | 0.328342 | 0.3283398 | 0.3283398 | 10−6 |
| 1.5 | 0.0940714 | 0.094071 | 0.094071 | 10−6 | |
| 2 | 0.0269518 | 0.02695179 | 0.02695179 | 10−6 | |
| 2.5 | 0.0077219 | 0.0077218 | 0.0077218 | 10−7 | |
| 3 | 0.0022124 | 0.0022123 | 0.0022123 | 10−7 | |
| (1.5, 2) | 1 | 0.1207896 | 0.1207895 | 0.1207895 | 10−6 |
| 1.5 | 0.0209900 | 0.0209900 | 0.0209900 | 10−6 | |
| 2 | 0.003647528 | 0.003647527 | 0.003647527 | 10−6 | |
| 2.5 | 0.00063385 | 0.000633845 | 0.000633845 | 10−7 | |
| 3 | 0.000110147 | 0.000110146 | 0.000110146 | 10−7 | |
| (2, 2.5) | 1 | 0.044436 | 0.04443599 | 0.04443599 | 10−6 |
| 1.5 | 0.00468353 | 0.00468352 | 0.00468352 | 10−6 | |
| 2 | 0.0004936392 | 0.0004936392 | 0.000493639 | 10−6 | |
| 2.5 | 5.20291906 × 10−5 | 5.20291906 × 10−5 | 5.20291906 × 10−5 | 10−7 | |
| 3 | 5.4838363 × 10−6 | 5.4838363 × 10−46 | 5.4838363 × 10−6 | 10−7 |
| CPU (s) | ||||||
|---|---|---|---|---|---|---|
| Order | Order | |||||
| 0.5 | 2.05 × 10−3 | --- | 1.6 × 10−3 | --- | 0.05 | |
| 0.1 | 2.59 × 10−4 | 2.0193 | 4.05 × 10−4 | 1.98207 | 0.07 | |
| 0.05 | 6.56 × 10−5 | 1.9812 | 9.62 × 10−5 | 2.0738 | 0.09 | |
| 0.025 | 1.57 × 10−5 | 2.0629 | 2.35 × 10−5 | 2.0333 | 0.15 | |
| Earlier numerical solutions [13,42,43,59] | 0.1 | 2.57 × 10−2 | --- | 1.56 × 10−2 | --- | --- |
| 0.05 | 5.5 × 10−3 | 2.2243 | 3.2 × 10−3 | 2.2854 | --- | |
| 0.025 | 7.41 × 10−4 | 2.4894 | 7.97 × 10−4 | 2.0056 | --- | |
| 0.0125 | 1.66 × 10−4 | 2.1565 | 1.9977 × 10−4 | 1.9961 | --- | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mustafa, A.; Ragb, O.; Salah, M.; Salama, R.S.; Mohamed, M. Distinctive Shape Functions of Fractional Differential Quadrature for Solving Two-Dimensional Space Fractional Diffusion Problems. Fractal Fract. 2023, 7, 668. https://doi.org/10.3390/fractalfract7090668
Mustafa A, Ragb O, Salah M, Salama RS, Mohamed M. Distinctive Shape Functions of Fractional Differential Quadrature for Solving Two-Dimensional Space Fractional Diffusion Problems. Fractal and Fractional. 2023; 7(9):668. https://doi.org/10.3390/fractalfract7090668
Chicago/Turabian StyleMustafa, Abdelfattah, Ola Ragb, Mohamed Salah, Reda S. Salama, and Mokhtar Mohamed. 2023. "Distinctive Shape Functions of Fractional Differential Quadrature for Solving Two-Dimensional Space Fractional Diffusion Problems" Fractal and Fractional 7, no. 9: 668. https://doi.org/10.3390/fractalfract7090668
APA StyleMustafa, A., Ragb, O., Salah, M., Salama, R. S., & Mohamed, M. (2023). Distinctive Shape Functions of Fractional Differential Quadrature for Solving Two-Dimensional Space Fractional Diffusion Problems. Fractal and Fractional, 7(9), 668. https://doi.org/10.3390/fractalfract7090668











