Heart Rhythm Analysis Using Nonlinear Oscillators with Duffing-Type Connections
Abstract
1. Introduction
2. Mathematical Modeling
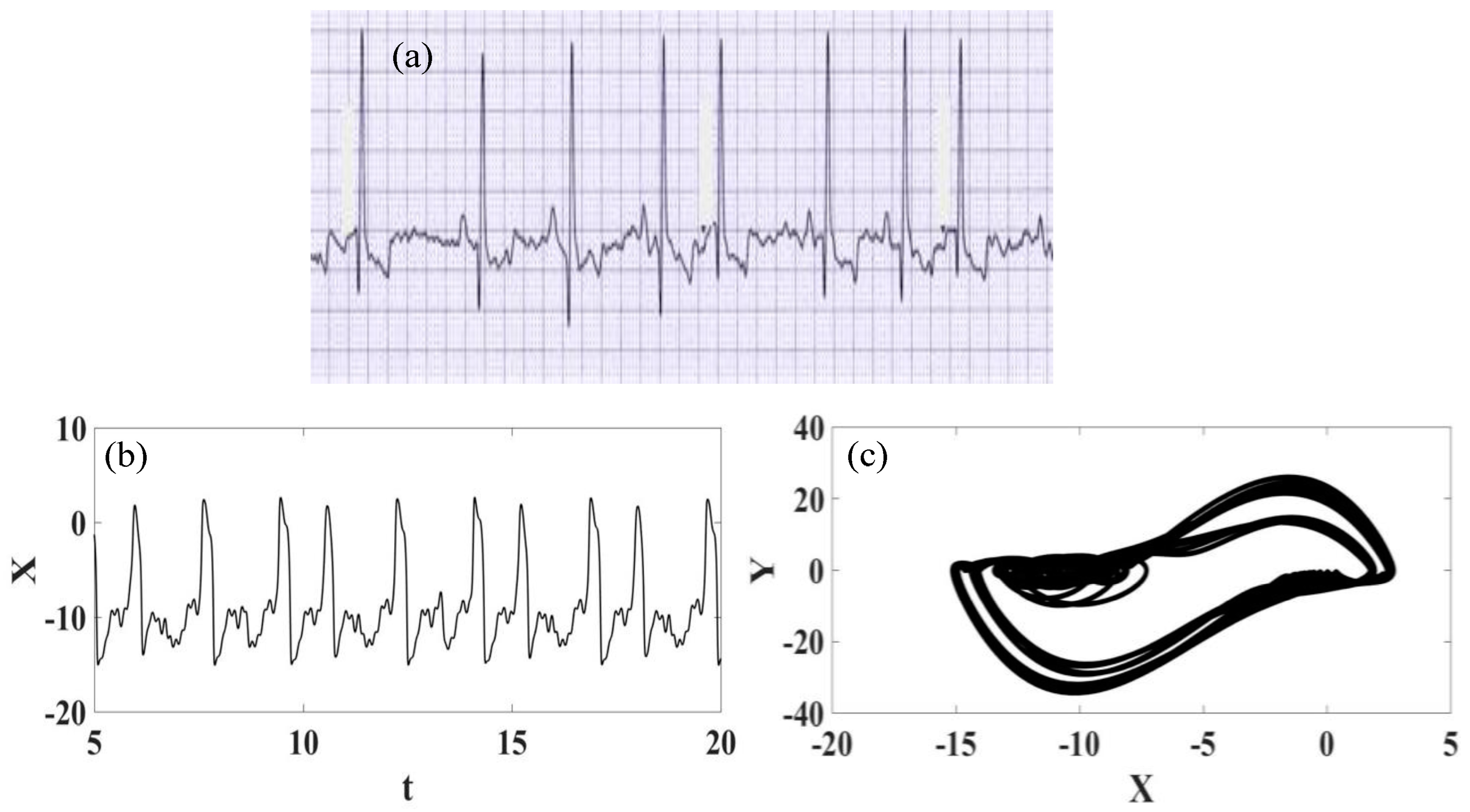
3. Normal Synthetic ECG
4. Pathological Synthetic ECGs
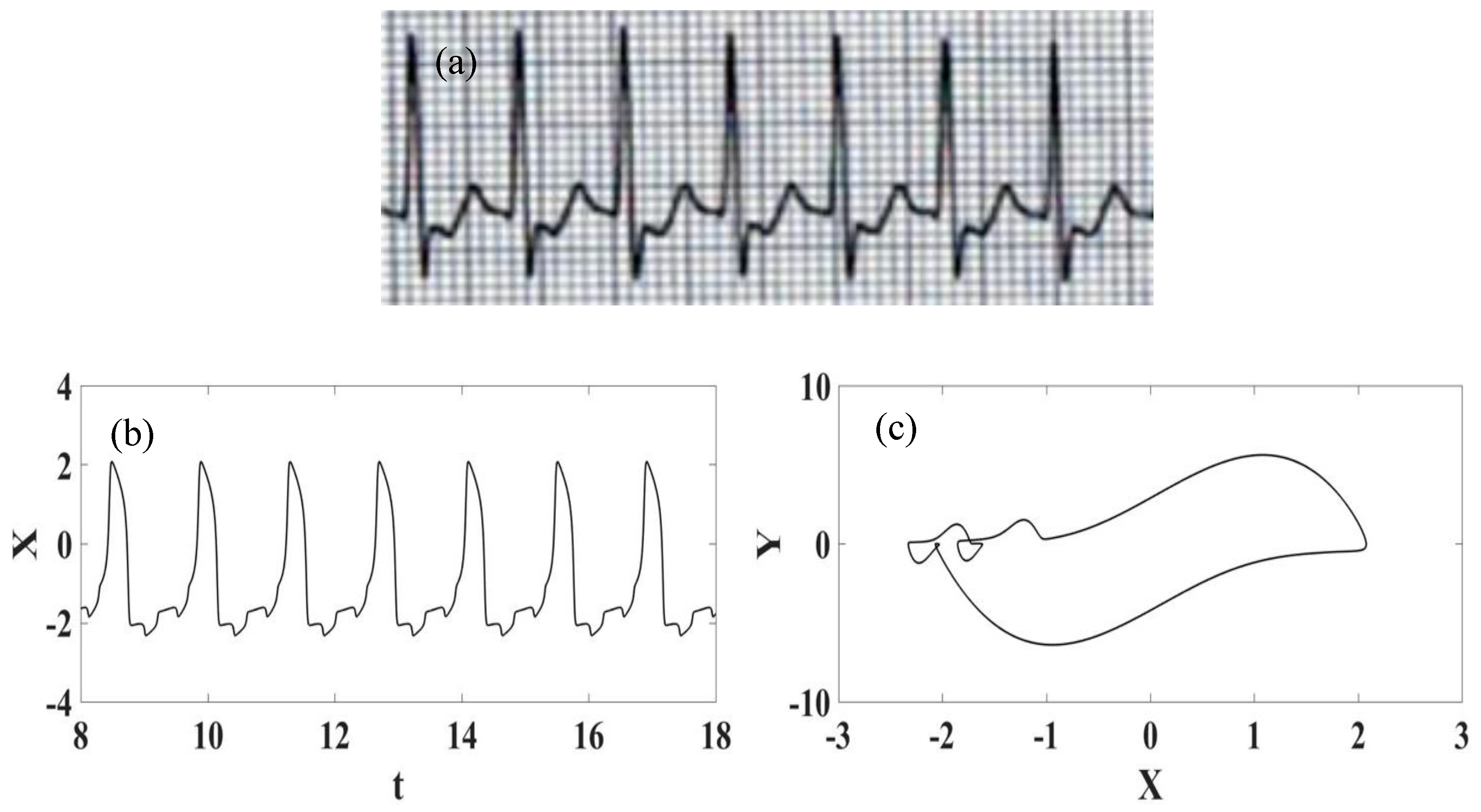
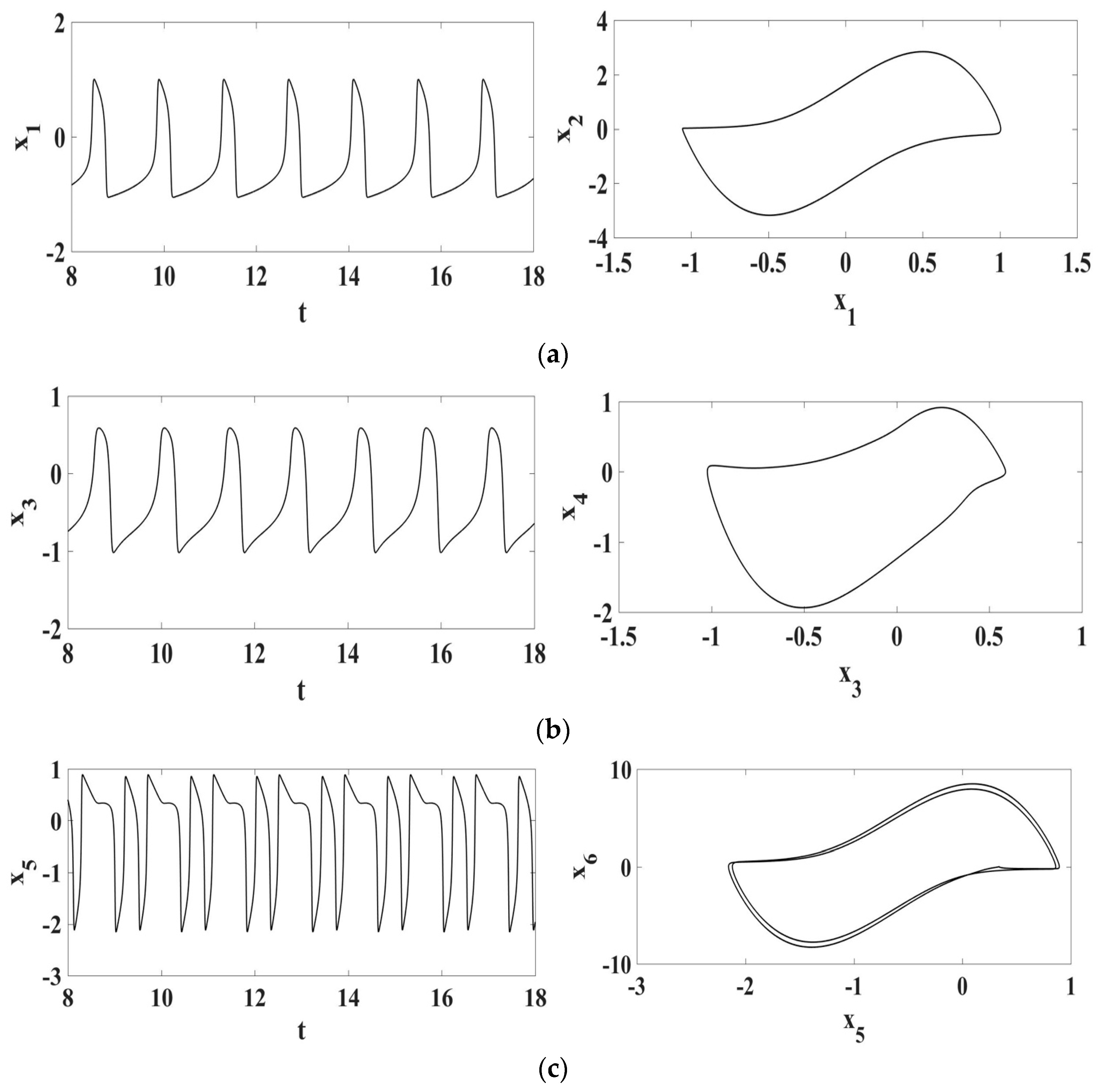
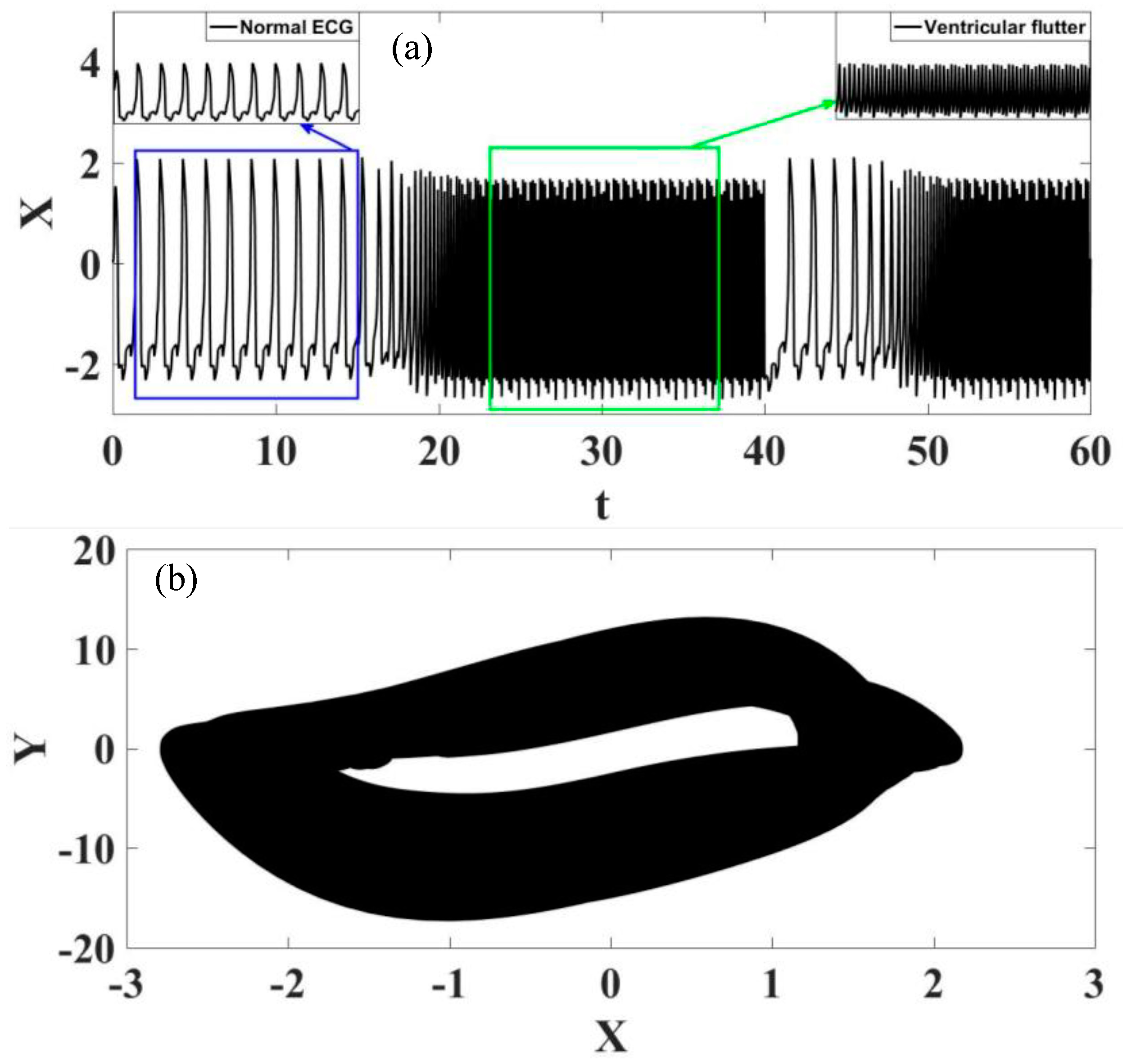
4.1. Ventricular Flutter
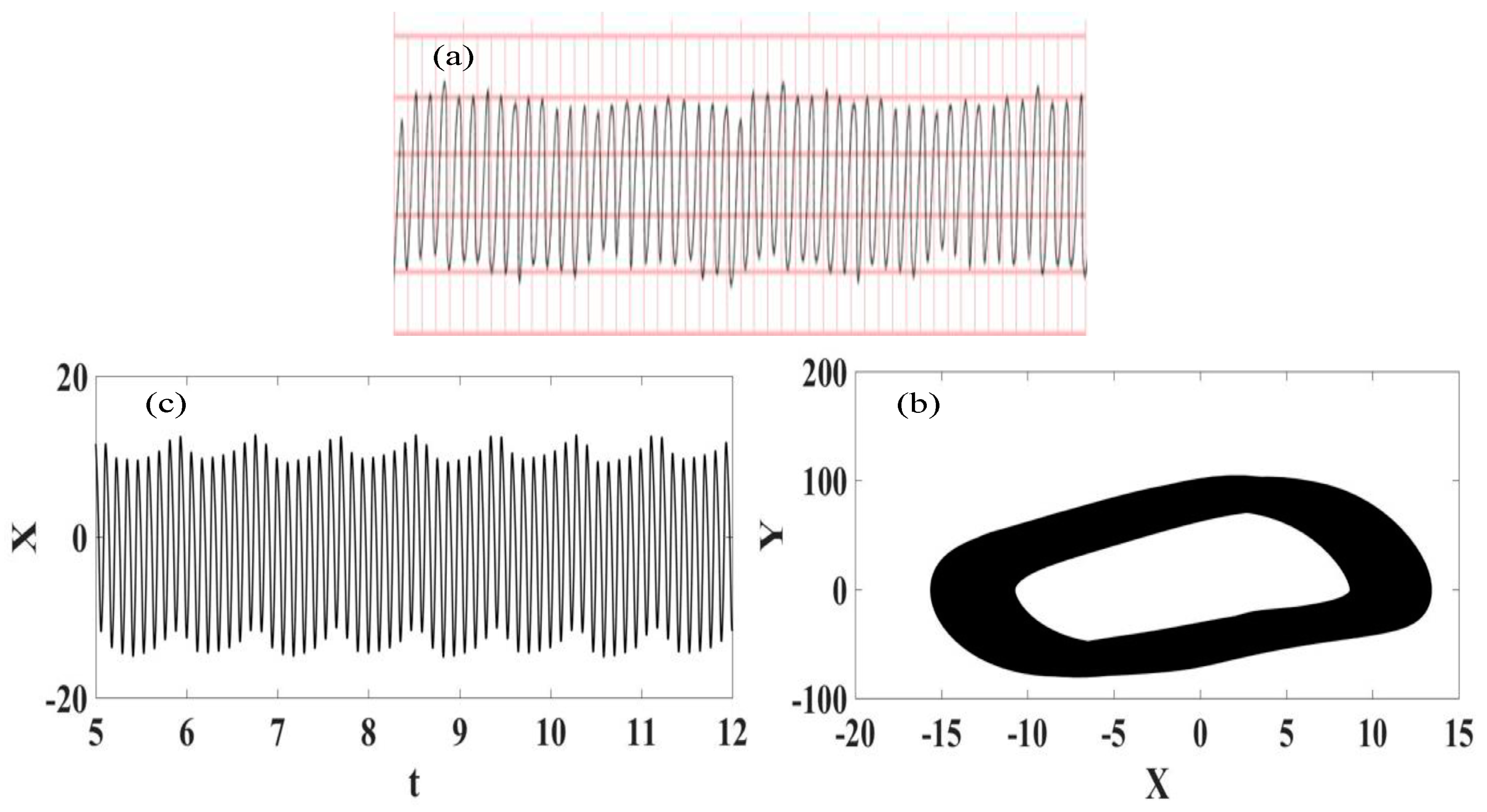
4.2. Torsade de Pointe (TdP)
4.3. Atrial Flutter
4.4. Atrial Fibrillation
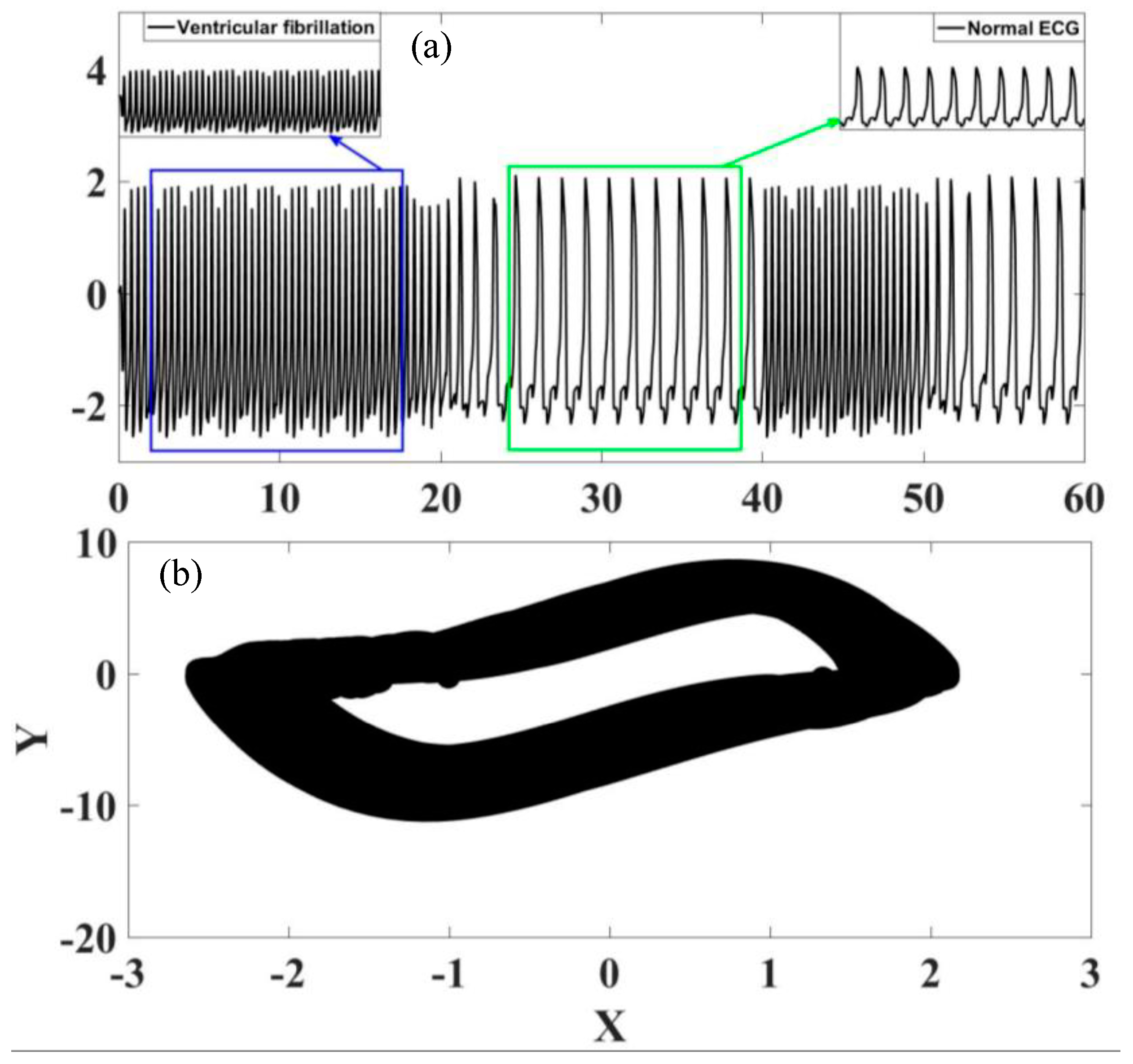
4.5. Ventricular Fibrillation
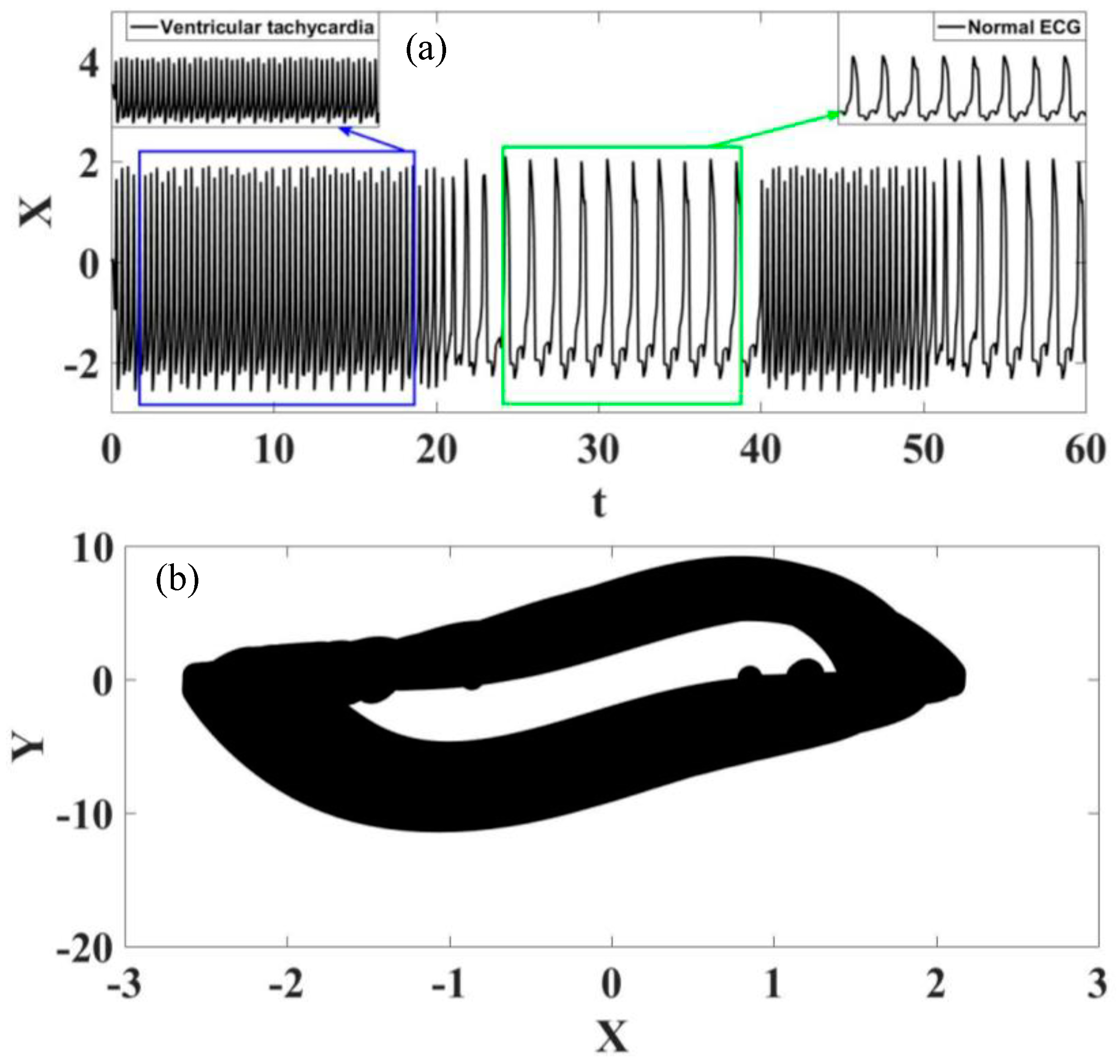
4.6. Polymorphic Ventricular Tachycardia
4.7. Supraventricular Extrasystole
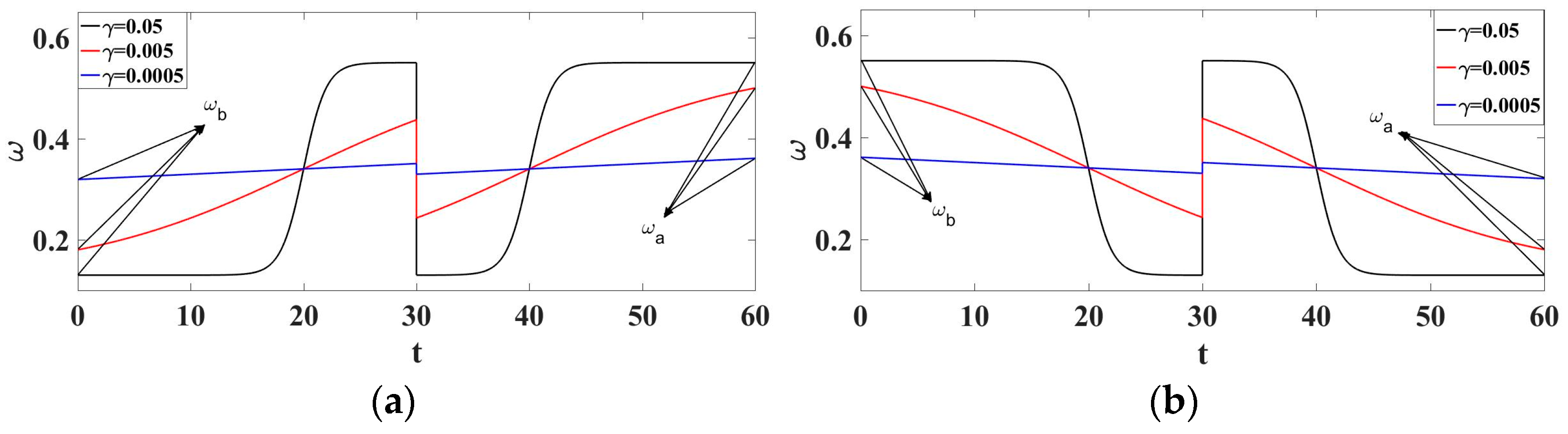
5. Frequency Transition State
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nazari, S.; Heydari, A.; Khaligh, J. Modified modeling of the heart by applying nonlinear oscillators and designing an appropriate control signal. Appl. Math. 2013, 4, 972–978. [Google Scholar] [CrossRef]
- Cheffer, A.; Savi, M.A.; Pereira, T.L.; de Paula, A.S. Heart rhythm analysis using a nonlinear dynamics perspective. Appl. Math. Model. 2021, 96, 152–176. [Google Scholar] [CrossRef]
- Fonkou, R.F.; Kengne, R.; Fotsing Kamgang, H.C.; Talla, P.K. Dynamical behavior analysis of the heart system by the bifurcation structures. Heliyon 2023, 9, e12887. [Google Scholar] [CrossRef] [PubMed]
- Cheffer, A.; Savi, M.A. Analysis of Cardiovascular Rhythms Using Mathematical Models. J. Cardiovasc. Med. 2021, 5, 022. [Google Scholar]
- La Sala, L.; Crestani, M.; Garavelli, S.; de Candia, P.; Pontiroli, A.E. Does microRNA disruption control the mechanisms linking obesity and diabetes? Implications for cardiovascular risk. Int. J. Mol. Sci. 2021, 22, 143. [Google Scholar] [CrossRef]
- Britin, S.N.; Britina, M.A.; Vlasenko, R.Y. Cardiac Conduction System: A Generalized Electrical Model. Biomed. 2021, 55, 41–45. [Google Scholar] [CrossRef]
- Savi, M.A. Chaos and order in biomedical rhythms. J. Braz. Soc. Mech. Sci. Eng. 2005, 27, 157–169. [Google Scholar] [CrossRef]
- Cheffer, A.; Savi, M.A. Biochaos in Cardiac Rhythms. Eur. Phys. J. Spec. Top. 2022, 231, 833–8455. [Google Scholar] [CrossRef]
- Ferreira, B.B.; Savi, M.A.; De Paula, A.S. Chaos control applied to cardiac rhythms represented by ECG signals. Phys. Scr. 2014, 89, 105203. [Google Scholar] [CrossRef]
- Baleanu, D.; Sajjadi, S.S.; Asad, J.H.; Jajarmi, A.; Estiri, E. Hyperchaotic behaviors, optimal control and synchronization of a non-autonomous cardiac conduction system. Adv. Differ. Equ. 2021, 2021, 157. [Google Scholar] [CrossRef]
- De Almeida, M.C.; Shumpei Mori, S.; Anderson, R.H. Three-dimensional visualization of the bovine cardiac conduction system and surrounding structures compared with arrangements in the human heart. J. Anat. 2021, 238, 1359–1370. [Google Scholar] [CrossRef]
- Adrian, E.D.; Bronk, D.W. Discharges in mammalian sympathetic nerves. J. Physiol. 1932, 2, 115–133. [Google Scholar] [CrossRef] [PubMed]
- Park, D.S.; Fishman, G.I. The cardiac conduction system. Circulation 2011, 8, 904–915. [Google Scholar] [CrossRef]
- Poon, C.-S.; Barahona, M. Titration of chaos with added noise. Proc. Natl. Acad. Sci. USA 2001, 98, 7107–7112. [Google Scholar] [CrossRef] [PubMed]
- Radhakrishna, R.K.A.; Dutt, D.N.; Yeragan, V.K. Nonlinear measures of heart rate time series: Influence of posture and controlled breathing. Auton. Neurosci. Basic Clin. 2000, 83, 148–158. [Google Scholar] [CrossRef] [PubMed]
- Van der Schouw, Y.T.; Van der Graaf, Y.; Steyerberg, E.W.; Eijkemans, M.J.C.; Banga, J.D. Age at menopause as a risk factor for cardiovascular mortality. Lancet 1996, 347, 714–718. [Google Scholar] [CrossRef]
- Venkat Narayan, K.M.; Thompson, T.J.; Boyle, J.P.; Beckles, G.L.A.; Engelgau, M.M.; Vinicor, F.; Williamson, D.F. The use of population attributable risk to estimate the impact of prevention and early detection of type 2 diabetes on population-wide mortality risk in US males. Health Care Manag. Sci. 1999, 2, 223–227. [Google Scholar] [CrossRef]
- Wåhlin, A.; Nyberg, L. At the Heart of Cognitive Functioning in Aging. Trends Cogn. Sci. 2019, 23, 717–720. [Google Scholar] [CrossRef]
- Santos, A.M.; Lopes, S.R.; Viana, R.L. Rhythm synchronization and chaotic modulation of coupled Van der Pol oscillators in a model for the heartbeat. Phys. A 2004, 338, 335–355. [Google Scholar] [CrossRef]
- Fonkou, R.F.; Louodop, P.; Talla, P.K. Dynamic behaviour of the cardiac conduction system under external disturbances: Simulation based on microcontroller technology. Phys. Scr. 2022, 97, 025001. [Google Scholar] [CrossRef]
- Richter, Y.; Edelman, E.R. Cardiology Is Flow. Circulation 2006, 113, 2679–2682. [Google Scholar] [CrossRef] [PubMed]
- Kik, M.J.L.; Path, D.V.; Mitchell, M.A. Reptile cardiology: A review of anatomy and physiology, diagnostic approaches, and clinical disease. Semin. Avian Exot. Pet Med. 2005, 14, 52–60. [Google Scholar] [CrossRef]
- Plonsey, R.; Barr, R.C. Mathematical modeling of electrical activity of the heart. J. Electrocardiol. 1987, 20, 219–226. [Google Scholar] [CrossRef]
- Banerjee, A.; Newman, D.R.; Van den Bruel, A.; Heneghan, C. Diagnostic accuracy of exercise stress testing for coronary artery disease: A systematic review and meta-analysis of prospective studies. Int. J. Clin. Pract. 2012, 66, 477–492. [Google Scholar] [CrossRef]
- Wiener, D.A.; Ryan, T.J.; McCabe, C.H.; Ward Kennedy, J.; Schloss, M.; Tristani, F.; Chaitman, B.R.; Fisher, L.D. Exercise Stress Testing—Correlations among History of Angina, ST-Segment Response and Prevalence of Coronary-Artery Disease in the Coronary Artery Surgery Study (CASS). N. Engl. J. Med. 1979, 301, 230–235. [Google Scholar] [CrossRef]
- Bonow, R.O.; Carabello, B.A.; Chatterjee, K.; de Leon, A.C., Jr.; Faxon, D.P.; Freed, M.D.; Gaasch, W.H.; Lytle, B.W.; Nishimura, R.A.; O’Gara, P.T.; et al. 2008 Focused Update Incorporated Into the ACC/AHA 2006 Guidelines for the Management of Patients With Valvular Heart Disease. Circulation 2008, 118, e523–e661. [Google Scholar] [CrossRef]
- Fonkou, R.F.; Louodop, P.; Talla, P.K. Nonlinear oscillators with variable state damping and elastic coefficients. Pramana J. Phys. 2021, 95, 210. [Google Scholar] [CrossRef]
- Bozkurt, S. Mathematical modeling of cardiac function to evaluate clinical cases in adults and children. PLoS ONE 2019, 14, e0224663. [Google Scholar] [CrossRef]
- Barrio, R.; Shilnikov, A. Parameter-sweeping techniques for temporal dynamics of neuronal systems: Case study of Hindmarsh-Rose model. J. Math. Neurosci. 2011, 1, 6. [Google Scholar] [CrossRef]
- Traver, J.E.; Nuevo-Gallardo, C.; Tejado, I.; Fernández-Portales, J.; Ortega-Morán, J.F.; Pagador, J.B.; Vinagre, B.M. Cardiovascular Circulatory System and Left Carotid Model: A Fractional Approach to Disease Modeling. Fractal Fract. 2022, 6, 64. [Google Scholar] [CrossRef]
- Suchorsky, M.; Rand, R. Three Oscillator of the heartbeat generator. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 2434–2449. [Google Scholar] [CrossRef]
- Hodgkin, A.L.; Huxley, A.F. A quantitative description of membrane current and its application to conduction and excitation in nerve. J. Physiol. 1952, 117, 500–544. [Google Scholar] [CrossRef] [PubMed]
- FitzHugh, R. Impulses and physiological states in theorical models of nerve membrane. Biophys. J. 1961, 1, 445–466. [Google Scholar] [CrossRef]
- Nagumo, J.; Arimoto, S.; Yoshizawa, S. An active pulse transmission line simulating nerve axon. Proc. IRE 1962, 50, 2061–2070. [Google Scholar] [CrossRef]
- Kuate, G.C.G.; Fotsin, H.B. On the nonlinear dynamics of a cardiac electrical conduction system model: Theoretical and experimental study. Phys. Scr. 2022, 97, 045205. [Google Scholar] [CrossRef]
- Moe, G.K.; Rheinboldt, W.C.; Abildskov, J.A. A computer model of atrial fibrillation. Am. Heart J. 1964, 67, 200–220. [Google Scholar] [CrossRef]
- Krinsky, V.I. Mathematical models of cardiac arrhythmias (spiral waves). Pharmacol. Ther. Part B 1978, 3, 539–555. [Google Scholar] [CrossRef]
- Fonkou, R.F.; Louodop, P.; Talla, P.K.; Woafo, P. Analysis of the dynamics of new models of nonlinear systems with state variable damping and elastic coefficients. Heliyon 2022, 8, e10112. [Google Scholar] [CrossRef]
- Fonkou, R.F.; Louodop, P.; Talla, P.K.; Woafo, P. Van der Pol equation with sinusoidal nonlinearity: Dynamic behaviour and real-time control of a target trajectory. Phys. Scr. 2021, 96, 125203. [Google Scholar] [CrossRef]
- Krstacic, G.; Krstacic, A.; Smalcelj, A.; Milicic, D.; Jembrek-Gostovic, M. The chaos theory and nonlinear dynamics in heart rate variability analysis: Does it work in short-time series in patients with coronary heart disease? Ann. Noninvasive Electrocardiol. 2007, 12, 130–136. [Google Scholar] [CrossRef]
- Ernst, J.; Bar-Joseph, Z. STEM: A tool for the analysis of short time series gene expression data. BMC Bioinform. 2006, 7, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Tobón, D.P.; Jayaraman, S.; Falk, T.H. Spectro-temporal electrocardiogram analysis for noise-robust heart rate and heart rate variability measurement. IEEE J. Transl. Eng. Health Med. 2017, 5, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Shiraishi, Y.; Katsumata, Y.; Sadahiro, T.; Azuma, K.; Akita, K.; Isobe, S.; Yashima, F.; Miyamoto, K.; Nishiyama, T.; Tamura, Y.; et al. Real-time analysis of the heart rate variability during incremental exercise for the detection of the ventilatory threshold. J. Am. Heart Assoc. 2018, 7, e006612. [Google Scholar] [CrossRef]
- Wang, Y.; Wei, S.; Zhang, S.; Zhang, Y.; Zhao, L.; Liu, C.; Murray, A. Comparison of time-domain, frequency-domain and non-linear analysis for distinguishing congestive heart failure patients from normal sinus rhythm subjects. Biomed. Signal Process. Control. 2018, 42, 30–36. [Google Scholar] [CrossRef]
- Hu, B.; Wei, S.; Wei, D.; Zhao, L.; Zhu, G.; Liu, C. Multiple time scales analysis for identifying congestive heart failure based on heart rate variability. IEEE Access 2019, 7, 17862–17871. [Google Scholar] [CrossRef]
- Ueno, H.; Totoki, Y.; Matsuo, T. ECG characterization of sinus bradycardia and ventricular flutter using malthusian parameter and recurrence plot. ICIC Express Lett. Part B Appl. Int. J. Res. Surv. 2018, 9, 23–30. [Google Scholar]
- Costa, M.D.; Goldberger, A.L. Heart rate fragmentation: Using cardiac pacemaker dynamics to probe the pace of biological aging. Am. J. Physiol. Heart Circ. Physiol. 2019, 316, H1341–H1344. [Google Scholar] [CrossRef]
- Van Der Pol, B. On relaxation-oscillations. Philos. Mag. J. Sci. Ser. 1926, 2, 978–992. [Google Scholar] [CrossRef]
- Van Der Pol, B.; Van Der Mark, J. The heartbeat considered as a relaxation oscillation, and an electrical model of the heart. Philos. Mag. J. Sci. Ser. 1928, 6, 763–775. [Google Scholar] [CrossRef]
- Grudzinski, K.; Zebrowski, J.J. Modeling cardiac pacemakers with relaxation oscillators. Phys. A 2004, 336, 153–162. [Google Scholar] [CrossRef]
- Gois, S.R.S.M.; Savi, M.A. An analysis of heart rhythm dynamics using a three-coupled oscillator model. Chaos Solitons Fractals 2009, 41, 2553–2565. [Google Scholar] [CrossRef]
- Cheffer, A.; Savi, M.A. Random effects inducing heart pathological dynamics: An approach based on mathematical models. Biosystems 2020, 196, 104–177. [Google Scholar] [CrossRef] [PubMed]
- Cheffer, A.; Ritto, T.G.; Savi, M.A. Uncertainty analysis of heart dynamics using Random Matrix Theory. Int. J. Non-Linear Mech. 2021, 129, 103653. [Google Scholar] [CrossRef]
- Waldmann, V.; Marijon, E. Troubles du rythme cardiaque: Diagnostic et prise en charge. Cardiac arrhythmias: Diagnosis and management. La Rev. De Médecine Interne 2016, 37, 608–615. [Google Scholar] [CrossRef] [PubMed]
- Kim, Y.G.; Choi, Y.Y.; Han, K.D.; Min, K.; Choi, H.Y.; Shim, J.; Choi, J.I.; Kim, Y.H. Atrial fibrillation is associated with increased risk of lethal ventricular arrhythmias. Sci. Rep. 2021, 11, 18111. [Google Scholar] [CrossRef]
- Carrarini, C.; Di Stefano, V.; Russo, M.; Dono, F.; Di Pietro, M.; Furia, N.; Onofrj, M.; Bonanni, L.; Faustino, M.; De Angelis, M.V. ECG monitoring of post-stroke occurring arrhythmias: An observational study using 7-day Holter ECG. Sci. Rep. 2022, 12, 228. [Google Scholar] [CrossRef]
- PhysioNet Databases. 2020. Available online: https://physionet.org/about/database/ (accessed on 10 December 2020).
- Niimi, N.; Yuki, K.; Zaleski, K. Long QT Syndrome and Perioperative Torsades de Pointes: What the Anesthesiologist Should Know. J. Cardiothorac. Vasc. Anesth. 2022, 36, 286–302. [Google Scholar] [CrossRef]
- Diamant, M.J.; Andrade, J.G.; Virani, S.A.; Jhund, P.S.; Petrie, M.C.; Hawkins, N.M. Heart failure and atrial flutter: A systematic review of current knowledge and practices. ESC Heart Fail. 2021, 8, 4484–4496. [Google Scholar] [CrossRef]
- Davies, M.J.; Pomerance, A. Pathology of Atrial fibrillation in man. Br. Heart J. 1972, 34, 520–525. [Google Scholar] [CrossRef]
- Wiggers, C.J. The mechanism and nature of ventricular fibrillation. Am. Heart J. 1940, 20, 399–412. [Google Scholar] [CrossRef]
- Viskin, S.; Chorin, E.; Viskin, D.; Hochstadt, A.; Schwartz, A.L.; Rosso, R. Polymorphic Ventricular Tachycardia: Terminology, Mechanism, Diagnosis, and Emergency Therapy. Circulation 2021, 144, 823–839. [Google Scholar] [CrossRef] [PubMed]


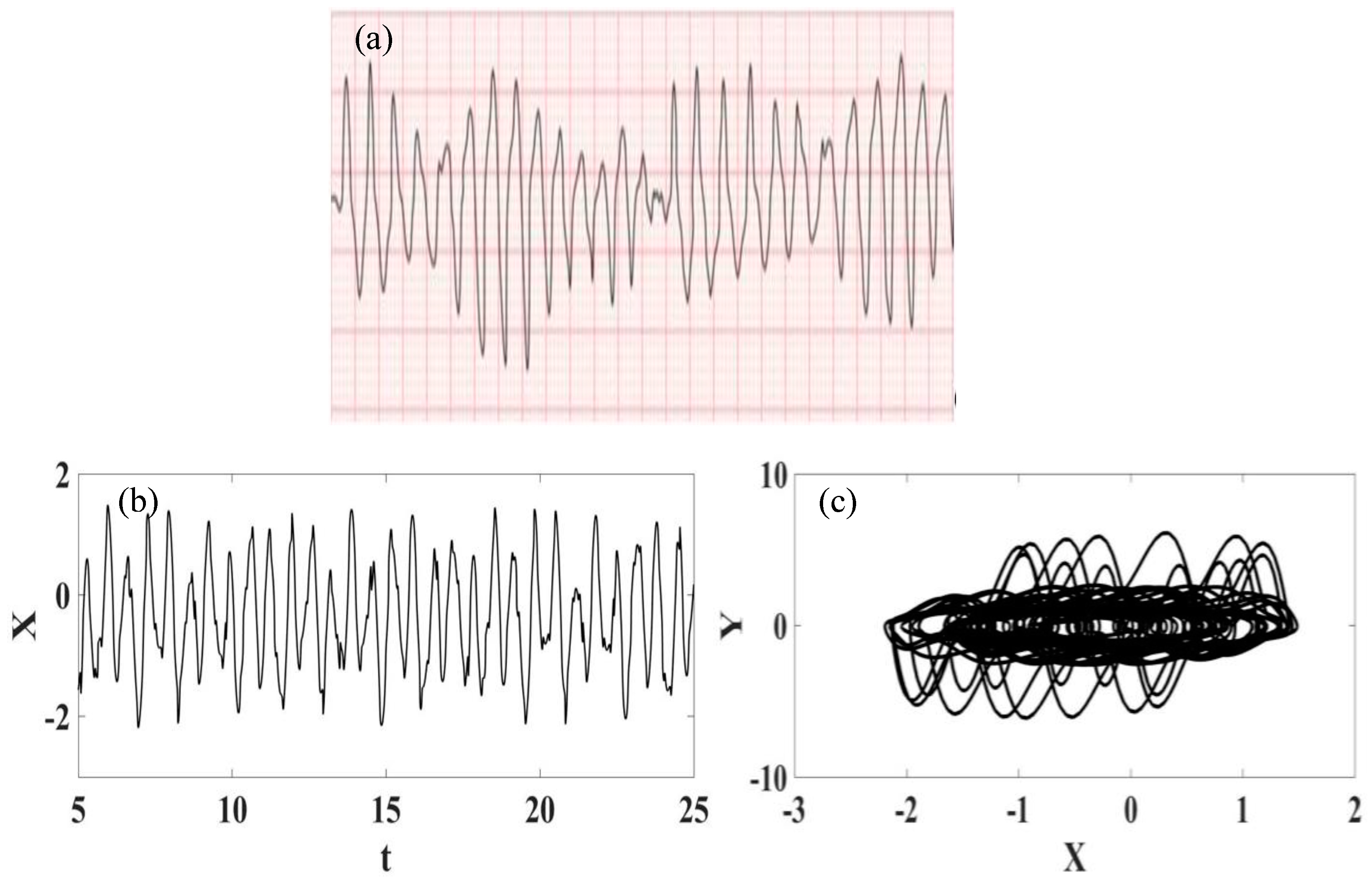
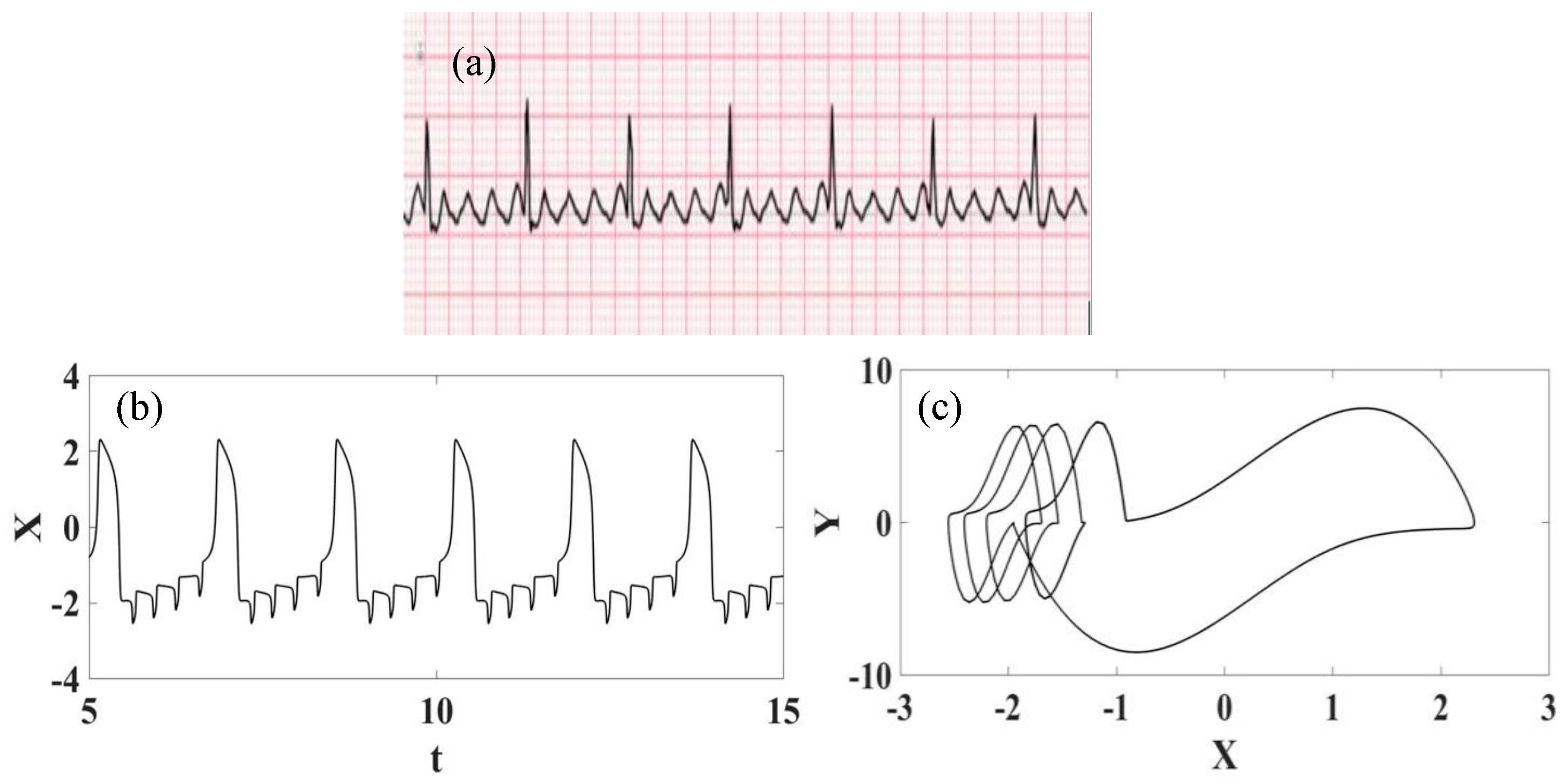
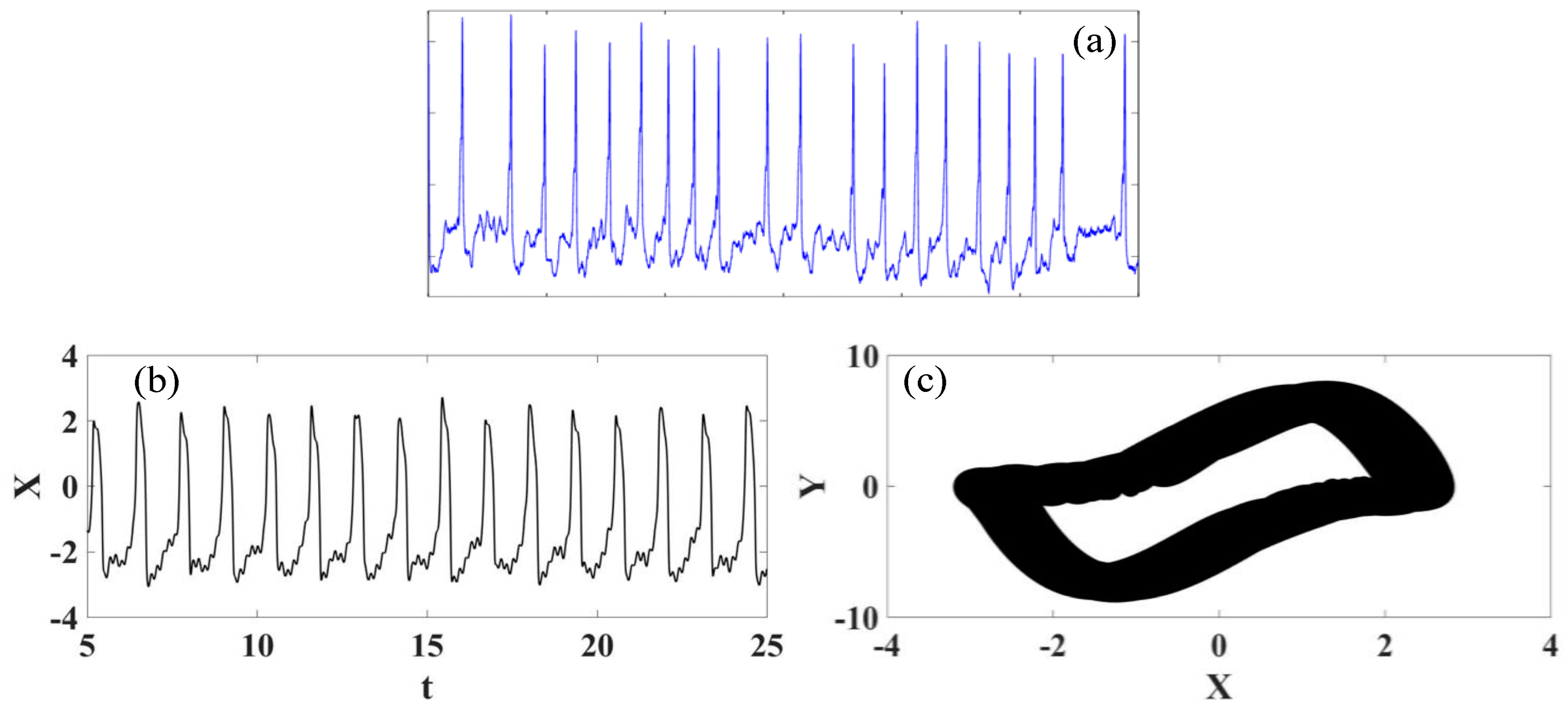



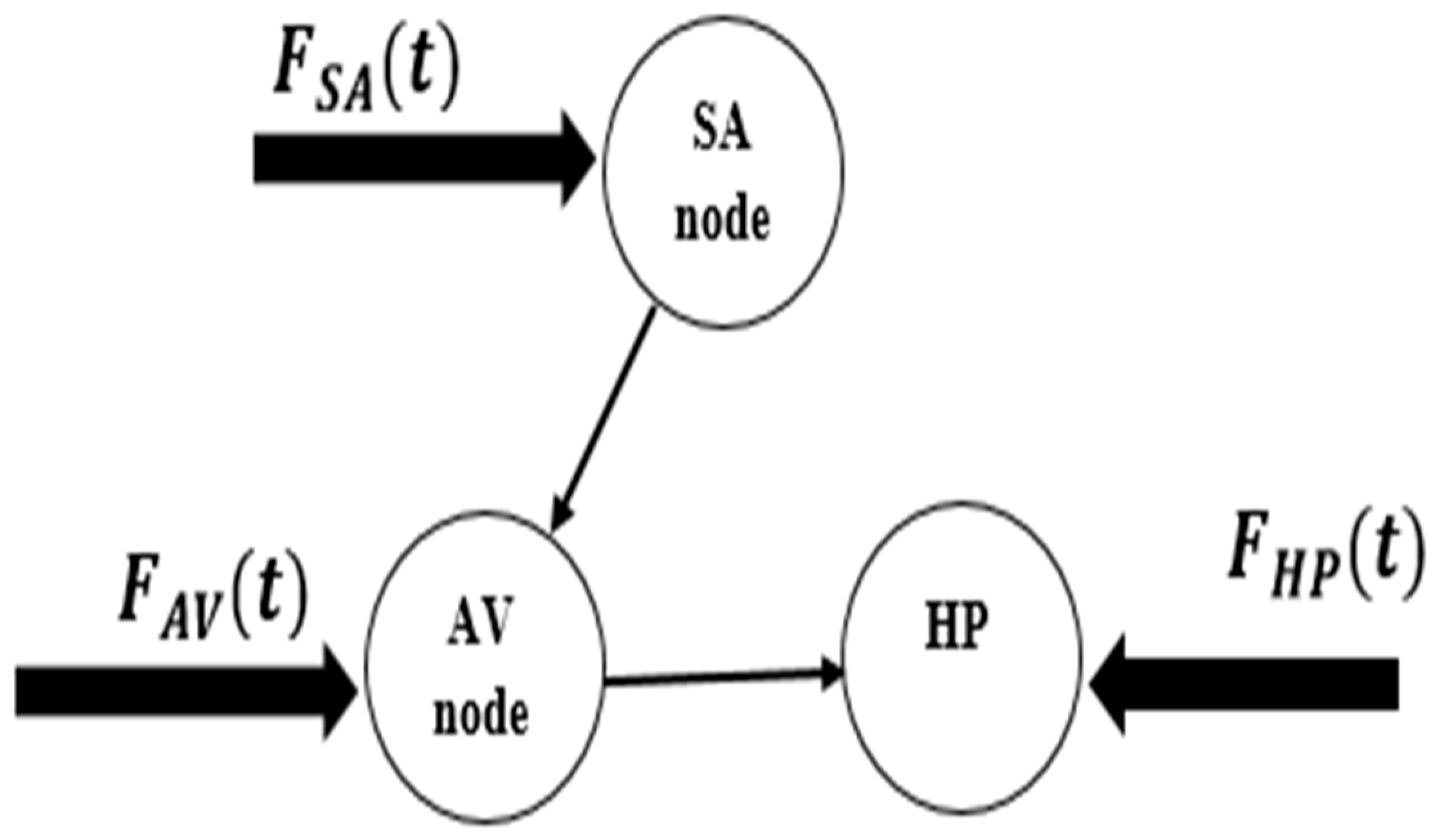

| Coupling Parameters | SA Oscillator | AV Oscillator | HP Oscillator | |
|---|---|---|---|---|
| SA Oscillator | AV Oscillator | HP Oscillator | External Stimuli | Coupling Terms |
|---|---|---|---|---|
| Ventricular flutter | ||||
| Torsade de Pointe | ||||
| Atrial flutter | ||||
| Atrial fibrillation | ||||
| Ventricular fibrillation | ||||
| Polymorphic ventricular tachycardia | ||||
| Supraventricular extrasystole | ||||
| Behavior of the Cardiac System | Transition from Normal Rhythm to Atrial Flutter | Transition from Normal Rhythm to Ventricular Flutter | Transition from Ventricular Fibrillation to Normal Rhythm | Transition from Ventricular Tachycardia to Normal Rhythm |
|---|---|---|---|---|
| ωb | 1.0 | 1.0 | 6.0 | 7.8 |
| ωa | 0.4 | 35.0 | 0.95 | 0.85 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fonkou, R.F.; Savi, M.A. Heart Rhythm Analysis Using Nonlinear Oscillators with Duffing-Type Connections. Fractal Fract. 2023, 7, 592. https://doi.org/10.3390/fractalfract7080592
Fonkou RF, Savi MA. Heart Rhythm Analysis Using Nonlinear Oscillators with Duffing-Type Connections. Fractal and Fractional. 2023; 7(8):592. https://doi.org/10.3390/fractalfract7080592
Chicago/Turabian StyleFonkou, Rodrigue F., and Marcelo A. Savi. 2023. "Heart Rhythm Analysis Using Nonlinear Oscillators with Duffing-Type Connections" Fractal and Fractional 7, no. 8: 592. https://doi.org/10.3390/fractalfract7080592
APA StyleFonkou, R. F., & Savi, M. A. (2023). Heart Rhythm Analysis Using Nonlinear Oscillators with Duffing-Type Connections. Fractal and Fractional, 7(8), 592. https://doi.org/10.3390/fractalfract7080592







