On a System of Equations with General Fractional Derivatives Arising in Diffusion Theory

Abstract

A novel two-compartment model for drug release was formulated. The general fractional derivatives of a specific type and distributed order were used in the formulation. Earlier used models in pharmacokinetics with fractional derivatives follow as special cases of the model proposed here. As a first application, we used this model to study the release of gentamicin from poly(vinyl alcohol)/chitosan/gentamicin (PVA/CHI/Gent) hydrogel aimed at wound dressing in the medical treatment of deep chronic wounds. As a second application, we studied the release of gentamicin from antibacterial biodynamic hydroxyapatite/poly(vinyl alcohol) /chitosan/gentamicin (HAP/PVA/CS/Gent) coating on a titanium substrate for bone tissue implants, which enables drug delivery directly to the infection site. In both cases. a good agreement is obtained between the measured data and the data calculated from the model proposed here. The form of the general fractional derivatives used here results in an additional parameter in the compartmental model used here. This, as a consequence, leads to a better approximation of the experimental data with only a slightly more complicated numerical procedure in obtaining the solution.

1. Introduction

Compartmental models are often used as a standard method in pharmacokinetics [1]. They have been applied in various drug delivery systems [2,3,4,5,6,7,8,9,10,11,12,13,14,15,16]. Since both Riemann–Liouville and Caputo fractional derivatives are non local linear operators, they are also applied in many problems in physics, mechanics and biology [17,18,19,20,21], where memory or action on distance effects is important. The concept of general fractional calculus (GFC) has recently gained increasing interest. We refer to the work of Tarasov [22], who systematically presented the generalization of the Riemann–Liouville and Caputo fractional integrals and derivatives, as well as to the papers by A. N. Kochubei [23], Y. Luchko [24,25] and Yang [17,18]. The generalization of GFC that includes both the general fractional derivatives of arbitrary order of the Riemann–Liouville type and the regularized general fractional derivatives of arbitrary order was recently presented in [26].

We give the basic definition of GFC that is needed for our analysis. The main idea of the GFC concept is, as in the case of Riemann–Liouville and Caputo fractional derivatives, to describe dynamical systems with non-locality in time and space but in a more general way.

The definitions of a general fractional integral, general fractional derivative and general fractional derivative of Caputo type are [22,24,27].

$$\begin{array}{ccc}\hfill I_{\left(M\right)}^t\left[\tau \right]f\left(\tau \right)& =& \left(M\ast f\right)\left(t\right)={\int }_0^{t}M\left(t-\tau \right)f\left(\tau \right)d\tau ,\hfill \\ \hfill D_{\left(K\right)}^t\left[\tau \right]f\left[\tau \right]& =& \frac{d}{dt}\left(K\ast f\right)\left(t\right)=\frac{d}{dt}{\int }_0^{t}K\left(t-\tau \right)f\left(\tau \right)d\tau ,\hfill \\ \hfill {}^C{D}_{\left(K\right)}^t\left[\tau \right]f\left[\tau \right]& =& \left(K\ast f^{\left(1\right)}\right)\left(t\right)={\int }_0^{t}K\left(t-\tau \right)f^{\left(1\right)}\left(\tau \right)d\tau ,t>0.\hfill \end{array}$$

(1)

In (1), we use $f^{\left(1\right)}\left(t\right)=\frac{df}{dt}$ to denote the first derivative and $f\ast g$ to denote the convolution of the functions f and g. Further, the first equation in (1) is the definition of the integral in GFC, while the second and third equations in (1) define the general fractional derivatives of the Riemann–Liouville type and general fractional derivative of the Caputo type, respectively. In addition, we denote by M and K in (1) the kernels. They are functions of the Sonine type. We recall now the definition of the Sonine kernels. Suppose that $a<b$. Let

$$C_{\left(a,b\right)}\left(0,\infty \right)=\left\{f:f\left(t\right)=t^{p}Y\left(t\right),a<p<b,Y\left(t\right)\in C[0,\infty )\right\}.$$

(2)

In (2), we denote by $C[0,\infty )$ the space of continuous functions on $[0,\infty )$. Then, the set of Sonine kernels $S_{-1}$ is defined as a set of pairs of functions $\left(M\left(t\right),K\left(t\right)\right)$ such that $M\left(t\right),K\left(t\right)\in C_{\left(-1,0\right)}\left(0,\infty \right),$ and $\left(M\left(t\right),K\left(t\right)\right)$ satisfy the following equation:

$$\left(M\ast K\right)\left(t\right)={\int }_0^{t}M\left(t-\tau \right)K\left(\tau \right)d\tau =1,t\ge 0.$$

(3)

Then, $\left(M\left(t\right),K\left(t\right)\right)$ is called a Sonine pair. It could be shown [28] that, under certain conditions,

$$\underset{t\to 0}{lim}tM\left(t\right)=0,\underset{t\to 0}{lim}tK\left(t\right)=0,$$

and

$${\int }_0^t{M}^{\left(1\right)}\left(\tau \right)\left[K\left(t\right)-K\left(t-\tau \right)\right]d\tau =K\left(t\right)M\left(t\right),$$

(4)

for all $t>0$, where $M^{\left(1\right)}$ and $K^{\left(1\right)}$ exist. The condition (3) provides the central condition of GFC, which reads

$$\begin{array}{ccc}\hfill D_{\left(K\right)}^t{I}_{\left(M\right)}^t\left[\tau \right]f\left(\tau \right)& =& f\left(t\right),\hfill \\ \hfill I_{\left(M\right)}^t{D}_{\left(K\right)}^t\left[\tau \right]f\left[\tau \right]& =& f\left(t\right)-f\left(0\right),t>0.\hfill \end{array}$$

(5)

For the importance of (5), see [23,29]. In addition, (3) leads to

$$s\tilde{M}\left(s\right)\tilde{K}\left(s\right)=1.$$

(6)

In (6), we use $\widetilde{(·)}$ to denote Laplace transform. Equation (6) may be used to determine K if M is given and vice versa; see [23]. Note that K and M may interchange roles in (1).

The Riemann–Liouville fractional calculus follows from GFC as a special case if we choose

$$M\left(t\right)=\frac{t^{\alpha -1}}{\mathrm{\Gamma }\left(\alpha \right)},K\left(t\right)=\frac{t^{-\alpha }}{\mathrm{\Gamma }\left(1-\alpha \right)},t>0.$$

It is easy to show that (3), (4) and (6) are satisfied by this choice of M and K. Next, consider the Sonine pair, proposed by Zacher [22,30]. It reads

$$\begin{array}{ccc}\hfill M\left(t\right)& =& \left[\frac{t^{\alpha -1}}{\mathrm{\Gamma }\left(\alpha \right)}exp\left(-\mu t\right)+\mu {\int }_0^t\frac{{\tau }^{\alpha -1}}{\mathrm{\Gamma }\left(\alpha \right)}exp\left(-\mu \tau \right)d\tau \right],\hfill \\ \hfill K\left(t\right)& =& \frac{t^{-\alpha }}{\mathrm{\Gamma }\left(1-\alpha \right)}exp\left(-\mu t\right),t\ge 0,\hfill \end{array}$$

(7)

where $\alpha \in \left(0,1\right),\mu \ge 0$ and $\mathrm{\Gamma }$ is the Euler gamma function [31]. The Laplace transforms, i.e.,

$$\mathcal{L}\left(f\right)\left(s\right)=\tilde{f}\left(s\right)={\int }_0^{\infty }exp(-ts)f\left(t\right)dt,\left|f\left(t\right)\right|\le Cexp\left(Bt\right),C>0,s\in \mathbb{C},\Re s>B,$$

of the functions $M_1$ and $K_1$ are

$$\tilde{M}\left(s\right)=\frac{1}{{\left(s+\mu \right)}^{\alpha }}\left(1+\frac{\mu }{s}\right),\tilde{K}\left(s\right)=\frac{1}{{\left(s+\mu \right)}^{1-\alpha }},\Re s>0,$$

(8)

$\Re s$ denotes the real part of s. We shall formulate a two-compartment model of pharmacokinetics with a general fractional derivative with kernel $K_1$ and distributed-order general fractional derivative with kernel $K_1$.

In this paper, we shall continue the analysis of the applicability of GFC that we started in our previously published papers [14,32].

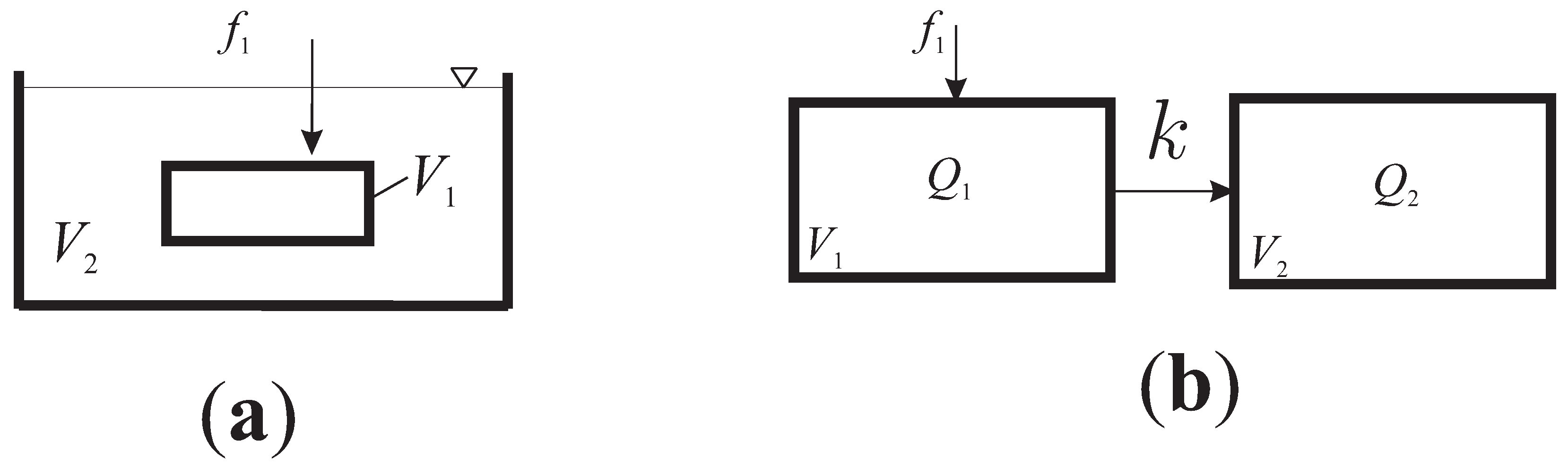
2. Two-Compartment System with General Fractional Derivatives

We recall the kinetics of a classical two-compartment model—see [1]—of pharmacokinetics, with different volumes of compartments. In Figure 1a, we show the physical model that we used, while, in Figure 1b, we show the corresponding two-compartment model.

From [1], we write

$$\begin{array}{ccc}\hfill \frac{d{m}_1}{dt}& =& -k\left[\frac{m_1\left(t\right)}{V_1}-\frac{m_2\left(t\right)}{V_2}\right]+f_1\left(t\right),\hfill \\ \hfill \frac{d{m}_2}{dt}& =& k\left[\frac{m_1\left(t\right)}{V_1}-\frac{m_2\left(t\right)}{V_2}\right],\hfill \end{array}$$

(9)

where $m_i,V_i,i=1,2$ represent the mass and volume of the compartments 1 and 2, respectively, $k>0$ is a constant connected with the diffusion coefficient and $f_1$ is the mass intake in the compartment 1. Recall that, in a compartmental system, perfect mixing is assumed, i.e., there is no spatial dependence of the masses/concentrations in the compartments. The connection between k and the diffusion coefficient D may me derived as follows. It is easily seen that, in (9), $dim\left[k\right]=\frac{L^3}{s}$, where L is the unit length. Since the diffusion coefficient is defined as the amount of a particular substance that diffuses across a unit area in 1 s under the influence of a gradient of one unit, we first define the gradient in (9) as $\left[\frac{m_1\left(t\right)}{V_1}-\frac{m_2\left(t\right)}{V_2}\right]\frac{1}{\delta },$ with $dim\left[\delta \right]=L$. Then, (9) becomes

$$\begin{array}{ccc}\hfill \frac{d{m}_1}{dt}& =& -k\delta \left[\frac{m_1\left(t\right)}{V_1}-\frac{m_2\left(t\right)}{V_2}\right]\frac{1}{\delta }+f_1\left(t\right),\hfill \\ \hfill \frac{d{m}_2}{dt}& =& k\delta \left[\frac{m_1\left(t\right)}{V_1}-\frac{m_2\left(t\right)}{V_2}\right]\frac{1}{\delta }.\hfill \end{array}$$

Let A denote the area through which the diffusion takes place. Then, $dim\left[D\right]=\frac{k\delta }{A}=\frac{L^{3}L}{s{L}^2}=\frac{L^2}{s}$. We shall use this expression in calculating D from the values of k. Note that the characteristic length $\delta$ that transforms the difference in the concentrations on the right hand side of (9) to the form of the gradient of concentrations remains unspecified. To (9), we adjoin the following initial conditions

$$m_1\left(0\right)=m_{10},m_2\left(0\right)=m_{20}.$$

(10)

We generalize (9) by introducing the distributed-order general fractional derivatives. First, by using the second equation in (7) in the second equation in (1), we obtain the general fractional derivative as

$${}_0^C{D}_t^{\alpha ,\lambda }f\left(t\right)=\frac{1}{\mathrm{\Gamma }\left(1-\alpha \right)}{\int }_0^t\frac{exp\left(-\lambda \tau \right)}{{\tau }^{\alpha }}{f}^{\left(1\right)}\left(t-\tau \right)d\tau ,t>0.$$

(11)

With (11), we define a distributed-order general fractional derivative as

$${}_0^C{\overline{D}}_t^{\varphi ,\lambda }f\left(t\right)={\int }_0^1\varphi {\left(\gamma \right)}_0^C{D}_t^{\gamma ,\lambda }f\left(t\right)d\gamma ,$$

(12)

where $\varphi >0,\gamma \in \left[0,1\right]$ is the weight function, or distribution. The values of the weight function specify the importance of the $\gamma$-th fractional derivative in the diffusion process. It may be connected with the characteristic time of the compartment; see [15].

The weighting function must have dimension ${[dimt]}^{\gamma }$ in order to obtain dimensional homogeneity. Note that, for

$$\varphi \left(\gamma \right)=\delta \left(\gamma -\beta \right),0\le \gamma \le 1$$

from (12), we obtain

$${}_0^C{\overline{D}}_t^{\varphi ,\lambda }f\left(t\right)={}_0^C{D}_t^{\beta ,\lambda }f\left(t\right).$$

Applying the Laplace transform to (11), it follows that

$$\mathcal{L}\left({}_0^C{\overline{D}}_t^{\varphi ,\lambda }f\left(t\right)\right)\left(s\right)={\int }_0^1\varphi \left(\gamma \right)\mathcal{L}\left({}_0^C{D}_t^{\gamma ,\lambda }f\right)\left(s\right)d\gamma .$$

(13)

Next, we determine $\mathcal{L}\left({}_0^C{D}_t^{\gamma ,\lambda }f\right)\left(s\right)$. In addition, from (11), we obtain

$$\mathcal{L}\left({}_0^C{D}_t^{\gamma ,\lambda }f\right)\left(s\right)=\mathcal{L}\left(\frac{1}{\mathrm{\Gamma }\left(1-\gamma \right)}\frac{exp\left(-\lambda t\right)}{t^{\gamma }}\right)\left(s\right)\mathcal{L}\left(f^{\left(1\right)}\right)\left(s\right).$$

Since

$$\mathcal{L}\left(\frac{1}{\mathrm{\Gamma }\left(1-\alpha \right)}\frac{exp\left(-\lambda t\right)}{t^{\gamma }}\right)\left(s\right)=\frac{1}{{\left(s+\lambda \right)}^{1-\gamma }},\lambda \ge 0,0\le \gamma \le 1,$$

and $\mathcal{L}\left(f^{\left(1\right)}\right)\left(s\right)=s\tilde{f}\left(s\right)-f\left(0\right)$, we obtain

$$\mathcal{L}\left({}_0^C{D}_t^{\gamma ,\lambda }f\right)\left(s\right)=\frac{s}{{\left(s+\lambda \right)}^{1-\gamma }}\tilde{f}\left(s\right)-\frac{1}{{\left(s+\lambda \right)}^{1-\gamma }}f\left(0\right).$$

(14)

Therefore, from (13), it follows that

$${}_0^C{\overline{D}}_t^{\varphi ,\lambda }f\left(t\right)={\mathcal{L}}^{-1}\left[{\int }_0^1\varphi \left(\gamma \right)\left(\frac{s}{{\left(s+\lambda \right)}^{1-\gamma }}\tilde{f}\left(s\right)-\frac{1}{{\left(s+\lambda \right)}^{1-\gamma }}f\left(0\right)\right)d\gamma \right]\left(t\right).$$

(15)

In the applications that follow, we shall choose, for the function $\varphi \left(\gamma \right),\gamma \in \left[0,1\right]$, the following forms:

$${\varphi }_1^a\left(\gamma \right)=a^{\alpha }\delta \left(\gamma -\alpha \right)$$

(16)

where $\alpha$ is given constant, $\delta$ is the Dirac distribution and $0\le \alpha \le 1$. In addition, let

$${\varphi }_2^a\left(\gamma \right)=a^{\gamma }$$

(17)

where a is a constant with a dimension of time. For the case (16), (15) gives

$${}_0^C{\overline{D}}_t^{{\varphi }_1^a,\lambda }f\left(t\right)={\mathcal{L}}^{-1}\left[\frac{a^{{\alpha }_1}s}{{\left(s+\lambda \right)}^{1-\alpha }}\tilde{f}\left(s\right)-\frac{a^{{\alpha }_1}}{{\left(s+\lambda \right)}^{1-\alpha }}f\left(0\right)\right]\left(t\right).$$

(18)

To obtain the expression for ${}_0^C{\overline{D}}_t^{{\varphi }_2^a,\lambda }f\left(t\right)$, when we use (17), we define $K\left(s\right)$ as

$$K\left(s\right)={\int }_0^1\frac{d\gamma }{{\left[a\left(s+\lambda \right)\right]}^{1-\gamma }}=\frac{a\left(s+\lambda \right)-1}{a\left(s+\lambda \right)ln\left[a\left(s+\lambda \right)\right]}.$$

Then, for (17), Equation (15) becomes

$$\begin{array}{ccc}\hfill {}_0^C{\overline{D}}_t^{{\varphi }_2^a,\lambda }f\left(t\right)& =& {\mathcal{L}}^{-1}\left[aK\left(s\right)\left(s\tilde{f}\left(s\right)-f\left(0\right)\right)\right]\left(t\right)\hfill \\ & =& {\mathcal{L}}^{-1}\left[\frac{a\left(s+\lambda \right)-1}{\left(s+\lambda \right)ln\left[a\left(s+\lambda \right)\right]}\left(s\tilde{f}\left(s\right)-f\left(0\right)\right)\right]\left(t\right).\hfill \end{array}$$

(19)

In the formulation of the generalized two-compartment system, we shall use (18) and (19).

We now propose the generalized two-compartment system of pharmacokinetics by replacing the integer-order derivatives on the left hand side of (9) by a linear combination of two general fractional derivatives (18) and a linear combination of general fractional derivatives (18) and (19). Therefore, we define two linear operators as

$$\begin{array}{ccc}\hfill {\mathcal{A}}_{1}f\left(t\right)& =& {}_0^C{\overline{D}}_t^{{\varphi }_1^a,{\lambda }_1}f\left(t\right){+}_0^C{\overline{D}}_t^{{\varphi }_1^b,{\lambda }_2}f\left(t\right)\hfill \\ & =& \frac{1}{\mathrm{\Gamma }\left(1-\alpha \right)}{\int }_0^t{a}^{\alpha }\frac{exp\left(-{\lambda }_1\tau \right)}{{\tau }^{\alpha }}{f}^{\left(1\right)}\left(t-\tau \right)d\tau \hfill \\ & & +\frac{1}{\mathrm{\Gamma }\left(1-\beta \right)}{\int }_0^t{b}^{\beta }\frac{exp\left(-{\lambda }_2\tau \right)}{{\tau }^{\beta }}{f}^{\left(1\right)}\left(t-\tau \right)d\tau \hfill \\ & =& {\mathcal{L}}^{-1}\left[\frac{a^{\alpha }s}{{\left(s+{\lambda }_1\right)}^{1-\alpha }}\tilde{f}\left(s\right)-\frac{a^{\alpha }}{{\left(s+{\lambda }_1\right)}^{1-\alpha }}f\left(0\right)\right]\left(t\right)\hfill \\ & & +{\mathcal{L}}^{-1}\left[\frac{b^{\beta }s}{{\left(s+{\lambda }_2\right)}^{1-\beta }}\tilde{f}\left(s\right)-\frac{b^{\beta }}{{\left(s+{\lambda }_2\right)}^{1-\beta }}f\left(0\right)\right]\left(t\right),\hfill \end{array}$$

(20)

and

$$\begin{array}{ccc}\hfill {\mathcal{A}}_{2}f\left(t\right)& =& {}_0^C{\overline{D}}_t^{{\varphi }_1^a,{\lambda }_1}f\left(t\right)+{}_0^C{\overline{D}}_t^{{\varphi }_2^b,{\lambda }_2}f\left(t\right)\hfill \\ & =& \frac{1}{\mathrm{\Gamma }\left(1-\alpha \right)}{\int }_0^t{a}^{\alpha }\frac{exp\left(-{\lambda }_1\tau \right)}{{\tau }^{\alpha }}{f}^{\left(1\right)}\left(t-\tau \right)d\tau \hfill \\ & & +{\int }_0^1{b}^{\gamma }\left[\frac{1}{\mathrm{\Gamma }\left(1-\gamma \right)}{\int }_0^t\frac{exp\left(-{\lambda }_2\tau \right)}{{\tau }^{\gamma }}{f}^{\left(1\right)}\left(t-\tau \right)d\tau \right]d\gamma \hfill \\ & =& {\mathcal{L}}^{-1}\left[\frac{a^{\alpha }s}{{\left(s+{\lambda }_1\right)}^{1-\alpha }}\tilde{f}\left(s\right)-\frac{a^{\alpha }}{{\left(s+{\lambda }_1\right)}^{1-\alpha }}f\left(0\right)\right]\left(t\right)\hfill \\ & & +{\mathcal{L}}^{-1}\left[b\frac{b\left(s+{\lambda }_2\right)-1}{b\left(s+\lambda \right)ln\left[b\left(s+{\lambda }_2\right)\right]}\left(s\tilde{f}\left(s\right)-f\left(0\right)\right)\right]\left(t\right).\hfill \end{array}$$

(21)

Therefore, the system of equations that generalize (9) reads

• Case I

$$\begin{array}{ccc}\hfill {\mathcal{A}}_1{m}_1\left(t\right)& =& -k\left[\frac{m_1\left(t\right)}{V_1}-\frac{m_2\left(t\right)}{V_2}\right]+f_1\left(t\right).\hfill \\ \hfill {\mathcal{A}}_1{m}_2\left(t\right)& =& k\left[\frac{m_1\left(t\right)}{V_1}-\frac{m_2\left(t\right)}{V_2}\right],\hfill \end{array}$$

(22)

with the initial conditions (10).

• Case II

$$\begin{array}{ccc}\hfill {\mathcal{A}}_2{m}_1& =& -k\left[\frac{m_1\left(t\right)}{V_1}-\frac{m_2\left(t\right)}{V_2}\right]+f_1\left(t\right).\hfill \\ \hfill {\mathcal{A}}_2{m}_2& =& k\left[\frac{m_1\left(t\right)}{V_1}-\frac{m_2\left(t\right)}{V_2}\right],\hfill \end{array}$$

(23)

with the initial conditions (10). Note that we multiplied the derivatives on the right hand side of (21) with $a^{\alpha }$ and $b^{\gamma }$ so that the dimension of ${\mathcal{A}}_2{m}_1$, for example, is the same as in the classical case (9). This will be used in determining the diffusion coefficient from k. Equations (9) and (22) represent a system of fractional differential equations with a general fractional derivative of the type (11), i.e., with Sonine kernels. The procedure, based on the operational calculus of the Mikusisnski type, for the solution of differential equations with general fractional derivatives with the Sonine kernels is presented in [25]. Equations (9) and (23) represent a system of fractional differential equations with a general fractional derivative of the distributed order (12). These types of systems are, to our knowledge, not treated for the case of kernels of arbitrary Sonine type. In addition, the presence of two parameters $\lambda$ and $\alpha$ in (11) gives the possibility of modeling the memory of the system more effectively.

Finally, we comment on the special cases of the systems (22) and (23). If we set ${\lambda }_1={\lambda }_2=0$ in (22), we obtain the fractional compartmental model used in [9,15], for example. Further, if we set ${\lambda }_1={\lambda }_2=0$ and $\alpha =\beta =1$ in (20), we obtain the classical compartmental system (9).

The systems (22) and (23) are our proposed generalization of the classical two-compartment model of pharmacokinetics (9).

3. Solution of (10), (22) and (10), (23)

• We first treat (22). By using (17), we obtain

  $$\begin{array}{ccc}& & \left[a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}+b\frac{s}{{\left(s+{\lambda }_2\right)}^{\beta }}\right]{\tilde{m}}_1\left(s\right)\hfill \\ & =& -k\left[\frac{{\tilde{m}}_1\left(s\right)}{V_1}-\frac{{\tilde{m}}_2\left(s\right)}{V_2}\right]+\left[a\frac{1}{{\left(s+{\lambda }_1\right)}^{\alpha }}+b\frac{1}{{\left(s+{\lambda }_2\right)}^{\beta }}\right]m_{10},\hfill \\ & & \left[a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}+b\frac{s}{{\left(s+{\lambda }_2\right)}^{\beta }}\right]{\tilde{m}}_2\left(s\right)\hfill \\ & =& k\left[\frac{{\tilde{m}}_1\left(s\right)}{V_1}-\frac{{\tilde{m}}_2\left(s\right)}{V_2}\right].\hfill \end{array}$$

  (24)

  where we assume that $f_1=0$. By adding the first and the second equation in (24) we obtain

  $$\left[{\tilde{m}}_1\left(s\right)+{\tilde{m}}_2\left(s\right)\right]=\frac{m_{10}}{s},$$

  so that the conservation of mass for the system (22), (10) reads

  $$m_1\left(t\right)+m_2\left(t\right)=m_{10},t\ge 0.$$

  (25)

Solving (24), we obtain

$$\begin{array}{ccc}\hfill {\tilde{m}}_2\left(s\right)& =& m_{10}\frac{k}{V_1}\frac{1}{s\left[a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}+b\frac{s}{{\left(s+{\lambda }_2\right)}^{\beta }}+k\left(\frac{1}{V_1}+\frac{1}{V_2}\right)\right]},\hfill \\ \hfill {\tilde{m}}_1\left(s\right)& =& \frac{m_{10}}{s}-m_{10}\frac{k}{V_1}\frac{1}{s\left[a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}+b\frac{s}{{\left(s+{\lambda }_2\right)}^{\beta }}+k\left(\frac{1}{V_1}+\frac{1}{V_2}\right)\right]}.\hfill \end{array}$$

(26)

We numerically invert (26) to obtain $m_2\left(t\right)$ and then determine $m_1\left(t\right)$ from (25). From the final value theorems for the Laplace transform [33], we obtain that, if the limits $m_i\left(0\right),m_i(\infty ),i=1,2$ exist, they satisfy

$$\underset{s\to \infty }{lim}s{\tilde{m}}_1\left(s\right)=m_1\left(0\right)=m_{10};\underset{s\to 0}{lim}s{\tilde{m}}_1\left(s\right)=m_1(\infty )=m_{10}\frac{V_1}{V_1+V_2},$$

$$\underset{s\to 0}{lim}s{\tilde{m}}_2\left(s\right)=m_2(\infty )=m_{10}\frac{V_2}{V_1+V_2}.$$

Therefore, the final concentrations in each compartment $c_i=\frac{m_i}{V_i},i=1,2$ are equal:

$$c_1\left(\infty \right)=c_2\left(\infty \right)=\frac{m_1\left(\infty \right)}{V_1}=\frac{m_2\left(\infty \right)}{V_2}=\frac{m_{10}}{V_1+V_2},$$

having in mind Fick’s model of diffusion.

• Applying the Laplace transform to (23) and using (17), we obtain

  $$\begin{array}{ccc}& & \left[\frac{a}{{\left[a\left(s+{\lambda }_1\right)\right]}^{1-\beta }}+\frac{b\left(s+{\lambda }_2\right)-1}{\left(s+{\lambda }_2\right)ln\left[b\left(s+{\lambda }_2\right)\right]}\right]\left[s{\tilde{m}}_1\left(s\right)-m_{10}\right]\hfill \\ & =& -k\left[\frac{{\tilde{m}}_1\left(s\right)}{V_1}-\frac{{\tilde{m}}_2\left(s\right)}{V_2}\right],\hfill \\ & & \left[\frac{a}{{\left[a\left(s+{\lambda }_1\right)\right]}^{1-\beta }}+\frac{b\left(s+{\lambda }_2\right)-1}{\left(s+{\lambda }_2\right)ln\left[b\left(s+{\lambda }_2\right)\right]}\right]s{\tilde{m}}_2\left(s\right)\hfill \\ & =& k\left[\frac{{\tilde{m}}_1\left(s\right)}{V_1}-\frac{{\tilde{m}}_2\left(s\right)}{V_2}\right],\hfill \end{array}$$

  (27)

  for $f_1=0$. Again, from (27), we obtain $\left[{\tilde{m}}_1\left(s\right)+{\tilde{m}}_2\left(s\right)\right]=\frac{m_{10}}{s}$, so that $m_1\left(t\right)+m_2\left(t\right)=m_{10},t\ge 0$. Solving (27) for ${\tilde{m}}_1\left(s\right)$ and ${\tilde{m}}_2\left(s\right)$, we obtain

  $$\begin{array}{ccc}\hfill {\tilde{m}}_2\left(s\right)& =& \frac{1}{V_1}\frac{k{m}_{10}}{s\left[s\frac{a}{{\left[a\left(s+{\lambda }_1\right)\right]}^{1-\beta }}+s\frac{b\left(s+{\lambda }_2\right)-1}{\left(s+{\lambda }_2\right)ln\left[b\left(s+{\lambda }_2\right)\right]}+k\left(\frac{1}{V_1}+\frac{1}{V_2}\right)\right]},\hfill \\ \hfill {\tilde{m}}_1\left(s\right)& =& \frac{m_{10}}{S}-\frac{1}{V_1}\frac{k{m}_{10}}{s\left[s\frac{a}{{\left[a\left(s+{\lambda }_1\right)\right]}^{1-\beta }}+s\frac{b\left(s+{\lambda }_2\right)-1}{\left(s+{\lambda }_2\right)ln\left[b\left(s+{\lambda }_2\right)\right]}+k\left(\frac{1}{V_1}+\frac{1}{V_2}\right)\right]}.\hfill \\ & & \hfill \end{array}$$

  (28)

By using the final value theorems for the Laplace transform, as in the previous case, we conclude that, for $t\to \infty$, the concentrations in each compartment are equal.

To obtain the solution in the time domain, we apply the Mellin’s inverse formula to the first equation in (26) so that

$$m_2\left(t\right)=m_{10}\frac{k}{V_1}\underset{T\to \infty }{lim}\frac{1}{2\pi i}{\int }_{x_0-iT}^{x_0+iT}\frac{exp\left(its\right)}{s\left[a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}+b\frac{s}{{\left(s+{\lambda }_2\right)}^{\beta }}+k\left(\frac{1}{V_1}+\frac{1}{V_2}\right)\right]}ds,$$

(29)

where $x_0$ is greater than the real part of all singularities of

$$F_1\left(s\right)=\frac{1}{s\left[a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}+b\frac{s}{{\left(s+{\lambda }_2\right)}^{\beta }}+k\left(\frac{1}{V_1}+\frac{1}{V_2}\right)\right]}.$$

To prove this, let $s=Rexp\left(i\theta \right)$. Then, $s+{\lambda }_1=R_{a}exp\left(i{\theta }_a\right),s+{\lambda }_2=R_{b}exp\left(i{\theta }_b\right)$, with ${\theta }_a,{\theta }_b\le \theta$. Therefore,

$$a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}=a\frac{R}{R_a^{\alpha }}exp\left(i\left(\theta -\alpha {\theta }_a\right)\right),b\frac{s}{\left(s+{\lambda }_2\right)\beta }=a\frac{R}{R_b^{\beta }}exp\left(i\left(\theta -\beta {\theta }_b\right)\right),$$

so that, if $\theta =0$, we have $\Re \left[a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}+b\frac{s}{{\left(s+{\lambda }_2\right)}^{\beta }}+k\left(\frac{1}{V_1}+\frac{1}{V_2}\right)\right]>0$ since $a\ge 0,b\ge 0,k>0$. In addition,

$$\begin{array}{ccc}\hfill \Im \left(a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}+b\frac{s}{{\left(s+{\lambda }_2\right)}^{\beta }}\right)& \ge & 0\mathrm{if}\theta \in \left[0,\frac{\pi }{2}\right],\hfill \\ \hfill \Im \left(a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}+b\frac{s}{{\left(s+{\lambda }_2\right)}^{\beta }}\right)& \le & 0\mathrm{if}\theta \in \left[-\frac{\pi }{2},0\right].\hfill \end{array}$$

where $\Im \left(z\right)$ denotes the imaginary part of z. Therefore, if $s=Rexp\left(i\theta \right),\theta \in \left[-\frac{\pi }{2},\frac{\pi }{2}\right],R\ne 0$, the function $F_1\left(s\right)$ does not have singular points. Consequently, we conclude that (29) represents the solution to (22) for any $x_0>0$. Using (25), we obtain

$$m_1\left(t\right)=m_{10}-m_2\left(t\right).$$

(30)

Therefore, (29) and (30) represent the solution to (22). Similarly, for the first equation in (28) we obtain

$$m_2\left(t\right)=m_{10}\frac{k}{V_1}\underset{T\to \infty }{lim}\frac{1}{2\pi i}{\int }_{x_0-iT}^{x_0+iT}\frac{exp\left(its\right)}{s\left[s\frac{a}{{\left[a\left(s+{\lambda }_1\right)\right]}^{1-\beta }}+s\frac{b\left(s+{\lambda }_2\right)-1}{\left(s+{\lambda }_2\right)ln\left[b\left(s+{\lambda }_2\right)\right]}+k\left(\frac{1}{V_1}+\frac{1}{V_2}\right)\right]}ds.$$

(31)

and (30) as a solution of (23). For the numerical inversion of the Laplace transform given by (31), we used the standard Mathcad 15 program with $x_0=0.1$ and $T=150$.

4. Numerical Results

We present an application of the models (22) and (23) to two problems of pharmacokinetics. Our examples present a generalization of the results presented in [14].

4.1. Gentamicin Release from Poly(vinyl Alcohol)/Chitosan/Gentamicin (PVA/CHI/Gent) Hydrogel

In this section, we use the model (22) to study the release of gentamicin from poly(vinyl alcohol)/chitosan/gentamicin (PVA/CHI/Gent) hydrogel aimed at wound dressing in the medical treatment of deep chronic wounds. The PVA/CHI/Gent hydrogel was prepared by the physical cross linking of poly(vinyl alcohol)/chitosan dispersion using the freezing–thawing method according to the procedure that we published earlier [14]. Thus, obtained hydrogels were cut into discs with diameters $d=9$ mm and thicknesses $\delta =4$mm and were swollen in gentamicin solution at 37 °C for 48 h. For the drug release assay, PVA/CHI/Gent hydrogel was immersed in $1{\mathrm{cm}}^3$ deionized water and kept at 37 °C. The concentration of released gentamicin was determined using high-performance liquid chromatography (HPLC) (Thermo Fisher Scientific, Waltham, MA, USA) coupled with an ion trap (LCQ Advantage, Thermo Fisher Scientific) as a mass spectrometer (MS). The ratio between the concentration of released gentamicin and initial concentration of gentamicin in the hydrogel was monitored during the time in order to obtain the gentamicin release profile. The measured data are new and are given in

Table 1. Measured values of $m_2$ and calculated values of $m_1=1-m_2$ at different time instants for experiment Section 4.1.

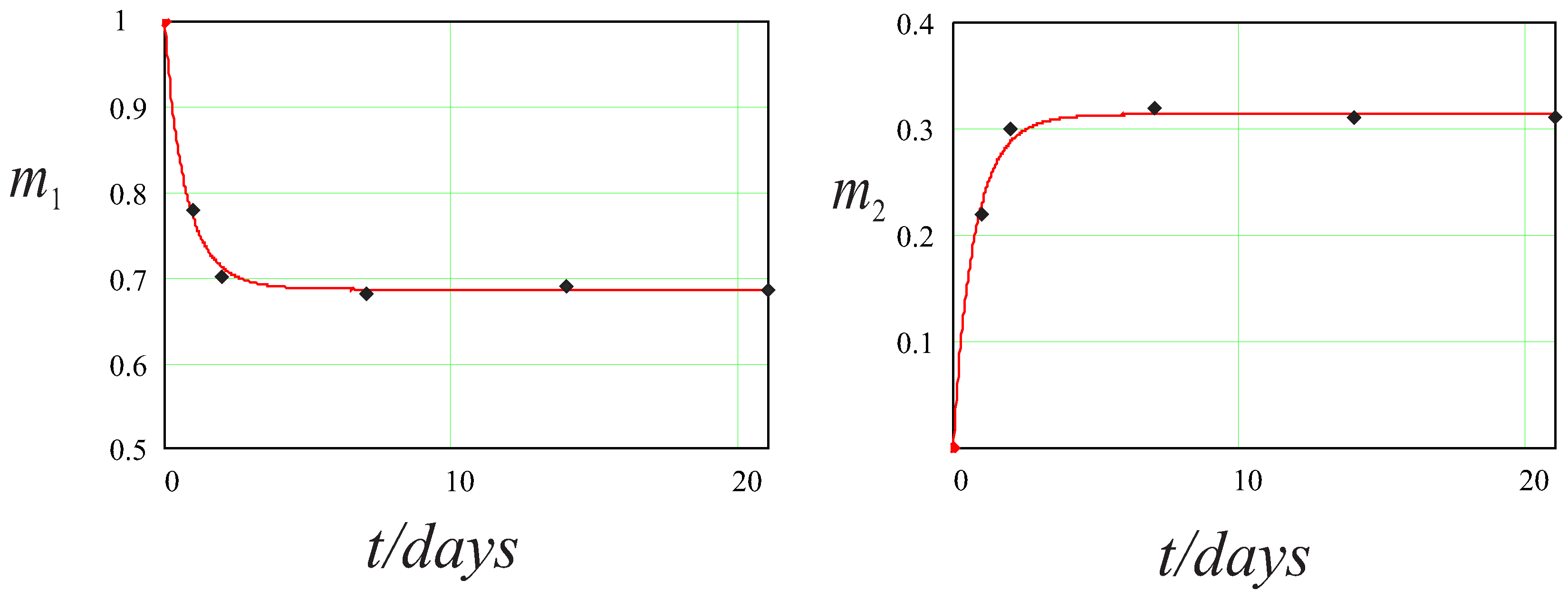
t (Days)	m${}_1$ calculated	m${}_2$ measured
0	1	0
1	0.72389	0.27611
2	0.33093	0.69067
4	0.2612	0.73880
7	0.2522	0.74771
14	0.25164	0.74836

and Figure 2. The values of mass in the compartments are given in the dimensionless form $\frac{m_i}{m_{10}},i=1,2$. The measured values of mass $m_2$ were divided by the initial and total mass of gentamicin, $m_{10}=2.4551\mathrm{mg}$. In our experiments, we used the hydrogel disc volume, $V_1=254.5{\mathrm{mm}}^3$, and the volume of deionized water where hydrogel was immersed, $V_2=1000{\mathrm{mm}}^3$.

We transformed (29) by dividing with $m_{10}$ so that the relative amount of gentamicin is given as

$$m_2\left(t\right)=\frac{k}{V_1}\underset{T\to \infty }{lim}\frac{1}{2\pi i}{\int }_{x_0-iT}^{x_0+iT}\frac{exp\left(its\right)}{s\left[a\frac{s}{{\left(s+{\lambda }_1\right)}^{\alpha }}+k\left(\frac{1}{V_1}+\frac{1}{V_2}\right)\right]}ds.$$

(32)

Parameters $\left(a,\alpha ,{\lambda }_1,b,\beta ,{\lambda }_2,k\right)$ were determined by the least square method, i.e., the sum of the squared residuals between the measured and calculated values of $m_2$ at five measured points is minimized; that is,

$$\underset{\left(a,\alpha ,{\lambda }_1,b,\beta ,{\lambda }_2,k\right)}{min}Z\left(a,\alpha ,{\lambda }_1,b,\beta ,{\lambda }_2,k\right)=Z\left(a^{\ast },{\alpha }^{\ast },{\lambda }_1^{\ast },b^{\ast },{\beta }^{\ast },{\lambda }_2^{\ast },k^{\ast }\right)$$

(33)

where

$$Z\left(a,\alpha ,{\lambda }_1,b,\beta ,{\lambda }_2,k\right)=\sum _{j=0}^5{\left(m_2\left(t_j\right)-m_2\left(t_{jmeasured}\right)\right)}^2.$$

(34)

In the minimization process, we took into account the restrictions

$$0<\alpha \le 1,0<\beta \le 1,{\lambda }_1\ge 0,{\lambda }_2\ge 0,a\ge 0,b\ge 0,k>0.$$

The values of parameters obtained from (34) are

$$\begin{array}{ccc}\hfill {\alpha }^{\ast }& =& 1,{\beta }^{\ast }=0.001,{\lambda }_1^{\ast }=8.7\times {10}^{-7}{\mathrm{s}}^{-1},{\lambda }_2^{\ast }=6.7562{\mathrm{s}}^{-1},a^{\ast }=0.0008898\mathrm{s},\hfill \\ \hfill b^{\ast }& =& 0.03772\mathrm{s},k^{\ast }\delta =0.005193{\mathrm{cm}}^4/\mathrm{day}.\hfill \end{array}$$

(35)

The corresponding diffusion coefficient, D, was calculated as follows. Since the shape of the hydrogel is cylindrical with a diameter of 9 mm and a height (thickness) of 4 mm, the area of the diffusion was calculated to be $A=2.4{\mathrm{cm}}^2$. Therefore, the diffusion coefficient is $D=k\delta /A$ = 2.504 ×${10}^{-8}{\mathrm{cm}}^2/\mathrm{s}$. In Figure 2, we present solution (32) with parameters (35). As can be seen, the values obtained from the model agree very well with the measured values, enabling a determination of the diffusion coefficient of gentamicin through the hydrogel disc.

Gentamicin release profiles verified the initial burst release effect of gentamicin from the hydrogel, i.e., a 70% loaded antibiotic was released within the first 48 h, which could be very useful in preventing biofilm formation, followed by a slow release of gentamicin at a later time period. This behavior is in favor of both application requirements, namely, for effective wound dressing with antibacterial properties, an initial burst release can be favorable to quickly suppress the bacterial adhesion and biofilm formation in the wound environment, whereas a sustained release over a longer period would ensure the maintained sterility of the wound and lower the dressing replacement frequency.

4.2. Gentamicin Release from Bioceramic Hydroxyapatite/Poly(vinyl Alcohol)/Chitosan/Gentamicin (HAP/PVA/CHI/Gent) Coating on Titanium

Electrophoretic deposition (EPD) was performed in an aqueous suspension consisting of hydroxyapatite (1.0 wt%), chitosan (0.05 wt%), poly(vinyl alcohol) (0.1 wt%) and gentamicin sulphate (0.1 wt%). A cathodic EPD process was performed on a titanium plate (dimensions 1 × 1) using a constant voltage method according to the procedure that we recently published [34]. The coating thickness, $\delta$, was $3.1$ $\mathsf{\mu }$m. For the gentamicin release measurements, the HAP/PVA/CS/Gent coating on titanium was immersed in 1 ${\mathrm{cm}}^3$ deionized water and kept at 37 °C. The concentration of released gentamicin was determined using high-performance liquid chromatography (HPLC) (Thermo Fisher Scientific, Waltham, MA, USA) coupled with an ion trap (LCQ Advantage, Thermo Fisher Scientific) as a mass spectrometer (MS).

In

Table 2. Measured values of $m_2$ and calculated values of $m_1=1-m_2$ at different time instants for experiment Section 4.2.

t (Days)	m${}_1$calculated	m${}_2$measured
0	1	0
1	0.78	0.22
2	0.70	0.30
7	0.68	0.32
14	0.69	0.31
21	0.69	0.31

we present numerical values of $m_1$ and $m_2$. The values of mass in the compartments are given in the dimensionless form $\frac{m_i}{m_{10}},i=1,2$. The measured values of mass $m_2$ were divided by the initial and total mass of gentamicin, $m_{10}=163.52$ mg. In our experiments, we used the coating volume, $V_1=3.1$×${10}^{-1}{\mathrm{mm}}^3$, and the volume of deionized water where coating was immersed, $V_2=1000{\mathrm{mm}}^3$.

We write (31) for the relative amount of gentamicin in compartment 2 as

$$m_2\left(t\right)=\frac{k}{V_1}\underset{T\to \infty }{lim}\frac{1}{2\pi i}{\int }_{x_0-iT}^{x_0+iT}\frac{exp\left(its\right)}{s\left[s\frac{a}{{\left[a\left(s+{\lambda }_1\right)\right]}^{1-\beta }}+s\frac{b\left(s+{\lambda }_2\right)-1}{\left(s+{\lambda }_2\right)ln\left[b\left(s+{\lambda }_2\right)\right]}+k\left(\frac{1}{V_1}+\frac{1}{V_2}\right)\right]}ds.$$

(36)

The values of parameters were determined from the least square method (33), which now becomes

$$\underset{\left(a,\beta ,{\lambda }_1,b,{\lambda }_2,k\right)}{min}Z\left(a,\beta ,{\lambda }_1,b,{\lambda }_2,k\right)=Z\left(a^{\ast },{\beta }^{\ast },{\lambda }_1^{\ast },b^{\ast },{\lambda }_2^{\ast },k^{\ast }\right)$$

(37)

The minimization of (37) leads to the following values:

$$\begin{array}{ccc}\hfill a^{\ast }& =& 0.1581115\mathrm{s},{\beta }^{\ast }=1.00,{\lambda }_1^{\ast }=0.170161163{\mathrm{s}}^{-1}{\lambda }_2^{\ast }=0.000847587115{\mathrm{s}}^{-1},\hfill \\ \hfill b^{\ast }& =& 0.0000773445158\mathrm{s},k^{\ast }\delta =0.064412530254{\mathrm{cm}}^4/\mathrm{day}.\hfill \end{array}$$

(38)

The corresponding diffusion coefficient, D, was calculated as follows. Since the coating area was calculated to be $A=1{\mathrm{cm}}^2$, the diffusion coefficient is $D=k\delta /A$ = 4.337 ×${10}^{-7}{\mathrm{cm}}^2/s$.

In Figure 3 we present solution (36) with parameters (38). As can be seen, the values obtained from the model agree very well with the measured values, enabling a determination of the diffusion coefficient of gentamicin through the coating.

The models described by (22) and (23) with the initial condition (10) shows excellent agreement with the measured values. The presence of additional parameters ${\lambda }_{1,2}$ in (22) and (23) is the advantage of the present models when compared with the classical fractional derivative models used; for example, in [10,11,12,21]. In addition, the results shown in Figure 3 confirm that the distributed-order general fractional derivative—see (23) and (36)—which is used for the first time in this work, represents the measured values especially well. Experimental gentamicin release profiles verified the initial burst release effect of gentamicin from the coating, i.e., a 30 % loaded antibiotic was released within the first 48 h, which could be useful in preventing biofilm formation, followed by a slow release of gentamicin at a later time period. This behavior is in favor of both application requirements, namely, for effective bone implant, an initial burst release can be favorable to quickly suppress the bacterial adhesion and biofilm formation in the implant environment, whereas a sustained release over a longer period would ensure the maintained sterility of the implant.

5. Conclusions

In this work, we formulated a new mathematical model for a two-compartment system of pharmacokinetics that includes a general fractional derivative of distributed order, given in the form (12). We solved the resulting system of equations that determines the mass transfer between compartments in the simple case of a two-compartment system. We have shown that the generalized formulation proposed here satisfies the mass balance equation. Two applications of the models are presented in detail. The first one is gentamicin release from PVA/CHI/Gent hydrogel aimed at wound dressing and the medical treatment of deep chronic wounds. The second one is gentamicin release from HAP/PVA/CHI/Gent coating on titanium substrate aimed at bone tissues implants, which enables drug delivery directly to the infection site. The gentamicin release study indicated a “burst” release effect in the first 48 h, which is beneficial for the blockage of biofilm formation, followed by a slow and steady release in the later time period. The experimental gentamicin release curves are described very well with the models proposed here. In addition, we determined the values of the diffusion coefficient of gentamicin on the basis of the models approximating the entire time period of the release. Further work on other applications of the models (10), (22) and (10), (23) to different physical systems and on the formulation of the procedure to determine the diffusion coefficient from the parameter k is now in progress.