Circuit Realization of the Fractional-Order Sprott K Chaotic System with Standard Components
Abstract
1. Introduction
2. Generalized Sprott K System and a Brief Mathematical Background on Fractional Calculus
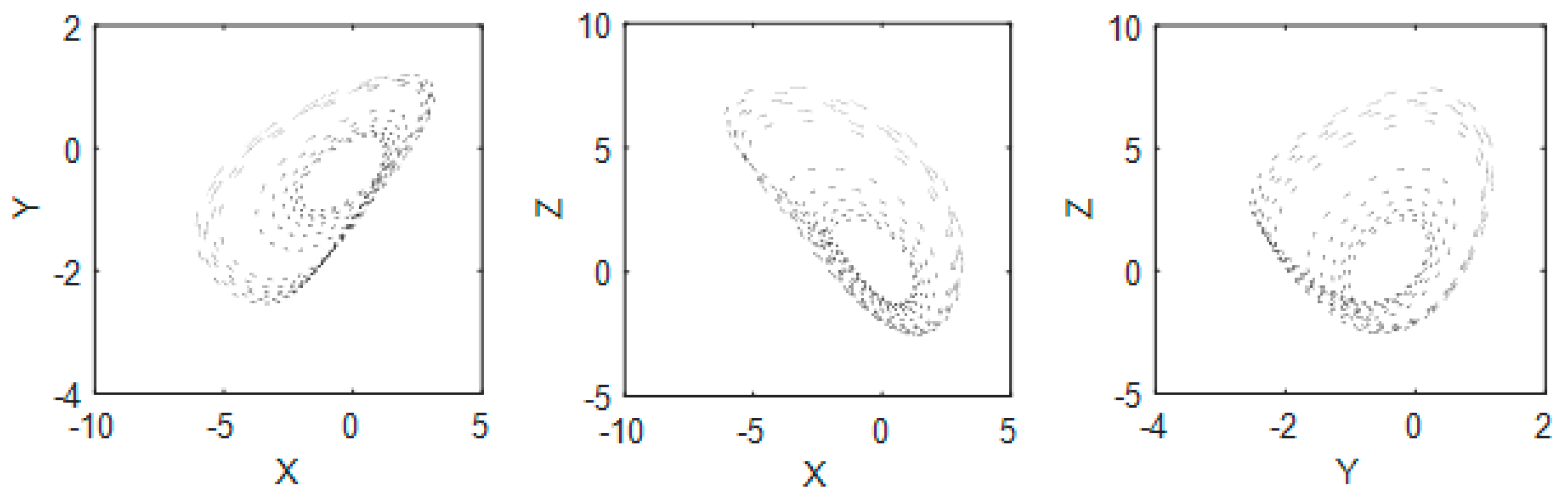
- It is possible to explore the chaotic behaviour of the system for different fractional-order values.
- The system can be linearly scaled to keep the amplitudes of the output voltages between −10 and +10 V while implementing the circuit.
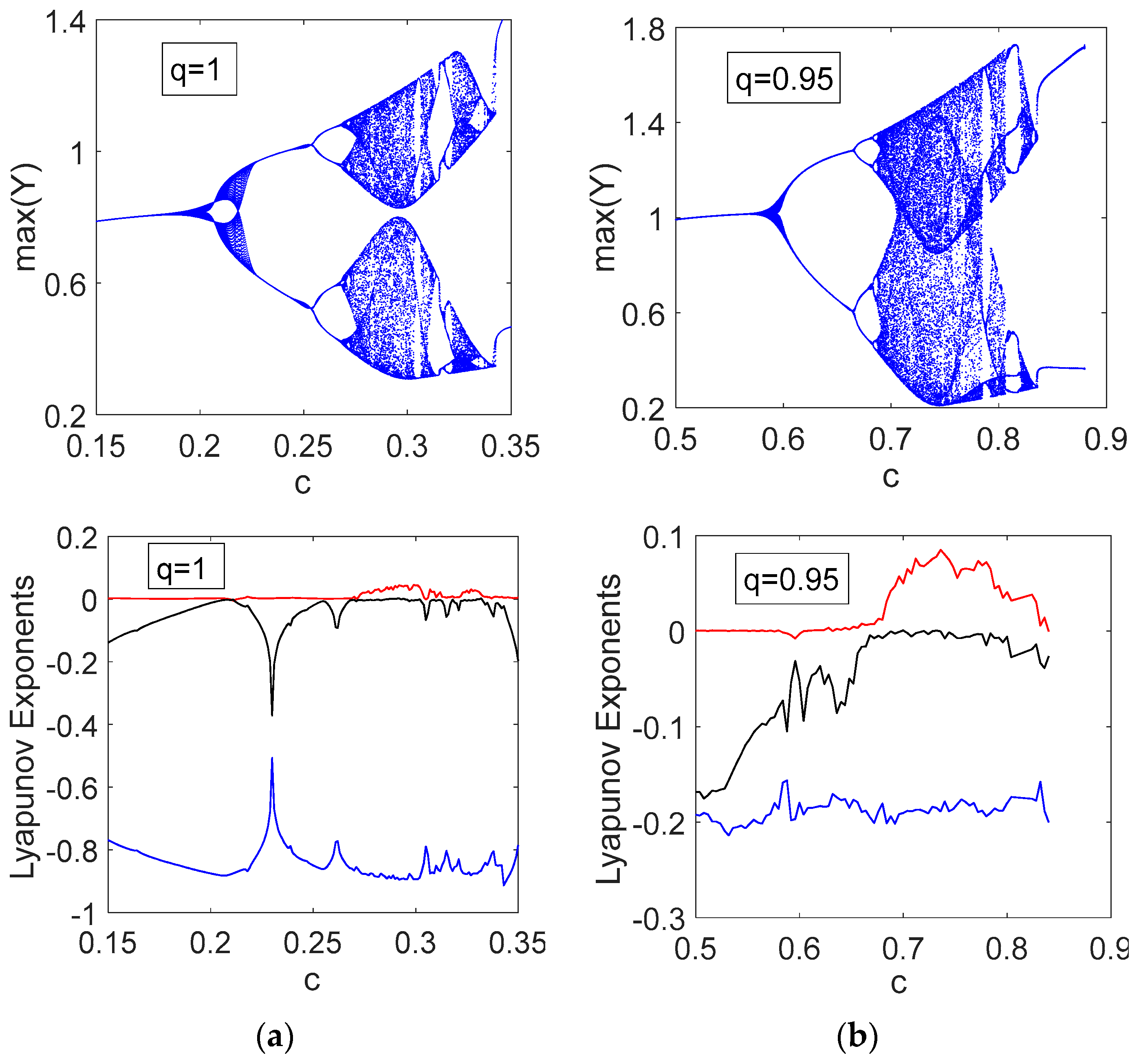
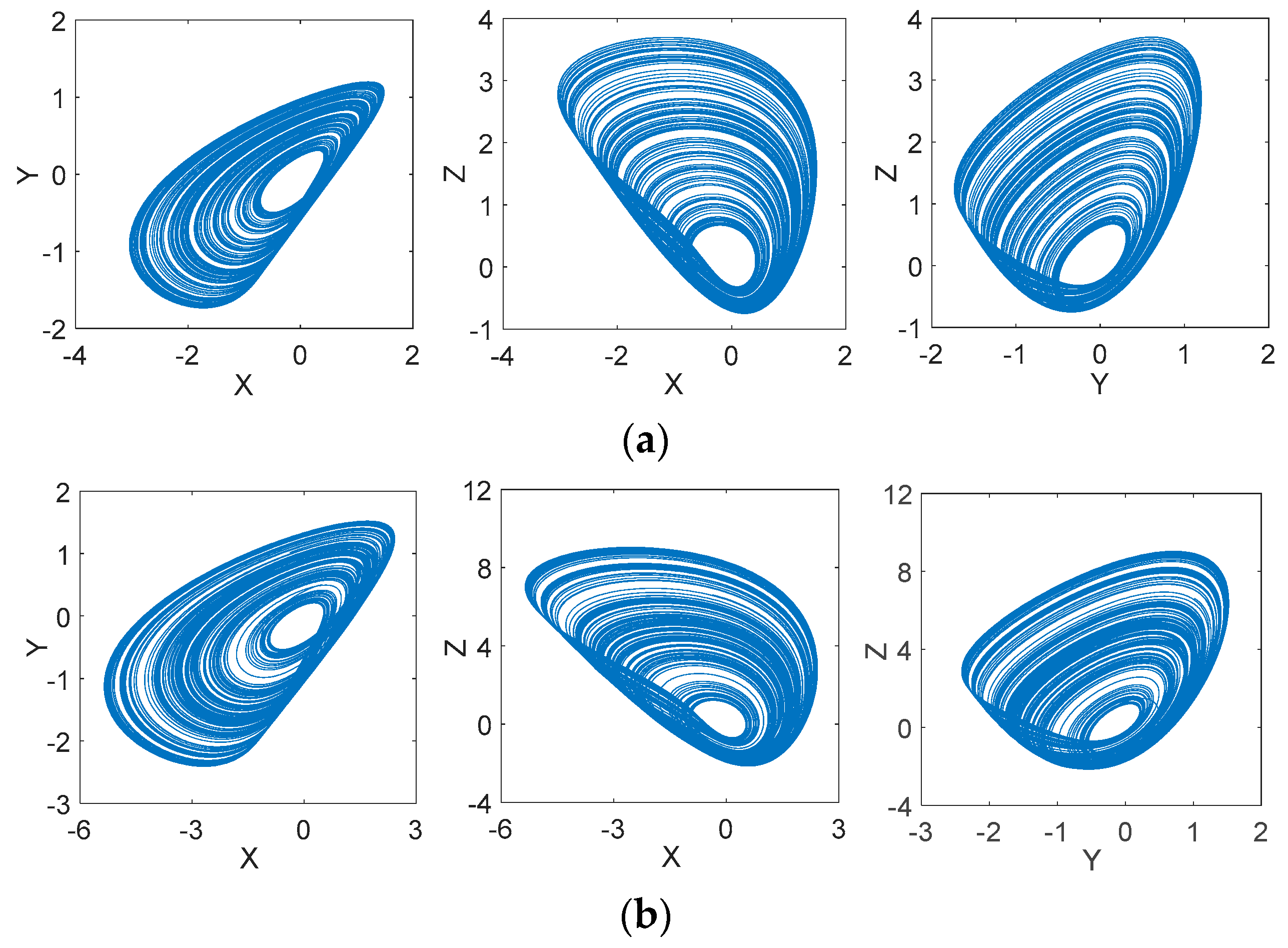
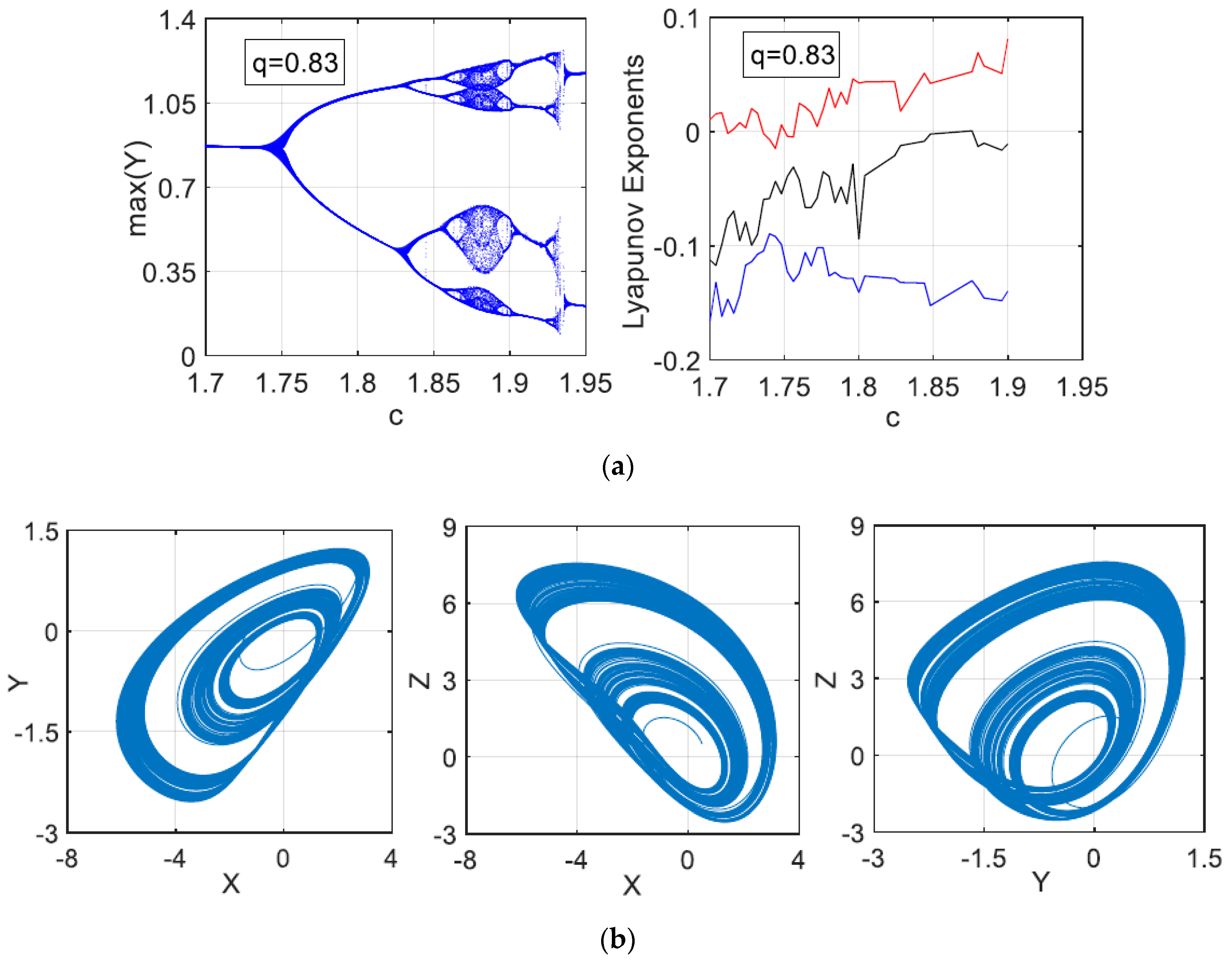
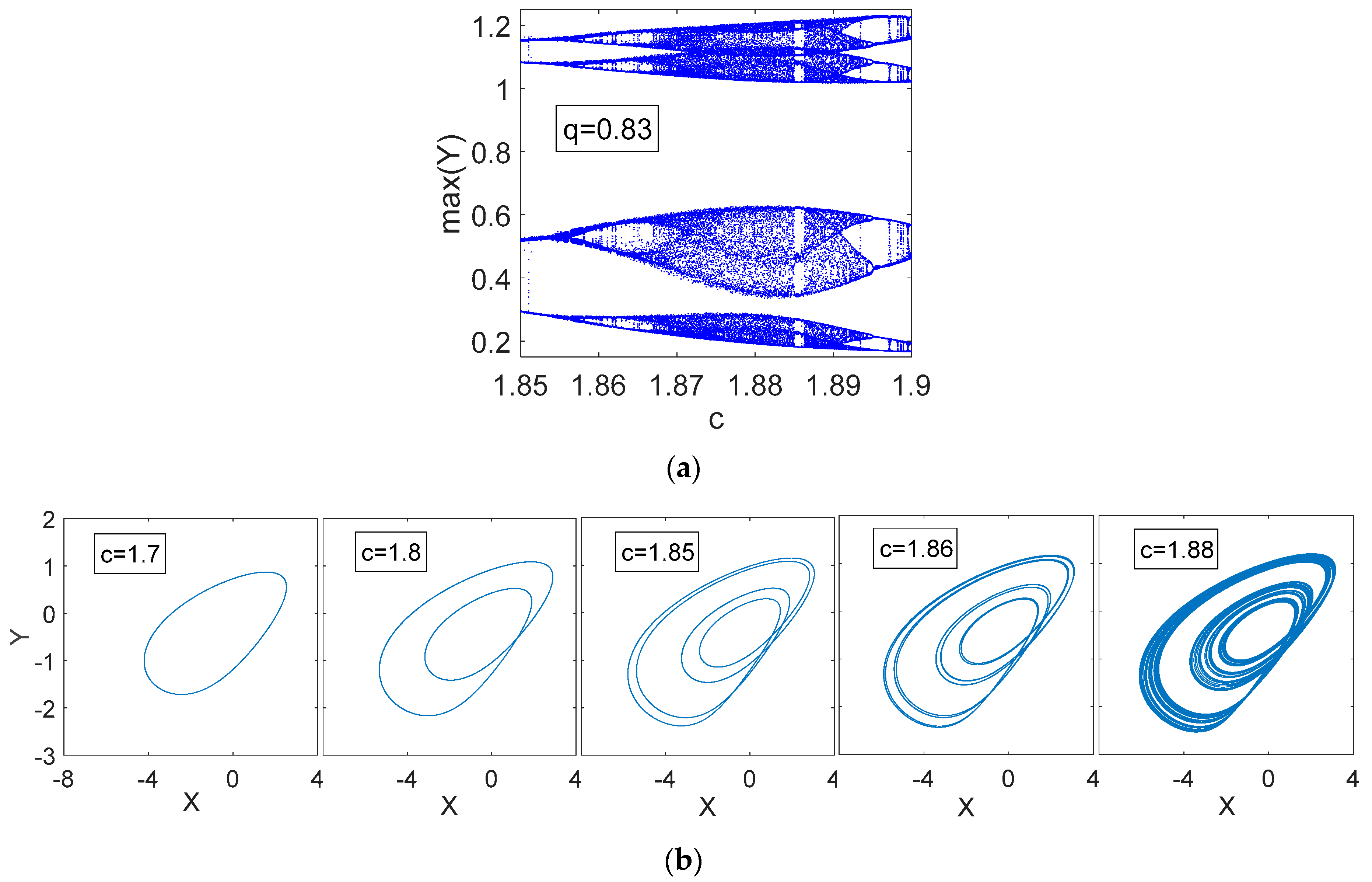
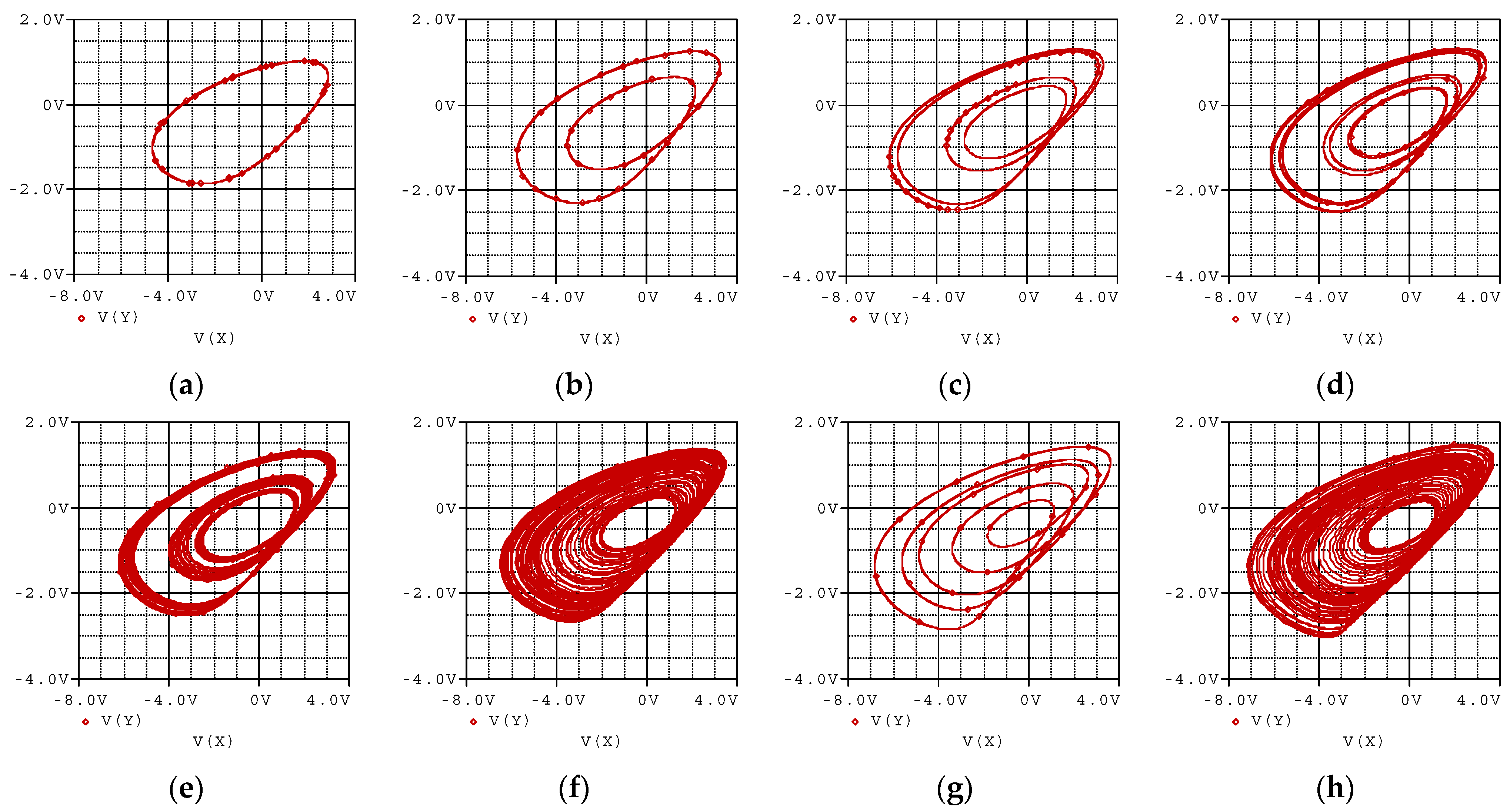
3. Dynamical Analyses of the Fractional-Order Sprott K System
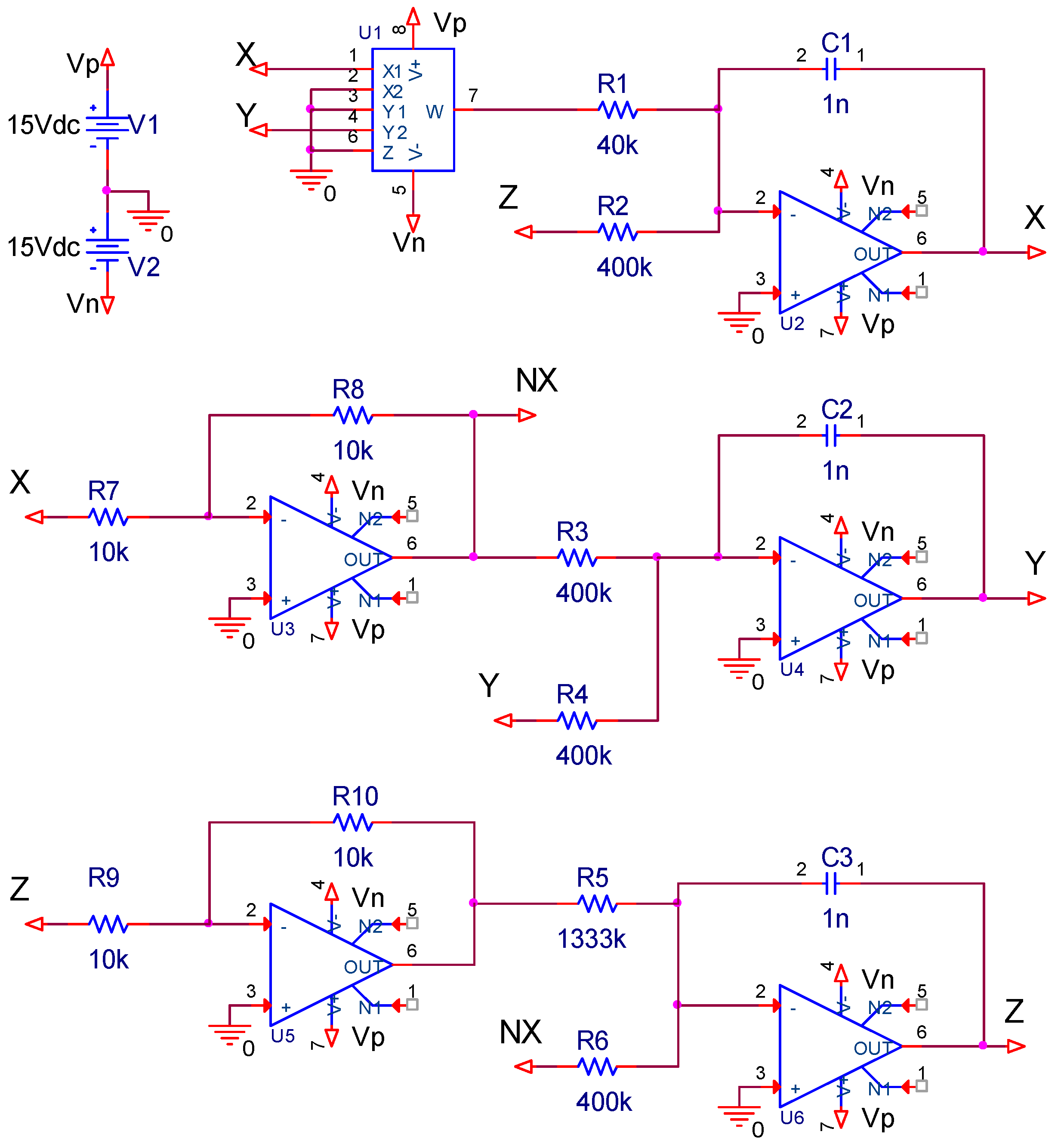
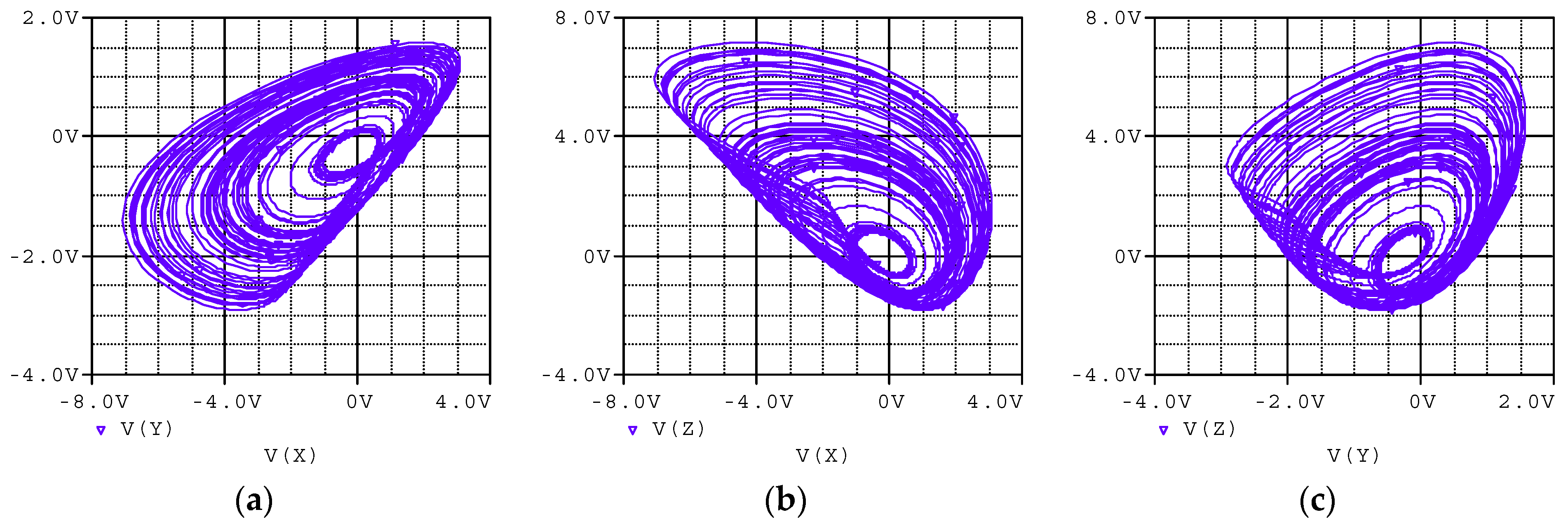
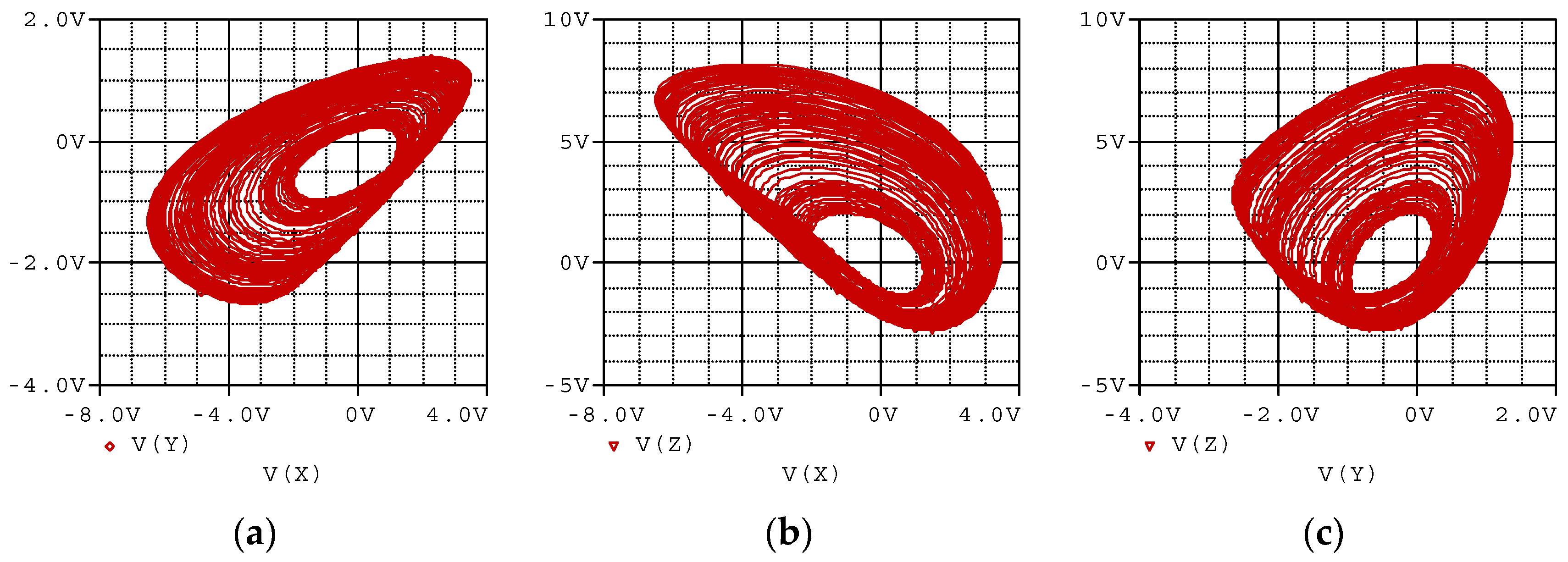
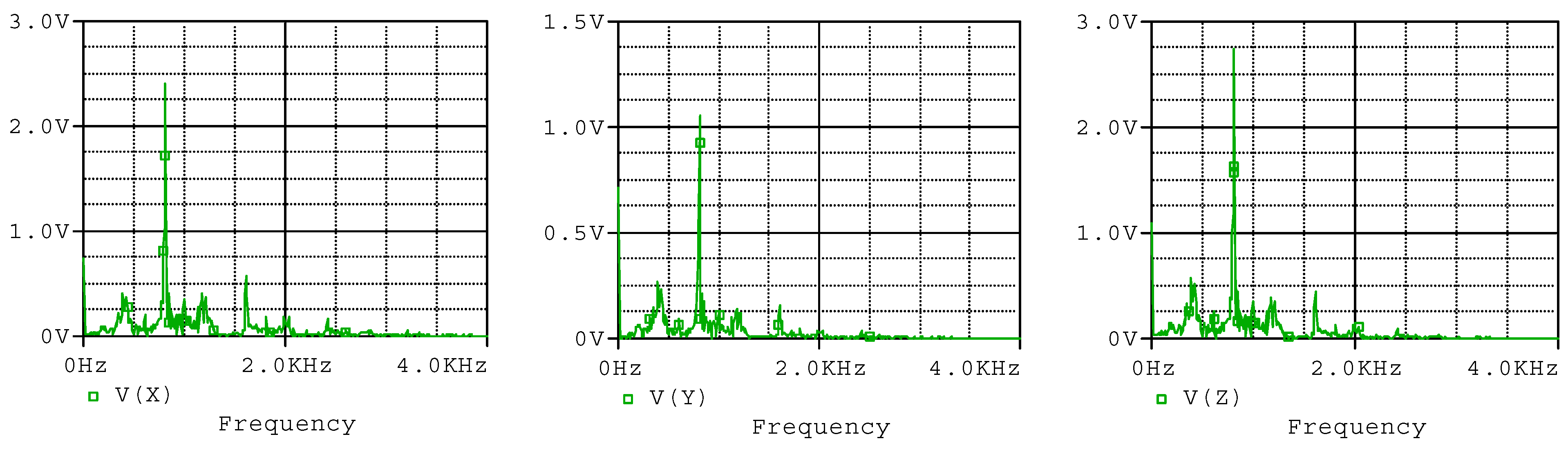
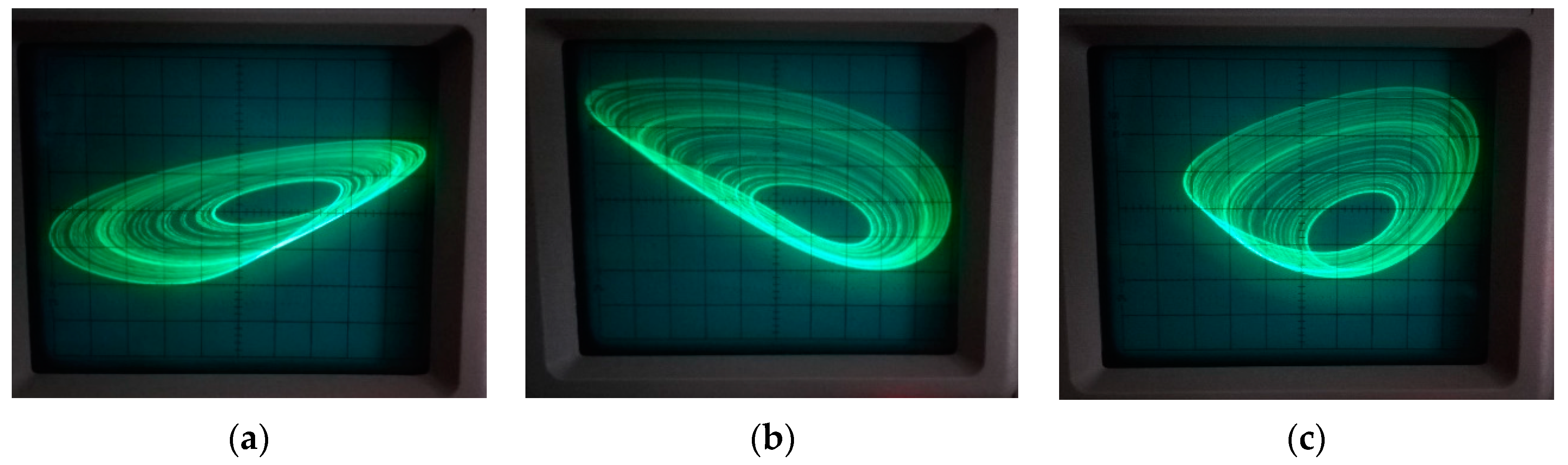
4. Electronic Circuit Realization of the Fractional-Order Sprott K Chaotic System
5. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yang, F.; Wang, X. Dynamic characteristic of a new fractional-order chaotic system based on the Hopfield Neural Network and its digital circuit implementation. Phys. Scr. 2021, 96, 035218. [Google Scholar] [CrossRef]
- Ahmad, W.M.; Sprott, J. Chaos in fractional-order autonomous nonlinear systems. Chaos Solitons Fractals 2003, 16, 339–351. [Google Scholar] [CrossRef]
- Peng, Z.; Yu, W.; Wang, J.; Wang, J.; Chen, Y.; He, X.; Jiang, D. Dynamic analysis of seven-dimensional fractional-order chaotic system and its application in encrypted communication. J. Ambient. Intell. Humaniz. Comput. 2020, 11, 5399–5417. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhou, T. Three schemes to synchronize chaotic Fractional-order Rucklidge systems. Int. J. Mod. Phys. B 2007, 21, 2033–2044. [Google Scholar] [CrossRef]
- Trikha, P.; Jahanzaib, L.S.; Nasreen; Baleanu, D. Dynamical analysis and triple compound combination anti-synchronization of novel fractional chaotic system. J. Vib. Control. 2021, 28, 1057–1073. [Google Scholar] [CrossRef]
- Guang-Chao, Z.; Chong-Xin, L.; Yan, W. Dynamic analysis and finite time synchronization of a fractional-order chaotic system with hidden attractors. Acta Phys. Sin. 2018, 67, 050502. [Google Scholar] [CrossRef]
- Akgul, A.; Adiyaman, Y.; Gokyildirim, A.; Aricioglu, B.; Pala, M.A.; Cimen, M.E. Electronic Circuit Implementations of a Fractional-Order Chaotic System and Observing the Escape from Chaos. J. Circuits Syst. Comput. 2022, 32, 2350085. [Google Scholar] [CrossRef]
- Wang, J.; Xiao, L.; Rajagopal, K.; Akgul, A.; Cicek, S.; Aricioglu, B. Fractional-Order Analysis of Modified Chua’s Circuit System with the Smooth Degree of 3 and Its Microcontroller-Based Implementation with Analog Circuit Design. Symmetry 2021, 13, 340. [Google Scholar] [CrossRef]
- Chen, D.; Liu, C.; Wu, C.; Liu, Y.; Ma, X.; You, Y. A New Fractional-Order Chaotic System and Its Synchronization with Circuit Simulation. Circuits Syst. Signal Process. 2012, 31, 1599–1613. [Google Scholar] [CrossRef]
- Wang, M.; Liao, X.; Deng, Y.; Li, Z.; Su, Y.; Zeng, Y. Dynamics, synchronization and circuit implementation of a simple fractional-order chaotic system with hidden attractors. Chaos Solitons Fractals 2019, 130, 109406. [Google Scholar] [CrossRef]
- Xiang-Rong, C.; Chong-Xin, L.; Fa-Qiang, W. Circuit realization of the fractional-order unified chaotic system. Chin. Phys. B 2008, 17, 1664–1669. [Google Scholar] [CrossRef]
- Li, X.; Li, Z.; Wen, Z. One-to-four-wing hyperchaotic fractional-order system and its circuit realization. Circuit World 2020, 46, 107–115. [Google Scholar] [CrossRef]
- Gokyildirim, A.; Calgan, H.; Demirtas, M. Fractional-Order sliding mode control of a 4D memristive chaotic system. J. Vib. Control. 2023. [Google Scholar] [CrossRef]
- Liu, T.; Yan, H.; Banerjee, S.; Mou, J. A fractional-order chaotic system with hidden attractor and self-excited attractor and its DSP implementation. Chaos Solitons Fractals 2021, 145, 110791. [Google Scholar] [CrossRef]
- Chen, D.; Wu, C.; Iu, H.H.C.; Ma, X. Circuit simulation for synchronization of a fractional-order and integer-order chaotic system. Nonlinear Dyn. 2013, 73, 1671–1686. [Google Scholar] [CrossRef]
- Yao, J.; Wang, K.; Huang, P.; Chen, L.; Machado, J.T. Analysis and implementation of fractional-order chaotic system with standard components. J. Adv. Res. 2020, 25, 97–109. [Google Scholar] [CrossRef]
- Rajagopal, K.; Nazarimehr, F.; Guessas, L.; Karthikeyan, A.; Srinivasan, A.; Jafari, S. Analysis, Control and FPGA Implementation of a Fractional-Order Modified Shinriki Circuit. J. Circuits Syst. Comput. 2019, 28, 1950232. [Google Scholar] [CrossRef]
- Pham, V.-T.; Kingni, S.T.; Volos, C.; Jafari, S.; Kapitaniak, T. A simple three-dimensional fractional-order chaotic system without equilibrium: Dynamics, circuitry implementation, chaos control and synchronization. AEU Int. J. Electron. Commun. 2017, 78, 220–227. [Google Scholar] [CrossRef]
- Hammouch, Z.; Mekkaoui, T. Circuit design and simulation for the fractional-order chaotic behavior in a new dynamical system. Complex Intell. Syst. 2018, 4, 251–260. [Google Scholar] [CrossRef]
- Altun, K. FPAA Implementations of Fractional-Order Chaotic Systems. J. Circuits Syst. Comput. 2021, 30, 1–23. [Google Scholar] [CrossRef]
- Altun, K. Kesir Dereceli Sprott-K Kaotik Sisteminin Dinamik Analizi ve FPGA Uygulaması. Eur. J. Sci. Technol. 2021, 25, 392–399. [Google Scholar] [CrossRef]
- Dang, H.G. Dynamics and Synchronization of the Fractional-Order Sprott E System. Adv. Mater. Res. 2013, 850–851, 876–879. [Google Scholar] [CrossRef]
- Dang, H.G. Adaptive Synchronization of the Fractional-Order Sprott N System. Adv. Mater. Res. 2013, 850–851, 872–875. [Google Scholar] [CrossRef]
- Sprott, J.C. Some simple chaotic flows. Phys. Rev. E 1994, 50, R647–R650. [Google Scholar] [CrossRef] [PubMed]
- Altun, K. Multi-Scroll Attractors with Hyperchaotic Behavior Using Fractional-Order Systems. J. Circuits Syst. Comput. 2021, 31, 22500852. [Google Scholar] [CrossRef]
- Lu, J.G.; Chen, G. A note on the fractional-order Chen system. Chaos Solitons Fractals 2006, 27, 685–688. [Google Scholar] [CrossRef]
- Pandey, A.; Baghel, R.K.; Singh, R.P. Analysis and circuit realization of a new autonomous chaotic system. Int. J. Electron. Commun. Eng. 2012, 5, 487–495. [Google Scholar]
- Silva-Juárez, A.; Tlelo-Cuautle, E.; de la Fraga, L.G.; Li, R. FPAA-based implementation of fractional-order chaotic oscillators using first-order active filter blocks. J. Adv. Res. 2020, 25, 77–85. [Google Scholar] [CrossRef] [PubMed]
- Du, M.; Wang, Z.; Hu, H. Measuring memory with the order of fractional derivative. Sci. Rep. 2013, 3, 3431. [Google Scholar] [CrossRef] [PubMed]
- Ilten, E.; Demirtas, M. Fractional order super-twisting sliding mode observer for sensorless control of induction motor. COMPEL—Int. J. Comput. Math. Electr. Electron. Eng. 2019, 38, 878–892. [Google Scholar] [CrossRef]
- Ozdemir, N.; Avci, D.; Iskender, B.B. The Numerical Solutions of a Two-Dimensional Space-Time Riesz-Caputo Fractional Diffusion Equation. Int. J. Optim. Control. Theor. Appl. IJOCTA 2011, 1, 17–26. [Google Scholar] [CrossRef]
- Calgan, H.; Demirtas, M. A robust LQR-FOPIλDµ controller design for output voltage regulation of stand-alone self-excited induction generator. Electr. Power Syst. Res. 2021, 196, 107175. [Google Scholar] [CrossRef]
- Yang, N.; Liu, C. A novel fractional-order hyperchaotic system stabilization via fractional sliding-mode control. Nonlinear Dyn. 2013, 74, 721–732. [Google Scholar] [CrossRef]
- Demirtas, M.; Ilten, E.; Calgan, H. Pareto-based multi-objective optimization for fractional order PI^λ speed control of induction motor by using Elman neural network. Arab J. Sci. Eng. 2019, 44, 2165–2175. [Google Scholar] [CrossRef]
- Ilten, E.; Demirtas, M. Off-Line Tuning of Fractional Order PIλ. J. Control. Eng. Appl. Inform. 2016, 18, 20–27. [Google Scholar]
- Garrappa, R. Numerical Solution of Fractional Differential Equations: A Survey and a Software Tutorial. Mathematics 2018, 6, 16. [Google Scholar] [CrossRef]
- Tepljakov, A. FOMCON: Fractional-Order Modeling and Control Toolbox. Fractional-Order Model. In Fractional-Order Modeling and Control of Dynamic Systems; Springer: Berlin/Heidelberg, Germany, 2017; pp. 107–129. [Google Scholar] [CrossRef]
- Valério, D.; Sá da Costa, J. Ninteger: A non-integer control toolbox for MatLab. In Proceedings of the First IFAC Workshop on Fractional Differentiation and Applications, Bordeaux, France, 19–21 July 2004; pp. 208–213. [Google Scholar]
- Danca, M.-F.; Kuznetsov, N. Matlab Code for Lyapunov Exponents of Fractional-Order Systems. Int. J. Bifurc. Chaos 2018, 28, 1850067. [Google Scholar] [CrossRef]
- Wolf, A.; Swift, J.B.; Swinney, H.L.; Vastano, J.A. Determining Lyapunov exponents from a time series. Phys. D Nonlinear Phenom. 1985, 16, 285–317. [Google Scholar] [CrossRef]
- He, S.; Sun, K.; Wang, H. Complexity Analysis and DSP Implementation of the Fractional-Order Lorenz Hyperchaotic System. Entropy 2015, 17, 8299–8311. [Google Scholar] [CrossRef]
- Xiong, P.-Y.; Jahanshahi, H.; Alcaraz, R.; Chu, Y.-M.; Gómez-Aguilar, J.; Alsaadi, F.E. Spectral Entropy Analysis and Synchronization of a Multi-Stable Fractional-Order Chaotic System using a Novel Neural Network-Based Chattering-Free Sliding Mode Technique. Chaos Solitons Fractals 2021, 144, 110576. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations, Mathematics in Science and Engineering; Academic Press: New York, NY, USA, 1999. [Google Scholar]






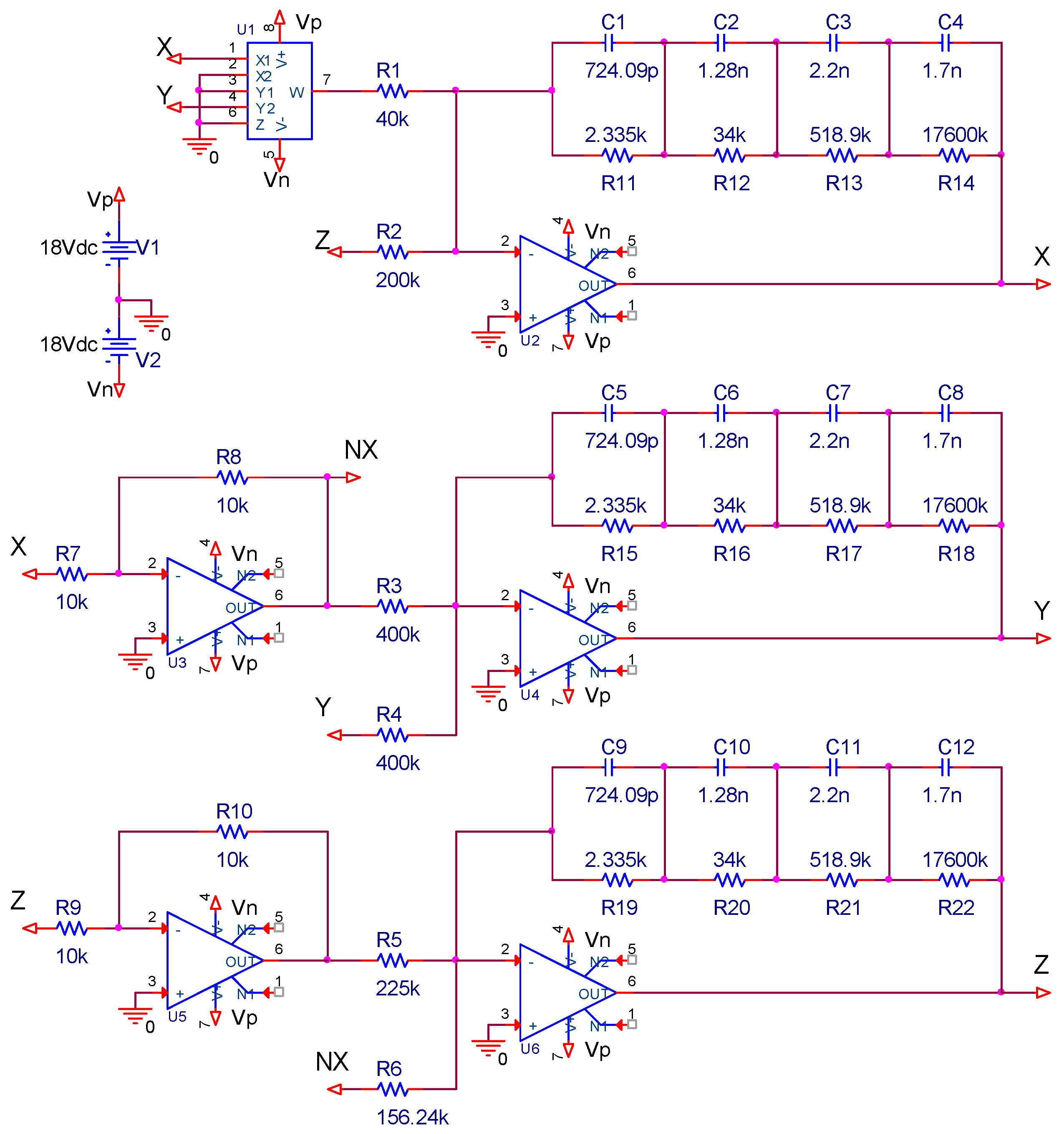
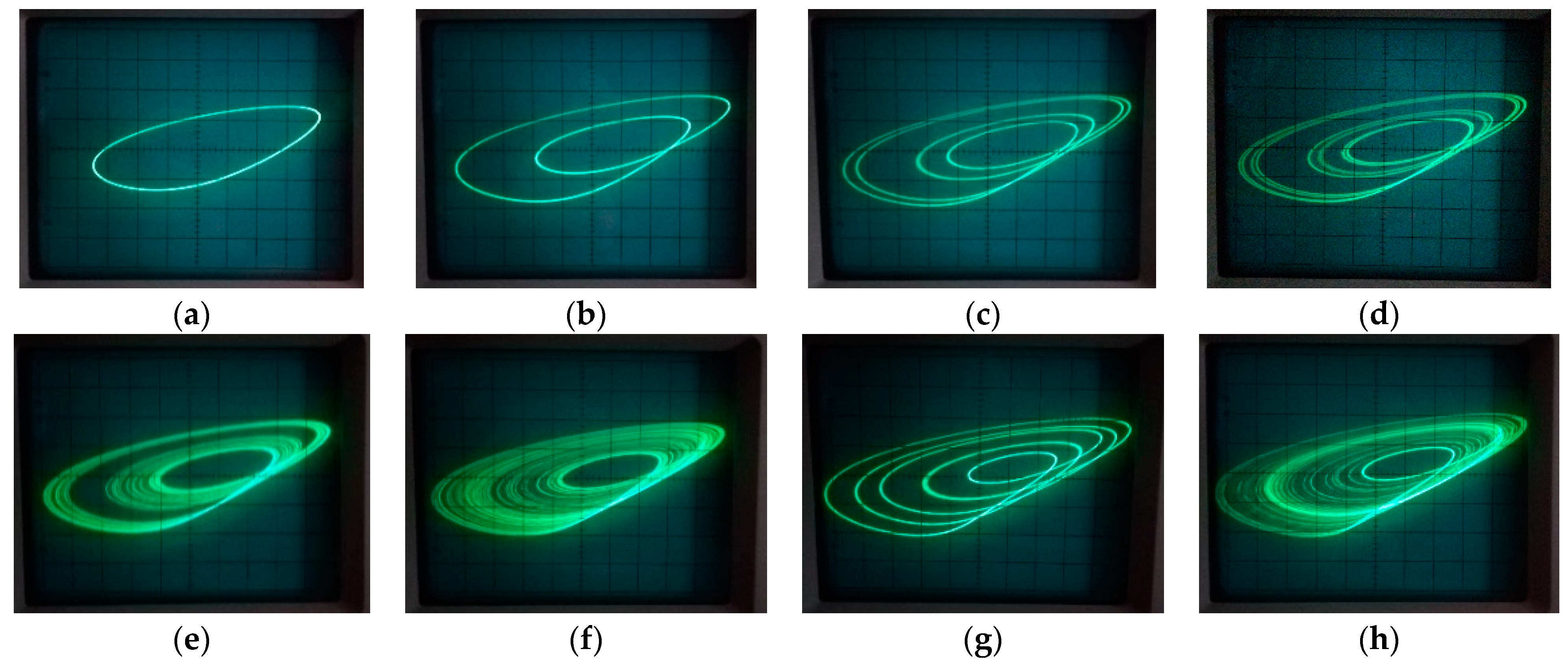
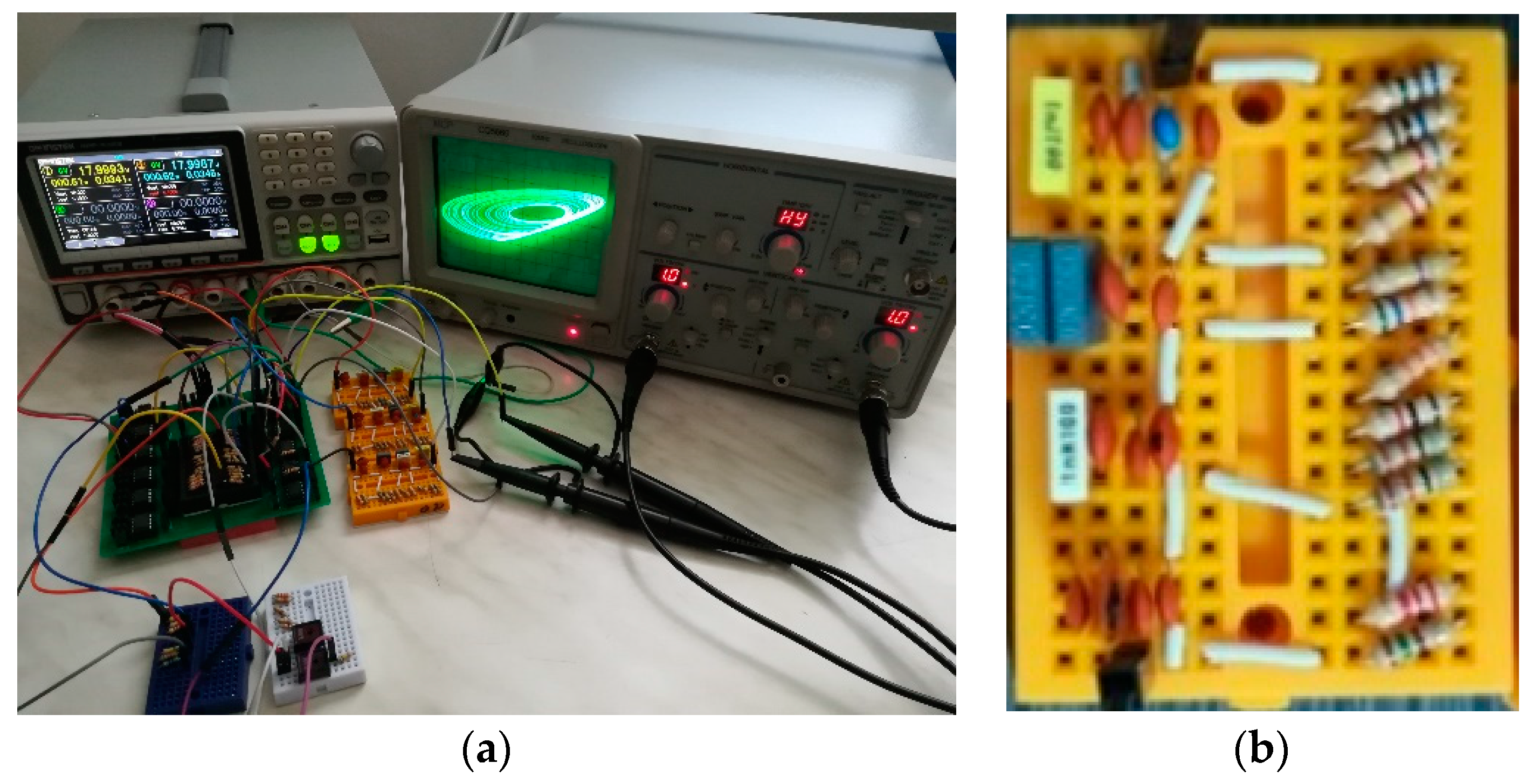
| q = 0.83 | q = 0.9 | |
|---|---|---|
| Ra | 2.335 kΩ | 2.53 kΩ |
| Rb | 34 kΩ | 253 kΩ |
| Rc | 518.9 kΩ | 62.922 MΩ |
| Rd | 17.6 MΩ | |
| Ca | 724.09 pF | 1.0984 nF |
| Cb | 1.28 nF | 1.833 nF |
| Cc | 2.2 nF | 1.23 nF |
| Cd | 1.7 nF |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gokyildirim, A. Circuit Realization of the Fractional-Order Sprott K Chaotic System with Standard Components. Fractal Fract. 2023, 7, 470. https://doi.org/10.3390/fractalfract7060470
Gokyildirim A. Circuit Realization of the Fractional-Order Sprott K Chaotic System with Standard Components. Fractal and Fractional. 2023; 7(6):470. https://doi.org/10.3390/fractalfract7060470
Chicago/Turabian StyleGokyildirim, Abdullah. 2023. "Circuit Realization of the Fractional-Order Sprott K Chaotic System with Standard Components" Fractal and Fractional 7, no. 6: 470. https://doi.org/10.3390/fractalfract7060470
APA StyleGokyildirim, A. (2023). Circuit Realization of the Fractional-Order Sprott K Chaotic System with Standard Components. Fractal and Fractional, 7(6), 470. https://doi.org/10.3390/fractalfract7060470






