On a Quadratic Nonlinear Fractional Equation
Abstract
1. Introduction
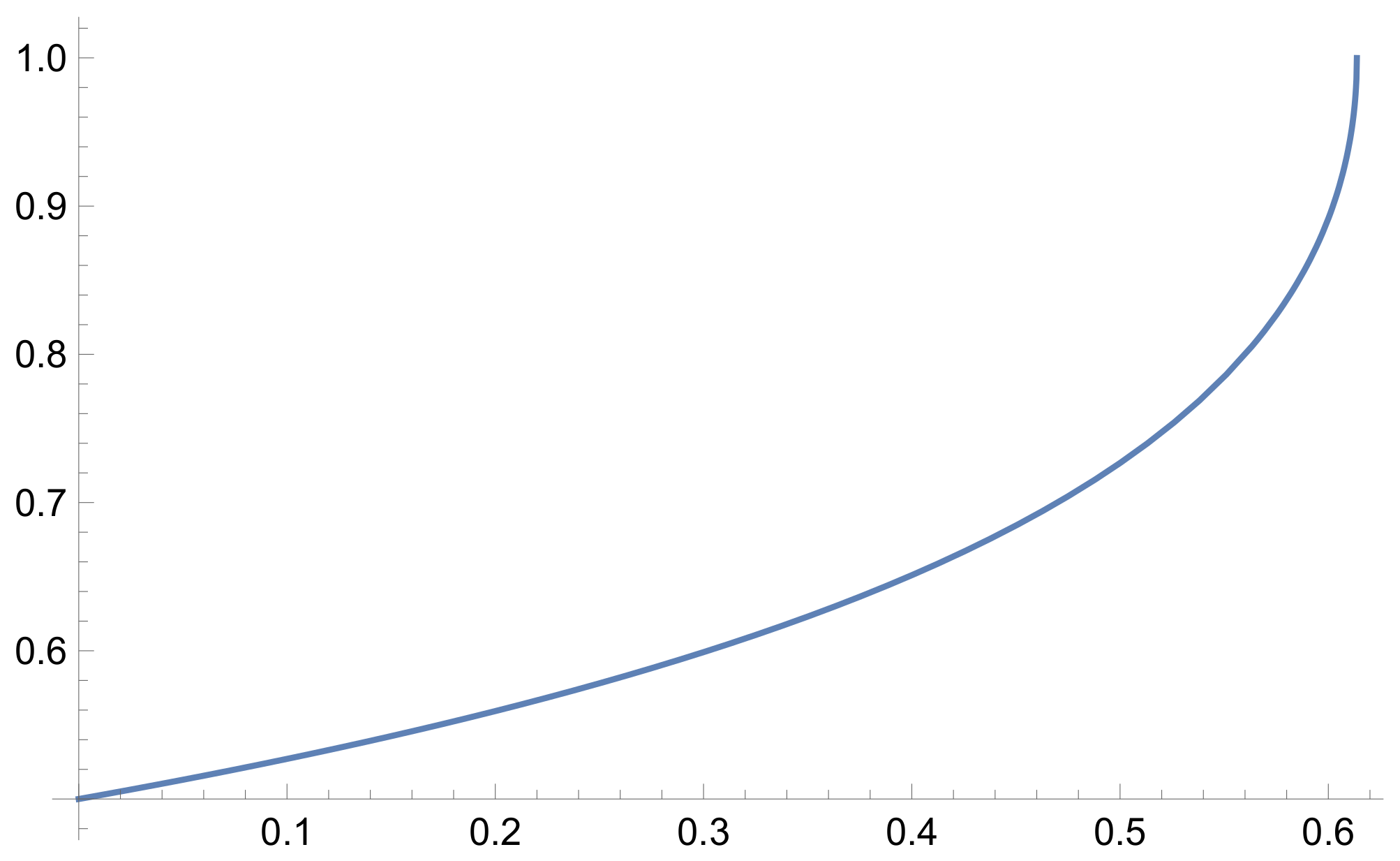
2. Example
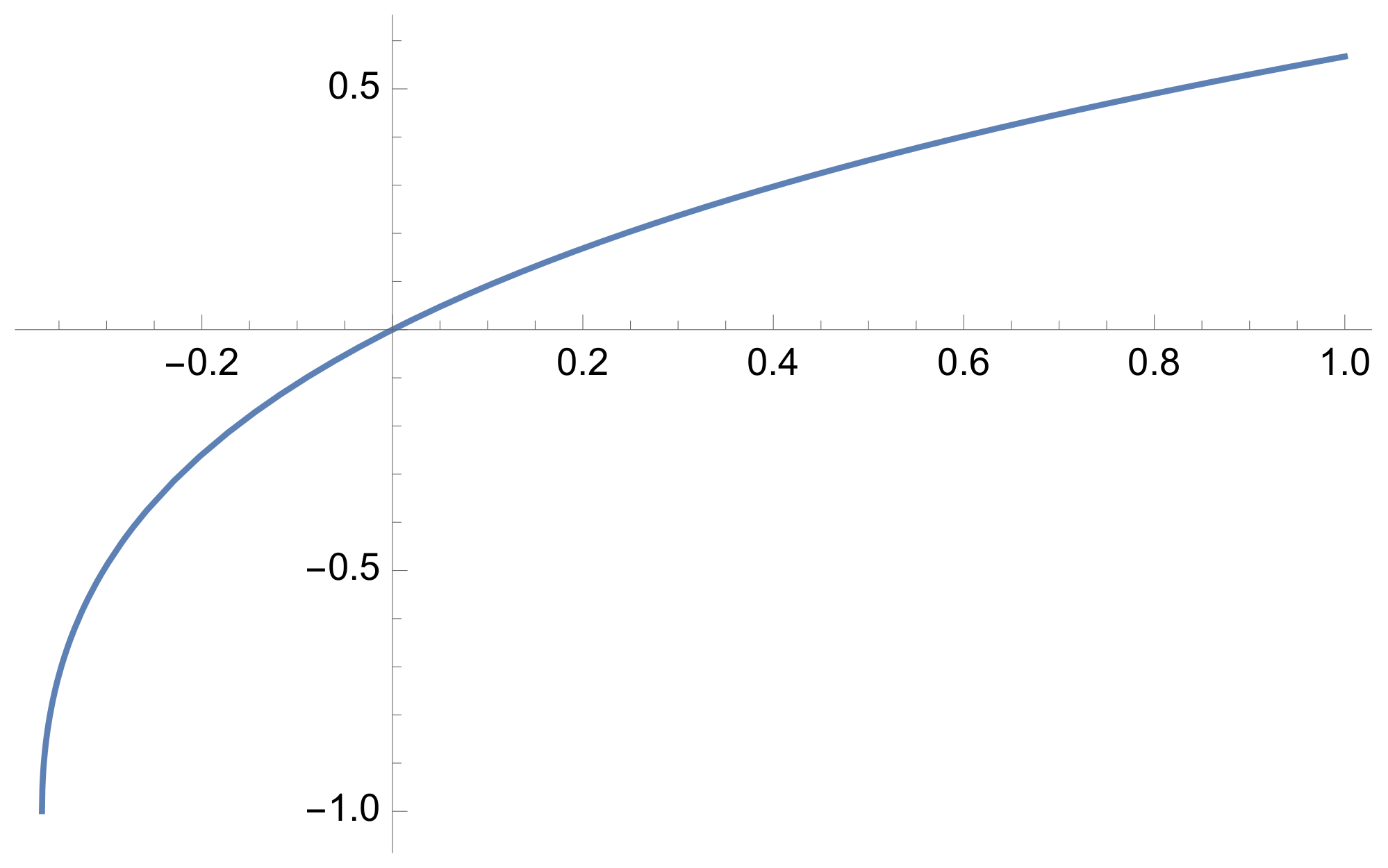
3. Lambert Function
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Area, I.; Nieto, J.J. Power series solution of the fractional logistic equation. Phys. A 2021, 573, 125947. [Google Scholar] [CrossRef]
- Wang, X.-S.; Wu, J.; Yang, Y. Richards model revisited: Validation by and application to infection dynamics. J. Theor. Biol. 2012, 313, 12–19. [Google Scholar] [CrossRef] [PubMed]
- Luo, X.; Duan, H.; Xu, K. A novel grey model based on traditional Richards model and its application in COVID-19. Chaos Solitons Fractals 2021, 142, 110480. [Google Scholar] [CrossRef] [PubMed]
- Smirnova, A.; Pidgeon, B.; Chowell, G.; Zhao, Y. The doubling time analysis for modified infectious disease Richards model with applications to COVID-19 pandemic. Math. Biosci. Eng. 2022, 19, 3242–3268. [Google Scholar] [CrossRef] [PubMed]
- Nieto, J.J. Solution of a fractional logistic ordinary differential equation. Appl. Math. Lett. 2022, 123, 107568. [Google Scholar] [CrossRef]
- Romashchenko, M.I.; Bohaienko, V.O.; Matiash, T.V.; Kovalchuk, V.P.; Krucheniuk, A.V. Numerical simulation of irrigation scheduling using fractional Richards equation. Irrig. Sci. 2021, 39, 385–396. [Google Scholar] [CrossRef]
- Berardi, M.; Difonzo, F.V.; Pellegrino, S.F. A numerical method for a nonlocal form of Richards’ equation based on peridynamic theory. Comput. Math. Appl. 2023, 143, 23–32. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of the Fractional Differential Equations. In North-Holland Mathematics Studies; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Caputo, M.; Fabrizio, M. On the singular kernels for fractional derivatives. Some applications to partial differential equations. Prog. Fract. Differ. Appl. 2021, 7, 79–82. [Google Scholar]
- Slimane, I.; Nazir, G.; Nieto, J.J.; Yaqoob, F. Mathematical analysis of Hepatitis C virus infection model in the framework of non-local and non-singular kernel fractional derivative. Int. J. Biomath. 2023, 16, 2250064. [Google Scholar] [CrossRef]
- Olver, F.W.J.; Daalhuis, A.B.O.; Lozier, D.W.; Schneider, B.I.; Boisvert, R.F.; Clark, C.W.; Mille, B.R.; Saunders, B.V.; Cohl, H.S.; McClain, M.A. NIST Digital Library of Mathematical Functions [Internet]. 2020. Available online: http://dlmf.nist.gov/ (accessed on 1 April 2023).
- Bronstein, M.; Corless, R.M.; Davenport, J.H.; Jeffrey, D.J. Algebraic properties of the Lambert W function from a result of Rosenlicht and of Liouville. Integral Transform. Spec. Funct. 2008, 19, 709–712. [Google Scholar] [CrossRef]
- Brauer, F.; Castillo-Chavez, C.; Feng, Z. Mathematical Models in Epidemiology; Springer: New York, NY, USA, 2019. [Google Scholar]
- Prodanov, D. Chapter 10-Analytical solutions and parameter estimation of the SIR epidemic model. In Mathematical Analysis of Infectious Diseases; Agarwal, P., Nieto, J., Torres, D., Eds.; Academic Press: Cambridge, MA, USA, 2022; pp. 163–189. ISBN 978-0-323-90504-6. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Area, I.; Nieto, J.J. On a Quadratic Nonlinear Fractional Equation. Fractal Fract. 2023, 7, 469. https://doi.org/10.3390/fractalfract7060469
Area I, Nieto JJ. On a Quadratic Nonlinear Fractional Equation. Fractal and Fractional. 2023; 7(6):469. https://doi.org/10.3390/fractalfract7060469
Chicago/Turabian StyleArea, Iván, and Juan J. Nieto. 2023. "On a Quadratic Nonlinear Fractional Equation" Fractal and Fractional 7, no. 6: 469. https://doi.org/10.3390/fractalfract7060469
APA StyleArea, I., & Nieto, J. J. (2023). On a Quadratic Nonlinear Fractional Equation. Fractal and Fractional, 7(6), 469. https://doi.org/10.3390/fractalfract7060469







