Efficient Solution of Fractional System Partial Differential Equations Using Laplace Residual Power Series Method
Abstract
:1. Introduction
2. Preliminaries
- 1.
- 2.
- 3.
3. LRPS Methodology
- and for each
- .
4. Application
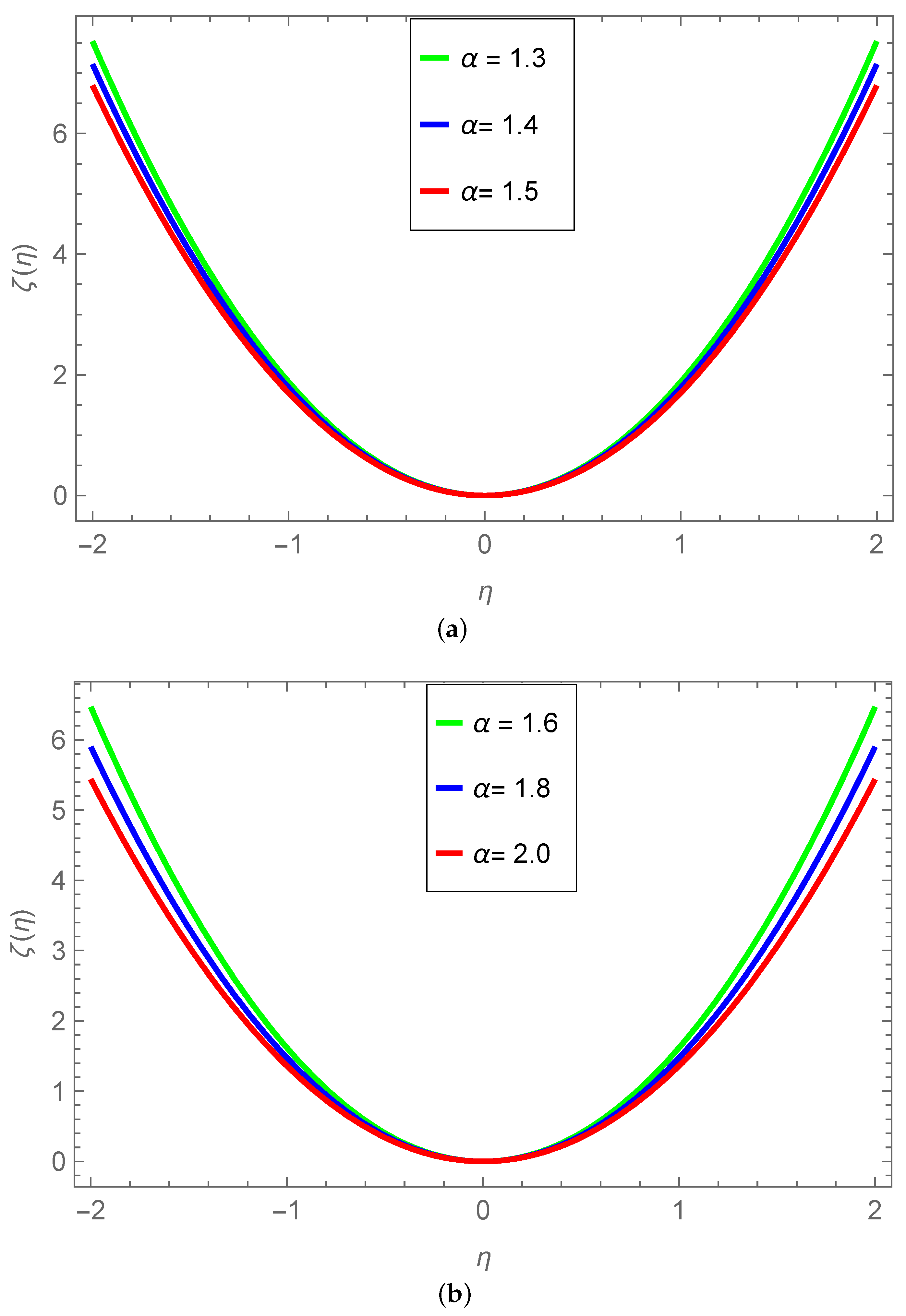
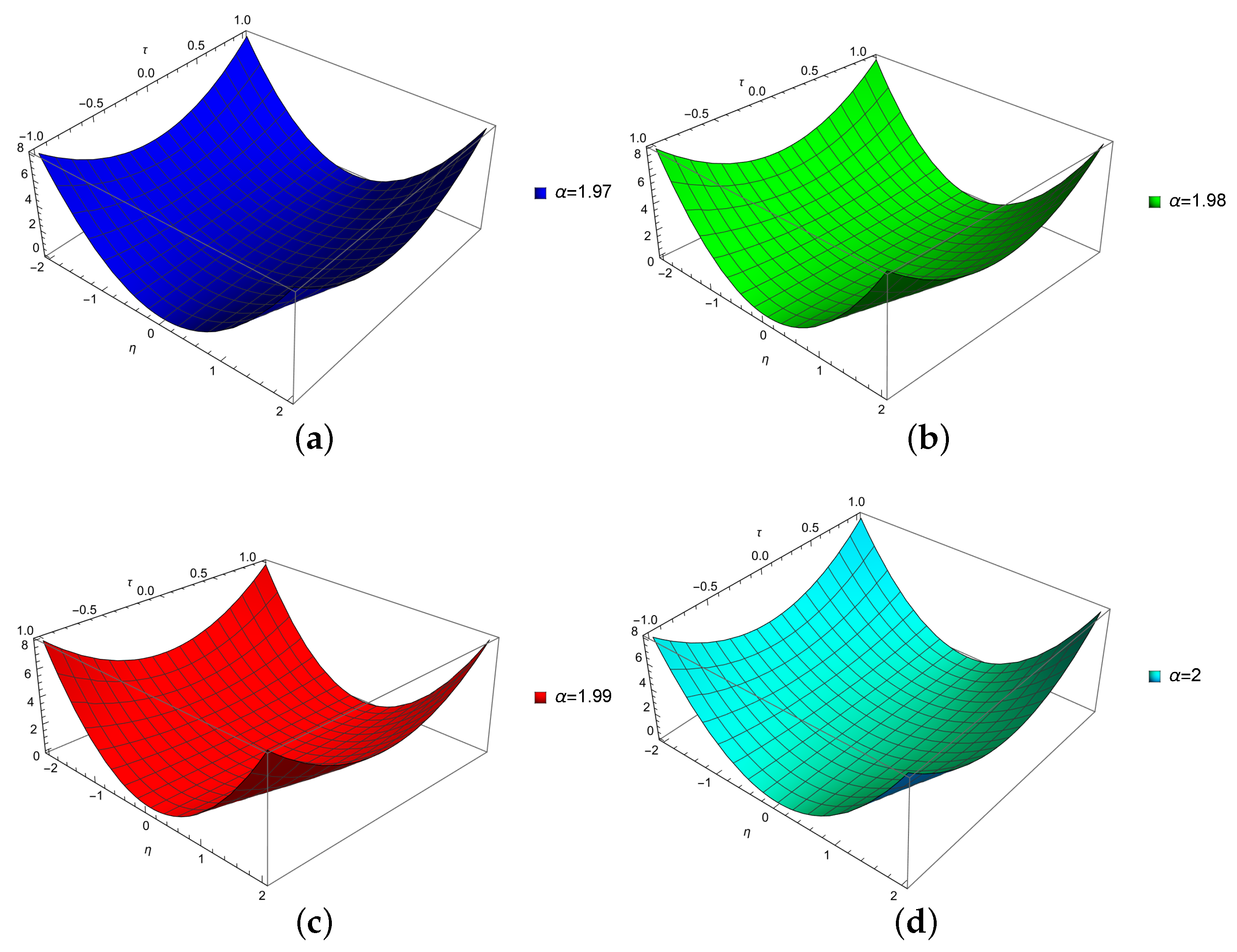
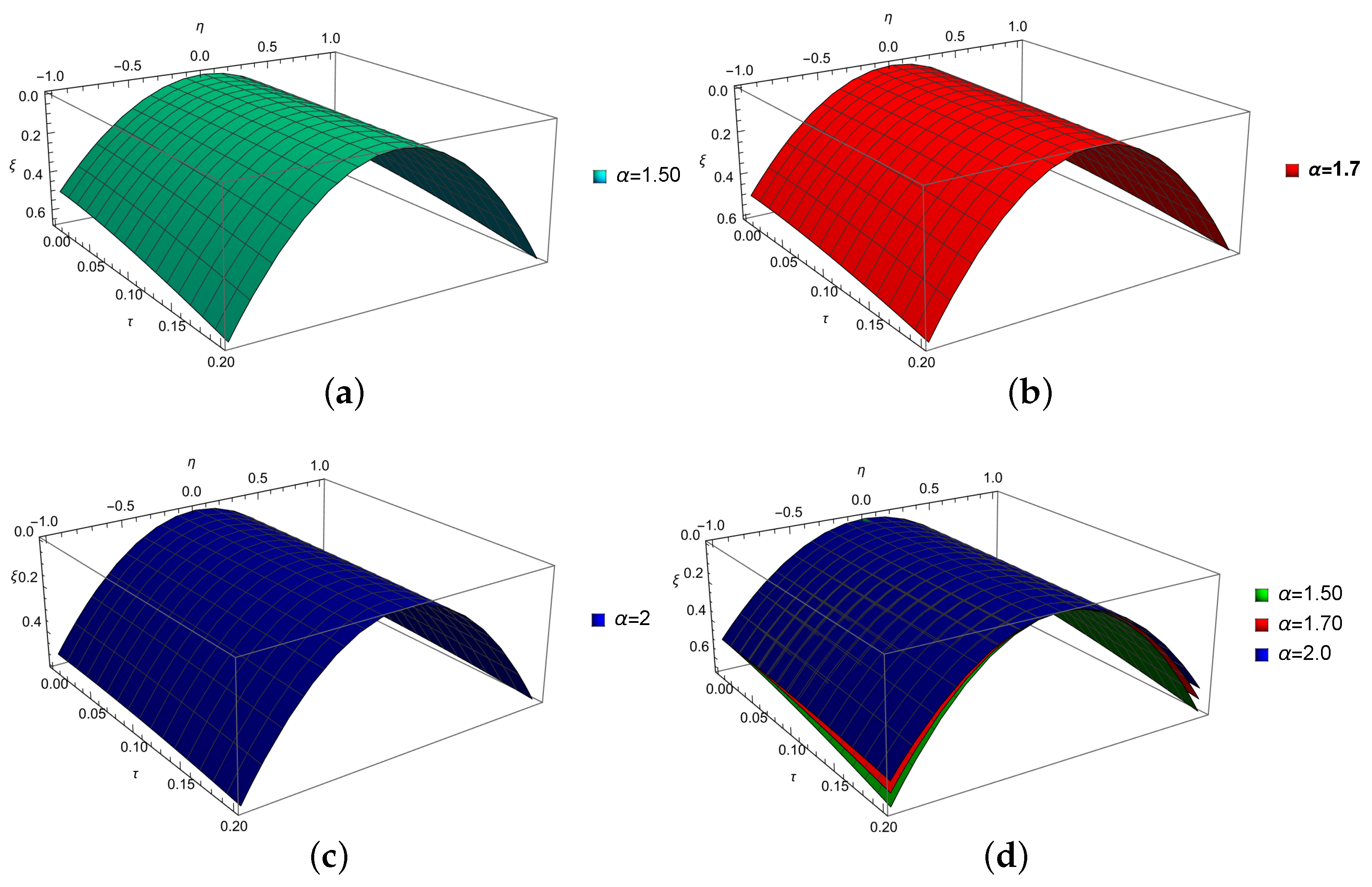
Problem
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chen, Y.; Liu, X. Numerical solution of time-fractional partial differential equations with multi-point differential inequalities. J. Comput. Appl. Math. 2020, 374, 114–127. [Google Scholar]
- Li, X.; Zhang, Y. A fractional gradient method for solving nonlinear partial differential equations with multi-point boundary conditions. J. Sci. Comput. 2021, 80, 474–498. [Google Scholar]
- Wang, L.; Zhou, X. A multi-point differential inequality based approach for solving fractional partial differential equations with variable coefficients. Commun. Nonlinear Sci. Numer. Simul. 2022, 85, 104835. [Google Scholar]
- Zhou, J.; Wang, X. A fractional calculus based method for solving partial differential equations with multi-point differential inequalities and variable coefficients. J. Math. Anal. Appl. 2020, 485, 1223–1247. [Google Scholar]
- Chen, Y.; Liu, X. A multi-point differential inequality based method for solving time-fractional partial differential equations with variable coefficients. Appl. Math. Comput. 2021, 401, 125532. [Google Scholar]
- Pitolli, F.; Sorgentone, C.; Pellegrino, E. Approximation of the Riesz-Caputo derivative by cubic splines. Algorithms 2022, 15, 69. [Google Scholar] [CrossRef]
- Izadi, M.; Srivastava, H.M. A discretization approach for the nonlinear fractional logistic equation. Entropy 2020, 22, 1328. [Google Scholar] [CrossRef] [PubMed]
- Shymanskyi, V.; Protsyk, Y. Simulation of the heat conduction process in the claydite-block construction with taking into account the fractal structure of the material. In Proceedings of the 2018 IEEE 13th International Scientific and Technical Conference on Computer Sciences and Information Technologies (CSIT), Lviv, Ukraine, 11–14 September 2018; Volume 1, pp. 151–154. [Google Scholar]
- Jin, H.; Wang, Z.; Wu, L. Global dynamics of a three-species spatial food chain model. J. Differ. Equ. 2022, 333, 144–183. [Google Scholar] [CrossRef]
- Li, Q.; Lin, H.; Tan, X.; Du, S. H ∞ Consensus for Multiagent-Based Supply Chain Systems Under Switching Topology and Uncertain Demands. IEEE Trans. Syst. Man Cybern. Syst. 2020, 50, 4905–4918. [Google Scholar] [CrossRef]
- Zhang, J.; Xie, J.; Shi, W.; Huo, Y.; Ren, Z.; He, D. Resonance and bifurcation of fractional quintic Mathieu-Duffing system. Chaos Interdiscip. J. Nonlinear Sci. 2023, 33, 23131. [Google Scholar] [CrossRef]
- Liu, L.; Wang, J.; Zhang, L.; Zhang, S. Multi-AUV Dynamic Maneuver Countermeasure Algorithm Based on Interval Information Game and Fractional-Order DE. Fractal Fract. 2022, 6, 235. [Google Scholar] [CrossRef]
- Dang, W.; Liao, S.; Yang, B.; Yin, Z.; Liu, M.; Yin, L.; Zheng, W. An encoder-decoder fusion battery life prediction method based on Gaussian process regression and improvement. J. Energy Storage 2023, 59, 106469. [Google Scholar] [CrossRef]
- Alyousef, H.A.; Shah, R.; Shah, N.A.; Chung, J.D.; Ismaeel, S.M.; El-Tantawy, S.A. The fractional analysis of a nonlinear mKdV equation with Caputo operator. Fractal Fract. 2023, 7, 259. [Google Scholar] [CrossRef]
- Gu, Q.; Tian, J.; Yang, B.; Liu, M.; Gu, B.; Yin, Z.; Zheng, W. A Novel Architecture of a Six Degrees of Freedom Parallel Platform. Electronics 2023, 12, 1774. [Google Scholar] [CrossRef]
- Naeem, M.; Yasmin, H.; Shah, R.; Shah, N.A.; Nonlaopon, K. Investigation of Fractional Nonlinear Regularized Long-Wave Models via Novel Techniques. Symmetry 2023, 15, 220. [Google Scholar] [CrossRef]
- Liu, M.; Gu, Q.; Yang, B.; Yin, Z.; Liu, S.; Yin, L.; Zheng, W. Kinematics Model Optimization Algorithm for Six Degrees of Freedom Parallel Platform. Appl. Sci. 2023, 13, 3082. [Google Scholar] [CrossRef]
- Alderremy, A.A.; Shah, R.; Shah, N.A.; Aly, S.; Nonlaopon, K. Comparison of two modified analytical approaches for the systems of time fractional partial differential equations. AIMS Math. 2023, 8, 7142–7162. [Google Scholar] [CrossRef]
- Ye, R.; Liu, P.; Shi, K.; Yan, B. State Damping Control: A Novel Simple Method of Rotor UAV with High Performance. IEEE Access 2020, 8, 214346–214357. [Google Scholar] [CrossRef]
- Alshehry, A.S.; Shah, R.; Shah, N.A.; Dassios, I. A reliable technique for solving fractional partial differential equation. Axioms 2022, 11, 574. [Google Scholar] [CrossRef]
- Li, X.; Dong, Z.; Wang, L.; Niu, X.; Yamaguchi, H.; Li, D.; Yu, P. A magnetic field coupling fractional step lattice Boltzmann model for the complex interfacial behavior in magnetic multiphase flows. Appl. Math. Model. 2023, 117, 219–250. [Google Scholar] [CrossRef]
- Shah, N.A.; Agarwal, P.; Chung, J.D.; El-Zahar, E.R.; Hamed, Y.S. Analysis of optical solitons for nonlinear Schrodinger equation with detuning term by iterative transform method. Symmetry 2020, 12, 1850. [Google Scholar] [CrossRef]
- He, H.M.; Peng, J.G.; Li, H.Y. Iterative approximation of fixed point problems and variational inequality problems on Hadamard manifolds. UPB Bull. Ser. A 2022, 84, 25–36. [Google Scholar]
- Shah, N.A.; Alyousef, H.A.; El-Tantawy, S.A.; Shah, R.; Chung, J.D. Analytical investigation of fractional-order Korteweg-De-Vries-type equations under Atangana-Baleanu-Caputo operator: Modeling nonlinear waves in a plasma and fluid. Symmetry 2022, 14, 739. [Google Scholar] [CrossRef]
- He, H.; Peng, J.; Li, H. Implicit viscosity iterative algorithm for nonexpansive mapping on Hadamard manifolds. Fixed Point Theory 2023, 24, 213–220. [Google Scholar] [CrossRef]
- Shah, N.A.; Hamed, Y.S.; Abualnaja, K.M.; Chung, J.D.; Shah, R.; Khan, A. A comparative analysis of fractional-order kaup-kupershmidt equation within different operators. Symmetry 2022, 14, 986. [Google Scholar] [CrossRef]
- Shah, N.A.; El-Zahar, E.R.; Akgul, A.; Khan, A.; Kafle, J. Analysis of fractional-order regularized long-wave models via a novel transform. J. Funct. Spaces 2022, 2022, 2754507. [Google Scholar] [CrossRef]
- Yuan, Q.; Kato, B.; Fan, K.; Wang, Y. Phased array guided wave propagation in curved plates. Mech. Syst. Signal Process. 2023, 185, 109821. [Google Scholar] [CrossRef]
- Aljarrah, H.; Alaroud, M.; Ishak, A.; Darus, M. Approximate solution of nonlinear time-fractional PDEs by Laplace residual power series method. Mathematics 2022, 10, 1980. [Google Scholar] [CrossRef]
- Oqielat, M.A.N.; Eriqat, T.; Al-Zhour, Z.; Ogilat, O.; El-Ajou, A.; Hashim, I. Construction of fractional series solutions to nonlinear fractional reaction-diffusion for bacteria growth model via Laplace residual power series method. Int. J. Dyn. Control. 2023, 11, 520–527. [Google Scholar] [CrossRef]
- Alderremy, A.A.; Iqbal, N.; Aly, S.; Nonlaopon, K. Fractional Series Solution Construction for Nonlinear Fractional Reaction-Diffusion Brusselator Model Utilizing Laplace Residual Power Series. Symmetry 2022, 14, 1944. [Google Scholar] [CrossRef]
- Gao, L. Free vibration analysis of non-uniform beams using Laplace residual power series method. J. Sound Vib. 2015, 337, 317–327. [Google Scholar]
- Zhang, Y.; Li, Y.; Liu, Y. Analysis of nonlinear dynamic systems by Laplace residual power series method. Commun. Nonlinear Sci. Numer. Simul. 2017, 44, 365–375. [Google Scholar]
- Liu, Y.; Li, Y.; Zhang, Y. Solutions of partial differential equations by Laplace residual power series method. J. Math. Anal. Appl. 2018, 456, 711–722. [Google Scholar]
- Liu, Y.; Li, Y.; Zhang, Y. Laplace residual power series method for modeling of nonlinear systems in control engineering. Nonlinear Dyn. 2019, 96, 543–559. [Google Scholar]
- Chen, X.; Liu, Y.; Li, Y. Analysis of nonlinear vibrations in mechanical systems by Laplace residual power series method. J. Vib. Control. 2020, 26, 1455–1467. [Google Scholar]
- Alquran, M.; Ali, M.; Alsukhour, M.; Jaradat, I. Promoted residual power series technique with Laplace transform to solve some time-fractional problems arising in physics. Results Phys. 2020, 19, 103667. [Google Scholar] [CrossRef]
- Alaroud, M. Application of Laplace residual power series method for approximate solutions of fractional IVP’s. Alex. Eng. J. 2022, 61, 1585–1595. [Google Scholar] [CrossRef]
- Kumar, R.; Koundal, R.; Ali Shehzad, S. Modified homotopy perturbation approach for the system of fractional partial differential equations: A utility of fractional Wronskian. Math. Methods Appl. Sci. 2022, 45, 809–826. [Google Scholar] [CrossRef]
| LRPSM | Exact | Abs. Error (LRPSM) | Abs. Error (GLSHP) | |
|---|---|---|---|---|
| 0.1 | 0.01 | 0.01 | 3.95169 | 4.59771 |
| 0.2 | 0.04 | 0.04 | 3.80677 | 4.59655 |
| 0.3 | 0.09 | 0.09 | 3.56523 | 4.59463 |
| 0.4 | 0.16 | 0.16 | 3.22707 | 4.59194 |
| 0.5 | 0.25 | 0.25 | 2.7923 | 4.58848 |
| 0.6 | 0.36 | 0.36 | 2.26091 | 4.58426 |
| 0.7 | 0.49 | 0.49 | 1.6329 | 4.57926 |
| 0.8 | 0.64 | 0.64 | 9.0828 | 4.5735 |
| 0.9 | 0.81 | 0.81 | 8.70417 | 4.56696 |
| 1.0 | 1.0 | 1.0 | 8.30813 | 4.55966 |
| LRPSM | Exact | Abs. Error (LRPSM) | Abs. Error (GLSHP) | |
|---|---|---|---|---|
| 0.1 | 0.005 | 0.005 | 1.92708 | 2.61859 |
| 0.2 | 0.02 | 0.02 | 1.70834 | 2.61798 |
| 0.3 | 0.045 | 0.045 | 1.34376 | 2.61698 |
| 0.4 | 0.08 | 0.08 | 8.33348 | 2.61557 |
| 0.5 | 0.125 | 0.125 | 1.77107 | 2.61377 |
| 0.6 | 0.18 | 0.18 | 6.24967 | 2.61156 |
| 0.7 | 0.245 | 0.245 | 1.57287 | 2.60895 |
| 0.8 | 0.32 | 0.32 | 2.66661 | 2.60594 |
| 0.9 | 0.405 | 0.405 | 3.90617 | 2.60253 |
| 1 | 0.5 | 0.5 | 5.29157 | 2.59871 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shafee, A.; Alkhezi, Y.; Shah, R. Efficient Solution of Fractional System Partial Differential Equations Using Laplace Residual Power Series Method. Fractal Fract. 2023, 7, 429. https://doi.org/10.3390/fractalfract7060429
Shafee A, Alkhezi Y, Shah R. Efficient Solution of Fractional System Partial Differential Equations Using Laplace Residual Power Series Method. Fractal and Fractional. 2023; 7(6):429. https://doi.org/10.3390/fractalfract7060429
Chicago/Turabian StyleShafee, Ahmad, Yousuf Alkhezi, and Rasool Shah. 2023. "Efficient Solution of Fractional System Partial Differential Equations Using Laplace Residual Power Series Method" Fractal and Fractional 7, no. 6: 429. https://doi.org/10.3390/fractalfract7060429
APA StyleShafee, A., Alkhezi, Y., & Shah, R. (2023). Efficient Solution of Fractional System Partial Differential Equations Using Laplace Residual Power Series Method. Fractal and Fractional, 7(6), 429. https://doi.org/10.3390/fractalfract7060429









