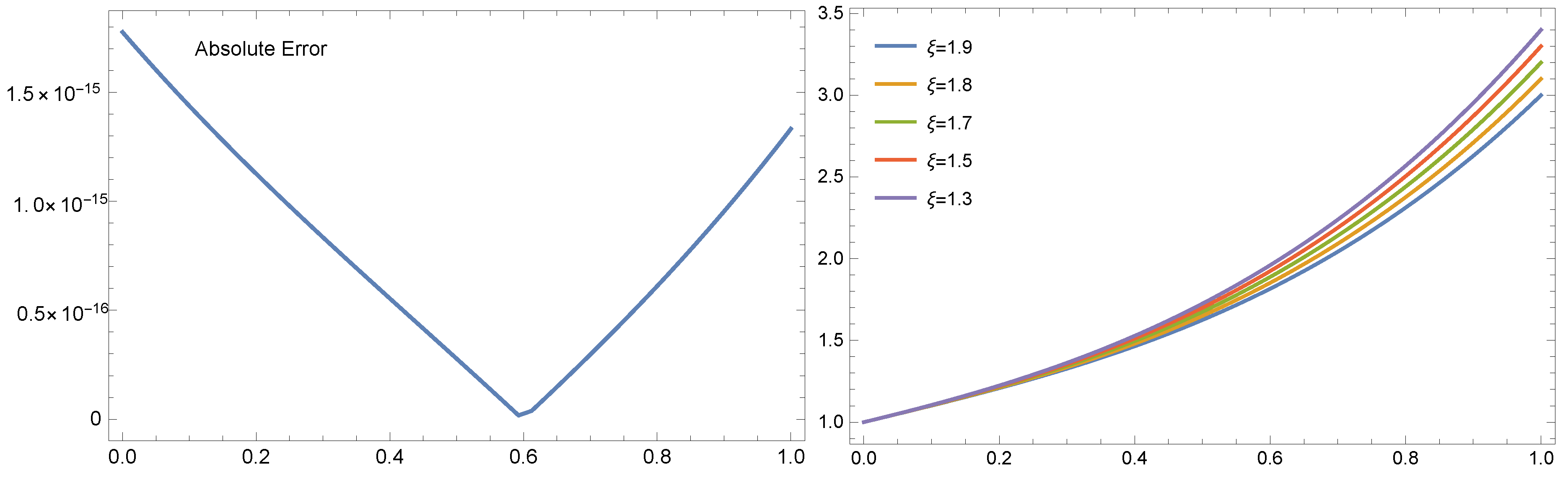1. Introduction
Fractional calculus is a branch of mathematics that deals with the study of derivatives and integrals of non-integer order. It has been around since the late 17th century when Gottfried Leibniz first proposed the concept of fractional derivatives, which has developed into a powerful tool for simulating different physical problems in many areas such as physics, chemistry, engineering, economics, and biology. The concept of fractional calculus was initially met with skepticism due to its unfamiliarity and lack of intuitive understanding. However, over time, its usefulness has been recognized and there have been various definitions and properties. These definitions vary depending on the context in which it is used, which in general have a common definition as the study of derivatives and integrals with non-integer orders. This means that instead of taking derivatives or integrals concerning a single variable (as in traditional calculus), fractional calculus allows for derivatives or integrals to be taken concerning multiple variables simultaneously. This allows for more complex phenomena such as memory effects, diffusion processes, and chaotic systems that might be difficult to solve using traditional definitions. In addition, fractional derivatives are useful in many fields such as physics, engineering, economics, and finance. For example, Zhao et al. [
1] investigated the possible application of fractional definitions to simulate a class of nonlinear fractional Langevin equations with important application in fluid dynamics. In addition, Zhang et al. [
2] employed an exponential Euler scheme for simulating the multi-delay Caputo–Fabrizio fractional-order differential equations with application in control theory. Additionally, other applications of fractional calculus in several branches of science and engineering include the simulation of the model of viscoelastic materials in engineering applications and financial markets in economics. They can also be used to describe chaotic systems in physics and other fields. There are various definitions of the fractional order including the Riemann–Liouville operator [
3], Grünwald–Letnikov operator [
4], Liouville–Caputo operator [
5] and Weyl–Riesz operator [
6]. Each of these definitions adheres to some advantages and disadvantageous over the other and the most widely used of these applications is the Liouville–Caputo and Riemann–Liouville operators. There is a close relationship between these two definitions since they can be converted through some regularity assumption [
7]. The Liouville–Caputo fractional operator is considered a powerful tool for solving fractional differential equations (FDEs) that have been used widely for simulating different complex problems. The Liouville–Caputo fractional operator is a generalization of the classical derivative operator and can be used to solve FDEs with non-integer order derivatives. It has the advantage of simulating physical phenomena that involve memory effects or non-local interactions. In addition, it allows for more accurate solutions since it takes into account memory effects and it provides more flexibility when solving FDEs; because it can be applied to any function that can be expressed as a power expansion series. This was one of the reasons to be used for the simulation of non-integer models. For example, the definition of the Liouville–Caputo operator has been used in simulating disease models. Bonyah et al. [
8] simulated the definition of the Liouville–Caputo for investigating the dynamics of the COVID-19 infection. In addition, the time-dependent influenza model has been studied in [
9] to provide insight into the dynamics of such a model and to provide measure precautions to stop its spread. Additionally, Gao et al. [
10] proposed a new fractional numerical differentiation formula for the Liouville–Caputo fractional derivative and tested the new formulae for multiple applications. Additionally, the constant proportional Liouville–Caputo operators were employed in [
11] for simulating the dynamics of the HIV disease model to understand its dynamics and ways of spread. Han et al. identified some solutions for the variable-coefficient fractional-in-space KdV equation in [
12]. Some basic therapies and applications of the fractional differential equations have been illustrated in [
13] while [
14,
15] provided some parametric and argument variations of the operators related to fractional calculus. With the importance of such definitions, the Liouville–Caputo operator is of importance in helping to understand such behavior of complex models.
Numerical simulation using collocation and spectral methods is a powerful tool for solving complex problems in engineering and science. It is a method of approximating solutions to differential equations by utilizing computational techniques such as collocation and spectral methods. Collocation methods are used to approximate solutions to various differential equations by representing them as a linear combination of basis functions. Spectral methods are used to approximate solutions to differential equations by representing them as an infinite series of (orthogonal) polynomials. Both of these techniques have been widely used in the field of computational science, with applications ranging from fluid dynamics to quantum mechanics. Collocation techniques have been widely used for acquiring accurate results for these models using different types of bases. The main idea of this technique is that the solution to a differential equation can be represented as a linear combination of basis functions. These basis functions can be chosen from a variety of sources including polynomials or other types. For example, Izadi et al. [
16] investigated the solution of the waste plastic management model in the ocean system using the Morgan–Voyce polynomials. In addition, Adel et al. [
17] employed a collocation method of Genocchi polynomials for simulating the solution to the fourth-order singular singularly perturbed and Emden–Fowler problems, which have significant importance in physics. Izadi et al. [
18] developed a collocation approach with a new definition of the Chelyshkov polynomials to solve the fractional delay differential equations. Additionally, El-Gamel et al. [
19] adapted the Genocchi collocation method for solving a class of high-order boundary value problems. The B-spline bases have been used to simulate physical models as well as other basis functions. For example, De Boor [
20] was the first to introduce the basic definitions of the B-spline basis, and then researchers have been using it to simulate real-life models. Kaur et al. [
21] employed the adaptive wavelet optimized finite difference technique combined with the B-spline polynomial for the solution of random partial differential equations. Zahra et al. [
22] developed a robust uniform B-spline collocation method for solving the generalized PHI-four equation. In addition, a cubic B-spline collocation algorithm has been used to solve the Newell–Whitehead–Segel type equations in [
23]. Additionally, the combination of the wavelet along with other polynomials has been used in the simulation of different models [
24] and Alqhtani et al. [
25] simulated a high-dimensional chaotic Lorenz system using the Gegenbauer wavelet polynomials. The coefficients in the linear combination are determined by solving an optimization problem that minimizes the error between the approximate solution and the exact solution. This approach is particularly useful when dealing with boundary value problems since it allows for accurate approximations near the boundaries without having to solve for all points in between. Spectral methods on the other hand are based on the idea that solutions to differential equations can be represented as an infinite series of orthogonal polynomials. These polynomials can be chosen from a variety of sources including the Chebyshev polynomials [
26] which have been used in the simulation of the fractional diffusion-wave equation by Atta et al. [
27]. Another type of polynomials is the Legendre polynomials [
28], which is also used for solving the linear Fredholm integro-differential equations accompanied by the Galerkin method by Fathy et al. [
29]. In addition, Abdelhakem et al. [
30] employed the pseudo-spectral matrices method for treating some models using the Legendre polynomials. More general orthogonal polynomials such as Hermite [
31] or Laguerre polynomials [
32] have also been used in practical simulations. This approach is particularly useful when dealing with initial value problems since it allows for accurate approximations at all points in time without having to solve for all points in between. Both of these techniques have been extensively studied over the past few decades, leading to significant advances in their accuracy and efficiency. They have become essential tools for solving complex problems in engineering and science, allowing researchers to accurately simulate physical phenomena with unprecedented accuracy and speed.
One of these polynomials that prove to have an effective role in simulating and acquiring efficient results is the Morgan–Voyce polynomials (MVP). This type of polynomial is a family of polynomials that was developed in the early 20th century by the American mathematician and physicist, Edward L. Morgan, see [
33]. Polynomials were initially developed as a tool to study the behavior of certain physical systems, such as electrical circuits and mechanical systems. These polynomials have since become an important tool in many areas of mathematics; for example, in algebraic geometry to study curves and surfaces defined by polynomial equations and in number theory to study Diophantine equations and prime numbers. Many researchers have recently been using the MVP accompanied by the collocation technique to solve engineering problems. For example, the MVP has been used to simulate a class of high-order differential equations by Türkyilmaz et al. in [
34]. Additionally, Tarakci et al. [
35] adapted a combination of the MVP with cubic and quadratic terms with the collocation strategy to simulate the nonlinear ordinary differential equations. Functional integro-differential equations of Volterra-type have been solved using the MVP collocation approach by Özel et al. [
36]. In addition, Izadi et al. [
37] employed the MVP to simulate the fractional Lotka–Volterra population model. Furthermore, Izadi et al. [
38] employed the shifted MVP for solving a class of nonlinear diffusion equations. Additionally, Bushra et al. [
39] proposed a collocation scheme for solving the Bratu problem with the aid of the MVP. With the little work on the application of the MVP, we are interested in expanding the application of such polynomials to fractional models.
In this research study, we are mainly interested in finding an accurate solution to a class of fractional order boundary value problems in the form
with the initial conditions
Here,
,
, and
in Equation (
1) are constant coefficients depending on the application type and the source term, respectively. In addition,
and
are the starting values for the problem’s solution and
is the fractional-order operator defined in Liouville–Caputo sense with the fractal value
. To the best of our knowledge, this is the first time that the MVP is utilized for solving the model (
1). This model incorporates a different form of fractional differential equations. One of the main models represented by the model (
1) is the Bagley–Torvik model. This model has been used to simulate the motion of a rigid plate immersed in a Newtonian fluid and also describes the behavior of a system of coupled oscillators and was first discovered by Torvik and Begly [
40]. Since then, it has been widely studied and applied to various fields such as nonlinear optics, fluid mechanics, and plasma physics. With the importance of this type of model, numerous analytical and numerical techniques have been employed to find accurate solutions to these problems. For examples, we mention neuro-swarming computational solver [
41], cubic B-spline method [
42], Haar wavelet [
43], fractional Meyer neural network [
44] and other related techniques. For more details and information, the reader may refer to the works [
45,
46,
47] and references therein.
In this paper, we interfered in simulating this model using the MVP with the definition of the fractional order in terms of the Liouville–Caputo fractional derivative. We adapt the proposed collocation method accompanied by the Tau method for simulating a different model of fractional order having real-life applications. We use MVP as the basis function in the collocation method because it has multiple advantages. Some of the advantages of the proposed technique using the MVP are the ability to accurately approximate functions with fewer terms than other methods, their ability to represent complex functions with a single equation, and their ability to be used in a wide variety of applications. Additionally, they can be used to solve equations that would otherwise be difficult or impossible to solve using traditional methods. On the other hand, there are some drawbacks to the complexity of the equations involved and they may not always provide an optimal solution for certain types of problems. The novelty of the paper lies within the following few points:
A novel operational matrix of fractional order is derived in the sense of the Liouville–Caputo fractional derivative for the MVP.
The technique is a combination of the collocation technique with the Tau method.
The method converts the nonlinear fractional differential equation into a system of algebraic equations that are solved easily.
The convergence analysis is performed to prove the error bound for the technique.
The proposed technique is adapted for solving various examples with the application including the Bagley–Torvik and Bratu models.
The acquired results prove that the technique is better than the other methods in terms of error and computational cost.
The proposed algorithm can be extended to more complex problems having real-life applications.
The organization of the rest of this paper can be summarized as the following.
Section 2 provides the basic definitions and preliminaries related to the fractional calculus that will be used later in the subsequent sections. The main relations and definitions of the MVP are introduced in
Section 3 with a derivation of the new fractional order matrix of differentiation. In
Section 4, the derivation of the integer and fractional operational matrix of derivatives is illustrated in detail and the Tau-collocation technique is demonstrated for solving the general model. In addition, the convergence analysis for the proposed technique is provided in detail in the same section to prove the convergence of the developed method. Several examples are introduced in
Section 5 to validate the theistical results in light of the absolute error and computational time. Finally,
Section 6 presents the conclusion of the study and some possible future work for the study.
5. Numerical Simulations
This section presents several examples that are solved numerically using our proposed method, i.e., the Morgan–Voyce operational matrix method (MVOMM). The numerical results of these examples support the analytical investigation and demonstrate the feasibility of the introduced technique. In the paper, two types of errors are used to evaluate the performance of the model: the error and the error. The error measures the average squared difference between the true values and the predicted values, while the error measures the maximum absolute difference between the true values and the predicted values. Additionally, the simulations were run using a Core-i7 laptop with 16 GB RAM and the used software is Mathematica 11.0.
Example 1 ([
51,
52,
53]).
Consider the following inhomogeneous Bagley–Torvik initial value problem:whereThe exact solution of Equation (30) is given by . We apply the investigated method to have the following result as described. In
Table 1, we present numerical results for
and its approximation
at various points in the interval
, obtained using the MVOMM with
. The results in this table demonstrate the high accuracy of the MVOM method. The CPU time that takes through obtaining these results at
m = 3 is 3.766 s. For comparison, we also include results obtained using the VIM and FIM methods from Mekkaouii and Hammouch [
52]. The last column in the table shows the exact values problem. As can be seen from the table, the MVOM technique with
yields more accurate results than the VIM, FIM, and LDG approaches.
Table 2 presents
-error and
-error results using our suggested method MVOMM in addition to the comparison with these results obtained via Lucas wavelet scheme (LWS) [
53]. From these results, we obtain the accuracy of the proposed method. For further illustration, we introduce
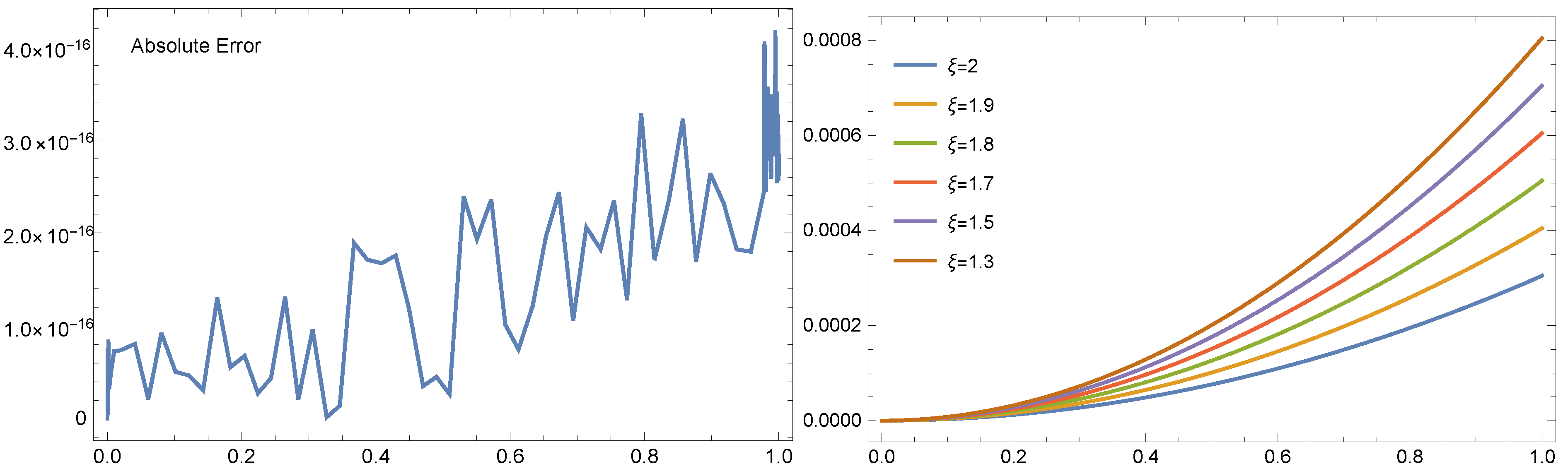
Figure 1, which shows the absolute error (left), and (right) the approximate solutions
for Example 1 with
. Clearly, from
Figure 1, the accuracy and efficiency of the MVOMM is useful for obtaining the numerical solutions in several cases. In addition, it can be noticed from the figure that while changing the value of the fractional order
, the value of the solution is increasing. This proves that the change in the fractional order has an impact on the simulation of the results.
Example 2 ([
51]).
In our next experiment, we will show that MVOM method can handle problems with discontinuities. To keep things simple, we will consider a model problem that only has one discontinuous point, but it is possible to extend the method to handle a larger number of discontinuities. We will consider a fractional-order Bagley–Torvik equation with an initial value and a discontinuous right-hand side.whereIn this case, is a point where the discontinuity occurs. The exact solutions to the problem are for the variable t in the interval and for t in the interval . We will assume that the discontinuous point coincides with a mesh node. For the purposes of this example, we will set
and
. Using
, we obtain the following approximations:
For Example 2, through both intervals
,
and with
, we obtain all the results via our suggested technique (MVOMM), reported in Equation (
32),
Table 3,
Figure 2 and
Figure 3. The results that were obtained by Equation (
32) indicate the approximate solutions were approximately consistent with the analytical solutions.
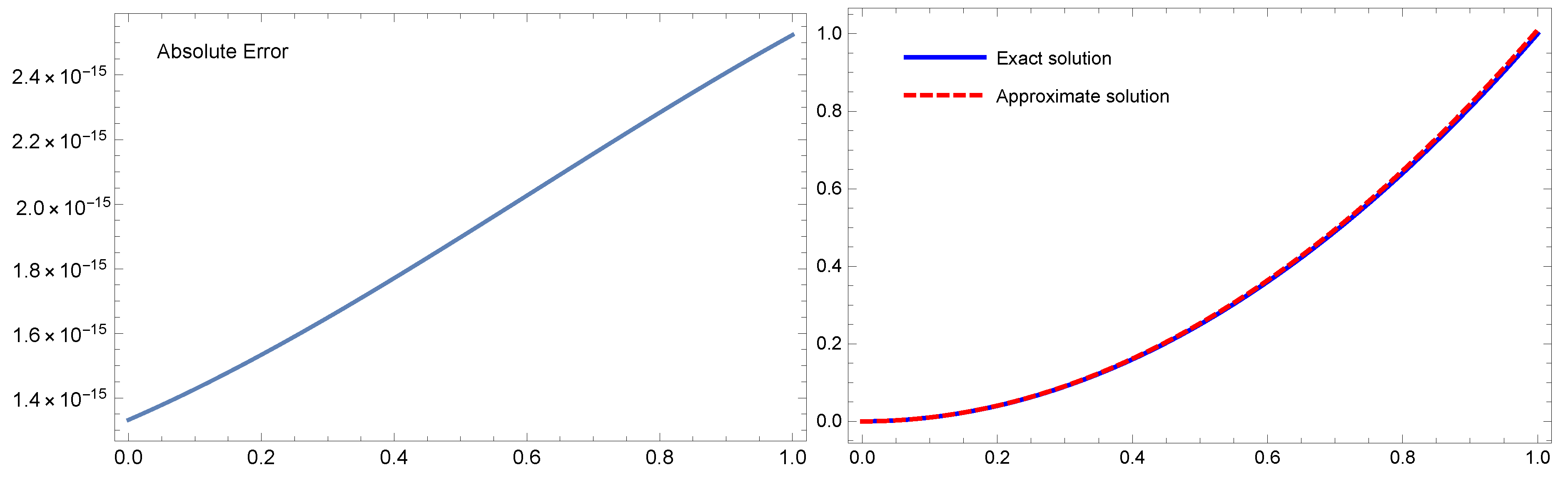
Table 3 represented the absolute error, which is very tiny.
Figure 2 shows the exact and approximate solutions (right), and the absolute error (left) at
.
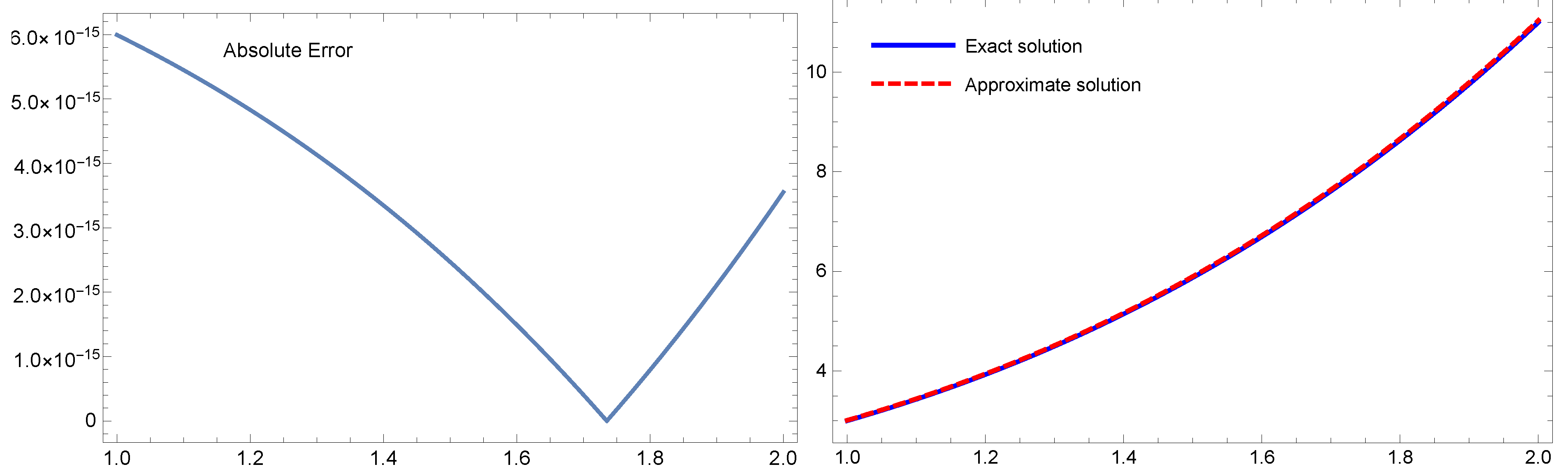
Figure 3 presents the exact and approximate solutions (right), and the absolute error (left) where
. Moreover, when
, the CPU time required to produce these results at
,
are
s,
s, respectively. Based on the presented results, we can say that our proposed algorithm gives high accuracy and efficiency.
Example 3 ([
51]).
For the last examination, we choose a case study that is representative of the types of issues encountered in the modeling of electrical and mechanical oscillations, in order to make the example more applicable and realistic to real-world situations:with the original state In our analysis of Equation (
33), we only considered the cases where the forcing function is a sinusoidal wave with an amplitude of
and a frequency of
. Using the MVOM scheme, we examined three different vibration problems for
and
values of 1. We obtain the numerical solution for these cases as the following:
Case I: and . The analytical solution is .
Case II: and . The precise solution .
Case III: and . The true solution is ,
where . Here, we have .
The proposed approach that was explained in the preceding Section is used to compute the absolute error for the three cases of Example 3. It is visible from analyzing the outcomes of
Table 4 that were generated by the proposed methodology and the results produced by the method provided in [
51] that the results provided by the proposed scheme are more accurate than those published in [
51]. Additionally, the CPU time of our method is better than of [
51] because we have few terms of the expansion series
only. We obtain the CPU time in these different cases (Case I, Case II, and Case III), which are
, and
s, respectively. A great degree of precision is also provided by the proposed method for solving oscillation problems.
Figure 4,
Figure 5 and
Figure 6 are reported at
for Example 3 through three different cases of oscillations.
Figure 4 gives the absolute error on left, the analytic and an approximation solutions on right for Case I.
Figure 5 presents the absolute error on left, the exact and numerical solutions on right for Case II.
Figure 6 indicates the absolute error on left, the exact and an approximation solutions on right for Case III. At first glance of these three figures, we notice a great degree of agreement between the exact and the numerical solution.
Example 4 ([
53,
54]).
Let us select the initial-value Bagley–Torvik equation of the fractional orderwith the boundary conditionsThe corresponding exact solution for Example 4 takes the form . We use the suggested method for solving this problem numerically. Additionally, some comparisons are made between the obtained results of MVOMM and the LWS and the reproducing kernel Hilbert space (RKHS) reported in [
53,
54]. By using the LWS, the obtained solution is [
53]
While the results with
using our method are as follows:
For Example 4 at
, we acquire all findings using our recommended technique (MVOMM), which is provided in Equation (
35),
Table 5,
Table 6 and
Figure 7. Equation (
35) shows that the approximations were roughly congruent with the analytical solutions.
Table 5, represented a comparison of the present study’s exact solution with the absolute inaccuracy of the techniques developed in [
53,
54].
Table 6, displays findings for
-error and
-error utilizing our recommended approach MVOMM as well as a comparison to results obtained via [
53].
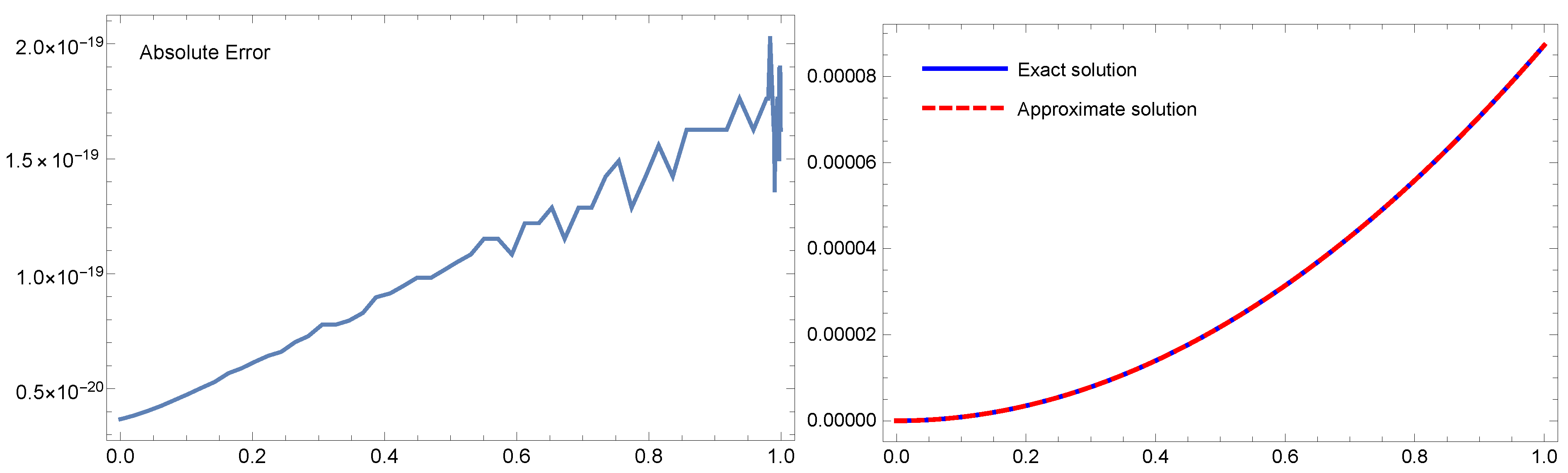
Figure 7 presents the absolute error (left) and the numerical and true solutions (right). All results are obtained with CPU time
s (including all numerical results and plotting the figures). The results derived from these Tables and Figures for Example 4 provide a strong indication of the superiority of the presented Tau-collocation algorithm. In terms of accuracy and efficiency, the proposed method was found to outperform the other methods considered.
Example 5 ([
55,
56,
57]).
Finally, we turn our attention to another form of equation, which is know as the fractional-order Bratu differential equation type:with the initial conditionsThe exact solution that corresponds to Example 5 at is . The following results are obtained by applying our recommended approach for solving this problem numerically and comparing the outcomes with those reported in [
55,
56,
57]. The developed methods are the compact finite difference method (CFDM), the reproducing kernel Hilbert space method (RKM), and the combined spectral Bessel quasilinearization method (Bessel-QLM), respectively. For Example 5, we obtain all the results utilizing our advised method (MVOMM), and these are presented in
Table 7 and
Table 8 and
Figure 8.
Table 7, introducing comparisons of the absolute error between the current methodology and the other research approaches, CFDM and RKM published in [
55,
56] with
. The computational time (CPU time) in the case of
is
s using our suggested method.
Table 8 reported a comparison between the recommended approach MVOMM and this given in [
55] with
-error and
-error at
and diverse values of
m. Additionally, the CPU time in different values of
m is reported in the last column of
Table 8.
Figure 8, listed the achieved absolute error for
(left) and the numerical values at several values of the fractional-order term
(right) with
. These Tables and Figures for numerical results of Example 5 give clear evidence of the proposed method’s superiority. The proposed method was found to perform better than the other existing numerical procedures taken into consideration in terms of efficiency and accuracy.