Pricing European Options under a Fuzzy Mixed Weighted Fractional Brownian Motion Model with Jumps

Abstract

This study investigates the pricing formula for European options when the underlying asset follows a fuzzy mixed weighted fractional Brownian motion within a jump environment. We construct a pricing model for European options driven by fuzzy mixed weighted fractional Brownian motion with jumps. By converting the partial differential equation (PDE) into a Cauchy problem, we derive explicit solutions for both European call options and European put options. The figures and tables demonstrating the effectiveness of the results highlight the suitability of the fuzzy mixed weighted fractional Brownian motion with jump model for option pricing.

1. Introduction

Since Black and Scholes [1] proposed the Black–Scholes model in 1973, a pioneering development of an option pricing model, the modern theory of option pricing has undergone rapid advancement. However, the assumptions within the Black–Scholes model, namely the geometric Brownian motion of the underlying asset price and constant volatility, fail to conform to the realities of financial markets. Consequently, substantial disparities between the theoretical prices calculated using the Black–Scholes model and actual market prices have emerged. As a result, scholars have undertaken efforts to enhance and expand the Black–Scholes model, as evidenced in scholarly works [2,3,4], where researchers have proposed the utilization of fractional Brownian motion (fBm in short) models exhibiting long-memory features as an enhancement to the classical Black–Scholes model.

Fractional Brownian motion represents a self-similar Gaussian process with stationary increments, characterized by the Hurst parameter H. The value of this parameter determines the degree of long-term dependence within the process. Recently, Mehrdoust and Najafi [5] succeeded in deducing explicit solutions for the fractional Black–Scholes model, characterized by a somewhat less resilient payoff function. Although fBm could effectively encompass the extended correlations among returns spanning different days, Rogers [6] showcased that it concurrently introduced the potential for arbitrage opportunities. To address this challenge and accommodate the enduring memory characteristic, EI-Nouty [7] and Mishura [8] illustrated the feasibility of employing a mixed fractional Brownian motion (mfBm in short), thereby capturing the intermittent variations in financial assets. Moreover, Cheridito [9] established the equivalence between the mfBm and a Brownian motion under the condition of the Hurst exponent $H\in (\frac{3}{4},1)$. This mathematical equivalence removes the potential for arbitrage, enabling valid financial use. For more applications of mfBm on financial models, please refer to [10,11,12]. Recently, another type of fractional Gaussian process–sub-fractional Brownian motion was also used in applications for option pricing, as seen in [13,14,15]. For example, Guo et al. [15] studied the issue of time-varying implied volatility of options and applied its model to option pricing under sub-mixed fractional Brownian motion. The article concluded, through comparative analysis of four models, namely Brownian motion, fractional Brownian motion, sub-fractional Brownian motion, and sub-mixed fractional Brownian motion, that the model of sub-mixed fractional Brownian motion exhibited the best pricing performance.

Bojedcki et al. [16] proposed an extension to fractional Brownian motion called weighted fractional Brownian motion (weighted-fBm in short). This extension incorporates a weighting function and exhibits non-stationary increments, thus offering a more flexible dependency structure. From this vantage point, the model of weighted-fBm might be better suited for capturing the behavior of financial systems. In such systems, investors often delay decisions until information surpasses a particular threshold. Nevertheless, according to the literature [17], it can be understood that the pricing model of the underlying asset driven by a fractional Gaussian process may lead to arbitrage opportunities. To avoid this issue, the commonly employed method is to construct a linear combination of a standard Brownian motion and a fractional Gaussian process (for example, a fractional Brownian motion or a sub-fractional Brownian motion), as described in [9,10,11,12,13,14,15], among others. This research also adopts such an approach, constructing a mixture of standard Brownian motion and weighted-fBm as a linear combination. Khalaf et al. [18] pointed out that this mixture, along with the asymptotic stationary properties for weighted-fBm, opened up the possibility of the mixture being a martingale or equivalent to Brownian motion, as it is the same as mfBm. This indicates that the pricing model of the underlying asset driven by a mixed weighted fractional Brownian motion is arbitrage-free.

Furthermore, the uncertainty in financial markets stems not only from stochasticity but also ambiguity. In practice, recorded financial data contain inaccuracies, especially for risk-free rates and volatility. For instance, the risk-free rate used in pricing European options is only an approximation, since different institutions have varying rates. Zadeh [19] pioneered fuzzy set theory in 1965, providing a key technique for modeling ambiguous markets. Wu [20] then incorporated fuzzy sets into option pricing, proposing fuzzy pricing models for European options. Fuzzy set theory can be employed to quantify uncertainty and risk, facilitating a more comprehensive assessment of option prices. By introducing fuzzy random variables, the impact of various factors on option prices can be considered, contributing to a more accurate reflection of market uncertainty. In this study, the triangular fuzzy number is introduced for the estimation of relevant parameters. The choice of employing the triangular fuzzy number is based on their relatively simple representation and form, making them more easily interpretable and understandable. The triangular fuzzy number offers an intuitive grasp of the boundaries and central tendencies of a fuzzy set.

Recently, Zhang et al. [21] discussed the pricing problem of European options under the assumption that the underlying stock price, the risk-free interest rate, the volatility, the jump intensity, and the mean value and variance of jump magnitudes are all fuzzy numbers. Bian and Li [22] used stochastic analysis, fractal theory, and fuzzy set theory to construct a European option pricing model based on the long-term memory property of the financial market in an uncertain environment, and the conclusions showed that the European option pricing model with long-term memory property was more suitable for financial markets in an uncertain environment. More related studies can be referred to in [23,24,25]. To tackle these challenges, we propose a new framework for pricing European options under a fuzzy mixed weighted-fBm model with jumps. This flexible model incorporates both mixed weighted-fBm to capture long-range dependence and jumps to represent sudden price changes, while using fuzzy sets to model inherent data uncertainty. By capturing a wider range of asset price behaviors, our approach is similar to the treatment of [21] in enhancing the accuracy of options pricing.

The subsequent sections of this paper are structured as follows: Section 2 expounds on the mixed weighted-fBm and fuzzy set theory, providing essential preliminary knowledge. In Section 3, we derive the corresponding It$\widehat{\mathrm{o}}$’sformula for the asset price propelled by the mixed weighted-fBm with jumps, and provide the expressions for the underlying asset price. In Section 4, we acquire the Black–Scholes partial differential equation along with the solution in a closed-form for European options. In Section 5, we present a fuzzy stochastic process that employs fuzzy random variables to define the underlying asset price process, and deduce the $\alpha$-cut of the fuzzy price of European options. Section 6 presents numerical results using European call options. The findings demonstrate the sensitivity of the fuzzy option prices to changes in b and $\alpha$. Section 7 provides a conclusion.

2. Preliminaries

Let $\{\mathrm{\Omega },{\mathcal{F}}_t,P\}$ be a complete probability space, endowed with a filtration ${\left\{{\mathcal{F}}_t\right\}}_{t\ge 0}$ that adheres faithfully to the usual conditions.

2.1. Mixed Weighted-fBm

 Definition 1. 

The mixed weighted-fBm  ${\xi }_t={\left\{{\xi }_t({\sigma }_1,{\sigma }_2)\right\}}_{t\ge 0}$  is a linear combination of the Brownian motion  ${\left\{B_t\right\}}_{t\ge 0}$  and the weighted-fBm  ${\left\{B_t^{a,b}\right\}}_{t\ge 0}$,  which can be expressed as 

$${\xi }_t({\sigma }_1,{\sigma }_2)={\sigma }_1{B}_t+{\sigma }_2{B}_t^{a,b},\forall t\ge 0,$$

 where  $a,b$  are the index and satisfy the condition  $a>-1,\left|b\right|<1,\left|b\right|<a+1$. ${\sigma }_1,{\sigma }_2$  are positive constants;  ${\left\{B_t\right\}}_{t\ge 0}$  and  ${\left\{B_t^{a,b}\right\}}_{t\ge 0}$  are independent of each other.  ${\left\{B_t^{a,b}\right\}}_{t\ge 0}$ is defined by [26].

In what follows, we present certain attributes of the mixed weighted-fBm through the proposition delineated hereafter. For more detailed information about the properties of the mixed weighted-fBm, one can refer to [18,26].

 Proposition 1. 

The mixed weighted-fBm  ${\xi }_t={\left\{{\xi }_t({\sigma }_1,{\sigma }_2)\right\}}_{t\ge 0}$  has the following properties: 

• ${\left\{{\xi }_t({\sigma }_1,{\sigma }_2)\right\}}_{t\ge 0}$  is a central Gaussian process. 
• When  $t=0$, ${\xi }_o({\sigma }_1,{\sigma }_2)={\sigma }_1{B}_0+{\sigma }_2{B}_0^{a,b}=0$.
• $\forall t,s\ge 0$,  the covariance of  ${\xi }_t({\sigma }_1,{\sigma }_2)$  and  ${\xi }_s({\sigma }_1,{\sigma }_2)$  is 

$$\begin{array}{ccc}& & Cov\left({\xi }_t({\sigma }_1,{\sigma }_2),{\xi }_s({\sigma }_1,{\sigma }_2)\right)\hfill \\ & & ={\sigma }_1^2\left(t\wedge s\right)+\frac{1}{2\mathbb{B}(a+1,b+1)}{\sigma }_2^2{\int }_0^{t\wedge s}{u}^a\left({(t-u)}^a+{(s-u)}^b\right)du,\hfill \end{array}$$

where  $t\wedge s=\frac{1}{2}\left(t+s-|t-s|\right)$,  and  $\mathbb{B}(·,·)$  is the beta function. 

2.2. Fuzzy Set Theory

 Definition 2 

(Fuzzy number [20]). $\tilde{a}$  represents a fuzzy subset of the set of real numbers, denoted by R.  $\tilde{a}$  earns the appellation of a fuzzy number under the following conditions: 

 (1) 

$\tilde{a}$  qualifies as both a normal fuzzy set and a convex fuzzy set; 

 (2) 

The membership function  ${\mu }_{\tilde{a}}$ of $\tilde{a}$  exhibits upper semi-continuity; 

 (3) 

The α-level set  ${\tilde{a}}_{\alpha }$ of $\tilde{a}$  remains confined within boundaries. 

If $\tilde{a}$ is a fuzzy number, its $\alpha$-cut set ${\tilde{a}}_{\alpha }$ is a bounded closed interval. We can denote ${\tilde{a}}_{\alpha }=\left[{\tilde{a}}_{\alpha }^L,{\tilde{a}}_{\alpha }^U\right]$. When ${\tilde{a}}_{\alpha }^L={\tilde{a}}_{\alpha }^U=a$, then we have ${\tilde{a}}_{\alpha }=a$.

 Definition 3 

(Triangle fuzzy number [27]). Allow  $\tilde{a}=(a_1,a_2,a_3)$   to denote a veritable fuzzy set within the realm of Group R, and it earns the distinguished title of a triangular fuzzy number when its membership function is meticulously defined as such: 

$$\begin{array}{c}\hfill {\mu }_{\tilde{a}\left(x\right)}=\left\{\begin{array}{cc}\frac{x-a_1}{a_2-a_1},\hfill & a_1\le x\le a_2,\hfill \\ \frac{x-a_3}{a_2-a_3},\hfill & a_2\le x\le a_3,\hfill \\ 0,\hfill & x>a_3.\hfill \end{array}\right.\end{array}$$

The utmost despondent extent of uncertainty, denoted as $\tilde{a}$, is embodied by $a_1$, while the zenith of optimism is marked by $a_3$, and at the heart of it all lies $a_2$, symbolizing the most probable value. The $\alpha$-cut of $\tilde{a}$ manifests itself as a sealed interval, elegantly articulated as follows:

$${\tilde{a}}_{\alpha }=\left[(1-\alpha )a_1+\alpha a_2,(1-\alpha )a_3+\alpha a_2\right],$$

where ${\tilde{a}}_{\alpha }^L=(1-\alpha )a_1+\alpha a_2,{\tilde{a}}_{\alpha }^U=(1-\alpha )a_3+\alpha a_2.$

3. Asset Pricing Model

In this research, we embrace the venerable theory of financial stochastic analysis, weaving extensions into the fabric of the Black–Scholes model. Moreover, the ensuing suppositions endure as follows:

(i)

The temporality of transactions and the magnitude of assets persist in a seamless continuum;

(ii)

Transaction expenses and fiscal levies remain conspicuously absent from the equation;

(iii)

The dealing involving assets faces no constriction, allowing for both short selling and short buying without hindrance;

(iv)

The return of risk-free assets in time period t  is

$$d{M}_t=r{M}_{t}dt,$$

(1)

where constant r  is the risk-free interest rate;

(v)

The risk assets (stocks) price $S_t$ is driven by the mixed weighted-fBm with jumps:

$$\begin{array}{cc}\hfill d{S}_t& =\mu S_{t}dt+S_{t}d{\xi }_t({\sigma }_1,{\sigma }_2)+\gamma S_{t}d{J}_t\hfill \\ \hfill & =\mu S_{t}dt+{\sigma }_1{S}_{t}d{B}_t+{\sigma }_2{S}_{t}d{B}_t^{a,b}+\gamma S_{t}d{J}_t,\hfill \end{array}$$

(2)

where $\mu$ is the instantaneous expected return of the stock; ${\sigma }_1,{\sigma }_2$, and $\gamma$ represent the volatility of stock prices; and ${\left\{J_t\right\}}_{t\ge 0}$ is a compensated Poisson process with intensity $\lambda$. ${\left\{B_t\right\}}_{t\ge 0},{\left\{B_t^{a,b}\right\}}_{t\ge 0}$, and ${\left\{J_t\right\}}_{t\ge 0}$ are independent of each other.

 Theorem 1. 

Let us postulate that  $Y_t$  takes the form of  $Y_t={\xi }_t({\sigma }_1,{\sigma }_2)+\gamma J_t$  with an initial value of zero, while  $f(t,Y_t)$  exhibits second-order differentiability. Consequently, It $\widehat{\mathrm{o}}$’s formula for the mixed weighted fractional Brownian motion with jumps can be elegantly articulated as such: 

$$\begin{array}{ccc}\hfill f(t,Y_t)& & =f(0,0)+{\int }_0^t\left\{\frac{\partial f}{\partial s}+\left[\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}+\frac{(1+a+b){\sigma }_2^2}{2}{s}^{a+b}\right]\frac{{\partial }^{2}f}{\partial Y^2}\right\}ds\hfill \\ & & +{\sigma }_1{\int }_0^t\frac{\partial f}{\partial Y}d{B}_s+{\sigma }_2{\int }_0^t\frac{\partial f}{\partial Y}d{B}_s^{a,b}+\gamma {\int }_0^t\frac{\partial f}{\partial Y}d{J}_s.\hfill \end{array}$$

 Proof. 

By virtue of It$\widehat{\mathrm{o}}$’s formula for weighted fractional Brownian motion (Refer to Theorem 2.1 in Reference [26] for details) and the analytical technique pertaining to the jump process [28], we derive the following:

$$\begin{array}{cc}\hfill f(t,Y_t)& =f(0,0)+{\int }_0^t\frac{\partial f}{\partial s}ds+{\int }_0^t\frac{\partial f}{\partial s}d{\overline{Y}}_s+\frac{1}{2}{\int }_0^t\frac{{\partial }^{2}f}{\partial s^2}d{\left({\overline{Y}}_s\right)}^2+\sum _{s\le t}\left[f(s,Y_s)-f(s-,Y_{s-})\right]\hfill \\ \hfill & =f(0,0)+{\int }_0^t\left[\frac{\partial f}{\partial s}-\lambda \gamma \frac{\partial f}{\partial Y}\right]ds+{\int }_0^t\left[\frac{{\sigma }_1^2}{2}+\frac{(1+a+b){\sigma }_2^2}{2}{s}^{a+b}\right]\frac{{\partial }^{2}f}{\partial Y^2}ds\hfill \\ \hfill & +{\sigma }_1{\int }_0^t\frac{\partial f}{\partial Y}d{B}_s+{\sigma }_2{\int }_0^t\frac{\partial f}{\partial Y}d{B}_s^{a,b}+\sum _{s\le t}\left[f(s,Y_s)-f(s-,Y_{s-})\right].\hfill \end{array}$$

(3)

The following identities are used by

$$d{\overline{Y}}_t={\sigma }_{1}d{B}_t+{\sigma }_{2}d{B}_t^{a,b}-\lambda \gamma dt,$$

$${\left(d{\overline{Y}}_t\right)}^2=\left[{\sigma }_1^2+(1+a+b){\sigma }_2^2{t}^{a+b}\right]dt.$$

and ${\overline{Y}}_t={\sigma }_1{B}_t+{\sigma }_2{B}_t^{a,b}-\lambda \gamma t$ is the continuous part of $Y_t$.

Assuming that $g\left(x\right)$ exhibits second-order differentiability, and considering the Poisson process ${\left\{N_t\right\}}_{t\ge 0}$ with intensity $\lambda$, which possesses second-order moment increments, we can invoke a generalized It $\widehat{\mathrm{o}}$’s formula to assert

$$\sum _{s\le t}\left[g\left(N_s\right)-g\left(N_{s-}\right)\right]={\int }_0^t\frac{\partial g}{\partial N}d{N}_s+\frac{\lambda }{2}{\int }_0^t\frac{{\partial }^{2}g}{\partial N^2}ds.$$

Combining $Y_t={\xi }_t({\sigma }_1,{\sigma }_2)+\gamma J_t={\sigma }_1{B}_t+{\sigma }_2{B}_t^{a,b}+\gamma N_t-\lambda \gamma t$, we obtain

$$\sum _{s\le t}\left[f(s,Y_s)-f(s-,Y_{s-})\right]=\gamma {\int }_0^t\frac{\partial f}{\partial Y}d{N}_s+\frac{\lambda {\gamma }^2}{2}{\int }_0^t\frac{{\partial }^{2}f}{\partial Y^2}ds.$$

(4)

We can substitute Equation (4) back into Equation (3), which yields

$$\begin{array}{ccc}\hfill f(t,Y_t)& & =f(0,0)+{\int }_0^t\left\{\frac{\partial f}{\partial s}+\left[\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}+\frac{(1+a+b){\sigma }_2^2}{2}{s}^{a+b}\right]\frac{{\partial }^{2}f}{\partial Y^2}\right\}ds\hfill \\ & & +{\sigma }_1{\int }_0^t\frac{\partial f}{\partial Y}d{B}_s+{\sigma }_2{\int }_0^t\frac{\partial f}{\partial Y}d{B}_s^{a,b}+\gamma {\int }_0^t\frac{\partial f}{\partial Y}d{J}_s.\hfill \end{array}$$

This completes the proof. □

 Theorem 2. 

The stock price, which complies with Equation (2), unveils an explicit solution as follows: 

$$S_t=S_{0}exp\left\{\mu t-\left[\left(\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}\right)t+\frac{{\sigma }_2^2}{2}{t}^{a+b+1}\right]+{\sigma }_1{B}_t+{\sigma }_2{B}_t^{a,b}+\gamma J_t\right\}.$$

 Proof. 

Let $f(t,Y_t)=S_{0}exp\left\{\mu t-\left[\left(\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}\right)t+\frac{{\sigma }_2^2}{2}{t}^{a+b+1}\right]+Y_t\right\}$.

According to Theorem 1, we obtain

$$\begin{array}{cc}\hfill df(f,Y_t)& =\left\{\frac{\partial f}{\partial t}+\left[\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}+\frac{{\sigma }_2^2}{2}{t}^{a+b+1}\right]\frac{{\partial }^{2}f}{\partial Y^2}\right\}dt+\frac{\partial f}{\partial Y}d{Y}_t\hfill \\ \hfill & =\mu f(t,Y_t)dt+f(t,Y_t)d{Y}_t\hfill \\ \hfill & =\mu f(t,Y_t)dt+f(t,Y_t)d{\xi }_t({\sigma }_1,{\sigma }_2)+\gamma f(t,Y_t)d{J}_t,\hfill \end{array}$$

(5)

where $\frac{\partial f}{\partial t}=\left\{\mu -\left[\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}+\frac{{\sigma }_2^2}{2}{t}^{a+b+1}\right]\right\}f(t,Y_t),$ $\frac{\partial f}{\partial Y}=f(t,Y_t),\frac{{\partial }^{2}f}{\partial Y^2}=f(t,Y_t).$

Comparing Equation (2) and Equation (5), we can infer that $d{S}_t=df(t,Y_t)$, with the values matching $f(0,Y_0)=S_0$. Therefore,

$$S_t=S_{0}exp\left\{\mu t-\left[\left(\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}\right)t+\frac{{\sigma }_2^2}{2}{t}^{a+b+1}\right]+{\sigma }_1{B}_t+{\sigma }_2{B}_t^{a,b}+\gamma J_t\right\}.$$

This completes the proof. □

4. Pricing Formula for European Option under the Mixed Weight-fBm Model with Jump

Equipped with the unequivocal solution for the stock price $S_t$, in this section, we shall derive the pricing formula for European call and put options.

 Theorem 3. 

Supposing that the price of the underlying asset,  $S_t$,  adheres to Equation (2), then the value of the contingent claim, denoted as  $V_t=V(t,S_t)$,  complies with the ensuing partial differential equation: 

$$\frac{\partial V}{\partial t}+r{S}_t\frac{\partial V}{\partial S}+\left[\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}+\frac{(a+b+1){\sigma }_2^2}{2}{t}^{a+b}\right]S_t^2\frac{{\partial }^{2}V}{\partial S^2}-r{V}_t=0.$$

Proof. 

Using the self-financing strategy ${\mathrm{\Pi }}_t=({\theta }_t^1,{\theta }_t^2)$, we maintain a number of ${\theta }_t^1$ bonds and ${\theta }_t^2$ stocks to construct the wealth process, the value of which at time t  is

$$V_t={\theta }_t^1{M}_t+{\theta }_t^2{S}_t.$$

(6)

Combining Equation (1) and Equation (2), we obtain

$$\begin{array}{cc}\hfill d{V}_t& ={\theta }_t^{1}d{M}_t+{\theta }_t^{2}d{S}_t\hfill \\ \hfill & =\left(r{\theta }_t^1{M}_t+\mu {\theta }_t^2{S}_t\right)dt+{\theta }_t^2{S}_t\left({\sigma }_{1}d{B}_t+{\sigma }_{2}d{B}_t^{a,b}+\gamma d{J}_t\right).\hfill \end{array}$$

(7)

Similarly, applying Theorems 1 and 2 gives

$$\begin{array}{cc}\hfill d{V}_t& =\frac{\partial V}{\partial t}dt+\frac{\partial V}{\partial S}d{S}_t+\frac{1}{2}\frac{{\partial }^{2}V}{\partial S^2}{\left(d{S}_t\right)}^2\hfill \\ \hfill & =\left\{\frac{\partial V}{\partial t}+\mu S_t\frac{\partial V}{\partial S}+\left[\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}+\frac{(a+b+1){\sigma }_2^2}{2}{t}^{a+b}\right]S_t^2\frac{{\partial }^{2}V}{\partial S^2}\right\}dt\hfill \\ \hfill & +S_t\frac{\partial V}{\partial S}\left({\sigma }_{1}d{B}_t+{\sigma }_{2}d{B}_t^{a,b}+\gamma d{J}_t\right),\hfill \end{array}$$

(8)

where ${\left(d{S}_t\right)}^2=S_t^2\left[({\sigma }_1^2+\lambda {\gamma }^2)+(a+b+1){\sigma }_2^2{t}^{a+b}\right]dt.$

Comparing Equation (7) and Equation (8), ${\theta }_t^1$ and ${\theta }_t^2$ are given as

$$\left\{\begin{array}{c}{\theta }_t^1={\left(r{M}_t\right)}^{-1}\left\{\frac{\partial V}{\partial t}+\left[\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}+\frac{(a+b+1){\sigma }_2^2}{2}{t}^{a+b}\right]S_t^2\frac{{\partial }^{2}V}{\partial S^2}\right\},\hfill \\ {\theta }_t^2=\frac{\partial V}{\partial S}.\hfill \end{array}\right.$$

(9)

From Equation (6), we obtain

$${\theta }_t^1=\frac{V_t-{\theta }_t^2{S}_t}{M_t}.$$

(10)

Combining Equation (9) and Equation (10), Theorem 3 is proved.

This completes the proof. □

 Theorem 4. 

With the underlying asset price  $S_t$  following Equation (2), the price at time t of a European call option with strike price K and maturity T is 

$$C(t,S_t)=S_t\mathrm{\Phi }\left(d_1\right)-K{e}^{-r(T-t)}\mathrm{\Phi }\left(d_2\right),$$

(11)

where 

$$\begin{array}{ccc}& & d_1=\frac{ln\frac{S_t}{K}+r(T-t)+(\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2})(T-t)+\frac{{\sigma }_2^2}{2}(T^{a+b+1}-t^{a+b+1})}{\sqrt{({\sigma }_1^2+\lambda {\gamma }^2)(T-t)+{\sigma }_2^2(T^{a+b+1}-t^{a+b+1})}},\hfill \\ & & d_2=d_1-\sqrt{({\sigma }_1^2+\lambda {\gamma }^2)(T-t)+{\sigma }_2^2(T^{a+b+1}-t^{a+b+1})},\hfill \end{array}$$

and  $\mathrm{\Phi }(·)$  denotes the standard normal cumulative distribution function. 

 Proof. 

The valuation of the European call option adheres to the ensuing differential equation

$$\left\{\begin{array}{c}\frac{\partial V}{\partial t}+r{S}_t\frac{\partial V}{\partial S}+\left[\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}+\frac{(a+b+1){\sigma }_2^2}{2}{t}^{a+b}\right]S_t^2\frac{{\partial }^{2}V}{\partial S^2}-r{V}_t=0,\hfill \\ C(T,S_T)={\left(S_T-K\right)}^{+}.\hfill \end{array}\right.$$

(12)

Through the utilization of variable substitution, Equation (12) metamorphoses into a Cauchy problem. Let $S_t=S=e^x$ and $V(t,S_t)=C(t,x)$; then, $x=lnS$.

$$\frac{\partial V}{\partial t}=\frac{\partial C}{\partial t},\frac{\partial V}{\partial S}=\frac{1}{S}\frac{\partial C}{\partial x},\frac{{\partial }^{2}V}{\partial S^2}=\frac{1}{S^2}\left(\frac{{\partial }^{2}C}{\partial x^2}-\frac{\partial C}{\partial x}\right).$$

By substituting the above formula into Equation (12), it can be obtained that

$$\left\{\begin{array}{c}\frac{\partial V}{\partial t}+r\frac{\partial C}{\partial x}+\left[\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}+\frac{(a+b+1){\sigma }_2^2}{2}{t}^{a+b}\right]\left(\frac{{\partial }^{2}C}{\partial x^2}-\frac{\partial C}{\partial x}\right)-rC=0,\hfill \\ C(T,x)={\left(e^x-K\right)}^{+}.\hfill \end{array}\right.$$

(13)

Let

$$\left\{\begin{array}{c}u(s,z)=C(t,x)e^{r(T-t)},\hfill \\ s=\left(\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}\right)(T-t)+\frac{{\sigma }_2^2}{2}\left(T^{a+b+1}-t^{a+b+1}\right),\hfill \\ z=x+r(T-t)-\left(\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}\right)(T-t)-\frac{{\sigma }_2^2}{2}\left(T^{a+b+1}-t^{a+b+1}\right).\hfill \end{array}\right.$$

(14)

Then, we have

$$\begin{array}{cc}\hfill & C(t,x)=u(s,z)e^{-r(T-t)},\hfill \\ \hfill & \frac{\partial C}{\partial t}=e^{-r(T-t)}\left\{\left[ru+\frac{{\sigma }_1^2}{2}+\frac{\lambda {\gamma }^2}{2}+\frac{(a+b+1){\sigma }_2^2}{2}{t}^{a+b}\right](\frac{\partial u}{\partial z}-\frac{\partial u}{\partial s})-\frac{\partial u}{\partial z}r\right\},\hfill \\ \hfill & \frac{\partial C}{\partial x}=e^{-r(T-t)}\frac{\partial u}{\partial z},\hfill \\ \hfill & \frac{{\partial }^{2}C}{\partial x^2}=e^{-r(T-t)}\frac{{\partial }^{2}u}{\partial z^2}.\hfill \end{array}$$

By substituting the above formula into Equation (13), it can be obtained that

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\frac{{\partial }^{2}u}{\partial z^2}=\frac{\partial u}{\partial s},\hfill \\ u(0,z)={\left(e^z-K\right)}^{+}.\hfill \end{array}\right.\end{array}$$

In accordance with the classical principles of thermal dynamics, there exists a unique strong solution

$$\begin{array}{cc}\hfill u(s,z)& =\frac{1}{2\sqrt{\pi s}}{\int }_{-\infty }^{+\infty }u(0,\tau )e^{\frac{{(\tau -z)}^2}{4s}}d\tau \hfill \\ \hfill & =\frac{1}{2\sqrt{\pi s}}{\int }_{-\infty }^{+\infty }{e}^{\tau -K}{e}^{\frac{{(\tau -z)}^2}{4s}}d\tau .\hfill \end{array}$$

Then,

$$\begin{array}{cc}\hfill V(t,S_t)=C(t,x)& =u(s,z)e^{-r(T-t)}\hfill \\ \hfill & =\frac{e^{-r(T-t)}}{2\sqrt{\pi s}}{\int }_{lnK}^{+\infty }{(e^{\tau }-K)}^{+}{e}^{\frac{{(\tau -z)}^2}{4s}}d\tau \hfill \\ \hfill & =\frac{e^{-r(T-t)}}{2\sqrt{\pi s}}{\int }_{lnK}^{+\infty }{e}^{\tau }{e}^{\frac{{(\tau -z)}^2}{4s}}d\tau -\frac{K{e}^{-r(T-t)}}{2\sqrt{\pi s}}{\int }_{lnK}^{+\infty }{e}^{\frac{{(\tau -z)}^2}{4s}}d\tau \hfill \\ \hfill & =I_1-I_2.\hfill \end{array}$$

(15)

For $I_2$, let $y=\frac{\tau -z}{\sqrt{\pi s}}$; we have $\sqrt{2s}dy=d\tau$. Then,

$$\begin{array}{cc}\hfill I_2& =\frac{K{e}^{-r(T-t)}}{2\sqrt{\pi s}}{\int }_{lnK}^{+\infty }{e}^{\frac{{(\tau -z)}^2}{4s}}d\tau \hfill \\ \hfill & =\frac{K{e}^{-r(T-t)}}{2\sqrt{\pi s}}{\int }_{\frac{lnK-z}{\sqrt{2s}}}^{+\infty }{e}^{-\frac{y^2}{2}}dy\hfill \\ \hfill & =K{e}^{-r(T-t)}\mathrm{\Phi }\left(\frac{z-lnK}{\sqrt{2s}}\right).\hfill \end{array}$$

Similarly, for $I_1$, let $\varpi =\frac{\tau -z-2s}{\sqrt{2s}}$; we have $\sqrt{2s}d\varpi =d\tau$.

$$\begin{array}{cc}\hfill I_1& =\frac{e^{-r(T-t)}}{2\sqrt{\pi s}}{\int }_{lnK}^{+\infty }{e}^{\tau }{e}^{\frac{{(\tau -z)}^2}{4s}}d\tau \hfill \\ \hfill & =\frac{e^{-r(T-t)}}{2\sqrt{\pi s}}{e}^{z+s}{\int }_{\frac{lnK-z-2s}{\sqrt{2s}}}^{+\infty }{e}^{-\frac{{\varpi }^2}{2}}d\varpi \hfill \\ \hfill & =e^{-r(T-t)}{e}^{z+s}\mathrm{\Phi }\left(\frac{z+2s-lnK}{\sqrt{2s}}\right).\hfill \end{array}$$

By substituting the expressions for s  and z  from Equation (14) into $I_1$ and $I_2$, and subsequently substituting $I_1$ and $I_2$ into Equation (15), this completes the proof. □

 Remark 1. 

The relationship of parity between the prices of European call option  $C_t$  and European put option  $P_t$  is expressed as  $P_t$  is  $C_t-P_t=S_t-K{e}^{-r(T-t)}$,  which enables us to determine the price of a European put option at time t. 

 Remark 2. 

If  $a=0,\gamma =0$,  let  $H=\frac{b+1}{2}$,  then the mixed weighted-fBm with the jump model reduces to the mfBm Black–Scholes model with the Hurst index H, which is consistent with the result in [29,30]. 

5. European Option Pricing in a Fuzzy Framework

According to Theorem 4 and reference [31], we can readily attain the subsequent findings regarding the fuzzy price, denoted as ${\tilde{C}}_t$, of the European call option.

 Theorem 5. 

 With fuzzy interest rate  $\tilde{r}$,  fuzzy volatility  ${\tilde{\sigma }}_1,{\tilde{\sigma }}_2$  and  $\tilde{\gamma }$ , fuzzy jump intensity  $\tilde{\lambda }$ , and fuzzy stock price  ${\tilde{S}}_t$ , the fuzzy European call price  ${\tilde{C}}_t$  at time t is 

$${\tilde{C}}_t={\tilde{S}}_t\mathrm{\Phi }\left({\tilde{d}}_1\right)-K{e}^{-\tilde{r}(T-t)}\mathrm{\Phi }\left({\tilde{d}}_2\right),$$

 where 

$$\begin{array}{ccc}& & {\tilde{d}}_1=\frac{ln\frac{{\tilde{S}}_t}{K}+\tilde{r}(T-t)+(\frac{{\tilde{\sigma }}_1^2}{2}+\frac{\tilde{\lambda }{\tilde{\gamma }}^2}{2})(T-t)+\frac{{\tilde{\sigma }}_2^2}{2}(T^{a+b+1}-t^{a+b+1})}{\sqrt{({\tilde{\sigma }}_1^2+\tilde{\lambda }{\tilde{\gamma }}^2)(T-t)+{\tilde{\sigma }}_2^2(T^{a+b+1}-t^{a+b+1})}},\hfill \\ & & {\tilde{d}}_2={\tilde{d}}_1-\sqrt{({\tilde{\sigma }}_1^2+\tilde{\lambda }{\tilde{\gamma }}^2)(T-t)+{\tilde{\sigma }}_2^2(T^{a+b+1}-t^{a+b+1})}.\hfill \end{array}$$

 Remark 3. 

 The relationship of parity between the prices of European call option  ${\tilde{C}}_t$  and European put option  ${\tilde{P}}_t$  is expressed as  ${\tilde{C}}_t-{\tilde{P}}_t={\tilde{S}}_t-K{e}^{-\tilde{r}(T-t)}$ , which enables us to determine the price of a European put option at time t. 

 Theorem 6. 

 The respective interval endpoints of the α-cut for the price of a European call option  ${\tilde{C}}_t$  are 

$$\begin{array}{ccc}& & {\left({\tilde{C}}_t\right)}_{\alpha }^L=f\left({\left({\tilde{S}}_t\right)}_{\alpha }^L,T,t,a,b,K,{\left(\tilde{r}\right)}_{\alpha }^L,{\left({\tilde{\sigma }}_1\right)}_{\alpha }^L,{\left({\tilde{\sigma }}_2\right)}_{\alpha }^L,{\left(\tilde{\gamma }\right)}_{\alpha }^L,{\left(\tilde{\lambda }\right)}_{\alpha }^L\right),\hfill \\ & & {\left({\tilde{C}}_t\right)}_{\alpha }^U=f\left({\left({\tilde{S}}_t\right)}_{\alpha }^U,T,t,a,b,K,{\left(\tilde{r}\right)}_{\alpha }^U,{\left({\tilde{\sigma }}_1\right)}_{\alpha }^U,{\left({\tilde{\sigma }}_2\right)}_{\alpha }^U,{\left(\tilde{\gamma }\right)}_{\alpha }^U,{\left(\tilde{\lambda }\right)}_{\alpha }^U\right).\hfill \end{array}$$

 Proof. 

The $\alpha$-cut set ${\left({\tilde{C}}_t\right)}_{\alpha }$ of ${\tilde{C}}_t$ can be articulated as ${\left({\tilde{C}}_t\right)}_{\alpha }=\left[{\left({\tilde{C}}_t\right)}_{\alpha }^L,{\tilde{C}}_t{)}_{\alpha }^U\right]$.

With regard to the $\alpha$-cut set of fuzzy variables encompassing fuzzy interest rate $\tilde{r}$, fuzzy volatility ${\tilde{\sigma }}_1,{\tilde{\sigma }}_2$ and $\tilde{\gamma }$, as well as the fuzzy jump intensity $\tilde{\lambda }$, the fuzzy stock price ${\tilde{S}}_t$ can be delineated as follows: ${\tilde{r}}_{\alpha }=[{\tilde{r}}_{\alpha }^L,{\tilde{r}}_{\alpha }^U]$, ${\left({\tilde{\sigma }}_1\right)}_{\alpha }=[{\left({\tilde{\sigma }}_1\right)}_{\alpha }^L,{\left({\tilde{\sigma }}_1\right)}_{\alpha }^U]$, ${\left({\tilde{\sigma }}_2\right)}_{\alpha }=[{\left({\tilde{\sigma }}_2\right)}_{\alpha }^L,{\left({\tilde{\sigma }}_2\right)}_{\alpha }^U]$, ${\tilde{\lambda }}_{\alpha }=[{\tilde{\lambda }}_{\alpha }^L,{\tilde{\lambda }}_{\alpha }^U]$, ${\tilde{\gamma }}_{\alpha }=[{\tilde{\gamma }}_{\alpha }^L,{\tilde{\gamma }}_{\alpha }^U]$, ${\left({\tilde{S}}_t\right)}_{\alpha }=[{\left({\tilde{S}}_t\right)}_{\alpha }^L,{\left({\tilde{S}}_t\right)}_{\alpha }^U]$.

It can be seen from Equation (11) that

$\frac{\partial f}{\partial S}=\mathrm{\Phi }\left(d_1\right)>0$, $\frac{\partial f}{\partial r}=(T-t)e^{-r(T-t)}\mathrm{\Phi }\left(d_2\right)>0$, $\frac{\partial f}{\partial {\sigma }_1}=\frac{\partial f}{\partial \lambda }=\frac{\partial f}{\partial \gamma }=S\sqrt{T-t}{\mathrm{\Phi }}^{\prime }\left(d_1\right)>0$, and $\frac{\partial f}{\partial {\sigma }_2}=S\sqrt{T^{a+b+1}-t^{a+b+1}}{\mathrm{\Phi }}^{\prime }\left(d_1\right)>0$.

One may ascertain that  f  is indeed an ascending function with respect to $S,r,{\sigma }_1,{\sigma }_2,\lambda$, and $\gamma$. Consequently, the theorem stands as duly established.

This completes the proof. □

The triangle fuzzy number is commonly used to represent fuzzy variables. In this study, the fuzzy interest rate, fuzzy volatility, fuzzy jump intensity, and fuzzy stock price are all modeled as triangle fuzzy numbers to capture their inherent uncertainty. There is $\tilde{r}=(r_1,r_2,r_3)$, ${\tilde{\sigma }}_1=({\sigma }_{11},{\sigma }_{12},{\sigma }_{13})$, ${\tilde{\sigma }}_2=({\sigma }_{21},{\sigma }_{22},{\sigma }_{23})$, $\tilde{\lambda }=({\lambda }_1,{\lambda }_2,{\lambda }_3)$, $\tilde{\gamma }=({\gamma }_1,{\gamma }_2,{\gamma }_3)$, and ${\tilde{S}}_t=(S_1,S_2,S_3)$. From Definition 3, we can obtain that

${\left(\tilde{S}\right)}_{\alpha }=\left[(1-\alpha )S_1+\alpha S_2,(1-\alpha )S_3+\alpha S_2\right]$;

${\left(\tilde{r}\right)}_{\alpha }=\left[(1-\alpha )r_1+\alpha r_2,(1-\alpha )r_3+\alpha r_2\right]$;

${\left({\tilde{\sigma }}_1\right)}_{\alpha }=\left[(1-\alpha ){\sigma }_{11}+\alpha {\sigma }_{12},(1-\alpha ){\sigma }_{13}+\alpha {\sigma }_{12}\right]$;

${\left({\tilde{\sigma }}_2\right)}_{\alpha }=\left[(1-\alpha ){\sigma }_{21}+\alpha {\sigma }_{22},(1-\alpha ){\sigma }_{23}+\alpha {\sigma }_{22}\right]$;

${\left(\tilde{\lambda }\right)}_{\alpha }=\left[(1-\alpha ){\lambda }_1+\alpha {\lambda }_2,(1-\alpha ){\lambda }_3+\alpha {\lambda }_2\right]$;

${\left(\tilde{\gamma }\right)}_{\alpha }=\left[(1-\alpha ){\gamma }_1+\alpha {\gamma }_2,(1-\alpha ){\gamma }_3+\alpha {\gamma }_2\right].$

 Theorem 7. 

 When representing the interest rate, volatility, jump intensity, and stock price as triangular fuzzy numbers, the α-cut set for the fuzzy European call price  ${\tilde{C}}_t$  is characterized by its interval endpoints  $[{\left({\tilde{C}}_t\right)}_{\alpha }^L,{\left({\tilde{C}}_t\right)}_{\alpha }^U]$:

$${\left({\tilde{C}}_t\right)}_{\alpha }^L={\left({\tilde{S}}_t\right)}_{\alpha }^L\mathrm{\Phi }\left({\tilde{d}}_1^L\left(\alpha \right)\right)-K{e}^{-{\tilde{r}}_{\alpha }^L(T-t)}\mathrm{\Phi }\left({\tilde{d}}_2^L\left(\alpha \right)\right),$$

(16)

$${\left({\tilde{C}}_t\right)}_{\alpha }^U={\left({\tilde{S}}_t\right)}_{\alpha }^U\mathrm{\Phi }\left({\tilde{d}}_1^U\left(\alpha \right)\right)-K{e}^{-{\tilde{r}}_{\alpha }^U(T-t)}\mathrm{\Phi }\left({\tilde{d}}_2^U\left(\alpha \right)\right).$$

(17)

where 

$$\begin{array}{ccc}& & {\tilde{d}}_1^L\left(\alpha \right)=\frac{ln\frac{{\left({\tilde{S}}_t\right)}_{\alpha }^L}{K}+{\tilde{r}}_{\alpha }^L(T-t)+(\frac{{\left[{\left({\tilde{\sigma }}_1\right)}_{\alpha }^L\right]}^2}{2}+\frac{{\tilde{\lambda }}_{\alpha }^L{\left[{\left(\tilde{\gamma }\right)}_{\alpha }^L\right]}^2}{2})(T-t)+\frac{{\left[{\left({\tilde{\sigma }}_2\right)}_{\alpha }^L\right]}^2}{2}(T^{a+b+1}-t^{a+b+1})}{\sqrt{({\left[{\left({\tilde{\sigma }}_1\right)}_{\alpha }^L\right]}^2+{\tilde{\lambda }}_{\alpha }^L{\left[{\left(\tilde{\gamma }\right)}_{\alpha }^L\right]}^2)(T-t)+{\left[{\left({\tilde{\sigma }}_2\right)}_{\alpha }^L\right]}^2(T^{a+b+1}-t^{a+b+1})}},\hfill \\ & & {\tilde{d}}_2^L\left(\alpha \right)={\tilde{d}}_1^L\left(\alpha \right)-\sqrt{({\left[{\left({\tilde{\sigma }}_1\right)}_{\alpha }^L\right]}^2+{\tilde{\lambda }}_{\alpha }^L{\left[{\left(\tilde{\gamma }\right)}_{\alpha }^L\right]}^2)(T-t)+{\left[{\left({\tilde{\sigma }}_2\right)}_{\alpha }^L\right]}^2(T^{a+b+1}-t^{a+b+1})},\hfill \\ & & {\tilde{d}}_1^U\left(\alpha \right)=\frac{ln\frac{{\left({\tilde{S}}_t\right)}_{\alpha }^U}{K}+{\tilde{r}}_{\alpha }^U(T-t)+(\frac{{\left[{\left({\tilde{\sigma }}_1\right)}_{\alpha }^U\right]}^2}{2}+\frac{{\tilde{\lambda }}_{\alpha }^U{\left[{\left(\tilde{\gamma }\right)}_{\alpha }^U\right]}^2}{2})(T-t)+\frac{{\left[{\left({\tilde{\sigma }}_2\right)}_{\alpha }^U\right]}^2}{2}(T^{a+b+1}-t^{a+b+1})}{\sqrt{({\left[{\left({\tilde{\sigma }}_1\right)}_{\alpha }^U\right]}^2+{\tilde{\lambda }}_{\alpha }^U{\left[{\left(\tilde{\gamma }\right)}_{\alpha }^U\right]}^2)(T-t)+{\left[{\left({\tilde{\sigma }}_2\right)}_{\alpha }^U\right]}^2(T^{a+b+1}-t^{a+b+1})}},\hfill \\ & & {\tilde{d}}_2^U\left(\alpha \right)={\tilde{d}}_1^U\left(\alpha \right)-\sqrt{({\left[{\left({\tilde{\sigma }}_1\right)}_{\alpha }^U\right]}^2+{\tilde{\lambda }}_{\alpha }^U{\left[{\left(\tilde{\gamma }\right)}_{\alpha }^U\right]}^2)(T-t)+{\left[{\left({\tilde{\sigma }}_2\right)}_{\alpha }^U\right]}^2(T^{a+b+1}-t^{a+b+1})}.\hfill \end{array}$$

6. Numerical Experiments

This section thoroughly investigates how the parameter b  affects the pricing model by utilizing the control variable method. It provides an exhaustive examination of the sensitivity and stability of the European option pricing model, particularly considering its enduring memory characteristics amidst the backdrop of uncertainty. For simplicity, the numerical experiments use European call option pricing as an example, with European put options following analogous arguments. The parameter values match the benchmark model in

Table 1. Values of benchmark parameters.

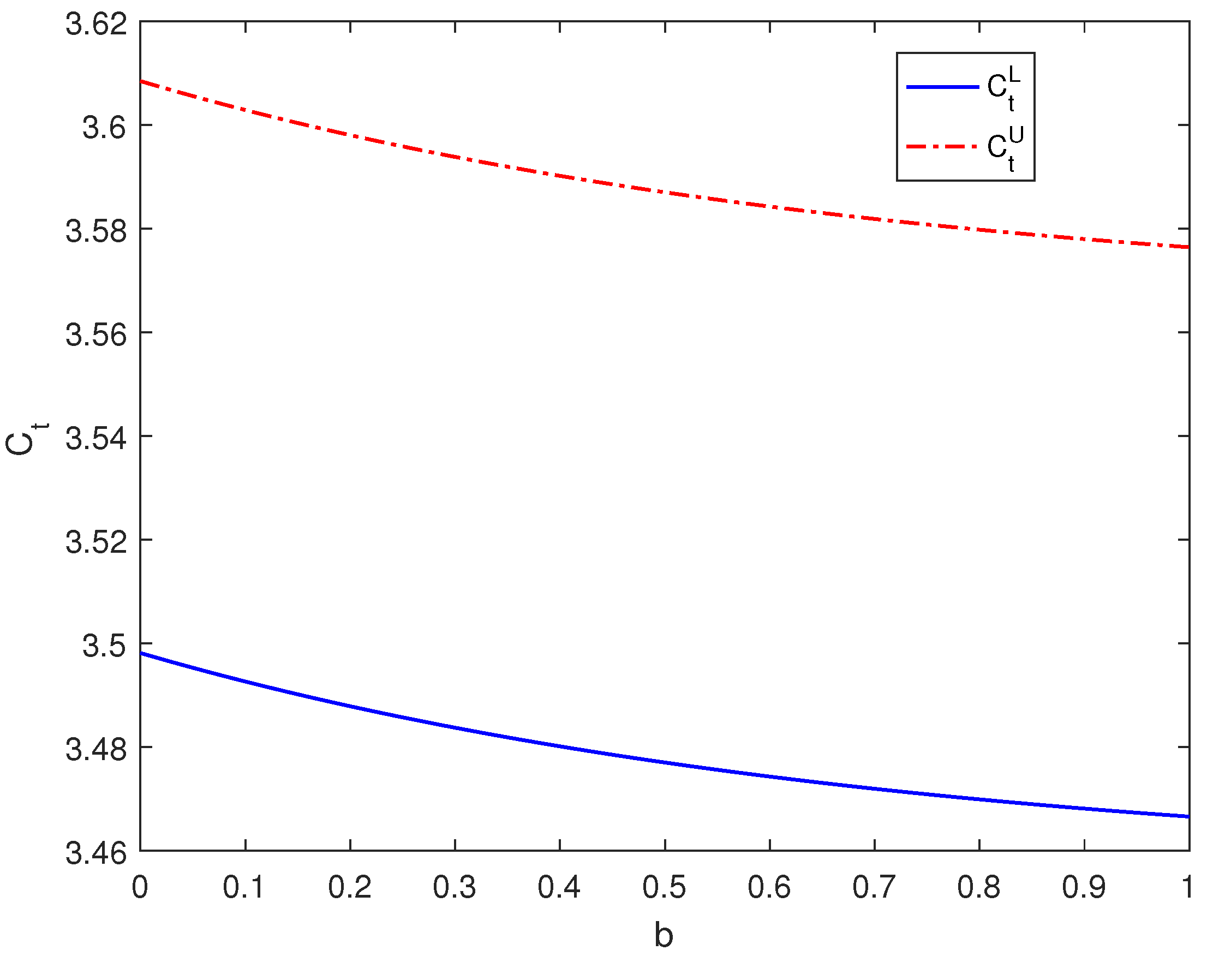
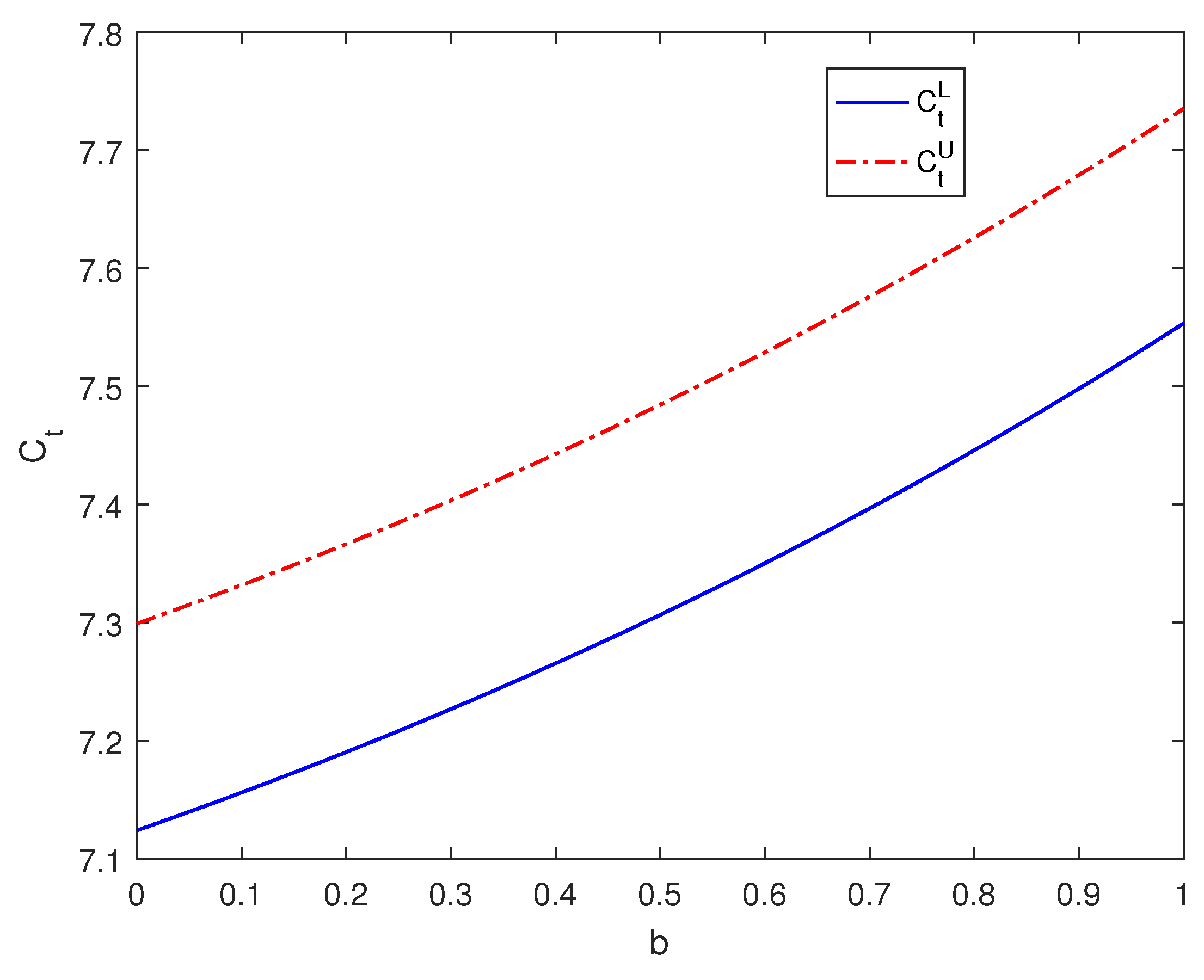
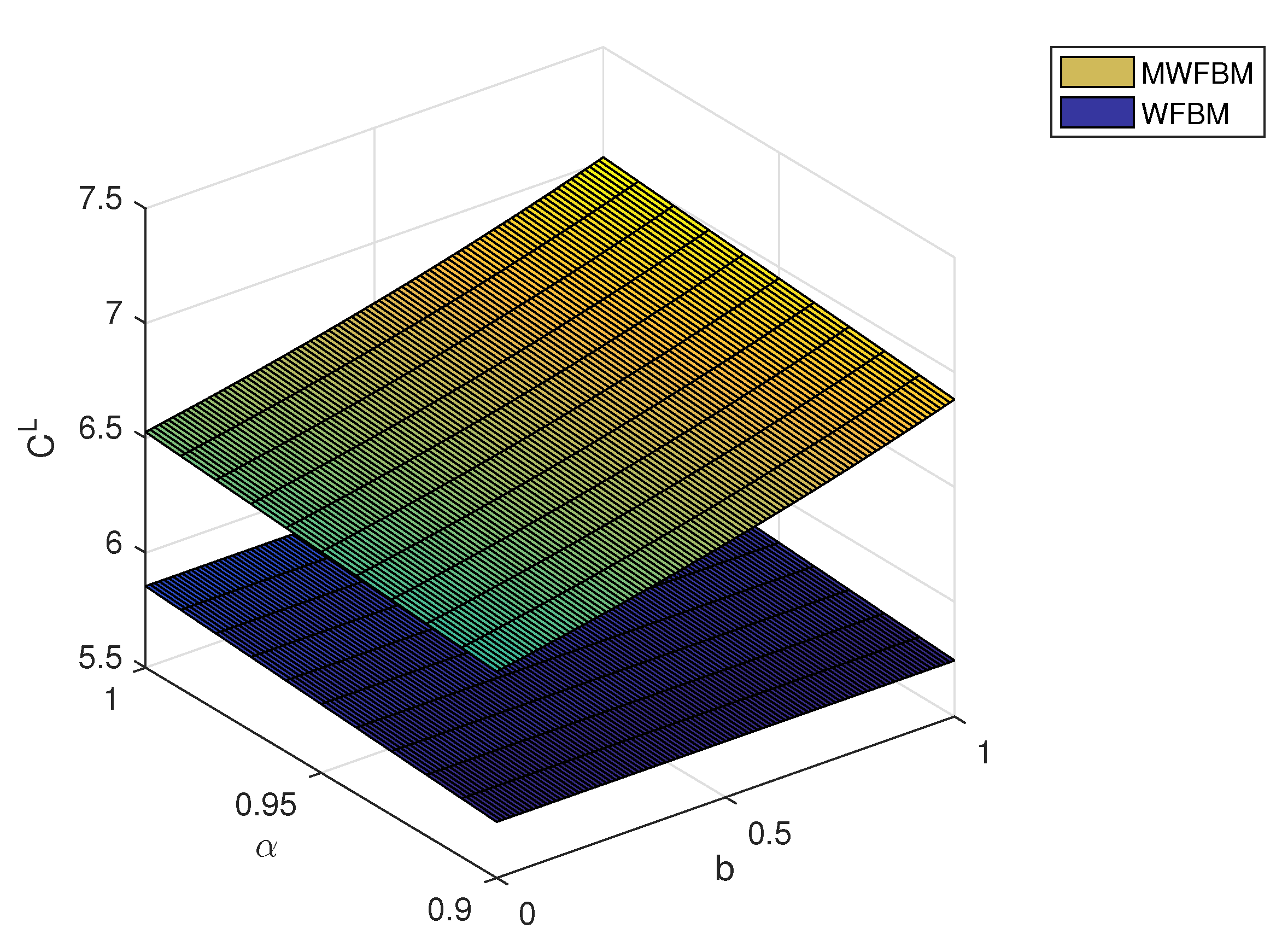
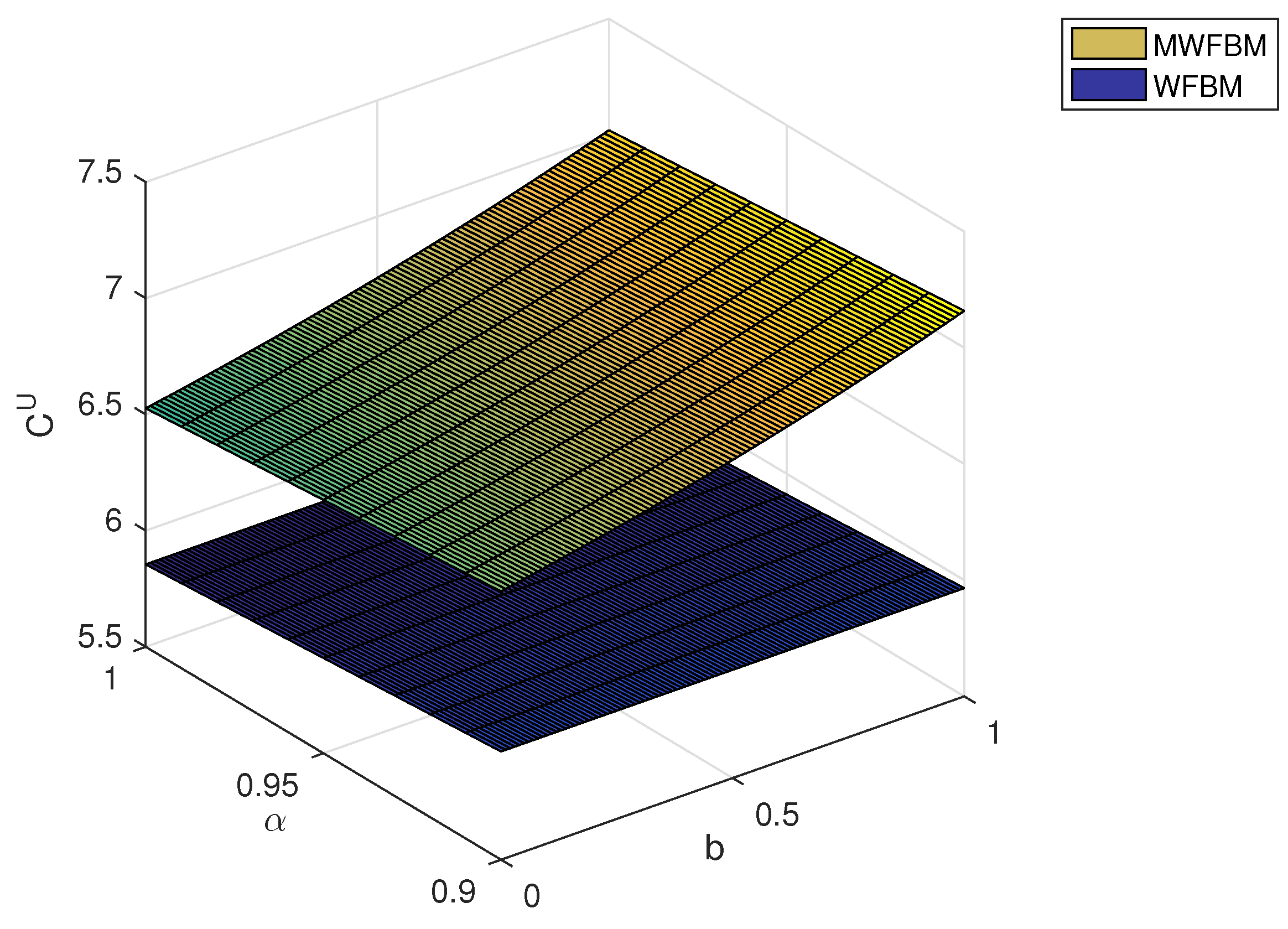
Parameter	Symbols	Parameter	Symbols
Strike price	$K=30$	Fuzzy stock price	$\tilde{S}=(32,33,34)$
Initial time	$t=0$	Fuzzy volatility	${\tilde{\sigma }}_1={\tilde{\sigma }}_2=\tilde{\gamma }=(0.08,0.1,0.12)$
Degree of confidence	$\alpha =0.95$	Fuzzy jump intensity	$\tilde{\lambda }=(1,2,3)$
Parameter of weighted-fBm	$a=0.5$	Fuzzy risk-free rate	$\tilde{r}=(0.048,0.05,0.052)$

. $C_t^L$ and $C_t^U$ represent, respectively, the upper and lower boundaries of European call option prices, and their associated connections can be expressed as follows: $C_t^L={\left({\tilde{C}}_t\right)}_{\alpha }^L$, $C_t^U={\left({\tilde{C}}_t\right)}_{\alpha }^U$.

As depicted in Figure 1 and Figure 2, we observe that when the maturity date is set at $T=0.25$, the triangular fuzzy price of European call options experiences a decline as parameter b  increases. When the maturity date is set at $T=2$, the triangular fuzzy price of European call options experiences an increase as parameter b  increases. By combining the results obtained from

Table 2. The interval of triangular fuzzy price $\alpha$-cut set for European call option varies across different values of b .

$T=0.25$		$\mathit{T}=2$
$\mathit{b}$	${\left({\tilde{\mathit{C}}}_{\mathbf{0}}\right)}_{\mathit{\alpha }}$	$\mathit{b}$	${\left({\tilde{\mathit{C}}}_{\mathbf{0}}\right)}_{\mathit{\alpha }}$
0.55	[3.4756,3.5856]	0.55	[7.3283,7.5065]
0.60	[3.4743,3.5843]	0.60	[7.3504,7.5290]
0.65	[3.4731,3.5830]	0.65	[7.3733,7.5522]
0.70	[3.4719,3.5819]	0.70	[7.3968,7.5761]
0.75	[3.4709,3.5808]	0.75	[7.4211,7.6007]
0.80	[3.4699,3.5798]	0.80	[7.4461,7.6261]
0.85	[3.4690,3.5788]	0.85	[7.4718,7.6522]
0.90	[3.4681,3.5780]	0.90	[7.4982,7.6791]
0.95	[3.4673,3.5772]	0.95	[7.5255,7.7068]

, we can draw the following conclusions: in the range where $0<T<1$, an increase in parameter b  leads to a reduction in the triangular fuzzy price intervals of European call options. Conversely, in cases where $T>1$, an increase in parameter b  results in an expansion of the triangular fuzzy price intervals of European call options.

Finally, we compare the proposed pricing model to a model based on weighted fractional Brownian motion (WFBM in short). For simplicity, we ignore jumps and focus on the mixed weighted fractional Brownian motion (MWFBM in short) case. Figure 3 and Figure 4 illustrate how the fuzzy price varies with the parameters b  and $\alpha$. The MWFBM model yields higher option prices compared with the WFBM model.

Table 3. A comprehensive analysis of the $\alpha$-cut set intervals of the fuzzy price of European call options under various models.

WFBM		MWFBM
$\mathit{\alpha }$	${\left({\tilde{\mathit{C}}}_{\mathbf{0}}\right)}_{\mathit{\alpha }}$	$\mathit{\alpha }$	${\left({\tilde{\mathit{C}}}_{\mathbf{0}}\right)}_{\mathit{\alpha }}$
0.99	[6.3881,6.4131]	0.99	[6.7478,6.7744]
0.98	[6.3757,6.4256]	0.98	[6.7345,6.7878]
0.97	[6.3632,6.4381]	0.97	[6.7212,6.8011]
0.96	[6.3507,6.4506]	0.96	[6.7079,6.8144]
0.95	[6.3382,6.4630]	0.95	[6.6946,6.8277]
0.94	[6.3257,6.4755]	0.94	[6.6813,6.8410]
0.93	[6.3133,6.4880]	0.93	[6.6680,6.8543]
0.92	[6.3008,6.5005]	0.92	[6.6547,6.8676]
0.91	[6.2883,6.5130]	0.91	[6.6414,6.8809]
0.90	[6.2758,6.5255]	0.90	[6.6281,6.8943]

presents the $\alpha$-cut set intervals of the fuzzy European call price $\left(\tilde{C}t\right)\alpha$ under the two models for different confidence levels $\alpha$. As shown in Table 3, the MWFBM intervals are greater than the WFBM ones. With the current model excluding jumps, if a confidence level of 0.97 is acceptable, any price between 6.7212 and 6.8011 can be chosen. In the event that the market value descends below 6.7212, the individual responsible for making choices regards the price as being undervalued and may opt for the acquisition of the option. Conversely, if the market price surpasses 6.8011, the decision-maker views the price as overvalued and may decide to exercise the option.

7. Conclusions

In this research, a fuzzy pricing model for European options is constructed within the framework of mixed weighted fractional Brownian motion with jumps. Using a self-financing strategy, we establish a partial differential equation (PDE) akin to the Black–Scholes model to assess these financial derivatives. We then obtain European option values by applying transform techniques. Similarly, put–call parity holds for the European options. Section 6 presents numerical experiments using European call options. The results demonstrate the sensitivity of fuzzy option prices to b  and $\alpha$. Additionally, the MWFBM model is compared with the WFBM model. The results indicate that, for investors, the MWFBM model carries less risk compared with the WFBM model. Based on the conclusions drawn in this study, the next steps in planned research questions and directions include the following:

(i) Improvement of Fuzzy random variables. Despite the advantages of triangular fuzzy number in terms of simplicity and ease of interpretation, their limitation lies in the potential inability to accurately depict some more complex fuzzy set distributions. Future considerations may involve exploring more intricate forms of fuzzy numbers, such as trapezoidal fuzzy numbers or generalized fuzzy number forms, to better capture the characteristics of fuzziness.

(ii) Empirical applications. On the one hand, there is potential to apply the mixed weighted fractional Brownian motion with the jumps model proposed in this paper to other more complex option types, such as Asian options, barrier options, and compound options. On the other hand, empirical simulations could involve comparing the MWFBM model with other long-memory models, such as MFBM (Mixed Fractional Brownian Motion) and MSFBM (Mixed Sub-fractional Brownian Motion), analyzing the accuracy of various models in simulating real financial market conditions.